1 Introduction

The objective of this paper is to develop an approach to the continuum mechanics of fracture viewed as the modeling of discontinuities and singularities of the (in-principle, measurable) mass-density gradient field by everywhere-continuous fields. In adopting this approach, we make a strict conceptual separation between discontinuities and singularities in the deformation of a body—that relate to dislocations, grain and phase boundaries, and generalized disclinations leading to plasticity and phase transformations in solids—and those that will be discussed here as representative of fracture. As well, the treatment of fracture evolution here unequivocally relates not to motion of 2-dimensional surfaces but to the motion of the terminating 1-dimensional curves of crack surfaces.

Fracture mechanics is by no means a new subject, see the monograph by Freund [15] which contains a brief historical review and detailed accounts of the major advances in the subject; a recent perspective viewing brittle fracture as a standard dissipative process, including computational considerations, can be found in [37]. It has also been treated from a dislocation mechanics point of view [5, 43]. There is a reasonably successful methodology for studying interesting physical problems involving complex fracture patterns based on the cohesive zone methodology, see [4, 11, 26, 31, 33, 35, 36] for some examples. Developing partial-differential-equation (pde) based or variational models for fracture that do not place restrictions on crack paths and allow practical computational schemes is a somewhat recent enterprise [6, 10, 16, 19, 28, 34, 42].Footnote 1 Also notable is the peridynamics approach of Silling (see [39]) with the associated mathematical work of Lipton [23] that have led to computational implementations. The utility of such approaches capable of modeling crack evolution in 3-d can be appreciated from the reviews of atomistic and experimental aspects of fracture in [8, 22]. The works [18, 41] relate to the concerns of our work but they involve tracking individual crack surfaces/tips. Developed as a computational model for linear elastic fracture mechanics, it is important also to note the developments in the Extended finite element methodology for fracture [27, 40]. In Sect. 5, we point out the complementary contributions of our work in relation to the literature mentioned above.

For the purpose of this work, we restrict attention to the ‘small-deformation’ theory. We use standard/obvious notations of vector calculus and work with tensor components w.r.t. a fixed orthonormal frame, unless mentioned otherwise. The \(\mathit{curl}\) of a second-order tensor field is interpreted as row-wise \(\mathit{curls}\) of the matrix field of the tensor w.r.t. the basis of a rectangular Cartesian coordinate system (this definition has an invariant meaning).

2 Kinematic Descriptors of Fracture and Their Physical Motivation

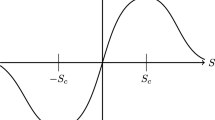
With reference to Fig. 1, consider the following situation: we consider the region \(c_{\sup}\) as divided into a set of disjoint ‘vertical’ neighborhoods as shown by the blue lines, each centered around a point \({\boldsymbol {x}}_{S} \in S\). We refer to each such neighborhood as \(N({\boldsymbol {x}}_{S})\). We think of measuring the mass density field \(\tilde{\rho}\) around \({\boldsymbol {x}}_{S}\) and call it the local mass density field in \(N({\boldsymbol {x}}_{S})\). We assume this locally measured mass density field to be continuous in the neighborhood (possibly taking on the value of 0 at some points). At the scale of observation, let it go to 0 on the crack surface \(S\). Assume the locally measured density variation at each \({\boldsymbol {x}}_{S}\) along the normal direction to the surface \(S\) be of the form as shown in Fig. 1(b). The macroscopic crack surface of 0-thickness actually is spread over the region \(c_{\sup}\) where the density may (or may not) be smooth/differentiable; but we assume that we are unable to resolve the variation of the measured density gradient in \(c_{\sup}\). Thus, \(\mathit{grad} \tilde{\rho}\) appears discontinuous across the crack surface. However, since \(\tilde{\rho}\) is continuous

$$ [\!\![\mathit{grad} \tilde{\rho} ]\!\!]= \varphi{\boldsymbol {n}}\quad \mbox{necessarily}, $$
(1)

where \({\boldsymbol {n}}\) is an arbitrarily chosen orientation for the crack surface and \(\varphi\) is a scalar field on \(S \cap N({\boldsymbol {x}}_{S})\), i.e., \(\mathit{grad} \tilde{\rho}\) can jump only in the normal direction to the surface.

Fig. 1
figure 1

Geometry of an idealized crack

Full size image

Assume that we are able to choose the orientation field \({\boldsymbol {n}}\) for the crack surfaces in the body at any given time in a continuous way except possibly at points where \(\varphi= 0\). We now define the crack field as

$$ {\boldsymbol {c}}({\boldsymbol {x}}) := \textstyle\begin{cases} \frac{\varphi({\boldsymbol {x}}_{S}) {\boldsymbol {n}}({\boldsymbol {x}}_{S})}{c_{w}} &\mbox{for}\ {\boldsymbol {x}}\in N({\boldsymbol {x}}_{S}) \in c_{\sup} \\ 0 & \mbox{for} \ {\boldsymbol {x}}\ \mbox{outside} \ c_{\sup}, \end{cases} $$

where \(c_{w}\) is the width of the region \(c_{\sup}\) measured along the direction \({\boldsymbol {n}}\), pointwise, and the crack tip field as

$$ {\boldsymbol {t}}:= -\mathit{curl} {\boldsymbol {c}}, \quad\mbox{globally on the body} $$
(2)

(with the minus sign for convenience). Assuming \({\boldsymbol {n}}\) to be oriented in the direction \({\boldsymbol {e}}_{2}\) in Fig. 1, \(\varphi({\boldsymbol {x}}) = 2a\) for \({\boldsymbol {x}}\in c_{\sup}\backslash t_{core}\). Thus, by thinking of the jump of the local density-gradient to be spread out over the layer, we may interpret the field \({\boldsymbol {c}}\) as an approximation to the directional derivative of the local density gradient field in the direction \({\boldsymbol {n}}\).

With the above argument as physical motivation, we now consider \({\boldsymbol {c}}\) and \({\boldsymbol {t}}\) as continuous fields for the sake of developing the mechanical model (as customary in mechanics). This would require some form of smoothing of the field \({\boldsymbol {c}}\) in the above description (involving an arbitrarily fine discretization of \(S\) into the set of points \({\boldsymbol {x}}_{S}\) and some natural mollification on the vertical boundaries of \(c_{\sup}\)); however, these details are not central to our postulation of the smooth \({\boldsymbol {c}}\) field as a model ingredient—we could as well have started from this premise without the physical justification. What is important is the fact that \({\boldsymbol {c}}\) is a vector field generated from a local gradient of a locally defined density gradient field, and by no means does it follow that the result should actually be compatible with an appropriately defined second-gradient of any, globally-defined, continuous scalar field on the whole body. We return to this point in Sect. 3.1.

To justify the terminology for the crack tip field, with reference to Fig. 1, let the density gradient go from some constant value in \(c_{\sup}\), say \(\varphi_{0}\) (\(\varphi_{0} = 2a\) in the example considered in Fig. 1), to 0 over the length of the region \(t_{core}\). Let \({\boldsymbol {c}}\) vary in-plane for simplicity. Then

$$ \begin{aligned} & {\boldsymbol {c}}= c_{2}\, {\boldsymbol {e}}_{2}\\ & -\mathit{curl} {\boldsymbol {c}}= {\boldsymbol {t}}= - e_{312}\, c_{2,1} \, {\boldsymbol {e}}_{3} = \frac{\varphi_{0} - 0}{l_{c} \cdot c_{w}} {\boldsymbol {e}}_{3}, \end{aligned} $$

and this is non-vanishing only in the region \(t_{core}\). Thus \(\mathit{curl} {\boldsymbol {c}} \) identifies the crack-tip region. It is also important to note that the \(\mathit{curl}\) is insensitive to the large gradients in \({\boldsymbol {c}}\) in the vertical direction across the horizontal boundaries of the layer.

3 Governing Field Equations

We begin this section by providing some heuristic motivation for the fundamental relation (3) which is subsequently derived on fundamental kinematic grounds.

Keeping crack extension through crack-tip motion in mind, we note first that

$$ {\boldsymbol {t}}= -\mathit{curl} {\boldsymbol {c}}\quad\implies\quad\dot{{\boldsymbol {t}}} = -\mathit{curl} \dot{{\boldsymbol {c}}} $$

and \(\dot{{\boldsymbol {c}}}\) should be a function of the crack-tip field \({\boldsymbol {t}}\) and the crack-tip velocity (with respect to the material) \({\boldsymbol {V}}\), postulated to be a field in this model. The crack-tip is identified by the field \({\boldsymbol {t}}\) and keeping within the confines of ‘local’ and simplest theory, it is natural to look for a relation of the type \(\dot {{\boldsymbol {c}}} = {\boldsymbol {f}}({\boldsymbol {t}}, {\boldsymbol {V}})\).

Possibly the simplest vector-valued function of two vectors that vanishes when either of them vanishes is \({\boldsymbol {t}}\times {\boldsymbol {V}}\). Noting that this is dimensionally consistent with the physical dimensions of \(\dot{{\boldsymbol {c}}}\), we assume, in this first instance, the relationship

$$ \dot{{\boldsymbol {c}}} = - \mathit{curl} {\boldsymbol {c}}\times{\boldsymbol {V}}. $$
(3)

To check its validity in a simple case, consider our previous ansatz of

$${\boldsymbol {c}}(x,y,t) = w(x,t) {\boldsymbol {e}}_{2} \quad\mbox{with}\quad {\boldsymbol {c}}(x,y,0) = w(x,0) {\boldsymbol {e}}_{2} = {\boldsymbol {c}}_{0}(x,y) := w_{0} (x) {\boldsymbol {e}}_{2}, $$

where \(w_{0}\) is a prescribed initial condition (assume in the form of a terminating ‘layer’ function), and

$${\boldsymbol {t}}= -\mathit{curl} {\boldsymbol {c}}= - e_{312} w_{,1} { \boldsymbol {e}}_{3} = - \partial_{x} w \, {\boldsymbol {e}}_{3}. $$

Now assume \({\boldsymbol {V}}= v {\boldsymbol {e}}_{1}\), \(v\) is a constant. Then

$$\dot{{\boldsymbol {c}}} = \partial_{t} w {\boldsymbol {e}}_{2} = {\boldsymbol {t}}\times{\boldsymbol {V}}= - v \partial _{x} w {\boldsymbol {e}}_{2} \implies {\boldsymbol {c}}(x,y,t) = w(x,t) {\boldsymbol {e}}_{2} = w_{0}(x-vt) {\boldsymbol {e}} _{2} = {\boldsymbol {c}}_{0}(x - vt, y), $$

and

$${\boldsymbol {t}}(x,y,t) = -\partial_{x} w (x,t)\, {\boldsymbol {e}}_{3} = - \partial_{x} w_{0} (x - vt) \, { \boldsymbol {e}}_{3} = {\boldsymbol {t}}_{0}(x-vt, y). $$

Thus, the crack-tip translates in the direction of \({\boldsymbol {V}}\), dragging out the crack-surface layer behind it.

If the previous argument for choosing the evolution equation (3) seemed somewhat ad-hoc and wanting regarding the general 3-d case, there exists a more fundamental argument justifying its form as we show in the following.

We note first that the local, point-wise statement (2) arising purely from the kinematics of the modeling implies

  1. 1.

    that \(\mathit{div} \,{\boldsymbol {t}}= 0\) which has the physical meaning that a crack-tip ‘line’ cannot end within the body—it either runs from boundary to boundary or forms a closed-loop. Given that the crack-tip is the boundary between a cracked and uncracked region, this makes sense, by physical definition.

  2. 2.

    A crack-tip carries a topological charge (given by \((\varphi _{0} - 0)\) in the case of the example considered before in Sect. 2). This is given by

    $$\int_{A} {\boldsymbol {t}}\cdot{\boldsymbol {n}}\, da = \int_{\partial A} - {\boldsymbol {c}}\cdot d{\boldsymbol {x}} $$

    by Stokes’ theorem and this holds for any area patch \(A\) (with oriented unit normal field \({\boldsymbol {n}}\)) threaded by the crack-tip, regardless of the size and orientation of the patch. We call it a topological property as it is a fixed property of any loop that encircles the core (viewed equivalently as the closed bounding curve of an area patch), regardless of the shape or size of the loop. This can be deduced simply by considering Fig. 2 and connecting the two end-patches by a cylindrical surface, and applying the divergence theorem to \(\mathit{div} \, {\boldsymbol {t}}= 0\) on the enclosed body and subsequently using Stokes’ theorem.

    Fig. 2
    figure 2

    Topological charge of a crack-tip

    Full size image

The local statement (2) implies the conservation law of evolution

$$ \frac{d}{dt} \int_{A} {\boldsymbol {t}}\cdot{\boldsymbol {n}}\, da = - \int_{\partial A} \dot{{\boldsymbol {c}} } \cdot d{\boldsymbol {x}}\quad \mbox{for any area patch} \ A. $$
(4)

So what should the flow \(- \dot{{\boldsymbol {c}}}\) on the boundary curves of arbitrary patches be? Now, \(\int_{A} {\boldsymbol {t}}\cdot {\boldsymbol {n}}\,da\) is the net topological charge of all crack-tips threading the patch \(A\), its time-derivative is the rate of change of the total topological charge content of the patch, and it is a physical tautology that this rate of change should be equal to the charge carried in minus what is carried out by crack-tips entering and exiting the patch through its bounding curve (we discuss the possibility of nucleation at the end of this section). To see what this amounts to, consider the patch \(A\) and a local orthonormal frame \(({\boldsymbol {p}}_{1}, {\boldsymbol {p}}_{2}, {\boldsymbol {p}}_{3})\) as shown in Fig. 3 based at a point on the boundary curve of \(A\). We consider the infinitesimal tangent patch as shown. We also choose the orientation of the frame such that \({\boldsymbol {p}}_{1}\) points along the tangent to \(\partial A\) and neither \({\boldsymbol {p}}_{2}\) nor \({\boldsymbol {p}}_{3}\) are parallel to the area patch (this can always be arranged and represents no loss of generality). Now consider the term \(- {\boldsymbol {t}}\times{\boldsymbol {V}}\) as a candidate for the flow:

$$ \begin{aligned} - ({\boldsymbol {t}}\times{\boldsymbol {V}}) \cdot d{ \boldsymbol {x}}&= - ({\boldsymbol {t}}\cdot{{\boldsymbol {p}}}_{i}) ({{ \boldsymbol {p}}}_{i} \times{\boldsymbol {V}}) \cdot dx {{\boldsymbol {p}}}_{1} \\ &= - dx ({\boldsymbol {t}}\cdot{{\boldsymbol {p}}}_{1}) \bigl\{ { \boldsymbol {p}}_{1} \times ( V_{1} {\boldsymbol {p}}_{1} + V_{2} {\boldsymbol {p}}_{2} + V_{3} {\boldsymbol {p}}_{3} ) \bigr\} \cdot{\boldsymbol {p}}_{1} \\ &\quad{} - dx ({\boldsymbol {t}}\cdot{{\boldsymbol {p}}}_{2}) \bigl\{ { \boldsymbol {p}}_{2} \times ( V_{1} {\boldsymbol {p}}_{1} + V_{2} {\boldsymbol {p}}_{2} + V_{3} {\boldsymbol {p}}_{3} ) \bigr\} \cdot {\boldsymbol {p}}_{1} \\ &\quad{}- dx ({\boldsymbol {t}}\cdot{{\boldsymbol {p}}}_{3}) \bigl\{ {\boldsymbol {p}}_{3} \times ( V_{1} {\boldsymbol {p}}_{1} + V_{2} {\boldsymbol {p}}_{2} + V_{3} {\boldsymbol {p}}_{3} ) \bigr\} \cdot {\boldsymbol {p}}_{1} \end{aligned} $$

The first term is obviously 0; physically, \({\boldsymbol {p}}_{1}\) moving in direction \({\boldsymbol {p}}_{1}\) does not move segment \({\boldsymbol {p}}_{1}\) into the patch; \({\boldsymbol {p}}_{1}\) moving in the direction \({\boldsymbol {p}}_{2}\) does not produce an intersection of \({\boldsymbol {p}}_{1}\) with the tangent patch \(A\), and similarly \({\boldsymbol {p}}_{1}\) moving in the direction \({\boldsymbol {p}}_{3}\) does not either. Arguing similarly, for the second term we have that the only term that contributes is

$$-dx({\boldsymbol {t}}\cdot{\boldsymbol {p}}_{2}) V_{3} ({ \boldsymbol {p}}_{2} \times{\boldsymbol {p}}_{3}) \cdot{ \boldsymbol {p}}_{1} $$

and the third contributes

$$-dx({\boldsymbol {t}}\cdot{\boldsymbol {p}}_{3}) V_{2} ({ \boldsymbol {p}}_{3} \times{\boldsymbol {p}}_{2}) \cdot{ \boldsymbol {p}}_{1}. $$

Therefore,

$$-({\boldsymbol {t}}\times{\boldsymbol {V}}) \cdot d{\boldsymbol {x}}= - dx({ \boldsymbol {t}}\cdot{\boldsymbol {p}}_{2}) V_{3} + dx ({ \boldsymbol {t}}\cdot{\boldsymbol {p}}_{3}) V_{2}. $$

The first term identifies the crack-tip curve \({\boldsymbol {t}}\)’s component along \({\boldsymbol {p}}_{2}\) bringing in charge \(- ({\boldsymbol {t}}\cdot {\boldsymbol {p}}_{2}) V_{3} dx\) into the area patch per unit time (the minus sign since \({\boldsymbol {p}}_{2}\) is directed inwards into the projection, of the infinitesimal area with normal \({\boldsymbol {n}} \), on the plane spanned by \({\boldsymbol {p}}_{1}\) and \({\boldsymbol {p}}_{3}\)) and a similar argument holds for the other term.

Fig. 3
figure 3

Set-up for rationalizing flow of crack-tip topological charge across patch boundaries

Full size image

Therefore, \(-{\boldsymbol {t}}({\boldsymbol {x}}) \times {\boldsymbol {V}}({\boldsymbol {x}}) \cdot d{\boldsymbol {x}}\) is exactly the flow of topological charge per unit time brought in by the field \({\boldsymbol {t}}({\boldsymbol {x}})\) crossing the element \(d{\boldsymbol {x}}\) of \(\partial A\) at the point \({\boldsymbol {x}}\) with velocity \({\boldsymbol {V}}({\boldsymbol {x}})\) (and this quantity depends only on \({\boldsymbol {t}}\), \({\boldsymbol {V}}\) and \(\partial A\)). Consequently, localizing the conservation statement (4) we obtain its local statement

$$ \dot{{\boldsymbol {t}}} = - \mathit{curl} ({\boldsymbol {t}}\times{\boldsymbol {V}}). $$
(5)

While (5) implies an evolution equation for the crack field \({\boldsymbol {c}}\) of the form

$$ \dot{{\boldsymbol {c}}} = -\mathit{curl} {\boldsymbol {c}}\times{\boldsymbol {V}} $$
(6)

up to a gradient, our derivation for the flow shows that the gradient actually vanishes, when the crack field \({\boldsymbol {c}}\) is restricted to evolve only by motion of the crack-tip field \({\boldsymbol {t}}\) (and not by transverse motion of the crack surfaces, for example—see [2] Sect. 5.4.3).

In the conservation law \(\dot{{\boldsymbol {t}}} = - \mathit{curl} ({\boldsymbol {t}}\times{\boldsymbol {V}})\) one could allow for a source-term allowing nucleation that would represent the emergence of crack-tips in the area patch but always “within a \(\mathit{curl}\)” so as not to violate the relation \(\dot{{\boldsymbol {t}}} = - \mathit{curl} \dot{{\boldsymbol {c}} }\). This also implies that as long as crack-tips are not inserted from outside the whole body under consideration, the crack-tips nucleated must be closed loops or ensure that the total topological charge within the whole body does not evolve in time.

Thus, the governing equations for the model are

$$ \begin{aligned} & \rho= \frac{\rho_{0}}{J} \quad\Leftrightarrow \quad\dot{\rho} = - \rho\, \mathit{div} \, {\boldsymbol {v}} \\ & \rho_{0} \ddot{{\boldsymbol {u}}} = \mathit{div} \, {\boldsymbol {T}}+ { \boldsymbol {b}} \\ & \dot{{\boldsymbol {c}}} = - \mathit{curl} {\boldsymbol {c}}\times{\boldsymbol {V}}, \end{aligned} $$
(7)

where \(\rho_{0}\) is the time-independent mass density field corresponding to the reference configuration of the body from which all displacements are measured, \(\rho\) is the evolving density field on the body, \({\boldsymbol {T}}\) is the symmetric stress tensor (a small deformation approximation to the First-Piola Kirchhoff stress tensor), \({\boldsymbol {b}}\) is the body force density per unit volume of the reference configuration, \({\boldsymbol {u}}\) is the displacement field, \(\dot{{\boldsymbol {u}}} = {\boldsymbol {v}}\) is the material velocity field, and \(J\) is the determinant of the deformation gradient field. Also, all differential operators \(\mathit{div}\), \(\mathit{curl}\) are written with respect to a fixed reference configuration.

While it is entirely possible at this level of theorizing to include a crack-nucleation related source term with an attendant thermodynamic driving force obtained by standard methods, experience with dislocation mechanics [17, 29] shows that homogeneous nucleation may not be related to thermodynamic driving forces, but to linear instability of the finite deformation version of transport equations of the type (7)2 through a coupling of the \({\boldsymbol {c}}\) field to the material velocity field through a convected derivative, in the complete absence of a source term. While there are substantial differences between a crack-tip with a crack in its wake and a dislocation with a slipped region behind it, both crack-tips and dislocations induce strong stress concentrations around their core (albeit the crack stress ‘singularity’ is milder) and conditions on the state that may be assumed to provide nucleation based on thermodynamic grounds in a core can typically persist in the core after nucleation, thus creating an obvious inconsistency in the prediction of nucleation. For this reason, we refrain from including an explicit source term for crack nucleation in the model at this time.

3.1 The Crack Field \({\boldsymbol {c}}\) and Its Compatibility with a Scalar Density Field

The crack field \({\boldsymbol {c}}\) has been physically rationalized as some second-gradient measure of a local density field. We think of the mass density field on the body that enters the fundamental laws of balance of mass and balance of linear momentum as the field \(\rho\) of (7). It is then natural to ask whether the crack field \({\boldsymbol {c}} \) may correspond to any global density field(s) on the body, and what connection, if any, may exist between such fields and the mass density field \(\rho\). In other words, we also ask whether the introduction of a new field is really necessary and if its introduction actually brings degrees of freedom not available through the mass density field alone.

Our fundamental modeling philosophy is that the equations of classical continuum mechanics are incapable of describing the dynamics of its basic fields when they develop, at the ‘macroscopic’ scale of observation, discontinuities along surfaces, and discontinuities of these ‘surface-discontinuities’ along lines, except for certain very special cases; they are best adapted to modeling continuous situations. In essence, discontinuities and singularities of macroscopic fields are extra degrees of freedom whose kinematics and evolution need to be defined as necessary, and we wish to do this by using at least integrable representations of the gradients of the discontinuous fields. In order to do this, we adopt the standpoint that the gradient of an \(n\)th-order macroscopic field is replaced by a pair of \((n+1)\)th-order ‘microscopic’ tensor fields consisting of a smooth gradient of an \(n\)th-order tensor field and an \((n+1)\)th-order tensor field, the latter referred to as the ‘layer’ field. The theory then has to be designed so that the layer field, which is not a priori a gradient, resembles a smoothed representation of the singular macroscopic gradient over an interfacial layer, with support on the interfacial layer. For all occurrences of the original macroscopic gradient in specific energy (or its derivative) response functions, we replace the original occurrence by the difference of the microscopic gradient and the layer field.Footnote 2 We then let the burden of representing the energetics of the layer region be carried by appropriate constitutive behavior dependent on the layer field and its gradients. The evolution (and equilibria) of the layer field is further governed by additional equations dictated by a kinematic understanding of the ‘motion’ of the layer field. Coupling of the microscopic gradient and the layer field along with other macroscopic fields occurs through thermodynamics-guided constitutive response and the enhanced set of governing field equations (often, the macroscopic balance law for the (in general, tensor) potential field that generates the macroscopic gradient under consideration serves as the governing equation for the potential field for the microscopic gradient). Specific examples of this approach in representing the dynamics and interactions of discrete, non-singular line defects within a pde setting can be found in [45, 46].

We now ask the question of the existence of a scalar field, say \(\rho ^{*}\), the directional derivative of whose gradient field in the direction of \({\boldsymbol {n}}= \frac{{\boldsymbol {c}}}{|{\boldsymbol {c}}|}\) is the field \({\boldsymbol {c}}\), i.e.,

$$ \rho^{*}_{,ij} c_{j} = (\sqrt{c_{k} c_{k}}) c_{i}; \qquad \mathit{grad} \, \mathit{grad} \, \rho ^{*} \frac{{\boldsymbol {c}}}{|{\boldsymbol {c}}|} = {\boldsymbol {c}}, $$
(8)

with \({\boldsymbol {c}}\) assumed as a given field.

We first note that when the crack field \({\boldsymbol {c}}= \mathbf{0}\), any scalar field is a candidate solution for \(\rho^{*}\). Thus, when there are no cracks, the actual mass density field \(\rho\) serves as a trivial solution to the compatibility equation (8), which is a reassuring consistency check.

When \({\boldsymbol {c}}\neq\mathbf{0}\), (8) amounts to three equations in one variable, and smooth solutions may not be expected in general for all smoothly specified fields \({\boldsymbol {c}}\).

At any rate, the possibilities are that a smooth \(\rho^{*}\) field may exist satisfying (8), a continuous \(\rho^{*}\) satisfying (8) may not exist on the body but may be constructed satisfying the equation in an appropriate approximate sense (which needs to be made precise), and in either case they may not equal the field \(\rho\). Thus, the model separates the ‘macroscopic’ density field \(\rho\) from the ‘microscopic’ crack field \({\boldsymbol {c}}\) and any (possibly discontinuous) density field \(\rho^{*}\) that may be deemed consistent with the crack field. Of course, \(\rho\) and \(\rho^{*}\) may be penalized to be close energetically if so desired on physical grounds, or \((\mathit{grad}\,\mathit{grad}\, \rho) {\boldsymbol {c}}\) may be similarly penalized to be close to \(|{\boldsymbol {c}}| {\boldsymbol {c}}\), following the motivation provided earlier in this section.

We now consider the construction of the various possibilities for the field \(\rho^{*}\) for a given field \({\boldsymbol {c}}\).

A necessary condition for the existence of \(\rho^{*}\) satisfying (8) is that \({\boldsymbol {c}}\) be an eigenvector of \(\mathit{grad}\, \mathit{grad}\,\rho ^{*}\) with \(|{\boldsymbol {c}}|\) the corresponding eigenvalue. Hence, \(\mathit{grad}\,\mathit{grad}\,\rho ^{*}\) must be of the form

$$ {\boldsymbol {B}}+ |{\boldsymbol {c}}| \frac{{\boldsymbol {c}}}{|{\boldsymbol {c}}|} \otimes \frac{{\boldsymbol {c}}}{|{\boldsymbol {c}}|} \quad\mbox{with} \quad {\boldsymbol {B}}\ \mbox{symmetric, and}\ {\boldsymbol {B}} {\boldsymbol {c}}= \mathbf{0}. $$
(9)

Consequently, a necessary and sufficient condition for twice-continuously differentiable solutions to (8) to exist in a simply connected domain is that smooth solutions exist to the Pfaffian system of equations

$$ \begin{aligned} \partial_{i} \rho^{*} & = f_{i} \\ \partial_{j} f_{i} & = B_{ij} + \frac{1}{|{\boldsymbol {c}}|} c_{i} c_{j}, \end{aligned} $$
(10)

with the stipulated requirements on the field \({\boldsymbol {B}}\) mentioned in (9). The compatibility condition for existence of solutions to this system is

$$ \mathit{curl} {\boldsymbol {B}}= - \mathit{curl} \biggl( \frac{1}{|{\boldsymbol {c}}|} { \boldsymbol {c}}\otimes{\boldsymbol {c}} \biggr). $$
(11)

We note that the requirements that \({\boldsymbol {B}}\) be a symmetric tensor and \({\boldsymbol {B}}{\boldsymbol {c}}= \mathbf{0}\) prevent application of standard methods, e.g., the Riemann-Graves operator method [12] or [21] without modification to obtain a solution. The question of existence of (local) solutions appears to lie in the domain of applications of the Cartan-Kähler theorem [20] which is beyond the scope of this paper. In any case, if a solution to (11) can be constructed, then smooth solutions \(\rho^{*}\) satisfying (8) can also be constructed, compatible with \({\boldsymbol {c}}\).

Suppose sufficiently smooth solutions to (11) cannot be constructed. We then look into the possibility of characterizing the class of discontinuous solutions of (10) that may be constructed consistent with \({\boldsymbol {c}}\) in the class for which the \(\mathit{curl}\) of the right-hand-side of (10)2 is assumed to be non-vanishing only in a (topologically equivalent shape to a) cylinder of small radius in the body that runs from boundary to boundary (a torus along a loop would also work).

With reference to the discussion in Sect. 2, if \(\varOmega \) is the body with the \(z\)-axis running through it, the layer \(L = \varOmega\cap\{ (x,y,z) \mid x \leq0, -\frac{c_{w}}{2} \leq y \leq\frac {c_{w}}{2} \}\), the crack-tip \(\varOmega_{c} = \varOmega\cap\{ (x,y,z) \mid - l_{c} \leq x \leq0, -\frac{c_{w}}{2} \leq y \leq\frac{c_{w}}{2} \}\) and we define the field \({\boldsymbol {c}}\) in \(\varOmega\backslash\varOmega_{c}\) as

$$ {\boldsymbol {c}}({\boldsymbol {x}}) = \textstyle\begin{cases} \frac{\varphi{\boldsymbol {e}}_{2}}{c_{w}} &\mbox{for}\ {\boldsymbol {x}}\in L \backslash\varOmega_{c} \\ \mathbf{0}&\mbox{in}\ \varOmega\backslash L, \end{cases} $$
(12)

with \(\varphi\) a constant, then

$$ \begin{aligned} \biggl\{ \mathit{curl} \biggl( \frac{1}{|{\boldsymbol {c}}|} {\boldsymbol {c}} \otimes{\boldsymbol {c}} \biggr) \biggr\} _{ri} & = e_{ijk} \biggl( \frac{1}{\sqrt{c_{m} c_{m}}} c_{r} c_{k} \biggr)_{,j} \\ & = e_{ijk} \biggl[ -\frac{1}{|{\boldsymbol {c}}|^{3}} c_{m} c_{m,j} c_{r} c_{k} + \frac {1}{|{\boldsymbol {c}}|} c_{r,j} c_{k} + \frac{1}{|{\boldsymbol {c}}|} c_{r} c_{k,j} \biggr] \end{aligned} $$

in \(\varOmega\backslash\varOmega_{c}\). Since outside \(\varOmega_{c}\) the field \({\boldsymbol {c}}\) varies only in the 2-direction and is itself oriented in the same direction, the first two terms in parenthesis vanish. The \(\mathit{curl}\) of \({\boldsymbol {c}}\) vanishes outside the core (despite the large gradients in \({\boldsymbol {c}}\) across the lateral layer boundaries) and so \(\mathit{curl} \, {\boldsymbol {B}}\) has to vanish as well here. Furthermore, since \({\boldsymbol {c}}\) is a constant vector field in the layer, it is easy to construct (a family) of symmetric \({\boldsymbol {B}}\) fields that satisfy \({\boldsymbol {B}}{\boldsymbol {c}}= \mathbf{0}\) in the body with crack-tip cylinder excluded, e.g., let \({\boldsymbol {B}}= a_{1} {\boldsymbol {e}}_{1} \otimes{\boldsymbol {e}} _{1} + a_{3} {\boldsymbol {e}}_{3} \otimes{\boldsymbol {e}}_{3}\), where \({\boldsymbol {e}}_{1}, {\boldsymbol {e}}_{3}\) are constant unit vectors perpendicular to \({\boldsymbol {c}}= {\boldsymbol {e}}_{2}\) and \(a_{1}, a_{3}\) are any scalar constants. Every pair of such a \({\boldsymbol {B}}\) field and the \({\boldsymbol {c}}\) field would be an explicit example of a generator of the right-hand-side of (10)2 that does not satisfy (11) at most within the crack-tip cylinder.

We first consider some results for the case of general \({\boldsymbol {c}}\) fields that satisfy the conditions mentioned in the paragraph before the last outside crack-tip cylinders, i.e., corresponding to the \({\boldsymbol {c}}\) field, we assume a \({\boldsymbol {B}}\) can be constructed such that (11) is satisfied in \(\varOmega\backslash\varOmega_{c}\). We continue to refer to the body as \(\varOmega\) and the crack-tip cylinder as \(\varOmega_{c}\). We have that \(\varOmega\backslash\varOmega_{c}\) is non-simply connected on which the right-hand-side of (10)2 is \(\mathit{curl}\)-free. Let \(S\) define a ‘cut-surface’ in \(\varOmega\backslash\varOmega_{c}\) as a surface that connects a curve on the boundary of \(\varOmega_{c}\) to another on the boundary of \(\varOmega\) such that

$$(\varOmega\backslash\varOmega_{c})\backslash S =: D^{S} $$

is rendered simply-connected. On each such cut-surface-induced simply-connected domain \(D^{S}\), a vector field \({\boldsymbol {f}}^{S}\) can be constructed satisfying (10)2 by elementary arguments of vector analysis. Furthermore, due to the symmetry of \(f^{S}_{i,j}\) in \(\{ i,j \}\), a \(\rho^{*S}\) can also be constructed on the same domain \(D^{S}\).

Following the line of argument in Sect. 5.4.7 of [2] exactly, it is now possible to show that the jump \([\!\![{\boldsymbol {f}}^{S} ]\!\!]\) in \({\boldsymbol {f}}^{S}\) across its corresponding cut-surface \(S\) is a constant vector, say \(\boldsymbol{\delta} \), regardless of the \(S\) and \({\boldsymbol {f}}^{S}\) involved, i.e., \(\boldsymbol{\delta}\) is independent of the cut-surface, the function induced by it, and the position on any cut-surface. It can also be shown that the jump in each \(\rho^{*S}\) (whose definition does depend on the cut-surface chosen) can be characterized as

$$\bigl[\!\!\bigl[\rho^{*S} \bigr]\!\!\bigr]({\boldsymbol {x}}) = \bigl[\!\!\bigl[\rho^{*S} \bigr]\!\!\bigr]({\boldsymbol {x}}_{0}) + \boldsymbol{ \delta}\cdot ({\boldsymbol {x}}- {\boldsymbol {x}}_{0}) $$

for arbitrary choices of \({\boldsymbol {x}}\in S\) and \({\boldsymbol {x}}_{0} \in S\). This may be considered an analog of Weingarten’s theorem in dislocation theory [30] for the treatment of fracture presented herein. To see the non-trivial dependence of the constructed, possibly discontinuous, family of \(\rho^{*S}\) on the surface \(S\), note that a choice of \(S\) as a planar surface perpendicular to \(\boldsymbol{\delta}\neq\mathbf {0}\) implies that the discontinuity of the corresponding \(\rho^{*S}\) is constant on the surface. However, on an admissible \(S\) that does not satisfy this orthogonality condition, e.g., a non-planar cut-surface (with \(\boldsymbol{\delta} \neq \mathbf{0}\)), the jump in \(\rho^{*S}\) cannot be constant on the surface. It can also be shown (following the arguments in Sect. 6.2 of [44]) that when \(\boldsymbol{\delta}= \mathbf{0}\) the jump \([\!\![\rho^{*S} ]\!\!]\) is a constant scalar, regardless of the \(S\) and \(\rho^{*S}\) chosen to define it.

Thus, even if (11) were to be satisfied in all of the domain except a thin cylinder, the corresponding \({\boldsymbol {c}}\) field would, in general, correspond to a (non-trivial) multitude of discontinuous scalar \(\rho^{*}\) fields. Such ‘non-uniqueness’ is not typically eliminated by the application of standard devices like boundary conditions on \(\partial\varOmega\) and this provides an intuitive sense of the problems that arise if macroscopic gradients were to develop singularities of the type being discussed here when solved in conjunction with macroscopic governing equations of balance. The exercise also answers the question asked at the beginning of this section as to whether the introduction of the \({\boldsymbol {c}}\) field can in general be accommodated by simply considering higher-gradients of a smooth scalar density field.

We end this section by explicitly calculating the jumps of the \(\rho ^{*S}\) and \({\boldsymbol {f}}^{S}\) fields corresponding to the specific \({\boldsymbol {c}}\) field considered in Sect. 2, with a natural choice of the surface \(S\) and \({\boldsymbol {B}}= \mathbf{0}\).

With reference to Fig. 4 and (12), \(S\) is chosen as

$$ S = \varOmega\cap \bigl\{ (x,y,z)\bigm| y = 0, x < -l_{c} \bigr\} . $$
(13)

Let \({\boldsymbol {x}}_{0} \in S\) be a point on the cut-surface \(S\). Let \({\boldsymbol {x}}_{0}^{+}\) and \({\boldsymbol {x}}_{0}^{-}\) be points in \(D^{S}\), arbitrarily close to \({\boldsymbol {x}}_{0}\), and above and below \({\boldsymbol {x}}_{0}\), respectively. Defining \({\boldsymbol {C}}:= \frac{1}{|{\boldsymbol {c}} |} {\boldsymbol {c}}\otimes{\boldsymbol {c}}\), we need to compute the line integral

$$\boldsymbol{\delta}= \lim_{{\scriptstyle {\boldsymbol {x}}^{+}_{0} \rightarrow{\boldsymbol {x}}_{0} \atop\scriptstyle {\boldsymbol {x}}^{-}_{0} \rightarrow{\boldsymbol {x}}_{0}}} \int_{{\boldsymbol {x}}_{0}^{-}}^{{\boldsymbol {x}}_{0}^{+}} {\boldsymbol {C}}d{\boldsymbol {x}} $$

along a contour shown in Fig. 4. As mentioned before, solutions to (10) exist on \(D^{S}\) (when (11) is satisfied there). Any such function \({\boldsymbol {f}}^{S}\) can be represented as \({\boldsymbol {f}}^{S}({\boldsymbol {x}}; {\boldsymbol {y}}) = {\boldsymbol {f}}^{S} ({\boldsymbol {y}}) + \int_{{\boldsymbol {y}}}^{{\boldsymbol {x}}} {\boldsymbol {C}}d{\boldsymbol {x}}\) for arbitrarily fixed \({\boldsymbol {y}}\in D^{S}\) and is unique up to a constant vector field on \(D^{S}\). We now define

$$ {\boldsymbol {f}}^{S}({\boldsymbol {x}}; {\boldsymbol {x}}_{0}) := \lim_{{\boldsymbol {x}}^{-}_{0} \rightarrow{\boldsymbol {x}}_{0}} \biggl( {\boldsymbol {f}} ^{S} \bigl({\boldsymbol {x}}_{0}^{-} \bigr) + \int_{{\boldsymbol {x}}_{0}^{-}}^{{\boldsymbol {x}}} {\boldsymbol {C}}d{\boldsymbol {x}} \biggr), $$
(14)

along any path from \({\boldsymbol {x}}^{-}_{0}\) to \({\boldsymbol {x}}\) contained in \(D^{S}\), and in the following we drop the superscript \(S\) and the parametric dependence on \({\boldsymbol {x}}_{0}\) of \({\boldsymbol {f}}^{S}\) (and later \(\rho^{*S}\)) for notational simplicity.

Fig. 4
figure 4

Contour for calculating jumps in \({\boldsymbol {f}}^{S}\) and \(\rho^{*S}\)

Full size image

We also denote the constant value of \({\boldsymbol {c}}\) in the layer by \({\boldsymbol {c}}_{0}\). Integrating along the path \({\boldsymbol {x}}(s) = {\boldsymbol {x}}_{o}^{-} - s {\boldsymbol {e}}_{2}\),

$$\begin{aligned} \lim_{{\boldsymbol {x}}^{-}_{0} \rightarrow{\boldsymbol {x}}_{0}} {\boldsymbol {f}} \biggl( {\boldsymbol {x}}^{-}_{0} - \frac{c_{w}}{2} {\boldsymbol {e}}_{2} \biggr) &= \lim_{{\boldsymbol {x}}^{-}_{0} \rightarrow {\boldsymbol {x}}_{0}} {\boldsymbol {f}} \bigl({\boldsymbol {x}}_{0}^{-} \bigr) + \int_{0}^{\frac{c_{w}}{2}} \biggl( \frac{{\boldsymbol {c}}_{0}}{|{\boldsymbol {c}}_{0}|} \otimes|{ \boldsymbol {c}}_{0}| {\boldsymbol {e}}_{2} \biggr) (- { \boldsymbol {e}}_{2}) ds \\& = \lim_{{\boldsymbol {x}}^{-}_{0} \rightarrow{\boldsymbol {x}}_{0}} {\boldsymbol {f}} \bigl({\boldsymbol {x}}_{0}^{-} \bigr) - \frac{c_{w}}{2} {\boldsymbol {c}}_{0}. \end{aligned}$$
(15)

\({\boldsymbol {f}}\) remains constant from \({\boldsymbol {x}}_{0} - \frac {c_{w}}{2} {\boldsymbol {e}}_{2}\) to \({\boldsymbol {x}}_{0} + \frac{c_{w}}{2} {\boldsymbol {e}}_{2}\) along the path shown since \({\boldsymbol {c}}\) vanishes there. The computation is essentially the same as before for the path from \({\boldsymbol {x}}_{0} + \frac{c_{w}}{2} {\boldsymbol {e}}_{2}\) to \({\boldsymbol {x}}_{0}^{+}\) and hence we obtain

$$\begin{aligned} \lim_{{\boldsymbol {x}}^{+}_{0} \rightarrow{\boldsymbol {x}}_{0}} {\boldsymbol {f}} \bigl({\boldsymbol {x}}_{0}^{+} \bigr)& = \lim_{{\boldsymbol {x}}^{+}_{0} \rightarrow{\boldsymbol {x}}_{0}} \biggl( {\boldsymbol {f}} \biggl( {\boldsymbol {x}}^{+}_{0} + \frac{c_{w}}{2} {\boldsymbol {e}}_{2} \biggr) + \int_{{\boldsymbol {x}}^{+}_{0} + \frac{c_{w}}{2} {\boldsymbol {e}}_{2}}^{{\boldsymbol {x}}_{0}^{+}} {\boldsymbol {C}}d{\boldsymbol {x}} \biggr) \\ & = \lim_{{\boldsymbol {x}}^{-}_{0} \rightarrow{\boldsymbol {x}}_{0}} {\boldsymbol {f}} \biggl( {\boldsymbol {x}}^{-}_{0} - \frac{c_{w}}{2} {\boldsymbol {e}}_{2} \biggr) - \frac{c_{w}}{2} {\boldsymbol {c}}_{0}. \end{aligned}$$
(16)

Combining (15) and (16) we have

$$ [\!\![{\boldsymbol {f}}]\!\!]({\boldsymbol {x}}_{0}) = \lim_{{\boldsymbol {x}}^{+}_{0} \rightarrow{\boldsymbol {x}} _{0}} {\boldsymbol {f}} \bigl({ \boldsymbol {x}}_{0}^{+} \bigr) - \lim_{{\boldsymbol {x}}^{-}_{0} \rightarrow{\boldsymbol {x}}_{0}} {\boldsymbol {f}} \bigl({\boldsymbol {x}}_{0}^{-} \bigr) = -c_{w} {\boldsymbol {c}}_{0}, $$
(17)

and, as mentioned before, this is the value of the constant \(\boldsymbol{\delta}\) for the \({\boldsymbol {c}}, {\boldsymbol {B}}\) considered here, independent of \({\boldsymbol {x}}_{0} \in S\) as well as of \(S\).

We now wish to construct the jump of \(\rho^{*S}\) across \(S\) (recalling the notational convenience set up earlier). Further, we also use the short-hand

$$g_{0}^{+/-} := \lim_{{\boldsymbol {x}}_{0}^{+/-} \rightarrow{\boldsymbol {x}}_{0}} g \bigl({ \boldsymbol {x}} _{0}^{+/-} \bigr), $$

for any function \(g\) defined on \(D^{S}\). We have

$$\rho^{*}({\boldsymbol {x}}) = \rho^{*-}_{0} + \lim _{{\boldsymbol {x}}_{0}^{-} \rightarrow{\boldsymbol {x}}_{0}} \int _{{\boldsymbol {x}}_{0}^{-}}^{{\boldsymbol {x}}} {\boldsymbol {f}}\cdot d{\boldsymbol {x}}. $$

The function \(\rho^{*}\) satisfies \(\mathop{\rm \mathit{grad}}\nolimits\rho^{*} = {\boldsymbol {f}}\) on \(D^{S}\) regardless of \({\boldsymbol {x}}_{0} \in S\) and the value \(\rho^{*-}\) and since all admissible \({\boldsymbol {f}}\) can only differ by a constant vector field, a solution \(\rho^{*}\) is unique up to an affine function on \(D^{S}\).

Along the path \({\boldsymbol {x}}(s) = {\boldsymbol {x}}_{0} - s {\boldsymbol {e}}_{2}, 0 \leq s \leq\frac {c_{w}}{2}\), \({\boldsymbol {f}}(s) = -s {\boldsymbol {c}}_{0} + {\boldsymbol {f}}_{0}^{-}\). Hence,

$$\begin{aligned} \rho^{*} \biggl({\boldsymbol {x}}_{0} - \frac{c_{w}}{2} {\boldsymbol {e}}_{2} \biggr) & = \rho^{*-} + \int _{0}^{\frac{c_{w}}{2}} - s |{\boldsymbol {c}}_{0}| {\boldsymbol {e}}_{2} \cdot (-{\boldsymbol {e}}_{2}) \, ds - \frac {c_{w}}{2} {\boldsymbol {f}}^{-}_{0} \cdot{\boldsymbol {e}}_{2} \\ & = \rho^{*-}_{0} + |{\boldsymbol {c}}_{0}| \frac{c_{w}^{2}}{8} - \frac {c_{w}}{2} {\boldsymbol {f}}^{-}_{0} \cdot{ \boldsymbol {e}}_{2}. \end{aligned}$$
(18)

Since \({\boldsymbol {f}}(s)\) takes the constant value \({\boldsymbol {f}}^{-}_{0} - \frac{c_{w}}{2} |{\boldsymbol {c}} _{0}| {\boldsymbol {e}}_{2}\) from \({\boldsymbol {x}}_{0} - \frac{c_{w}}{2} {\boldsymbol {e}}_{2}\) to \({\boldsymbol {x}}_{0} + \frac {c_{w}}{2} {\boldsymbol {e}}_{2}\) along the path, using (18) we have

$$\begin{aligned} \rho^{*} \biggl({\boldsymbol {x}}_{0} + \frac{c_{w}}{2} {\boldsymbol {e}}_{2} \biggr) & = \rho^{*} \biggl({\boldsymbol {x}} _{0} - \frac{c_{w}}{2} {\boldsymbol {e}}_{2} \biggr) + \int_{{\boldsymbol {x}}_{0} - \frac{c_{w}}{2} {\boldsymbol {e}} _{2}}^{{\boldsymbol {x}}_{0} + \frac{c_{w}}{2} {\boldsymbol {e}}_{2}} {\boldsymbol {f}}\cdot d{\boldsymbol {x}} \\ & = \rho^{*-}_{0} + |{\boldsymbol {c}}_{0}| \frac{c_{w}^{2}}{8} - \frac{c_{w}}{2} {\boldsymbol {f}}^{-}_{0} \cdot{ \boldsymbol {e}}_{2} - |{\boldsymbol {c}}_{0}| \frac{c_{w}^{2}}{2} + c_{w} {\boldsymbol {f}}^{-}_{0} \cdot{\boldsymbol {e}}_{2}. \end{aligned}$$
(19)

Now

$$\begin{aligned} \int^{{\boldsymbol {x}}_{0}}_{{\boldsymbol {x}}_{0} + \frac {c_{w}}{2}{\boldsymbol {e}}_{2}} {\boldsymbol {f}}\cdot d{\boldsymbol {x}}& = \int _{0}^{\frac{c_{w}}{2}} \biggl[ {\boldsymbol {f}} \biggl( { \boldsymbol {x}}_{0} + \frac{c_{w}}{2} {\boldsymbol {e}}_{2} \biggr) - s {\boldsymbol {c}}_{0} \biggr] \cdot(-{\boldsymbol {e}}_{2}) ds \\ & = \int^{\frac{c_{w}}{2}}_{0} \biggl[ {\boldsymbol {f}}^{-}_{0} - \frac {c_{w}}{2} |{\boldsymbol {c}}_{0}| { \boldsymbol {e}} _{2} - s|{\boldsymbol {c}}_{0}|{\boldsymbol {e}}_{2} \biggr] \cdot (-{\boldsymbol {e}}_{2}) ds \\ & = \frac{c_{w}^{2}}{4} |{\boldsymbol {c}}_{0}| - \frac{c_{w}}{2} { \boldsymbol {f}}^{-}_{0} \cdot{\boldsymbol {e}}_{2} + \frac{c_{w}^{2}}{8} |{\boldsymbol {c}}_{0}|, \end{aligned}$$
(20)

and combining (19) and (20) we obtain

$$\begin{aligned} &\rho^{*+}_{0} = \rho^{*} \biggl({\boldsymbol {x}}_{0} + \frac{c_{w}}{2} {\boldsymbol {e}}_{2} \biggr) + \int ^{{\boldsymbol {x}}_{0}}_{{\boldsymbol {x}}_{0} + \frac{c_{w}}{2}{\boldsymbol {e}}_{2}} {\boldsymbol {f}}\cdot d{\boldsymbol {x}}= \rho ^{*-}_{0} \Longrightarrow\bigl[\!\!\bigl[\rho^{*} \bigr]\!\!\bigr]({\boldsymbol {x}}_{0}) := \rho ^{*+}_{0} - \rho^{*-}_{0} = 0 \\ & \quad \mbox{for}\ {\boldsymbol {x}}_{0} \in S. \end{aligned}$$
(21)

Let \((\rho_{1}^{*}, {\boldsymbol {f}}_{1})\), \((\rho_{2}^{*}, {\boldsymbol {f}}_{2})\) be two pairs of solutions that satisfy (10) on \(D^{S}\). Since any two \({\boldsymbol {f}}\) that satisfy these equations can only differ by a constant vector, we have

$$\begin{aligned} &{\boldsymbol {f}}_{1} \bigl({\boldsymbol {x}}^{+} \bigr) - {\boldsymbol {f}}_{2} \bigl({\boldsymbol {x}}^{+} \bigr) = {\boldsymbol {f}}_{1} \bigl({\boldsymbol {x}}^{-} \bigr) - {\boldsymbol {f}}_{2} \bigl({\boldsymbol {x}}^{-} \bigr), \quad\mbox{for}\ {\boldsymbol {x}}^{+}, {\boldsymbol {x}}^{-} \in D^{S} \\ \Longrightarrow&{\boldsymbol {f}}_{1} \bigl({\boldsymbol {x}}^{+} \bigr) - {\boldsymbol {f}}_{1} \bigl({\boldsymbol {x}}^{-} \bigr) = { \boldsymbol {f}}_{2} \bigl({\boldsymbol {x}}^{+} \bigr) - {\boldsymbol {f}}_{2} \bigl({\boldsymbol {x}}^{-} \bigr), \end{aligned}$$

and now choosing sequences of \({\boldsymbol {x}}^{+}\) and \({\boldsymbol {x}}^{-}\) converging to \({\boldsymbol {x}} \in S\) from above and below \(S\) and taking limits we obtain that the jump function \([\!\![{\boldsymbol {f}}]\!\!]\) on \(S\) is unique. Since any two admissible \({\boldsymbol {f}}\)s can differ by a constant vector, say \({\boldsymbol {a}}\), then the corresponding \(\rho^{*}\)s can only differ affinely, i.e., \(\rho_{1}^{*}({\boldsymbol {y}}) - \rho_{2}^{*}({\boldsymbol {y}}) = {\boldsymbol {a}}\cdot( {\boldsymbol {y}}-{\boldsymbol {y}}_{0}) + (\rho_{1}^{*} -\rho_{2}^{*})({\boldsymbol {y}}_{0})\) for all \({\boldsymbol {y}}\in D^{S}\) and any arbitrarily fixed \({\boldsymbol {y}}_{0} \in D^{S}\). Then

$$ \begin{aligned} & \bigl(\rho_{1}^{*} \bigl({\boldsymbol {x}}^{+} \bigr) - \rho_{2}^{*} \bigl({\boldsymbol {x}}^{+} \bigr) \bigr) - \bigl( \rho _{1}^{*} \bigl({\boldsymbol {x}}^{-} \bigr) - \rho_{2}^{*} \bigl({ \boldsymbol {x}}^{-} \bigr) \bigr) = {\boldsymbol {a}}\cdot \bigl({\boldsymbol {x}}^{+} - { \boldsymbol {x}}^{-} \bigr),\quad {\boldsymbol {x}}^{+}, {\boldsymbol {x}}^{-} \in D^{S} \\ \Longrightarrow& \bigl(\rho_{1}^{*} \bigl({\boldsymbol {x}}^{+} \bigr) - \rho _{1}^{*} \bigl({\boldsymbol {x}}^{-} \bigr) \bigr) - \bigl( \rho_{2}^{*} \bigl({\boldsymbol {x}}^{+} \bigr) - \rho_{2}^{*} \bigl({ \boldsymbol {x}}^{-} \bigr) \bigr) = {\boldsymbol {a}}\cdot \bigl({\boldsymbol {x}}^{+} - { \boldsymbol {x}}^{-} \bigr), \end{aligned} $$

and again taking sequences of \({\boldsymbol {x}}^{+}\) and \({\boldsymbol {x}}^{-}\) converging to \({\boldsymbol {x}} \in S\), we find that the jump function \([\!\![\rho^{*} ]\!\!]\) on \(S\) is unique. Of course, the uniqueness of the jump functions \([\!\![{\boldsymbol {f}}]\!\!]\) and \([\!\![\rho ^{*} ]\!\!]\) also follows from the observation that the results (17) and (21) hold for any pair \(({\boldsymbol {f}}, \rho ^{*})\) that solves (10) on \(D^{S}\) as shown.

We find that in this specific case (corresponding to \({\boldsymbol {c}}\) defined in (12) and \(S\) in (13)) leading up to (21), the \(\rho^{*S}\) field is continuous in \(\varOmega \backslash\varOmega_{c}\) but its gradient is not. We have established here the ‘converse’ of the heuristic construction of the \({\boldsymbol {c}}\) field from the \(\tilde{\rho}\) field in Sect. 2 outside the crack-tip region; the exercise also provides an example of a cut-surface \(S\) and its corresponding \(\rho^{*S}\) field with jump in \(\rho^{*S}\) constant on \(S\) when \(\boldsymbol{\delta}\neq\mathbf{0}\).

4 Reversible Response Functions and Driving Forces for Dissipation

We consider mechanical effects only. Assume a free-energy density function (per unit volume of reference configuration) given as

$$\psi \bigl(\boldsymbol{\varepsilon}^{e}, {\boldsymbol {c}},{\boldsymbol {t}} \bigr) $$

where \(\boldsymbol{\varepsilon}^{e} = \boldsymbol{\varepsilon}- \boldsymbol{\varepsilon}^{p}\) and \(\boldsymbol{\varepsilon}= \mathit{sym}(\mathit{grad}{\boldsymbol {u}})\), with \({\boldsymbol {u}}\) being the displacement field, and \(\boldsymbol {\varepsilon}^{p}\) is the symmetric plastic strain tensor (that we include herein simply to show that the crack-driving force derived subsequently accommodates general, inelastic material response in the bulk).

The mechanical dissipation is defined as the power supplied by the external forces (tractions and body forces) less the rate of change of kinetic energy and the power stored in the body:

$$ \mathsf{D} = \int_{\partial V} {\boldsymbol {t}}\cdot\dot {{\boldsymbol {u}}} \,da + \int_{V} {\boldsymbol {b}} \cdot\dot{{\boldsymbol {u}}}\, dv - \frac{d }{d t} \int_{V} \psi\, dv - \frac{d }{d t} \int _{V} \frac{1}{2} \rho_{0} |\dot{{ \boldsymbol {u}}}|^{2} \,dv. $$
(22)

Using the governing equations (7), the dissipation can be expressed as

$$\begin{aligned} \mathsf{D} &= \int_{V} ( {\boldsymbol {T}}- \partial_{\boldsymbol {\varepsilon}^{e}} \psi ) : \mathit{grad}{\boldsymbol {v}}\,dv \\ &\quad{} + \int_{V} {\boldsymbol {T}}: \dot{\boldsymbol{ \varepsilon}}^{p} \, dv + \int_{V} \bigl\{ [ - \partial_{{\boldsymbol {c}}} \psi+ \mathit{curl}\, \partial_{{\boldsymbol {t}}} \psi ] \times{\boldsymbol {t}} \bigr\} \cdot{\boldsymbol {V}} \, dv \\ &\quad{} + \int_{\partial V} {\boldsymbol {V}}\cdot \bigl[ ( \partial _{{\boldsymbol {t}}} \psi \times{\boldsymbol {n}} ) \times{\boldsymbol {t}} \bigr] \, da. \end{aligned}$$
(23)

On demanding classical hyperelasticity be recovered in the absence of plasticity and crack evolution, we obtain the stress relation

$${\boldsymbol {T}}= \partial_{\boldsymbol{\varepsilon}^{e}} \psi $$

and note the driving forces in the bulk for the mechanisms of plasticity (in the absence of any further kinematic assumption on the nature of plastic straining like, e.g., volume-preservation) and the crack-tip advance as

$$ \begin{aligned} \dot{\boldsymbol{\varepsilon}}^{p} & \leadsto{\boldsymbol {T}} \\ {\boldsymbol {V}}& \leadsto [ - \partial_{{\boldsymbol {c}}} \psi+ \mathit{curl}\, \partial_{{\boldsymbol {t}}} \psi ] \times{\boldsymbol {t}}. \end{aligned} $$

A driving force on a crack-tip at the boundary also emerges as

$${\boldsymbol {V}}\leadsto ( \partial_{{\boldsymbol {t}}} \psi\times {\boldsymbol {n}} ) \times {\boldsymbol {t}}. $$

Ignoring plasticity and the boundary velocities for the moment, the closed, governing equations of the model become

$$ \begin{aligned} \psi& = \hat{\psi}(\boldsymbol{ \varepsilon}, {\boldsymbol {c}}, \mathit{curl}{\boldsymbol {c}}) \\ \rho_{0} \ddot{{\boldsymbol {u}}} & = \mathit{div}\, \partial_{\boldsymbol {\varepsilon}} \hat{\psi} + {\boldsymbol {b}} \\ \dot{{\boldsymbol {c}}} & = \mathit{curl}\,{\boldsymbol {c}}\times{\boldsymbol {M}} \bigl[ \bigl\{ - \partial_{{\boldsymbol {c}}} \hat{\psi} - \mathit{curl} (\partial_{\mathit{curl} {\boldsymbol {c}}} \hat{ \psi } ) \bigr\} \times \mathit{curl} {\boldsymbol {c}} \bigr], \end{aligned} $$
(24)

where \({\boldsymbol {M}}\) is a symmetric, positive definite tensor of crack mobility that could take the isotropic form \({\boldsymbol {M}}= \frac {1}{B} {\boldsymbol {I}} \), where \(B > 0 \) is a scalar drag coefficient. A typical candidate for the energy density would be

$$ \hat{\psi} = \frac{1}{2} \boldsymbol{\varepsilon}:{ \boldsymbol {C}}(|{\boldsymbol {c}}|) \boldsymbol{\varepsilon}+ \eta(|{\boldsymbol {c}}|) + \epsilon|\mathit{curl}{\boldsymbol {c}}|^{2} $$
(25)

where \({\boldsymbol {C}}\) is the (possibly anisotropic) tensor of elastic moduli degraded to represent damage by its dependence on \({\boldsymbol {c}}\), \(\eta\) is a non-convex function representing an energy barrier to damage from an undamaged state, and \(\epsilon\) is a small parameter regularizing the crack-tip (but not the crack layer).

5 Concluding Remarks

  • A partial-differential-equation based model of fracture has been developed that allows naturally for crack evolution through the motion of non-singular, localized crack-tips in a brittle or ductile solid. Cracks and their tips are identified as smooth localizations of fields, related through kinematics. The crack-field is linked to the idea of regularized mass-density gradients. This identification with a physically observable field has the potential of identifying crack-tip mobility functions linking driving force to crack velocity from experiment. A primary contribution of this work is the essentially kinematic rationalization of a kinetic equation for crack-tip motion in the form of a pde. This pde form of the theory allows for seamless multiphysics coupling with similar defect-mediated, dynamical models of plasticity and phase transformations, allowing the study of complex defect interactions, e.g., automatic arrest of dislocations inside a body on crossing a crack-flank due to depletion of stress, without tracking free-boundaries.

  • Any reasonable energy/stress constitutive assumption in the theory will involve degradation of elastic modulus to negligible values where the crack field magnitude attains its damaged value and beyond, as dictated by the function \(\eta\) in (25). Thus, any compact region, say an elliptical cavity in the shape of a penny shaped-crack, where this occurs serves as an Eshelby inhomogeneity [13], and therefore our model will predict commonly accepted (non-singular) crack stress fields.

  • The model kinematically restricts fracture evolution as arising solely from the motion of crack-tips. The model delivers an explicit driving-force for crack-tip motion. Furthermore, the equation of evolution of the crack field is such that the crack evolves instantaneously only in regions where a crack-tip is present, and does not at other points regardless of the level of energy, stress, or damage at such points. Likewise, the dissipation is manifestly localized at crack-tips, regardless of how close to thermodynamic equilibrium the state of the body is in, e.g., in the presence of significant material inertia. This is in contrast to models where the driving force is simply the variational derivative of the energy density with respect to the order parameter/phase field (see [46], which may also be considered as a preliminary proof-of-concept that the type of model presented here has the potential of delivering on its objectives). This feature has similarities with the viewpoint of classical fracture mechanics viewed as a standard dissipative process [37], but without requiring the postulate that the driving force for crack extension is related to (a priori known) stress intensity factors (requiring a singular crack tip field) conjugate to crack extension, known to be valid for linear elastic fracture mechanics. This feature (i.e., the crack evolves only at locations \({\boldsymbol {x}}\) where \({\boldsymbol {t}}({\boldsymbol {x}}) \neq\bf0\)) has implications for the need (or not) for surface-energy regularization in a damaged zone behind a crack tip. The conjecture is that, in the canonical case of such a region being produced by the transport of a ‘diffuse’ crack-tip along a layer, the \(\mathit{curl} {\boldsymbol {c}}\) field would vanish in the layer behind the tip and therefore, regardless of the softening stress-strain response there due to degradation of elastic modulus, no further evolution of \({\boldsymbol {c}}\) would take place (with the attendant narrowing and localization of the layer-width).

  • The kinematic representation of a crack and its tip is such that an existing crack has a driving force on it for all of Modes I, II, and III loadings or any combination thereof. This is in contrast to some models of fracture based on eigendeformation concepts representing total displacement discontinuities (including modes other than the Mode I opening), as in such situations particular loading modes can extend only particular types of cracks, the latter identified by the eigendeformation, i.e., as in dislocation theory, a shear stress in the 1-direction on a plane with normal in the 2-direction can only drive an existing displacement discontinuity if it has a component in the 1-direction.

  • As shown by Weertman [43], the problem statement of a dislocation mechanics based approach to fracture (that does not degrade elastic modulus) requires the dislocation density field as well as the distortion field to be considered as unknowns, this arising from the enforcement of traction-free conditions on the crack flanks. This is in contrast to the dislocation problem where the dislocation density is specified and one only needs to solve for the distortion field, since no extra conditions are required to be satisfied in the wake of the dislocation line. These observations make the adoption of general pde methods for solving 3-d problems for dislocation statics non-transferable to the fracture context without significant conceptual and practical changes. In the present approach, the field \({\boldsymbol {c}}\) is intended to degrade the elastic modulus and this along with the equation of balance of linear momentum ensures the satisfaction of the correct traction conditions on the flanks of the cracked region.

  • The theory is time-dependent and does not rely on assumptions of rate-independence, especially in dynamic settings considering material inertia where rate-independence becomes a dubious notion (implying time-scales of crack motion are much faster than scales associated with elastic wave propagation).

  • The model does not involve tracking of line-like objects and neither of surfaces.

  • As stated, the present model will not predict crack nucleation (for most reasonable constitutive assumptions for \({\boldsymbol {V}}\) which would necessarily have the property that \({\boldsymbol {V}}= \mathbf{0}\) if \({\boldsymbol {t}}= \mathbf{0} \)). However, the geometrically exact form of the evolution equation for the crack-tip field \({\boldsymbol {t}}\) in the Eulerian setting is (cf. [1])Footnote 3

    $$\partial_{t} {\boldsymbol {t}}= -\mathit{curl} \bigl({\boldsymbol {t}}\times({ \boldsymbol {v}}+ {\boldsymbol {V}}) \bigr) $$

    and on linearization about a crack-free state (\({\boldsymbol {c}}=\mathbf{0}\)) it can be shown, following the arguments of [17], that the coupling between \({\boldsymbol {t}}\) and \({\boldsymbol {v}}\) allows for nucleation in such models. The upshot is that even in the small deformation setting, changing (7)3 to \(\dot{{\boldsymbol {c}}} = \mathit{curl} {\boldsymbol {c}}\times ( {\boldsymbol {V}}+ {\boldsymbol {v}} )\) would allow for nucleation. The additional term involving the material velocity, \(\mathit{curl} {\boldsymbol {c}}\times {\boldsymbol {v}}\), may be expected to have beneficial effects in predictions of crack-branching as well. It can be shown that restricting attention to problems with field variations in the plane and for isotropic mobility \({\boldsymbol {M}}\) in (24), if the energy \(\hat{\psi}\) were to depend on \({\boldsymbol {c}}\) only through \(|{\boldsymbol {c}}|\) then branching (i.e., \({\boldsymbol {V}}\) not orthogonal to \({\boldsymbol {c}}\)) can be driven only by the term \(\mathit{curl} (\partial_{\mathit{curl} {\boldsymbol {c}}} \hat{\psi} ) \times \mathit{curl} {\boldsymbol {c}}\) that is, roughly speaking, to be considered not a dominant term in driving the crack motion but simply one that regularizes the tip. An anisotropic energetic dependence on \({\boldsymbol {c}}\) (e.g., through a quadratic form in \({\boldsymbol {c}} \)) and anisotropic mobilities would produce branching effects, but instabilities arising from the dependence on the material velocity can induce branching even in materially isotropic material, especially in situations involving rapid crack-motions accounting for material inertia. Such explorations will be subjects of future work.

  • As alluded to in Sect. 3.1, the structure of the model suggests energetic penalization of values of \(|\,\mathit{grad} \mathit{grad} \rho\, {\boldsymbol {c}} - |{\boldsymbol {c}}| {\boldsymbol {c}}\,|\) away from 0. Then the formalism would change the thermodynamic stress relation and the driving force for crack-tip velocity to naturally include interesting couplings to higher gradients in the mass density, reminiscent of Korteweg theory. This has the potential of producing very interesting patterned equilibria and dynamics of (7), including the mass density \(\rho\) field.

  • The literature listed in Sect. 1 is well-developed and has resulted in several models well-adapted to practical numerical computation. The model presented herein provides complementary benefits to this body of work, but clearly much work remains to be done to implement the model and assess its practical capability. Also important will be the exploration of connections of the model with micromechanics of damage by void nucleation and growth [3, 9, 25, 32, 38].