Fully nonlinear heavy ion-acoustic solitary waves in astrophysical degenerate relativistic quantum plasmas

Abstract

Fully nonlinear features of heavy ion-acoustic solitary waves (HIASWs) have been investigated in an astrophysical degenerate relativistic quantum plasma (ADRQP) containing relativistically degenerate electrons and non-relativistically degenerate light ion species, and non-degenerate heavy ion species. The pseudo-energy balance equation is derived from the fluid dynamical equations by adopting the well-known Sagdeev-potential approach, and the properties of arbitrary amplitude HIASWs are examined. The small amplitude limit for the propagation of HIASWs is also recovered. The basic features (width, amplitude, polarity, critical Mach number, speed, etc.) of HIASWs are found to be significantly modified by the relativistic effect of the electron species, and also by the variation of the number density of electron, light ion, and heavy ion species. The basic properties of HIASWs, that may propagated in some realistic astrophysical plasma systems (e.g., in white dwarfs), are briefly discussed.

1 Introduction

The propagation characteristics of nonlinear excitations (in the form of solitary waves) in an extremely dense plasmas (Chandrasekhar 1931, 1935, 1939, 1964; Chandrasekhar and Tooper 1964) has received a renewed interest to the plasma physics researchers because of the availability of such plasma not only in astrophysical environments (Shapiro and Teukolsky 1983; Koester and Chanmugam 1990; Chabrier et al. 2006; Potekhin et al. 1999), but also in experimental plasma environments (Ichimaru 1982; Fortov 2009; Drake 2009, 2010; Marklund and Shukla 2006). In extremely (ultra-) dense plasmas, the mean inter-particle distance is smaller than or is of the same order as the de Broglie wavelength, and the quantum effect becomes an important parameter to be considered. On the other hand, the Fermi velocity of the lighter particles (e.g., electrons, positrons, etc.) at extreme density (Shukla and Mamun 2010) is comparable to the speed of light in vacuum. Therefore, plasmas at extreme conditions (ultra-dense plasmas) (Chandrasekhar 1931, 1935, 1939, 1964; Chandrasekhar and Tooper 1964) that fulfil the above mentioned criteria may termed as degenerate relativistic quantum plasma (DRQP). The degenerate particles (e.g., electrons, positrons, and/or holes in the context of semiconductors) may be present in astrophysical compact stars (white dwarfs, neutron stars, etc.) (Shapiro and Teukolsky 1983; Koester and Chanmugam 1990) and also in dense matter (Ichimaru 1982; Fortov 2009). The pressure in such plasmas arises due to the continuous motion of degenerate particles around their positions, and is termed as degenerate or degeneracy pressure (Shukla and Eliasson 2011). The degenerate pressure sometimes becomes comparable to or even larger than the thermal gas pressure at ultra-high densities. It is worth to mention that the basic features of solitary waves in DRQP medium are quite different than those in classical thermal plasma medium. The nonlinear propagation of solitary waves in an astrophysical degenerate relativistic quantum plasma (ADRQP) medium seems to be an interesting topic of research to the plasma physics researchers. Based on the increasing interest of nonlinear dynamics of solitary waves in an ADRQP, it is now important to develop the theory of solitary waves that may propagate in such systems. Most of the solitary waves are modeled via the Korteweg de Vries (KdV) equation (Washimi and Taniuti 1966; Hirota 1971), Kadomtsev Petviasvili (KP) equation (Alexander et al. 1997), Zakharov Kadomtsev (ZK) equation (Wazwaz 2005), pseudo-energy balance equation (Sagdeev 1966), etc., where in all cases, the mutual balance between the nonlinear term and the dispersion term form the solitary structure.

There has been a great deal of interests in studying the linear and nonlinear wave properties in the ionic time and length scale in a multi species ADRQP systems (Duvinov and Dubinova 2007; Masood and Eliasson 2011; Roy et al. 2012; Rahman et al. 2013; Hossem et al. 2014; Kerr et al. 2016; Islam et al. 2017), as well as in the next generation of compressed plasma which may create due to the interactions of intense laser beams with the dense solid materials. It should be essential to mention that in many ADRQP systems (e.g., in white dwarfs, neutron stars, etc.), the number density of degenerate electrons or/and positrons are high enough that the plasma can be considered as degenerate fluids, and the fluid dynamical approach can be used to study the characteristics of solitary waves in such plasmas. A vast number of works has already been conducted theoretically to study the properties of solitary waves in ADRQP medium via the fluid dynamical approach (Masood and Eliasson 2011; Roy et al. 2012; Rahman et al. 2013; Hossem et al. 2014; Kerr et al. 2016; Islam et al. 2017). It is reported that most of the theoretical works on the dynamics of solitary waves in ADRQP systems have been considered for the ion time and length scale (Lee 2008; Rahman et al. 2015; El-Labany et al. 2016; Atteya et al. 2017). A very few has considered the propagation of nonlinear waves (e.g., solitary waves, shock waves, etc.) in the presence of heavy ions or/and nucleus of heavy elements (Atteya et al. 2017; Islam et al. 2017; Mamun et al. 2016, 2017; Sultana et al. 2018). Interestingly, it has been reported (Horn 1991; Witze 2014; Vanderburg et al. 2015) that heavy nuclei/ions, e.g., ${}^{56}_{26}\mbox{Fe}$ or/and ${}^{85}_{37}\mbox{Rb}$ or/and ${}^{96}_{42}\mbox{Mo}$, etc. are present in many astrophysical degenerate relativistic quantum plasma (ADRQP) systems such as white dwarfs and neutron stars. It has also been predicted (Chandrasekhar and Tooper 1964; Shapiro and Teukolsky 1983; Koester and Chanmugam 1990) that the core of white dwarfs may contain degenerate electrons and also degenerate light nuclei/ions, e.g., ${}^{1}_{1}\mbox{H}$ or/and ${}^{4}_{2}\mbox{He}$ or/and ${}^{12}_{6}\mbox{C}$ or/and ${}^{16}_{8}\mbox{O}$, etc. Therefore, the main aim of the present attempt is to develop a generalized model to investigate the properties of arbitrary amplitude heavy ion-acoustic solitary waves in an ADRQP system composed of three distinct particle populations, namely inertial non-degenerate heavy ion/nucleus species (e.g., ${}^{56}_{26}\mbox{Fe}$ or ${}^{85}_{37}\mbox{Rb}$ or ${}^{96}_{42}\mbox{Mo}$, etc.), inertialess relativistically degenerate electron and non-relativistically degenerate light ion/nucleus species (e.g., ${}^{1}_{1}\mbox{H}$ or ${}^{4}_{2}\mbox{He}$ or ${}^{12}_{6}\mbox{C}$ or ${}^{16}_{8}\mbox{O}$, etc.).

The manuscript is organized in the following manner: The basic plasma fluid formalism of our considered plasma system is presented in Sect. 2. The linear dispersion relation is derived, and the linear properties of heavy ion-acoustic waves (HIAWs) are analyzed in Sect. 3. The fully nonlinear HIASWs are modelled via the Sagdeev type pseudo-potential approach, and the characteristics of HIASWs are investigated for different plasma configuration parameters in Sect. 4. The small amplitude approximation for HIASWs is also discussed in Sect. 4. Finally, a brief summary of results is given in Sect. 5.

2 Plasma fluid formalism

We consider a three-component ADRQP system containing non-degenerate cold mobile heavy ion fluid (of charge $z_{h}e$ and mass $m_{h}$), relativistically degenerate electrons (of charge $-e$ and mass $m_{e}$) and non-relativistically degenerate light ion species (of charge $z_{i}e$ and mass $m_{i}$), where $z_{h}\ (z_{i})$ is the charge state of the heavy (light) ion species and $e$ is the magnitude of an electron charge. Thus, the charge neutrality condition at equilibrium reads $z_{i}n_{i0}+z_{h}n_{h0}=n_{e0}$, where $n_{s0}$ is the unperturbed number density of the plasma species $s$ and the indices $s=e, i, h$ refer to the electron, light ion, heavy ion, respectively. The heavy ion-acoustic wave, in which the inertia (the restoring force) is provided by the mass density of the heavy ion species (the degenerate pressures of the electron and light ion species), is described by the following one dimensional normalized equations

$$\begin{aligned}& \frac{\partial n_{h}}{\partial t}+\frac{\partial (n_{h} u_{h})}{ \partial x} = 0, \end{aligned}$$

(1)

$$\begin{aligned}& \frac{\partial u_{h}}{\partial t}+ u_{h} \frac{\partial u_{h}}{ \partial x}= - \frac{\partial \phi }{\partial x} , \end{aligned}$$

(2)

$$\begin{aligned}& K_{1} \frac{\partial n_{i}^{\gamma _{i}}}{\partial x}+ n_{i} \frac{ \partial \phi }{\partial x}= 0 , \end{aligned}$$

(3)

$$\begin{aligned}& K_{2} \frac{\partial n_{e}^{\gamma _{e}}}{\partial x}- n_{e} \frac{ \partial \phi }{\partial x}= 0 , \end{aligned}$$

(4)

$$\begin{aligned}& \frac{\partial ^{2}\phi }{\partial x^{2}} = (1+\alpha ) n_{e} - \alpha n_{i} - n_{h} , \end{aligned}$$

(5)

where the number density $n_{s}$ is normalized by its equilibrium value $n_{s0}$, heavy ion fluid velocity $u_{h}$ by the heavy ion sound speed $c_{0}=(z_{h} m_{e} c^{2}/m_{h})^{1/2}$, the electrostatic potential $\phi$ by $m_{e} c^{2}/e$. The space $x$ and the time $t$ are normalized by the Debye length $\lambda _{0}=(m_{e} c^{2}/4\pi e^{2}z _{h} n_{h0})^{1/2}$ and the plasma period $\omega _{ph}^{-1}=(4\pi z _{h}^{2}e^{2}n_{h0}/m_{h})^{-1/2}$ of the heavy ion species, respectively. Other parameters are defined as $\alpha =z_{i} n_{i0}/z _{h} n_{h0}$, $K_{1}=K_{i} n_{i0}^{\gamma _{i}-1}/z_{i} m_{e} c^{2}$, and $K_{2}=K_{e} n_{e0}^{\gamma _{e}-1}/m_{e} c^{2}$. Expressions of $K_{1}$ and $K_{2}$ have been obtained from the arbitrary degeneracy pressure $P_{j}=K_{j} n_{j}^{\gamma }$ (Chandrasekhar 1931, 1935, 1939, 1964; Chandrasekhar and Tooper 1964) of the plasma species $j$, here $j=e$ for the electron and $i$ for light ion in our case. The $P_{j}$ has been explicitly explained by Chandrasekhar for two limits, namely the non-relativistic and the ultra-relativistic limits; and the expression of the parameter $K_{j}$ for ultra-relativistic limit, i.e., for $\gamma =\frac{4}{3}$ leads to the form $K_{j}=\frac{3}{4} \hbar c$ (Shukla and Eliasson 2011) and for non-relativistic limit, i.e., for $\gamma =\frac{5}{3}$ leads to the form $K_{j}=\frac{3}{5} \frac{ \pi \hbar ^{2}}{m_{j}}$ (Shukla and Eliasson 2011), where $\hbar$ is the reduced Planck constant, $m_{j}$ is the rest mass of the plasma species $j$, and $c$ is the speed of light in vacuum.

We note that the normalized parameter $\alpha$ can be either $>1$ or $<1$ since $z_{i}/z_{h}<1$ and $n_{i0}/n_{h0}\geq 1$ for most of the ADRQP systems like white dwarfs and neutron stars, where the plasma quasi-neutrality condition $z_{h} n_{h0}+z_{i} n_{i0}-n_{e0}=0$ is always satisfied. For example, one may consider $n_{e0}=38\times 10^{30}~\mbox{cm}^{-3}$, $n_{i0}=10^{30}~\mbox{cm}^{-3}$, $n_{h0}=10^{30}~\mbox{cm}^{-3}$ for electron-hydrogen ion-rubidium ion plasma, where $z_{i}=1$ and $z_{h}=37$ or $n_{e0}=2.42\times 10^{32}~\mbox{cm}^{-3}$, $n_{i0}=10^{32}~\mbox{cm}^{-3}$, $n_{h0}=10^{30}~\mbox{cm}^{-3}$ for electron-helium ion-molybdenum ion plasma, where $z_{i}=2$ and $z_{h}=42$, etc. It is also noted that the existence of different heavier ions/nuclei (e.g., ${}^{56}_{26}\mbox{Fe}$ or ${}^{85}_{37}\mbox{Rb}$ or ${}^{96}_{42}\mbox{Mo}$, etc.) possess different masses, and one can describe the acoustic wave for the individual mobile ion/nucleus species (e.g., molybdenum-acoustic wave for the mobile molybdenum ion species, rubidium-acoustic wave for mobile rubidium ion species, and so on). However, the plasma model (given in Eqs. (1)–(5)) under consideration is valid for any pair of light and heavy ion species (viz. hydrogen ion ($z_{i}=1$) as light ion and iron ion ($z_{h}=26$) as heavy ion or carbon ($z_{i}=6$) as light ion and molybdenum ($z _{h}=42$) as heavy ion, etc. Horn 1991; Witze 2014; Vanderburg et al. 2015), which is a general degenerate plasma model applicable to many astrophysical plasma systems like white dwarfs and neutron stars. It is then appropriate to use a generalize name like “heavy ion-acoustic wave” to model the nonlinear wave associated with the inertial heavy ion species.

We now integrate Eqs. (3) and (4) over $x$, and obtain the number densities (normalized) of the inertialess degenerate light ion $n_{i}$ and electron $n_{e}$, in terms of the electric potential $\phi$, as

$$\begin{aligned}& n_{i}= \biggl(1-\frac{\gamma _{i}-1}{\gamma _{i} K_{1}} \phi \biggr)^{1/(\gamma _{i}-1)} , \end{aligned}$$

(6)

$$\begin{aligned}& n_{e}= \biggl(1+\frac{\gamma _{e}-1}{\gamma _{e} K_{2}} \phi \biggr)^{1/(\gamma _{e}-1)} , \end{aligned}$$

(7)

where $\gamma _{i} \ (\gamma _{e})$ represents the relativistic index of the light ion (electron) species, and $\gamma _{i}=5/3$ will be considered for the non-relativistically degenerate light ion species in later sections for our analysis purposes. Now, substituting Eqs. (6) and (7) in Eq. (5), we obtain

$$\begin{aligned} \frac{\partial ^{2}\phi }{\partial x^{2}} =& (1+\alpha ) \biggl(1+\frac{ \gamma _{e}-1}{\gamma _{e} K_{2}} \phi \biggr)^{1/(\gamma _{e}-1)} \\ &{}- \alpha \biggl(1-\frac{\gamma _{i}-1}{\gamma _{i} K_{1}} \phi \biggr)^{1/(\gamma _{i}-1)} - n_{h} . \end{aligned}$$

(8)

The plasma fluid model given in Eqs. (1)–(5) is now reduced to a coupled equations given by the heavy ion continuity equation (1), motion equation for the heavy ion plasma fluid (2), and the Poisson’s equation (8). That is, equations (1), (2), and (8) represent a basis set of equations, which describes the dynamics of HIASWs associated with the HIAWs in an ADRQP system.

3 Linear waves

The linear properties of heavy ion-acoustic waves can be analyzed via the linear dispersion relation (DR). We use the normal mode analysis to derive the DR of HIAWs in an ADRQP medium. We linearize Eqs. (1), (2), and (8), and obtain a DR in the form

$$ \omega ^{2}=\frac{k^{2}}{k^{2}+\mu } , $$

(9)

where $\mu =\frac{1+\alpha }{\gamma _{e} K_{2}}+\frac{\alpha }{\gamma _{i} K_{1}}$. It is now clear in the dispersion relation (9) that the linear characteristics of the HIAWs in an ADRQP with relativistically degenerate electron and non-relativistically degenerate light ion species appear to modify for the relevant parameters of the considered plasma medium, e.g., the relativistic effect via $\gamma _{e}$, the total charge density of the light-to-heavy ion species $\alpha$, electrons number density via $K_{2}$, light ion number density via $K_{1}$, etc. To analyze the influence of different plasma configuration parameters on the linear properties of HIAWs, we first demonstrate the variation of the wave frequency $\omega$ (given in Eq. (9)) against the wavenumber $k$ in an ADRQP system containing ultra-relativistically degenerate electron and non-relativistically degenerate light ion species for different values of $\alpha$, as shown in Fig. 1. The wave frequency (or phase speed of HIAWs) is observed to decrease for the decreasing (increasing) values of the heavy (light) ion charge density $z_{h}n_{h0}$ ($z_{i}n_{i0}$). That is, the increasing (decreasing) the number density or the number of protons in the inertial heavy ion (degenerate light ion) leads to a faster (slower) HIAWs (as depicted in Fig. 1), which agrees with the earlier work (Islam et al. 2017) on shock dynamics in a degenerate relativistic plasma containing electrons, light ions and heavy ions.

Fig. 1

Effect of $\alpha$ on the wave frequency $\omega$ in an ADRQP containing ultra-relativistically degenerate electron and non-relativistically degenerate light ion species

In Fig. 2, we depict the variation of the wave frequency $\omega$ against the wavenumber $k$ for different degenerate electron number density $n_{e0}$ via $K_{2}$. We see in Fig. 2 that the wave frequency $\omega$ (i.e., the phase speed) of the HIAWs increases with the degenerate electrons number density $n_{e0}$. That is, the wave frequency (in other words, the phase speed) of the HIAWs is seen to higher in an ADRQP containing ultra-relativistically degenerate electron and non-relativistically degenerate light ion species (i.e., $\gamma _{i}=5/3$ and $\gamma _{e}=4/3$) with more population of degenerate electron than in an ADRQP with less population of degenerate electron, as shown in Fig. 2.

Fig. 2

Effect of the degenerate electron number density $n_{e0}$ on the wave frequency $\omega$ in an ADRQP containing ultra-relativistically degenerate electron and non-relativistically degenerate light ion species

It is now appropriate to consider the long wavelength limit (i.e., $k^{2}\ll \mu$). In the long wavelength approximation, the wave frequency in Eq. (9) reduces to $\omega =k/\mu ^{1/2}$, which then lead to the effective phase speed of the HIAWs in an ADRQP medium in the form $V_{ph}^{eff}=\mu ^{-1/2}$. We now recover the dimension of the phase velocity for the sake of physical transparency of our plasma model. The phase velocity $V_{ph}$ in dimensional form reads $v_{ph}=\mu ^{-1/2} (z_{h} m_{e}c^{2}/m_{h})^{1/2}$, which, in fact, suggests the heavy ion-acoustic wave propagation $\mu ^{-1/2}$ times than that of the heavy ion sound speed in a three component astrophysical degenerate relativistic quantum plasma.

4 Fully nonlinear HIASWs

We adopt pseudo-potential approach to study the fully nonlinear HIASWs in an ADRQP medium, and assume that all independent variables in the evolution equations (1), (2), and (8) depend on a single travelling variable $\xi =x-Mt$ (where $M$ is Mach number, normalized by the heavy ion sound speed $c_{0}$). We now follow the mathematical procedure given in Sultana et al. (2010, 2012) to derive the pseudo-energy balance equation. By applying the steady state condition, and imposing the appropriate boundary conditions (namely, $n_{h}\rightarrow 1, u_{h}\rightarrow 0,\phi \rightarrow 0$, and $d\phi /d\xi \rightarrow 0$ at $\xi \rightarrow \pm \infty$), our plasma model equations (1), (2), and (8) reduce to the energy integral in the form

$$ \frac{1}{2} \biggl( \frac{d \phi }{d \xi } \biggr) ^{2} + \Psi ( \phi ; M,\alpha ,\gamma _{i},\gamma _{e},K_{1},K_{2})=0 , $$

(10)

where the (Sagdeev-type) pseudo-potential (Sagdeev 1966) in an ADRQP system is given by

$$\begin{aligned}& \Psi (\phi ; M,\alpha ,\gamma _{i},\gamma _{e},K_{1},K_{2}) \\& \quad = M^{2} \biggl[1- \biggl(1-\frac{2\phi }{M^{2}} \biggr)^{1/2} \biggr] \\& \quad \quad {}+\alpha K_{1} \biggl[1- \biggl(1-\frac{\gamma _{i}-1}{\gamma _{i} K _{1}} \phi \biggr)^{\gamma _{i}/(\gamma _{i}-1)} \biggr] \\& \quad \quad {}+(1+\alpha )K_{2} \biggl[1- \biggl(1+\frac{\gamma _{e}-1}{\gamma _{e} K_{2}} \phi \biggr)^{\gamma _{e}/(\gamma _{e}-1)} \biggr] . \end{aligned}$$

(11)

It is clear in Eq. (11) that $\Psi (\phi )= d\Psi (\phi )/d \phi =0$ at $\phi =0$, represents the equilibrium state and also the local maximum of the pseudo-potential $\Psi (\phi )$. That is, the solitary wave solution of (10) may exist if (i) the convexity requirement of the second derivative is negative at the origin, i.e., $d^{2}\Psi (\phi )/d \phi ^{2}|_{\phi =0}\leq 0$, which in fact, defines a velocity values above (below) which solitary excitations exist (do not exist), and (ii) $\Psi (\phi )$ must be negative in the region $0<\phi <\phi _{m} (\phi _{m}<\phi <0)$ for the positive (negative) potential solitary structures to exist, where $\phi _{m}$ is the amplitude of the solitary structures. Therefore, by expanding the Sagdeev potential $\Psi (\phi )$ near equilibrium (i.e., at $\phi =0$), one can find the critical Mach number $M_{1}$ (at which the second derivative changes the sign) in the form

$$ M_{1} = \sqrt{\frac{\gamma _{i} \gamma _{e} K_{1} K_{2}}{\gamma _{i} K _{1}+\alpha \gamma _{i} K_{1}+\alpha \gamma _{e} K_{2}} } =\mu ^{-1/2} . $$

(12)

It is expected and also clear in Eq. (12) that the propagation of solitary waves associated with HIAWs do not exist below the effective phase speed $V_{ph}^{(eff)}$ (=$\mu ^{-1/2}$), as solitary waves are super-acoustic by nature, and exist above the actual sound speed, which, in fact, the heavy ion sound speed in an ADRQP containing inertialess relativistically degenerate electron and non-relativistically degenerate light ion species, and inertial non-degenerate heavy ion species. We can, therefore, say that the HIASWs may exist only for the Mach number $M>M_{1}$ for any fixed set of relevant plasma parameters (e.g., $\alpha , \gamma _{e}, \gamma _{i}, z_{h}, z_{i}, n_{h0}, n_{e0}$, etc.), i.e., solitary waves must be travelling at speed exceeding the actual sound speed of the considered plasma.

The upper limit of the velocity $M_{2}$ for the existence of HIASWs, above which the nonlinear propagation of HIASWs is forbidden, can be found by using the density compression limit. That is, we assume a condition $\Psi (\phi _{c})\geq 0$ with $\phi _{c}=M^{2}/2$ being the minimum value of $\phi$ beyond which the inertial heavy ion number density is undefined. This can be fulfilled only if

$$\begin{aligned}& M_{2}^{2}+\alpha K_{1} \biggl[1- \biggl(1- \frac{\gamma _{i}-1}{\gamma _{i} K_{1}} \frac{M_{2}^{2}}{2} \biggr)^{\gamma _{i}/(\gamma _{i}-1)} \biggr] \\& \quad {}+(1+\alpha )K_{2} \biggl[1- \biggl(1+\frac{\gamma _{e}-1}{\gamma _{e} K_{2}} \frac{M_{2}^{2}}{2} \biggr)^{\gamma _{e}/(\gamma _{e}-1)} \biggr] = 0, \end{aligned}$$

(13)

where the upper limit of the velocity $M_{2}$ for the existence of the HIASWs can be found by solving Eq. (13) numerically, and it is seen that the upper limit $M_{2}$ is also a function of the total charge density of light-to-heavy ion species $\alpha$, relativistic effects (via $\gamma _{e}$), number density of degenerate electron $n_{e0}$ and light ion $n_{i0}$, etc. That is, the lower threshold $M_{1}$ as well as the upper limit $M_{2}$ of the velocity (also the accessible velocity domain, let us define $[M_{1}, M_{2}]$) for the propagation of the HIASWs are predicted to modify due to the variation of relevant parameters (e.g., $\alpha , \gamma _{e}, \gamma _{i}, n_{h0}, n_{e0}$, etc.).

We now depict the variation of the Mach number $M$ (i.e., $M_{1}$ and $M_{2}$) against the total charge density ratio of light to that of heavy ion species $\alpha$ for three different plasma compositions in Fig. 3 to trace the influence of degenerate electrons population on the lower and the upper velocity, and also on the accessible velocity domain $[M_{1}, M_{2}]$ for the propagation of HIASWs in an ADRQP medium. It is seen that the lower as well as the upper velocity are seen to lower in a plasma containing less population of degenerate electrons than in a plasma containing more population of degenerate electrons, as shown in Fig. 3. The existence velocity region $[M_{1}, M_{2}]$ is found to enhance with the increasing values of degenerate electron number density $n_{e0}$, i.e., the existence region for the propagation of HIASWs becomes narrower while HIASWs propagated from a high dense degenerate electron populated plasma to a less dense degenerate electron populated plasma. We have investigated a significant influence of $\alpha$ on $M$ ($M_{1}$ and $M_{2}$) and also on the existence velocity domain $[M_{1}, M_{2}]$, which can also be seen in Fig. 3. The $M_{1}$ and $M_{2}$ are seen to decrease as $\alpha$ increases. The accessible velocity region $[M_{1}, M_{2}]$ is observed to be larger in a plasma containing more number of inertial heavy ion $n_{h0}$ (or heavy ion with more number of protons residing on its surface, i.e., for larger $z_{h}$) than in a plasma containing less number of inertial heavy ion $n_{h0}$ (or heavy ion with less number of protons residing on its surface, i.e., for smaller $z_{h}$), as depicted in Fig. 3.

Fig. 3

Variation of the lower threshold $M_{1}$ (lower three curves) and the upper limit $M_{2}$ (upper three curves) of the velocity for the propagation of HIASWs with $\alpha$ for different electron number density $n_{e0}$ in a plasma containing ultra-relativistically degenerate electron and non-relativistically degenerate light ion species

4.1 Small amplitude approximation

It may be important to recover the small amplitude limit for the propagation of the heavy ion-acoustic solitary waves (given via the pseudo-potential in Eq. (11)). For the small amplitude approximation (i.e., for the limit $\phi \ll 1$), one may expand (11) as a McLaurin series, and obtain an expression for the pseudo-potential at $\phi \approx 0$ in the form

$$ \Psi _{s}(\phi ; M,\alpha ,\gamma _{i},\gamma _{e},K_{1},K_{2}) \approx -\frac{1}{2} S_{1}\phi ^{2}-\frac{1}{6} S_{2}\phi ^{3} , $$

(14)

where

$$\begin{aligned}& S_{1}=- \frac{1}{M^{2}}+\frac{\alpha }{\gamma _{i} K_{1}}+\frac{1+ \alpha }{\gamma _{e} K_{2}} , \\& S_{2}=-\frac{3}{M^{4}}-\frac{\alpha (2-\gamma _{i})}{\gamma _{i}^{2} K _{1}^{2}}+\frac{(1+\alpha )(2-\gamma _{e})}{\gamma _{e}^{2} K_{2}^{2}} . \end{aligned}$$

Now, substituting Eq. (14) in Eq. (10), and then after some algebraic calculation of the resultant equation (under consideration of some appropriate boundary conditions), one may obtain a solution in the form of the solitary wave solution as

$$ \phi =\phi _{m} \mathrm{sech}^{2}(\xi /\delta ) , $$

(15)

where $\phi _{m}=-3S_{1}/S_{2}$ is the amplitude, and $\delta =2/\sqrt{S _{1}}$ is the width of the heavy ion-acoustic solitary potential for the small amplitude approximation in an ADRQP medium. Thus, one may predict from Eq. (15) that the amplitude as well as the width, and hence the properties of the small amplitude HIASWs in ADRAQP medium are significantly influenced by the relevant plasma configuration parameters, e.g., the light and heavy ion species number density, degenerate electron number density, soliton propagation speed, etc. We shall give a brief parametric analyses on the basis of these relevant parameters of the considered plasma medium in later Sect. 4.2 for the arbitrary amplitude HIASWs (given by the pseudo-potential equation (11)) as our main focus here is to characterize the fully nonlinear features of HIASWs in an ADRQP medium.

4.2 Heavy ion-acoustic soliton characteristics

We now study the characteristics of fully nonlinear HIASWs in an ADRQP system via the numerical integration of (11). In the framework of our plasma model, it is seen that the Sagdeev-type pseudo-potential (11) is a function of the electric potential $\phi$, and also the relevant plasma configuration parameters, e.g., the total charge density of the light-to-heavy ion species $\alpha$, electron’s degeneracy effects (via $\gamma _{e}$), electron number density $n_{e0}$, light (heavy) ion number density $n_{i0}$ ($n_{h0}$), etc. It is essential to mention that the solitary pulse in terms of the electric potential $\phi$ can be obtained by the numerical integration of the pseudo-potential (11), and hence the associated electric field $E$ ($=-\nabla \phi$) of the solitary waves. We should also note that the pulse amplitude is predicted from the pseudo-potential root, while the pulse width is predicted from the maximum value of the slope of potential curve $\phi (\xi )$.

Effect of the light and heavy ion number density We have analyzed the influence of the light and the heavy ion number density via $\alpha$ ($=z_{i}n_{i0}/z_{h} n_{h0}$) on the arbitrary amplitude HIASWs characteristics in an ADRQP medium by plotting the pseudo-potential $\Psi (\phi )$, given in Eq. (11), for a fixed set of plasma parameters in Fig. 4. The soliton speed $M$ is considered a fixed value within the accessible velocity domain for three different plasma compositions (for different values of $\alpha$). It is numerically seen that the accessible velocity domain $[M_{1}, M _{2}]$ for the propagation of arbitrary amplitude solitary excitations are $[0.513, 1.041], [0.436, 0.883], [0.385, 0.779]$ for $\alpha =1, 1.5, 2$, respectively; and we have chosen a fixed soliton propagation speed $M=0.7$ to analyze the effect of $\alpha$ on the solitary waves characteristics. We have observed numerically and also seen in Fig. 3 that the existence velocity domain for the propagation of HIASWs becomes wider for the decreasing (increasing) values of $z_{i}$ or $n_{i0}$ ($z_{h}$ or $n_{h0}$). It is found in Figs. 4(a) and 4(b) for a fixed solitons propagation speed $M$ that the plasma with less (more) inertial heavy ion charge density $z_{h} n_{h0}$ (non-relativistically degenerate light ion charge density $z_{i}n_{i0}$) leads to a taller but steeper solitary excitations than a plasma with more (less) inertial heavy ion charge density (non-relativistically degenerate light ion charge density). The amplitude of the solitary excitations is found to be higher in a more (less) populated heavy (light) ion species plasma for the fixed values of $z_{h}$, $z_{i}$, and $M$, and the resulting electric field is found to be weaker in a more heavy ion populated plasma than in a less heavy ion populated plasma. It is now essential to recall here that the lower Mach number threshold $M_{1}$ for the propagation of HIASWs is found to higher for lower $\alpha$, and the true solitary pulse speed $M-M_{1}$ is actually investigated to higher in a less populated heavy ion plasma for fixed $M$. It is well established (Sultana et al. 2010) and also agreed by our numerical results that the taller solitary pulse moves faster than the smaller pulse and vice versa.

Fig. 4

Effect of $\alpha$ (a) on the pseudo-potential $\Psi (\phi )$ in ADRQP containing ultra-relativistically degenerate electron and non-relativistically degenerate light ion species for $M=0.7$, (b) the corresponding electrostatic potential $\phi$, and (c) the associated electric field $E$, obtained numerically

The Mach number threshold $M_{1}$, below which the solitary waves can not be propagated, depends on the various relevant parameters of the plasma under consideration. We can, therefore, say that the solitary pulse propagation speed in a fixed travelling frame with speed $M_{1}$ or the super-acoustic excess speed is $M-M_{1}$ (where $M$ is the pulse propagation speed). It is then appropriate to trace the effect of $\alpha$ on the access super-acoustic speed $M-M_{1}$, and hence the effect of $M-M_{1}$ on the solitons’ characteristics. We note here that the pseudo-potential $\Psi (\phi ; M,\alpha ,\gamma _{i},\gamma _{e},K _{1},K_{2})$, given in Eq. (11), satisfies $\partial \Psi / \partial M<0$, and hence $\partial \phi _{\mathrm{max}}/\partial M>0$ (Verheest 2010; Verheest and Hellberg 2010), which actually predicting that the maximum amplitude $\phi _{\mathrm{max}}$ of a solitary pulse varies linearly with the access super-acoustic speed $M-M_{1}$ or is an increasing function of $M-M_{1}$ (Sultana et al. 2012). In Fig. 5, we depict the variation of $\phi _{\mathrm{max}}$ with $M-M_{1}$ for different values of $\alpha$ in a plasma containing ultra-relativistically degenerate electron and non-relativistically degenerate light ion species (i.e., we consider $\gamma _{i}=5/3$ and $\gamma _{e}=4/3$). The maximum amplitude of the solitary pulse is seen to vary approximately linearly with the access super-acoustic speed of the solitary pulse $M-M_{1}$, which agrees with earlier work for obliquely propagating electron-acoustic solitary waves in non-thermal plasmas (Sultana et al. 2012). The pulse amplitude is investigated to be higher in more populated heavy ion plasma (lower $\alpha$) than in a less populated heavy ion plasma (higher $\alpha$). At a first glance, it seems to be contradictory with Figs. 4(a) and 4(b). It is physically expected and is actually due to the fact that the Mach number threshold $M_{1}$ is higher (in other word, the true acoustic speed $M/M_{1}$ is lower) for lower values of $\alpha$ and for fixed $M$ (discussed above), and therefore results in a smaller and wider solitary excitations; as shown in Fig. 4. On the other hand, we have chosen a range of values of $M$ within the existence velocity domain and explored the variation of $\phi _{\mathrm{max}}$ with $M-M_{1}$ in Fig. 5.

Fig. 5

Variation of the soliton’s amplitude $\phi _{\mathrm{max}}$ (absolute value) with soliton’s propagation speed in the moving frame $M-M_{1}$ for different $\alpha$ in a plasma containing ultra-relativistically degenerate electron and non-relativistically degenerate light ion species

Effect of degenerate electron number density We have analyzed the dependencies of the degenerate electron number density $n_{e0}$ via $K_{2}$ ($=K_{e} n_{e0}^{\gamma _{e}-1}/m_{e} c ^{2}$) on the characteristics of arbitrary amplitude HIASWs (on the pseudo-potential, and hence on the solitary pulses and associated electric fields) in an ADRQP medium in Fig. 6 for a fixed soliton propagation speed $M$ within the accessible velocity range. In Fig. 6, we see that the amplitude (width) of the solitary pulse in a plasma containing ultra-relativistically degenerate electron and non-relativistically degenerate light ion species is seen to smaller (wider) for the increasing values of $n_{e0}$, see Figs. 6(a) and 6(b). That is, an astrophysical degenerate relativistic quantum plasma containing less population of ultra-relativistically degenerate electron leads to a taller and steeper solitary excitation than in an ADRQP of more population of ultra-relativistically degenerate electron for a fixed soliton propagation speed $M$.

Fig. 6

Effect of degenerate electron number density $n_{e0}$ (a) on the pseudo-potential $\Psi (\phi )$ with $\phi$ for $M=1.05$ and $\alpha =1$, (b) the corresponding electrostatic potential perturbations $\phi$, and (c) the associated electric field $E$, obtained numerically

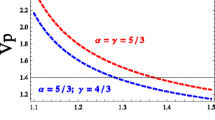
Relativistic effects via $\gamma _{e}$ To trace the influence of relativistic effect via the relativistic index of the electron species $\gamma _{e}$, we have considered a realistic plasma environment (Horn 1991; Witze 2014; Vanderburg et al. 2015) that may exist in many astrophysical objects (e.g., white dwarfs, neutron stars, etc.) containing inertialess relativistically degenerate electron and non-relativistically degenerate hydrogen ion (as light ion) species, and non-degenerate inertial iron (as heavy ion) species. It is numerically seen that the accessible velocity domain $[M_{1}, M_{2}]$ for the propagation of heavy ion-acoustic solitary excitations in a plasma containing ultra-relativistically degenerate electron and non-relativistically degenerate hydrogen ion species is $[0.385, 1.041]$, and in a plasma containing non-relativistically degenerate electron and hydrogen ion is $[0.454, 0.955]$. The accessible velocity domain for the propagation of HIASWs is seen to be wider in a plasma containing ultra-relativistically degenerate electron and non-relativistically degenerate light ion species than in a plasma containing non-relativistically degenerate electron and light ion species for a fixed $\alpha$. Our analytical and numerical results, therefore, suggest a faster heavy ion-acoustic solitary pulse in a plasma containing ultra-relativistically degenerate electron and non-relativistically degenerate light ion species than in a plasma containing non-relativistically degenerate electron and light ion species. The variation of the pseudo-potential $\Psi (\phi )$ with the electric potential $\phi$ (see Fig. 7(a)) and also the electric potential $\phi$ with $\xi$ (see Fig. 7(b)) is depicted for two plasma compositions. A taller and steeper solitary excitation is predicted to propagate in an ADRQP medium containing ultra-relativistically degenerate electron and non-relativistically degenerate light ion species (i.e., for $\gamma _{i}=5/3$ and $\gamma _{e}=4/3$) in comparison to an ADRQP medium containing non-relativistically degenerate electron and light ion species (i.e., for $\gamma _{i}=\gamma _{e}=5/3$) for the fixed soliton propagation speed $M$.

Fig. 7

Relativistic effect: (a) showing the variation of the pseudo-potential $\Psi (\phi )$ with $\phi$ for $M=0.5$ and $\alpha =2$, and (b) the corresponding electrostatic potential perturbations, obtained numerically. Red solid curve is for ultra-relativistically degenerate electron and non-relativistically degenerate light ion species, while the blue dashed curve is for non-relativistically degenerate electron and light ion species

5 Discussion

In this manuscript, we have investigated the characteristics of arbitrary amplitude HIASWs associated with the HIAWs in which inertia is provided by the mass density of heavy ion species, and the restoring force is provided by the degenerate pressures of relativistically degenerate electron and non-relativistically degenerate light ion species. We have derived a linear dispersion relation via the normal mode analysis to characterize the linear wave properties, while a energy balance equation has been derived by adopting a well known Sagdeev type pseudo-potential approach to model the arbitrary amplitude HIASWs in an ADRQP medium. Our results show that not only the linear properties of HIAWs but also the basic properties of arbitrary amplitude HIASWs (e.g., amplitude, width, speed, etc.), which may formed/propagated in an ADRQP medium, are significantly influenced by the relevant parameters of the considered plasma. The wave frequency is observed to increase for the increasing (decreasing) of inertial heavy ion (inertialess degenerate light ion) number density. On the other hand, the wave frequency is seen to increase as the degenerate electron number density increases.

The main emphasis of this manuscript is the study of exact nonlinear theory, and hence the analysis of arbitrary amplitude HIASWs in an ADRQP medium for different intrinsic plasma composition parameters. To characterize the HIASWs, we first determined and analyzed the accessible velocity domain (defined as $[M_{1}, M_{2}]$) for the propagation of HIASWs in an ADRQP system, in the form of a lower Mach number threshold $M_{1}$ and an upper velocity limit $M_{2}$, both of which are seen to depend on the number densities of heavy ion, light ion, and also on the number density of the relativistically degenerate electron species. The existence domain of HIASWs is observed to larger in a more heavy ion as well as in a more degenerate electron populated plasma than in a less populated heavy ion and degenerate electron plasma.

We have investigated that our plasma model can admit only compressive (positive potential) heavy ion-acoustic solitary structures. We have observed that as we increase (decrease) the background degenerate light ion (electron) number density, the amplitude of HIASWs increases for constant values of Mach number $M$. The accessible velocity domain $[M_{1}, M_{2}]$ and also the super-acoustic excess speed $M-M_{1}$ are seen to reduce for the decreasing values of inertial heavy ion number density $n_{h0}$. It is seen that a plasma containing non-relativistically degenerate electron and light ion species leads to the formation/propagation of smaller but wider heavy ion-acoustic solitary excitations than in a plasma containing ultra-relativistically degenerate electron and non-relativistically degenerate light ion species. Excess super-acoustic speed is also seen to be lower in a non-relativistically degenerate electron and light ion species plasma than in a ultra-relativistically degenerate electron and non-relativistically degenerate light ion plasma. To conclude, we may say that the plasma model under consideration in this manuscript would be very important to understand the propagation of localized disturbances in extremely dense astrophysical and experimental plasmas, in which the ratio of charge state (number density) of light to that of heavy ion species $z_{i}/z_{h}<1$ ($n_{i0}/n_{h0}\geq 1$), and the relativistic and quantum effects are important parameters to be considered.