Abstract
Both atomistic and experimental studies reveal the dependence of collagen fibril mechanics on biochemical and biophysical features such as, for instance, cross-link density, water content and protein sequence. In order to move toward a multiscale structural description of biological tissues, a novel analytical model for collagen fibril mechanics is herein presented. The model is based on a multiscale approach that incorporates and couples: thermal fluctuations in collagen molecules; the uncoiling of collagen triple helix; the stretching of molecular backbone; the straightening of the telopeptide in which covalent cross-links form; slip-pulse mechanisms due to the rupture of intermolecular weak bonds; molecular interstrand delamination due to the rupture of intramolecular weak bonds; the rupture of covalent bonds within molecular strands. The effectiveness of the proposed approach is verified by comparison with available atomistic results and experimental data, highlighting the importance of cross-link density in tuning collagen fibril mechanics. The typical three-region shape and hysteresis behavior of fibril constitutive response, as well as the transition from a yielding-like to a brittle-like behavior, are recovered with a special insight on the underlying nanoscale mechanisms. The model is based on parameters with a clear biophysical and biochemical meaning, resulting in a promising tool for analyzing the effect of pathological or pharmacological-induced histochemical alterations on the functional mechanical response of collagenous tissues.
Similar content being viewed by others
Avoid common mistakes on your manuscript.
1 Introduction
Collagen is an ubiquitous protein, representing the main constituent of connective tissues in vertebrates. It can be found in more than 27 forms, but type I collagen is the most abundant in the human body, being the most important for maintaining the structural integrity and a functional mechanical behavior of structural tissues, such as cartilage, bones, tendons, ligaments, and vessel walls, (Fratzl 2008).
Type I collagen molecule is made up by three polypeptide strands which form a triple-helix quaternary structure, stabilized by interstrand hydrogen weak bonds. These molecules exhibit hydroxyproline-deficient sequences characterized by 60 residues (about 20 nm long), referred to as labile domains or molecular kinks (Miles and Bailey 2001; Fratzl 2008). Molecular kinks are activated by thermal fluctuations (Misof et al. 1997) and can be extended by forces at molecular ends that counteract thermal undulations. This mechanism is related to the flexural behavior of macromolecular segments and is known as entropic elasticity (Marko and Siggia 1995; Buehler and Wong 2007; Fratzl 2008). It couples with elastic mechanisms associated with the uncoiling of collagen triple helix and the stretching of molecular backbone, known as energetic elasticity (Buehler 2006; Holzapfel and Ogden 2010; Maceri et al. 2012b; Marino and Vairo 2013).
Collagen molecules assemble themselves in ordered and staggered arrays (Holmes et al. 2001). The latter are, in turn, arranged to form a supertwisted and discontinuous right-handed microfibril which interdigitates with neighboring microfibrils and form thin (20–500 nm) and long (\({>}1\) mm) fibrils at the mesoscale (nano-to-micro). Fibrils collect themselves in form of fibers at the scale of micrometers (Orgel et al. 2006; Fratzl 2008).
Aside from weak interactions among molecules, the fibrillar structure is stabilized by intermolecular covalent cross-links of which two main types have been identified (Eyre and Wu 2005; Avery and Bailey 2008). A first type results from advanced glycation end products (AGEs) whose sources, formation pathways and chemical structure are yet not fully characterized (Reiser et al. 1996; Bailey et al. 1998; Bailey 2001; Depalle et al. 2014). AGEs accumulate with age and diabetes and may impair fibrils normal function, being associated with pathological alterations in tendon stiffness and viscoelasticity (Andreassen et al. 1981; Li et al. 2013), in bone toughness and ductility (Zimmermann et al. 2011), in cartilage stiffness and fragility (Verzijl et al. 2002; DeGroot et al. 2004).
A second covalent cross-link type (namely, the enzymatic cross-link) is essential in the maturation and the physiological mechanical function of collagen fibrils (Brüel et al. 1998; Bailey 2001; Eyre and Wu 2005; Fessel et al. 2012; Svensson et al. 2013; Depalle et al. 2014). It is promoted by the enzyme lysyl oxidase acting on specific lysine amino acids of molecular non-helical ends (both at N- and C-terminal). The resulting allysine reacts with a specific lysine of an adjacent molecule, forming a divalent intermolecular bond (Bailey 2001; Eyre et al. 2008; Wess 2008). Using X-ray diffraction techniques, it has been shown that the C-terminal cross-link forms on a telopeptide that takes a folded hook-shaped configuration, adding nonlinearities to fibril mechanical behavior (Orgel et al. 2000; Wess 2008; Uzel and Buehler 2011; Marino and Vairo 2014a). These divalent bonds are referred to as immature. In fact, over time, these may further react with another telopeptide aldehyde group, forming a trivalent mature bond linking three collagen molecules (Bailey 2001; Avery and Bailey 2008; Eyre et al. 2008). Bailey et al. (1998) proposed that mature cross-links form between molecules on different microfibrils and fibrils (Avery and Bailey 2008).
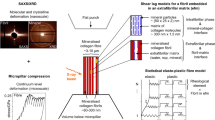
In this study, enzymatic cross-links are focused only and Fig. 1 schematically shows the features of fibril hierarchically organized structure herein addressed. Significant variations in enzymatic mature cross-links concentrations have been reported in different tissues (Eyre et al. 1984; Saito et al. 1997). For instance, Saito et al. (1997) reports that the density of trivalent cross-links for middle-age men is 280 mmol/mol in skin, 390 mmol/mol in bone and 1800 mmol/mol in articular cartilage. On the other hand, the density of immature cross-links appears to be fairly constant across different tissues resulting 1340 mmol/mol in cartilage, 1350 mmol/mol in bone and 1590 mmol/mol in skin for middle-age men (Saito et al. 1997).
Left Fibril multiscale structure. The hierarchical organization of triple-helix collagen polypeptides, cross-linked by immature and mature enzymatic cross-links. Definition of the representative unit system (RUS). Right Typical stress–strain responses of collagen fibrils from human-patellar tendon (HPT) and rat-tail tendon (RTT) where a different density of mature covalent cross-links occurs. Three distinct regions (denoted as I, II, and III) are experimentally observed
The load transmission within fibrils is highly affected by the presence or by the lack of mature cross-links because these cross-links between twisted microfibrils prevent intrafibrillar sliding mechanisms (Yang et al. 2012). Accordingly, the alteration in the biochemistry of these cross-links is an effective strategy for tuning the mechanical properties of tissues such as, for instance, their strength and stiffness (Carmo et al. 2002; Fratzl 2008; Fessel et al. 2012; Yang et al. 2012; Svensson et al. 2013). In general, fibrils subjected to a monotonic uniaxial traction present a stress–strain constitutive response characterized by three distinct regions: an initial rise in modulus (region I) followed by a plateau with reduced modulus (region II), which is finally followed by an even greater increase in stress and modulus before failure (region III; Svensson et al. 2013). Nevertheless, different mature cross-link density has been associated with significant differences in fibril constitutive response: fibrils in adult human-patellar tendons (HPTs) are characterized by about 890 mmol/mol mature cross-links and display a well-rendered region III of increasing modulus at high strains before abrupt failure (Svensson et al. 2013). In contrast, the density of mature cross-links in fibrils of rat-tail tendons (RTTs) is very low (about 8.7 mmol/mol) and their constitutive response displays a plateau leading up to failure with region III being almost absent (Svensson et al. 2013). Because of these different curve shapes and as schematically shown in Fig. 1, human-patellar fibrils fail at significantly higher stress than rat-tail fibrils and appear more brittle.
Understanding the relationship between biochemistry and mechanics in collagen fibrils is an open topic which is nowadays widely investigated (Uzel and Buehler 2011; Svensson et al. 2013; Marino and Vairo 2014a; Depalle et al. 2014). In fact, it would open toward the better comprehension of: physiological functional behavior of tissues in living and remodeling organs; pathological tissue mechanical dysfunctions associated with histochemical alterations; self-healing processes or pharmacological treatments. To this aim, internal deformation and failure mechanisms have to be elucidated, and their effects on fibril behavior should be described and analyzed. A wide literature exists on this field by following both experimental studies (Sasaki and Odajima 1996; Saito et al. 1997; Orgel et al. 2000; Bozec and Horton 2005; Orgel et al. 2006; Svensson et al. 2013) and atomistic computational approaches (Buehler 2006; Buehler and Wong 2007; Buehler 2008; Uzel and Buehler 2011; Depalle et al. 2014). Both approaches are incomparable resources for clarifying the in vivo, in vitro and in silico mechanical behavior of collagen molecules, cross-linked molecular assemblies, and entire fibrils. Nevertheless, from both aforementioned approaches, some limitations arise due to the complexity of the systems under investigations and the wide differences in the involved length scales. In fact, experimental techniques reach their limits in distinguishing molecular and intermolecular effects occurring within fibrils. On the other hand, molecular dynamics simulations (MDSs) at the atomic scale are feasible in terms of computational costs only for short cross-linked assemblies (long only few hundreds of nanometers; Uzel and Buehler 2011; Gautieri et al. 2014) even employing coarse-grained molecular descriptions (Buehler 2008; Depalle et al. 2014). Moreover, atomistic modeling requires the use of large deformation rates that often lead to underestimate molecular entropic effects and to overestimate the forces computed during the simulations (Klepeis et al. 2009; Depalle et al. 2014). Finally, the high computational cost of MDS prevents from the development of extensive parametric simulation campaigns and makes these simulations unhelpful at the scale of tissues and organs.
In this paper, starting from well-established experimental and atomistic computational evidence, an analytical model of collagen fibrils is presented, opening to parametric and low computing-cost simulations with a detailed insight on the elasto-damage mechanisms at nanoscale. Reference is made to the fibril multiscale model proposed by Marino and Vairo (2014a), in the following referred to as the MV-model, which allows to analyze the general influence of intermolecular effects on fibril mechanics. The MV-model, based on an internal constrained variational framework, starts from the definition of a nanoscale representative unit system (RUS, see Fig. 1) for the fibril. The RUS describes the main mechanical features that have to be upscaled, and it is analogous to the representative volume element in homogenization approaches (Kouznetsova et al. 2001; Hain and Wriggers 2008; Lehmann et al. 2012; Marino and Vairo 2014b). The definition of interscale compatibility relationships and of constitutive responses for the nanoscale constituents allows to obtain fibril constitutive response, explicitly based on the actual histochemical environment through model parameters with a clear physical sense. Moreover, this analytical approach might be employed within a structural multiscale approach for developing refined microscale models of collagen fibers and macroscale models of biological tissues (Maceri et al. 2010, 2012a, 2013; Marino and Vairo 2013, 2014b, c), opening to simulations of entire organs.
Nevertheless, the MV-model does not account for molecular entropic/energetic nonlinearities, and model outcomes have not been compared with fibril experimental constitutive responses. Since the multiscale approach proposed by Marino and Vairo (2014a) can straightforwardly incorporate refinements in the description of nanoscale constituents, the MV-model is here enriched. Both weak and covalent intermolecular interactions are addressed and coupled with a refined molecular description involving both entropic and energetic elasticity (Maceri et al. 2012b). Damage mechanisms involving both collagen molecules and their interactions are taken into account. Accordingly, the role of density and maturation of enzymatic cross-links on fibril elasto-damage response are investigated by showing their influence on the dominant mechanisms determining fibril internal elasto-damage mechanisms and, thereby, on their overall response. Model outcomes are compared with available atomistic results (Buehler 2008) and experimental data (Svensson et al. 2013), proving model effectiveness in capturing fibril mechanical behavior at different cross-link-related biochemical conditions. Moreover, the typical hysteresis behavior of collagen fibrils is recovered.
2 Multiscale model
The model is developed within the context of standard generalized materials (Germain et al. 1983; Frémond 2002) and by adopting a time-incremental operative framework. Accordingly, introducing the time variable \(\tau \) and the time increment \(d\tau \), the value of a given quantity x at the actual time \(\tau =t\) is obtained by superimposing the perturbation \(dx=\dot{x}d\tau \) to the reference value \(\bar{x}\) relevant to the time \(\bar{t}=t-d\tau \), where \(\dot{x}\) is the left-hand time derivative of x at t, in agreement with the causality principle. Moreover, \((O,{\varvec{\xi }}_1, {\varvec{\xi }}_2, {\varvec{\xi }}_3)\) is the three-dimensional Cartesian frame, with \({\xi }_1\), \({\xi }_2\), and \({\xi }_3\) being the corresponding coordinates, and \(f_{/3}\) denotes the partial derivative of f with respect to \(\xi _3\).
As a notation rule, subscript o identifies quantities in the initial configuration (i.e., at \(\tau =0\)), subscript k will take values in \(\{1,2\}\), and j in \(\{1,2,3\}\). Finally, \(H_a(x)\) denotes the Heaviside function centered at \(x=a\), with \(H_a(x)=0\) for \(x\le a\) and \(H_a(x)=1\) for \(x>a\).
After the description of fibril elasto-damage mechanisms that are herein addressed (see Sect. 2.1), the multiscale approach introduced by Marino and Vairo (2014a) in the MV-model is described in Sect. 2.2. Then, in the following Sects. 2.3 and 2.4, fibril and RUS model are, respectively, presented together with their coupling terms.
2.1 Evidence-based elasto-damage mechanisms
At fibril scale and starting from experimental observations, two in-series deformation mechanisms are introduced analogously to the MV-model. In fact, fibril strain can be experimentally measured by means of both small-angle and wide-angle X-ray diffraction patterns (Sasaki and Odajima 1996). In the latter case, when employing a reflection corresponding to the distance of few nanometers, a lower value of fibril strain is obtained with respect to the one obtained through small-angle X-rays (Sasaki and Odajima 1996). This evidence indicates that fibril deformation is governed at least by two distinct mechanisms.
Moreover, in order to account for the variability of yielding-like and brittle-like failure mechanisms observed for fibrils, (Svensson et al. 2013), three damage mechanisms are introduced: a perfectly-yielding behavior (yielding at constant stress), yielding with softening, and a brittle-like behavior. This choice generalizes the MV-model where only two fibril damage mechanisms were introduced allowing for the description only of either perfectly-yielding/softening-yielding or perfectly-yielding/brittle behaviors.
At nanoscale, starting from experimental and atomistic evidence and analogously to the MV-model, present model accounts for
-
slip-pulse (SP): localized breaking of weak cross-links and their continuous reactivation among adjacent residues during molecular sliding (Buehler 2006);
-
the delayed activation of enzymatic cross-links: the latter can indeed transmit the force and hold back molecular sliding only when the hook-shaped cross-linked telopeptide straightens out (Uzel and Buehler 2011; Depalle et al. 2014);
-
interstrand delamination (ID): a polypeptide strand within a collagen molecule slides with respect to the other two because the force transmitted through the covalent cross-link (acting upon a single strand) breaks the weak bonds inside the triple-helix structure. Accordingly, a strand pull-out mechanism altering molecular quaternary structure is promoted, (Buehler 2006; Uzel and Buehler 2011).
Moreover, as an enhancement with respect to the MV-model, further nanoscale mechanisms are accounted for:
-
the nonlinear coupling of entropic and energetic molecular deformation mechanisms (Buehler and Wong 2007; Fratzl 2008; Maceri et al. 2012b);
-
molecular covalent bond rupture (MR): molecular damage associated with the breaking of covalent bonds within the polypeptide strands (Buehler 2006; Maceri et al. 2012b);
-
a distinct behavior for mature and immature enzymatic cross-links (Depalle et al. 2014);
-
a refined and nonlinear activation rate for slip-pulse mechanisms.
Figure 2 represents a schematic representation of the elasto-damage internal mechanisms at nanoscale that are herein addressed for describing fibril mesoscale constitutive response.
Evidence-based fibril internal elasto-damage mechanisms. Left Molecular sliding related to slip-pulse (SP). Center Delayed activation of enzymatic covalent cross-links (both mature and immature) due to the straightening of the cross-linked telopeptide; interstrand delamination (ID) associated with a strand pull-out mechanism. Right Nonlinear molecular elasticity related to entropic (thermal fluctuations) and energetic (triple-helix uncoiling and stretching of molecular backbone) mechanisms; molecular covalent bond rupture (MR)
As interscale relationships and in agreement with Sasaki and Odajima (1996), the deformation mechanism associated with wide-angle X-ray diffraction is related to molecular elongation mechanisms because this technique allows to measure strains associated with the distance between neighboring amino acids along the collagen helix. Accordingly, the second deformation mechanism (the difference between the deformations associated with small-angle and wide-angle X-ray diffraction) is associated with molecular sliding.
Finally, fibril perfectly-yielding behavior is associated with SP (Buehler 2006; Uzel and Buehler 2011), softening-yielding failure with ID (Buehler 2006; Uzel and Buehler 2011), and brittle rupture with MR (Buehler 2006; Buehler and Wong 2007; Buehler 2008; Svensson et al. 2013).
2.2 Marino–Vairo’s multiscale approach
A collagen fibril in the actual configuration (at \(\tau =t\)) is regarded as a right circular cylinder \({\mathcal {F}}\) of radius \(r_f\) (assumed to be constant in time) and length \(\ell _f\) (assumed to be variable in time), whose axis is \({\varvec{\xi }}_3\). Thereby,
where \(A_f=\{(\xi _1,\xi _2)\, | \, \xi _1^2+\xi _2^2 \le r_{f}^2 \}\) is the fibril cross-sectional domain, whose constant measure is \({\mathcal {A}}_f=\pi r_f^2\).
Collagen molecules in the actual configuration are regarded, in turn, as cylinder-like sub-domains whose axes are aligned with the fibril’s one and whose measures of cross-sectional area, length and volume are denoted by \({\mathcal {A}}_m\) (assumed to be constant in time), \(\ell _m\) (assumed to be variable in time), and \(\varOmega _{m}={\mathcal {A}}_m \ell _m\), respectively.
The choice of employing a cylindrical shape for both molecules and fibrils is done in order to maintain the model simple and, thereby, to allow for an effective upscaling toward macroscale tissue mechanical modeling. Nevertheless, thanks to refined expressions of interscale compatibility relationships and constitutive laws in Sect. 2.4, dominant mechanisms related to the non-cylindrical geometry of fibril constituents are properly described. Results, shown in Sect. 3, highlight that this approach is effective for capturing essential nonlinear mechanisms of nanoscale constituents that affect fibril mechanics.
With reference to the initial configuration (at time \(\tau =0\)), average quantities describing the molecular arrangement within fibrils are introduced (Marino and Vairo 2014a):
-
\({\mathcal {O}}_{c}(\xi _1,\xi _2)\): molecular density along the \(\varvec{\xi }_3\)-direction;
-
\({\mathcal {A}}_c=\int _{A_f} {\mathcal {O}}_{c}(\xi _1,\xi _2) d\xi _1 d\xi _2\): average load-bearing area;
-
\(n_s=\ell _{f,o}/\ell _{m,o}\): average molecular number along \(\xi _3\);
-
\(n_a={\mathcal {A}}_c/{\mathcal {A}}_m\): average molecular number in \(A_f\);
-
\(\lambda _c^T\): average number of trivalent mature cross-links per collagen molecule.
-
\(\lambda _c^D\): average number of divalent immature cross-links per collagen molecule.
Fibril deformation and damage behavior are obtained by introducing a suitable set of state variables \({\mathcal {S}}_f=\{S_f^j \text { with } j=1,\ldots ,M_f\}\) that describes the mechanisms observed at the mesoscale. Dual to \({\mathcal {S}}_f\), there exist static quantities whose equilibrium relationships among themselves and with external actions can be straight obtained by means of the application of the principle of virtual power (PVP, Frémond 2002; Marino 2013).
Fibril elasto-damage response is obtained from equilibrium equations solved by employing suitable constitutive laws, namely relationships between state quantities and the corresponding dual static quantities. Denoting with \(\dot{{\mathcal {S}}}_f\) the set collecting the time derivatives of \({\mathcal {S}}_f\) and following Frémond (2002), constitutive laws are obtained by subdifferentiation of fibril free energy \(\psi _f=\psi _f({\mathcal {S}}_f)\) (with the unit of work per unit length) and pseudo-potential of dissipation \(\phi _f=\phi _f(\dot{{\mathcal {S}}}_f)\) (with the unit of power per unit length). These functions are defined by adopting a multiscale rationale based on the detailed description of fibril constituents’ behavior and employing the definition of the RUS.
Fibril RUS is herein chosen as: one collagen molecule (\({\mathcal {M}}\)); \(\lambda _c^T\) and \(\lambda _c^D\) covalent cross-links among \({\mathcal {M}}\) and surrounding molecules; a linear density of weak interactions among \({\mathcal {M}}\) and surrounding molecules, constant along the length of \({\mathcal {M}}\).
Then, the RUS is described by introducing nanoscale state variables collected in \({\mathcal {S}}_R=\{{\mathcal {S}}_m,{\mathcal {S}}_c,{\mathcal {S}}_w\}\), where \({\mathcal {S}}_m =\{s_m^n \text { with } n=1,\ldots ,M_m\}\), \({\mathcal {S}}_c=\{s_c^n \text { with } n=1,\ldots ,M_c\}\) and \({\mathcal {S}}_w=\{s_w^n \text { with } n=1,\ldots ,M_w\}\) are the sets of the state variables describing \({\mathcal {M}}\), covalent cross-links (both mature and immature) and intermolecular weak bonds, respectively. Deformation and damage at the fibril’s scale are related to nanoscale mechanisms within the RUS by introducing interscale compatibility relationships between \({\mathcal {S}}_R\) and \({\mathcal {S}}_f\). Nanoscale state variables are assumed to be constant within a single RUS (hence, modeled as a zero-dimensional domain) and in \(A_f\), but they can depend on \(\xi _3\) when referred to RUSs located at different values of \(\xi _3\).
Mechanics of nanoscale constituents of the RUS is, in turn, described by means of free energies \(\varPsi _m({\mathcal {S}}_m)\), \({\mathcal {E}}_c^T({\mathcal {S}}_c)\), \({\mathcal {E}}_c^D({\mathcal {S}}_c)\), \({\mathcal {E}}_w({\mathcal {S}}_w)\), and pseudo-potential of dissipation \(\varPhi _m(\dot{{\mathcal {S}}}_m)\), \({\mathcal {D}}_c^T(\dot{{\mathcal {S}}}_c)\), \({\mathcal {D}}_c^D(\dot{{\mathcal {S}}}_c)\), \({\mathcal {D}}_w(\dot{{\mathcal {S}}}_w)\) for molecules, mature covalent cross-links, immature covalent cross-links, and intermolecular weak bonds, respectively.
Accordingly, RUS free energy (with the unit of work per unit length) is defined as
where \(\bar{\psi }_R=\psi _R|_{\tau =\bar{t}}\) is RUS reference free energy,
and where an analogous notation holds for the term involving \(F_c^T\) (resp., \(F_c^D\) and \(F_w\)) by employing \(s_c^n\), \({\mathcal {S}}_c\), \(M_c\), \({\mathcal {E}}_c^T\) (resp., \(s_c^n\), \({\mathcal {S}}_c\), \(M_c\), \({\mathcal {E}}_c^D\), and \(s_w^n\), \({\mathcal {S}}_w\), \(M_w\), \({\mathcal {E}}_w\)) instead of \(s_m^n\), \({\mathcal {S}}_m\), \(M_m\), \(\varPsi _m\).
Thereby, fibril free energy is defined as
where \({\mathcal {I}}_d\) is an interaction free-energy contribution.
Finally, RUS pseudo-potential of dissipation is defined as
and fibril pseudo-potential of dissipation is
2.3 Fibril model
Since fibrils mainly undergo uniaxial traction, fibril deformation at mesoscale is described by introducing centerline axial displacement \(u=u(\xi _3)\) which can be observed through small-angle X-ray diffraction. As previously described and following experimental evidence (see Sect. 2.1), the two in-series mechanisms, contributing to fibril axial deformation, are described by
-
\(u_1\), related to wide-angle X-ray diffraction,
-
\(u_2\), related to the difference between small-angle and wide-angle X-ray diffraction,
with \(du_1+du_2=du\). In agreement with classical bar’s theories, natural strain measures \(e_1\) and \(e_2\) are introduced, by defining their perturbations as \(de_1=du_{1/3}\) and \(de_2=du_{2/3}\).
Fibril damage is described by means of variables
-
\(\beta _1(\xi _3)\) and \(\gamma _1(\xi _3)\), related to yielding with softening,
-
\(\beta _2(\xi _3)\) and \(\gamma _2(\xi _3)\), related to perfectly yielding behavior,
-
\(\beta _3(\xi _3)\) and \(\gamma _3(\xi _3)\), related to a brittle behavior,
with \(\beta _j\) valued in [0, 1], where \(\beta _j=1\) means that the damage mechanism is not activated and \(\beta _j=0\) that it is fully activated. Variables \(\gamma _j\) are introduced to describe damage diffusion, by defining \(\gamma _j=\beta _{j/3}\).
Accordingly, fibril state variables are
The static quantities associated with \({\mathcal {S}}_f\) are the normal forces \(N_k=N_k(\xi _3)\) (dual to \(e_k\)), the sub-mesoscale damage forces \(b_j=b_j(\xi _3)\) (dual to \(\beta _j\)), and the sub-mesoscale damage works \(h_j=h_j(\xi _3)\) (dual to \(\gamma _j\)).
Consider \(u|_{\xi _3=0}=0\) as a displacement boundary condition, and let the fibril be loaded by the force \(\mathbf{F}=F_f{\varvec{\xi }}_3\) at \(\xi _3=\ell _f\) and by the axial force density \(\mathbf{q}(\xi _3)=q_f(\xi _3){\varvec{\xi }}_3\). Moreover, let \(a_f=a_f(\xi _3)\) be a non-mechanical source of damage (e.g., biochemical, electrical, magnetic) distributed along the fibril length. Accordingly, through the application of the PVP (Marino 2013; Marino and Vairo 2014a), the equations governing the equilibrium of the afore-introduced static quantities are:
with boundary conditions:
Finally, constitutive laws are:
Following Eq. (2), only the interaction free-energy contribution has to be defined at the fibril scale, and it is herein chosen as:
where \(k_j\) denotes damage diffusion coefficient. The other quantities for the definition of \(\psi _f\) and \(\phi _f\) as in Eqs. (2) and (3) will straightly derive from the nanoscale model, described in what follows.
2.4 RUS model
Before introducing RUS state variables, some auxiliary quantities are conveniently introduced: molecular nominal strain measure \(\varepsilon _m=\ell _m/\ell _{m,o}-1\) (\(\ell _{m,o}\) being molecular length at \(\tau =0\)) and molecular sway \(\delta \). Accordingly, actual RUS length is \(\ell _R=\ell _m+\delta \) (with \(\ell _{R,o}=\ell _{m,o}\) being RUS length at \(\tau =0\)) and nominal strain is described by \(\varepsilon _R=\ell _{R}/\ell _{R,o}-1=\varepsilon _m+\varepsilon _{CL}\) where \(\varepsilon _{CL}=\delta /\ell _{m,o}\).
Moreover, two contributions for molecular sliding are introduced: \(\delta _w\), being molecular sway associated with weak bonds deformation, and \(\delta _c\), being molecular sway associated with covalent bonds deformation. In detail, it results:
being

the activation function of covalent cross-links. Thereby, two parameters have been introduced: \(\mu \in {\mathbb {R}}^+\) is an activation shape parameter and \(\delta _o\) is a measure of the molecular sway inducing the telopeptide straightening, responsible for the delayed activation of the covalent cross-link stretch (Uzel and Buehler 2011; Marino and Vairo 2014a).
The deformation of covalent cross-links is assumed to be elastic and thereby fully recovered upon loading removal. Mature and immature cross-links are assumed to act as in-parallel, and thereby they can be described introducing a unique strain measure. The deformation of both weak cross-links and molecules is regarded as the series of elastic (denoted by superscript e) and inelastic contributions. Inelastic contributions are not recovered upon unloading: plastic-like mechanisms (denoted by superscript p) are associated with residual strains due to either SP or ID; brittle-like mechanisms (denoted by superscript b) are associated with molecular fracture due to MR. Damage of molecular structural integrity (namely, ID and MR) is associated with relaxation mechanisms (that is, a decay of elastic strain with time).
Accordingly, the RUS elasto-damage mechanisms are herein described by
-
for weak cross-links:
-
the elastic weak-related molecular sliding \(\delta _w^e\), or equivalently \(\varepsilon _w^e=\delta _w^e/\ell _{m,o}\);
-
the SP-inelastic weak-related molecular sliding \(\delta _w^p\), or equivalently \(\varepsilon _w^p=\delta _w^p/\ell _{m,o}\);
-
damage parameter \(\beta _w\) associated with the rupture of intermolecular weak bonds and thereby with SP;
-
-
for covalent cross-links:
-
the elastic covalent-related molecular sliding \(\varepsilon _c=\delta _c/\ell _{m,o}\);
-
-
for collagen molecules:
-
the elastic molecular strain \(\varepsilon _m^e\);
-
the ID-inelastic molecular strain \(\varepsilon _m^p\) associated with a molecular relaxation mechanism governed by the decay time constant \(\tau _o^{ID}\);
-
the MR-inelastic molecular strain \(\varepsilon _m^b\) associated with a molecular relaxation mechanism governed by the decay time constant \(\tau _o^{MR}\);
-
damage parameter \(\beta _d\) associated with the rupture of molecular interstrand weak bonds and thereby with ID;
-
damage parameter \(\beta _m\) associated with the rupture of molecular intrastrand covalent bonds and thereby with MR.
-
With respect to the MV-model, \(\varepsilon _m^b\) and \(\beta _m\) enrich molecular description. In detail, when \(\beta _m=1\), intramolecular covalent bonds are considered to be sound, possible molecular strain increment is either elastic or ID-inelastic (depending on the value of \(\beta _d\)), and MR-inelastic strain is not activated (that is, \(\dot{\varepsilon }_m^b=0\)); when \(\beta _m=0\), intramolecular covalent bonds are considered to be totally broken, and possible molecular strain increment is neither elastic nor ID-inelastic (that is, \(\dot{\varepsilon }_m^e=\dot{\varepsilon }_m^p=0\)); when \(0<\beta _m<1\), intramolecular covalent bonds are considered to be partially broken, and elastic, ID-inelastic and MR-inelastic mechanisms may occur.
The relationships between \(\varepsilon _m^p\) and \(\beta _d\) and between \(\varepsilon _w^p\) and \(\beta _w\) are analogous to the one previously described for \(\varepsilon _m^b\) and \(\beta _m\) (Marino and Vairo 2014a).
In Fig. 3, nanoscale elasto-damage mechanisms described by present model are schematically represented. In summary, RUS state variables are herein chosen as:
2.4.1 Constitutive models at the nanoscale
Constitutive choices are introduced by following an internal constrained approach. Physical restrictions are introduced directly in the variational formulation by means of suitable indicator functions and employing arguments from convex analysis (see Appendix section “Basics of convex analysis”).
Similar to the MV-model, covalent cross-links are assumed to behave as linearly elastic. Trivalent mature (superscript T) and divalent immature (superscript D) cross-links are described by:
where \(K_c^T=k_c^T\ell _{m,o}^2\), \(K_c^D=k_c^D\ell _{m,o}^2\), and \(k_c^T\) (resp., \(k_c^D\)) is the mature (resp., immature) covalent cross-link stiffness.
The mechanics of weak cross-links is herein described by defining
where \({\mathcal {E}}_w^{el}(\varepsilon _w^e) = K_w [\varepsilon _w^e]^2/2\) is the weak-bonds elastic energy, with \(K_w=k_w \ell _{m,o}^2\), \(k_w\) is the weak-bond stiffness, \(w_w\) and \(c_w\) are the activation threshold and viscosity of intermolecular weak-bonds damage, respectively. Moreover, \(\hbox {I}_{[0,1]}\) is introduced to enforce as an internal constraint the restriction \(\beta _w \in [0,1]\), and \({\mathcal {E}}_w^{sp}\) is the free-energy part contributing to SP, here chosen as \({\mathcal {E}}_w^{sp} = {\mathcal {E}}_w^{el}(\bar{\varepsilon }_w^e)\). The aforementioned terms correspond to the ones proposed in the MV-model. Nevertheless, a new term is here introduced, namely
-
\(\hbox {I}^+(\dot{\beta }_w+\bar{\beta }_w^\omega v_w)\) with \(v_w,\, \omega \in {\mathbb {R}}^+\) that enforces the restriction
$$\begin{aligned} \dot{\beta }_w \ge - \bar{\beta }_w^\omega v_w. \end{aligned}$$(11)Accordingly, a controlled activation rate of slip-pulse, nonlinearly depending on damage amount, is introduced. Results will clearly show that this choice straightly follows from experimental data. It is also worth pointing out that no upper bound for damage rate of weak bonds is introduced and thereby damage evolution can be lower or higher than zero (namely, damage is reversible). Accordingly, weak cross-links may break and reform.
Constitutive response of \({\mathcal {M}}\) is defined by:
Analogously to the MV-model, intramolecular hydrogen-bonds damage is governed by threshold \(w_d\), viscosity \(c_d\), and free-energy part contributing to damage onset \(\varPsi _m^{ID}\). Introducing
and in order to account for mature and immature cross-links, \(\varPsi _m^{ID}\) is assumed to be:
No restrictions are introduced for ID damage rate.
Moreover, new terms are introduced in the present paper for the refined modeling of molecular behavior
-
the activation threshold \(w_m\) and viscosity \(c_m\) of intramolecular covalent bonds damage;
-
\(\text {I}_{[-v_m,0]}(\dot{\beta }_m)\) with \(v_m \in {\mathbb {R}}^+\), introduced to enforce the restriction \(\dot{\beta }_m \le 0\), that is molecular damage is irreversible, but also that maximum damage rate is limited, namely \(\dot{\beta }_m\ge -v_m\), as previously shown by Maceri et al. (2012b);
-
the nonlinearly elastic free-energy contribution \(\varPsi _m^{e}\),
$$\begin{aligned} \varPsi _m^{e}\left( \varepsilon _m^e\right) :=\bar{\varPsi }_m^{e} + \bar{\sigma }_m d\varepsilon _m^e +\frac{E_m\left( \bar{\varepsilon }_m^e\right) \left[ d\varepsilon _m^e\right] ^2}{2}, \end{aligned}$$(13)from which elastic molecular stress results
$$\begin{aligned} \sigma _m\left( \varepsilon _m^{e}\right) :=\frac{\partial \varPsi ^{e}_m}{\partial d\varepsilon _m^e}=\bar{\sigma }_m+E_m\left( \bar{\varepsilon }_m^e\right) d\varepsilon _m^e, \end{aligned}$$(14)and where \(\bar{\varPsi }_m^{e}=\varPsi _m^{e}|_{\tau =\bar{t}}\), \(\bar{\sigma }_m=\sigma _m|_{\tau =\bar{t}}\), and function \(E_m(\varepsilon _m^e)\) is the molecular tangent elastic modulus, depending on entropic–energetic in-series mechanisms (Maceri et al. 2012b). The entropic mechanism is associated with strain contribution \(\varepsilon _m^{e,s}\) and tangent modulus \(E_m^s(\varepsilon _m^{e,s})\), the energetic mechanism with strain \(\varepsilon _m^{e,h}\) and modulus \(E_m^h(\varepsilon _m^{e,h})\). Accordingly, \(E_m\) is defined as
$$\begin{aligned} E_m\left( \varepsilon _m^e\right) :=\frac{E_m^s\left( \varepsilon _m^{e,s}\right) E_m^h\big (\varepsilon _m^{e,h}\big )}{E_m^s\left( \varepsilon _m^{e,s}\right) + E_m^h\big (\varepsilon _m^{e,h}\big )}, \end{aligned}$$(15a)where \(\varepsilon _m^{e,s}\) and \(\varepsilon _m^{e,h}\) are obtained from solving the following differential problem:
$$\begin{aligned} \dot{\varepsilon }_m^{e,s}=\frac{E_m\big (\varepsilon _m^e\big ) \dot{\varepsilon }_m^e}{E_m^s\big (\varepsilon _m^{e,s}\big )}, \quad \dot{\varepsilon }_m^{e,h}=\frac{E_m\left( \varepsilon _m^e\right) \dot{\varepsilon }_m^e}{E_m^h\big (\varepsilon _m^{e,h}\big )}. \end{aligned}$$(15b)Entropic stiffness \(E_m^s\) models thermal fluctuations by recovering the well-established worm-like chain model (Marko and Siggia 1995; Maceri et al. 2012b), and it is defined as
$$\begin{aligned} E_m^s\left( \varepsilon _m^{e,s}\right) := \frac{k_B T}{{\mathcal {A}}_m \ell _p} \left\{ \frac{r_{\ell }}{2\left[ 1-r_{\ell }\left( 1+\varepsilon _m^{e,s}\right) \right] ^3} + r_{\ell } \right\} , \end{aligned}$$(15c)where \(k_B\) is the Boltzmann constant, T the absolute temperature, \(\ell _p\) molecular persistence length, and \(r_{\ell }=\ell _{m,o}/\ell _c\) with \(\ell _c\) the molecular contour length. Finally, \(E_m^h\) models triple-helix uncoiling and stretching of molecular backbone (Buehler 2006; Maceri et al. 2012b), and it is defined as
$$\begin{aligned} E_m^h\big (\varepsilon _m^{e,h}\big ) := \frac{\hat{E}r_{\ell }}{1+e^{-\eta \left( r_{\ell }\varepsilon ^{e,h}_m - \varepsilon _o^h\right) }} + \hat{E}_o r_{\ell }, \end{aligned}$$(15d)where \(\hat{E}\), \(\hat{E}_o\), \(\varepsilon _o^h\), and \(\eta \) are model parameters associated with the uncoiling of the triple-helix collagen structure.
-
\(\varPsi _m^b\): free-energy part contributing to damage of intramolecular covalent bonds, herein chosen as
$$\begin{aligned} \varPsi _m^{b} := {\varPsi }_m^{e}\left( \tilde{\varepsilon }_m^{e}\right) \quad \text { with} \quad \tilde{\varepsilon }_m^{e}:=\underset{\tau \in [0,\bar{t}]}{\text {max}}\left\{ \varepsilon _m^e(\tau )\right\} . \end{aligned}$$(16)
2.5 Interscale compatibility relationships
Nanoscale state variables in \({\mathcal {S}}_R\) are related to the fibril’s, \({\mathcal {S}}_f\), by means of incremental interscale compatibility relationships in agreement with the afore-described interscale evidence (see Sect. 2.1).
Firstly, let the following convenient control functions be introduced
The first mesoscale deformation mode \(e_1\) is associated with molecular elongation through
resulting
On the other hand, the second mesoscale deformation mode \(e_2\) is associated with molecular sway by choosing \(d\delta =\ell _{m,o} d\varepsilon _{CL}=\bar{\ell }_R de_2\) and, distinguishing the different mechanisms,
Thereby, since \(d\delta _w = \ell _{m,o}(d\varepsilon _w^e + d\varepsilon _w^p)\) and \(d\delta _c =\ell _{m,o} d\varepsilon _c\),
Finally, fibril and nanoscale damage perturbations are related each other as
2.6 Governing equations
For obtaining an expression for fibril free energy within the present multiscale framework, it is firstly worth pointing out that \({\mathcal {E}}_c^T={\mathcal {E}}_c^T(\varepsilon _c)\) and \({\mathcal {E}}_c^D={\mathcal {E}}_c^D(\varepsilon _c)\) from Eq. (9a), \({\mathcal {E}}_w={\mathcal {E}}_w(\varepsilon _w^e,\beta _w)\) from Eq. (10a), and \(\varPsi _m=\varPsi _m(\varepsilon _m^e,\beta _d,\beta _m)\) from Eq. (12a). Accordingly, among the full set of interscale compatibility relationships, the functional dependencies \(\varepsilon _m^e=\varepsilon _m^e(e_1)\) from Eq. (17a), \(\varepsilon _w^e=\varepsilon _w^e(e_2)\) from Eq. (18a), \(\varepsilon _c=\varepsilon _c(e_2)\) from Eq. (18c), \(\beta _d=\beta _1\), \(\beta _w=\beta _2\), and \(\beta _m=\beta _3\) from Eq. (20) are highlighted for deriving constitutive relationships. Moreover, it is noted that, in the present incremental formulation, the derivative with respect to a given quantity (e.g., x) corresponds to the derivative with respect to its perturbation (namely, dx).
Based on the definition (1) and on previous consideration, fibril free energy in Eq. (2) results equal to
where Eq. (7) has been employed. Moreover, from Eqs. (9b), (10b), and (12b), pseudo-potential of dissipation \(\phi _f\) in Eq. (3) straight results equal to
Accordingly, from Eqs. (6a) and (21), fibril axial forces are:
where \(\bar{E}_m=E_m(\bar{\varepsilon }_m^e)\) and \(k_c^{eq}= \lambda _c^T k_c^T + \lambda _c^D k_c^D\). Moreover, from Eqs. (6b), (6c) and (22), static quantities associated with fibril damage are
Accordingly, governing equations of fibril’s elasto-damage behavior are obtained by combining equilibrium conditions (5) with interscale compatibility relationships (17), (18), and (20) and constitutive relationships (23) and (24).
For evaluating model performance, MDS/experimental data on homogenous uniaxial traction only are available in literature. Accordingly, in the present work, equilibrium relationships are solved by considering \(F_f\ne 0\) and \(q_f=a_f=0\) in order to reproduce numerical/experimental conditions.
In this simplifying case, equilibrium Eq. (5a) with boundary conditions (5c) reduces to
where \(\rho _a={\mathcal {A}}_c/{\mathcal {A}}_f\) is the ratio between load-bearing area and total fibril cross-sectional area, \(\sigma _f\) is fibril nominal stress related to molecular stress \(\sigma _m=\sigma _m(\varepsilon _m^e)\) [see Eq. (14)], weak cross-links stress \(\sigma _w=k_w \delta _w^e/{\mathcal {A}}_m\), and covalent cross-links stress \(\sigma _c=\lambda _c^D \sigma _c^D + \lambda _c^T\sigma _c^T\) where \(\sigma _c^D=k_c^D \delta _c/{\mathcal {A}}_m\) and \(\sigma _c^T=k_c^T \delta _c/{\mathcal {A}}_m\) refer to immature divalent and mature trivalent cross-links, respectively.
Governing equation for fibril stretch is obtained by combining Eqs. (17), (18), (23), and (25), and it results
where \(\bar{r}_c= k_c^{eq}\bar{\delta }_c\) and \(\bar{r}_w^e = k_w\bar{\delta }_w^e\).
Moreover, let introduce
It is worth pointing out that, from previous relationships, the quantity \(d\check{\beta }_2/d\tau +\bar{\beta }_w^\omega v_w \ge 0\) is a priori in the definition domain of \(\partial \hbox {I}^+\). Similarly, \(-v_w \le d\check{\beta }_3/d\tau \le 0\), and thereby, the quantity \(d\check{\beta }_3/d\tau \) is a priori in the definition domain of \(\partial \hbox {I}_{[-v_m,0]}\). Accordingly, by denoting \(\check{\beta }_j=\bar{\beta _j}+d\check{\beta }_j\) and from the definition domain of \(\partial \text {I}_{[0,1]}\), damage parameters \(\beta _j\) are obtained from
being solutions for Eq. (5b), combined with Eqs. (20) and (24). It is worth pointing out that, under homogenous uniaxial traction, \(\beta _{1/3}=\beta _{2/3}=\beta _{3/3}=0\) for any \(\xi _3\in [0,\ell _f]\), because all quantities are constant in space. Accordingly, results are not affected by damage diffusion coefficients \(k_j\).
In Eq. (27), the onset of damage is governed by damage thresholds \(w_w\), \(w_d\), and \(w_m\). Useful relationships for setting the values of the latter starting from available data are:
with \(K_{ID}=H_0(\lambda _c^T)K_c^T +H_0(\lambda _c^D)K_c^D\) and where, referring to the onset of SP, ID and MR, \(\varepsilon _{*}\) and \(\sigma _{*}\) are the strain (normalized with respect to \(\ell _{m,o}\)) and the stress (normalized with respect to \({\mathcal {A}}_m\)) at constituent scale, while \(\sigma _f^*\) is the stress (normalized with respect to \({\mathcal {A}}_f\)) at fibril scale (with \(*=\{SP,\, ID,\, MR\}\)). In other words, introducing the ID-related covalent cross-link-stress
a damage mechanism activates when the corresponding stress reaches its threshold value: when \(\sigma _w=\sigma _{SP}\), then SP activates; when \(\sigma _c^{ID}=\sigma _{ID}\), then ID activates; when \(\sigma _m=\sigma _{MR}\), then MR activates.
3 Results
The outcomes obtained by proposed model are compared with both results from atomistic computations and experimental data on fibril monotonic uniaxial traction. Moreover, an application reproducing a traction loading–unloading test is proposed.
In a displacement-based approach where fibril nominal strain \(\varepsilon _f:=u/\ell _{f,o}\) is the control variable, Eqs. (26) and (27) can be solved in the variables \(de_k\) and \(\beta _j\) by accounting for the compatibility incremental relationship:
The influence on fibril mechanics of the values of
-
cross-link densities \(\lambda _c^D\) and \(\lambda _c^T\),
-
parameters \(\omega \), \(c_w/w_w\) and \(v_w\),
-
molecular relaxation time constant \(\tau _o^{MR}\),
is shown in order to discuss on their sensitivity and to justify modeling choices. Parametric analyses for other model parameters are herein not addressed because they are already discussed in previous works (Maceri et al. 2012b; Marino and Vairo 2014a).
Results will be shown in terms of fibril constitutive relationship \(\sigma _f\) versus \(\varepsilon _f\) and the evolution of damage variables \(\beta _j\). Moreover, internal state variables \({\mathcal {S}}_R\) and stresses \(\sigma _m\) [see Eq. (14)], \(\sigma _c\), \(\sigma _c^{ID}\) and \(\sigma _w\) [see Eqs. (25) and (29)] are reported. Furthermore, the tangent modulus related to the fibril (\(E_f\)), to the weak cross-links (\(E_w\)) and to the covalent cross-links (\(E_c\)),
will be shown, obtaining also the overall intermolecular stiffness \(E_{CL}=E_c+E_w\), through the consideration that weak and covalent cross-links are in-parallel mechanisms. By referring to Eq. (15), molecular stiffness \(E_m(\varepsilon _m(\bar{\varepsilon }_f))\) will be reported together with entropic \(E_m^s(\varepsilon _m^{e,s}(\bar{\varepsilon }_f))\) and energetic \(E_m^h(\varepsilon _m^{e,h}(\bar{\varepsilon }_f))\) contributions. Finally, the values at fibril failure (assumed to occur when fibril stress starts to decrease with increasing strain) for \(\sigma _c^{ID}\), \( \sigma _m\), \(\sigma _f\), \(\varepsilon _c\), \(\varepsilon _m^e\) and \(\varepsilon _f\) will be denoted as \(s_c^{ID}\), \(s_m\), \(s_f\), \(d_c\), \(d_m^e\) and \(d_f\), respectively.
As a result of a sensitivity analysis, computational steps have been chosen in order to obtain strain increments \(d\varepsilon _f\) such that \(\varepsilon _{f}^{end}=2\cdot 10^4 |d\varepsilon _f|\) where \(\varepsilon _{f}^{end}\) is the fibril strain at the end of the simulation (\(\varepsilon _{f}^{end}=0.5\) for MDS and \(\varepsilon _{f}^{end}=0.26\) for experiments). All simulations are performed at the body temperature \(T=310\) K, employing \(\rho _a={\mathcal {A}}_c/{\mathcal {A}}_f=0.7\) from experimental data (Holmes et al. 2001; Marino and Vairo 2014a).
3.1 Comparison with MDS data
Model predictions are compared with results obtained by means of MDS approaches (Buehler 2008) where the uniaxial traction (with displacement rate \(\dot{\ell }_f=0.4\) m/s) of an array of \(2\times 5\) collagen molecules is considered (\(n_a=5\), \(n_s=2\), \(\ell _c=287\) nm, and \({\mathcal {A}}_m=1.41\) nm\(^2\)).
MDSs have been carried out by Buehler (2008) adopting coarse-grained models of collagen fibrils and employing some simplifying assumptions. The latter can be straightforwardly reproduced by the proposed model, and in order to have a meaningful comparison, values of model parameters are chosen in order to reproduce the atomistic numerical model adopted in Buehler’s simulations. The procedure adopted for setting the values of model parameters is explained in what follows.
Buehler includes weak cross-links through a Lennard-Jones potential, and covalent cross-links are modeled through an increased Lennard-Jones adhesion at the ends of each molecule where a constant proportionality factor of \(\varpi =12.5\) corresponds to one covalent cross-link for each molecule and \(\varpi =1\) to zero covalent cross-links for each molecule. Since Buehler’s model is bidimensional, immature divalent cross-links are considered only. Accordingly, cross-link density parameters are computed as \(\lambda _c^D=(\varpi -1)/11.5\), \(k_w=k_c^D/11.5\), \(\lambda _c^T=0\), and \(k_c^T\) is ineffective.
Moreover, the delayed activation of covalent cross-links is not considered in Buehler’s simulations. This is reproduced by employing \(f_c(x)=1\), instead of the expression given in Eq. (8b), in the interscale compatibility relationships. Furthermore, addressing collagen elasticity, Buehler neglects entropic mechanisms. Thereby, it is chosen \(\ell _{m,o} \rightarrow \ell _c\) in order to obtain \(E_m^s \rightarrow + \infty \) [see Eq. (15c)] and \(E_m \rightarrow E_m^h\) [see Eq. (15a)].
For setting the remaining parameters governing fibril response in the purely elastic regime \(\beta _1=\beta _2=\beta _3=1\) [that is, parameters in Eq. (15d), and \(k_c^D\)], Buehler’s data on functions \(E_m(\varepsilon _f)\) and \(E_f(\varepsilon _f)\), available for \(\varpi =25\), have been fitted by means of a trial-error procedure. Accordingly, as shown in Fig. 4 and by choosing \(\hat{E}_o = 8.5\) GPa, \(\hat{E} = 48\) GPa, \(\eta =60\), \(\varepsilon _o^h = 0.305\), and \(k_c^D=7\) nN/nm , the good agreement between obtained and available data for both \(E_m(\varepsilon _f)\) and \(E_f(\varepsilon _f)\) at \(\varpi =25\) proves the correspondence between Buehler’s and the proposed model for what concerns fibril elastic response.
Multiscale analytical model applied to coarse-grained atomistic conditions. Fibril \(E_f\) and molecular \(E_m\) tangent moduli versus fibril strain \(\varepsilon _f\). Comparison between present model and molecular dynamics simulations (MDSs) by Buehler (2008) employing the increased adhesion proportionality factor \(\varpi =25\), (namely, \(\lambda ^D_c=(\varpi -1)/11.5\approx 2\)). The values of model parameters are reported in text (see Sect. 3.1)
Addressing damage-related parameters in Eq. (27), their values are set by means of the following considerations:
-
damage thresholds \(w_w\), \(w_d\) and \(w_m\) are set on the basis of fibril strengths by means of Eq. (28). By referring to a given value of the increased adhesion proportionality factor \(\varpi \), \(s^{\varpi }\) denotes the ultimate stress value obtained by Buehler’s simulations, and it is chosen
$$\begin{aligned}&\sigma _f^{SP} =s^1, \quad \sigma _f^{ID}=\frac{s^5+s^{10}+s^{15}+s^{20}+s^{25}}{5}, \\&\sigma _f^{MR} =\frac{s^{35}+s^{40}}{2}, \, \end{aligned}$$because: \(\varpi =1\) corresponds to zero covalent cross-links and thereby to the activation of SP; \(\varpi =5,10, 15, 20\) and 25 are associated with softening-yielding behavior, herein associated with ID; \(\varpi =35\) and 40 correspond to fibril brittle rupture, related to MR. Accordingly, it results \(\sigma _f^{SP}=0.38\) GPa, \(\sigma _f^{ID}=2.29\) GPa, and \(\sigma _f^{MR}=6.29\) GPa.
-
damage rate is governed by the normalized damage viscosity parameters \(c_{*}/w_{*}\) (with \(*=\{w,d,m\}\)) and, for SP and MR, maximum damage rates \(v_w\) and \(v_m\). In agreement with the non-smoothness of Buehler’s data, a quasi-instantaneous damage is herein assumed by choosing \(c_{*}/w_{*}=1/v_w=1/v_m=1\) ps;
-
molecular relaxation time constant associated with ID is chosen by fitting the stress softening-rate for \(\varpi =10\) and its results equal to \(\tau _o^{ID}=0.6\) \(\upmu \)s; molecular relaxation time constant associated with MR is chosen from the slope of the stress drop obtained at fibril failure for \(\varpi =35\) and it results \(\tau _o^{MR}=10\) ns.
Addressing different values of covalent cross-link density, the comparison between obtained constitutive relationship and available MDS data is shown in Fig. 5. Addressing the entire range of values for cross-link density, the average error on fibril ultimate stress results to be equal to \(3.9\,\%\). Moreover, Fig. 6 shows the evolution of damage variables \(\beta _j\) as well as of nanoscale strain measures associated with the dominant mechanisms that govern fibril mechanics.
Multiscale analytical model applied to coarse-grained atomistic conditions. Stress \(\sigma _f\) versus strain \(\varepsilon _f\) constitutive relationship of a collagen fibril with varying cross-link density \(\lambda _c^D\): comparison between predictions obtained by means of proposed model (continuous lines) and of molecular dynamics simulations (dashed lines with symbols) by Buehler (2008). Different values of Buehler’s increased adhesion proportionality factor \(\varpi \), with \(\lambda _c^D=(\varpi -1)/11.5\), are addressed. The values of model parameters are reported in text (see Sect. 3.1)
Multiscale analytical model applied to coarse-grained atomistic conditions. State variables in a fibril representative unit system (RUS) versus fibril strain \(\varepsilon _f\) with different values of Buehler’s increased adhesion proportionality factor \(\varpi \), namely with varying cross-link density \(\lambda _c^D=(\varpi -1)/11.5\). The values of model parameters are reported in text (see Sect. 3.1)
3.2 Comparison with experimental data
Model predictions are compared with experimental data by Svensson et al. (2013) on the uniaxial traction of long collagen fibrils at constant elongation rate, \(\dot{\ell }_f=40\) \(\upmu \)m/s. Collagen fibrils from both human-patellar tendon (HPT, length \(\ell _{f,o}=33.05\) \(\upmu \)m and diameter \(2r_f=0.153\) \(\upmu \)m) and rat-tail tendon (RTT, length \(\ell _{f,o}=29.50\) \(\upmu \)m and diameter \(2r_f=0.190\) \(\upmu \)m) are addressed.
The values of model parameters employed in the present application are listed in Table 1. Biophysical parameters governing molecular elastic response are chosen on the basis of well-established results (Buehler and Wong 2007; Maceri et al. 2012b; Marino and Vairo 2013, 2014c). The density of mature cross-links is the one reported from biochemical analysis by Svensson et al. (2013), while immature cross-link density is chosen on the basis of experimental studies by Saito et al. (1997). Other model parameters are tuned in order to fit the available experimental data by following a trial-error procedure. A unique set of parameters governing the elasto-damage response of collagen molecules and of covalent cross-links (except for their density) are employed for HPT and RTT fibrils, while parameters governing the intermolecular weak interactions are kept independent for the two cases herein addressed.
The comparisons between experimental data and model outcomes are presented in Fig. 7 in terms of both \(E_f\) versus \(\varepsilon _f\) and \(\sigma _f\) versus \(\varepsilon _f\) relationships. Choosing experimental data as a reference, it is obtained an average error of about \(1\,\%\) for the \(\sigma _f\) versus \(\varepsilon _f\) curves on the overall strain range. Moreover, an average error of \(5\,\%\) on the \(E_f\) versus \(\varepsilon _f\) curves within \(\varepsilon _f \in [0,0.19]\) for HPT and \(\varepsilon _f \in [0,0.15]\) for RTT fibrils (namely, before fibril failure) is reported. Error estimates are obtained normalizing with respect to the maximum experimental stress and tangent modulus, respectively, for the case under investigation.
Comparison between experimental data (dashed lines with symbols) by Svensson et al. (2013) and predictions obtained by means of proposed model for collagen fibrils in human-patellar tendon (HPT) and rat-tail tendon (RTT). Left Fibril tangent modulus \(E_f\) versus strain \(\varepsilon _f\). Right Fibril stress \(\sigma _f\) versus strain \(\varepsilon _f\) constitutive relationship. The values of model parameters are reported in Table 1
The evolution of RUS state variables (both strain and damage variables) and internal stresses are reported in Fig. 8 where strong nonlinearities clearly appear and couple each other. Addressing the HPT case, stiffness nonlinearities of fibril constituents are shown in Fig. 9 and compared with the overall fibril stiffness, in order to highlight the mechanisms which governs fibril behavior at different strain-range values. Moreover, Fig. 9 shows also the dependence on cross-link density of stresses and strains (both for constituent and for the overall fibril) at failure. This allows to highlight the dependence of fibril mechanical response on cross-link biochemistry.
Stresses (top) and state variables (bottom) in a representative unit system (RUS) of fibrils in human-patellar (left) and rat-tail (right) tendons versus fibril strain \(\varepsilon _f\). It is worth pointing out that \(\varepsilon _m^p=0\) (resp., \(\varepsilon _m^b=0\)) during the overall test for human-patellar (resp., rat-tail) fibrils, and thereby the curve is not clearly visible. The values of model parameters are reported in Table 1
Analysis of internal stiffness and damage mechanisms. Left Fibril (\(E_f\)), molecular (\(E_m\), \(E_m^e\) and \(E_m^s\)), and intermolecular (\(E_{CL}\), \(E_{c}\) and \(E_{w}\)) tangent moduli versus fibril strain \(\varepsilon _f\). Stiffness of internal constituents are scaled with the load-bearing-to-total area ratio \(\rho _a={\mathcal {A}}_c/{\mathcal {A}}_f\). Right Normalized stress and strain at failure versus \(\lambda _c^T\) for: covalent cross-links (\(s_c^{ID}/\sigma _{ID}\) and \(d_c/\varepsilon _{ID}\)); molecules (\(s_m/\sigma _{MR}\) and \(d_m^e/\varepsilon _{MR}\)); fibril (\(s_f/\sigma _{MR}\) and \(d_f/\varepsilon _{MR}\)). If not differently specified, the values of model parameters are reported in Table 1 and refer to fibrils in human-patellar tendon. By employing Eq. (28), values of damage thresholds correspond to \(\sigma _{ID}=427.6\) MPa, \(\varepsilon _{ID}=1.96\,\%\), \(\sigma _{MR}=654.6\) MPa, \(\varepsilon _{MR}=6.94\,\%\)
Finally, Fig. 10 presents a parametric analysis on the values of parameters governing the weak cross-link damage law and molecular relaxation time constant following the activation of MR.
Parametric analyses on values of model parameters. Top Fibril stress \(\sigma _f\) (left), tangent modulus \(E_f\) and damage variable \(\beta _2\) (right) versus strain \(\varepsilon _f\) for different values of parameters governing weak cross-links damage laws. Bottom Fibril stress \(\sigma _f\) (left), elastic \(\varepsilon _m^e\) and MR-inelastic \(\varepsilon _m^b\) molecular strains (right) versus strain \(\varepsilon _f\) for different values of \(\tau _o^{MR}\). If not differently specified, the values of model parameters are reported in Table 1 and refer to fibrils in human-patellar tendon
3.3 Application: loading–unloading behavior
Three cycles of a traction loading–unloading displacement-driven test at constant elongation rate \(|\dot{\ell }_f|=40\) \(\upmu \)m/s are addressed. The values employed for model parameters coincide with the ones of the HPT case. Accordingly, \(\ell _{f,o}=33.05\) \(\upmu \)m, \(2r_f=0.190\) \(\upmu \)m and other model parameters are listed in Table 1. Figure 11 shows the applied strain-law \(\varepsilon _f(\tau )\) and the underlying deformation mechanisms occurring at nanoscale. Moreover, the evolution of damage variables is reported together with the fibril stress normalized with respect to \(\sigma _f^{MR}\). As a result of nanoscale mechanisms, fibril constitutive response (reported in the same figure) is characterized by hysteresis loops associated with SP (namely, \(\beta _w\)) up to final failure due to MR (namely, \(\beta _m\)).
Three loading–unloading cycles of a displacement-driven traction test at \(|\dot{\ell }_f|=40\) \(\upmu \)m/s. Left Evolution of applied fibril strain \(\varepsilon _f\), nanoscale strains \(\varepsilon _c\), \(\varepsilon _w^e\), \(\varepsilon _w^p\), \(\varepsilon _m^e\), \(\varepsilon _m^p\),and \(\varepsilon _m^b\), normalized fibril stress \(\sigma _f/\sigma _f^{MR}\), and damage variables \(\beta _d\), \(\beta _w\) and \(\beta _m\) versus time \(\tau \). It is worth pointing out that \(\varepsilon _m^p=0\) during the overall test, and thereby the curve is not clearly visible. Right Fibril stress \(\sigma _f\) versus strain \(\varepsilon _f\). The values of model parameters are reported in Table 1 and refer to fibrils in human-patellar tendon (\(\ell _{f,o}=33.05\) \(\upmu \)m and \(2r_f=0.190\) \(\upmu \)m )
4 Discussions
Present paper addresses collagen fibril mechanics with a special insight on elasto-damage mechanisms occurring at nanoscale. The role of cross-links on determining the well-established variability in fibril mechanical response is clearly highlighted through a multiscale modeling approach.
\(\bullet \) Reproduction of MDS data
The comparison with MDS data by Buehler (2008) clearly shows that model predictions agree well with coarse-grained atomistic computations of short fibrils (see Fig. 5). In fact, several features of available data are reproduced: for low cross-link density, fibril strength increases with cross-link density and damage behavior shows large yielding regimes; for high covalent cross-link density, fibril damage behavior is characterized by a brittle-like rupture and the overall mechanical response has very low sensitiveness to cross-link density. Moreover, the nanoscale mechanisms governing the response in the different cases addressed by Buehler can be straightforwardly analyzed thanks to the proposed multiscale analytical technique (see Fig. 6). When only weak interactions among molecules are present, SP activates (associated with \(\beta _2\)) and fibril behavior is mainly governed by molecular elastic deformation \(\varepsilon _m^e\) in the elastic regime and by permanent molecular slippage \(\varepsilon _w^p\) in the yielding regime; with increasing covalent cross-link density, ID occurs at fibril failure (associated with \(\beta _1\) and \(\varepsilon _m^p\)); when the density of covalent cross-links is high, fibril damage is due to the rupture of covalent bonds inside collagen triple-helix structure (namely, activation of \(\beta _3\) and \(\varepsilon _m^b\)) and there is the transition from a yielding to a brittle behavior.
It is worth to point out the good quantitative agreement between obtained results and available data for the entire range of values considered for covalent cross-link density. Clearly, with respect to MDS, the proposed analytical technique reduces significantly the computational costs, resulting in the order of a minute for each simulation on a laptop with 2.3 GHz Quad-core Intel i7 processor.
With reference to the yielding behavior for cross-links deficient-fibrils, obtained results agree well with the initial softening-rate obtained by Buehler’s coarse-grained data. Nevertheless, it is worth remarking that, for \(\varpi =1\) (namely, \(\lambda _c^D=0\)), proposed model predicts a perfectly yielding behavior without softening (due to SP onset), in contrast to available data. The choice of not including softening for SP is based on non-coarse-grained MDS results on the assembly of two tropocollagen segments (Buehler 2006; Uzel and Buehler 2011; Marino and Vairo 2014a). Nevertheless, different yielding behaviors for SP could be addressed by modifying only weak cross-links interscale compatibility relationships [namely, Eq. (18)] and by introducing a possible molecular relaxation (similar to ID and MR).
Despite MDSs allow to have an insight on fibril elasto-damage internal mechanisms, attention should be paid when quantitative predictions are addressed. For instance, the highest value of fibril stiffness predicted from the coarse-grained MDSs by Buehler (\(\approx \)40 GPa, see Fig. 4) is indeed higher than the one obtained from experimental data from well-established literature (in the range \(\approx \)1–10 GPa, Cusack and Miller 1979; Shen et al. 2008; Svensson et al. 2013). This occurrence is due to entropic effects and large-strain rates, (Buehler 2008). Accordingly, a comparison between model and experimental results is necessary for proving model capabilities in providing quantitative predictions.
\(\bullet \) Reproduction of experimental data
Model results have been also compared with experimental data by Svensson et al. (2013), revealing model capability in reproducing the mechanics of collagen fibrils in both human-patellar and rat-tail tendons. Model fits available data in an excellent way for both specimen types (see Fig. 7). Available biochemical data on the values of cross-link density (Saito et al. 1997; Svensson et al. 2013), are straightly incorporated in the simulations thanks to the multiscale approach within which present model is developed.
Molecular biophysical properties are chosen the same among HPT and RTT cases because collagen polypeptide pattern does not differ among different species and genetic disorders are herein not addressed. Similarly, a unique set for covalent cross-link properties is chosen, except from the density of mature cross-links. On the other hand, differences in the biochemical environment of different specimens (and especially fibrils from human-patella and rat-tails) justify the choice of allowing for different intermolecular weak interaction behaviors (Grant et al. 2009; Gautieri et al. 2012). Accordingly, model parameters governing weak cross-links properties are set independently for HPT and RTT fibrils. Nevertheless, as a further proof of consistency, the best fitting is obtained employing values of model parameters with the same order of magnitude among the two cases, and sometimes their values coincide.
\(-\) Analysis of fibril mechanics
The model allows to predict and to put in evidence the high nonlinearities occurring in the internal structure of HPT and RTT fibrils (see Fig. 8). Moreover, the analysis of constituent’s stiffness is useful for determining the mechanisms which mainly affect fibril response (see Fig. 9). When in-series mechanisms are considered, the one associated with a lower stiffness dominates fibril behavior. On the other hand, addressing in-parallel mechanisms, the stiffer one is the most important. From this point of view, fibril mechanics can be described as the result of the complex interplay of a number of nonlinear stiffnesses: some of them are in-series (namely, molecular \(E_m\) and intermolecular \(E_{CL}\) but also entropic \(E_m^s\) and energetic \(E_m^h\)) and others in-parallel (covalent \(E_c\) and weak \(E_w\) cross-links).
For strains in the range \(\varepsilon _f \in [0,0.02]\), fibril mechanics is governed by both molecular elastic deformation \(\varepsilon _m^e\) and reversible molecular sliding associated with weak cross-links deformation \(\delta _w^e\). Moreover, average molecular strain \(\varepsilon _m\) within this interval is \(1.61\,\%\) for HPT and \(1.47\,\%\) for RTT while sliding mechanisms contribute to the overall fibril deformation for the remaining part (that is, \(0.39\,\%\) for HPT and \(0.53\,\%\) for RTT). This agrees in an excellent way with X-ray diffraction studies by Sasaki and Odajima (1996) who, in \(2\,\%\) of overall strain, report a contribution from molecular elongation equal to \(1.7\,\%\) and from molecular rearrangement mechanisms equal to \(0.3\,\%\) for fibrils in Achilles tendon. This evidence is a strong proof for the effectiveness of the proposed approach since it highlights model capability in capturing a-posteriori a main experimental observation on nanostructural intra-fibril rearrangement during tensile loads in tendons. Thereby, in this low-strain range, fibril mechanics is mainly affected by molecular deformation, as also confirmed by the evidence that \(E_m<<E_{CL}\). Moreover \(E_{CL} \approx E_w\) because the covalent cross-link is not yet activated (\(E_c \approx 0\)). Furthermore, it is worth highlighting that entropic mechanisms (namely, thermal fluctuations) have a strong influence on the overall molecular behavior and, since \(E_m^s<E_m^h\), they significantly reduce molecular modulus with respect to the value obtained if only energetic mechanisms were considered. This justifies the need of incorporating the complex and refined molecular behavior herein addressed [see Eq. (15)].
For \(\varepsilon _f \in [0.02,0.1]\), fibril deformation is mainly related to SP activation (\(E_{CL} \approx E_w << E_m\)). The load reaches the bond strength of weak intermolecular cross-links and the irreversible sliding between collagen molecules occurs at the expense of the elastic one and of molecular deformation (namely, \(\varepsilon _w^p\) increases, \(\varepsilon _w^e\) and \(\varepsilon _m\) tend to remain constant). Clearly, SP is associated with a significant reduction in fibril stiffness.
For \(\varepsilon _f > 0.1\), fibril stiffness increases again up to the final failure because of the activation of intermolecular covalent cross-links (\(E_{CL} \approx E_c>> E_w\)). This delayed response is related to the straightening of the hook-shaped telopeptide in which covalent cross-links form, herein modeled through Eq. (8b), (Marino and Vairo 2014a). The stiffening effect is significant for HPT and negligible for RTT, suggesting that it is mainly due to the presence of mature trivalent cross-links which are responsible for transverse connections between different microfibrils (Bailey et al. 1998; Orgel et al. 2006; Yang et al. 2012), and in fact it results \(k_c^T >> k_c^D\).
\(-\) Biochemistry-dependent fibril failure
Fibril failure behavior is significantly affected by the density of mature cross-links. In fact, HPT failure is due to the activation of damage parameter \(\beta _3\) associated with MR-inelastic strain \(\varepsilon _m^b\). On the other hand, RTT failure is related to the activation of \(\beta _1\) associated with ID-inelastic strain \(\varepsilon _m^p\). The reason underlying the transition from ID to MR is well highlighted by means of present model and by taking into account that the onset of ID is associated to the force transmitted through the covalent cross-links, representing a pulling out force for the polypeptide strand where the covalent cross-link is formed. The latter force has to be not confused with the one that intervenes in fibril equilibrium (25), namely \(\sigma _c\), which is an homogenous stress measure representing the average force transmitted through covalent cross-links in the entire fibril. On the other hand, ID-onset is related to a stress localization mechanism which is relevant when different molecules within the fibril have significant differences in covalent cross-links occurrence. This likely occurs especially for low cross-link density (thereby, when ID intervenes in fibril mechanics). The ID-related localization mechanism is herein described through the free-energy contribution \(\varPsi _m^{ID}\) in Eq. (12d), and it is driven by stress \(\sigma _c^{ID}\) in Eq. (29). This modeling choice means that it is herein assumed that ID-onset occurs in correspondence of a molecule where one mature (if \(\lambda _c^T\ne 0\)) and one immature (if \(\lambda _c^D\ne 0\)) covalent cross-links are present.
Addressing MR-onset, the free-energy contribution \(\varPsi _m^b\) in Eq. (16), and thereby molecular stress \(\sigma _m\) (resp., strain \(\varepsilon _m^e\)) drives this source of damage. In this case, no localization mechanism is modeled because the stress at constituent scale is assumed to be fairly constant within fibrils for high cross-link density (when MR is relevant for fibril mechanics).
The density of mature cross-links is a main factor for the internal damage mechanism which is activated at fibril failure. In case of low mature cross-link density (as in RTT), intermolecular sliding is high (ratio \(\varepsilon _c/\varepsilon _m^e\) is closer to the unit with respect to the HPT case in Fig. 8), and thereby, the force transmitted through the covalent cross-links is sufficient for the onset of ID before the one of MR. On the other hand, high density of mature cross-links in HPT is an obstacle for intermolecular sliding with respect to molecular elongation (\(\varepsilon _c\) remains small compared to \(\varepsilon _m^e\) in Fig. 8), and thereby, \(\sigma _m\) (resp., \(\varepsilon _m^e\)) reaches \(\sigma _{MR}\) (resp., \(\varepsilon _{MR}\)) before that \(\sigma _c^{ID}\) (resp., \(\varepsilon _c\)) reaches \(\sigma _{ID}\) (resp., \(\varepsilon _{ID}\)).
Moreover, fibril ultimate stress increases with cross-link density for low cross-link density, and then, it results constant (see Fig. 9). This evidence is related to the transition from ID to MR failure mechanisms, elucidated in the latter figure. Addressing the range of values dominated by ID, when \(\lambda _c^T\) increases, \(\sigma _c^{ID}\) reaches \(\sigma _{ID}\) for higher values of \(\varepsilon _m^e\) and \(\sigma _m\) (because the higher is the density of mature cross-links, the lower is molecular sway with respect to molecular elongation). Accordingly, since molecular and fibril stress differ only due to the ratio between fibril cross-sectional area \({\mathcal {A}}_f\) and load-bearing area \({\mathcal {A}}_c\), fibril strength increases with \(\lambda _c^T\). On the other hand, addressing the range of values dominated by MR, fibril ultimate stress is constant with \(\lambda _c^T\) and related to molecular ultimate stress, resulting \(s_f=\rho _a \sigma _{MR}\). In this case, no further strengthening mechanism is present. Nevertheless, it is worth pointing out that fibril strain at failure, \(d_f\), is much higher than molecular failure strain \(\varepsilon _{MR}\) because of intermolecular sliding favored by slip-pulse mechanisms (see Fig. 8). Moreover, \(d_f\) is fairly constant with \(\lambda _c^T\) with a peak in correspondence of the ID-MR transition.
\(-\) Analysis of modeling choices
Present work couples the model for intermolecular interactions by Marino and Vairo (2014a) with the one for the nonlinear elasto-damage mechanics of collagen molecules by Maceri et al. (2012b). Accordingly, the sensitivity and the physical meaning of most model parameters have been already discussed in the existing literature and they are here only recalled. Moreover, some refinements for both models have been included starting from the need of fitting experimental data on collagen fibrils. In detail, these are a refined nonlinear activation law for weak cross-links damage (Eq. (11)) and molecular relaxation mechanisms associated with MR (governed by parameter \(\tau _o^{MR}\)).
The parametric analysis on the values of parameters governing weak cross-links damage highlights the effectiveness of modeling choice. Firstly, it is worth pointing out that from Eq. (11): when \(\omega =0\) and \(v_w \rightarrow \infty \), then an unlimited damage rate is considered; when \(\omega =0\) and \(v_w<+\infty \), then damage rate is limited by the constant \(v_w\). Figure 10 shows that the choice of either an unlimited or a constant damage rate significantly affects the obtained fibril constitutive response but also that, for both choices, SP activation couples with the straightening of the cross-linked telopeptide determining a sharp transition between the decreasing and the increasing branch of \(E_f\). This feature does not agree with experimental data that clearly indicate a smooth variation of \(E_f\). On the other hand, the smoothness of \(E_f\) is recovered considering a nonlinear damage activation law governed by Eq. (11) (with \(\omega \ne 0\) and \(v_w<+\infty \)), and it is associated with the convexity of \(\beta _2\) with \(\varepsilon _f\).
Figure 10 shows also that parameter \(\tau _o^{MR}\) governs fibril brittleness associated with MR. As a general rule of thumb, lower is the value of molecular relaxation decay time constants, higher is the stress decrease rate at failure, and thereby the mechanical response appears more brittle. Thereby, the transition from a yielding softening to a brittle behavior resides only in the difference between the associated molecular relaxation time constants. In this framework, in agreement with MDS data (Buehler 2008; Uzel and Buehler 2011), it is interesting to note that the fitting of experimental data corresponds to values \(\tau _o^{ID}>\tau _o^{MR}\), although the typical elongation rates employed for fibril uniaxial traction do not allow to appreciate significant differences in the stress decay rate at fibril failure (Marino and Vairo 2014a).
\(\bullet \) Reproduction of fibril loading–unloading behavior
Finally, the model is applied for predicting fibril response to a loading–unloading test (see Fig. 11). The obtained hysteresis loops are fully in agreement with experimental evidence (Shen et al. 2008; Svensson et al. 2010). Thanks to the adopted multiscale rationale, these hysteresis loops are herein related to nanoscale deformation mechanisms and are not described employing phenomenological viscous laws. In fact, the residual strains appearing at the end of each cycle are a straight consequence of the reversibility assumption on intermolecular weak bonds, associated with the activation and deactivation of SP.
\(\bullet \) Model strengths, limitations and future directions
In summary, accounting for nanoscale mechanisms, the model provides a special insight on the correlation between the histochemical features of collagen fibrils and their mechanics in terms of stiffness, nonlinearities, damage behavior and strength. A quantitative correlation between mature cross-link density and mechanical response is effectively obtained. Elasto-damage molecular and intermolecular mechanisms are explicitly modeled, providing a rational explanation to the nonlinear and viscoelastic mechanics of collagen fibrils.
Despite collagen molecules and fibrils are three-dimensional structures (Orgel et al. 2006; Pradhan et al. 2011), the model is developed within a mono-dimensional framework in order to describe several nanoscale mechanisms in a computational feasible way. Thereby, if coupled with multiscale homogenization methods, present approach allows for an effective upscaling of complex molecular and intermolecular effects in three-dimensional macroscale tissue models. This rationale has been already employed for the description of soft connective tissues (Maceri et al. 2010, 2012a, 2013; Marino and Vairo 2013, 2014b, c), but it is likely suitable also for homogenized models of bones (Hellmich et al. 2004; Grimal et al. 2011; Morin and Hellmich 2013; Morin et al. 2013; Morin and Hellmich 2014).
The model presents some limitations that will be faced in future works. Firstly, the mechanical behavior of non-enzymatic cross-links (namely, advanced glycation end products) is herein not addressed. Moreover, the best fitting of experimental data is obtained when a significant difference in the stiffness of mature (between molecules in different microfibrils/fibrils) and immature (between molecules within the same microfibril) cross-links is considered, resulting \(k_c^T/k_c^D\) \(\approx 20\). This outcome is likely related to a geometric effect: the assembly of cross-linked microfibrils (helically wounded each other and whose relative sliding is prevented by the presence of intermicrofibrils cross-links) is stiffer than the assembly of non-cross-linked microfibrils (which are free to slide each other due to the absence of intermicrofibrils cross-links). This three-dimensional geometric effect is effectively described in the present mono-dimensional framework by considering a higher stiffness for mature cross-links, but different strategies will be also investigated in future works, by explicitly addressing the microfibril sliding in the formulation of the model.
Furthermore, the model has been validated addressing only traction of fibrils. The analysis of their compressive behavior requires more investigations. Moreover, only two fibrils are employed for comparison between model outcomes and experimental data. Future works will address an higher number of samples. In this framework, a refined automatic procedure for parameter setting will be conceived and implemented. This will allow to obtain physiological ranges for biophysical and biochemical parameters, straightforwardly derived from mechanical measures. Finally, a quantitative correlation between the values of model parameters and more measurable biophysical and biochemical parameters (such as hydration state, pH, ionic environment and enzymatic activity) will be investigated.
In general, thanks to the multiscale rationale herein employed, new or different computational/experimental evidence at nanoscale could be straightforwardly incorporated in present model without affecting its general setting. For instance, interscale compatibility relationships or nanoscale constitutive laws only could be modified in order to obtain refined responses of fibril mechanics.
5 Conclusions
A great modeling challenge in biomechanics is to identify and to upscale molecular and intermolecular mechanisms that are likely to have a significant macroscopic influence. Addressing connective tissues, elasto-damage effects of collagen molecules and their interaction properties within fibrillar structures have to be analyzed across scales up to the continuum level.
In this study, an analytical model of collagen fibril mechanics is developed. The model enriches the model by Marino and Vairo (2014a) that allowed to describe: permanent molecular sliding associated to slip-pulse mechanisms; the delayed activation of the hook-shaped telopeptide in which the covalent cross-links form; interstrand delamination mechanisms associated with the rupture of intramolecular weak bonds. The model has been generalized by taking into account: different responses for mature and immature cross-links; molecular elastic nonlinearities associated with thermal fluctuations, helix uncoiling and stretching of molecular backbone; damage of intramolecular covalent bonds; refined nonlinear slip-pulse activation.
The proposed analytical approach allows to gain a better comprehension on the nanoscale intrafibrillar mechanisms where experimental techniques and atomistic computations reach their limits. Indeed, model effectively predicts how fibril nanostructure affects the nonlinear coupling of the internal mechanisms characterizing fibril mechanics and it allows to run simulations with a very low computational cost. Accordingly, present model might be effectively employed within a structural multiscale approach for developing refined macroscale models of biological tissues (Maceri et al. 2010, 2012a, 2013; Marino and Vairo 2013, 2014b, c). Thereby, it represents a powerful tool for investigating on the structure mechanics dependence in biological tissues. This opens toward the analysis of histochemical alterations associated with pathological tissue/organ behavior, as well as the investigation on the effectiveness of pharmacological treatments favoring the homeostatic histochemical condition.
References
Andreassen T, Seyer-Hansen K, Bailey A (1981) Thermal stability, mechanical properties and reducible cross-links of rat tail tendon in experimental diabetes. Biochim Biophys Acta Gen Subj 677:313–317
Avery NC, Bailey AJ (2008) Restraining cross-links responsible for the mechanical properties of collagen fibers: natural and artificial. In: Fratzl P (ed) Collagen: structure and mechanics. Springer, Berlin, pp 81–110
Bailey AJ, Paul RG, Knott L (1998) Mechanisms of maturation and ageing of collagen. Mech Ageing Dev 106:1–56
Bailey AJ (2001) Molecular mechanisms of ageing in connective tissues. Mech Ageing Dev 122:735–755
Bozec L, Horton M (2005) Topography and mechanical properties of single molecules of type I collagen using atomic force microscopy. Biophys J 88:4223–4231
Brüel A, Ørtoft G, Oxlund H (1998) Inhibition of cross-links in collagen is associated with reduced stiffness of the aorta in young rats. Atherosclerosis 140:135–145
Buehler MJ (2006) Atomistic and continuum modeling of mechanical properties of collagen: elasticity, fracture, and self-assembly. J Mater Res 21:1947–1961
Buehler MJ (2008) Nanomechanics of collagen fibrils under varying cross-link densities: atomistic and continuum studies. J Mech Behav Biomed Mater 1:59–67
Buehler MJ, Wong SY (2007) Entropic elasticity controls nanomechanics of single tropocollagen molecules. Biophys J 93:37–43
Carmo M, Colombo L, Bruno A, Corsi FRM, Roncoroni L, Cuttin MS, Radice F, Mussini E, Settembrini PG (2002) Alteration of elastin, collagen and their cross-links in abdominal aortic aneurysms. Eur J Vasc Endovasc Surg 23:543–549
Cusack S, Miller A (1979) Determination of the elastic constants of collagen by Brillouin light scattering. J Mol Biol 135:39–51
DeGroot J, Verzijl N, Wenting-Van Wijk MJG, Jacobs KMG, Van El B, Van Roermund PM, Bank RA, Bijlsma JWJ, TeKoppele JM, Lafeber FPJG (2004) Accumulation of advanced glycation end products as a molecular mechanism for aging as a risk factor in osteoarthritis. Arthritis Rheum 50:1207–1215
Depalle B, Qin Z, Shefelbine SJ, Buehler MJ (2014) Influence of cross-link structure, density and mechanical properties in the mesoscale deformation mechanisms of collagen fibrils. J Mech Behav Biomed Mater. doi:10.1016/j.jmbbm.2014.07.008
Eyre DR, Wu JJ (2005) Collagen cross-links. In: Brinckmann J, Notbohm H, Müller PK (ed) Collagen: primer in structure, processing and assembly. Topics in current chemistry, vol 247. Springer, Berlin, pp 207–229
Eyre DR, Koob TJ, Van Ness KP (1984) Quantitation of hydroxypyridinium crosslinks in collagen by high-performance liquid chromatography. Anal Biochem 137:380–388
Eyre DR, Weis MA, Wu JJ (2008) Advances in collagen cross-link analysis. Methods 45:65–74
Fessel G, Gerber C, Snedeker JG (2012) Potential of collagen cross-linking therapies to mediate tendon mechanical properties. J Shoulder Elbow Surg 21:209–217
Fratzl P (2008) Collagen: structure and mechanics. Springer, Berlin
Frémond M (2002) Non-smooth thermomechanics. Springer, Berlin
Gautieri A, Pate MI, Vesentini S, Redaelli A, Buehler MJ (2012) Hydration and distance dependence of intermolecular shearing between collagen molecules in a model microfibril. J Biomech 45:2079–2083
Gautieri A, Redaelli A, Buehler MJ, Vesentini S (2014) Age-and diabetes-related non enzymatic cross links in collagen fibrils: candidate amino acids involved in Advanced Glycation End-products. Matrix Biol 34:89–95
Germain P, Nguyen QS, Suquet P (1983) Continuum thermodynamics. J Appl Mech 50:1010–1020
Grant CA, Brockwell DJ, Radford SE, Thomson NH (2009) Tuning the elastic modulus of hydrated collagen fibrils. Biophys J 97:2985–2992
Grimal Q, Rus G, Parnell WJ, Laugier P (2011) A two-parameter model of the effective elastic tensor for cortical bone. J Biomech 44:1621–1625
Hain M, Wriggers P (2008) Numerical homogenization of hardened cement paste. Comput Mech 42:197–212
Hellmich C, Barthélémy JF, Dormieux L (2004) Mineral-collagen interactions in elasticity of bone ultrastructure–a continuum micromechanics approach. Eur J Mech A Solids 23:783–810
Holmes DF, Graham HK, Trotter JA, Kadler KE (2001) STEM/TEM studies of collagen fibril assembly. Micron 32:273–285
Holzapfel GA, Ogden RW (2010) On the bending and stretching elasticity of biopolymer filaments. J Elast 104:319–342
Klepeis JL, Lindorff-Larsen K, Dror RO, Shaw DE (2009) Long-timescale molecular dynamics simulations of protein structure and function. Curr Opin Chem Biol 19:120–127
Kouznetsova V, Brekelmans WAM, Baaijens FPT (2001) An approach to micro-macro modeling of heterogenous materials. Comput Mech 27:37–48
Lehmann E, Loehnert S, Wriggers P (2012) Computational homogenization of polycrystalline elastoplastic microstructures at finite deformation. Tech Mech 37:369–379
Li Y, Fessel G, Georgiadis M, Snedker JG (2013) Advanced glycation end-products diminish tendon collagen fiber sliding. Matrix Biol 32:169–177
Maceri F, Marino M, Vairo G (2010) A unified multiscale mechanical model for soft collagenous tissues with regular fiber arrangement. J Biomech 43:355–363
Maceri F, Marino M, Vairo G (2012a) An insight on multiscale tendon modeling in muscle-tendon integrated behavior. Biomech Model Mechanobiol 11:505–517
Maceri F, Marino M, Vairo G (2012b) Elasto-damage modeling of biopolymer molecules response. CMES 87:461–481
Maceri F, Marino M, Vairo G (2013) Age-dependent arterial mechanics via a multiscale elastic approach. Int J Comput Methods Eng Sci Mech 14:141–151
Marino M (2013) Pseudopotentials and thermomechanical response of materials and structures: a convex analysis approach. Dissertation, Department of Civil Engineering and Computer Science, University of Rome “Tor Vergata”
Marino M, Vairo G (2013) Multiscale elastic models of collagen bio-structures: from cross-linked molecules to soft tissues. In: Gefen A (ed) Computational modelling of biomechanics and biotribology in the musculoskeletal system. Stud. Mechanobiol. Tissue Eng Biomater, vol 14. Springer, Berlin, pp 73–102
Marino M, Vairo G (2014a) Influence of inter-molecular interactions on the elasto-damage mechanics of collagen fibrils: a bottom-up approach towards macroscopic tissue modeling. J Mech Phys Solids 73:38–54
Marino M, Vairo G (2014b) Stress and strain localization in stretched collagenous tissues via a multiscale modelling approach. Comput Methods Biomech Biomed Eng 17:11–30
Marino M, Vairo G (2014c) Computational modeling of soft tissues and ligaments. In: Jin Z (ed) Computational modelling of biomechanics and biotribology in the musculoskeletal system. Woodhead Publ Ser Biomat, vol 81. Woodhead Publishing Limited, Cambridge, pp 141–172
Marko JF, Siggia ED (1995) Stretching DNA. Macromolecules 28:8759–8770
Miles CA, Bailey AJ (2001) Thermally labile domains in the collagen molecule. Micron 32:325–332
Misof K, Rapp G, Fratzl P (1997) A new molecular model for collagen elasticity based on synchrotron X-ray scattering evidence. Biophys J 72:1376–1381
Morin C, Hellmich C, Henits P (2013) Fibrillar structure and elasticity of hydrating collagen: a quantitative multiscale approach. J Theor Biol 317:384–393
Morin C, Hellmich C (2013) Mineralization-driven bone tissue evolution follows from fluid-to-solid phase transformations in closed thermodynamic systems. J Theor Biol 335:185–197
Morin C, Hellmich C (2014) A multiscale poromicromechanical approach to wave propagation and attenuation in bone. Ultrasonics 54:1251–1269
Orgel J, Wess TJ, Miller A (2000) The in situ conformation and axial location of the intermolecular cross-linked non-helical telopeptides of type I collagen. Structure 8:137–142
Orgel JPRPO, Irving TC, Miller A, Wess T (2006) Microfibrillar structure of type I collagen in situ. Proc Nat Acad Sci USA 103:9001–9005
Pradhan SM, Katti DR, Katti KS (2011) Steered molecular dynamics study of mechanical response of full length and short collagen molecules. J Nanomech Micromech 1:104–110
Reiser K, McCormick RJ, Rucker RB (1996) Enzymatic and nonenzymatic cross-linking of collagen and elastin. FASEB J 6:2439–2449
Saito M, Marumo K, Fujii K, Ishioka N (1997) Single-column high-performance liquid chromatographic-fluorescence detection of immature, mature, and senescent cross-links of collagen. Anal Biochem 253:26–32
Sasaki N, Odajima S (1996) Elongation mechanism of collagen fibrils and force-strain relations of tendon at each level of structural hierarchy. J Biomech 29:1131–1136
Shen ZL, Dodge MR, Kahn H, Ballarini R, Eppell SJ (2008) Stress-strain experiments on individual collagen fibrils. Biophys J 95:3956–3963
Svensson RB, Hassenkam T, Hansen P, Magnusson SP (2010) Viscoelastic behavior of discrete human collagen fibrils. J Mech Behav Biomed Mater 3:112–115
Svensson RB, Mulder H, Kovanen V, Magnusson SP (2013) Fracture mechanics of collagen fibrils: influence of natural cross-links. Biophys J 104:2476–2484
Uzel S, Buehler MJ (2011) Molecular structure, mechanical behavior and failure mechanism of the C-terminal cross-link domain in type I collagen. J Mech Behav Biomed Mater 4:153–161
Verzijl N, DeGroot J, Ben ZC, Brau-Benjamin O, Maroudas A, Bank RA, Mizrahi J, Schalkwijk CG, Thorpe SR, Baynes JW, Bijlsma JWJ, Lafeber FPJG, TeKoppele JM (2002) Crosslinking by advanced glycation end products increases the stiffness of the collagen network in human articular cartilage: a possible mechanism through which age is a risk factor for osteoarthritis. Arthritis Rheum 46:114–123
Wess TJ (2008) Collagen fibrillar structure and hierarchies. In: Fratzl P (ed) Collagen: structure and mechanics. Springer, Berlin, pp 49–80
Yang L, van der Werf KO, Dijkstra PJ, Feijen J, Bennink ML (2012) Micromechanical analysis of native and cross-linked collagen type I fibrils supports the existence of microfibrils. J Mech Behav Biomed Mater 6:148–158
Zimmermann E, Schaible E, Bale H, Barth HD, Tang SY, Reichert P, Busse B, Alliston T, Ager JW III, Ritchie RO (2011) Age-related changes in the plasticity and toughness of human cortical bone at multiple length scales. Proc Nat Acad Sci USA 108:14416–14421
Acknowledgments
The author gratefully acknowledges Prof. Peter Wriggers, Prof. Franco Maceri and Prof. Giuseppe Vairo for fruitful discussions on the topics addressed by present paper. Present study was supported by the Italian Minister of University and Research MIUR (PRIN, Grant Number F11J12000210001) and by a research fellowship for postdoctoral researchers of the Alexander von Humboldt Foundation.
Author information
Authors and Affiliations
Corresponding author
Appendix: Basics of convex analysis
Appendix: Basics of convex analysis
In agreement with classical arguments of convex analysis, introduce the set \(\bar{\mathbb {R}}={\mathbb {R}} \cup \{+ \infty \}\) where the regular addition is completed by the rules: \(a+(+\infty )=+\infty \) (\(\forall \; a \in {\mathbb {R}}\)) and \(+\infty +(+\infty )=+\infty \), while multiplication by strictly positive numbers is completed by \(a \times (+\infty )=+\infty \) (\(\forall \; a \in {\mathbb {R}}^+\)), (Frémond 2002). In this framework, introduce the indicator functions
with their subdifferential sets being
Rights and permissions
About this article
Cite this article
Marino, M. Molecular and intermolecular effects in collagen fibril mechanics: a multiscale analytical model compared with atomistic and experimental studies. Biomech Model Mechanobiol 15, 133–154 (2016). https://doi.org/10.1007/s10237-015-0707-8
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10237-015-0707-8