Downstream rivals’ competition, bargaining, and welfare

Abstract

We analyze the effect of downstream competition (or cooperation) in the presence of decentralized bargaining between two downstream firms and an upstream monopolist over a two-part tariff input price. The major findings are as follows: (i) the relationship between the profits of the upstream monopolist (resp. the downstream firms) and the intensity of competition is U-shaped (resp. inverted U-shaped), irrespective of the competition modes in the downstream product market; (ii) if the intensity of competition is sufficiently high, the downstream firms’ profits are higher under Bertrand competition, whereas if the intensity of competition is sufficiently low, the downstream firms’ profits are higher under Cournot competition; and (iii) a market under Cournot competition is more efficient than a market under Bertrand competition, in the sense that both consumer surplus and social welfare are higher in the case of the former.

1 Introduction

In their seminal work, Singh and Vives (1984) argued that in the presence of perfectly competitive markets, for the input of production, a firm’s dominant strategy is a quantity (price) contract if the goods are substitutes (complements), and they demonstrated that social welfare and consumer surpluses are higher under Bertrand competition with a linear demand structure. This traditional view has been subsequently challenged by a number of theoretical studies. For example, Vives (1985) showed that when the demand structure is symmetric and both Bertrand and Cournot equilibria are unique, then prices and profits are higher and quantities are smaller in Cournot competition than in Bertrand competition. Arya et al. (2008) found that when a retail competitor secures an essential input from a vertically integrated provider of substitute goods, Bertrand competition can produce higher retail prices, higher industry profit, and lower levels of consumer surplus and total surplus than Cournot competition. Mukherjee et al. (2012) showed that in a vertical structure with a profit-maximizing upstream firm, whether the profits in the downstream market are higher under Bertrand competition or under Cournot competition depends on the differences in technology between the downstream firms and on the pricing strategy (namely, uniform pricing or price discrimination) of the upstream firm—social welfare is always higher under Bertrand competition than under Cournot competition. Considering two-part tariff vertical contracts, Alipranti et al. (2014) and Basak and Wang (2016) found that whether downstream Cournot or Bertrand competition yields a dominant strategy in a vertically related market with an upstream monopoly depends on whether it is bargaining via a decentralized or centralized two-part tariff. In sum, the first line of research has mainly explored the links between the profits of firms and competition modes.

The second line of research has examined the links between the factors underlying the market structure and competition modes. Miller and Pazgal (2001) demonstrated that the differences between the outcomes of price and quantity competition dissolve if managerial incentive schemes are linear combinations of the firm’s own profit and its rival firm’s profit. Symeonidis (2010) found that when competition is in quantities (prices), a merger between downstream firms may raise (lower) consumer surplus and overall welfare. Pal (2015) examined the effects of network externalities on the endogenous choices of downstream competition modes in a vertically related market and found that in the presence of positive (negative) network externalities, Bertrand (Cournot) competition yields lower prices and profits and higher quantities, consumer surpluses, and welfare than Cournot (Bertrand) competition.

The studies of Symeonidis (2008), Alipranti et al. (2014), Basak and Wang (2016), and Vetter (2017) are similar to ours. Symeonidis (2008) has explored the effects of downstream competition in a vertically related market and found that when bargaining is over a two-part tariff, a decrease in the intensity of competition reduces downstream firms’ profits and upstream firms’ utility but raises consumer surplus and overall welfare. However, the competition intensity in Symeonidis’ paper was restricted to the competitiveness between monopoly and duopoly levels and did not consider the competitiveness between duopoly and perfectly competitive levels. Examining these issues in detail yields results that differ from those of Symeonidis (2008).

Although Alipranti et al. (2014) have shown that Cournot competition is more efficient than Bertrand competition in a vertically related market with an upstream monopoly and decentralized bargaining via two-part tariffs, when Basak and Wang (2016) considered the centralized two-part tariff bargaining with an upstream input supplier they revealed that choosing Bertrand competition is the dominant strategy taken by downstream firms irrespective of whether the goods are substitutes or complements. Assuming that downstream firms produce under a soft capacity restriction, Vetter (2017) indicated that the balance between price and quantity in downstream firms’ strategies is endogenous and the monopolist’s charge for input co-determines downstream market conduct. Different from Vetter (2017), who assumed that the upstream monopolist decides the price of the input, in this paper, the upstream monopolist participates in decentralized bargaining with downstream firms to determine the terms of the two-part tariff contracts.

Chen (2017) examined the welfare implications of input price discrimination in a vertically related market when the downstream duopolists produce quality-differentiated products at different marginal costs. This revealed that in a linear pricing regime, discriminatory pricing by an upstream monopolist does not affect aggregate output, although it does cause ambiguous welfare effects, depending on the sizes of the downstream quality gap and cost difference. Hence, discriminatory pricing by an upstream monopolist in the linear pricing regime can be socially desirable, because the more efficient downstream firm can sell relatively more output. However, in the two-part tariff regime, a ban on price discrimination may increase the aggregate output and social welfare. The papers cited above did not consider the effects of competition intensity in the downstream market with differentiated products.¹ Therefore, our results differ in one significant way, i.e., the competition mode (Cournot or Bertrand) that yields higher profits for the downstream firms crucially depends on the intensity of competition (or cooperation) in the downstream market.

The current paper extends the existing literature by comparing the effects of competition (or cooperation) intensity in the downstream market on the downstream firms’ profits under different competition modes in a vertically related market with an upstream monopolist.² With respect to the market structure, one typical example is the automobile industry, where the number of brands is few, the number of independent car manufacturing firms is even fewer, and virtually every important electrical component originates from Bosch, a leading global supplier of automotive and industrial technology. Likewise, there are relatively few laptop manufacturers and Intel seems to have monopoly power in the market on the semiconductor chips used in laptop production.

Our model is not restricted to the effects of collusion (or merger) (see Ziss 1995; Fershtman and Pakes 2000)³ but analyzes more generally the effects of changes in the competition intensity in the downstream market. Similar to Matsumura and Matsushima (2012), Matsumura et al. (2013), Matsumura and Okamura (2015), and Hirose and Matsumura (2016), we capture the intensity of rivalry in the downstream duopolistic market using a continuous variable that contains three standard models: monopoly, duopoly, and a perfectly competitive market. Specifically, in our benchmark model, two downstream firms compete in a horizontally differentiated product market. Prior to that, each of the two downstream firms separately bargains with the upstream monopolist over a two-part tariff. Similar to Milliou and Petrakis (2007), Milliou and Pavlou (2013), Alipranti et al. (2015), and Li and Shuai (2017), we assume that the per-unit input price can be negative, that is, that the upstream firms subsidize their downstream customers.⁴ We find that the relationship between the profits of the upstream monopolist (the downstream firms) and the intensity of competition is U-shaped (inverted U-shaped), irrespective of the competition modes in the downstream product market. As for the profit comparison of downstream firms under different competition modes, if the intensity of competition is sufficiently high, the downstream firms’ profits are higher under Bertrand competition. If the intensity of competition is sufficiently low, the downstream firms’ profits are higher under Cournot competition. Moreover, consumer surplus and social welfare are higher under Cournot competition than under Bertrand competition.

This paper is structured as follows. Section 2 formulates our basic model. In Sect. 3, we present the equilibrium results under Cournot and Bertrand competition. In Sect. 4, we compare the equilibrium outcomes under Cournot and Bertrand competition in the downstream market. Section 5 concludes this paper.

2 The model

Consider a vertical market structure where an upstream monopolist $U$ supplies a homogeneous intermediate input to two downstream firms—denoted by $D_{1}$ and $D_{2}$, respectively—through two-part tariff contracts involving an upfront fixed fee and a per-unit price.⁵ The two downstream firms sell differentiated products in the final goods market. We denote the output of downstream firm $i$ by $q_{i} (i = 1,2)$. We assume that one unit of input is required to produce one unit of output, and $D_{1}$ and $D_{2}$ can convert the inputs into the final goods without incurring any further cost.

The underlying utility function of the representative consumer is assumed to be $\Lambda (q_{1} ,q_{2} ) = (q_{1} + q_{2} ) - \frac{{q_{2}^{2} }}{2} - \frac{{q_{1}^{2} }}{2} - \gamma q_{1} q_{2} .$

Utility maximization yields the following inverse demand function for downstream firm i:$p_{i} = 1 - q_{i} - \gamma q_{j}$, where $i = 1,2,$ and $\gamma \in (0,1)$ measures the degree of product differentiation; a lower value of $\gamma$ indicates a higher degree of product differentiation, $\gamma = 1$ implies that the goods of the two downstream firms are perfect substitutes, and $\gamma = 0$ implies that their products are completely different.

We model the bargaining between the upstream monopolist and two downstream firms by invoking the Nash equilibrium of simultaneous generalized Nash bargaining problems, in which the bargaining power of $U$ and $D_{i}$ is given by $\beta$ and $( 1- \beta )$, respectively, with $\beta \in \left( {0,{ 1}} \right]$. The intensity of competition (or manager cooperation) between downstream firms is modeled as follows: we allow for a continuum of degrees of competition (or manager cooperation) by assuming that at the second stage, each firm maximizes the sum of its own profits and a fraction λ of the profits of its rival, i.e.,$v_{i} = \pi_{i} + \lambda \pi_{j} (i,j = 1,2 \, i \ne j)$. The parameter $\lambda$, $\lambda \in ( - 1,1)$, is an inverse measure of the intensity of competition.⁶ In a symmetric situation, the equilibrium outcome for $\lambda = - 1$ is identical to that in a perfectly competitive market. By construction, the model is reduced to the standard duopoly case where $\lambda = 0$. If $\lambda = 1$, each firm chooses an output to maximize joint profits, and thus the outcome corresponds to that of collusion or monopoly. Hence, $\lambda \in (0,1)$ implies an intermediate competitiveness between monopoly and duopoly levels, and $\lambda \in ( - 1,0)$ implies an intermediate competitiveness between duopoly and perfectly competitive levels. Thus, a smaller $\lambda$ indicates a more competitive market. The model enables us to treat competitiveness as a continuous variable, and it contains three standard models as special cases: monopoly, duopoly, and a perfectly competitive market.⁷

We consider a two-stage game. In the first stage, the upstream firm $U$ is involved in decentralized bargaining with downstream firms to determine the terms of the two-part tariff contracts involving an upfront fixed fee, $F_{i}$, and a per-unit price, $\omega_{i}$, where $i = { 1},{ 2}$. In the second stage, $D_{1}$ and $D_{2}$ choose their quantities (Cournot competition) or their prices (Bertrand competition) simultaneously, given the result of stage 1. We solve the game through backward induction.

To ensure that the upstream monopolist’s and downstream firms’ profits are always non-negative, we adopt the following assumption in the subsequent analysis:

Assumption 1

Either (a) the product substitutability between the downstream firms cannot be too high, i.e., $0 < \gamma \le \bar{\gamma }(\beta ) = 1 - \frac{1}{1 - \beta } + \sqrt {\frac{\beta }{{\left( {1 - \beta } \right)^{2} }}} ( < 1)$; or (b) the product substitutability between the downstream firms is sufficiently high, but the downstream market cannot be too non-competitive, i.e., $\gamma > \bar{\gamma }$ and $- 1 < \lambda < \bar{\lambda }(\beta ,\gamma ) = \sqrt {\frac{{\beta \left( {8 + \beta } \right) + 2\left( {1 - \beta } \right)\beta \gamma + \left( {1 - \beta } \right)^{2} \gamma^{2} }}{{\gamma^{2} \left( {2 + \gamma - \beta \gamma } \right)^{2} }}} - \frac{{\beta + \gamma + \beta \gamma + \gamma^{2} - \beta \gamma^{2} }}{\gamma (2 + (1 - \beta )\gamma )}( < 1)$.

3 Equilibrium analysis

We start by solving the last stage of the game, first under Cournot competition and then under Bertrand competition.

3.1 Cournot competition

In stage 2, the downstream firm $D_{i} (i = 1,2)$ chooses its output to maximize $v_{i}$:

$$\begin{aligned} & \mathop {Max}\limits_{{q_{i} }} v_{i} = \pi_{i} + \lambda \pi_{j} = [(1 - q_{i} - \gamma q_{j} )q_{i} - (\omega_{i} q_{i} + F_{i} )]\\ &\quad + \lambda \left[ {(1 - q_{j} - \gamma q_{i} )q_{j} - (\omega_{j} q_{j} + F_{j} )} \right],\quad (i,j = 1,2 \, i \ne j).\end{aligned} $$

(1)

Solving the first-order conditions, we obtain the equilibrium output of $D_{i} (i = 1,2)$:

$$ q_{i}^{C} = \frac{{2 - \gamma - \gamma \lambda - 2\omega_{i} + \gamma (1 + \lambda )\omega_{j} }}{{4 - \gamma^{2} (1 + \lambda )^{2} }} , (i,j{ = 1,2 }i \ne j ). $$

(2)

In stage 1, $U$, the upstream monopolist and $D_{i} (i = 1,2)$ adopt the terms of the two-part tariff contract by maximizing the following generalized Nash bargaining expression:

$$ \mathop {Max}\limits_{{F_{i} ,\omega_{i} }} [\Pi_{U}^{{}} - \Pi_{{U_{j} }}^{{}} ]^{\beta } [\pi_{i}^{{}} ]^{1 - \beta } ,\, (i \, ,j = 1, 2i \ne j) $$

(3)

where $\Pi_{U}^{{}} = \mathop \sum \nolimits_{d = 1}^{2} \left( {q_{d} \omega_{d} + F_{d} } \right)$ denotes the profits of the upstream monopolist when there are two firms in the downstream market, and $\pi_{i}^{{}} = (p_{i}^{{}} - \omega_{i}^{{}} )q_{i}^{{}} - F_{i}^{{}}$ denotes the profits of $D_{i} .$$\Pi_{{U_{j} }}^{{}} = \omega_{j} \frac{{(1 - \omega_{j} )}}{2} + F_{j}$ is $U'{\text{s}}$ profit when its negotiations with $D_{i}$ break down and $D_{j}$ acts as a monopolist in the downstream market, i.e., it produces the monopoly quantity.

According to (3), the equilibrium per-unit input price and the equilibrium fixed fees are as follows:

$$\begin{aligned} \omega_{i}^{C} &= \frac{{\gamma [\gamma + \left( {2 + \gamma } \right)\lambda ]}}{{2\gamma \lambda + \gamma^{2} (1 + \lambda )(2 + \lambda ) - 4}},\\ F_{i}^{C} &= \frac{{(2 - \gamma - \gamma \lambda )(\beta (2 - \gamma - \gamma \lambda )(2 - \gamma^{2} (1 + \lambda )) - \gamma^{2} (1 + \lambda )(\gamma + (2 + \gamma )\lambda ))}}{{(4 - 2\gamma \lambda - \gamma^{2} (1 + \lambda )(2 + \lambda ))^{2} }},\\ &\quad (i,j{ = 1,2 }i \ne j ).\end{aligned} $$

(4)

According to (2) and (4), we can obtain the equilibrium output and the equilibrium price of $D_{i} (i = 1,2)$:

$$\begin{aligned} & q_{i}^{C} = \frac{2 - \gamma - \gamma \lambda }{{4 - 2\gamma \lambda - \gamma^{2} (1 + \lambda )(2 + \lambda )}},\, p_{i}^{C} = \frac{(1 - \gamma - \gamma \lambda )(2 + \gamma + \gamma \lambda )}{{4 - 2\gamma \lambda - \gamma^{2} (1 + \lambda )(2 + \lambda )}}, \\ & \quad (i,j{ = 1,2 }i \ne j )\end{aligned} $$

(5)

The profits of the upstream monopolist and the downstream firms are

$$ \begin{aligned} \Pi_{U}^{C} & = \frac{{(2 - \gamma - \gamma \lambda )(\beta (2 - \gamma - \gamma \lambda )(2 - \gamma^{2} (1 + \lambda )) - \gamma^{2} (1 + \lambda )(\gamma + (2 + \gamma )\lambda ))}}{{[4 - 2\gamma \lambda - \gamma^{2} (1 + \lambda )(2 + \lambda )]^{2} }}, \\ \pi_{i}^{C} & = \frac{{\left( {1 - \beta } \right)\left( {2 - \gamma - \gamma \lambda } \right)^{2} \left[ {2 - \gamma^{2} \left( {1 + \lambda } \right)} \right]}}{{2\left[ {4 - 2\gamma \lambda - \gamma^{2} \left( {1 + \lambda } \right)\left( {2 + \lambda } \right)} \right]^{2} }}. \\ \end{aligned} $$

(6)

Next, we work out the consumer surplus and the social welfare, respectively, which are as follows:

$$ \begin{aligned} CS^{C} & = \Lambda (q_{1}^{C} ,q_{2}^{C} ) - (p_{1}^{C} q_{1}^{C} + p_{2}^{C} q_{2}^{C} ) = \frac{{(1 + \gamma )(2 - \gamma - \gamma \lambda )^{2} }}{{[4 - 2\gamma \lambda - \gamma^{2} \left( {1 + \lambda } \right)\left( {2 + \lambda } \right)]^{2} }} \\ SW^{C} & = CS^{C} + \sum\limits_{i = 1}^{2} {\pi_{i}^{C} + \Pi_{U}^{C} = } \frac{{\left( {2 - \gamma - \gamma \lambda } \right)[6 - \gamma - 3\gamma \lambda - \gamma^{2} \left( {1 + \lambda } \right)\left( {3 + 2\lambda } \right)]}}{{[4 - 2\gamma \lambda - \gamma^{2} \left( {1 + \lambda } \right)\left( {2 + \lambda } \right)]^{2} }} \\ \end{aligned} $$

(7)

3.2 Bertrand Competition

To solve the Bertrand game, we derive the direct demand function $q_{i} = \frac{{(1 - \gamma ) - p_{i} + \gamma q_{j} }}{{1 - \gamma^{2} }}$. Accordingly, the representative downstream firm chooses its price to maximize the following objective function in stage 2:

$$ \begin{aligned} \mathop {Max}\limits_{{p_{i} }} v_{i} = \pi_{i} + \lambda \pi_{j} = \left( {p_{i} - \omega_{i} } \right)\frac{{\left( {1 - \gamma } \right) - p_{i} + \gamma q_{j} }}{{1 - \gamma^{2} }} - F_{i} \hfill \\ \, + \lambda \left[ {\left( {p_{j} - \omega_{j} } \right)\frac{{\left( {1 - \gamma } \right) - p_{j} + \gamma q_{i} }}{{1 - \gamma^{2} }} - F_{j} } \right], (i,j{ = 1,2 }i \ne j ). \hfill \\ \end{aligned} $$

(8)

Solving the first-order conditions, we obtain the equilibrium price of the ith firm:

$$ p_{i}^{B} = \frac{{\left( {1 - \gamma } \right)\left( {2 + \gamma + \gamma \lambda } \right) + \left( {2 - \gamma^{2} \lambda \left( {1 + \lambda } \right)} \right)\omega_{i} + \gamma \left( {1 - \lambda } \right)\omega_{j} }}{{4 - \gamma^{2} \left( {1 + \lambda } \right)^{2} }}, (i,j{ = 1,2 }i \ne j ). $$

(9)

In stage 1, similar to Cournot competition, the upstream firm $U$ becomes involved in a decentralized bargaining process with the downstream firms to determine the terms of the two-part tariff contracts. We can then obtain the equilibrium per-unit input price and the equilibrium fixed fees as:

$$ \begin{aligned} \omega_{i}^{B} & = \frac{{\gamma [2\lambda - \gamma + \gamma \lambda - \gamma^{2} \lambda^{2} (1 + \lambda )]}}{{4 + \gamma \lambda [6 + \gamma (3 + \lambda ) - \gamma^{2} (1 + \lambda )(1 + 2\lambda )]}}, \\ F_{i}^{B} & = \frac{(1 - \gamma \lambda )(2 + \gamma + \gamma \lambda )[N + \beta (4 - 2\gamma (1 + 5\lambda ) + \gamma M)]}{{2(1 + \gamma )(4 - \gamma \lambda (6 + \gamma (3 + \lambda ) - \gamma^{2} (1 + \lambda )(1 + 2\lambda )))^{2} }} \\ \end{aligned} $$

(10)

where

$$\begin{aligned} M &= \gamma (2\lambda (1 + 3\lambda ) - \gamma (1 - \gamma - (5 - (4 - \gamma )\gamma )\lambda^{2} \hfill \\ \, &\quad + 2(2 - \gamma )(1 - \gamma )\lambda^{3} - (3 - \gamma )\gamma \lambda^{4} )) \hfill \\ N &= \gamma [2 - \gamma + \gamma^{2} + \gamma \lambda ( - 3 + \gamma )](2\lambda - \gamma (1 - \lambda + \gamma \lambda^{2} (1 + \lambda ))) \end{aligned}$$

According to (9) and (10), we can obtain the equilibrium price and equilibrium output of the final product:

$$ \begin{aligned} p_{i}^{B} & = \frac{(2 - \gamma - \gamma \lambda )[1 - \gamma \lambda (1 + \gamma + \gamma \lambda )]}{{4 - \gamma \lambda [6 + \gamma \left( {3 + \lambda } \right) - \gamma^{2} \left( {1 + \lambda } \right)\left( {1 + 2\lambda } \right)]}} \\ q_{i}^{B} & = \frac{{(1 - \gamma \lambda )^{2} (2 + \gamma + \gamma \lambda )}}{{(1 + \gamma )(4 - \gamma \lambda (6 + \gamma \left( {3 + \lambda } \right) - \gamma^{2} (1 + \lambda )(1 + 2\lambda )))}}. \\ \end{aligned} $$

(11)

The net equilibrium profits of $D_{i}$ and $U$ are

$$ \begin{aligned} \pi_{i}^{B} & = \frac{{\left( {1 - \beta } \right)\left( {1 - \gamma \lambda } \right)\left( {2 + \gamma + \gamma \lambda } \right)K}}{{2\left( {1 + \gamma } \right)\left( {4 - 6\gamma \lambda - 3\gamma^{2} \lambda + \gamma^{3} \lambda - \gamma^{2} \lambda^{2} + 3\gamma^{3} \lambda^{2} + 2\gamma^{3} \lambda^{3} } \right)^{2} }}, \\ \Pi_{U}^{B} & = \frac{{(1 - \gamma \lambda )(2 + \gamma + \gamma \lambda )[\beta (4 - 2\gamma - 10\gamma \lambda + \gamma^{2} H) - G]}}{{(1 + \gamma )(4 - \gamma \lambda (6 + \gamma (3 + \lambda ) - \gamma^{2} (1 + \lambda )(1 + 2\lambda )))^{2} }} \\ \end{aligned} $$

(12)

Where

$$ \begin{aligned} K &= 4 - 2\gamma - \gamma^{3} + \gamma^{4} - 10\gamma \lambda + 2\gamma^{2} \lambda + 6\gamma^{2} \lambda^{2} + 5\gamma^{3} \lambda^{2} - 4\gamma^{4} \lambda^{2} \hfill \\ &\quad + \gamma^{5} \lambda^{2} + 4\gamma^{3} \lambda^{3} - 6\gamma^{4} \lambda^{3} + 2\gamma^{5} \lambda^{3} - 3\gamma^{4} \lambda^{4} + \gamma^{5} \lambda^{4} \hfill \\ \end{aligned} ,$$

$$ G = (1 - \gamma )\gamma^{2} (1 + \lambda )(2\lambda - \gamma (1 - \lambda + \gamma \lambda^{2} (1 + \lambda ))) $$

$$ H = 2\lambda (1 + 3\lambda ) + \gamma [\gamma + 5\lambda^{2} - (4 - \gamma )\gamma \lambda^{2} + 2(2 - \gamma )(1 - \gamma )\lambda^{3} - (3 - \gamma )\gamma \lambda^{4} - 1]. $$

Next, we work out the consumer surplus and social welfare as follows:

$$ \begin{aligned} & CS^{B} = \Lambda (q_{1}^{B} ,q_{2}^{B} ) - (p_{1}^{B} q_{1}^{B} + p_{2}^{B} q_{2}^{B} )\\ &\quad = \frac{{(1 - \gamma \lambda )^{4} (2 + \gamma + \gamma \lambda )^{2} }}{{(1 + \gamma )(4 - 6\gamma \lambda - 3\gamma^{2} \lambda + \gamma^{3} \lambda - \gamma^{2} \lambda^{2} + 3\gamma^{3} \lambda^{2} + 2\gamma^{3} \lambda^{3} )^{2} }}, \\ & SW^{B} = CS^{B} + \sum\limits_{i = 1}^{2} {\pi_{i}^{B} + \Pi_{U}^{B}}\\ &\quad = \frac{{(1 - \gamma \lambda )^{2} (2 + \gamma + \gamma \lambda )(6 - \gamma - 9\gamma \lambda - 4\gamma^{2} \lambda + 2\gamma^{3} \lambda - 2\gamma^{2} \lambda^{2} + 5\gamma^{3} \lambda^{2} + 3\gamma^{3} \lambda^{3} )}}{{(1 + \gamma )(4 - 6\gamma \lambda - 3\gamma^{2} \lambda + \gamma^{3} \lambda - \gamma^{2} \lambda^{2} + 3\gamma^{3} \lambda^{2} + 2\gamma^{3} \lambda^{3} )^{2} }}. \\ \end{aligned} $$

(13)

We have the following lemma:

Lemma 1

The equilibrium price of the final product falls and the equilibrium output of the final product increases as $\lambda$ rises, i.e., as the intensity of downstream competition decreases.

Proof

From Eqs. (5) and (11) we obtain $\frac{{{\text{d}}p_{i}^{\rho *} }}{{{\text{d}}\lambda }} < 0$,$\frac{{{\text{d}}q_{i}^{\rho *} }}{{{\text{d}}\lambda }} > 0$$.$

Since $\frac{{\partial \omega_{i}^{{\rho^{*} }} }}{\partial \lambda } < 0$ and $\frac{{\partial F_{i}^{{\rho^{*} }} }}{\partial \lambda } > 0$, we can find that when downstream firms engage in decentralized bargaining with an upstream monopolist over two-part tariffs, the unit input price decreases and the fixed fee increases in $\lambda$, which is irrelevant to the competition modes of the downstream firms.⁸ The direct effect of an increase in $\lambda$ on the final product is that the price rises but output declines in order to gain more profits owing to the less intense competition in the downstream market. However, the indirect effect of an increase in $\lambda$ on the final product works through the decline in input price due to the downstream firms’ stronger incentives to avoid a higher input price. As the input price declines, the downstream firms will increase production and lower their prices to gain more profit. In this case, the indirect effect of an increase in $\lambda$ on the final product is stronger than the direct effect, which leads to a decline in price and a rise in production. Thus, we obtain a complete reversal of the standard result: when downstream firms bargain with an upstream monopolist over two-part tariffs, less intense competition between downstream firms, i.e., a larger $\lambda$, reduces the final product price de facto but increases the equilibrium of the final product output.

We now consider the effect of competition (or manager cooperation) on downstream profits. Intuitively, stronger competition should result in less profit for the downstream firms. However, this may not be true. This leads to the following proposition:

Proposition 1

The relationship between the profits of the downstream firms and the intensity of competition is U-shaped, irrespective of the competition modes in the downstream product market.

The total effect of a change in $\lambda$ on the profits of downstream firms is $\frac{{d\pi_{i} }}{d\lambda } = \frac{{\partial \pi_{i} }}{{\partial \omega_{i} }}\frac{{\partial \omega_{i} }}{\partial \lambda } + \frac{{\partial \pi_{i} }}{{\partial F_{i} }}\frac{{\partial F_{i} }}{\partial \lambda }$. The first term captures the indirect effect working through the change in input price. It is straightforward to check that $\frac{{\partial \pi_{i} }}{{\partial \omega_{i} }} < 0$ and $\frac{{\partial \omega_{i} }}{\partial \lambda } < 0$, so the first term is positive and the second term captures the indirect effect working through the change in the fixed fee. As $\frac{{\partial \pi_{i} }}{{\partial F_{i} }} < 0$ and $\frac{{\partial F_{i} }}{\partial \lambda } > 0$, the second term is negative. Hence, the overall effect of a change in $\lambda$ on downstream profits is not unambiguous, depending on the relative strength of the two effects mentioned earlier. We find that when $\lambda$ is sufficiently low, the effect working through the fixed fee is sufficiently strong and the standard result of oligopoly theory will be reversed. In this case, the profits of the downstream firms decrease in $\lambda$. On the other hand, when $\lambda$ is sufficiently high, the effect working through the input price is stronger and the profits of the downstream firms increase in $\lambda$. Thus, the relationship between the profits of the downstream firms and the intensity of competition is U-shaped.

Next, we examine the effects of the competitive regime on the profits of the upstream monopolist with the following proposition:

Proposition 2

The relationship between the utility of the upstream monopolist and the intensity of competition is inverted U-shaped, irrespective of the competition modes in the downstream market.

The total effect of a change in $\lambda$ on the profits of upstream firm is $\frac{{d\pi_{U}^{*} }}{d\lambda } = 2q_{i} \frac{{\partial \omega_{i}^{*} }}{\partial \lambda } + 2\omega_{i} \frac{{\partial q_{i}^{*} }}{\partial \lambda } + 2\omega_{i} \frac{{\partial q_{i}^{*} }}{{\partial \omega_{i}^{*} }}\frac{{\partial \omega_{i}^{*} }}{\partial \lambda } + 2\frac{{\partial F_{i}^{*} }}{\partial \lambda }$. The first term stands for the effect of a change in $\lambda$ on the equilibrium input price $\omega_{i}^{*}$. As $\frac{{\partial \omega_{i}^{*} }}{\partial \lambda } < 0$, this term is negative. The second term captures the effect of a change in $\lambda$ on the equilibrium level of output, $q_{i}^{*}$. As we know from Lemma 1, this effect is positive. The third term captures the indirect effect of a change in $\lambda$ on $q_{i}^{*}$ that works through the change in the input price. Because $\frac{{\partial q_{i}^{*} }}{{\partial \omega_{i}^{*} }} < 0$ and $\frac{{\partial \omega_{i}^{*} }}{\partial \lambda } < 0$, this term is positive. The fourth term captures the positive effect of a change in $\lambda$ on $F_{i}^{*}$. The overall effect of a change in $\lambda$ on profits is potentially ambiguous. When $\lambda$ is sufficiently low, the positive effect working through the output and the fixed fee is sufficiently strong, which dominates the negative effect captured by the first term and the standard result of oligopoly theory will be reversed. In this case, the profits of the upstream monopolist increase in $\lambda$. On the other hand, when $\lambda$ is sufficiently high, the negative effect working through the input price is stronger and the profit of the upstream monopolist decreases in $\lambda$. Hence, we find that the relationship between the utility of the upstream monopolist and $\lambda$ is inverted U-shaped.

4 Downstream competition, consumer surplus, and social welfare

We now turn to the comparison of the equilibrium outcomes under Cournot and Bertrand final market competition.

We first compare the impact of $\lambda$ on social welfare and consumer surplus under two competition modes. It is easy to show that $\frac{{dCS^{\rho *} }}{d\lambda } > 0$ and $\frac{{dSW^{\rho *} }}{d\lambda } > 0$. We move on to examine which competition mode is preferable from both consumer surplus and social welfare perspectives. Using Eqs. (7) and (13), we find that $\Delta CS = CS_{ }^{C*} - CS_{ }^{B*} > 0$ and $\Delta SW = SW_{ }^{C*} - SW_{ }^{B*} > 0$. We then have the following lemma:

Lemma 2

Consumer surplus and social welfare increase in $\lambda$ and both are higher under Cournot than under Bertrand competition.

The mechanism behind Lemma 2 is similar to that described by Symeonidis (2008). The effect of a change in $\lambda$ on consumer surplus can be decomposed as follows: $\frac{{dCS^{*} }}{d\lambda } = \frac{{\partial CS^{*} }}{{\partial q_{1}^{*} }}\frac{{\partial q_{1}^{*} }}{\partial \lambda } + \frac{{\partial CS^{*} }}{{\partial q_{2}^{*} }}\frac{{\partial q_{2}^{*} }}{\partial \lambda }$. The right-hand side of the equation stands for the indirect effect of a change in $\lambda$ on consumer surplus that works through the change in the output of the downstream firms. Because $\frac{{\partial {\text{CS}}^{*} }}{{\partial q_{i}^{*} }} > 0$ and $\frac{{\partial q_{i}^{*} }}{\partial \lambda } > 0$, this term is positive. Consumer surplus increases as the price declines and output rises. From Lemma 1, we know that the equilibrium price falls and the equilibrium output increases as the intensity of competition decreases. Hence, consumer surplus increases as $\lambda$ rises. On the other hand, social welfare is comprised of corporate profits and consumer welfare. Thus, the effect of $\lambda$ can be further decomposed into two different sub-effects: $\frac{{dSW^{*} }}{d\lambda } = \frac{{\partial \Pi^{*} }}{\partial \lambda } + \frac{{\partial CS^{*} }}{\partial \lambda }$, where $\Pi$ denotes the sum of the profits of the downstream firms and the upstream monopolist. The first term stands for the effect of a change in $\lambda$ on the aggregate profits of all firms. It is straightforward to check that $\frac{{\partial \Pi^{*} }}{\partial \lambda } > 0$, so the first effect is positive. The second term stands for the effect of a change in $\lambda$ on the consumer surplus, which is positive. Hence, the total effect is positive.

Moreover, we find that a market under Cournot competition is more efficient than a market under Bertrand competition, in the sense that both consumer surplus and social welfare are higher in the case of the former. Our finding is in sharp contrast with that of Mukherjee et al. (2012), who found that social welfare is always higher under Bertrand competition than under Cournot competition. Although our result is also obtained by Alipranti et al. (2014) in the case of decentralized two-part tariff vertical contracts, they did not account for the intensity of downstream competition. We find that their result holds even in the presence of downstream competition (or cooperation). The underlying mechanism is similar to that of Alipranti et al. (2014).

Define $\varvec{\varDelta}= \pi_{i}^{{C^{*} }} - \pi_{i}^{{B^{*} }}$, i.e., downstream firm i’s profit gap under Cournot and Bertrand competition. Let $\tilde{\lambda }^{ } = h(\gamma )$ denote the solution to the equation $\varvec{\varDelta}= 0$. We then make the following proposition:

Proposition 3

When $\lambda$ is sufficiently low, i.e., $\lambda < \tilde{\lambda }^{ }$ , the downstream firms’ profits are higher under Bertrand competition; when $\lambda$ is sufficiently high, i.e., $\lambda \ge \tilde{\lambda }^{ }$ , the downstream firms’ profits are higher under Cournot competition.

As shown in Fig. 1, the area above the blue line, i.e., Area A, denotes that the combination ($\lambda ,\gamma$) satisfies $\varvec{\varDelta}> 0$ and the downstream firms’ profits are higher under Cournot competition; the area below or on the line, i.e., Area B, denotes that the combination ($\lambda , \, \gamma$) satisfies $\varvec{\varDelta}\le 0$ and the downstream firms’ profits are higher under Bertrand competition. As shown in Proposition 1, the relationship between the profits of the downstream firms and $\lambda$ is U-shaped, irrespective of the competition modes in the downstream market. However, the profit loci of the downstream firms with respect to $\lambda$ are different under different competition modes.

Fig. 1

Comparison of downstream firms’ profits under Cournot competition and Bertrand competition

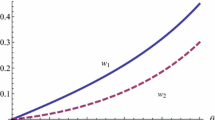
In Fig. 2, the blue dotted U-curve depicts how the profits of the downstream firms change with $\lambda$ under Bertrand competition, while the blue U-curve depicts how the profits of the downstream firms change with $\lambda$ under Cournot competition when $\gamma = 1/5$. When $- 1 < \lambda < \tilde{\lambda },$ each downstream firm’s profits under Bertrand competition are higher, i.e., $\pi_{i}^{{C^{*} }} < \pi_{i}^{{B^{*} }}.$ When $\tilde{\lambda }\le \lambda < 1$, each downstream firm’s profits under Cournot competition are higher, i.e., $\pi_{i}^{{C^{*} }} > \pi_{i}^{{B^{*} }}$. Considering the intensity of competition in the downstream market, our result differs from that of Alipranti et al. (2014), who showed that the equilibrium downstream profits are higher under Cournot than under Bertrand competition. It is also in contrast to the findings of Basak and Wang (2016), who demonstrated that the equilibrium downstream profits are the same under Cournot and Bertrand competition.

Fig. 2

Profit curves of the downstream firms with respect to $\lambda \, (\gamma = 1/5)$

The mechanism behind Proposition 3 is as follows. Each downstream firm’s profit motive is driven by the input price and upfront fixed fee payable to the upstream monopolist. Since $\frac{{\partial \pi_{i} }}{{\partial \omega_{i} }} < 0$ and $\frac{{\partial \pi_{i} }}{{\partial F_{i} }} < 0$, the effects of a change in $\omega_{i}$ and $F_{i}$ on the profits of the downstream firms, i.e., the input price effect and the fixed fee effect, are both negative. Clearly, the fixed fee is higher under Cournot competition i.e., $F_{i}^{{C^{*} }} < F_{i}^{{B^{*} }}$, whereas the input price is higher under Bertrand competition, i.e., $\omega_{i}^{{C^{*} }} > \omega_{i}^{{B^{*} }}$. Hence, the comparison of downstream firms’ profits under Bertrand competition and Cournot competition is ambiguous, depending on the relative strength of the fixed fee effect and the input price effect mentioned earlier. When $\lambda$ is sufficiently low, the fixed fee effect is stronger, implying that the profits of the downstream firms under Bertrand competition are higher than those under Cournot competition. On the other hand, when $\lambda$ is sufficiently high, the input price effect is stronger and the profits of each downstream firm are higher under Cournot competition.

$$ \tilde{\lambda }^{ } = h\left( \gamma \right) $$

We use a numerical example to illustrate the above propositions. We set $\gamma = 1/5$, $\beta = 1/2$, and $\lambda$ to be one of the following three values: − 0.9, − 0.6, or 0.9. Table 1 summarizes the equilibrium results.

Table 1 Numerical example

It is clear that Proposition 3 holds in this example.

5 Concluding remarks

In this paper, we have analyzed the effects of downstream competition intensity (or manager cooperation) in the presence of decentralized bargaining between two downstream firms and an upstream monopolist over a two-part tariff input price. We find that the relationship between the profits of the upstream monopolist (the downstream firms) and the intensity of competition is U-shaped (inverted U-shaped), irrespective of the competition modes in the downstream product market. As for the comparison of the competition modes in the downstream market, if the intensity of competition is sufficiently high, the downstream firms’ profits are higher under Bertrand competition; if the intensity of competition is sufficiently low, the downstream firms’ profits are higher under Cournot competition. We also show that a market under Cournot competition is more efficient than a market under Bertrand competition, in the sense that both consumer surplus and social welfare are higher in the former.

In our analysis, we have restricted our discussion to consider one firm in the upstream market and two firms in the downstream market, and we do not account for the possibility of free entry into the downstream market. Extending our model in this direction remains an issue for future research.