Introduction

Recently, there has been an intense interest in the dynamics of active, self-propelled particles, such as biological micro-organisms and synthetic colloidal motors (Ebbens and Howse 2010; Hong et al. 2010; Patra et al. 2013; Dey et al. 2016; Schwarz-Linek et al. 2016; Teo and Pumera 2016). Self-propelled particles have potential biomedical applications, including targeted drug delivery (Kline et al. 2005; Dhar et al. 2007; Sundararajan et al. 2008), where a drug is transported in or on an active particle through the bloodstream directly to a predetermined location, e.g., a cancerous tumor or region of inflammation. Furthermore, suspensions of self-propelled microscale particles serve as a paradigmatic model for active matter, which is inherently out of thermal equilibrium due to the activity of the particles (Tjhung et al. 2011; Stenhammar et al. 2014; Takatori et al. 2014; Spagnolie 2015). A natural question that arises is as follows: How does the activity of the particles impact the rheology, or state of the stress, in a flowing suspension? For example, how would the addition of active particles, for use in drug delivery, impact the rheology and subsequent blood flow in a patient? Conversely, what can the stress of an active suspension tell us about the activity (e.g., mechanism or strength of self-propulsion) of the particles in suspension?

In general, microscale self-propelled particles, at low Reynolds number, can be divided into two categories: pullers and pushers. Pullers, such as Chlamydymonus reinhardtii (CR), generate thrust from the front of their body; whereas, pushers, such as Escherichia coli (E. coli) or Bacillus subtilis (B. subtillis), generate thrust from their rear (Lauga and Powers 2009). Steady shear experiments on a dilute suspension of CR have shown that the effective viscosity of the suspension is increased, relative to both the viscosity of the suspending medium and the viscosity of a suspension of the same immotile (i.e., dead) bacteria (Rafaï et al. 2010; Mussler et al. 2013). Experiments conducted on dilute suspensions of pushers, E. coli (Gachelin et al. 2013; López et al. 2015) and B. subtilis (Sokolov and Aranson 2009), reported a steady shear viscosity less than that of a suspension of the same immotile bacteria and, more surprisingly, also less than the steady shear viscosity of the suspending medium. This indicates a negative particle contribution to the overall suspension viscosity.

From these experimental results, it is evident that the activity of the particles affects the stress in the suspension. The activity of the particles has an indirect effect on the stress by altering the microstructure, or spatio-temporal arrangement, of the suspension through tumbling, which acts to randomize the particle orientation, as observed in E. coli (Turner et al. 2000). The activity of the particles also has a direct effect on the stress via the force dipole (stresslet) generated from self-propulsion (Saintillan 2010; Takatori et al. 2014; Spagnolie 2015). One methodology to predict the stress of an active suspension is to calculate the disturbance flow created by the active particles. Then, from the energy dissipation associated with this, the effective viscosity can be inferred. Haines et al. (2008) utilized a two-dimensional hydrodynamics simulation to calculate the disturbance flow, and hence rheology, of a dilute, active suspension of disks. Hatwalne et al. (2004) utilized a coarse-grained model based on the field theory of nematic liquid crystals to predict the linear viscoelasticity of a dilute suspension of active disks or rods. Alternatively, one can explicitly determine how the activity of the particles affects the microstructure of the suspension and from there predict the microstructurally averaged stress. In this vein, Saintillan (2010) utilized a kinetic theory model to calculate the change in the orientational microstructure of a dilute suspension of active, slender rods, resulting from an imposed steady shear flow, rotational Brownian motion, and uncorrelated tumbling events (i.e., \(90^{\circ }\) changes in orientation due to tumbling). Bozorgi and Underhill (2014) calculated the stress in a dilute suspension of slender, active rods subject to a large-amplitude oscillatory shear (LAOS) deformation. They assumed that the tumbling events did not affect the microstructure of the suspension, but did include the direct stress contribution arising from the particle activity.

In this work, we calculate the linear viscoelasticity of a dilute suspension of active, spheroidal particles, of arbitrary aspect ratio, subject to a small-amplitude oscillatory shear (SAOS) deformation. We explicitly account for microstructural relaxation due to both rotational Brownian motion and correlated tumbling, as this is relevant for many biological systems. For instance, E. coli are Brownian, prolate bodies with an average change in orientation due to tumbling of \(58 \pm 40\)° (Turner et al. 2000). Oscillatory shear experiments are useful in that they allow for one to average the measured signal over multiple oscillation cycles and avoid step changes that occur during the start-up and cessation of a steady flow. SAOS experiments effectively allow one to take a spectral fingerprint of the linear response of a material. The frequency-dependent results obtained from a SAOS deformation can be used to predict steady shear behavior via the empirical Cox-Merz rule, which states that the magnitude of the frequency-dependent complex viscosity is equivalent to steady shear viscosity (Cox and Merz 1958). Thus, information from a SAOS deformation can be utilized to predict the steady shear viscosity of a material, which is advantageous as it is typically simpler to experimentally perform a frequency-sweep as opposed to a shear rate sweep (Macosko 1994). This could be especially true for active suspensions of biological organisms, where large shear rates could be detrimental to the organisms and inhibit activity. Gachelin et al. (2013) observed that the effective steady shear viscosity of both a motile and immotile dilute suspension of E. coli were equivalent at high shear rates, indicating that the active contribution to the steady shear viscosity is negligible compared to the passive contribution in this regime. There are cases where the Cox-Merz rule breaks down, namely colloidal dispersions at high shear rate and frequency (Al-Hadithi et al. 1992), and thus, it should be viewed as a “rule-of-thumb” rather than a strict rule.

For a homogeneous, oscillatory shear deformation, the strain-rate is given by \(\dot {\gamma } = \dot {\gamma }_{\scriptscriptstyle {0}}\cos (\omega t)\), where \(\dot {\gamma }_{\scriptscriptstyle {0}}\) is the strain-rate amplitude and \(\omega \) is the oscillation frequency. Two timescales arise: (1) the oscillation timescale, \(1/\omega \) and (2) the flow timescale, \(1/\dot {\gamma }_{\scriptscriptstyle {0}}\). The active suspension is also characterized by two independent relaxation mechanisms: Brownian rotation and tumbling. Therefore, we can form two ratios of the flow to relaxation timescales, or Weissenberg numbers: \(Wi_{\scriptscriptstyle {D}} = \dot {\gamma }_{\scriptscriptstyle {0}}/D_{r}\) and \(Wi = \dot {\gamma }_{\scriptscriptstyle {0}}/\tau ^{-1}\), where \(D_{r}\) is the rotary diffusion coefficient and \(\tau ^{-1}\) is frequency of tumbling. Furthermore, a relative rate of relaxation can be defined as \(\lambda =Wi/Wi_{\scriptscriptstyle {D}}= D_{r}/\tau ^{-1}\). For a dilute suspension of E. coli, \(\lambda \sim 0.1\) (using \(D_{r} \sim 0.1\) s\(^{-1}\) and \(\tau ^{-1} \sim 1\) s\(^{-1}\) (Leal and Hinch 1971; Gachelin et al. 2013)) and tumbling is therefore the dominant mode of microstructural relaxation. SAOS requires that the rate of relaxation is much greater than the rate of deformation, \(Wi \ll 1\). Thus, the linear viscoelasticity of a dilute suspension of active spheroids is examined in this regime.

In the “Governing equations” section, we detail the governing equations required to calculate the microstructure and linear viscoelasticity of the active suspension. In the “Asymptotic expansion for linear viscoelasticity” section, a solution for the microstructure is obtained via an asymptotic analysis for \(Wi \ll 1\). In the “Linear viscoelasticity” section, the deviatoric particle contribution to the suspension stress is calculated, and the combined effects of the imposed flow, Brownian rotation, and tumbling are discussed. Finally, in the “Comparison to previous work” section, we demonstrate that a Cox-Merz rule can be applied to an active particle suspension by comparing our linear viscoelastic results to (1) a theoretical prediction of the steady shear viscosity of active, slender rods (Saintillan 2010) and (2) experimental data for the steady shear viscosity of a dilute suspension of E. coli (López et al. 2015). We also demonstrate that from the linear viscoelasticity one can determine the method of self-propulsion (pusher or puller) and estimate the strength of self-propulsion and correlation between tumbling events.

Governing equations

A suspension of active particles is modeled as an ensemble of Brownian, rigid spheroids in a Newtonian fluid of viscosity \(\mu _{s}\) and density \(\rho \). The particles are assumed to be free of any externally applied force or torque and are characterized by an aspect ratio, r, defined as the ratio of the major (\(\ell \)) to minor (a) axes, r = ℓ/a. The hydrodynamic sphericity of the particles is given by the Bretherton constant,

$$ B = \frac{r^{2} -1}{r^{2} +1}\,, $$
(1)

which is negative for oblate spheroids, positive for prolate spheroids, and zero for spheres. The suspension is assumed to be dilute, such that interparticle interactions can be neglected. Thus, \(c = 4\pi n\ell a^{2}/3 \ll 1\), where c is the particle volume fraction and n is the number density. We will consider an oscillatory shear flow with velocity field \(\mathbf {v} = \dot {\gamma }_{\scriptscriptstyle {0}}y\cos (\alpha \tilde {t}\,)\mathbf {e}_{x}\), and velocity gradient \(\nabla \mathbf {v} = \dot {\gamma }_{\scriptscriptstyle {0}}\cos (\alpha \tilde {t}\,)\mathbf {e}_{y}\mathbf {e}_{x}\), where \(\mathbf {e}_{x}\), \(\mathbf {e}_{y}\), \(\mathbf {e}_{z}\) are Cartesian unit vectors in the flow, flow-gradient, and vorticity directions, respectively, \(\alpha \) is the dimensionless oscillation frequency , and \(\tilde {t}\) is dimensionless time (\(\alpha = \omega \tau \), \(\tilde {t} = t/\tau \)).

The microstructure of the suspension is characterized by the orientation distribution function, \(\psi (\mathbf {p},t)\), where \(\psi (\mathbf {p},t)\sin \theta \text {d}\theta \text {d}\phi \) represents the probability of finding a particle ensemble in a differential region about (\(\theta ,\phi \)) (Hinch and Leal 1972; Kim and Karrila 2005). Here, \(\mathbf {p}\) is a unit vector along the particle axis of symmetry, in spherical coordinates, and \(\theta \) and \(\phi \) are the polar and azimuthal angles, respectively. The orientation distribution function satisfies a conservation, or Fokker-Planck, equation (Subramanian and Koch 2009),

$$ \frac{\partial\psi}{\partial t} + \nabla_{\mathbf{p}}\cdot(\dot{\mathbf{p}}\,\psi)=D_{r}\nabla_{\mathbf{p}}^{2}\psi - \tau^{-1}\left( \psi - \int K(\mathbf{p}|\mathbf{p}^{\prime})\psi(\mathbf{p}^{\prime},\tilde{t}\,)\text{d}S^{\prime}\right), $$
(2)

where \(\nabla _{\mathbf {p}}\) is the surface gradient operator, and \(\dot {\mathbf {p}}\) is the deterministic evolution of the particle orientation due to the ambient shear (Jeffery 1922; Brenner and Condiff 1974; Kim and Karrila 2005),

$$ \dot{\mathbf{p}} = B(\mathbf{I}-\mathbf{pp})\cdot\mathbf{H}\cdot\mathbf{p}, $$
(3)

where \(\mathbf {I}\) is the identity tensor and \(\mathbf {H} = B^{-1}\boldsymbol {\Omega }+\mathbf {E}\). Here, \(\boldsymbol {\Omega }\) is the vorticity tensor and \(\mathbf {E}\) is the rate of strain tensor, such that \(\nabla \mathbf {v} = \mathbf {E} + \boldsymbol {\Omega }\). For an oscillatory shear deformation, \(\mathbf {H} = \dot {\gamma }_{\scriptscriptstyle {0}}\cos (\alpha \tilde {t}\,)\check {\mathbf {H}}\), where,

$$ \check{\mathbf{H}} = \frac{1}{2}\Big[(1-B^{-1})\mathbf{e}_{x}\mathbf{e}_{y} + (1+B^{-1})\mathbf{e}_{y}\mathbf{e}_{x}\Big]. $$
(4)

The first term on the right-hand-side (RHS) of Eq. 2 accounts for the randomization of particle orientation due to rotational Brownian diffusion. The last terms on the RHS, in parentheses, of Eq. 2 model the randomization of particle orientation due to tumbling events. Specifically, tumbling events are treated as a Poisson process (Subramanian and Koch 2009). Here, \(K(\mathbf {p}|\mathbf {p}^{\prime })\) is a conditional probability density function characterizing a tumble from orientation \(\mathbf {p}^{\prime }\) to orientation \(\mathbf {p}\), where the apostrophe indicates the pre-tumble orientation, and d\(S^{\prime } = \sin \theta ^{\prime }\text {d}\theta ^{\prime }\text {d}\phi ^{\prime }\) is the pre-tumble differential solid angle. Conservation of probability requires that,

$$ \int K(\mathbf{p}|\mathbf{p}^{\prime})\text{d}S = \int K(\mathbf{p}|\mathbf{p}^{\prime})\text{d}S^{\prime} = 1\,. $$
(5)

Following Subramanian and Koch (2009), we choose

$$ K(\mathbf{p}|\mathbf{p}^{\prime}) = \frac{\beta}{4\pi\sinh\beta}\exp\left( \beta\,\mathbf{p}\cdot\mathbf{p}^{\prime}\right), $$
(6)

where \(\beta \) is parameter that gauges the correlation between pre- and post-tumble configurations. In the limit of \(\beta \rightarrow 0\), \(K = 1/4\pi \), corresponding to uncorrelated tumbles (90\(^{\circ }\) changes in orientation), and the term in parentheses on the RHS of Eq. 2 reduces to the relaxation-time approximation used in the kinetic theory of gases (Chapman and Cowling 1991; McQuarrie 2000). This limit was previously investigated by Saintillan (2010) for slender, active rods in steady shear. In the limit of \(\beta \rightarrow \infty \), each tumbling event results in an infinitesimal change in orientation, and thus, microstructural relaxation is dominated by rotational diffusion. This regime was investigated by Bozorgi and Underhill (2014). The inclusion of finite, non-zero values of \(\beta \) allows one to account for correlated tumbles. For instance, the average change in orientation due to tumbling (\(<\theta >\)) for an E. coli cell is \(58 \pm 40\) \(^{\circ }\) (Turner et al. 2000), which corresponds to \(\beta \sim 1.5\), where (Subramanian and Koch 2009),

$$ <\theta> \;= \frac{\beta}{2\sinh\beta}{\int}_{0}^{\pi}\text{e}^{\beta\cos\theta}\theta\sin\theta\text{d}\theta. $$
(7)

A dimensionless conservation equation can be obtained by normalizing \(\mathbf {H}\) by the strain-rate amplitude (\(\mathbf {H} = \dot {\gamma }_{\scriptscriptstyle {0}}\tilde {\mathbf {H}}\)). Substitution of Eq. 3 into Eq. 2 yields,

$$ \frac{\partial \psi}{\partial \tilde{t}} + BWi\nabla_{\mathbf{p}}\cdot\Big[(\mathbf{I}-\mathbf{pp})\cdot\tilde{\mathbf{H}}\cdot\mathbf{p}\,\psi\Big] =\lambda\nabla_{\mathbf{p}}^{2}\psi - \left( \psi - \int K(\mathbf{p}|\mathbf{p}^{\prime})\psi(\mathbf{p}^{\prime},\tilde{t}\,)\text{d}S^{\prime}\right), $$
(8)

The orientation distribution function is also subject to a normalization condition, \(\int \psi (\mathbf {p},t)\text {d}S = 1\).

Asymptotic expansion for linear viscoelasticity

The conservation (8) is to be addressed in the limit of weak deformation rate, \(Wi\ll 1\). In this regime, the microstructure remains in a near-equilibrium state, and the orientation distribution function can be expressed as a regular perturbation expansion,

$$ \psi(\mathbf{p},t) = \frac{1}{4\pi}\Big(1+Wi\psi_{1}(\mathbf{p},t) + O(Wi^{2})\Big), $$
(9)

where \(Wi\psi _{1}\) represents the leading order departure from equilibrium. Substitution of Eq. 9 into Eq. 8 yields,

$$\begin{array}{@{}rcl@{}} \frac{\partial\psi_{1}}{\partial \tilde{t}} &=& \;3B\cos(\alpha\tilde{t}\,)\,\mathbf{pp}:\check{\mathbf{E}}\\ &&+\lambda\Bigg[(\mathbf{I}-\mathbf{pp}):\frac{\partial^{2}\psi_{1}}{\partial\mathbf{p}\partial\mathbf{p}}-\; 2\mathbf{p}\cdot\frac{\partial\psi_{1}}{\partial\mathbf{p}}\Bigg]\\ &&-\left( \psi_{1} - \int K\psi_{1}(\mathbf{p}^{\prime},\tilde{t}\,)\text{d}S^{\prime}\right), \end{array} $$
(10)

subject to \(\int \psi _{1}\text {d}S = 0\). Here, \(\mathbf {E} = \dot {\gamma }_{\scriptscriptstyle {0}}\cos (\alpha \tilde {t}\,)\check {\mathbf {E}}\) and \(\check {\mathbf {E}} =\frac {1}{2}\left (\mathbf {e}_{x}\mathbf {e}_{y} + \mathbf {e}_{y}\mathbf {e}_{x}\right )\). The solution to Eq. 10 should oscillate at the input frequency, \(\alpha \), and linearly depend on the imposed flow through the quadrupolar forcing \(3B\cos (\alpha \tilde {t}\,)\,\mathbf {pp}:\check {\mathbf {E}}\), which acts to align the microstructure along the principle axes of strain. Hence, we pose,

$$ \psi_{1}(\mathbf{p},\tilde{t}\,) = \Big(h_{1}\text{e}^{i\alpha\tilde{t}} + \hat{h}_{1}\text{e}^{-i\alpha\tilde{t}}\Big)\mathbf{pp}:\check{\mathbf{E}}, $$
(11)

where hatted quantities indicate complex conjugates. Substitution of Eq. 11 into Eq. 10 yields an algebraic equation for \(h_{1}\), with solution,

$$ h_{1} = \frac{3B}{2}\left[\frac{1-J(\beta)+6\lambda - i\alpha}{\alpha^{2} + \big(1-J(\beta)+6\lambda\big)^{2}}\right], $$
(12)

where \(i = \sqrt {-1}\) and

$$ J(\beta) = \frac{(3+\beta^{2})\sinh\beta - 3\beta\cosh\beta}{\beta^{2}\sinh\beta}. $$
(13)

To determine (12) and (13), we have used the result: \(\int K\mathbf {p}^{\prime }\mathbf {p}^{\prime }:\check {\mathbf {E}}\,\text {d}S^{\prime } = J(\beta )\mathbf {pp}:\check {\mathbf {E}}\). A derivation of this relation can be found in Appendix B of Subramanian and Koch (2009); in their equation (B8), the coefficient of \(\sinh \beta \) is incorrect and should read \(-(3\beta ^{2} + 6)\). The expression in Eq. 13 is even and is bounded by \(J(\beta ) = [0,1)\), corresponding to \(\beta = [0,\pm \infty )\). Next, we utilize our asymptotic solution to the orientation distribution function to calculate the linear viscoelasticity of the suspension.

Linear viscoelasticity

The particle contribution to the deviatoric stress of the suspension (\(\boldsymbol {\tau }^{p}\)) is obtained from an ensemble average of the stresslet, which can be decomposed into three contributions arising from: the imposed flow (\(\boldsymbol {\tau }^{F}\)), rotational Brownian motion (\(\boldsymbol {\tau }^{B}\)), and the active nature (self-propulsion) of the particles (\(\boldsymbol {\tau }^{A}\)),

$$ \boldsymbol{\tau}^{p} = \boldsymbol{\tau}^{F} + \boldsymbol{\tau}^{B} + \boldsymbol{\tau}^{A}. $$
(14)

The contribution from the imposed flow is given by Hinch and Leal (1976) and Kim and Karrila (2005),

$$\begin{array}{@{}rcl@{}} \boldsymbol{\tau}^{F} &=& \;2\mu_{s}c\Bigg[2A_{H}\Big(<\mathbf{pppp}> -\, \frac{1}{3}<\mathbf{pp}>\Big):\mathbf{E}\\ &&+2B_{H}\Big(\mathbf{E}\cdot<\mathbf{pp}> + <\mathbf{pp}>\cdot\mathbf{E}\\ &&+\frac{2\,\mathbf{I}}{3}\,\mathbf{E}:<\mathbf{pp}>\Big) + C_{H}\mathbf{E}\Bigg], \end{array} $$
(15)

where the angled brackets are orientational averages,

$$ <\mathbf{pp}> \;=\int \mathbf{pp}\,\psi(\mathbf{p},\tilde{t}\,)\text{d}S, $$
(16)

and similarly for \(<\mathbf {pppp}>\). The torque generated from rotational Brownian motion leads to the following stress contribution (Hinch and Leal 1976; Kim and Karrila 2005),

$$ \boldsymbol{\tau}^{B} = 2\mu_{s}cF_{H}D_{r}\Big(<\mathbf{pp}> - \frac{\mathbf{I}}{3}\Big). $$
(17)

Here, \(A_{H}\), \(B_{H}\), \(C_{H}\), and \(F_{H}\) are known scalar functions of the particle aspect ratio, r, Hinch and Leal (1972) and Kim and Karrila (2005). Finally, the self-propulsion of the particles exerts a force dipole onto the fluid, resulting in the active stress contribution (Saintillan and Shelley 2008),

$$ \boldsymbol{\tau}^{A} = n\sigma_{0}\Big(<\mathbf{pp}> - \frac{\mathbf{I}}{3}\Big), $$
(18)

where \(\sigma _{0}\) is a scalar dipole strength, in units of energy. The sign of \(\sigma _{0}\) indicates the mechanism of self-propulsion (i.e., \(\sigma _{0}<0\) for pushers and \(\sigma _{0}>0\) for pullers) and the magnitude of \(\sigma _{0}\) scales with the swimming speed of the particle (Saintillan and Shelley 2008)

Substitution of Eqs. 11 and 12 into Eqs. 15, 17 and 18 yields for the shear component of the stress, \(\tau _{yx}\),

$$ \frac{\tau_{yx}^{F}}{\dot{\gamma}_{\scriptscriptstyle{0}}\mu_{s}c} = \left( \frac{4A_{H}}{15} + \frac{2B_{H}}{3} + C_{H}\right)\cos(\alpha\tilde{t}\,) + O(Wi), $$
(19)
$$\begin{array}{@{}rcl@{}} \frac{\tau_{yx}^{B}}{\dot{\gamma}_{\scriptscriptstyle{0}}\mu_{s}c} &=& \frac{2F_{H}B\lambda}{5} \Bigg[\frac{1-J(\beta)+6\lambda}{\alpha^{2} + \big(1-J(\beta)+6\lambda\big)^{2}}\cos(\alpha\tilde{t}\;)\\ &&+\frac{\alpha}{\alpha^{2} + \big(1-J(\beta)+6\lambda\big)^{2}}\sin(\alpha\tilde{t}\;)\Bigg] + O(Wi),\\ \end{array} $$
(20)

and

$$\begin{array}{@{}rcl@{}} \frac{\tau_{yx}^{A}}{\dot{\gamma}_{\scriptscriptstyle{0}}\mu_{s}c} &=& \frac{zB}{5} \Bigg[\frac{1-J(\beta)+6\lambda}{\alpha^{2} + \big(1-J(\beta)+6\lambda\big)^{2}}\cos(\alpha\tilde{t}\;)\\ &&+\frac{\alpha}{\alpha^{2} + \big(1-J(\beta)+6\lambda\big)^{2}}\sin(\alpha\tilde{t}\;)\Bigg] + O(Wi).\\ \end{array} $$
(21)

Note that the shear component of the stress is all that is obtained from linear viscoelasticity; normal stress differences can be obtained at \(O(Wi^{2})\), requiring knowledge of \(\psi _{2}\). Here, \(z = \sigma _{0}\tau /\mu _{s}V_{p}\) is a dimensionless dipole moment and \(V_{p}\) is the volume of a single particle. For \(\lambda <1\) (\(\lambda >1\)), the frequency of tumbling is greater (less) than the frequency of rotational Brownian motion; the dominant relaxation mechanism is tumbling (rotational Brownian motion). The overall particle contribution to the deviatoric stress can be expressed as the sum of a viscous and elastic response,

$$ \frac{\tau_{yx}^{p}}{\dot{\gamma}_{\scriptscriptstyle{0}}} = \eta^{\prime}(\alpha)\cos(\alpha\tilde{t}\,) + \eta^{\prime\prime}(\alpha)\sin(\alpha\tilde{t}\,) + O(Wi), $$
(22)

where \(\eta ^{\prime }\) and \(\eta ^{\prime \prime }\) are the viscous and elastic components of the complex viscosity (\(\eta ^{*} = \eta ^{\prime } + i\eta ^{\prime \prime }\)), respectively, which are given by,

$$\begin{array}{@{}rcl@{}} \frac{\eta^{\prime}}{\mu_{s}c} &=& \frac{4A_{H}}{15} + \frac{2B_{H}}{3} + C_{H}\\ &&+\frac{B}{5}\left( 2F_{H}\lambda + z\right)\frac{1-J(\beta)+6\lambda}{\alpha^{2} + \big(1-J(\beta)+6\lambda\big)^{2}}, \end{array} $$
(23)

and

$$ \frac{\eta^{\prime\prime}}{\mu_{s}c} = \frac{B}{5}\left( 2F_{H}\lambda + z\right)\frac{\alpha}{\alpha^{2} + \big(1-J(\beta)+6\lambda\big)^{2}}. $$
(24)

Figure 1 shows the effect of correlated tumbling (\(\beta \neq 0\)) on the magnitude of the viscous and elastic components of the complex viscosity. Correlated tumbling events increase the magnitude of the Brownian and active stress response, compared to uncorrelated tumbling events, at small and moderate frequency values (\(\alpha \leq O(10)\)).

Fig. 1
figure 1

Effect of correlated tumbling (β ≠ 0) on a the viscous and b elastic components of the complex viscosity for λ = 0.5. Here, \(\eta ^{\prime }_{\scriptscriptstyle {\infty }} = 4A_{H}/15 + 2B_{H}/3 + C_{H}\) is the viscous complex viscosity in the limit of \(\alpha \rightarrow \infty \). Correlated tumbling events increase the magnitude of the Brownian and active stress contribution at small to moderate frequency values (\(\alpha \lesssim 10\)), resulting in an increased magnitude of the viscous and elastic components

Full size image

Since z can be positive or negative, depending on the mechanism of self-propulsion, we can define a critical dipole strength, \(z^{*}\), at which the particle contribution to the zero-frequency viscous complex viscosity is zero (\(\eta ^{\prime }(0) = 0\)),

$$\begin{array}{@{}rcl@{}} z^{*} &=&\frac{5}{B\big(1-J(\beta)+6\lambda\big)}\left( \frac{4A_{H}}{15} + \frac{2B_{H}}{3} + C_{H}\right)\\ &&+ \;2F_{H}\lambda, \end{array} $$
(25)

which is positive for prolate spheroids and negative for oblate spheroids, the latter since B, \(F_{H} < 0\) for \(r<1\). Thus, a dispersion of prolate pushers with dipole strength \(z < - z^{*}\) can have a negative contribution to the overall viscosity of the suspension, as previously predicted (Hatwalne et al. 2004; Haines et al. 2008; Saintillan 2010) and observed (Sokolov and Aranson 2009; Gachelin et al. 2013; López et al. 2015). However, we are not aware of prior experimental work indicating a negative contribution to the overall viscosity for oblate pullers, as is predicted here.

Figure 2 shows a schematic representation of the stresslet (force dipole exerted by the particle onto the fluid) for a prolate spheroid subject to a shear flow. The passive contribution to the stresslet, resulting from the imposed flow and Brownian rotation, always results in a compressional stresslet and therefore a positive contribution to the suspension viscosity. However, the active contribution, arising from the thrust force and compensating drag force generated from self-propulsion, can result in either a compressional or extensional stresslet, depending on the mechanism of self-propulsion. Prolate pullers, which generate thrust from the front, always have a compressional active stresslet, and therefore a compressional overall stresslet (Fig. 2a) and positive contribution to the suspension viscosity. Weak prolate pushers, which generate thrust from behind and are characterized by the dipole strength \(-z^{*}<z<0\), will have an extensional active stresslet, but a compressional overall stresslet (Fig. 2b). This results in a positive contribution to the suspension viscosity, but the magnitude of the viscosity increase is less than that of a passive suspension. Strong prolate pushers, characterized by \(z < -z^{*}<0\), will have an extensional active stresslet of greater magnitude than the passive stresslet contribution, resulting in an extensional overall stresslet (Fig. 2c) and a negative contribution to the suspension viscosity. Note that the overall viscosity of the suspension (\(\eta \)), which includes the contribution of the suspending medium (\(\mu _{s}\)), will remain positive for a dilute suspension of prolate pullers or pushers, regardless of the magnitude of the dipole, as \(\eta = \mu _{s}+c\,\eta ^{p}\), where \(\eta ^{p}\) is the particle contribution to the overall viscosity and c (\(\ll 1\)) is the particle volume fraction

Fig. 2
figure 2

Schematic of the stresslet for a prolate spheroid in steady shear flow for different self-propulsion mechanisms: a puller (0<z), b weak pusher (−z ∗<z<0), and c strong pusher (z<−z ∗<0). The solid black linesrepresent the straining component of the flow and the dashed black linesthe principle axes of strain. In the limit of Wi≪1, the flow, to leading order, acts to align the microstructure along the principle axes of strain. The passive contribution (left, red) to the stresslet, from the imposed flow and rotational Brownian motion, always results in a compressional force dipole from the particle onto the fluid. However, the active contribution (middle, green, and blue) to the stresslet can be either compressional or extensional, depending on the mechanism of self-propulsion. Thus, for pullers and weak pushers, the overall stresslet (right, magenta) is compressional. Whereas, for strong pushers, the overall stresslet is extensional, resulting in a negative viscosity contribution. Here, u s indicates the self-propelled swimming speed of particle

Full size image

The linear relaxation modulus, \(G_{I}(s)\), is defined as Macosko (1994),

$$ G_{I}(s) = \frac{2}{\pi}{\int}_{0}^{\infty}\eta^{\prime}(\alpha)\cos(\alpha s)\text{d}s , $$
(26)

and can be determined from Eq. 23 to be,

$$\begin{array}{@{}rcl@{}} G_{I}(\tilde{t}\,) &=& \Big(\frac{8A_{H}}{15} + \frac{4B_{H}}{3} + 2C_{H}\Big)\delta(\tilde{t}\,)\\ &&+\; \frac{B}{5}\Big(2F_{H}\lambda + z\Big)\text{e}^{-\big(1-J(\beta) + 6\lambda\big)\tilde{t}}. \end{array} $$
(27)

The linear relaxation modulus has the functional form of the Jeffrey’s model: an instantaneous response combined with stress relaxation via an exponentially fading memory, similar to that of passive spheroids (Abdel-Khalik et al. 1974; Bird et al. 1977; Bechtel and Khair 2017). In contrast to a suspension of passive spheroids, the rate of stress relaxation, \(1/t_{r}\), which is defined from the argument of the exponential in Eq. 27, is

$$ \frac{1}{t_{r}}= \frac{1}{t_{\scriptscriptstyle{T}}} + \frac{1}{t_{\scriptscriptstyle{B}}}, $$
(28)

where \(t_{\scriptscriptstyle {T}} = \tau /\big [1-J(\beta )\big ]\) is the timescale of tumbling events and \(t_{\scriptscriptstyle {B}} = 1/6D_{r}\) is the timescale of rotational Brownian motion. This combination of two stress relaxation mechanisms is analogous to a pair of resistors in parallel in an electrical circuit, where the overall resistance of the system (\(R_{total}\)) is the reciprocal of the sum of the reciprocal resistance of each resistor (\(R_{i}\)), \(1/R_{total} = {\sum }_{i} 1/R_{i}\). Here, the overall rate of stress relaxation is the sum of the reciprocal of the timescale of each relaxation mechanism. The combination of stress relaxation from tumbling events and rotational Brownian motion increases the overall rate of stress relaxation, relative to a suspension of non-Brownian, active particles or a suspension of Brownian, inactive particles.

Figures 3 and 4 depict the complex viscosity of a dilute suspension of prolate (\(r =3\)) and oblate (\(r=0.25\)) spheroids, respectively, as a function of self-propulsion mechanism, z, and relative importance of tumbling to rotational Brownian motion, \(\lambda \). For both prolate and oblate spheroids, the plateau frequency, which is defined as the frequency at which the material response no longer changes, increases as the strength of rotational Brownian motion increases (i.e., as \(\lambda \) increases). Furthermore, we also observe that prolate pullers and oblate pushers will always have a positive viscous and elastic response, independent of frequency. Whereas, prolate pushers and oblate pullers with a dipole strength greater than the critical dipole strength, defined in Eq. 25, can have a negative viscous and elastic response at low frequency.

Fig. 3
figure 3

Viscous (\(\eta ^{\prime }\), top) and elastic (\(\eta ^{\prime \prime }\), bottom) components of the complex viscosity for a dilute suspension of active prolate spheroids (r = 3) for (1) a puller: z = 50, (2) a weak pusher: z = −50, and (3) a strong pusher: z = −200.Here, the ratio of tumbling frequency to rotational Brownian motion is varied: a λ = 0, b λ = 0.1, and c λ = 1 and z ∗=−150.4, using λ = 1 and β = 1

Full size image
Fig. 4
figure 4

Viscous (\(\eta ^{\prime }\), top) and elastic (\(\eta ^{\prime \prime }\), bottom) components of the complex viscosity for a dilute suspension of active oblate spheroids (r = 0.25 ) for (1) a pusher: z = −50, (2) a weak puller: z = 50, and (3) a strong puller: z = 200. Here, the ratio of tumbling frequency to rotational Brownian motion is varied: a λ = 0, b λ = 0.1, and c λ = 1 and z ∗=198.5, using λ = 1 and β = 1

Full size image

Comparison to previous work

Steady shear of active, slender rods (\(r\rightarrow \infty \))

The particle contribution to the steady shear viscosity of a dilute suspension of active, slender rods (\(r \rightarrow \infty \)) was previously calculated by Saintillan (2010) for uncorrelated tumbling events (\(\beta = 0\)). We can compare these steady shear results to our linear viscoelastic response, via the Cox-Merz rule,

$$ \eta(Wi) = |\eta^{*}|(\alpha), $$
(29)

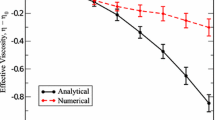
which states that, for \(Wi = \alpha \), the steady shear viscosity is equal to the magnitude of the frequency-dependent complex viscosity. Figure 5 shows a comparison of the linear viscoelasticity and the steady shear viscosity for a puller and a pusher at \(\lambda = 0.5\). In Fig. 5a, we observe that the Cox-Merz rule holds fairly well for prolate pullers, with a qualitative agreement overall all frequencies. For prolate pushers, which have a negative contribution to the overall viscosity of the suspension, the Cox-Merz rule does not hold, as the magnitude of the complex viscosity is always a positive quantity (Fig. 5b). However, we observe agreement between the steady shear viscosity and the viscous component of the complex viscosity up to \(O(10)\) in the shear rate (\(Wi\)) or frequency (\(\alpha \)). This indicates that the particle contribution to the steady shear viscosity is akin to the linear viscous response for an active suspension, up to moderate shear rate. That is, \(\eta (\dot {\gamma }_{\scriptscriptstyle {0}}) = \eta ^{\prime }(\omega )\) is applicable beyond the zero-shear and zero-frequency limit and can be viewed as a modified Cox-Merz rule for active suspensions with a negative viscosity increment. The deviation between the steady shear and both the viscous complex viscosity and magnitude of the complex viscosity, at larger \(Wi\) and frequency, is consistent with passive colloidal systems (Al-Hadithi et al. 1992).

Fig. 5
figure 5

Cox-Merz rule for active suspensions. Here, we compare our theoretical prediction to that of Saintillan (2010) (see his Figure 5a) for \( r \rightarrow \infty \), β = 0, λ = 0.5 (corresponding to \(\tilde {\tau } = 5\) and \(\tilde {d}_{r} = 0.1\) in the notation of Saintillan (2010)) for a puller (z = 4A H ) and b pusher (z = −4A H ). The circlesand squares are the digitized numerical results for the steady shear viscosity from Saintillan 2010. The solid line is the magnitude of the complex viscosity, from Eqs. 23–24, and the dot-dashed lineis the viscous component of the complex viscosity, from Eq. 23

Full size image

Experimental results for suspensions of E. coli

Now that we have demonstrated that a modified Cox-Merz rule is applicable to a dilute, active suspension, at least to moderate frequency and shear rate, we can extend our comparison to an experimental system. In Fig. 6, we show the digitized steady shear viscosity measurements of López et al. (2015) for E. coli suspensions (symbols), which includes the contribution of the suspending medium, compared to the viscous component of the complex viscosity (23), at varied volume fractions (solid lines). The experimental parameters reported by López et al. (2015) utilized in Eq. 23 are \(\tau ^{-1} = 10\) Hz, \(T = 298\) K, and \(\mu _{s} = 1.4\) mPa. To determine the relative frequency of Brownian rotation to tumbling (\(\lambda \)), we first calculated the rotational diffusion coefficient for a single particle (Leal and Hinch 1971),

$$ D_{r} = \frac{k_{B}T}{4\mu_{s}V_{p}}\left( \frac{r^{2} K_{3}(r) + K_{1}(r)}{r^{2} + 1}\right), $$
(30)

where,

$$ K_{1}(r) \equiv {\int}_{0}^{\infty}\frac{r\,\text{d}s}{\left( r^{2} + s\right)^{1/2}(1+s)^{2}}, $$
(31)
$$ K_{3}(r) \equiv {\int}_{0}^{\infty} \frac{r\,\text{d}s}{(r^{2} + s)^{3/2}(1+s)}, $$
(32)

and \(k_{B}\) is the Boltzmann constant. Substitution of the given values for temperature and suspending medium viscosity, along with an estimate of aspect ratio of an E. coli cell, \(r=20\) (\(\ell \sim 5\;\mu \)m and a∼0.25 μ m (Trueba and Woldringh 1980; Gachelin et al. 2013; López et al. 2015)), into Eq. 30 yields D r =0.009 s −1. Thus, \(\lambda = D_{r}/\tau ^{-1} = 9\times 10^{-4}\) and tumbling is the dominant mode of microstructural relaxation. Here, the estimate of the aspect ratio of an E. coli cell includes the length of the flagella tail that is responsible for self-propulsion and tumbling. Next, we utilized the steady shear viscosity measurements at \(Wi = 0.002\) and Wi = 0.007 and the modified Cox-Merz rule via (23) to obtain a system of two equations and two unknowns (β and z); solving this system of equations simultaneously obtains β and z, which are listed for each volume fraction in Table 1. The dotted line in Fig. 6 is the viscous complex viscosity given in Eq. 23 for \(\beta = 1.64\), corresponding to \(<\theta > = 58^{\circ }\) (Turner et al. 2000), and z = −155.5 , corresponding to a thrust force, F thrust = σ 0/ℓ = 0.57 pN (Chattopadhyay et al. 2006).

Fig. 6
figure 6

Comparison of the linear viscoelasticity of a suspension of pushers to experimental measurements of the steady shear viscosity of E. coli, which include the contribution of the suspending medium, for cell volume fractions, c, of a 0.11%, b 0.21%, c 0.44%, and d 0.67%. The symbols are experimental data from López et al. (2015) (see their Fig. 1b) for μ s =1.4 mPa, and T = 298. The solid line is the viscous complex viscosity in Eq. 23 using r = 20, τ = 0.1 s, λ = 9×10−4, and the appropriate β and z values from Table 1. The volume fraction-dependent values of β and z were obtained from the steady shear viscosity measurements at Wi = 0.002 and Wi = 0.007. Thedotted line is the viscous complex viscosity from Eq. 23, now using β = 1.64, which was obtained from fluorescence microscopy (Turner et al. 2000), and z = −155.5, which was obtained from optical trapping measurements (Chattopadhyay et al. 2006)

Full size image

From Fig. 6, we see a qualitative agreement between the steady shear viscosity measurements of López et al. (2015) and our prediction of the linear viscoelasticity, using the values of \(\beta \) and z in Table 1 at all values of \(Wi\) and \(\alpha \). However, the use of \(\beta \) obtained from fluorescence microscopy (Turner et al. 2000) and z from optical trapping of a single cell (Chattopadhyay et al. 2006) does not provide a reasonable agreement to the experimental data. This suggests that the measured self-propulsion characteristics of an active suspension are sensitive to the volume fraction of particles. Furthermore, the cell volume fractions utilized by López et al. (2015) are arguably beyond the dilute regime, where hydrodynamic interactions between cells is negligible; collective motion has been observed in suspensions of E. coli for \( n = O(10^{8})\) cells/mL (Wu et al. 2006), which corresponds to \(c\sim 0.01\%\). Despite the fact that the data reported by López et al. (2015) is at volume fractions above this threshold, we surprisingly observe good agreement between their experimental results and our dilute theory. Thus, the results from the SAOS of a dilute, active suspension are able to provide the self-propulsion characteristics of active particles; namely, the self-propulsion mechanism (pusher or puller), dipole strength (\(|\sigma _{0}|\)), and correlation between tumbling events (\(\beta \)).

Table 1 Volume fraction-dependent self-propulsion parameters of E. coli
Full size table

Summary

We calculated the linear viscoelasticity of a dilute suspension of active spheroids of arbitrary aspect ratio subject to a SAOS deformation. The active suspension is characterized by two relaxation mechanisms: rotational Brownian motion and correlated tumbling. For weak deformation (\(Wi \ll 1\)), the particle contribution to the stress was determined from ensemble averages of the stresslets due to the imposed flow, rotational Brownian motion, and tumbling. From a comparison to the steady shear result of Saintillan (2010) for slender rods, we show that a modified Cox-Merz rule is applicable to a dilute active suspension. Furthermore, through a comparison with the experimental results of López et al. (2015) for E. coli, we demonstrated that from the SAOS of an active suspension, one can determine the mechanism of self-propulsion (pusher or puller) and estimate the strength of the dipole moment (\(|\sigma _{0}|\)) and correlation between tumbling events (\(\beta \)) of the particles. A natural extension to the present work would be to quantify how correlated tumbling affects the nonlinear rheology of an active suspension, e.g., shear-thinning (or thickening) and normal stress coefficients.