Introduction

Micro- and nanofluidic devices have attracted considerable attention due to its versatile application like drug diagnostic [1, 2], separation of bio-macromolecules [3,4,5], DNA sequencing [6], and desalination of seawater [7] and environmental monitoring sensors [8] to name a few. These devices became growing more importance microelectromechanical system (MEMS), Lab-on-a-chip, etc., and it is designed as microchannels, micromixers, micropumps, nanopore, and microreaction chambers. When electrolyte solution comes in contact with charged nanopore wall, electric double layer (EDL) forms at the wall surface due to the redistribution of counter-ions and co-ions in the electrolyte medium. Many important features were experimentally observed in nanofluidic devices when characteristic height is comparable to the EDL thickness. Electroosmotic flow (EOF) is the bulk liquid motion under the application of external electric field [9]. These devices use the electrokinetic effects such as electroosomsis to transport electro-neutral solute through the soft polyelectrolyte-coated nanopore. When wall surface of the nanopore is covered with soft polymeric material named as soft nanopore where the polyelectrolyte layer (PEL) is sandwiched between the rigid nanopore wall and bulk electrolyte medium. Due to several advantages of EOF such as plug-like velocity profile, negligible axial dispersion and better flow control, many research group studied in different aspect of EOF under the applied electric field. A large volume of experimental, theoretical, and computational studies conducted for steady EOF and solute transport and mixing phenomena on the Newtonian fluid for different geometrical shape such as rectangular microchannel [10], cylindrical capillary [11], semi-circular microchannel [12], annulus [13], T-shape of microchannel [14], and surface-modulated microchannel [15] with heterogeneous surface potential. Relatively large DC electric field strength is required for such type of steady-state EOF which might not be always undesirable in many practical situation. By applying DC electric field in a microfluidic system, sometimes it may form gas bubbles. This may block the flow passage which may be suppressed under AC electric field. As a result, time-dependent EOF has become major growing attraction as an alternative mechanism for micro-fluidics transport. Dutta and Beskok [16] studied the time periodic EOF in two-dimensional rectangular microchannel and established the analytic solution for flow field under AC electric field. The control of time periodic flow rate under the pulsating electric fields through a circular microchannel has been studied by Chakraborty and Ray [17]. Jian et al. [18] analyzed the time periodic EOF through a cylindrical microannulus considering different zeta potentials in inner and outer cylinder using linear Poisson–Boltzmann equation for induced potential and Navier–Stokes equation for flow field. Huang and Lai [19] studied theoretically the enhancement of mass transport and species separation phenomena under an oscillatory EOF in a microchannel.

Polyelectrolyte-coated nanopore, nanometer size pore, or polyelectrolyte layer has motivated a great attraction among many research community for regulating ion transport and sensing single bio-polymers phenomena. EOF through a PEL-grafted nanochannel under the AC electric field has been investigated by Li et al. [20] using Debye–Hückel approximation. A theoretical studies on the ion transport phenomena through a poly electrolyte modified nanopore have been performed by Yeh et al. [21] using a continuum-based model. An analytic solution for transient EOF through the polyelectrolyte grafted nanochannel is derived by Li et al. [22] using Debye–Hückel approximation for Jeffrey fluid under the AC electric field. Chen and Das [23] investigated the electroosmotic transport through the pH-regulated polyelectrolyte-grafted nanochannel. Previously, it is considered that for low charging density in polyelectrolyte grafting which allows equal permittivity ratio between the electrolyte solution and PEL. Generally, the permittivity in the polyelectrolyte molecules is substantially less than the surrounding electrolyte solution, and hence depending on the PEL charge density, a significant impact occurs on the functional permittivity inside the PEL which creates the ion-partitioning effect. Physically, the Born energy [24], which transform the energy of an ion from bulk electrolyte solution to the PEL, determines the ion-partitioning effect. Poddar et al. [25] studied the Born energy effects on pressure gradient induced electrokinetic flow through a soft charged layer in narrow fluidic conduit. Ganjizade et al. [26] studied the ion-partitioning effect in a spherical soft particle in the presence of volumetric core charge density. Reshadi and Saidi [27] analyzed theoretically the electro-hydrodynamics characteristic on combined electroosmotic and pressure-driven flow through polyelectrolyte-grafted nanotube considering steric effects. Maurya et al. [28] theoretically investigate the ion-partitioning effects on electrophoresis of a soft particle made up of a charged hydrophobic inner core surrounded with PEL.

Although most of authors studied the EOF for the Newtonian fluids but saliva solution, blood sample, DNA sample which are used to analyse in BioMENS devices, cannot be treated as Newtonian fluids. Many microfluidic devices are also uses non-Newtonian bio-fluids to analyse the physiology and medical applicant. The Cauchy momentum equation with a proper constitutive law are used to describe the fluid characteristics of these kind of fluids instead of the Navier–Stokes equation. Peralta et al. [29] studied theoretically the mass transport rate of an electro-neutral solute in EOF under the applied periodic electric field through an annular micro-annular region between two concentric cylinders with asymmetric and symmetric zeta potentials for Maxwell fluids. Analytic solutions for transient EOF velocity between parallel plate micro channel and micro tube for Maxwell fluids have been derived by Li et al. [30]. Using the Debye–Hückel approximation in electric potential, Liu et al. [31] derived an analytic solution of the magneto-hydrodynamical EOF velocity between two parallel plates for Maxwell fluid.

The aim of the study is to investigate theoretically the ion-partitioning effects on electroosmotic flow and solute transport phenomena of Maxwell fluid through the PEL-coated conical nanopore under the influence of the imposed AC electric field. The medium near the polyelectrolytes medium attached with electrolyte molecules of an aqueous solution. Being different permittivity between two medium, ion-partitioning effect occurs which is determined by the Born formula. With considering the ion-partitioning effects, the linearized Poisson Boltzmann equation gives the distribution of the induced potential and the Cauchy momentum equation with Maxwell constitute equation provide the distribution of the flow pattern in the exterior and interior of the PEL. The analytic expression for the solutions for the distribution of the induced electric potential and axial fluid velocity are established in this study. The convection–diffusion equation is also solved analytically to study the transport behaviour of electro neutral solute. The comparison between the Newtonian and Maxwell fluids are also addressed in this study. The main objective in this paper is to analyse the influence of oscillating Reynolds number, the permittivity ratio between two layers, relaxation time, PEL fixed charge density, ionic concentration, softness parameter on electrokinetic flow and solute transport phenomena in Maxwell fluid.

Mathematical model

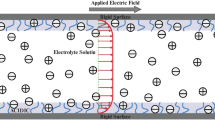
We have studied the unsteady EOF of a generalized Maxwell fluids under the influence of an external applied AC electric field through PEL grafted nanopore of radius a (Fig. 1). The nanopore is filled with an aqueous binary symmetric electrolyte of valence \(z_{i}\) \((i = 1,2)\) of density \(\rho\). The dielectric constant of the PEL and electrolyte medium are considered to be different and denoted as \(\epsilon _{\mathrm{p}}\) and \(\epsilon _{\mathrm{e}}\) respectively when \(\epsilon _{0}\) is the vacuum permittivity. A PEL of uniform thickness d is embedded in the wall surface of the nanopore which is bearing uniform negative surface potential \(\zeta ^{*}\). The PEL contains uniform volume charge density \(\rho _{\mathrm{fix}}\) which stem from ionization of PEL molecule where \(\rho _{\mathrm{fix}}= ZFN\), where F is the Faraday constant, Z is the valence and N is the molar concentration of the PEL ions. The EDL is formed at the interfaces between the electrolyte solution and the inner surface of the cylinder and EDL thickness (\(\lambda _{\mathrm{D}}\)) is the reciprocal of the Debye layer thickness \(\kappa (=1/\lambda _{\mathrm{D}}=\sqrt{\Sigma _{i}(z_{i}e)^{2}n_{0}/\epsilon _{0}\epsilon _{\mathrm{e}}k_{\mathrm{B}}T}\)). Here, \(k_{\mathrm{B}}\) is Boltzmann constant, T is absolute temparature, e is the elementary charge and \(n_{0}\) is the bulk ionic concentration. When the permittivity of the polyelectrolyte ions is significantly less than that of the surrounding electrolyte medium, a substantial impact occurs on the effective permittivity inside the PEL, depending upon the grafting charge density [32]. Due to different dielectric permittivity of the PEL and electrolyte medium, a partition effects of ions \(\Delta W_{i}\) [33] occur due to the change in self energy between these ions. The difference ionic concentration \(n_{i}\) of the ith species between the two medium can be related through the ion-partitioning coefficient \(f_{i}\) as \(n_{i}\mid _{r=a-d^{-}}=f_{i}n_{i}\mid _{r = a-d^{+}}\) [26, 32] The ion partition coefficient factor can be expressed as

$$\begin{aligned} \begin{aligned} f_{i}=\exp \left( -\Delta W_{i}/k_{\mathrm{B}}T\right) \end{aligned} \end{aligned}$$
(1)

The Born energy \(\Delta W_{i}\) determines the associated electrostatic free energy required to transfer an ion from the bulk solvent to the bulk of the PEL membrane, and it can be expressed as [33]

$$\begin{aligned} \Delta W_{i}= \frac{(z_{i}e)^{2}}{8\pi r_{i}\epsilon _{0}}\left( \frac{1}{\epsilon _{\mathrm{p}}}-\frac{1}{\epsilon _{\mathrm{e}}}\right) \end{aligned}$$
(2)

Here \(r_{i}\) is the radius of the ith ionic species. The flow is considered as axisymmetric and fully developed through the cylindrical nanopore. In this study, we consider cylindrical coordinate systems (r, z) where r denotes the radial direction and z is the axial direction. The externally imposed oscillating electric field, \(E_{z}(t^{*})\) along the z-direction is assumed to be \(E_{z}(t^{*}) =E_{0}\sin \omega t^{*}\) where \(E_{0}\) is the magnitude, \(\omega (=2\pi \pounds )\) is the oscillating frequency of the applied electric field, \(\pounds\) is the frequency in kHz and t is the time. The flow is driven by an oscillatory electroosmotic force induced by electric double layer and time-dependent applied AC electric field.

Fig. 1
figure 1

The schematic diagram of polyelectrolyte layer-coated canonical nanopore

Full size image

Analytic expression for induced potential

The background salt is assumed to be KCl, for which the hydrated radii of the corresponding ionic species \({\text{K}}^{+}\), \({\text{Cl}}^{-}\), \({\text{H}}^{+}\) and \({\text{OH}}^{-}\) are respectively 3.30, 3.32, 2.82 and 3.0 Å [34]. For computational simplicity, it considered that the hydrated radius of the dissociated ions \(r_{i} (=3.3)\) Å are all equal which leads \(\Delta W_{i}=\Delta W\) for all i and corresponding partition coefficients in Eq. (1) of the binary symmetric electrolyte ions become the same, i.e., \(f_{1}=f_{2}=f\). The bulk ionic concentration of the ith ionic species is denoted by \(n_{i}^{0}\). Far away from the charged surface \(n_{i}^{0}\) approaches to the bulk concentration \(n_{0}\) \((i.e., n_{+}^{0}=n_{-}^{0}=n_{0})\). For low-charge density, the ionic species are considered to follow the equilibrium Boltzmann distribution [35] which remains valid if the frequency of the external electric field is not very high (e.g., less than 1 MHz) [36]. Then the distribution of the ionic species inside and outside of the PEL can be written as:

$$\begin{aligned} n_i^{*}= {\left\{ \begin{array}{ll} n_i^0 \exp \left( \frac{-z_{i} e \phi ^{*}}{k_{\mathrm{B}}T}\right) &{}\quad { 0\le {r^{*}}<{a-d^{*}}} \\ n_i^0 \exp \left( \frac{-z_{i} e \phi ^{*}}{k_{\mathrm{B}}T}\right) \exp {\left( -\frac{\Delta W}{k_{\mathrm{B}}T}\right) }&{}\quad { {a-d^{*}}\le {r^{*}}\le {a}}. \end{array}\right. } \end{aligned}$$
(3)

Under the thin Debye layer assumption, the distribution of electrolyte potential within and outside PEL is governed by the Poisson equation which relates the net charge density in EDL due to mobile ions and fixed charged within PEL and expressed as

$$\begin{aligned} \nabla ^{2}\Phi ^{*} = {\left\{ \begin{array}{ll} -\frac{\Sigma z_{i}en_{i}^{*}}{\epsilon _{0}\epsilon _{\mathrm{e}}}&{}\quad { 0\le {r^{*}}<{a-d^{*}}} \\ -\frac{\Sigma z_{i}en_{i}^{*}}{\epsilon _{0}\epsilon _{\mathrm{p}}}\exp \left( -\frac{\Delta W}{k_{\mathrm{B}}T}\right) -\frac{\rho _{\mathrm{fix}}}{\epsilon _{0}\epsilon _{\mathrm{p}}} &{}\quad { {a-d^{*}}\le {r^{*}}\le {a}}. \end{array}\right. } \end{aligned}$$
(4)

Using the Debye–Hückel approximation for low surface charge i.e., \(\phi <k_{\mathrm{B}}T/e=0.025\) V and for symmetric binary electrolyte (\(z_{+}=-z_{-}=z\)), the Poisson–Boltzmann equation for the induced potential distribution can be linearized and expressed inside and outside of the PEL in the following non-dimensional form as

$$\begin{aligned}&\frac{1}{r}\frac{{\mathrm{d}}}{{\mathrm{d}} r}\left( r\frac{{\mathrm{d}} \phi _{\mathrm{e}}}{{\mathrm{d}} r} \right) =(\kappa a)^{2}\phi _{\mathrm{e}} \qquad 0\le {r}<{1-d} \end{aligned}$$
(5a)
$$\begin{aligned}&\frac{1}{r}\frac{{\mathrm{d}}}{{\mathrm{d}} r}\left( r\frac{{\mathrm{d}} \phi _{\mathrm{p}}}{{\mathrm{d}} r}\right) =\frac{1}{\epsilon _{\mathrm{r}}}\left[ (\kappa a)^{2}\exp {\left( -\Delta w\right) }\phi _{\mathrm{p}}-q_{\mathrm{fix}}\right] \qquad \nonumber \\&\quad \qquad {1-d}\le {r}\le {1} \end{aligned}$$
(5b)

Here, \(\epsilon _{\mathrm{r}}\) is the ratio of the relative dielectric permittivity of the PEL layer to the bulk electrolyte solution and is given by \(\epsilon _{\mathrm{r}}=\epsilon _{\mathrm{p}}/\epsilon _{\mathrm{e}}\). \(\phi _{\mathrm{e}}\) and \(\phi _{\mathrm{p}}\) represent the induced potential in electrolyte and PEL region, respectively. We have taken radius of the nanopore, a as a length scale. We scale potential \(\phi ^{*}\), \(\zeta ^{*}\) by thermal potential \(\phi _{0}(=k_{\mathrm{B}}T/e)\), ionic concentration \(n_{i}^{*}\) by bulk ionic concentration \(n_{0}\) and the Born energy \(\Delta W\) by \(k_{\mathrm{B}}T\). The scaled fixed charge density in PEL can be expressed as \(q_{\mathrm{fix}}=Ne^{2}zZa^{2}/\epsilon _{0}\epsilon _{\mathrm{e}}k_{\mathrm{B}}T\). The continuity of electrostatic potential and electric displacement are considered at the interface between the PEL and electrolyte region. The dimensionless boundary condition are given as follows

$$\begin{aligned} \begin{aligned}&\frac{{\mathrm{d}}\phi _{\mathrm{e}}}{{\mathrm{d}}r}\mid _{r=0}=0,\qquad \phi _{\mathrm{e}}\mid _{r=(1-d)^{-}}=\phi _{\mathrm{p}}\mid _{r=(1-d)^{+}}\\&\frac{{\mathrm{d}}\phi _{\mathrm{e}}}{{\mathrm{d}}r}\mid _{r=(1-d)^{-}}=\epsilon _{r}\frac{{\mathrm{d}}\phi _{\mathrm{p}}}{{\mathrm{d}}r} \mid _{r=(1-d)^{+}}, \qquad \phi _{\mathrm{p}}\mid _{r=1}=\zeta \end{aligned} \end{aligned}$$
(6)

In the present paper, in order to account for the ion-partitioning effects, we have introduced different relative dielectric permittivities of the PEL and the bulk solution. As a result, on the boundary between the PEL and the surrounding electrolyte solution, the electric field strength is no longer continuous and instead the electric displacement becomes continuous [37]. For the case when the relative dielectric permittivities of the PEL and the bulk solution are equal, this boundary condition becomes the continuity condition of the electric field strength. The solutions of the Poisson equation Eqs. (5a) and (5b), inside and outside PEL with the corresponding above boundary condition given in Eq. (6) are as follows

$$\begin{aligned} \phi _{\mathrm{e}}= & {} AI_{0}\left( \kappa ar\right) +BK_{0}\left( \kappa ar\right) \qquad 0\le {r}<{1-d} \end{aligned}$$
(7a)
$$\begin{aligned} \phi _{\mathrm{p}}= & {} CI_{0}\left( \kappa a\sqrt{\frac{{m}}{\epsilon _{r}}}r\right) +DK_{0}\left( \kappa a\sqrt{\frac{{m}}{\epsilon _{r}}}r\right) \nonumber \\&+\,\frac{q_{\mathrm{fix}}}{m(\kappa a)^{2}} \qquad {1-d}\le {r}\le {1} \end{aligned}$$
(7b)

here, \(I_{n}\) and \(K_{n}\) are the modified Bessel function of first and second kind of order n and we denote \(\exp {\left( -\Delta w\right) }\) as m. The values of the arbitrary constants are specified in Eqs. (7a) and (7b) are given in “Appendix 1”.

Analytic expression for velocity field

The nanopore is assumed to be very long and concentrating the study at the middle region far away from inlet/outlet, the flow is considered as unidirectional and fully developed. For unsteady unidirectional EOF in fully developed generalize Maxwell fluid, the modified Cauchy momentum equation with Darcy–Brinkman term in the exterior and interior PEL can be written as

$$\begin{aligned} \rho \frac{\partial }{\partial t^{*}}u_{\mathrm{e}}^{*}\left( r^{*},t^{*}\right)&=-\frac{1}{r^{*}}\frac{\partial }{\partial r^{*}} \left( r^{*}\tau _{rz}^{*}\right) +\rho _{\mathrm{e}}^{*}E_{z}(t^{*})\nonumber \\&\quad 0\le {r}^{*}<{a-d^{*}} \end{aligned}$$
(8a)
$$\begin{aligned} \rho \frac{\partial }{\partial t^{*}}u_{\mathrm{p}}^{*}\left( r^{*},t^{*}\right)&=-\frac{1}{r^{*}}\frac{\partial }{\partial r^{*}} \left( r^{*}\tau _{rz}^{*}\right) +\rho _{\mathrm{e}}^{*}E_{z}(t^{*})-\gamma u_{\mathrm{p}}^{*}(r^{*},t^{*})\nonumber \\&\quad a-d^{*}\le {r^{*}}\le {a} \end{aligned}$$
(8b)

here, \(u_{\mathrm{e}}^{*}\) and \(u_{\mathrm{p}}^{*}\) denote the velocity components in the electrolyte and PEL region respectively. \(\gamma\) is the hydrodynamics frictional coefficient [38] of the PEL. The stress tensor of Maxwell fluid \(\tau _{rz}^{*}\), satisfies the constitutive equation

$$\begin{aligned} \left( 1+\lambda \frac{\partial }{\partial t^{*}}\right) \tau _{r,z}^{*}=-\eta _{0}\frac{\partial u^{*}}{\partial r^{*}} \end{aligned}$$
(9)

where \(\lambda\) is the relaxation time [39] and \(\eta _0\) is the zero-shear-rate viscosity of the fluid. Corresponding boundary conditions are given as

$$\begin{aligned} \begin{aligned}&\frac{\partial u_{\mathrm{e}}^{*}}{\partial r^{*}}\mid _{r^{*}=0}=0,\quad u_{\mathrm{e}}^{*}\mid _{r^{*}=(a-d^{*})^{-}}=u_{\mathrm{p}}^{*}\mid _{r^{*}=(a-d^{*})^{+}}\\&\frac{\partial u_{\mathrm{e}}^{*}}{\partial r^{*}}\mid _{r^{*}=(a-d^{*})^{-}}=\frac{\partial u_{\mathrm{p}}^{*}}{\partial r^{*}}\mid _{r^{*}=(a-d^{*})^{+}}, \qquad u_{\mathrm{p}}^{*}\mid _{r^{*}=a}=0 \end{aligned} \end{aligned}$$
(10)

The velocity field \(u^{*}\) and time \(t^{*}\) are scaled by the Helmholtz–Smoluchowski velocity \(U_{\mathrm{HS}}(=\epsilon _{0}\epsilon _{\mathrm{e}} E_{0}\phi _{0}/\eta _{0})\) and \(1/\omega\) respectively. The stress coefficient \(\tau _{r,z}^{*}\) is scaled by \(\eta _{0} U_{\mathrm{HS}}/a\). The oscillating Reynolds number \({\text{Re}}_{w}\) is defined as \({\text{Re}}_{w}=\rho \omega a^{2}/\eta _{0}\) which represents as the ratio between the characteristic length scale associate with momentum diffusions length scale. Here, \(\nu =\eta _{0}/\rho\) is the kinematic viscosity. The non-dimensional parameter \(\beta\)=\(a/\lambda _{0}^{-1}\), represents a measure of the friction experienced by the fluid within the polyelectrolyte layer where \(\lambda _{0}^{-1}=\sqrt{\eta _{0}/\gamma }\) is the softness degree of the PEL.

To establish the analytic solution for the distribution of the induced potential and axial velocity of the present scenario under the AC electric field, we have considered the electric field and velocity in the following complex form as

$$\begin{aligned} E_{z}(t^{*})= & {} {\text{Im}}\left( E_{0}e^{ i\omega t^{*}}\right) \end{aligned}$$
(11)
$$\begin{aligned} u^{*}(r^{*},t^{*})= & {} {\text{Im}}\left( u_{0}^{*}(r^{*})e^{ i\omega t^{*}}\right) \end{aligned}$$
(12)

where \({\text{Im}}\) denotes the imaginary part of a complex number, and \(u_{0}^{*}(r^{*})\) is the complex amplitude of EOF velocity. The Cauchy momentum equation in the non-dimensional form can be written as

$$\begin{aligned} {\text{Re}}_{w}\frac{\partial u_{\mathrm{e}}}{\partial t}= & {} -\frac{1}{r}\frac{\partial }{\partial r}\left( r\tau _{rz}\right) \nonumber \\&-(\kappa a)^{2}\phi _{\mathrm{e}} {\text{Im}}(e^{it}) \qquad 0\le {r}<{1-d} \end{aligned}$$
(13a)
$$\begin{aligned} {\text{Re}}_{w}\frac{\partial u_{\mathrm{p}}}{\partial t}= & {} -\frac{1}{r}\frac{\partial }{\partial r}\left( r\tau _{rz}\right) -(\kappa a)^{2}m\phi _{\mathrm{p}} {\text{Im}}(e^{it})\nonumber \\&-\beta ^{2}u_{\mathrm{p}} \qquad {1-d}\le {r}\le {1} \end{aligned}$$
(13b)

The simplified dimensionless constitutive equation for Maxwell model is

$$\begin{aligned} \begin{aligned} \left( 1+{\text{Re}}_{w}\lambda _{1}\frac{\partial }{\partial t}\right) \tau _{rz}=-\frac{\partial u}{\partial r} \end{aligned} \end{aligned}$$
(14)

Here, \(\lambda _{1}=\lambda \eta _{0}/\rho a^{2}\) represents the competition between elastic and viscous effects of the fluid, called the Deborah number or elasticity number. The non-dimensional form of momentum equation inside and outside of the PEL can be written as

$$\begin{aligned}&\frac{{\mathrm{d}}^{2}(u_{0})_{\mathrm{e}}}{{\mathrm{d}}r^{2}}+\frac{1}{r}\frac{{\mathrm{d}}(u_{0})_{\mathrm{e}}}{{\mathrm{d}}r}-(u_{0})_{\mathrm{e}} \big (i{\text{Re}}_{w}-\lambda _{1}{\text{Re}}_{w}^{2}\big )\nonumber \\&\quad =(\kappa a)^{2}(1+i\lambda _{1}{\text{Re}}_{w})AI_{0} (\kappa ar)\qquad 0\le {r}<{1-d} \end{aligned}$$
(15a)
$$\begin{aligned}&\frac{{\mathrm{d}}^{2}(u_{0})_{\mathrm{p}}}{{\mathrm{d}}r^{2}}+\frac{1}{r}\frac{{\mathrm{d}}(u_{0})_{\mathrm{p}}}{{\mathrm{d}}r} -(u_{0})_{\mathrm{p}}\left( \beta ^{2}+\left( i{\text{Re}}_{w}-\lambda _{1}{\text{Re}}_{w}^{2}\right) \right) \nonumber \\&\quad =(\kappa a)^{2}m(1+i\lambda _{1} {\text{Re}}_{w}) \nonumber \\&\qquad *\left[ CI_{0}\left( \kappa a\sqrt{\frac{{m}}{\epsilon _{r}}}r\right) +DK_{0}\left( \kappa a\sqrt{\frac{{m}}{\epsilon _{r}}}r\right) +\frac{q_{\mathrm{fix}}}{\left( \kappa a\right) ^{2}m}\right] \nonumber \\&\qquad {1-d}\le {r}\le {1} \end{aligned}$$
(15b)

The corresponding dimensionless boundary conditions are given as

$$\begin{aligned} \begin{aligned}&\frac{{\mathrm{d}}(u_{0})_{\mathrm{e}}}{{\mathrm{d}}r}\mid _{r=0}\,=\,0,\qquad (u_{0})_{\mathrm{e}}\mid _{r=(1-d)^{-}}=(u_{0})_{\mathrm{p}}\mid _{r=(1-d)^{+}}\\&\frac{{\mathrm{d}}(u_{0})_{\mathrm{e}}}{{\mathrm{d}}r}\mid _{r=(1-d)^{-}}=\frac{{\mathrm{d}}(u_{0})_{\mathrm{p}}}{{\mathrm{d}}r}\mid _{r=(1-d)^{+}}, \qquad (u_{0})_{\mathrm{p}}\mid _{r=1}=0 \end{aligned} \end{aligned}$$
(16)

The solution of Eqs. (15a) and (15b) corresponding to the above boundary conditions Eq. (16) are given by

$$\begin{aligned} (u_{0})_{\mathrm{e}}\,=\, & {} C_{1}I_{0}\left( \sqrt{\big (i{\text{Re}}_{w}-\lambda _{1}{\text{Re}}_{w}^{2}\big )}r\right) \nonumber \\&+D_{1}K_{0}\left( \sqrt{\big (i{\text{Re}}_{w}-\lambda _{1}{\text{Re}}_{w}^{2}}\big )r\right) \nonumber \\&+GI_{0}\left( \kappa ar\right) \qquad 0\le {r}<{1-d} \end{aligned}$$
(17a)
$$\begin{aligned} (u_{0})_{\mathrm{p}}\,=\, & {} C_{2}I_{0}\left( \sqrt{\beta ^{2}+\big (i{\text{Re}}_{w}-\lambda _{1} {\text{Re}}_{w}^{2}\big )}r\right) \nonumber \\&+D_{2}K_{0}\left( \sqrt{\beta ^{2}+\big (i{\text{Re}}_{w}- \lambda _{1}{\text{Re}}_{w}^{2}\big )}r\right) \nonumber \\&+MI_{0}\left( \kappa a\sqrt{\frac{m}{\epsilon _{r}}}r\right) +NK_{0}\left( \kappa a\sqrt{\frac{m}{\epsilon _{r}}}r\right) \nonumber \\&-q_{\mathrm{fix}}\frac{1+i\lambda _{1} {\text{Re}}_{w}}{\beta ^{2}+\big (i{\text{Re}}_{w}-\lambda _{1}{\text{Re}}_{w}^{2}\big )} \quad {1-d}\le {r}\le {1} \end{aligned}$$
(17b)

The value of the arbitrary constants are specified in Eqs. (17) and (17b) are given in “Appendix 2”. We have calculated the dimensional volumetric flow rate per unit width through the nanopore [40] in the following as

$$\begin{aligned} \begin{aligned} q^{*}_{v}=\int _{0}^{a}\frac{u^{*}(r^{*}, t^{*})2\pi r^{*}{\mathrm{d}}r^{*}}{\pi a^{2}} \end{aligned} \end{aligned}$$
(18)

Introducing \(q_{v}^{*}\) as \(q^{*}_{v}={\text{Im}}\left( (q^{*}_{0})_{v}e^{i\omega t^{*}}\right)\), then

$$\begin{aligned} \begin{aligned} (q_{0}^{*})_{v}=2U_{\mathrm{HS}}a^{2}\int _{0}^{1}u_{0}(r)r{\mathrm{d}}r \end{aligned} \end{aligned}$$
(19)

We have introduced the scaling factor of the volumetric flow rate as \(U_{\mathrm{HS}}a^{2}\). Therefore, the dimensionless volume flow rate is given by

$$\begin{aligned} \begin{aligned} (q_{0})_{v}=2\int _{0}^{1}u_{0}(r)r{\mathrm{d}}r \end{aligned} \end{aligned}$$
(20)

Analytic expression for the concentration field

The concentration distribution of the electroneutral solute along the nanopore of length L is studied in the present analysis. Both ends of the nanopore are connected to a large reservoirs which is maintained with uniform concentration of neutral solute. The concentrations of the left and right reservoirs are \(c_{1}\) and \(c_{2}\) respectively where \(c_{1}>c_{2}\). The species concentration is also considered as infinitely dilute, so that the concentration gradient will not interfere with each other’s. In the absence of no chemical reaction or absorption of solute along the nanopore wall, the characteristic behaviour of the species in an isotropic medium is governed by convection–diffusion equation and is given by

$$\begin{aligned}&\frac{\partial c_{\mathrm{e}}^{*}}{\partial t^{*}}+u_{\mathrm{e}}^{*}\frac{\partial c_{\mathrm{e}}^{*}}{\partial z^{*}}=D\frac{1}{r^{*}}\frac{\partial }{\partial r^{*}}\left( r^{*}\frac{\partial c_{\mathrm{e}}^{*}}{\partial r^{*}}\right) \qquad 0\le {r}^{*}<{a-d^{*}} \end{aligned}$$
(21a)
$$\begin{aligned}&\frac{\partial c_{\mathrm{p}}^{*}}{\partial t^{*}}+u_{\mathrm{p}}^{*}\frac{\partial c_{\mathrm{p}}^{*}}{\partial z^{*}}=D\frac{1}{r^{*}}\frac{\partial }{\partial r^{*}}\left( r^{*}\frac{\partial c_{\mathrm{p}}^{*}}{\partial r^{*}}\right) \qquad a-d^{*}\le {r^{*}}\le {a} \end{aligned}$$
(21b)

where \(c_{\mathrm{e}}^{*}\) and \(c_{\mathrm{p}}^{*}\) are the concentration of the solute species in the electrolyte region and PEL, respectively. Here, D represents the diffusion coefficient of the solute species in the fluid. The non-uniformity of the velocity distribution will cause that the concentration field is no longer uniform at cross-section of the nanopore. We have considered here Chatwin approximation [41, 42] because of the linearity of Eqs. (21a) and (21b). Therefore, the the concentration field can be expressed as the sum of a linear concentration distribution and a concentration distribution caused by the periodic effect of the velocity field. The species distribution of solute can be written as follow

$$\begin{aligned} \begin{aligned} c^{*}(z^{*}, r^{*}, t^{*})= c_{1}+\frac{c_{2}-c_{1}}{L} z^{*}+c_{0}^{*}(r^{*}, t^{*}) \end{aligned} \end{aligned}$$
(22)

where \(c_{0}^{*}(r^{*}, t^{*})\) denotes the concentration distribution caused by the imposed oscillatory effect of the velocity. The boundary conditions at the two end are considered as

$$\begin{aligned} \begin{aligned} c^{*}= & {} c_{1}\quad {\text{at}}\quad z^{*}=0\\ c^{*}= & {} c_{2}\quad {\text{at}} \quad z^{*}=L \end{aligned} \end{aligned}$$
(23)

These boundary conditions of the above equation are not satisfied at the end of nanopore due to imposed flow oscillation effect \(c_{0}^{*}(r^{*}, t^{*})\). But it is observed that such approximation is still valid far from the nanopore ends [42, 43] by neglecting the end effect of a low-aspect-ratio configuration with \(a<<L\).

$$\begin{aligned}&\frac{\partial (c_{0})_{\mathrm{e}}^{*}}{\partial t^{*}}+u_{\mathrm{e}}^{*}\frac{c_{2}-c_{1}}{L}\nonumber \\&\quad =D\frac{1}{r^{*}}\frac{\partial }{\partial r^{*}}\left( r^{*}\frac{\partial (c_{0})_{\mathrm{e}}^{*}}{\partial r^{*}}\right) \qquad 0\le {r}^{*}<{a-d^{*}} \end{aligned}$$
(24a)
$$\begin{aligned}&\frac{\partial (c_{0})_{\mathrm{p}}^{*}}{\partial t^{*}}+u_{\mathrm{p}}^{*}\frac{c_{2}-c_{1}}{L}\nonumber \\&\quad =D\frac{1}{r^{*}}\frac{\partial }{\partial r^{*}}\left( r^{*}\frac{\partial (c_{0})_{\mathrm{p}}^{*}}{\partial r^{*}}\right) \qquad a-d^{*}\le {r^{*}}\le {a} \end{aligned}$$
(24b)

The boundary conditions for the above Eqs. (24a) and (24b) are given by

$$\begin{aligned} \begin{aligned}&\frac{\partial (c_{0})_{\mathrm{e}}^{*}}{\partial r^{*}}\mid _{r^{*}=0}=0, \qquad \frac{\partial (c_{0})^{*}_{\mathrm{p}}}{\partial r^{*}}\mid _{r^{*}=(a-d^{*})^{+}}=\frac{\partial (c_{0})_{\mathrm{e}}^{*}}{\partial r^{*}}\mid _{r^{*}=(a-d^{*})^{-}}\\&(c_{0})_{\mathrm{p}}^{*}\mid _{r^{*}=(a-d^{*})^{+}}= (c_{0})^{*}_{\mathrm{e}}\mid _{r^{*}=(a-d^{*})^{-}} \qquad \frac{\partial (c_{0})^{*}_{\mathrm{p}}}{\partial r^{*}}\mid _{r^{*}=a}=0 \end{aligned} \end{aligned}$$
(25)

The species concentrations \((c_{0})_{\mathrm{e}}^{*}\) and \((c_{0})_{\mathrm{p}}^{*}\) are scaled by \(\left( c_{2}-c_{1}\right)\). The species concentration is assumed to be of the form as \(c_{0}(r^{*}, t^{*})={\text{Im}}\left( g^{*}(r^{*})e^{i\omega t^{*}}\right)\) and accordingly the species conversion equations become

$$\begin{aligned}&\frac{{\mathrm{d}}^{2}g_{\mathrm{e}}}{{\mathrm{d}}r^{2}}+\frac{1}{r}\frac{{\mathrm{d}} g_{\mathrm{e}}}{{\mathrm{d}}r}-i{\text{Re}}_{w}{\text{Sc}} g_{\mathrm{e}}=\varepsilon {\text{Pe}} u_{\mathrm{e}} \qquad 0\le {r}<{1-d} \end{aligned}$$
(26a)
$$\begin{aligned}&\frac{{\mathrm{d}}^{2}g_{\mathrm{p}}}{{\mathrm{d}}r^{2}}+\frac{1}{r}\frac{{\mathrm{d}} g_{\mathrm{p}}}{{\mathrm{d}}r}-i{\text{Re}}_{w}{\text{Sc}} g_{\mathrm{p}}=\varepsilon {\text{Pe}} u_{\mathrm{p}} \qquad {1-d}\le {r}\le {1} \end{aligned}$$
(26b)

where \(g_{\mathrm{e}}\) and \(g_{\mathrm{p}}\) are, respectively, the dimensionless solute concentration in the electrolyte and PEL region. The aspect ratio is denoted as \(\varepsilon = a/L\), Schmidt number Sc is defined as \({\text{Sc}}=\nu /D\) and Pe is the Peclet number and defined by \({\text{Pe}}=U_{\mathrm{HS}}a/D\). The corresponding dimensionless boundary conditions are given as

$$\begin{aligned} \begin{aligned}&\frac{{\mathrm{d}}g_{\mathrm{e}}}{{\mathrm{d}}r}\mid _{r=0}\,=\,0, \qquad \frac{{\mathrm{d}}g_{\mathrm{p}}}{{\mathrm{d}}r}\mid _{r=(1-d)^{+}}=\frac{{\mathrm{d}}g_{\mathrm{e}}}{{\mathrm{d}}r}\mid _{r=(1-d)^{-}}\\&g_{\mathrm{p}}\mid _{r=(1-d)^{+}}= g_{\mathrm{e}}\mid _{r=(1-d)^{-}} \qquad \frac{{\mathrm{d}}g_{\mathrm{p}}}{{\mathrm{d}}r}|_{r=1}=0 \end{aligned} \end{aligned}$$
(27)

The solutions of the above Eqs. (26a) and (26b) corresponding to the boundary conditions Eq. (27) are given by

$$\begin{aligned} g_{\mathrm{e}}(r)=\, & {} G_{1}I_{0}\left( \sqrt{i{\text{Re}}_{w}{\text{Sc}}}r\right) +G_{2}K_{0} \left( \sqrt{i{\text{Re}}_{w}{\text{Sc}}}r\right) \nonumber \\&+G_{3}I_{0}\left( \sqrt{(i {\text{Re}}_{w}-\lambda _{1}{\text{Re}}_{w}^{2})}r\right) \nonumber \\&+G_{4}I_{0}\left( \kappa ar\right) \qquad 0\le {r}<{1-d} \end{aligned}$$
(28a)
$$\begin{aligned} g_{\mathrm{p}}(r)=\, & {} G_{9}I_{0}\left( \sqrt{i{\text{Re}}_{w}{\text{Sc}}}r\right) +G_{10}K_{0} \left( \sqrt{i{\text{Re}}_{w}{\text{Sc}}}r\right) \nonumber \\&+G_{5}I_{0}\left( \sqrt{\beta ^{2}+(i {\text{Re}}_{w}-\lambda _{1}{\text{Re}}_{w}^{2})}r\right) \nonumber \\&+G_{6}K_{0}\left( \sqrt{\beta ^{2}+(i {\text{Re}}_{w}-\lambda _{1}{\text{Re}}_{w}^{2})}r\right) \nonumber \\&+G_{7}I_{0}\left( \kappa a\sqrt{\frac{m}{\epsilon _{r}}}r\right) \nonumber \\&+G_{8}K_{0}\left( \kappa a\sqrt{\frac{m}{\epsilon _{r}}}r\right) \nonumber \\&+q_{\mathrm{fix}}\frac{\varepsilon (1+i\lambda _{1}{\text{Re}}_{w}){\text{Pe}}}{i\left( \beta ^{2}+(i {\text{Re}}_{w}-\lambda _{1}{\text{Re}}_{w}^{2})\right) {\text{Re}}_{w}{\text{Sc}}} {1-d}\le {r}\le {1} \end{aligned}$$
(28b)

The value of the arbitrary constants are specified in Eqs. (28a) and (28b) are given in “Appendix 3”.

For oscillatory electroosmotic flow, we have introduced the concept of tidal displacement \(\Delta z\) [19, 42]. Basically, tidal displacement represents the average moving distance of each cross section on full cycle of oscillation \(T(=2\pi /\omega )\) [19, 44] and defined as

$$\begin{aligned} \begin{aligned} \Delta z=\frac{2}{a^{2}\pi }\mid \int _{0}^{a}\int _{0}^{T}u^{*} r^{*} {\text{d}}r^{*}{\text{d}}t^{*} \mid \end{aligned} \end{aligned}$$
(29)

When the tidal displacement has to be considered the same value for each flow situation, then the imposed electric field, Helmholtz–Smoluchowski velocity and corresponding Peclet number will no longer remain constant. The Helmholtz–Smoluchowski velocity subjected into Eq. (29) can be redefined as

$$\begin{aligned} \begin{aligned} U_{\mathrm{HS}}=\frac{\Delta z \omega }{4\mid \int _{0}^{1}u_{0}r {\text{d}}r \mid } \end{aligned} \end{aligned}$$
(30)

A net mass transport exists from upstream to downstream, due to either the pure diffusion effect or the convective effect. The net mass transport is calculated based on the time and space average mass-transfer rate [19, 44, 45] for each cross-section of the tube in one period of oscillation

$$\begin{aligned} \begin{aligned} m_{\theta }^{*}=\frac{2}{a^{2}T}\int _{0}^{a}\int _{0}^{T} j_{z}r^{*}{\text{d}}r^{*}{\text{d}}t^{*} \end{aligned} \end{aligned}$$
(31)

The instant mass flux in the z-direction is denoted by \(j_{z}\) caused due to convection diffusion effects and defined as

$$\begin{aligned} \begin{aligned} j_{z}=-D\frac{c_{2}-c_{1}}{L}+\left( c_{1}+\frac{c_{2}-c_{1}}{L} z^{*}+c_{0}^{*}(r^{*}, t^{*})\right) u^{*} \end{aligned} \end{aligned}$$
(32)

Equivalently,

$$\begin{aligned} j_{z}= & {} -D\frac{c_{2}-c_{1}}{L}+c_{1}{\text{Im}}\left( u_{0}^{*}e^{i\omega t^{*}}\right) +z^{*}\frac{c_{2}-c_{1}}{L}{\text{Im}}\left( u_{0}^{*}e^{i\omega t^{*}}\right) \nonumber \\&+{\text{Im}}(g^{*}e^{i\omega t^{*}}){\text{Im}}\left( u_{0}^{*}e^{i\omega t^{*}}\right) \end{aligned}$$
(33)

The dimensionless form of Eq. (33) is given by

$$\begin{aligned} j_{z}= & {} -D\frac{c_{2}-c_{1}}{L}+U_{\mathrm{HS}}c_{1}{\text{Im}}\left( ue^{it}\right) +aU_{\mathrm{HS}}z\frac{c_{2}-c_{1}}{L}{\text{Im}}\left( u_{0}e^{it}\right) \nonumber \\&+ U_{\mathrm{HS}}\left( c_{2}-c_{1}\right) {\text{Im}}\left( ge^{it}\right) {\text{Im}}\left( u_{0}e^{it}\right) \end{aligned}$$
(34)

Introducing Eq. (34) in Eq. (31) and express \(m_{\theta }=m_{\theta }^{*}L/D\left( c_{1}-c_{2}\right)\) as dimensionless average mass transport rate in the following

$$\begin{aligned} m_{\theta }= & {} 2\int _{0}^{1} r{\text{d}}r -2\frac{{\text{Pe}}_{w}}{\epsilon }\int _{0}^{1} {\text{Im}}\left( ge^{it}\right) {\text{Im}}\left( u_{0}e^{it}\right) r{\text{d}}r \end{aligned}$$
(35)
$$\begin{aligned}= & {} 2\int _{0}^{1} r{\text{d}}r - 2\frac{{\text{Pe}}_{w}}{\epsilon }\int _{0}^{1}{\text{Re}}\left( u_{0}{\overline{g}}\right) r{\text{d}}r \end{aligned}$$
(36)

where \({\text{Re}}[.]\) denotes the real part of the product of the function \(u_{0}\) by the complex conjugate of the function g. We have redefined Peclet number based on the angular phenomena as \({\text{Pe}}_{w}=\frac{{\text{Re}}_{w}{\text{Sc}}\Delta Z}{\mid <u_{0}>\mid }\) where, \(\mid <u_{0}>\mid =\mid 4\int _{0}^{1}u_{0}r{\text{d}}r\mid\) and \(\Delta Z=\frac{\Delta z}{a}\). The dimensionless induced potential, axial velocity, average fluid velocity, concentration distribution and mass transfer rate have been evaluated numerically with MATLAB [46].

Results

In the previous section, we have established the analytic solution for the distribution of induced potential and axial velocity based on the modified Bessel function. These solutions rely some non-dimensional parameter such as oscillating Reynolds number \({\text{Re}}_{w}\), permittivity ratio \(\epsilon _{r}\), normalized relaxation time \(\lambda \omega\), Debye–Hückel parameter \(\kappa a\), softness parameter \(\beta\) and nanopore surface charge \(\zeta\) which control the electrokinetic flow dynamics for the present study. The nanopore wall has fixed zeta potential \(\phi ^{*}=\zeta ^{*}=-0.025\) V (i.e. \(\zeta =-1\)). We have considered the nanopore radius \(a=20\times 10^{-9}\) m, the thickness of the PEL \(d=0.2\), permittivity of the electrolyte solution \(\epsilon _{0}\epsilon _{\mathrm{e}}\) = \(79.8\times 8.854\times 10^{12}\) F m\(^{-1}\), density \(\rho\) = \(10^{3}\) kg/m\(^{3}\), zero shzre rate \(\eta _{0}\) = \(10^{-3}\) kg m\(^{-1}\) s\(^{-1}\), Boltzmann constant \(k_{\mathrm{B}}=1.3806\times 10^{-23}\,{\text{J}}\,{\mathrm{K}}^{-1}\), elementary charge \(e=1.602\times 10^{-19}\) C, Faraday’s constant \(F=96{,}472\) Cmol\(^{-1}\), aspect ratio \(\varepsilon = 0.001\), Peclet number \({\text{Pe}}=5000\), absolute temperature T=298 K, Helmholtz–Smoluchowski velocity \(U_{\mathrm{HS}}=0.18158\,{\text{m}}\,{\mathrm{s}}^{-1}\), and applied electric field \(E_{0}\)=\(10^{7}\) V/m. Generally, the parametric values of oscillation frequency in the applied AC electric field is \(10\le \omega \le 10^{3}\,{\mathrm{s}}^{-1}\) [39] and relaxation time is \(10^{-4}\le \lambda \le 10^{3}\,{\mathrm{s}}\) [47]. In order to ensure the fundamental assumption for undistributed EDL structure [22, 48] in steady state, the relaxation time \(\lambda\) must be smaller than the imposed oscillating frequency \(2\pi /\omega\) [39, 47]. This restriction can be expressed in the dimensionless form as \(\lambda _{1}{\text{Re}}_{w}\le 2\pi\) which implies the normalized relaxation time \(\lambda \omega \le 2\pi\). The variation of fluid velocity with time is one of the most significant characteristics in AC electroosmotic flow in a generalized Maxwell fluids through a nanopore. The instantaneous change in velocity profiles within a period of a time cycle (t varies from 0 to 2\(\pi\)) are illustrated in some profile figures at different scaled time. We have also verified the distribution of the axial velocity of EOF of a Newtonian fluid for a particular case of Maxwell fluid. We analyzed the neutral species transport phenomena through the carrier fluid without effecting the electric potential.

Velocity field and solute transport

Figure 2 depicts the scaled axial velocity and concentration profiles for different values of the Debye–Hückel parameter \(\kappa a(=5, 15, 50)\) when oscillating Reynolds number \({\text{Re}}_{w}=5\), permittivity ratio \(\epsilon _{r}=0.7\), PEL fixed charge density \(q_{\mathrm{fix}}=5\), softness parameter \(\beta =1\). First and second columns correspond to the axial velocity profiles for Newtonian \(\lambda =0\) and Maxwell fluid \(\lambda \omega =5\) respectively while third column indicates the concentration profiles corresponding to the Maxwell fluid \(\lambda \omega =5\). The axial velocity increases with the Debye–Hückel parameter \(\kappa a\) i.e., ionic concentration of the electrolyte solution for both Newtonian and Maxwell fluid but it almost remains constant for high ionic concentration. Due to the shearing-thinning effects, the magnitude of the axial velocity for Maxwell fluid is always greater than the Newtonian fluid. It is also noted in Newtonian fluid that the velocity gradient occurs near the nanopore surface wall region. The concentration is also increased with axial velocity for the Maxwell fluid.

Fig. 2
figure 2

Distribution of the dimensionless axial velocity profiles of Newtonian fluid \(\lambda =0\) (first column), Maxwell fluid \(\lambda \omega =5\) (second column) and corresponding dimensionless concentration profiles (third column) of the Maxwell fluid \(\lambda \omega =5\) for different values of Debye–Huckel parameter \(\kappa a\) \((=5,15,50)\) at different dimensionless time. Here, Schmidt number \({\text{Sc}}=2000\), oscillating Reynolds number \({\text{Re}}_{w}=5\), PEL fixed charge density \(q_{\mathrm{fix}}=5\), permittivity ratio \(\epsilon _{r}=0.7\) and softness parameter \(\beta =1\)

Full size image

The scaled axial velocity and concentration profiles are shown in Fig. 3 for different values of PEL scaled charge density \(q_{\mathrm{fix}}(=2, 5, 15)\). The surface potential of the nanopore wall is considered as \(\zeta =-1\) while the charge density of the PEL is positive. The increase in positive charge density of the PEL reduces the axial velocity both the Newtonian \(\lambda =0\) (first column) and generalized Maxwell fluid \(\lambda \omega =5\) (second column) for fixed Debye–Hückel parameter \(\kappa a=20\). Here, we considered the oscillating Reynolds number \({\text{Re}}_{w}=5\), permittivity ratio \(\epsilon _{r}=0.7\) and softness parameter \(\beta =1\). As shown in third column of Fig. 3, the increasing scaled charge density decreases concentration distribution.

Fig. 3
figure 3

Distribution of the dimensionless axial velocity profiles of Newtonian fluid \(\lambda =0\) (first column), Maxwell fluid \(\lambda \omega =5\) (second column) and corresponding dimensionless concentration profiles (third column) of the Maxwell fluid \(\lambda \omega =5\) for different values of scaled PEL fixed charge density \(q_{fix}\) \((=2,5,15)\) at different dimensionless time. Here, Schmidt number \({\text{Sc}}=2000\), oscillating Reynolds number \({\text{Re}}_{w}=5.0\), Debye–Hückel parameter \(\kappa a=20\), permittivity ratio \(\epsilon _{r}=0.7\) and softness parameter \(\beta =1\)

Full size image

Figure 4 displays the scaled axial velocity and concentration profiles for different values of permittivity ratio \(\epsilon _{r}(= 0.3, 0.6, 1.0)\) at different dimensionless time for fixed oscillating Reynolds number \({\text{Re}}_{w}=5\), PEL scaled fixed charge density \(q_{\mathrm{fix}}=5\), Debye–Hückel parameter \(\kappa a= 20\) and softness parameter \(\beta\) \(=1\). As permittivity ratio \(\epsilon _{r}\) indicates the dielectric permittivity ratio between the PEL layer and the electrolyte solution while \(\epsilon _{r}=1\) implies the same permittivity among these two adjacent layer. Due to the low dielectric permittivity of the PEL and the electrolyte solution, low counter ions penetrate in the PEL, as a result the ion-partitioning effects occurs. The axial velocity increases with the permittivity ratios and this effects is more prominent in Maxwell fluid \(\lambda \omega =5\). To analyse the influence of the permittivity ratio in concentration distribution, we plotted the concentration profiles of the generalized Maxwell fluid \(\lambda \omega =5\) in third column of Fig. 4. Similarly as axial velocity, the concentration distribution is also increased with the permittivity ratio.

Fig. 4
figure 4

Distribution of the dimensionless axial velocity profiles of Newtonian fluid \(\lambda =0\) (first column), Maxwell fluid \(\lambda \omega =5\) (second column) and corresponding dimensionless concentration profiles (third column) of the Maxwell fluid \(\lambda \omega =5\) for different values of permittivity ratio \(\epsilon _{r}\) \((=0.3,0.6,1.0)\) at different dimensionless time. Here, Schmidt number \({\text{Sc}}=2000\), oscillating Reynolds number \({\text{Re}}_{w}=5\), Debye–Hückel parameter \(\kappa a=20\), PEL scaled fixed charge density \(q_{\mathrm{fix}}=5\) and softness parameter \(\beta =1\)

Full size image

The effects of variation of softness parameter \(\beta (=1,3,5)\) on the axial velocity and concentration profiles are shown in Fig. 5 when the oscillating Reynolds number \({\text{Re}}_{w}=5\), permittivity ratio \(\epsilon _{r}=0.7\), Debye–Hückel parameter \(\kappa a=20\), PEL scaled fixed charge density \(q_{\mathrm{fix}}=5\). The scaled axial velocity decreases with the increase in the softness parameter for Newtonian fluid \(\lambda =0\) (first column) and generalized Maxwell fluid \(\lambda \omega =5\) (second column). The softness parameter mainly represents the frictional forces acting on the electrolyte solution flowing through the PEL, and it mainly effects the hydrodynamics behaviour inside the nanopore while the conductance is not affected crucially by the flow field. It is cleared form Fig. 5 (second and third column) that as axial scaled velocity decreases, the solute concentration is also decreased with the increase in softness parameter. 

The oscillating Reynolds numbers have a great impact on the fluid behaviours. To illustrate this effects, we plotted the variation of axial velocity and concentration profiles for different oscillating Reynolds number at different time for both Newtonian and generalized Maxwell fluids case when the permittivity ratio \(\epsilon _{r}=0.7\), Debye–Hückel parameter \(\kappa a=20\), PEL scaled fixed charge density \(q_{\mathrm{fix}}=5\) and softness parameter \(\beta =1\). For low oscillating Reynolds number, the viscoelasticity of the fluid does not affect the hydrodynamics practice in comparison with the Newtonian fluid (first column) and the axial velocity form plug-like profile shape in both fluids. The magnitude of the axial velocity profile of EOF decreases with the higher values of oscillating Reynolds number where as the flow oscillation increases for the generalized Maxwell fluid \(\lambda \omega =3\) as shown in second column of Fig. 6. The concentration profiles (third column) corresponding to this Maxwell fluid show that, concentration amplitude decreases but oscillation increases with the higher oscillating Reynolds number.

Fig. 5
figure 5

Distribution of the dimensionless axial velocity profiles of Newtonian fluid \(\lambda =0\) (first column), Maxwell fluid \(\lambda \omega =5\) (second column) and corresponding dimensionless concentration profiles (third column) of the Maxwell fluid \(\lambda \omega =5\) for different values of softness parameter \(\beta\) \((=1,3,5)\) at different dimensionless time. Here, Schmidt number \({\text{Sc}}=2000\), oscillating Reynolds number \({\text{Re}}_{w}=5\), Debye–Hückel parameter \(\kappa a =20\), permittivity ratio \(\epsilon _{r}=0.7\) and PEL scaled fixed charge density \(q_{\mathrm{fix}}=5\)

Full size image
Fig. 6
figure 6

Distribution of the dimensionless axial velocity profiles of Newtonian fluid \(\lambda =0\) (first column), Maxwell fluid \(\lambda \omega =3\) (second column) and corresponding dimensionless concentration profiles (third column) of the Maxwell fluid \(\lambda \omega =3\) for different values of oscillating Reynolds number \({\text{Re}}_w\) \((=0.1,20,40,60)\) at different dimensionless time. Here, Schmidt number \({\text{Sc}}=2000\), Debye–Hückel parameter \(\kappa a =20\), permittivity ratio \(\epsilon _{r}=0.7\), PEL scaled fixed charge density \(q_{\mathrm{fix}}=5\) and softness parameter \(\beta =1\)

Full size image

To show the influence of the normalized relaxation time \(\lambda \omega\), we illustrated the distribution of the axial velocity profiles (first row; a, b, c, d) and corresponding distribution of the concentration profiles (second row; e, f, g, h) for different dimensionless time in Fig. 7 when oscillating Reynolds number \({\text{Re}}_{w}=15\), permittivity ratio \(\epsilon _{r} =0.7\), Debye–Huckel parameter \(\kappa a=20\), PEL scaled fixed charge density \(q_{\mathrm{fix}}=5\) and softness parameter \(\beta =1\). For the higher value of the oscillating Reynolds number, flow oscillation occurs in both the Newtonian and generalized Maxwell fluids, but it’s occurrences are more rapid in generalized Maxwell fluid cases. The amplitude of the axial velocity increases with higher values of normalized relaxation time for generalized Maxwell fluids in different time. It is observed that the viscoelastic effects increase the axial fluid velocity due to higher fluidity. Since the oscillation time period is much smaller than the diffusion time scale and hence there is no sufficient time for the flow moment to diffuse away from the nanopore surface. As a result, the effects are only restricted only within a thin layer near the nanopore wall surface. The axial velocity and subsequent concentration distribution corresponding to the Newtonian fluid (\(\lambda =0\)) are shown in Fig. 7a and c, respectively. It can be observed from Fig. 7 that the increasing values of the relaxation time (\(\lambda \omega\)) enhance both of the solute concentration and oscillation.

Fig. 7
figure 7

Distribution of a dimensionless axial velocity profiles (first row) and b corresponding dimensionless concentration profiles (second row) for different values of normalized relaxation time \(\lambda \omega\) \((=0, 1.5, 3.0, 6.0)\) at different dimensionless time. Here, Schmidt number \({\text{Sc}}=2000\), oscillating Reynolds number \({\text{Re}}_{w}=15\), Debye–Hückel parameter \(\kappa a =20\), permittivity ratio \(\epsilon _{r}=0.7\), PEL scaled fixed charge density \(q_{\mathrm{fix}}=5\) and softness parameter \(\beta =1\)

Full size image

Volumetric flow rate, average mass transport and neutralization factor

In Fig. 8, we illustrated the scaled volumetric flow rate \((q_{0})_{v}\) as a function of normalized relaxation times \(\lambda \omega\) of Maxwell fluids for different values of permittivity ratio \(\epsilon _{r}\), PEL fixed charge density \(q_{\mathrm{fix}}\) and oscillating Reynolds number \({\text{Re}}_{w}\). The effects of the permittivity ratio on volumetric flow rate is shown in Fig. 8a when the PEL charge density \(q_{\mathrm{fix}}(=5)\), Debye–Hückel parameter \(\kappa a(=40)\) and oscillating Reynolds number \({\text{Re}}_{w} (=15)\) are fixed. The volumetric flow rate increases with permittivity ratio between PEL and electrolyte region. It has been shown in Fig. 8b that the scaled volumetric flow rate decreases with the increases of the positive charge density in PEL. We have also plotted the variation of the volumetric flow rate for different oscillating Reynolds number in Fig. 8c and it is observed that volumetric flow rate decreases with higher values of the oscillating Reynolds number. It can be seen from all theses in Fig. 8 that the volumetric flow rate has some peaks for some normalized relaxation time. Due to the elasticity, the fluid velocity decreases for some relaxation time and increases for others relaxation time.

Fig. 8
figure 8

Variation of volumetric flow rate \((q_{0})_{v}\) with different value of normalized relaxation time \(\lambda \omega\) of Maxwell fluid corresponding to different values of a permittivity ratio \(\epsilon _{r}\), b PEL scaled charge density \(q_{\mathrm{fix}}\) and c oscillating Reynolds number \({\text{Re}}_{w}\). Here, Debye–Hückel parameter \(\kappa a=40\) and softness parameter \(\beta =1\)

Full size image

The dimensionless average mass transport rate \(m_{\theta }\) is illustrated in Fig. 9a for different values of the tidal displacement \(\Delta Z\) when the Schmidt number \({\text{Sc}}=1000\) and oscillating Reynolds number \({\text{Re}}_{w}=15\) for generalized Maxwell fluids. It shows that the mass transport rate increases with normalized relaxation time. The increase in the tidal displacement intensifies the instantaneous convective and dispersion effects in the concentration field and promote the mass transport rate when Schmidt number and oscillating Reynolds number are fixed. To visualize the reasonable comparison of performance rate of the average mass transport, the tidal displacement \(\Delta Z\) has considered to be same as \(\Delta Z\) for each flow situation as shown in Fig. 9b and c. Figure 9b shows that the variation of the average mass transport rate increases with normalized relaxation times for different values of oscillating Reynolds number \({\text{Re}}_{w}(=20,40,60)\) for fixed Schmidt number \({\text{Sc}}(=1000)\) and tidal displacement \(\Delta Z(=1.0)\). It also shows that the mass transport rate decreases with the increase in the oscillating Reynold number and it is more prominent in higher values of the normalized relaxation time. The influence of Schmidt number on average mass transport rate is illustrated in Fig. 9c for fixed oscillating Reynolds number \({\text{Re}}_{w}(=15)\) and tidal displacement \(\Delta Z(=1)\). It is shown from this figure that the increase in Schmidt number enhance the mass transport rate. For small mass, diffusivity implies large Schmidt number \({\text{Sc}}\) which enhance the average mass transport rate and oscillatory EOF is more effective to intensify mass transport rate for large Schmidt number.

Fig. 9
figure 9

Variation of dimensionless average mass transport rate \(m_{\theta }\) with normalized relaxation time \(\lambda \omega\) corresponding to different values of a tidal displacement \(\Delta Z\) b oscillating Reynolds number \({\text{Re}}_{w}\) and c Schmidt number \({\text{Sc}}\). Here, Debye–Hückel parameter \(\kappa a=20\), permittivity ratio \(\epsilon _{r}=0.7\), PEL scaled charge density \(q_{\mathrm{fix}}=5\) and softness parameter \(\beta = 1\)

Full size image

To demonstrate the charge neutralization in PEL, we have calculated the neutralization factor \(\Gamma\) [49] and is defined as

$$\begin{aligned} \begin{aligned} \Gamma =1-\frac{Q_{\mathrm{eff}}}{Q_{\mathrm{fix}}} \end{aligned} \end{aligned}$$
(37)

where \(Q_{\mathrm{eff}}\) is the volume average effective charge density and defined as

$$\begin{aligned} \begin{aligned} Q_{\mathrm{eff}}=\frac{2}{\omega T}\int _{0}^{1}\int _{0}^{2\pi } \left[ (\kappa a)^{2}\exp (\Delta w_{i}) \phi +q_{\mathrm{fix}}\right] r{\text{d}}r {\text{d}}t \end{aligned} \end{aligned}$$
(38)

and \(Q_{\mathrm{fix}}\) signifies the volume average fixed charge density and defined as

$$\begin{aligned} \begin{aligned} Q_{\mathrm{fix}}=\frac{2}{\omega T}\int _{0}^{1}\int _{0}^{2\pi } q_{\mathrm{fix}} r{\text{d}}r {\text{d}}t \end{aligned} \end{aligned}$$
(39)

Figure 10a shows the variation of the neutralization factor with Debye–Hückel parameter \(\kappa a\) (ionic concentration of the electrolyte) for different values of permittivity ratio \(\epsilon _{r}\) for Maxwell fluids \(\lambda \omega =5\) when oscillating Reynolds number \({\text{Re}}_{w}=5\), PEL fixed charge density \(q_{\mathrm{fix}}=5\) and softness parameter \(\beta =1\). The charge neutralization factor \(\Gamma\) increases with the Debye–Hückel parameter \(\kappa a\) and it attains higher values when the permittivity between the PEL and electrolyte region are same, i.e., \(\epsilon _{r}=1\) as shown in Fig. 10a. This indicates that the neutralization of immobile charge occurs at a lower rate when the permittivity ratio is small in the PEL. The variation of the neutralization factor \(\Gamma\) with Debye–Hückel parameter \(\kappa a\) is illustrated in Fig. 10b for different values of PEL fixed charge density \(q_{\mathrm{fix}}\) for Maxwell fluids \(\lambda \omega =5\) when oscillating Reynolds number \({\text{Re}}_{w}=5\), permittivity ratio \(\epsilon _{r}=0.7\) and softness parameter \(\beta =1\). In Fig. 10b, it indicates that the neutralization factor \(\Gamma\) decreases with increases of the positive fixed charge density \(q_{\mathrm{fix}}\) in PEL but increases with Debye–Hückel parameter \(\kappa a\) i.e., ionic concentration of the electrolyte.

Fig. 10
figure 10

Variation of the neutralization factor \(\Gamma\) with Debye–Hückel parameters \(\kappa a\) of Maxwell fluids \(\lambda \omega =5\) for different values of a permittivity ratio \(\epsilon _{r}\) and b PEL scaled charge density \(q_{\mathrm{fix}}\). Here, oscillating Reynolds number \({\text{Re}}_{w}=5\) and softness parameter \(\beta = 1\)

Full size image

Conclusion

The motivation of the present study is to analyse the electrokinetic effects and solute transport phenomena for Maxwell fluids through the polyelectrolyte grafted nanopore considering ion-partitioning effects under AC electric field. Using Debye–Hückel approximation for linearizing modified Poisson–Boltzmann equation, an analytic expression for induced potential equation was established in the interior and exterior of the PEL. The analytic expression for axial velocity was also presented from the Cauchy momentum equation along with Maxwell constitutive equation in the interior and exterior of the PEL. The axial velocity increases with ionic concentration of the electrolyte solution but it decreases with the increase in the fixed positive charge density in the PEL for both Newtonian and Maxwell fluids cases. The increase in the permittivity ratio enhances the axial velocity for both fluids but its effects are more prominent in Maxwell fluids. The velocity decreases with the increase in the softness parameter. The analytic solution for the convection-diffusions equation was also established in full domain. For higher values of the relaxation time, the axial velocity, and species concentration are both increased. The higher values of the oscillating Reynolds number increase the flow oscillation, as a result the axial velocity decreases. The volumetric flow rate decreases with the increase in the oscillatory Reynolds number and PEL fixed positive charge density but increases with permittivity ratio and relaxation time. For Maxwell fluids, it is also noticed the undulatory distribution in axial velocity and concentration field in comparison to the Newtonian fluids. The average mass transport rate increases with tidal displacement for fixed Schmidt number and relaxation time. The average mass transport rate increases with Schmidt number as well as relaxation time. The neutralization factor increases with permittivity ratio and decreases with PEL fixed charge density.