Keywords

18.1 Propagation of the Gaussian Beam

Measuring the thickness of a thin film, supported only by the border is a difficult task as the film vibrates continuously; thus, especial techniques have been designed for this purpose [1–13]. In this report we describe a technique based on the defocusing properties of a Gaussian beam which is accurate and has the advantage of been immune to piston-like vibration of the film.

The propagation of a Gaussian beam is well-known. A brief description follows.

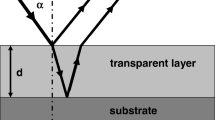
In Fig. 18.1 the system is adjusted to focus the laser beam at the plane of observation with coordinates (ξ, η) in the absence of the sample, by means of a focusing lens placed on a coordinate plane (x 1, y 1) at a distance z 0 from the laser output. For simplicity the lens is considered very thin and the aperture is large enough to allow neglecting truncation of the beam. The plane of observation is adjusted at a distance z T behind the lens to attain the best focusing conditions (minimum beam semi-width). We will refer to this plane as the best focusing plane. Once z T has been fixed, the sample with geometrical thickness t is introduced transversally to the optical axis z in the optical path. It can be seen that,

Fig. 18.1
figure 1

Propagation of the Gaussian beam . z T is set such that the plane (ξ, η) corresponds to the best focusing plane

Full size image
$$ {z}_T={z}_1+t+{z}_2, $$
(18.1)

where z 1 and z 2 are the distances depicted in Fig. 18.1 which can be chosen arbitrarily as it will be demonstrated below.

For illustrative purposes the width of the sample has been exaggerated in Fig. 18.1.

The propagation of the Gaussian beam throughout the system can be calculated by means of the Fresnel diffraction integral [14].

First, we consider a virtual sample, a slice with a refractive index equal to one and geometrical thickness t. For this condition we calculate the propagation from the laser up to the plane of observation and we plot the semi-width of the Gaussian beam as a function of distance z T . These calculations allow characterizing all the parameters involved in the propagation of the beam.

The value of z T in which the semi-width exhibits a minimum at the plane of observation will be referred as the best focusing distance and, as indicated, the corresponding transversal plane as the best focusing plane.

The calculations can be simplified by only characterizing the beam that emerges just after the lens. For this, we consider a circular amplitude distribution of the Gaussian just at the back surface of the lens as [15],

$$ {\varPsi}_1\left({x}_1,{y}_1\right)=A \exp \left(-\frac{x_1^2+{y}_1^2}{r^2}\right) \exp \left[-i\beta \left({x}_1^2+{y}_1^2\right)\right]. $$
(18.2)

In (18.2) A is a complex constant term, r is the semi-width of the Gaussian beam and β is the corresponding coefficient of the convergent quadratic phase.

The field given by (18.2) is propagated up to the plane of observation. We obtain for the corresponding semi-width (r A ) at this plane [15],

$$ {r}_A=\frac{\sqrt{\lambda^2{z_T}^2+{r}^4{\left(\beta \kern0.1em \lambda {z}_T-\pi \right)}^2}}{\pi r}. $$
(18.3)

We emphasize that (18.3) corresponds to the case of a virtual sample with a refractive index equal to one. r A , r, β and λ are determined experimentally as it will be described in Sect. 18.4. In this manner we propose a value for z T which matches with our experimental conditions and it is unique.

By maintaining z T constant, we now repeat the same calculation substituting the virtual sample by a film with a refractive index n and geometrical thickness t. The semi-width r B in this case is given as,

$$ {r}_B=\frac{\sqrt{\lambda^2{\left({z}_T-t+\frac{t}{n}\right)}^2+{r}^4{\left(\beta \kern0.1em \lambda\;\left[{z}_T-t+\frac{t}{n}\right]-\pi \right)}^2}}{\pi r}. $$
(18.4)

In the next section we describe the technique for measuring the corresponding semi-widths.

18.2 Homodyne Detector

As described in [13], the homodyne detector uses a vibrating knife-edge as depicted in Fig. 18.2. The knife-edge is placed at the detection plane. For our purposes, the lens has a working distance of 1 cm allowing the placement of the sample. An attenuator (A) is included to avoid damaging the optical components due to excessive heating. A photo diode whose sensitive area is much larger than the spot size of the beam is positioned behind the knife-edge. The knife-edge is fixed to a flexure piezoelectric transducer (PZT) to vibrate in a plane transversal to the optical axis at a low frequency (f), in our case 10 Hz, with small amplitude (δ 0) of 500 nm. The PZT is also used to displace in plane the knife-edge and has high resolution of about 50 nm. A flexure type PZT is preferred as it exhibits low tilt of less than 5 μrad.

Fig. 18.2
figure 2

Homodyne detector setup for measuring the semi-width and centroid of the transmitted Gaussian beam

Full size image

The vibrating knife-edge allows determining the semi-width of the Gaussian beam in a homodyne way as follows. First, when the knife-edge is not vibrating, due to the photodiode large sensitive area, the power collected (P) can be written as,

$$ P=A{\displaystyle \underset{\alpha }{\overset{\infty }{\int }} \exp \left(-2\frac{x^2}{r_0^2}\right)}\kern0.1em dx. $$
(18.5)

In (18.5) A is a constant scale factor and the lower limit of the integral (α) represents the initial position of the knife-edge. r 0 is the semi-width of the beam at the plane of detection. Equation 18.5 indicates that the photodiode integrates the overall beam excluding the portion covered by the knife-edge.

Now, when the knife-edge is vibrating, the lower limit is written as,

$$ \alpha ={x}_0+{\delta}_0 \cos \left(2\pi ft\right), $$
(18.6)

where x 0 is a static position. By substituting (18.6) in (18.5) and by only preserving the linear term of a series expansion of the resulting equation, we obtain,

$$ {P}_{\mathrm{linear}}\left({x}_0\right)=B \exp \left(-2\frac{{x_0}^2}{{r_0}^2}\right) \cos \left(2\pi ft\right), $$
(18.7)

where B is a constant. Only the first order term of the expansion has to be considered because the lock-in amplifier is tuned to the first harmonic. As a consequence, the signal detected by the lock-in results proportional to the intensity Gaussian profile evaluated at x 0 as indicated by (18.7).

To obtain the shape of the Gaussian beam with our homodyne detector it is necessary to displace the position of the knife-edge to different values of x 0. In this way, the profile and width of the Gaussian beam are obtained with high accuracy at the plane of detection.

In the next section we provide experimental results.

18.3 Experimental Section

The working conditions in our measurements were: \( \beta =4.971\times {10}^8\;{\mathrm{m}}^{-2} \), \( r=2.2\;\mathrm{mm} \), \( {r}_A=1.4\;\upmu \mathrm{m} \), \( {z}_T=1.0\;\mathrm{cm} \), \( \lambda =0.632\kern0.22em \upmu \mathrm{m} \). Refractive film index = 1.7. With these parameters r B can be determined. With the film sample present, \( {r}_B=8.77\;\upmu \mathrm{m} \). Figure 18.3 depicts the experimental intensity distributions.

Fig. 18.3
figure 3

Intensity distributions recorded with the homodyne detector normalized with respect to the virtual sample (continuous trace). The dashed trace corresponds to the measurement with the sample film

Full size image

The acquisition time for each profile is approximately 1 min and is a consequence of the slow vibrating rate. The acquisition time can be diminished by increasing the frequency vibration of the knife-edge. For the moment being we are working in this direction.

18.4 Conclusions

A technique for measuring the geometrical thickness of a semi-transparent thin film by means of the diffractive properties of a transmitted Gaussian beam has been described and tested. The technique is based on measuring the semi-width of the transmitted beam. We demonstrated that the technique is immune to piston-like vibrations of the film and as it is not interferometric, it showed to be immune to external noise. The technique was applied in determining experimentally the geometrical thickness of a commercially available stretch film.