The Loss Tangent of Visco-Elastic Models

Abstract

When modelling visco-elasticity, be it linearly (standard linear solid with two springs and one damper) or non-linearly (power and log models), it is important to know on which model parameters the viscous energy loss depends on. In this paper, the dependency of the loss tangent (tan δ, ratio of loss modulus to storage modulus) and the phase angle δ on elasticity E and viscosity η parameters and on the excitation frequency f is derived and evaluated in three visco-elastic models. In the Zener model (standard linear solid of Voight form), tan δ and δ depend on E, η, and f. f and η are linked together and always occur as the product fη. Tan δ is smaller than π/2. The transient part of the stress function is an exponential function; the steady state part comprises of sine and cosine functions. In the power model, tan δ and δ depend on η only; (0 ≤ η < 1). η has no relationship with f in tan δ. Tan δ is smaller than π/2. The transient part of the stress function is a Maclaurin series; the steady state part is a sine function with ηπ/2 phase shift. In the log model, tan δ and δ depend on E, η, and f; but at the same f, larger E/η have larger tan δ and δ. The viscosity constant appears as a stand alone η, and as the product of η and log 2πf. Tan δ can be larger than π/2 at small E/η (high viscosity) and small frequencies (large cycle periods with small strain rates). The transient part of the stress function comprises of cosine and sine integrals; steady state part consists of sine and cosine functions.

1 Introduction

Visco-elastic models are characterised by phenomena such as

• Velocity dependency: increase of stiffness with strain rate,

• Stress relaxation: decay of stress with time at constant strain,

• Creep: increase of strain with time at constant stress, and

• Loss of stored (elastic) energy due to inner friction resulting in unequal loading and unloading stress–strain curves, the area between the two curves corresponding to the dissipated energy (hysteresis).

Visco-elastic models can be classified in various ways, e.g.

• (1) Linear or non-linear models

  • Linear models with at least one elastic and one viscous element in parallel or in series;

  • QLV/quasi-linear “viscous” models such as Prony series (Wiechert model; Wiechert 1889, 1893);

  • Non-linear models such as logarithmic and power law models.

  or

• (2) According to the decay function of stress relaxation, which can be

  • Exponential: linear three-element model (standard linear solid),

  • Power: non-linear power law model, or

  • Logarithmic: non-linear logarithmic law model

Examples for the latter two non-linear models are:

• Power law model: biological tissues such as ligaments (Provenzano et al. 2001), foams with non-negative stiffness (Fuss 2009), cork in cricket balls (Fuss 2008a, 2012);

• Logarithmic law model: solid polymers (Findley et al. 1989), polymer golf balls (Fuss 2008b, 2012).

The loss tangent, tan δ, is defined as the tangent of the phase angle δ, which, in turn, is the ratio of loss modulus E″ to storage modulus E′.

$$ \tan \delta =\frac{E^{{\prime\prime} }}{E^{\prime }} $$

(6.1)

where

$$ {E}^{\prime }=\frac{\sigma_0}{\varepsilon_0} \cos \delta $$

(6.2)

$$ {E}^{{\prime\prime} }=\frac{\sigma_0}{\varepsilon_0} \sin \delta $$

(6.3)

and σ ₀ and ε ₀ are the peak amplitudes of stress σ and strain ε, respectively.

The complex modulus E ^* is defined as

$$ {E}^{*}=\frac{\sigma_0}{\varepsilon_0}{\mathrm{e}}^{i\delta}=\frac{\sigma_0}{\varepsilon_0}\left( \cos \delta +i \sin \delta \right)={E}^{\prime }+i{E}^{{\prime\prime} }=\left|{E}^{*}\right|{\mathrm{e}}^{i\delta} $$

(6.4)

where $i=\sqrt{-1}$, and |E ^*| is the dynamic modulus, the magnitude of E ^*, i.e. the resultant of loss modulus E″ and storage modulus E′

$$ \left|{E}^{*}\right|=\frac{\sigma_0}{\varepsilon_0} $$

(6.5)

The loss tangent, tan δ, is usually determined by subjecting a material or structure to sinusoidal strain ε

$$ \varepsilon ={\varepsilon}_0 \sin \left(2\uppi ft\right) $$

(6.6)

where f is the cyclic frequency (angular frequency ω = 2πf). The resulting reaction stress σ is equally sinusoidal, but out of phase with respect to the strain by the phase angle δ

$$ \sigma ={\sigma}_0 \sin \left(2\uppi ft+\delta \right) $$

(6.7)

A positive phase angle δ causes the stress peak to occur earlier than the strain peak (Fig. 6.1), resulting in the typical hysteresis effect of visco-elastic materials when plotting stress against strain (Fig. 6.2). The area of the hysteresis loop corresponds to the energy dissipated into the material as thermal energy.

Fig. 6.1

Sinusoidal strain curve (blue) imposed on a visco-elastic material and resulting stress curve (pink); σ₀: stress amplitude, maximal stress; ε₀: strain amplitude, maximal strain; δ: phase shift

Fig. 6.2

Hysteresis loop of stress–strain ellipse; σ₀: maximal stress; ε₀: maximal strain; δ: phase angle

When subjecting a material to cyclic (sinusoidal) strain, the peak stress, σ ₀, increases during the first cycles (transient part) until it reaches an equilibrium or steady state (Fig. 6.3). The phase angle δ is measured once the steady state has set in.

Fig. 6.3

Stress–time curve (red) during the first load cycles; blue curve: transient component; green curve: steadys state component; the stress–time curve (red) is the sum of transient and steady state components

It is evident, that the energy dissipated by inner friction depends on the viscosity parameter η. However, as the loss tangent is the ratio of loss to storage modulus, the strain rate independent elasticity parameter E is expected to influence the loss tangent too. Lastly, as the modulus (Young’s and tangent) increases with strain rate and thus with frequency f, the latter could contribute to the loss tangent as well.

The objective of this Book Chapter is to explore in how far the viscosity parameter η, the strain rate independent elasticity parameter E and the strain frequency f affect the loss tangent. The aim is to derive the loss tangent at steady state of the three visco-elastic models mentioned above, in order to understand the interaction between elasticity, viscosity and frequency and their effect on energy loss. The function of stress relaxation with time is not the only difference between the three models mentioned above. A further objective of this paper is to analyse the basic differences of these models and to understand their applicability and constraints.

2 Analysis

2.1 The Standard Linear Solid (Zener Model)

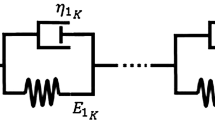
The standard linear solid (SLS of Voight form) consists of two Hookean springs and a viscous damper, where the spring with the modulus E ₁ is connected in series with a Kelvin–Voight model, with spring of modulus E ₂ and damper of viscosity constant η connected in parallel (Fig. 6.2).

From Fig. 6.4

Fig. 6.4

Standard linear solid of Voight form; σ: stress; ε: strain; η: viscosity constant; E ₁: modulus of series spring; E ₂: modulus of parallel spring; ε ₁: strain of series spring; ε ₂: strain of Kelvin–Voight model

$$ \varepsilon ={\varepsilon}_1+{\varepsilon}_2 $$

(6.8)

$$ \sigma ={\varepsilon}_1{E}_1 $$

(6.9)

$$ \sigma ={\varepsilon}_2{E}_2+{\dot{\varepsilon}}_2\eta $$

(6.10)

Taking the Laplace transform of Eqs. (6.8)–(6.10), eliminating ε ₁ and ε ₂ by substitution, and solving for $\widehat{\sigma}$ yields the constitutive equation of the standard linear solid

$$ \widehat{\sigma}=\widehat{\varepsilon}\frac{E_1{E}_2+ s\eta {E}_1}{E_1+{E}_2+ s\eta} $$

(6.11)

where the caret (^) denotes the transformed parameter.

The equation for stress relaxation results from applying a constant strain ε _c to the model through a Heaviside function H(t)

$$ \varepsilon ={\varepsilon}_c\mathrm{H}(t) $$

(6.12)

the Laplace transform of which is

$$ \widehat{\varepsilon}=\frac{\varepsilon_c}{s} $$

(6.13)

By substituting Eq. (6.13) into Eq. (6.11), we obtain

$$ \widehat{\sigma}={\varepsilon}_c\frac{E_1{E}_2+ s\eta {E}_1}{s\!\left({E}_1+{E}_2+ s\eta \right)} $$

(6.14)

the inverse Laplace transform of which yields the function of stress relaxation

$$ \frac{\sigma }{\varepsilon_c}={E}_1\frac{E_2+{E}_1{\mathrm{e}}^{-t\frac{\left({E}_1+{E}_2\right)}{\eta }}}{E_1+{E}_2} $$

(6.15)

where the stress σ is normalised to the constant strain ε _c . Equation (6.15) proves the exponential decay of stress with time, as mentioned above in the Introduction.

The loss tangent results from applying the sinusoidal strain of Eq. (6.6) to the constitutive Eq. (6.11). The Laplace transform of Eq. (6.6) is

$$ \widehat{\varepsilon}={\varepsilon}_0\frac{2\uppi f}{s^2+{\left(2\uppi f\right)}^2} $$

(6.16)

where ε ₀ is the peak amplitude of the strain.

By substituting Eq. (6.16) into the constitutive Eq. (6.11), we obtain

$$ \widehat{\sigma}={\varepsilon}_0\left(\frac{2\uppi f}{s^2+{\left(2\uppi f\right)}^2}\right)\left(\frac{E_1{E}_2+ s\eta {E}_1}{E_1+{E}_2+ s\eta}\right) $$

(6.17)

After rearranging

$$ \widehat{\sigma}={\varepsilon}_02\uppi f{E}_1\frac{E_2+ s\eta}{s^3\eta +{s}^2{E}_1+{s}^2{E}_2+ s\eta {\left(2\uppi f\right)}^2+{E}_1{\left(2\uppi f\right)}^2+{E}_2{\left(2\uppi f\right)}^2} $$

(6.18)

the inverse Laplace transform of Eq. (6.18) yields

$$ \sigma ={\varepsilon}_0{E}_1\frac{-{E}_1\eta 2\uppi f{\mathrm{e}}^{-t\frac{E_1+{E}_2}{\eta }}+{E}_1\eta 2\uppi f \cos \left(2\uppi ft\right)+\left({E}_1{E}_2+{E_2}^2+{\eta}^2{\left(2\uppi f\right)}^2\right) \sin \left(2\uppi ft\right)}{{E_1}^2+2{E}_1{E}_2+{E_2}^2+{\eta}^2{\left(2\uppi f\right)}^2} $$

(6.19)

The numerator of Eq. (6.19) comprises of a transient part (exponential function) and the steady state part (sine and cosine functions). At large times, if t → ∞, the transient part, i.e. the exponential term of the numerator, vanishes and the steady state sets in:

$$ \sigma =\frac{\varepsilon_0{E}_1}{{\left({E}_1+{E}_2\right)}^2+{\eta}^2{\left(2\uppi f\right)}^2}\left[{E}_1\eta 2\uppi f \cos \left(2\uppi ft\right)+\left({E}_1{E}_2+{E_2}^2+{\eta}^2{\left(2\uppi f\right)}^2\right) \sin \left(2\uppi ft\right)\right] $$

(6.20)

In order to obtain the loss tangent and the peak stress σ ₀, we apply the addition rules to Eq. (6.7)

$$ \sigma ={\sigma}_0 \sin \left(\delta \right) \cos \left(2\uppi ft\right)+{\sigma}_0 \cos \left(\delta \right) \sin \left(2\uppi ft\right) $$

(6.21)

where the unknowns are the two components of the peak stress σ ₀: σ ₀ sin δ and σ ₀ cos δ.

The loss tangent thus results from the ratio of the two components

$$ \tan \delta =\frac{\sigma_0 \sin \delta }{\sigma_0 \cos \delta } $$

(6.22)

and the resultant of the peak stress σ ₀ is obtained from

$$ {\sigma}_0=\sqrt{{\left({\sigma}_0 \sin \delta \right)}^2+{\left({\sigma}_0 \cos \delta \right)}^2} $$

(6.23)

From Eq. (6.20) it follows that

$$ {\sigma}_0 \sin \delta =\frac{\varepsilon_0{E}_1}{{\left({E}_1+{E}_2\right)}^2+{\eta}^2{\left(2\uppi f\right)}^2}{E}_1\eta 2\uppi f $$

(6.24)

and

$$ {\sigma}_0 \cos \delta =\frac{\varepsilon_0{E}_1}{{\left({E}_1+{E}_2\right)}^2+{\eta}^2{\left(2\uppi f\right)}^2}\left({E}_1{E}_2+{E_2}^2+{\eta}^2{\left(2\uppi f\right)}^2\right) $$

(6.25)

The loss tangent thus is

$$ \tan \delta =\frac{E_1\eta 2\uppi f}{E_1{E}_2+{E_2}^2+{\eta}^2{\left(2\uppi f\right)}^2} $$

(6.26)

and the peak stress σ ₀ results from

$$ {\sigma}_0=\frac{\varepsilon_0{E}_1}{{\left({E}_1+{E}_2\right)}^2+{\eta}^2{\left(2\uppi f\right)}^2}\sqrt{{E_1}^2{\eta}^2{\left(2\uppi f\right)}^2+{\left({E}_1{E}_2+{E_2}^2+{\eta}^2{\left(2\uppi f\right)}^2\right)}^2} $$

(6.27)

In both Eqs. (6.26) and (6.27) f and η are linked together and always occur as the product fη.

As the maximal strain rate ${\dot{\varepsilon}}_0$ of a sinusoidal strain function equals ε ₀2πf, the strain rate dependency of σ ₀ is given by

$$ {\sigma}_0=\frac{\varepsilon_0{E}_1}{{\left({E}_1+{E}_2\right)}^2+{\eta}^2{\left(\frac{{\dot{\varepsilon}}_0}{\varepsilon_0}\right)}^2}\sqrt{{E_1}^2{\eta}^2{\left(\frac{{\dot{\varepsilon}}_0}{\varepsilon_0}\right)}^2+{\left[{E}_1{E}_2+{E_2}^2+{\eta}^2{\left(\frac{{\dot{\varepsilon}}_0}{\varepsilon_0}\right)}^2\right]}^2} $$

(6.28)

The peak stress σ ₀ increases with f and η. If f or η → ∞, σ ₀ asymptotes to ε ₀ E ₁. If f or η → 0, σ ₀ → ε ₀(E ₁ ^–1 + E ₂ ^–1)^–1. The limits of σ ₀ are evident when considering that the peak strain rate changes with f. Thus, at zero strain rate or zero η, the standard linear solid reduces to two springs in series and the effective modulus of the model becomes (E ₁ ^–1 + E ₂ ^–1)^–1. At infinite strain rate or infinite η, the damper becomes rigid and the effective modulus of the model is just E ₁.

The peak stress σ ₀ increases with E ₁. If f or η → ∞ and E ₁ → 0 or ∞, both the effective modulus and σ ₀ become equally 0 or ∞, respectively. If f or η → 0 and E ₁ → 0 or ∞, the effective modulus and σ ₀ become zero in the former case, and E ₂ and ε ₀ E ₂ in the latter.

If E ₂ → 0, the standard linear solid reduces to a Maxwell model (with a spring and a damper in series), the modulus of which is (1) ∞ or (2) E ₁ or (3) 0, if (1) E ₁, and η or f, are ∞ or (2) η or f are ∞ or (3) E ₁, and η or f are 0, respectively.

At η > 0 and f < ∞, and E ₂ → 0, the peak stress reaches

$$ {\sigma}_0=\frac{2\uppi f{\varepsilon}_0{E}_1\eta }{\sqrt{{E_1}^2+{\eta}^2{\left(2\uppi f\right)}^2}} $$

(6.29)

If E ₂ → ∞, the modulus of the standard linear solid reduces to E ₁. σ ₀ shows a local minimum at a certain $\mathop{E_{2}\!}\mathop{}\limits_{0}$ (Fig. 6.5)

$$ {E}_{2_0}=\frac{\sqrt{{E_1}^2+4{\eta}^2{\left(2\uppi f\right)}^2}-{E}_1}{2} $$

(6.30)

resulting from equating the first E ₂ derivative of Eq. (6.27) with zero and solving for E ₂. Equation (6.30) is the only positive and real result of the fourth order polynomial nature of the first E ₂ derivative of Eq. (6.27).

Fig. 6.5

Maximal stress σ₀ against modulus E ₂ of parallel spring; f: frequency; η: viscosity constant; $\mathop{E_{2}\!}\mathop{}\limits_{0}$: E ₂ at which a local minimum of σ ₀ is found

The loss tangent, Eq. (6.26), reveals that the phase angle δ depends on all four parameters, E ₁, E ₂, η, and f, where the latter two always occur as the product fη.

If the frequency f → ∞ or 0, the phase angle δ → 0 in both cases. Thus, we expect a local maximum of δ at a certain frequency f ₀ (actually shown by Findley et al. 1989). Taking the first derivative of tan δ with respect to f in Eq. (6.26), equating it with zero and solving for f provides

$$ {f}_0=\frac{\sqrt{E_1{E}_2+{E_2}^2}}{2\uppi \eta } $$

(6.31)

Replacing f by f ₀ in Eq. (6.26),

$$ {\left( \tan \delta \right)}_{f_0}=\frac{E_1}{2\sqrt{E_1{E}_2+{E_2}^2}} $$

(6.32)

and equating its denominator with zero yields the scenario at which δ = 0.5 π, which is E ₁ = –E ₂ and E ₂ = 0. In both cases, however, f ₀ = 0, which means that a standard linear solid can never reach δ = 0.5π or an infinite loss tangent.

Rearranging Eqs. (6.31) and (6.32) shows that f ₀ depends on two ratios, R ₁ and R ₂, whereas tan δ at f ₀ (but also any other f at the same R ₁) depends only on R ₂ (Fig. 6.6)

$$ {f}_0=\frac{R_1}{2\uppi} $$

(6.33)

$$ {\left( \tan \delta \right)}_{f_0}=\frac{R_2}{2\sqrt{1+{R}_2}} $$

(6.34)

where

$$ {R}_1=\frac{E_2\sqrt{1+{R}_2}}{\eta } $$

(6.35)

and

Fig. 6.6

Phase angle δ against frequency f; η: viscosity constant; E ₁ and E ₂: moduli of series and parallel spring; f ₀: f at which a local maximum of δ is found

$$ {R}_2=\frac{E_1}{E_2} $$

(6.36)

Equation (6.34) shows that tan δ at f ₀ is a constant and independent of f ₀ (Fig. 6.6).

Changing η has the same effect as changing f has. If η → ∞ or 0, the phase angle δ → 0 in both cases and we obtain a local maximum of δ at a certain η ₀

$$ {\eta}_0=\frac{\sqrt{E_1{E}_2+{E_2}^2}}{2\uppi f} $$

(6.37)

Equation (6.37) is similar to Eq. (6.31) and the same principles apply.

The phase angle δ and tan δ increase with E ₁. If E ₁ → 0, tan δ → 0. If E ₁ → ∞, tan δ asymptotes to

$$ \underset{E_1\to \infty }{ \lim}\left( \tan \delta \right)=\frac{\eta 2\uppi f}{E_2} $$

(6.38)

The phase angle δ and tan δ decrease with increasing E ₂, asymptoting to 0 if E ₂ → ∞. If E ₂ → 0, tan δ reaches a limit of

$$ \underset{E_2\to 0}{ \lim}\left( \tan \delta \right)=\frac{E_1}{\eta 2\uppi f} $$

(6.39)

Lakes (2009) derived the loss tangent of the SLS of Maxwell form (with a spring connected in parallel with a Maxell model).

2.2 The Power Law Model

The power law model is characterised by a power decay of stress σ with time t:

$$ \frac{\sigma }{\varepsilon_c}=E{t}^{-\eta } $$

(6.40)

Stress σ is normalised to the constant strain ε _c applied by the Heaviside function H(t) of Eq. (6.12).

Taking Laplace transform of Eq. (6.40) yields

$$ \widehat{\sigma}={\varepsilon}_cE\frac{\Gamma \left(-\eta +1\right)}{s^{-\eta +1}} $$

(6.41)

where Γ denotes the Gamma function (Fuss 2008a, 2012).

By substituting Eq. (6.13) into Eq. (6.41), we obtain the constitutive equation of the power law of non-linear visco-elasticity (Fuss 2008a, 2012):

$$ \widehat{\sigma}={s}^{\eta}\widehat{\varepsilon}E\Gamma \left(-\eta +1\right) $$

(6.42)

By substituting the sinusoidal strain of Eq. (6.16) into the constitutive Eq. (6.42), we obtain

$$ \widehat{\sigma}={\varepsilon}_0E\Gamma \left(-\eta +1\right){s}^{\eta}\frac{2\uppi f}{s^2+{\left(2\uppi f\right)}^2} $$

(6.43)

Taking the inverse Laplace transform of Eq. (6.43), we obtain a general fractional derivative of the sine function

$$ \sigma ={\varepsilon}_0E\Gamma \left(-\eta +1\right)\frac{{\mathrm{d}}^{\eta }}{\mathrm{d}{t}^{\eta }} \sin \left(2\uppi ft\right) $$

(6.44)

where σ is the ηth time derivative of the strain function of Eq. (6.6) times a constant.

The generalised solution of $\frac{{\mathrm{d}}^{\eta }}{\mathrm{d}{t}^{\eta }} \sin (t)$ results from applying the inverse operation of the Riemann–Liouville fractional integration (with lower limit = 0),

$$ \frac{{\mathrm{d}}^{\eta }}{\mathrm{d}{t}^{\eta }} \sin (t)= \sin \left(t+\eta \frac{\pi }{2}\right)+\left[\frac{t^{-1-\eta }}{\Gamma \left(-\eta \right)}-\frac{t^{-3-\eta }}{\Gamma \left(-\eta -2\right)}+\dots \right], $$

(6.45)

i.e. Eq. 6.10.3 of Oldham and Spanier (1974)

consisting of steady state (sine function) and transient parts (Maclaurin series in square brackets). If η → 0 or 1, the denominators of the transient part approach ± ∞ (Gamma function of negative integers), the transient part reduces to 0, and a sine or cosine function, respectively, remains.

By replacing the lower limit of the inverse operation of the Riemann–Liouville fractional integration by –∞, which equals the inverse operation of the Weyl integral (Weyl 1917), we obtain the steady state part of the fractional derivative of Eq. (6.44)

$$ \sigma ={\varepsilon}_0E\Gamma \left(-\eta +1\right){\left(2\uppi f\right)}^{\eta } \sin \left(2\uppi ft+\eta \frac{\uppi}{2}\right) $$

(6.46)

Comparing Eq. (6.7) with the steady state Eq. (6.46), it becomes evident that the phase angle δ is

$$ \delta =\eta \frac{\uppi}{2} $$

(6.47)

the loss tangent is

$$ \tan \delta = \tan \left(\eta \frac{\uppi}{2}\right) $$

(6.48)

and the peak stress σ ₀ is

$$ {\sigma}_0={\varepsilon}_0E\Gamma \left(-\eta +1\right){\left(2\uppi f\right)}^{\eta } $$

(6.49)

The loss tangent depends solely on η, independent of E and f. If η → 1, both tan δ and σ ₀ → ∞, as both tan(0.5π) and Γ(0) are infinite. Thus, 0 ≤ η < 1.

The peak stress σ ₀ increases non-linearly with f and linearly with E and f ^η. The peak stress σ ₀ decreases non-linearly with η, reaching a limit of ε ₀ E, i.e. a Hookean spring, if η → 0. If η → 1, σ ₀ reaches infinity.

As the maximal strain rate ${\dot{\varepsilon}}_0$ of a sinusoidal strain function equals ε ₀2πf, the strain rate dependency of σ ₀ of Eq. (6.49) is given by

$${\sigma}_0={\varepsilon}_0\frac{E\Gamma \left(-\eta +1\right)}{{\left(2\uppi f\right)}^{1-\eta }}{\left(2\uppi f\right)}^{\eta }{\left(2\uppi f\right)}^{1-\eta }={\dot{\varepsilon}}_0\frac{E\Gamma \left(-\eta +1\right){\varepsilon_0}^{1-\eta }}{{{\dot{\varepsilon}}_0}^{1-\eta }}={{\dot{\varepsilon}}_0}^{\eta }E\Gamma \left(-\eta +1\right){\varepsilon_0}^{1-\eta}$$

(6.50)

In contrast to the fractional derivative approach shown above, Lakes (2009) solved the loss tangent of the power model from the ratio of the constitutive equations of loss to storage modulus.

2.3 The Logarithmic Law Model

The logarithmic law model is characterised by a logarithmic decay of stress σ with time t:

$$ \frac{\sigma }{\varepsilon_c}=E-\eta \log (t) $$

(6.51)

where “log” denotes the natural logarithm. Stress σ is normalised to the constant strain ε _c applied by the Heaviside function H(t) of Eq. (6.12).

Taking Laplace transform of Eq. (6.51) yields

$$ \widehat{\sigma}={\varepsilon}_c\frac{E}{s}-{\varepsilon}_c\eta \left(-\frac{\upgamma}{s}-\frac{ \log s}{s}\right) $$

(6.52)

where γ denotes the Euler–Mascheroni constant, i.e. 0.577215665… (Fuss 2008b, 2012).

By substituting Eq. (6.13) into Eq. (6.52), we obtain the constitutive equation of the logarithmic law of non-linear visco-elasticity (Fuss 2008b, 2012):

$$ \widehat{\sigma}=\widehat{\varepsilon}E+\widehat{\varepsilon}\eta \left(\upgamma + \log s\right) $$

(6.53)

By substituting the sinusoidal strain of Eq. (6.16) into the constitutive Eq. (6.53), we obtain

$$ \widehat{\sigma}=\frac{2{\varepsilon}_0\pi f}{s^2+{\left(2\pi f\right)}^2}\left(E+\upgamma \eta +\eta \log s\right) $$

(6.54)

after rearranging

$$ \widehat{\sigma}={\varepsilon}_0E\frac{2\pi f}{s^2+{\left(2\pi f\right)}^2}-{\varepsilon}_0\eta \left(-\frac{\upgamma}{s}-\frac{ \log s}{s}\right)\left(\frac{s2\pi f}{s^2+{\left(2\pi f\right)}^2}\right) $$

(6.55)

and taking inverse Laplace transform, we obtain

$$ \sigma ={\varepsilon}_0E \sin \left(2\pi f t\right)-{\varepsilon}_0\eta 2\pi f\left[ \log t\right]^*\left[ \cos \left(2\pi f t\right)\right] $$

(6.56)

where * denotes a convolution.

Applying the convolution integral, the convolution log (t) * cos (ωt), where ω = 2πf, is solved accordingly:

$$ \left[ \log t\right]*\left[ \cos \left(\omega t\right)\right]={\displaystyle {\int}_0^t \log \left(\tau \right) \cos \left[\omega \left(t-\tau \right)\right]}\mathrm{d}\tau $$

(6.57)

where τ is the dummy variable of the convolution integral.

Decomposition of cos(ωt – ωτ) according to the addition rules and subsequent partial integration yields:

$$ \left[ \log t\right]\!{*}\left[ \cos \left(\omega t\right)\right]\!{=}{\omega}^{-1}{\left[\mathrm{Ci}\left(\omega \tau \right) \sin \left(\omega t\right){-}\mathrm{Si}\left(\omega \tau \right) \cos \left(\omega t\right){-} \log \left(\tau \right) \sin \left(\omega t{-}\omega \tau \right)\right]}_0^t $$

(6.58)

where Ci and Si denote cosine and sine integrals respectively (definition of Ci and Si according to Abramowitz and Stegun 1972).

Solving Eq. (6.58) from 0 to t:

$$ \begin{array}{l}\left[ \log t\right]^*\left[ \cos \left(\omega t\right)\right]={\omega}^{-1}\Big[\mathrm{Ci}\left(\omega t\right) \sin \left(\omega t\right)-\mathrm{Si}\left(\omega t\right) \cos \left(\omega t\right)- \log (t) \sin (0)-\\ {}\kern8.5em \mathrm{Ci}\left(\omega 0\right) \sin \left(\omega t\right)+\mathrm{Si}\left(\omega 0\right) \cos \left(\omega t\right)+ \log (0) \sin \left(\omega t\right)\Big]\end{array} $$

(6.59)

Apparently, Eq. (6.59) contains an indeterminate form, as both log(0) and Ci(0) are, and thus the term log(0) sin(ωt) – Ci(ω0) sin(ωt), or sin(ωt) [log(0) – Ci(0)], delivers sin(ωt) (∞–∞).

However,

$$ \mathrm{Cin}(t)=\upgamma + \log (t)-\mathrm{Ci}(t) $$

(6.60)

where Cin denotes an alternative cosine integral (definition of Cin according to Schelkunoff 1944). As Cin(0) = 0, log(0) – Ci(0) = –γ.

Yet, the argument of the cosine integral in Eq. (6.58) is ωτ, in contrast to the one of the natural logarithm, which is just τ. Thus we have to consider the multiplier ω. This multiplier leads to a convergence constant other than simply –γ, if t → 0.

$$ \mathrm{Ci}\left(\omega t\right)=\upgamma + \log \left(\omega t\right)-\mathrm{Cin}\left(\omega t\right)=\upgamma + \log (t)+ \log \left(\omega \right)-\mathrm{Cin}\left(\omega t\right) $$

(6.61)

As Cin(0) = 0,

$$ \log (0)-\mathrm{Ci}(0)=-\upgamma - \log \left(\omega \right) $$

(6.62)

Thus,

$$ \underset{t\to 0}{ \lim}\left[ \log (t)-\mathrm{Ci}\left(\omega t\right)\right]=- \log \left(\omega \right)-\upgamma $$

(6.63)

Equation (6.59) can now be solved, considering that Si(0) = 0

$$ \left[ \log t\right]\!{^*}\!\left[ \cos \left(\omega t\right)\right]{=}{\omega}^{-1}\left\{\mathrm{Ci}\left(\omega t\right) \sin \left(\omega t\right){-}\mathrm{Si}\left(\omega t\right) \cos \left(\omega t\right){-} \sin \left(\omega t\right)\left[ \log \left(\omega \right)\!{+}\upgamma \right]\right\} $$

(6.64)

The solution of Eq. (6.56) after taking inverse Laplace follows

$$ \sigma {=}{\varepsilon}_0E \sin \left(\omega t\right){-}{\varepsilon}_0\eta \left\{\mathrm{Ci}\left(\omega t\right) \sin \left(\omega t\right){-}\mathrm{Si}\left(\omega t\right) \cos \left(\omega t\right){-} \sin \left(\omega t\right)\left[\left( \log \left(\omega \right){+}\upgamma \right)\right]\right\} $$

(6.65)

The steady state of Eq. (6.65) sets in at large times, or t → ∞. The transient part of Eqs. (6.64) and (6.65) comprises of the cosine and sine integrals. When considering the values at infinity of cosine and sine integrals, which are 0 and 0.5 π respectively, the convolution of Eqs. (6.64) and (6.65) yields at large times (steady state equation)

$$ \underset{t\to \infty }{ \lim}\left[ \log (t)\ast \cos \left(\omega t\right)\right]={\omega}^{-1}\left\{-\frac{\pi }{2} \cos \left(\omega t\right)- \sin \left(\omega t\right)\left[ \log \left(\omega \right)+\upgamma \right]\right\} $$

(6.66)

and

$$ \sigma ={\varepsilon}_0E \sin \left(\omega t\right)+{\varepsilon}_0\eta \frac{\pi }{2} \cos \left(\omega t\right)+{\varepsilon}_0\eta \sin \left(\omega t\right)\left[ \log \left(\omega \right)+\upgamma \right] $$

(6.67)

respectively.

After rearranging according to the procedure applied for determining the loss tangent of the standard linear solid, we obtain

$$ \tan \delta =\left(\frac{\pi }{2}\right)\frac{\eta }{E+\eta \left[ \log \left(2\pi f\right)+\upgamma \right]} $$

(6.68)

and

$$ {\sigma}_0={\varepsilon}_0\sqrt{0.25{\eta}^2{\pi}^2+{\left[E+\eta \log \left(2\pi f\right)+\eta \upgamma \right]}^2} $$

(6.69)

As the maximal strain rate ${\dot{\varepsilon}}_0$ of a sinusoidal strain function equals ε ₀2πf, the strain rate dependency of σ ₀ after rearranging Eq. (6.69) is given by

$$ {\sigma}_0={\varepsilon}_0\sqrt{0.25{\eta}^2{\pi}^2+{\left[E+\eta \log \left(\frac{{\dot{\varepsilon}}_0}{\varepsilon_0}\right)+\eta \upgamma \right]}^2} $$

(6.70)

The stress amplitude σ ₀ increases with E, η, and f. This fact is obvious, as E is the strain rate independent elasticity parameter, i.e. the modulus or stiffness; the viscosity parameter η is linked to the strain rate, i.e. at a given strain rate, σ increases with η; and the frequency f is linearly proportional to the strain rate applied periodically to the model, i.e. at a given η, σ increases with f.

If η → 0, σ ₀ → ε ₀ E, the stress equation of a Hookean spring. If η → ∞, σ ₀ → ∞.

If f → 0 or ∞, σ ₀ → ∞. This means that σ ₀ has a local minimum at a certain frequency f ₀ (Fig. 6.7). As the first frequency derivative of Eq. (6.69) contains the arguments f, log(2πf), and log²(2πf), there is no closed-form analytical solution, and the frequency, at which σ ₀ is at a minimum, can only be obtained numerically. Figure 6.7 shows that σ ₀ increases with η, and f ₀ increases with the ratio η/E. The ratio η/E is identical to the viscosity of a log law model, not to be confused with the viscosity constant η.

Fig. 6.7

Peak stress σ₀ against frequency f; η: viscosity constant; E: velocity independent elasticity parameter

The peak stress σ ₀ increases almost linearly with E.

If E → ∞, σ ₀ → ∞. If E → 0 (purely viscous material)

$$ \underset{E\to 0}{ \lim }{\sigma}_0={\varepsilon}_0\eta \sqrt{0.25{\pi}^2+{\left[ \log \left(2\pi f\right)+\upgamma \right]}^2} $$

(6.71)

and σ ₀ increases with η and f.

The loss tangent, Eq. (6.68), reveals that the phase angle δ depends on E, η, and f.

Increasing the elasticity parameter E reduces the phase angle δ, thereby asymptoting to 0, when E approaches infinity. This result is evident, as a perfectly rigid solid (E = ∞) does not deform and thus there are no losses (δ = 0).

Reducing E increases tan δ. If E approaches zero (purely viscous material), tan δ reaches a limit of

$$ \underset{E\to 0}{ \lim}\left( \tan \delta \right)=\left(\frac{\pi }{2}\right)\frac{1}{ \log \left(2\pi f\right)+\upgamma} $$

(6.72)

a constant, which is a function of f but independent of η. The magnitude of the viscosity η does not matter in this case, as the material is anyway purely viscous. The phase angle δ becomes 0.5 π if

$$ f=\frac{1}{2\pi \, {\mathrm{e}}^{\upgamma}} $$

(6.73)

Thus, if E = 0 and the loss tangent is positive, f must be ≥ 0.08936…Hz.

If f < 0.08936…Hz, δ > 0.5 π, and E′ (storage modulus) and tan δ are negative, which is impossible, as the stored energy is zero (as E = 0), and the energy dissipated cannot be negative.

If the frequency f → ∞ or 0, the loss tangent → 0 in both cases, and the phase angle δ → 0 or π, respectively. Thus, δ crosses π/2 at a certain frequency f ₀. Equating the denominator of Eq. (3.20) with zero yields f ₀ at which δ = 0.5 π:

$$ {f}_0=\frac{{\mathrm{e}}^{-\frac{E}{\eta }-\upgamma}}{2\pi } $$

(6.74)

The ratio E/η in Eq. (6.68) equals the reciprocal value of the viscosity of a log law model. The higher the viscosity, the smaller is the ratio E/η. Figure 6.8 shows E/η against the frequency, the f ₀ curve at which δ = 0.5 π, and the E/η and frequency ranges at which δ is < or > 0.5 π. If E/η → 0, f ₀ approaches the value of Eq. (6.73), which is f ₀ = 0.089359 Hz, i.e. a cycle period of 11.2 s. From Fig. 6.8, the loss tangent, and this the storage modulus, is negative at small E/η (high viscosity) and small frequencies (large cycle periods with small strain rates). This is in accordance with a negative (elastic) modulus of the log law model at very small strain rates (Eq. 5.73 of Fuss 2012).

Fig. 6.8

Ratio of E/η against frequency f; η: viscosity constant; E: velocity independent elasticity parameter; tan δ: loss tangent; f ₀: frequency at which tan δ = π/2

The reciprocal of Eq. (6.68)

$$ \cot \delta =\left(\frac{2}{\pi}\right)\left(\frac{E}{\eta }+ \log \left(2\pi f\right)+\upgamma \right) $$

(6.75)

shows that the higher E/η, the higher is cot δ. Thus, the higher η/E, the higher is tan δ. This explains that the viscosity of a log law model corresponds to the ratio η/E, and not to the viscosity constant η. Figure 6.9 shows that the higher η/E, the higher is the phase angle δ. The viscosity constant η alone has no influence on δ.

Fig. 6.9

Phase angle δ against log frequency; η: viscosity constant; E: velocity independent elasticity parameter

3 Summary

Material models are always simplified descriptions to assist calculations and assessment of mechanical behaviour. This means that a material does not necessarily have to follow the behaviour of its model. For example, the negative storage modulus of a log law model at small E/η and small frequencies may well be a mathematical phenomenon but does not necessarily exist in reality. The standard linear solid is certainly oversimplified with three elements (2 springs, 1 damper) and is usually expanded to more elements for better linear material characterisation (Prony series; Wiechert model; Wiechert 1889, 1893; Fuss 2012).

3.1 Loss Tangent and Viscosity

SLS: tan δ and δ depend on E, η, and f; but at the same f, tan δ and δ at the same R ₁ depend only on R ₂ (Eqs. 6.35 and 6.36), i.e. the ratio of moduli of series to parallel spring, but not on the viscosity constant η.

Power law model: tan δ and δ depend on η only; 0 ≤ η < 1.

Log law model: tan δ and δ depend on E, η, and f; but at the same f, larger E/η have larger tan δ and δ.

3.2 Relationship Between Frequency and Viscosity Constant

SLS: f and η are linked together and always appear as the product fη. Equations (6.26) and (6.27).

Power law model: η has no relationship with f in tan δ (as f does not influence tan δ), whereas for σ ₀, η appears in the gamma function, and is the exponent of f, i.e. f ^η.

Log law model: the viscosity constant appears as a stand alone η, and as the product of η and log 2πf.

3.3 Transient and Steady State Parts

SLS: transient part: exponential function; steady state part: sine and cosine functions.

Power law model: transient part: Maclaurin series; steady state part: sine function with ηπ/2 phase shift (resulting in sine and cosine functions after applying addition rules).

Log law model: transient part: cosine and sine integrals; steady state part: sine and cosine functions.

3.4 Negative Storage Modulus if tan δ > π/2

SLS: tan δ < π/2

Power law model: tan δ < π/2

Log law model: tan δ can be > π/2 at small E/η (high viscosity) and small frequencies (large cycle periods with small strain rates).

Ci:

Cosine integral function

Cin:

Alternative cosine integral function

cos:

Cosine function

cot:

Cotangent function

d:

Differential operator

E :

Modulus (velocity independent elasticity parameter)

E ₁, E ₂ :

Moduli of springs of a standard linear solid

E′:

Storage modulus

E″:

Loss modulus

E*:

Complex modulus

|E*|:

Dynamic modulus

e:

Exponential function

f :

Frequency

H:

Heaviside (unit step) function

i :

$\sqrt{-1}$

lim:

Limit

log:

Natural logarithm

R ₁, R ₂ :

Parameter ratios of a standard linear solid

s :

Complex variable of transformed functions

Si:

Sine integral function

sin:

Sine function

t:

Time

tan:

Tangent function

tan δ :

Loss tangent

Γ:

Gamma function

γ :

Euler–Mascheroni constant (0.577215665)

δ :

Phase shift angle

ε :

Strain

ε _c :

Constant strain

ε ₀ :

Amplitude of strain, peak strain

$\widehat{\varepsilon}$ :

Transformed strain

${\dot{\varepsilon}}_0$ :

Peak strain rate

η :

Viscosity constant

π:

pi (3.14159…)

σ :

Stress

$\widehat{\sigma}$ :

Transformed stress

σ ₀ :

Amplitude of stress, peak stress

τ :

Dummy variable of convolution integral

ω :

Angular frequency ω = 2πf

*:

Convolution operator

∞:

Infinity