Electron-Acoustic Solitons in a Multicomponent Superthermal Magnetoplasma

Abstract

In this paper, electron-acoustic solitons (EASs) in magnetized multicomponent plasma having fluid of cold electrons, positrons, superthermal electrons, and positive ions are examined. The nonlinear Zakharov-Kuznetsov (ZK) equation is derived by applying the reductive perturbation method (RPM). The effect of various plasma parameters (concentration of electrons, superthermality of hot electrons/positrons and magnetic field strength) on the characteristic properties of EASs is analysed.

1 Introduction

From the past many years, the study of electron-acoustic (EA) has become very fascinating among plasma physicists because of their pivotal role in different plasma environments e.g. astrophysical, laboratory and space plasmas  [1]. These waves are evolved due to the existence of two temperature electrons. Due to two distinct temperatures, pressure of hot electrons provide the required restoring force and cold electrons become inertial. Further, due to large mass of ions as compared to that of electrons, ions are considered to form a stationary background. Numerous investigation have already been done to examine the propagation properties of linear and nonlinear EASs. Yu and Shukla  [2] reported the characteristics of EASs in a magnetoplasma with multi-temperature electrons. Mace and Hellberg  [3] studied the EASs in a fluid model composed of two temperature electrons with magnetized and unmagnetized fluid ions. They discussed the propagation properties of KdV-ZK equation with plane and multidimensional solitary wave solutions. Danehkar et al. [4] developed a general plasma fluid model to describe the large amplitude EASs in superthermal plasma using pseudopotential method. They showed that only negative potential EASs are formed in a plasma. Devanandhan et al. [5] studied the EASs in a magnetized and plasma composed of hot ions and cold electrons obeying a kappa distribution. They observed that the magnetic field and other plasma parameters have strongly modified the characteristics of EASs. Various researchers have reported the properties of EASs in electron-positron-ion (e-p-i) plasmas. The characteristic properties of positron acoustic solitons in a multicomponent plasma have been examined by Alam et al.  [6]. They studied the basic features of Double layer, Gardner solitons with solitary wave solution of mKdV equation. Adnan et al.  [7] analysed the ion acoustic waves in a superthermal e-p-i plasma under the influence of magnetic field. They found that the effect of positron concentration and superthermality has modified the ion acoustic solitary waves. Ferdousi et al.  [8] studied the ion acoustic solitons in a magnetized plasma composed of nonextensive positrons and electrons. It was observed that nonextensive parameter has altered the propagation properties of ion acoustic solitons. Saha and Tamang  [9] analysed the behaviour of positron acoustic waves in a multicomponent plasma containing inertial positrons and Kaniadakis distributed positrons and hot electrons. They observed that the effect of different parameters have modified the nonlinear structures. Bansal et al.  [10] examined the characteristic properties of EASs in a magnetoplasma composed of superthermal distributed two temperature electrons, positrons and uniform stationary background ions. The results showed that the nonplanar EASs are significantly modified due to the effect of positron densities as well as positron temperature and other components. The dissipative effects of ion acoustic solitons in a multicomponent collisional e-p-i plasma with non-thermal electrons and isothermal positrons were studied by Gul and Ahmed  [11].

Electron-acoustic waves gain more importance when high energy particles in plasmas come into picture. The occurrence of these high energy particles are well explained by kappa distribution function. The superthermal distribution function was first well explained by Vasyliunas  [12]. Various researchers have examined the role of high energy superthermal particles in nonlinear dynamics. The characteristic properties of ion acoustic waves with two fluid ions in superthermal plasma were studied by Shahmansouri and Tribeche  [13]. The nonlinearity and dispersion properties of ion acoustic solitons are significantly enhanced with change in superthermality parameter. Singh and Sethi  [14] studied the characteristic properties of mKdV equation in a collisionless plasma composed of negatively charged dust, two temperature kappa distributed electrons and hot ions. Singh and Saini  [15] investigated the EA shock waves in a magnetized multicomponent plasma consists of cold electrons as a fluid, hot positrons and superathermal electrons. They analysed that the strength of EA shocks is increased with increase in superthermality of electrons. The aim of our present work is to study nonlinear dynamics of EASs in an e-p-i superthermal magnetoplasma. The paper is arranged as follows: Sect. 2 presents the basic fluid model equations. The derivation of ZK equation and its solution are illustrated in Sect. 3. Numerical analysis is illustrated in Sect. 4. Conclusions are mentioned in the Sect. 5.

2 Basic Fluid Equations

The dimensionless expressions of densities of superthermal positrons and hot electrons are given as  [15]

$$\begin{aligned} n_{p}=1-\gamma b_{1}\phi +\gamma ^2 b_{2}\frac{\phi ^2}{2}+... \end{aligned}$$

(1)

$$\begin{aligned} n_{h}=1+a_{1}\phi +a_{2}\frac{\phi ^2}{2}+.. \end{aligned}$$

(2)

Here, $a_{1}=\left( \frac{\kappa _{e}-\frac{1}{2}}{\kappa _{e}-\frac{3}{2}}\right)$, $a_{2}=\left( \frac{\kappa _{e}^2-\frac{1}{4}}{(\kappa _{e}-\frac{3}{2})^2}\right)$, $b_{1}=\left( \frac{\kappa _{p}-\frac{1}{2}}{\kappa _{p}-\frac{3}{2}}\right)$, $b_{2}=\left( \frac{\kappa _{p}^2-\frac{1}{4}}{(\kappa _{p}-\frac{3}{2})^2}\right)$. Here, $\kappa _{e,p}$ are the superthermality spectral indices of electrons and positrons. The Maxwellian case can be obtained as $\kappa _{e,p}\rightarrow \infty$.

At equilibrium $n_{oh} + n_{oc} = n_{oi} + n_{op}$, where $n_{oj}$(for $j=c,p,i,h)$ are undisturbed number density of cold electrons, hot positrons, stationary ions and hot electrons respectively. The wave is propagating in the x-z plane. We consider the dimensionless equations as  [15]:

$$\begin{aligned} \frac{\partial n_c}{\partial t}+\frac{\partial (n_c u_{cx})}{\partial x}+\frac{\partial (n_c u_{cz})}{\partial z}=0, \end{aligned}$$

(3)

$$\begin{aligned} \frac{\partial u_{cx}}{\partial t}+u_{cx}\frac{\partial u_{cx}}{\partial x}+u_{cz}\frac{\partial u_{cx}}{\partial z}=\frac{\partial \phi }{\partial x}-\Omega u_{cy}, \end{aligned}$$

(4)

$$\begin{aligned} \frac{\partial u_{cy}}{\partial t}+u_{cx}\frac{\partial u_{cy}}{\partial x}+u_{cz}\frac{\partial u_{cy}}{\partial z}= \Omega u_{cx}, \end{aligned}$$

(5)

$$\begin{aligned} \frac{\partial u_{cz}}{\partial t}+u_{cx}\frac{\partial u_{cz}}{\partial x}+ u_{cz}\frac{\partial u_{cz}}{\partial z}=\frac{\partial \phi }{\partial z}, \end{aligned}$$

(6)

$$\begin{aligned} \frac{\partial ^2 \phi }{{\partial x}^2}+\frac{\partial ^2 \phi }{{\partial z}^2}= 1- \alpha + n_{c}\sigma - \delta +\phi {\left( a_{1}+\alpha \gamma b_{1}\right) } \nonumber \\ + \frac{\phi ^2}{2}{\left( a_{2}-\alpha \gamma ^2 b_{2}\right) }, \end{aligned}$$

(7)

The fluid velocity $u_{c}$, and electrostatic potential $\phi$, are normalized with respect to EA speed, $C_{e}=(\frac{T_{h}}{m_{e}})^\frac{1}{2}$, and $\frac{T_{h}}{e}$, respectively. The space coordinate (x) is normalized by electron Debye length $\lambda _{D}=(\frac{T_{h}}{4\pi n_{oh}e^2})^\frac{1}{2}$ and time coordinate (t) is scaled by inverse of plasma frequency of electrons, $\omega _{ph}=(\frac{4\pi n_{oh}e^2}{m_{e}})^\frac{1}{2}$. The gyrofrequency of electron, $\omega _{c}=\frac{eB}{m_{e}c}$ is scaled with respect to $\omega _{ph}$, $\Omega =\frac{\omega _{c}}{\omega _{ph}}$. $\alpha =\frac{n_{op}}{n_{oh}}$, $\sigma =\frac{n_{oc}}{n_{oh}}$, $\delta =\frac{n_{oi}}{n_{oh}}$ and $\gamma =\frac{T_{h}}{T_{p}}$.

3 Derivation of ZK Equation and Its Solution

To study the dynamics of EASs with weak dispersion and of weak nonlinearity, we assume $\omega$ (or k) $<<$ 1. All physical quantities vary slowly in space and vary more slowly in time. We have used the RPM to find the ZK equation. The stretched coordinates are given as  [16, 17]:

$$\begin{aligned} \xi = \epsilon ^\frac{1}{2}\left( z-Vt\right) , \quad \zeta =\epsilon ^\frac{1}{2}x, \quad \textrm{and} \quad \tau = \epsilon ^\frac{3}{2}t \end{aligned}$$

(8)

The expansions used are given as:

$$\begin{aligned} n_{c}=1+\epsilon n_{c1}+\epsilon ^2 n_{c2}+\epsilon ^3 n_{c3}+..., \end{aligned}$$

(9)

$$\begin{aligned} u_{cx}=\epsilon ^\frac{3}{2} u_{cx1}+\epsilon ^2 u_{cx2}+\epsilon ^\frac{5}{2} u_{cx3}+..., \end{aligned}$$

(10)

$$\begin{aligned} u_{cy}=\epsilon ^\frac{3}{2}u_{cy1}+\epsilon ^2 u_{cy2}+\epsilon ^\frac{5}{2} u_{cy3}+..., \end{aligned}$$

(11)

$$\begin{aligned} u_{cz}=\epsilon u_{cz1}+\epsilon ^2 u_{cz2}+\epsilon ^3 u_{cz3}+..., \end{aligned}$$

(12)

$$\begin{aligned} \phi =\epsilon \phi _{1}+\epsilon ^2\phi _{2}+\epsilon ^3\phi _{3}+..., \end{aligned}$$

(13)

using Eqs. ( 8)–( 13) in Eqs. (3)–(7) neutrality condition is obtained as: $(\delta +\alpha ) =(1+\sigma )$. After simplifying, we get the first order equations as:

$$\begin{aligned} n_{c1}=-\phi _{1}Q , \end{aligned}$$

(14)

$$\begin{aligned} u_{cy1}=\frac{1}{\sigma }\frac{\partial \phi _{1}}{\partial \zeta }, \end{aligned}$$

(15)

$$\begin{aligned} u_{cz1}=-V\phi _{1}, \end{aligned}$$

(16)

$$\begin{aligned} V=\frac{1}{\sqrt{Q}}, \end{aligned}$$

(17)

where, V is the phase velocity of EASs and $Q=\frac{\left( a_{1}+\alpha \gamma b_{1}\right) }{\sigma }$. By equating the quantities for higher orders of $\epsilon$ and doing rigorous calculations, we have obtained the following ZK equation as:

$$\begin{aligned} \frac{\partial \phi }{\partial \tau }+ A\phi \frac{\partial \phi }{\partial \xi }+B\frac{\partial ^3\phi }{\partial \xi ^3}+C\frac{\partial }{\partial \xi }\left( \frac{\partial ^2\phi }{\partial \zeta ^2}\right) =0, \end{aligned}$$

(18)

where, $\phi _{1}=\phi$ and nonlinear coefficient $A=B\left( -3\sigma Q^2-(a_{2}-\alpha \gamma ^2 b_{2})\right)$, dispersion coefficient $B=\frac{1}{2VQ^2\sigma }$, and transverse dispersion coefficient $C=B\left( 1+\frac{\sigma }{\Omega ^2}\right)$.

We consider a transformation $Y=l_{x}\zeta +l_{z}\xi -\Lambda \tau$, ($l_{x}$, $l_{z}$ are the direction cosines), to evaluate the solution of Eq. (18). $\Lambda$ denotes the velocity of solitons w.r.t. moving frame scaled with $C_{e}$. The solution of ZK equation is obtained as [18]:

$$\begin{aligned} \phi =\phi _{0} sech^{2}{\left( \frac{Y}{\Delta }\right) }, \end{aligned}$$

(19)

where $\phi _{0}=\frac{3\Lambda }{A l_{z}}$ is maximum amplitude and $\Delta ={\left( \frac{4F l_{z}}{\Lambda }\right) }^\frac{1}{2}$ is the width of EASs.

Here, $F=B l_{z}^2+C{\left( 1-l_{z}^2\right) }$.

4 Numerical Analysis

To carry out numerical analysis, the range of various physical parameters in laboratory and astrophysical/space plasmas  [19] is chosen as: $n_{op}\sim$(1.5–3) $\mathrm{{cm}}^{-3}$, $n_{oc}\sim$(0.1–0.4) $\mathrm{{cm}}^{-3}$, $T_{h}\sim$(200–1,000) eV, $n_{oh}\sim$(1.5–3) $\mathrm{{cm}}^{-3}$, and $T_{p}\sim$ (200–1,000) eV. The propagation properties of EASs are strongly influenced by the change in the value of any parameter.

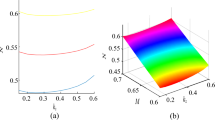
Figure 1, describes the behaviour of phase velocity (V) with superthermality index of positrons (via $\kappa _{p}$) and superthermality index of hot electrons (via $\kappa _{e}$). It is found that with increment in $\kappa _{p}$ and $\kappa _e$ (i.e., decrease in the superthermality of positrons/electrons), the phase velocity of EASs is enhanced. It is clear that superthermality effects have significantly modified the dispersion properties of EASs and makes the wave to propagate slowly in case of more superthermal charged particles.

Fig. 1

Plot for the phase velocity (V) with superthermality of positrons ($\kappa _{p}$) and with electrons ($\kappa _{e}$)

Fig. 2

Plot for the nonlinear coefficient (A) with superthermality index of positrons (via $\kappa _{p}$) and with superthermality index of electrons ($\kappa _{e}$)

Fig. 3

Plot for EASs profile ($\phi$) with superthermality index of electrons ($\kappa _{e}$), here $\gamma =4$, $\delta =0.1$, $\kappa _{p}=4$, $\sigma =0.2$, $l_{z}=0.6$, $\omega =0.2$, $\Lambda =0.3$

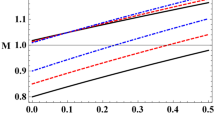
Figure 2, describes the nature of coefficient (A) with superthermality index of positrons($\kappa _{p}$) and superthermal index of hot electrons (via $\kappa _{e}$). It is seen that with increase in $\kappa _{p}$ and $\kappa _e$, magnitude of A increases. It is found that A is negative, so only negative potential EASs are reported in the considered plasma model.

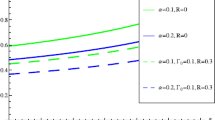
In Fig. 3, we have analysed the characteristics of EASs profile ($\phi$) with superthermality of electrons (via $\kappa _{e}$). It is noticed that with increase in the value of $\kappa _{e}$, the amplitude and width of EASs are increased along negative axis. This variation in the properties of EASs occurs due to the change in nonlinearity and dispersion effects.

In Fig. 4, depicts the nature of EASs profile ($\phi$) with number density ratio of positron to hot electron $\alpha (=n_{op}/n_{oh}$) and shows that amplitude(width) of EASs is increased (decreased) with increase in $\alpha$. It is noteworthy to mention that any change in number density ratios makes variation in the nonlinear coefficient A that further modifies the profile of solitons.

Figure 5, describes the profile of EASs ($\phi$) with magnetic field strength (via $\Omega$). The width of EASs increases with the increase in the value of magnetic field strength, whereas the amplitude remains same. It is clear that the dispersion effects are more pronounced with the variation of magnetic field strength.

Figure 6, describes the variation of profile of EASs ($\phi$) for multiple values of ($\kappa _{p}$). It is analysed that with rise in superthermality index of positrons (via $\kappa _{p}$), the magnitude of EASs decreases. This emphasizes that superthermality index has strongly influenced the properties of EASs with change in different nonlinear effects.

Figure 7, depicts the variation of EASs profile ($\phi$) for multiple values of temperature ratio of hot electrons to positrons $\gamma (=\frac{T_{h}}{T_{p}}$) and highlights that width and amplitude of EASs are decreased with increase in the value of $\gamma (=\frac{T_{h}}{T_{p}}$). This highly change in width and amplitude of EA solitons is noticed due to the effect of temperature ratio on the nonlinear coefficient A.

Figures 8 and 9, represent the 3D profiles of EASs with magnetic field strength ($\Omega$) and superthermality of positrons ($\kappa _{p}$) respectively. These figures further confirm the modification in the profile of EA solitons with the variation of different parameters simultaneously.

Fig. 4

Plot for EASs profile ($\phi$) with number density ratio $\alpha (=n_{op}/n_{oh}$)

Fig. 5

Plot for EASs profile ($\phi$) with magnetic field strength ($\Omega$)

Fig. 6

Plot for EASs profile ($\phi$) with superthermality index of positrons ($\kappa _{p}$)

Fig. 7

Plot for EASs profile ($\phi$) with temperature ratio of hot electrons to positrons $\gamma (=\frac{T_{h}}{T_{p}}$)

Fig. 8

Plot for 3D EASs profile ($\phi$) with magnetic field strength ($\Omega$)

Fig. 9

Plot for 3D EASs profile ($\phi$) with superthermality index of positrons ($\kappa _{p}$)

5 Conclusion

We have studied the salient features of EASs in a magnetized multicomponent plasma having inertial cold electrons, inertialess ions and superthermal positrons as well as electrons. The RPM is adopted to develop the nonlinear ZK equation and its solution to describe the dynamics of EASs. Only EASs with negative polarity exist. The effects of various plasma parameters such as $\kappa _{p}$, $\kappa _e$, $\alpha$, $\gamma$ and $\Omega$ have significantly influence the characteristic properties of EASs. The phase velocity of solitons is enhanced with increase in $\kappa _{p}$ and $\kappa _e$. Nonlinear coefficient (A) flourishes with increase in $\kappa _{p}$ and $\kappa _e$. The width and amplitude of EASs are increased with increase in $\kappa _{e}$ and $\alpha$. The width of EASs increases with increase in the value of strength of magnetic field ($\Omega$). With the increase in the value of ratio of temperature of hot electrons to positrons $\gamma (=\frac{T_{h}}{T_{p}}$), the width and amplitude of EASs are decreased. Negative potential EASs are significantly influenced with the change in dispersion and nonlinearity effects.

The outcome of present study can be beneficial for the indepth understanding of EASs with superthermal positrons and electrons in Van Allen radiation belts, auroral zone, planetary magnetospheres  [20,21,22,23].