Introduction

The concept of Environmentally conscious manufacturing and product recovery (ECMPRO) is interpreted as the manufacturers’ responsibility regarding take-back legislation, customers’ awareness of green products, and economics [1]. Based on the literature surveys in the year 1999 [10] and 2010 [13], researchers focused on environmentally conscious design and production, material recycling, remanufacturing, reverse and closed-loop supply chains. However, fewer of them discussed the marketing-related issues for remanufactured products, especially for short lifecycle items. Hi-tech products, such as smartphones and laptops, are considered short lifecycle items due to market competition and customers’ behavior. Manufacturers launch newer generation models intensively to meet customers’ requirements on fashion design and technology innovation. Likewise, high-end customers stop using old models and purchase new products. A product that the customer no longer wishes to use and reaches the end of a use cycle is called an end-of-use (EOU) product [21]. These end-of-use products returned by high-end customers often have significant functional and material value remaining, which are valuable suppliers for remanufacturing operations. Then remanufactured products are sold to price-sensitive or green customers. High-end customers’ throwing behavior creates a healthy business cycle but intensifies the e-waste problem. However, the remanufacturing practice has mitigated this dilemma situation, avoids massive use of new materials, excavates the value of end-of-use parts or cores, reduces the burden of the environment, and creates a unique business opportunity by producing remanufactured products.

Remanufacturing is a comprehensive and rigorous industrial process by which a previously sold, worn, or non-functional product or component is returned to a “like-new” or “better-than-new” condition and warranted in performance level and quality [4]. The topics of remanufacturing are not limited to techniques of disassembly, reassembly, and inspection. They are extended to several interdisciplinary areas, such as design for remanufacturing with lifecycle thinking [11] and decision-making methods in three supply chain management levels [22]. Market issues, such as pricing decision, is critical to the survival of remanufacturing industry. Marketing the remanufactured products is challengeable because customers’ acceptance towards remanufactured products is lower than the brand-new products and expect a lower price. The price difference between remanufactured products and similar new products is between 30% and 40% [14]. Andre-Munot, et al. even indicate a more massive gap, between 45%–65% [1]. However, remanufactured high-technology products are slightly different from other durable goods. New models with the latest technology and design are released yearly. Remanufactured products belonging to the newest generation are available when the unsold old models are still in stock. Therefore, remanufactured items can surpass the new products belonging to the early generation due to the novel technology despite a lower perceived quality. Heterogeneous customers perceive differently on distinct goods. Quality-conscious customers prefer the brand-new product rather than remanufactured items, while technology-savvies pay more attention to technology innovation than quality. To manage the overall profit for all kinds of products well, manufacturers need to understand customer’s needs and identify the market position of different items, selling them with differentiation prices to avoid cannibalization with new generation models.

The success of a remanufacturing practice is dependent on a complete closed-loop supply chain. The supply chain members play their roles and influence others. Some retailers play a dominant role in the system, while the downstream members are the followers. Some manufacturers have more power than the retailer. The manufacturers and retailers create a vertical competition in a supply chain. Members at the same structure line also compete horizontally. The manufacturer and remanufacturer are in the same downstream line. They compete but also coordinate for the vertical competition. The retailer, manufacturer, remanufacturer need to find an optimal pricing strategy to maximize the profit in a centralized or decentralized supply chain structure. A successful marketing policy for new and remanufactured products should address the interests of all supply chain members. However, a limited number of studies about price decisions for new and remanufactured products belonging to different generations has been conducted, especially in the supply chain’s scope. Fewer studies analyzed how customers value technology depreciation towards outdated new products and acceptance towards remanufactured products, and how do these perceptions and customer populations in different segments influence the pricing strategy.

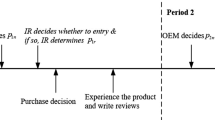
This paper addressed wholesale and retail price decisions for the new products belonging to the early generation (Type 1 product) and the remanufactured products belonging to the latest generation (Type 2 product) in a supply chain system with a manufacturer, a remanufacturer, and a retailer. The two products’ market is separated from the premium market, which sells new products belonging to the latest technology. Retail prices are determined by customers’ perceived value on quality and technology. The customers are segmented into two groups, quality-conscious and technology savvies. Each group of people assess the products differently. Five game theory models are implemented to determine optimal prices. They are Vertical Nash Equilibrium model (VN), Manufacturer-Stackelberg balanced power model (MNS), Manufacturer-Stackelberg manufacturer leading model (MMS), Retailer-Stackelberg balanced power model (RNS), and Retailer-Stackelberg manufacturer leading model (RMS).

In the VN model, the retailer, manufacturers, and the remanufacture announce the prices simultaneously by anticipating competitors’ prices. In the Manufacturer-Stackelberg model, the manufacturer and remanufacturer will notify the wholesale prices first by predicting the retailer’s prices for both products, while in the Retailer-Stackelberg model, the sequence will be in the other way. We will consider two scenarios In Manufacturer-Stackelberg and Retailer-Stackelberg models, respectively. The first scenario is assumed as a balanced power between the manufacturer and the remanufacturer. They announced the wholesale prices co-currently. The second scenario shows that the manufacturer acts as a leader that notifies the price ahead of the remanufacturer. Then the remanufacturer foresees the wholesale prices for both products and declares a wholesale price. In the real world, Type 1 product announces a discounted price when the new model is launched; Type 2 product will be available later when some new models are returned. The price of Type 2 product is determined with a known price of Type 1 product, or the manufacturer negotiates with the remanufacturer for balanced power. Therefore, we do not consider the remanufacturer to lead the market. The study will compare the profits for the three supply chain members and the whole supply chain. We will also implement numerical analysis to explore how optimal pricing decisions would vary depending on different value perceptions of two customer segments and relative size of each group.

This research aims to determine optimal prices for Type 1 and Type 2 products. The study helps understand how different coordination and competition strategies among manufacturers and retailers affect optimal price decisions for profit maximization. It also explores how to price based on different value perceptions of two customer segments and the relative size of each group. The result provides information to industrial decision-makers for choosing an optimal strategy based on the specific market situation.

In the following sections, the literature review summarizes the previous papers regarding marketing policies for heterogeneous customers, supply chain structure policies for the system members, and competition among supply chain members. The models show the customer perceived-value demand functions for two types of commodities and profits for the retailer, manufacturer, remanufacturer, and the whole supply chain. The factor analysis sheds light on the impact of customer segment ratio and the difference in willingness to pay. The conclusion illustrates the managerial insights and future study.

Literature review

Many researchers focused on pricing decisions for new and remanufactured products. Finding an optimal price helps balance the trade-off between generating revenue and losing business opportunities. In the hi-tech industry or apparel industry, manufacturers release new generation models or seasonal products in regular cycles. Prices of commodities differ from various generations and seasons. Price decisions are also dependent on the product’s condition. Remanufactured and used products usually have a lower price than the new product due to the inferior perceived quality. Researchers searched for the optimal price for high-technology or seasonal products by understanding customer’s reservation value and purchase behavior. Researchers also adjusted price decisions based on vertical and horizontal competition and cooperation among different manufacturers and retailers.

The customer’s acceptance of the products and willingness to pay (WTP) is critical to the pricing strategy. To understand people’s purchase behavior, Kuo and Huang (2012) examined the dynamic retail price decisions for the products from two different generations considering inventory level and remaining selling periods [18]. They generalized the Nash bargaining solution model in two scenarios, posted-pricing-first and Negotiation-first to determine the optimal posted price. Customers are classified into two segments, price-takers and bargainers. Authors suggested an increased posted price when bargainers are the majorities, and negotiable price for the earlier generation is the optimal strategy for short lifespan electronic products. Zhu and Yu (2018) also conducted a case study on new, remanufactured, and refurbished electronic products to analyze customers’ buying behavior, but they paid more attention to the service level [33]. Under the dynamic game model, they found that the service differentiation among three products is reduced when the consumer preference for service increases. Liu et al. (2016) examined the customers’ WTP and acceptance level for the remanufactured products by setting the independent price for new products in the first cycle period, then ruling the joint strategy for both new and remanufactured products in the second cycle [19]. They extended the study into a dual-channel system with one manufacturer, one retailer, and one e-retailer, discussed the influence of bargaining power between the manufactured and the retailer. The result shows that all supply chain members receive benefits from selling remanufactured products. Customer’s acceptance level for remanufactured products is positively correlated with the manufacturer’s profit growth significantly more than with that of the retailer and the e-retailer. The same phenomenon is summarized by Gan et al. (2017) [6]. They established a two-time period model to find optimal prices for new and remanufactured products in a separate sales-channel, where the remanufactured products are sold through a direct channel, while the new products are sold via the retailer. They pointed out that lower remanufacture acceptance contributes to higher profit for the retailer but lower profit for the manufacturer. However, if the acceptance level is high, the manufacturer can leverage the power as the Stackelberg leader to gain more benefits than the retailer. They also found that a dual-channel brings more profit than single-channel to the whole supply chain. Moreover, most recently, a study indicated that influencing factors towards purchasing remanufactured products include changing customer’s attitude, evaluation, and product remaining function [15]. However, no previous research focused on how technology development influences the acceptance level towards remanufactured products in different customer segments.

Except for the customer acceptance towards remanufactured products, other papers discussed price strategies in a dual-channel supply chain system and channel selection. Manufacturers and retailers are critical members of a product supply chain. Customers purchase items in different ways. Some people prefer to place an order online, while others enjoy retailer shopping. Both manufacturers and retailers have an option to operate a dual-channel supply chain system. Different sale channels vary the pricing policies. Chen et al. (2013) focused on the channel and brand competitions in an environment of one manufacturer with internet and traditional channels, and one retailer who sells two substitutable products [3]. They built up Nash and Stackelberg game models and applied sensitivity analysis of an equilibrium solution. They believe that brand loyalty is the key to profitability; both the manufacturer and the retailer prefer an appropriate cooperation strategy rather than the Nash strategy. Soleimani (2016) built a manufacturer-leader Stackelberg game pricing model with fuzzy cost and customer demand to determine the optimal retail price and the wholesale price [24]. The result shows with a decrease fuzzy degree of the parameter, the optimal prices decrease, and the profits of the manufacturer and the entire channel increase while the profit of the retailer drops. Rodriguez and Aydin (2015) studied the same system but built an inventory cost and rested-logit customer demand model for pricing and assortment decisions [23]. They found contradictory results on the preferences of the manufacturer and the retailer. Xiao and Shi (2016) developed game theoretic models to determine pricing and channel priority strategies when the retailer faces insufficient supply from the manufacturer caused by random yield [25]. They indicated that the dual-channel policy has an advantage in alleviating the retailer’s pressure on inadequate supply. Supply priority decision varies in different conditions. Ding et al. (2016) assumed that the manufacturer acts as Stackelberg’s leader, found an optimal joint strategy for a wholesale price, retailer price, and direct channel price by hierarchical pricing decision process [5]. They indicated that operating a dual-channel system only brings more benefits to the manufacturer. An equal-price policy for both sales channels is not optimal for the manufacturer. Gao et al. (2016) worked on a similar problem but analyzed customer acceptance of the direct channel on pricing decision by building Nash game, manufacturer Stackelberg game, and retailer Stackelberg game models [7]. They explored that when customers’ acceptance of the direct channel increases, the wholesale price, retail price, and expected profits of the retailer decrease, while the direct sales price and manufacturer’s expected earnings in the retailer-Stackelberg game all increase. Except for price decisions, Wang et al. (2016) looked for a new way to market new and remanufactured products by identifying the optimal channel strategy [27]. They believe the manufacturer prefers to open a direct online channel to differentiate new and remanufactured products while the dual-channel system benefits the customer but sacrifices the retailer.

The above papers only examined the pricing strategy in a forward supply chain. Some researchers extended the problem to a closed-loop supply chain (CLSC). The same with Soleimani (2016) [24], Karimabadi et al. (2019) examined the optimal pricing decisions by building game theory models in one manufacturer and one retailer environment with fuzzy variables. However, they extended the study to CLSC when the manufacturer operates dual channels in selling new products and collecting used parts [16]. The results show that with a decrease in the fuzzy degree of price sensitivity in the Manufacturer-Stackelberg model, the expected profit for the manufacturer and the retailer increase. Giri et al. (2017) considered a system with one manufacturer, one retailer, and one third-party, where the manufacturer sells new products through retailer and e-retailer. In contrast, third-party and e-retailer are responsible for collecting used parts [8]. They decided optimal retail, wholesale, acquisition prices, and return product collection rate in five scenarios, including centralized, Nash game, manufacturer-led, retailer-led, and third party-led. They approved that a retailer-led decentralized scenario provides more benefits than other decentralized scenarios.

Apart from the study on an environment of one manufacturer and one retailer, another volume of papers keeps eyes on the horizontal competition between two manufacturers or two retailers, and the vertical competition between the manufacturer and the retailer. Ke and Cai (2019) developed price decision models with collection-relevant demand in a closed-loop supply chain. One manufacturer acts as the Stackelberg leader, and two competing retailers are assumed to be the followers [17]. They indicated that the demand is a more significant factor than the cost-saving from remanufacturing for motivation to recycling. Hsiao and Chen (2014) described vertical competition and horizontal competition between retailers and e-retailers by analyzing internet channels, pricing strategies, and channel structure using Nash Equilibrium method [12]. They indicated that although the manufacturer’s online channel encroaches the retailer’s benefit, the manufacturer is willing to give up the direct channel and leverage on retailer competition. Other researchers considered the system with two manufacturers and one retailer. Gu and Gao (2012) conducted a study on the closed-loop supply chain in an environment with two manufacturers and one retailer [9]. They believe the retailer is the optimal collector for used cores. Zhao et al. (2017) investigated the remanufacturing decision for two substitutable products, new products produced by raw materials and remanufactured products incorporated used parts [28]. They examined the research problem in Manufacturer-Stackelberg and Retailer-Stackelberg models with several sub-conditions, such as two manufacturers have equal or different market power with subgame perfect Nash equilibrium solution concept. They found that the whole supply chain receives the highest profit in Manufacturer-Stackelberg when two manufacturers have equal force. Luo et al. (2017) conducted a related study but focused on the two differentiated brand products [20]. They indicated that manufacturers’ competition benefits the retailer after analyzing seven game models. The whole supply chain receives more profit by increased acceptance of the average brand. Aydin et al. (2016) extended the research to the closed-loop supply chain in a multi-objective optimization problem [2]. They proposed a case study on tablet PCs in a system containing a manufacturer, a remanufacturer, and a chain retailer, aim to find pricing decisions, product return rate, and product line solutions by Stackelberg game theory and Genetic algorithm. Except for the price decision across the competition and the channel power, some researchers focused on the service’s impact. Wang Z. and Wang Y. (2015) established the Retailer-Stackelberg, Manufacturer-Stackelberg, and the Nash Equilibrium models in a monopoly market with one manufacturer, one remanufacturer, and one retailer. They exhibited that the customer’s acceptance growth on remanufactured products leads to lower prices, higher service levels, and lower profit for the members with less power [26].

Most studies about pricing remanufactured products are focused on negotiating between sellers and buyers with different bargain powers. Researchers discussed more customer’s acceptance towards remanufacturing. Some researchers expanded the scope to the whole supply chain, explore the price influence by horizontal and vertical competitions among retailers and manufacturers. However, Fewer studies take notice of the technology advantage for high-technology remanufactured products, compared to the outdated unsold new products. Both technology innovation and quality conditions could influence customers’ willingness to pay differently in distinct segments. This study fills the research gap, focusing on pricing new and remanufactured hi-tech products belonging to different generations, exhibiting how horizontal competition between the manufacturer and remanufacturer, and vertical competition with the retailer influence the price strategy.

Model

The manufacturer sells Type 1 products to the retailer at a wholesale price w1, while the remanufacturer sells Type 2 products to the same retailer at w2. The retailer sells the product to customers at different retail prices p1 and p2. Figure 1 shows the supply chain structure. The cost of manufacturing a new product is c1, while a remanufactured product is c2. Assume c1 >  c2.

Fig. 1
figure 1

Supply chain structure

Full size image

Customers are classified into two segments. The first segment is the quality-conscious that customers are concerned more about quality than technology. The second segment is the technology-savvy that takes more attention to technology development than the product condition. Type 1 products depreciate in technology, while Type 2 products are inferior in quality. Customers in different segments have different perceived discounts for Type 1 and Type 2 products. α1 and α2 are the inferiority of generation obsolescence for quality-conscious customers and technology-savvies, respectively. β1 and β2 are the discount factors of remanufacturing inferiority for quality-conscious and technology-savvy customers. We assume that α1 >  α2, β1 < β2. An item is sold only when the price is less than the perceived value. Supply constraint is not considered.

As described in Ref. [20], the authors consider one product is priced at p; the customer perceived value ν is uniformly distributed over [0, 1]. Therefore, the demand of the product is \( Q={\int}_P^1 d\nu =1-p \), for 0 < = p < = 1. We develop the model for two types of products with different generations and product condition. We assume that ν is the perceived value for the new product belonging to the latest generation. Table 1 shows the perceived value from customers in each segment. Table 2 exhibits how customers in each group select products. We assume δ is the proportion of the customers in the quality-conscious segment; the proportion of technology-savvy group is 1-δ. Therefore, the piecewise demand function can be derived.

Table 1 Perceived value of two types of products from customers in segment
Full size table
Table 2 Customers’ selection
Full size table

Demand function

When p1 < p2, all the quality-conscious customers will buy Type 1 products; the technology-savvy customers will choose Type 1 items if β2-α2 < p2-p1.

When p2 < p1, all the technology-savvy customers will buy Type 2 products; quality-conscious customers will choose Type 2 items if α1-β1 < p1-p2.

Therefore, the demand functions of Type 1 and Type 2 products are as follows in function (1) to (4).

  • Condition 1: p1 < p2

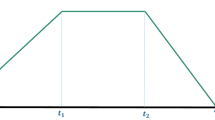
  • Region 1: When 0\( <\frac{\beta_2}{\alpha_2}<\frac{p_2}{p_1}<1 \) and P1 < α1, P1 < α2, all customers will buy Type 1 products. Figure 2 expresses the demand of Type 1 product. Q1,1 means the demand from quality-conscious customers, while Q1,2 represents the demand from technology-savvies.

Fig. 2
figure 2

Demand for Type 1 product in the two customer segments

Full size image
$$ \left\{\begin{array}{l}{\mathrm{D}}_1\ \left({\mathrm{p}}_1,{\mathrm{p}}_2\right)=\updelta\ \left({\upalpha}_1-{\mathrm{p}}_1\right)+\left(1-\updelta \right)\ \left({\upalpha}_1-{\mathrm{p}}_1\right)\\ {}{\mathrm{D}}_2\ \left(\mathrm{p}1,\mathrm{p}2\right)=0\end{array}\right. $$
(1)

  • Region 2: When\( \kern1em \frac{\alpha_2}{\beta_2}<\frac{p_1}{p_2}<1 \) and p1 < α1, p1 < α2, p2 < β2, all quality-conscious customers will buy Type 1 products, some technology savvies will purchase Type 1 products instead of Type 2 products.

$$ \left\{\begin{array}{l}{\mathrm{D}}_1\ \left({\mathrm{p}}_1,{\mathrm{p}}_2\right)=\updelta\ \left({\upalpha}_1-{\mathrm{p}}_1\right)+\left(1-\updelta \right)\left(\frac{p_2-{p}_1}{\beta_2-{\alpha}_2}-\frac{p_1}{\alpha_2}\right)\\ {}{\mathrm{D}}_2\ \left({\mathrm{p}}_1,{\mathrm{p}}_2\right)=0+\left(1-\updelta \right)\ \left({\upbeta}_2-\kern0.5em \frac{P_2-{P}_1}{\kern0.75em {\beta}_2-{\alpha}_2}\right)\end{array}\right. $$
(2)

  • Condition 2: p 1 > p 2 ,

  • Region 3: When 1 <\( \frac{\alpha_1}{\beta_1}\le \frac{p_1}{\ {p}_2} \) and p2 < β1, p2 < β2, all customers will buy Type 2 products. Figure 3 shows the demand of Type 2 product from each customer group.)

Fig. 3
figure 3

Demand for Type 2 product in the two customer segments

Full size image
$$ \left\{\begin{array}{l}{\mathrm{D}}_1\ \left({\mathrm{p}}_1,{\mathrm{p}}_2\right)=0\ \\ {}{\mathrm{D}}_2\ \left({\mathrm{p}}_1,{\mathrm{p}}_2\right)=\updelta \left({\upbeta}_1-{\mathrm{p}}_2\right)+\left({\upbeta}_2-{\mathrm{p}}_2\right)\end{array}\right. $$
(3)

  • Region 4: When \( 1<\frac{p_1}{\ {p}_2}<\frac{\alpha_1}{\beta_1} \), some quality conscious customers will purchase Type 2 product instead of Type 1 product.

$$ \left\{\begin{array}{l}{\mathrm{D}}_1\ \left({\mathrm{p}}_1,{\mathrm{p}}_2\right)=\delta\ \left({\alpha}_1-\frac{p_1-{p}_2}{\alpha_1-{\beta}_1}\right)\\ {}{\mathrm{D}}_2\ \left({\mathrm{p}}_1,{\mathrm{p}}_2\right)=\kern0.5em \delta\ \left(\frac{p_1-{p}_2}{\alpha_1-{\beta}_1}-\frac{p_2}{\beta_1}\right)+\left(1-\delta \right)\left({\beta}_2-{p}_2\right)\end{array}\right. $$
(4)

The profit models for manufacturer, remanufacturer, and retailer can be generated based on the demand functions.

Profit model

Manufacturer 1’s profit model:

$$ {\Pi}_{\mathrm{m}1}=\left({\mathrm{w}}_1-{\mathrm{c}}_1\right)\times {\mathrm{D}}_1\ \left({\mathrm{p}}_1,{\mathrm{p}}_2\right) $$
(5)

Manufacturer 2’s profit model (Remanufacturer):

$$ {\Pi}_{\mathrm{m}2}=\left({\mathrm{w}}_2-{\mathrm{c}}_2\right)\times {\mathrm{D}}_2\ \left({\mathrm{p}}_1,{\mathrm{p}}_2\right) $$
(6)

Retailer’s profit model:

$$ {\Pi}_{\mathrm{r}}=\left({\mathrm{p}}_1-{\mathrm{w}}_1\right)\times {\mathrm{D}}_1\ \left({\mathrm{p}}_1,{\mathrm{p}}_2\right)+\left({\mathrm{p}}_2-{\mathrm{w}}_2\right)\times {\mathrm{D}}_2\ \left({\mathrm{p}}_1,{\mathrm{p}}_2\right) $$
(7)

Based the profit models above, the results of five game theory models could be derived in the four regions. In the Vertical Nash Model, all supply chain members will announce simultaneously. The manufacturer and remanufacturer determine the prices, anticipating the retailer’s margins. At the same time, the retailer decides retail prices to maximize its profit by predicting the wholesale prices of two types of the products. In the manufacturer-Stackelberg model, we will analyze the two scenarios. The first scenario is that the manufacturer and the remanufacturer are shared the same power (MNS). The second scenario is that the manufacturer acts as a Stackelberg leader (MMS). In the model of MNS, the manufacturers and the remanufactures declare the wholesale prices to the retailer simultaneously, anticipating the retailer’s prices for both Type 1 and Type 2 products. In the second step, the retailer decides the retail prices with the given wholesale prices. In the case of MMS, manufacturer-Stackelberg Leader Case (MMS), the manufacturer announces the wholesale price of Type 1 product, anticipating the wholesale price of Type 2 product and the retail prices of the two types of products. Then given the wholesale price for Type 1 product and anticipating the retail prices, the remanufacturer decides its wholesale price. Third, the retailer determines the optimal retail prices with known wholesale prices. In the Retailer-Stackelberg model, we will also analyze the condition of balanced power (RNS) and being led by the manufacturer (RMS). In the RNS case, the retailer states the price by foreseeing the wholesale prices, then the manufacturer and remanufacturer show the wholesale prices simultaneously by anticipating the retailer’s margin. In the RMS, the manufacturer determines the price after the retailer’s announcement by anticipating the wholesale price of Type 2 products, and then the remanufacturer decides the optimal price. The results of each model in two conditions and four regions are given in the next part. The solution steps are shown in Appendix 1.

Optimal solutions

Condition 1, region 1

Retailer’s profit model:

Πr = (p1 − w1) × [δ   (α1 − p1) + (1 − δ)   (α2 − p1)]

Manufacturer’s profit model:

$$ {\Pi}_{\mathrm{m}1}=\left({\mathrm{w}}_1-{\mathrm{c}}_1\right)\times \left[\updelta\ \left({\upalpha}_1-{\mathrm{p}}_1\right)+\left(1-\updelta \right)\ \left({\upalpha}_2-{\mathrm{p}}_1\right)\right] $$

Remanufacturer’s profit model:

$$ {\Pi}_{\mathrm{m}2}=\left({\mathrm{w}}_2-{\mathrm{c}}_2\right)\times 0=0 $$

VN model:

$$ {\displaystyle \begin{array}{c}\ {w}_1^{VN-R1}=\frac{\delta {\alpha}_1+\left(1-\delta \right){\alpha}_2+2{c}_1}{3}\\ {}{p}_1^{VN-R1}=\kern0.5em \frac{2\delta {\alpha}_1+2\left(1-\delta \right){\alpha}_2+{c}_1}{3}\end{array}} $$

To maintain the positive demand, \( \updelta <\frac{\alpha_2-{c}_1}{2\left({\alpha}_1-{\alpha}_2\right)} \). To maintain the positive demand for all values of \( \updelta, \frac{\alpha_2-{c}_1}{2\left({\alpha}_1-{\alpha}_2\right)}\ge 1 \); the relationship between α1 and α2 should be satisfy the condition that \( {\alpha}_2\ge \frac{2{\alpha}_1+{c}_1}{3} \). To make non-negative profits for all supply chain members, \( \delta >\frac{c_1-{\alpha}_2}{\alpha_1-{\alpha}_2} \).

MNS model:

$$ {\displaystyle \begin{array}{c}{w}_1^{MNS-R1}=\frac{\delta {\alpha}_1+\left(1-\delta \right){\alpha}_2+{c}_1}{2}\\ {}{p}_1^{MNS-R1}=\frac{3\delta {\alpha}_1+3\left(1-\delta \right){\alpha}_2+{c}_1}{4}\end{array}} $$

To maintain the non-negative demand and profits, \( \frac{c_1-{\alpha}_2}{\alpha_1-{\alpha}_2}<\delta <\frac{\alpha_2-{c}_1}{3\left({\alpha}_1-{\alpha}_2\right)} \).

RNS model:

$$ {\displaystyle \begin{array}{c}{p}_1^{RNS-R1}=\frac{c_1+3\delta {\alpha}_1+3\left(1-\delta \right){\alpha}_2+\delta }{4}\\ {}{w}_1^{RNS-R1}=\frac{3{c}_1+\delta {\alpha}_1+\left(1-\delta \right){\alpha}_2+3\delta }{4}\end{array}} $$

To maintain the non-negative demand and profits, \( \frac{c_1-{\alpha}_2}{\alpha_1-{\alpha}_2+3}<\delta <\frac{\alpha_2-{c}_1}{3\left({\alpha}_1-{\alpha}_2\right)+1} \).

Condition 1, region 2

Retailer’s profit model:

$$ {\Pi}_{\mathrm{r}}=\left({\mathrm{p}}_1-{\mathrm{w}}_1\right)\times \left[\updelta \times \left({\alpha}_1-{\mathrm{p}}_1\right)+\left(1-\updelta \right)\times \left(\frac{p_2-{p}_1}{\ {\beta}_2-{\alpha}_2}-\frac{p_1}{\alpha_2}\right)\right]+\left({\mathrm{p}}_2-{\mathrm{w}}_2\right)\times \left[\left(1-\updelta \right)\times \left({\beta}_2-\frac{p_2-{p}_1}{\beta_2-{\alpha}_2}\right)\right] $$

Manufacturer’s profit model:

$$ {\Pi}_{\mathrm{m}1}=\left({\mathrm{w}}_1-{\mathrm{c}}_1\right)\times \left[\updelta \times \left({\alpha}_1-{\mathrm{p}}_1\right)+\left(1-\updelta \right)\times \left(\frac{p_2-{p}_1}{\beta_2-{\alpha}_2}-\frac{p_1}{\alpha_2}\right)\right] $$

Remanufacturer’s profit model:

$$ {\Pi}_{\mathrm{m}2}=\left({\mathrm{w}}_2-{\mathrm{c}}_2\right)\times \left(1-\updelta \right)\times \left({\beta}_2-\frac{p_2-{p}_1}{\beta_2-{\alpha}_2}\right) $$

VN model:

$$ {\displaystyle \begin{array}{l}\mathrm{p}{1}^{\mathrm{VN}-\mathrm{R}2}=\frac{1}{2}{w}_1^{VN-R2}+\frac{1}{2}\times \frac{\alpha_2{\beta}_2\left(1-\delta \right)+\delta {\alpha}_1{\alpha}_2}{\delta {\alpha}_2+1-\delta}\\ {}\mathrm{p}{2}^{\mathrm{VN}-\mathrm{R}2}=\frac{1}{6}{w}_1^{VN-R2}+\frac{1}{3}{c}_2+\frac{1}{2}\times \frac{\alpha_2{\beta}_2\left(1-\delta \right)+\delta {\alpha}_1{\alpha}_2}{\delta {\alpha}_2+1-\delta }+\frac{2}{3}{\beta}_2\left({\beta}_2-{\alpha}_2\right)\\ {}\begin{array}{l}\mathrm{w}{1}^{\mathrm{VN}-\mathrm{R}2}=\frac{6\times \left[\left({\beta}_2-{\alpha}_2\right)\left(\delta {\alpha}_2+1-\delta \right)+{\alpha}_2\left(1-\delta \right)\right]}{9\times \left({\beta}_2-{\alpha}_2\right)\left(\delta {\alpha}_2+1-\delta \right)+8\times {\alpha}_2\left(1-\delta \right)}\times {c}_1\\ {}+\frac{2{\alpha}_2\left(1-\delta \right)}{9\times \left({\beta}_2-{\alpha}_2\right)\left(\delta {\alpha}_2+1-\delta \right)+8\times {\alpha}_2\left(1-\delta \right)}\times {c}_2+\frac{\left({\beta}_2-{\alpha}_2\right)\left[3\delta {\alpha}_1{\alpha}_2+{\alpha}_2{\beta}_2\left(1-\delta \right)\right]}{9\times \left({\beta}_2-{\alpha}_2\right)\left(\delta {\alpha}_2+1-\delta \right)+8\times {\alpha}_2\left(1-\delta \right)}\\ {}\mathrm{w}{2}^{\mathrm{VN}-\mathrm{R}2}\kern0.5em =\frac{1}{3}{w}_1^{VN-R2}+\frac{2}{3}{c}_2+\frac{1}{3}{\beta}_2\left({\beta}_2-{\alpha}_2\right)\end{array}\end{array}} $$

MNS model:

$$ {\displaystyle \begin{array}{l}{w}_1^{MNS-R2}=\frac{2\left({\beta}_2-{\alpha}_2\right)\left(\delta {\alpha}_2+1-\delta \right)+2{\alpha}_2\left(1-\delta \right)\Big]}{4\times \left({\beta}_2-{\alpha}_2\right)\left(\delta {\alpha}_2+1-\delta \right)+3\times {\alpha}_2\left(1-\delta \right)}\times {c}_1+\frac{\alpha_2\left(1-\delta \right)}{4\times \left({\beta}_2-{\alpha}_2\right)\left(\delta {\alpha}_2+1-\delta \right)+3\times {\alpha}_2\left(1-\delta \right)}\\ {}\times {c}_2+\frac{\left({\beta}_2-{\alpha}_2\right)\left[2\delta {\alpha}_1{\alpha}_2+\left(1-\delta \right){\alpha}_2{\beta}_2\right]}{4\times \left({\beta}_2-{\alpha}_2\right)\left(\delta {\alpha}_2+1-\delta \right)+3\times {\alpha}_2\left(1-\delta \right)}\\ {}\begin{array}{l}{w}_2^{MNS-R2}=\frac{1}{2}{w}_1^{MNS-R2}+\frac{1}{2}{c}_2+\frac{1}{2}{\beta}_2\left({\beta}_2-{\alpha}_2\right)\\ {}{p}_1^{MNS-R2}=\frac{1}{2}{w}_1^{MNS-R2}+\frac{\alpha_2\left[\delta {\alpha}_1+\left(1-\delta \right){\beta}_2\right]}{2\left(\delta {\alpha}_2+1-\delta \right)}\\ {}{p}_2^{MNS-R2}=\frac{1}{2}{w}_2^{MNS-R2}+\frac{\alpha_2\left[\delta {\alpha}_1+\left(1-\delta \right){\beta}_2\right]}{2\left(\delta {\alpha}_2+1-\delta \right)}+\frac{1}{2}{\beta}_2\left({\beta}_2-{\alpha}_2\right)\end{array}\end{array}} $$

MMS model:

$$ {\displaystyle \begin{array}{l}{w}_1^{MMS-R2}=\frac{1}{2}{c}_1+\frac{1}{2}\times \frac{\left(1-\delta \right){\alpha}_2}{2\left({\beta}_2-{\alpha}_2\right)\left(\delta {\alpha}_2+1-\delta \right)+\left(1-\delta \right){\alpha}_2}{c}_2+\frac{\left({\beta}_2-{\alpha}_2\right)\left[\delta {\alpha}_1{\alpha}_2+\frac{1}{2}\left(1-\delta \right){\alpha}_2{\beta}_2\right]}{2\left({\beta}_2-{\alpha}_2\right)\left(\delta {\alpha}_2+1-\delta \right)+\left(1-\delta \right){\alpha}_2}\\ {}{w}_2^{MMS-R2}=\frac{w_1^{MMS}+{c}_2+{\beta}_2\left({\beta}_2-{\alpha}_2\right)}{2}\\ {}\begin{array}{l}{p}_1^{MMS-R2}=\frac{1}{2}{w}_1^{MMS}+\frac{\alpha_2\left[\delta {\alpha}_1+\left(1-\delta \right){\beta}_2\right]}{2\left(\delta {\alpha}_2+1-\delta \right)}\\ {}{p}_2^{MMS-R2}=\frac{1}{4}{w}_1^{MMS}+\frac{1}{4}{c}_2+\frac{3}{4}{\beta}_2\left({\beta}_2-{\alpha}_2\right)+\frac{\alpha_2\left[\delta {\alpha}_1+\left(1-\delta \right){\beta}_2\right]}{2\left(\delta {\alpha}_2+1-\delta \right)}\end{array}\end{array}} $$

RNS model:

$$ {\displaystyle \begin{array}{l}{w}_1^{RNS-R2}=-{p}_1^{RNS-R2}+\frac{\left(1-\delta \right){\alpha}_2}{A}{p}_2^{RNS-R2}+{c}_1+\frac{\delta {\alpha}_1{\alpha}_2\left({\beta}_2-{\alpha}_2\right)}{A}\\ {}{w}_2^{RNS-R2}={p}_1^{RNS-R2}-{p}_2^{RNS-R2}+{c}_2+{\beta}_2\left({\beta}_2-{\alpha}_2\right)\\ {}\begin{array}{l}{p}_1^{RNS-R2}=\frac{A\times \left[B-3\left(1-\delta \right){\alpha}_2\right]}{2C}{c}_1+\frac{\left(1-\delta \right){\alpha}_2\left(3A-B\right)}{2C}{c}_2+\frac{\alpha_2\left({\beta}_2-{\alpha}_2\right)\times B\times \left[3\delta {\alpha}_1-2\left(1-\delta \right){\beta}_2\right]}{2C}-\frac{3\left(1-\delta \right){\alpha}_1{\alpha}_2^2\left({\beta}_2-{\alpha}_2\right)}{C}+\frac{9\left(1-\delta \right){\alpha}_2{\beta}_2\left({\beta}_2-{\alpha}_2\right)\times A}{2C}\\ {}{p}_2^{RNS-R2}=\frac{2A+\left(1-\delta \right){\alpha}_2}{3\left(1-\delta \right){\alpha}_2}{p}_1^{RNS-R2}-\frac{A}{6\left(1-\delta \right){\alpha}_2}{c}_1+\frac{1}{6}{c}_2-\frac{\left({\beta}_2-{\alpha}_2\right)\left[3\delta {\alpha}_1-2\left(1-\delta \right){\beta}_2\right]}{6\left(1-\delta \right)}\end{array}\end{array}} $$

Where

$$ {\displaystyle \begin{array}{l}\mathrm{A}=\delta {\alpha}_2\left({\beta}_2-{\alpha}_2\right)+\left(1-\delta \right){\beta}_2\\ {}\mathrm{B}=2\mathrm{A}+\left(1-\updelta \right)\upalpha 2\\ {}\mathrm{C}={B}^2-9\left(1-\delta \right){\alpha}_2\times A\end{array}} $$

RMS model:

$$ {\displaystyle \begin{array}{l}{w}_1^{RMS-R2}=-\frac{\delta {\alpha}_2\left({\beta}_2-{\alpha}_2\right)+\left(1-\delta \right){\beta}_2}{\left({\beta}_2-{\alpha}_2\right)\left(\delta {\alpha}_2+1-\delta \right)}{p}_1^{RMS-R2}+\frac{\left(1-\delta \right){\alpha}_2}{\left({\beta}_2-{\alpha}_2\right)\left(\delta {\alpha}_2+1-\delta \right)}{p}_2^{RMS-R2}+{c}_1+\frac{\delta {\alpha}_1{\alpha}_2}{\delta {\alpha}_2+1-\delta}\\ {}{w}_2^{RMS-R2}={p}_1^{RMS-R2}-{p}_2^{RMS-R2}+{c}_2+{\beta}_2\left({\beta}_2-{\alpha}_2\right)\\ {}\begin{array}{l}{p}_1^{RMS-R2}=\frac{\left[\left(1-\delta \right){\alpha}_2\left(2F+A\right)-A\left(F+A\right)\right]\times F}{G}\times {c}_1-\frac{\left(1-\delta \right){\alpha}_2\times F\times F}{G}\times {c}_2-\frac{\delta {\alpha}_1{\alpha}_2\left({\beta}_2-{\alpha}_2\right)\left[\left(F+2A\right)\left(F+A\right)-2\left(1-\delta \right){\alpha}_2\left(2F+A\right)\right]}{G}-\frac{\left(1-\delta \right){\alpha}_2{\beta}_2\left({\beta}_2-{\alpha}_2\right)\times F\times \left(4F+A\right)}{G}\\ {}{p}_2^{RMS-R2}=\frac{2F+A}{F+A}\times {p}_1^{RMS-R2}-\frac{F}{2\left(F+A\right)}{c}_1+\frac{F}{2\left(F+A\right)}{c}_2+\frac{3{\beta}_2\left({\beta}_2-{\alpha}_2\right)\times F}{2\left(F+A\right)}-\frac{\delta {\alpha}_1{\alpha}_2\left({\beta}_2-{\alpha}_2\right)}{F+A}\end{array}\end{array}} $$

Where

$$ {\displaystyle \begin{array}{l}\mathrm{A}=\delta {\alpha}_2\left({\beta}_2-{\alpha}_2\right)+\left(1-\delta \right){\beta}_2,\\ {}\mathrm{F}=A-\left(1-\delta \right){\alpha}_2,\\ {}G=2\left(1-\delta \right){\alpha}_2{\left(2F+A\right)}^2-\left[4{A}^2-2{\left(1-\delta \right)}^2{\alpha_2}^2\right]\left[F+A\right]\end{array}} $$

Condition 2, region 3

Retailer’s profit model:

$$ {\Pi}_{\mathrm{r}}=\left({\mathrm{p}}_2-{\mathrm{w}}_2\right)\times \Big[\updelta \times \left({\upbeta}_1-{\mathrm{p}}_2\right)+\left(1-\updelta \right)\times \left(\upbeta 2-\mathrm{p}2\right) $$

Manufacturer’s profit model:

$$ {\Pi}_{\mathrm{m}1}=\left({\mathrm{w}}_1-{\mathrm{c}}_1\right)\times 0=0 $$

Remanufacturer’s profit model:

$$ {\Pi}_{\mathrm{m}2}=\left({\mathrm{w}}_2-{\mathrm{c}}_2\right)\times \left[\updelta \times \left({\upbeta}_1-{\mathrm{p}}_2\right)+\left(1-\updelta \right)\times \left({\upbeta}_2-{\mathrm{p}}_2\right)\right] $$

VN model:

$$ {\displaystyle \begin{array}{c}\mathrm{p}{2}^{\mathrm{VN}-\mathrm{R}3}=\frac{2\delta {\beta}_1+2\left(1-\delta \right){\beta}_2+{c}_2}{3}\\ {}\mathrm{w}{2}^{\mathrm{VN}-\mathrm{R}3}=\frac{\delta {\beta}_1+\left(1-\delta \right){\beta}_2+2{c}_2}{3}\end{array}} $$

In the Vertical Nash model, to maintain the feasibility, \( \delta <\frac{\beta_2-{c}_2}{\beta_2-{\beta}_1} \). To maintain the positive demand from at least one of the customer segments, \( \delta >\frac{\beta_2-{c}_2}{2\left({\beta}_1-{\beta}_2\right)} \).

MNS model:

$$ {\displaystyle \begin{array}{l}{w}_2^{MNS-R3}=\frac{c_2+\delta {\beta}_1+\left(1-\delta \right){\beta}_2}{2},\\ {}{p}_2^{MNS-R3}=\frac{c_2+3\delta {\beta}_1+3\left(1-\delta \right){\beta}_2}{4}\end{array}} $$

In the Manufacturer-Stackelberg model, \( \frac{\beta_2-{c}_2}{3\left({\beta}_1-{\beta}_2\right)}<\delta <\frac{\beta_2-{c}_2}{\beta_2-{\beta}_1} \).

RNS model:

$$ {\displaystyle \begin{array}{c}{p}_2^{RNS-R1}=\frac{c_2+3{\delta \beta}_1+3\left(1-\delta \right){\beta}_2}{4}\\ {}{w}_2^{RNS-R1}=\frac{3{c}_2+{\delta \beta}_1+\left(1-\delta \right){\beta}_2}{4}\end{array}} $$

In the Retailer-Stackelberg model, \( \frac{\beta_2-{c}_2}{3\left({\beta}_1-{\beta}_2\right)}<\delta <\frac{\beta_2-{c}_2}{\beta_2-{\beta}_1} \).

Condition 2, region 4

Retailer’s profit model:

$$ \varPi r=\left(p1-w1\right)\times \left[\delta\ \left({\alpha}_1-\frac{p_1-{p}_2}{\alpha_1-{\beta}_1}\right)\right]+\left({p}_2-{w}_2\right)\times \left[\delta\ \left(\frac{p_1-{p}_2}{\alpha_1-{\beta}_1}-\frac{p_2}{\beta_1}\right)+\left(1-\delta \right)\left({\beta}_2-{p}_2\right)\right] $$

Manufacturer’s profit model:

\( {\Pi}_{\mathrm{m}1}=\left({\mathrm{w}}_1-{\mathrm{c}}_1\right)\times \left[\updelta\ \right({\alpha}_1-\frac{p_1-{p}_2}{\alpha_1-{\beta}_1} \))]

Remanufacturer’s profit model:

\( {\Pi}_{\mathrm{m}2}=\left({w}_2-{\mathrm{c}}_2\right)\times \Big[\updelta\ \left(\frac{p_1-{p}_2}{\alpha_1-{\beta}_1}-\frac{p_2}{\beta_1}\right)+\left(1-\delta \right)\left({\beta}_2-{p}_2\right) \)].

VN model:

$$ {\displaystyle \begin{array}{l}{\mathrm{p}}_1^{\mathrm{VN}-\mathrm{R}3}=\frac{1}{6}{w}_2+\frac{1}{3}{c}_1+\frac{2}{3}{\alpha}_1\left({\alpha}_1-{\beta}_1\right)+\frac{\delta {\alpha}_1{\beta}_1+\left(1-\delta \right){\beta}_1{\beta}_2}{2\delta +2{\beta}_1\left(1-\delta \right)}\\ {}{\mathrm{p}}_2^{\mathrm{VN}-\mathrm{R}3}=\frac{1}{2}{w}_2+\frac{\delta {\alpha}_1{\beta}_1+\left(1-\delta \right){\beta}_1{\beta}_2}{2\delta +2{\beta}_1\left(1-\delta \right)}\\ {}\begin{array}{l}{\mathrm{w}}_1^{\mathrm{VN}-\mathrm{R}3}=\frac{1}{3}{w}_2+\frac{2}{3}{c}_1+\frac{\alpha_1\left({\alpha}_1-{\beta}_1\right)}{3}\\ {}{\mathrm{w}}_2^{\mathrm{VN}-\mathrm{R}3}=\kern0.5em \frac{2\delta {\beta}_1}{8\delta {\beta}_1+7\left({\alpha}_1-{\beta}_1\right)\left[\delta +\left(1-\delta \right){\beta}_1\right]}{c}_1+\frac{6\left({\alpha}_1-{\beta}_1\right)\left[\delta +{\beta}_1\left(1-\delta \right)\right]+6\delta {\beta}_1}{8\delta {\beta}_1+7\left({\alpha}_1-{\beta}_1\right)\left[\delta +{\beta}_1\left(1-\delta \right)\right]}{c}_2+\frac{\beta_1\left({\alpha}_1-{\beta}_1\right)\left[7{\alpha}_1\delta +9{\beta}_2\left(1-\delta \right)\right]}{7\left({\alpha}_1-{\upbeta}_1\right)\left[\delta +{\beta}_1\left(1-\delta \right)\right]+8\delta {\beta}_1}\end{array}\end{array}} $$

MNS model:

$$ {\displaystyle \begin{array}{l}{w}_1^{MNS-R4}=\frac{w_2+{c}_1+{\alpha}_1\left({\alpha}_1-{\beta}_1\right)}{2}\\ {}{w}_2^{MNS-R4}=\frac{\delta {\beta}_1}{4\left({\alpha}_1-{\beta}_1\right)\left[\left(1-\delta \right){\beta}_1+\delta \right]+3\delta {\beta}_1}\ {c}_1+\frac{2\left({\alpha}_1-{\beta}_1\right)\left[\left(1-\delta \right){\beta}_1+\delta \right]+2\delta {\beta}_1}{4\left({\alpha}_1-{\beta}_1\right)\left[\left(1-\delta \right){\beta}_1+\delta \right]+3\delta {\beta}_1}\ {c}_2+\frac{\left({\alpha}_1-{\beta}_1\right)\left[\delta {\alpha}_1{\beta}_1+2\left(1-\delta \right){\beta}_1{\beta}_2\right]}{4\left({\alpha}_1-{\beta}_1\right)\left[\left(1-\delta \right){\beta}_1+\delta \right]+3\delta {\beta}_1}\\ {}\begin{array}{l}{p}_1^{MNS-R4}=\frac{w_2+{c}_1+3{\alpha}_1\left({\alpha}_1-{\beta}_1\right)}{4}+\frac{1}{2}\times \frac{\delta {\alpha}_1{\beta}_1+\left(1-\delta \right){\beta}_1{\beta}_2}{\delta +\left(1-\delta \right){\beta}_1}\\ {}{p}_2^{MNS-R4}=\frac{1}{2}{w}_2^{MNS-R4}+\frac{1}{2}\times \frac{\delta {\alpha}_1{\beta}_1+\left(1-\delta \right){\beta}_1{\beta}_2}{\delta +\left(1-\delta \right){\beta}_1}\end{array}\end{array}} $$

MMS model:

$$ {\displaystyle \begin{array}{l}{w}_1^{MMS-R4}=\frac{1}{2}{c}_1+\frac{\left(1-\delta \right){\beta}_1\left({\alpha}_1-{\beta}_1\right)+\delta {\alpha}_1}{\left({\alpha}_1-{\beta}_1\right)\left[2\left(1-\delta \right){\beta}_1+\delta \right]}{c}_2+\frac{\left(1-\delta \right){\beta}_1{\beta}_2\left({\alpha}_1-{\beta}_1\right)}{4\left[\left(1-\delta \right){\beta}_1\left({\alpha}_1-{\beta}_1\right)+\delta {\alpha}_1\right]-2\delta {\beta}_1}+\frac{\frac{1}{2}{\alpha}_1\left({\alpha}_1-{\beta}_1\right)}{1-\frac{1}{2}\times \frac{\delta {\beta}_1}{\left(1-\delta \right){\beta}_1\left({\alpha}_1-{\beta}_1\right)+\delta {\alpha}_1}}\\ {}{w}_2^{MMS-R4}=\frac{\frac{1}{2}\delta {\beta}_1}{\left(1-\delta \right){\beta}_1\left({\alpha}_1-{\beta}_1\right)+\delta {\alpha}_1}{w}_1^{MMS-R4}+{c}_2+\frac{\frac{1}{2}\left(1-\delta \right){\beta}_1{\beta}_2\left({\alpha}_1-{\beta}_1\right)}{\left(1-\delta \right){\beta}_1\left({\alpha}_1-{\beta}_1\right)+\delta {\alpha}_1}\\ {}\begin{array}{l}{p}_1^{MMS-R4}=\frac{1}{2}{w}_1^{MMS-R4}+\frac{1}{2}\times \frac{\delta {\alpha}_1{\beta}_1+\left(1-\delta \right){\beta}_1{\beta}_2}{\delta +\left(1-\delta \right){\beta}_1}+\frac{1}{2}{\alpha}_1\left({\alpha}_1-{\beta}_1\right)\\ {}{p}_2^{MMS-R4}=\frac{1}{2}{w}_2^{MMS-R4}+\frac{1}{2}\times \frac{\delta {\alpha}_1{\beta}_1+\left(1-\delta \right){\beta}_1{\beta}_2}{\delta +\left(1-\delta \right){\beta}_1}\end{array}\end{array}} $$

RNS model:

$$ {\displaystyle \begin{array}{l}{w}_1^{RNS-R4}={w}_1=-{p}_1^{RNS-R4}+{p}_2^{RNS-R4}+{c}_1+{\alpha}_1\left({\alpha}_1-{\beta}_1\right)\\ {}{w}_2^{RNS-R4}=\frac{\delta {\beta}_1}{D}{p}_1^{RNS-R4}-{p}_2^{RNS-R4}+{c}_2+\frac{\left(1-\delta \right){\beta}_1{\beta}_2\left({\alpha}_1-{\beta}_1\right)}{D}\\ {}\begin{array}{l}{p}_1^{RNS-R4}=\frac{2D+\delta {\beta}_1}{3\delta {\beta}_1}\ {p}_2^{RNS-R4}+\frac{1}{6}{c}_1-\frac{D}{6\delta {\beta}_1}{c}_2+\frac{\left({\alpha}_1-{\beta}_1\right)\left[2\delta {\alpha}_1-3\left(1-\delta \right){\beta}_2\right]}{6\delta}\\ {}{p}_2^{R\mathrm{N}S-R4}=\frac{\delta {\beta}_1\left(D-\delta {\beta}_1\right)}{2E}{c}_1+\frac{D\left(D-\delta {\beta}_1\right)}{E}{c}_2-\frac{\beta_1\left({\alpha}_1-{\beta}_1\right)\left[2\delta {\alpha}_1\left(2D+\delta {\beta}_1\right)-3\left(1-\delta \right){\beta}_2\left(2D-\delta {\beta}_1\right)\right]}{2E}+\frac{9\delta {\alpha}_1{\beta}_1\left({\alpha}_1-{\beta}_1\right)D}{2E}\end{array}\end{array}} $$

Where D = (1 − δ)β1(α1 − β1) + δα1, and E = (2D + δβ1)2 − 9δβ1D.

RMS model:

$$ {\displaystyle \begin{array}{l}{w}_1^{RMS-R4}=-\frac{D}{D-\delta {\beta}_1}{p}_1^{RMS-R4}+\frac{D}{D-\delta {\beta}_1}{p}_2^{RMS-R4}+{c}_1+\frac{\alpha_1\left({\alpha}_1-{\beta}_1\right)\times D}{D-\delta {\beta}_1}\\ {}\begin{array}{l}{w}_2^{RMS-R4}=\frac{\delta {\beta}_1}{D}{p}_1^{RMS-R4}-{p}_2^{RMS-R4}+{c}_2+\frac{\left(1-\delta \right){\beta}_1{\beta}_2\left({\alpha}_1-{\beta}_1\right)}{D}\\ {}{p}_1^{RMS-R4}=\frac{\left[{\delta}^2{\beta}_1\left(3D-2\delta {\beta}_1\right)-\delta D\left(2D-\delta {\beta}_1\right)\right]\times \left(D-\delta {\beta}_1\right)}{H}{c}_1+\frac{\delta D\left(-D+\delta {\beta}_1\right)\left(D-\delta {\beta}_1\right)}{H}{c}_2-\frac{\updelta {\alpha}_1D\left(3D-\delta {\beta}_1\right)\left(2D-\delta {\beta}_1\right)\left({\alpha}_1-{\beta}_1\right)}{H}+\frac{2{\delta}^2{\alpha}_1{\beta}_1D\left(3D-2\delta {\beta}_1\right)\left({\alpha}_1-{\beta}_1\right)}{H}+\frac{\delta \left(1-\delta \right){\beta}_1{\beta}_2\left(-5D+4\delta {\beta}_1\right)\left(D-\delta {\beta}_1\right)\left({\alpha}_1-{\beta}_1\right)}{H}\\ {}{p}_2^{RMS-R4}=\frac{\delta {\beta}_1\left(3D-2\delta {\beta}_1\right)}{D\left(2D-\delta {\beta}_1\right)}{p}_1^{RMS-R4}-\frac{\delta {\beta}_1\left(D-\delta {\beta}_1\right)}{2D\left(2D-\delta {\beta}_1\right)}{c}_1+\frac{D\left(D-\delta {\beta}_1\right)}{2D\left(2D-\delta {\beta}_1\right)}{c}_2-\frac{\delta {\alpha}_1{\beta}_1\left({\alpha}_1-{\beta}_1\right)}{\left(2D-\delta {\beta}_1\right)}+\frac{3\left(1-\delta \right){\beta}_1{\beta}_2\left(D-\delta {\beta}_1\right)\left({\alpha}_1-{\beta}_1\right)}{2D\left(2D-\delta {\beta}_1\right)}\end{array}\end{array}} $$

Where D = ( 1 − δ ) β1 ( α1 − β1 ) + δα1, and H= 2 δ2 β1 ( 3D − 2 δ β1 )2 − 2 δ ( 2 D2 − δ2 β12 ) ( 2D − δ β1 )

Factor analysis

This section will apply numerical examples in the four regions to explore the impact of the two factors. The first factor is different value perceptions between quality-conscious customers and technology-savvies. The perceived quality of remanufactured products is different between the two segments. Customer knowledge development could vary the difference over time. The same applies to the distinct perception between the two customer groups towards technology depreciation. The technology innovation of the new generation does not always move at the same pace. The perception of technology advantage towards remanufactured products in the two segments changes differently based on how large the new generation takes a step towards innovation. The perception differences in quality and technology are discussed in the following paragraph. The second factor is the relative size of each customer segment. The population of quality-conscious customers and technology-savvies in the potential markets varies by region. Therefore, δ, which represented the proportion of quality-conscious customers, has different values in various area. We will analyse the impact of the different relative sizes of each group.

All models in the four regions comply with the non-negative demand and non-negative profit rules. The solution is not applicable if δ is out of range. we assure α1 = β2 = 1, c1 = 0.2, c2 = 0.1. The difference between the perceived value of the two customer segments ranged from 0.1 to 0.3. The profits for the retailer, manufacturer, and the supply chain in each region under different conditions are exhibited in Fig. 4 through Fig. 13.

In Region 1, only the retailer and manufacturer are in the game. As shown in Fig. 4, the lower acceptance level for Type 1 products from technology-savvies decreases all earnings. The higher proportion of quality-conscious customers contributes to less profit for the manufacturer and the supply chain but more for the retailer in the Retailer-Stackelberg model. However, in the Nash Equilibrium model and the Manufacturer-Stackelberg model, all members receive more benefit with a higher ratio of quality-conscious customers. This phenomenon is due to theoretically negative demands from technology-savvies, calculated as zero when the quality-conscious group is the majority. In terms of each supply chain member, the retailer will obtain maximum profit in the Retailer-Stackelberg model when δ is less than 0.1. However, with an increased value of δ, the Nash Equilibrium model provides more to the retailer. For the manufacturer, the Manufacturer-Stackelberg model is always optimal. Given the whole supply chain, the Nash Equilibrium is the optimal game system.

In Region 2, all supply chain members are in the game. Figure 5 through Fig. 8 exhibit the profit for the retailer, manufacturer, remanufacturer, and the supply chain in different game theory models under different value differentiations. The retailer’s earning is decreased with a higher perceived value difference between two customer groups in all game theories. When δ value is up, the profits are decreased in general, except for the RNS model, which allows a limited range of δ value. The result of the MNS model is more sensitive to δ. When the difference is as low as 0.1, the MNS model brings the retailer the most benefit, while when δ rises to 0.3, the MNS becomes the least optimal model. Instead, the VN model is optimal when δ is 0.2 and 0.3.

According to the figures for the manufacturer, the tendency is reverse to that of the retailer. VN model is generally the least optimal for the manufacturer because Type 1 product is unattractive to technology-savvies in this numerical example. Besides, when δ is going up, the profit climbs, except for the RNS model. That is understandable when the quality-conscious group’s population is getting more generous, and more people are willing to buy Type 1 products at a higher price as the perceived value is higher than the other group of customers. MNS model is optimal when the difference is more negligible, and δ is at the lower rate. RMS is optimal for the rest of the condition, except for when δ is higher than 0.9, MMS replaces it as an optimal model.

As a horizontal competitor, the remanufacturer, when δ is going up, the profit is decreased in general. RMS model is the selected policy when the difference is low. Type 2 product is welcomed by using price level to attract some quality-conscious customers; even the manufacturer is a leader. However, with an enlarging perceived value differentiation, less quality-conscious customers will choose Type 2 products, and MMS is selected. RNS is optimal at the restricted δ value range around 0.8 when the value difference is 0.2.

Given the whole supply chain, the MNS model is optimal only when the perceived value differences between the two customer segments are slight. When the difference gets larger, the VN model is better if the quality-conscious customer population ratio is less than 50 %; otherwise, the RMS model is more favorite.

In Region 3, the retailer and remanufacturer are in the game. The applicable conditions are w2 > c2, p2 > w2, and p2 <β2. If β2 >p2 >β1, the demand from quality-conscious customers is zero.

As expressed in Fig. 9, an increased value of δ leads to less benefit for all. The results are consistent with intuition. The retailer prefers the RS model while the remanufacture gets more from the MS model. The profit of the supply chain picks the NV model. The inconsiderable difference between two customer groups helps all supply chain members gain more.

In Region 4, we calculated the wholesale prices and retail price for each product and the profits for the retailer, manufacturer, remanufacturer, and supply chain when the value differentiation between α1 and β1 is 0.1, 0.2, and 0.3, respectively. The RMS model is not applicable when the difference is 0.1. Figure 10 through Fig. 13 show the trends.

The tendency of the retailer’s profit is close to that of Region 2. However, the optimal price strategy is various. When the difference in perceived value is slight, the RNS, VN, and the MMS model are optimal in the different specific ranges of δ. When the difference in perceived value is moderate or considerable, the RNS model is selected if quality-conscious customers are the majority. In contrast, the VN model is optimum if technology-savvies occupy the main population. The VN model is always superior to the manufacturer, especially when the value gap is significant. For the remanufacturer, the MMS model is selected in most of the conditions. The VN model replaces the optimal selection in some situations, as shown in the figure. In the supply chain, the VN model is recommended in most practices. However, if the quality-conscious customers’ population is too substantial or too bare, the MMS model may take place mainly when the value difference is minor.

Conclusion

This study analyzes the price strategies for new and remanufactured high-technology products across generations, particularly for the new products belonging to the early generation and the remanufactured products belonging to the latest generation. The manufacturer sells new commodities to the retailer while the remanufacturer sells remanufactured items to the same retailer. Two types of items have different inferiors. The remanufactured item has a less perceived quality while the new item has an obsolesced technology. Customers categorized as quality-conscious and technology-savvy are heterogeneous in their valuation of the two products. The two items’ demands are based on the customer’s perceived value and price, divided into four regions. In the first region, customers only buy Type 1 products. In the second region, quality-conscious customers only buy Type 1, and some technology-savvies will choose Type 1. In the third region, the retailer only sells Type 2 products. In the fourth region, technology-savvies only buy Type 2 while some quality-conscious customers would like to purchase Type 2. The different five game theory models determine the distinct optimal wholesale prices and retail prices. The results provide interesting implications.

First, Type 1 and Type 2 products co-exist when prices are appropriately determined. If the price difference between the two items is more significant than the perceived value gap, the market will eliminate one item. Second, an optimal intuitional solution is not always be selected. Balancing the power of all supply chain members is the optimal choice in general. For the vertical competition, the retailer prefers the VN model rather than the Retailer-Stackelberg model; in some conditions, the retailer even decides the Manufacturer-Stackelberg model. The manufacturer and remanufacturer have the same phenomenon. The manufacturer picks the VN model but sometimes chooses the Retailer-Stackelberg strategy. The remanufacturer prefers the Retailer-Stackelberg policy more. However, if only one type of commodity is in the market (Region 1 and 3), leading the market is optimal. In the horizontal competition between the manufacturer and remanufacturer, making a balanced power is better than leading the manufacturer market. Remanufacturer even prefers the manufacturer to lead the game. Therefore, it is wise to mitigate competition among all the supply chain members in most cases. Leading the market is not knowledgeable in typical cases, mainly when two products are in the market. Sometimes, acting as a follower is the right choice. Although the VN model is optimal in the prominent cases for the whole supply chain, the Retailer-Stackelberg and Manufacturer-Stackelberg model are also recommended in some conditions. Third, to make all supply chain members’ profits non-negative, the value of δ has the lower and upper boundary in disparate perceived value gaps and different game models. The applicability is highly dependent on the state of customers’ willingness to pay and their segments. Finally, the retailer and the joint supply chain will receive more benefit if the proportion of quality-conscious customers is smaller than that of the technology-savvies in most cases. Therefore, customer education about remanufacturing is essential to the general market performance.

Due to the variation in each game theory model under different conditions, the adequate understanding of customer’s perceived value on technology and quality and the proper investigation of the proportion of customer group population is essential to make a pricing policy. The decision-maker can find an optimal strategy based on the figures in Appendix 2 after taking a survey about perceptions from the targeted people and calculating each segment’s population in the specific region. Additionally, expanding the technology-savvies group helps the retailer and the supply chain gain more profit unless the manufacturer has significant leading power. Therefore, increasing customers’ acceptance of remanufacturing is critical to improving the circular marketing and remanufacturing strategy.

This paper is the first pricing decision study for new and remanufactured products across generations under different game theory models to the best of the knowledge. Only a limited amount of research articles pay attention to the new and remanufactured products across generations [29,30,31,32]. It pointed out the critical market issues for new and remanufactured high-technology products with different quality and technology advantages. New products are not always superior to remanufactured products. The study provides recommendations to maximize the profits for all supply chain members in different situations. However, only two product generations are engaged in the research. Future studies could extend the price model to a multiple generation line, which is more like real-world condition.