A comparative study for convective flow of basefluid (gasoline oil), nanomaterial (SWCNTs) and hybrid nanomaterial (SWCNTs + MWCNTs)

Abstract

In this communication, we have investigated flow of hybrid nanomaterial (SWCNTs + MWCNTs) by a curved stretched surface. Relative analysis of nanomaterial (SWCNTs) and hybrid nanomaterial (SWCNTs + MWCNTs) is performed. Gasoline oil is treated as basefluid. Heat transfer features are elaborated via thermal radiation and convection. Equations relevant to flow field (PDEs) are transmitted into ODEs through adequate transformations. Solutions are developed via the shooting method. Furthermore, relative analysis of basefluid (gasoline oil), nanomaterial (SWCNTs) and hybrid nanomaterial (SWCNTs + MWCNTs) is presented through higher estimations of influential flow parameters during observation of flow, skin friction coefficient, temperature and Nusselt number.

Introduction

Recent developments in industrial and thermal processes directly depend upon the requirement of processing more compact and efficient heat transfer equipments. To fulfill such requirements the scientists and engineers have made many attempts to design various equipment and fluids for the advance of heat transfer rate. The consequence of such attempts is that solid materials are better thermal conductors when compared with liquids. Presently various liquids are used for cooling purposes as cooling agents. To improve thermal conductance of such liquids, the scientists and engineers added some small (nano) sized particles into it. Such nano-sized particles are referred as nanoparticles. Nanoparticles are made of metal (copper, gold etc.), carbides, oxides (alumina, titania) and copper oxides etc. Kerosine oil, ethylene glycol, bioliquids, water and some lubricants are utilized as traditional liquids. The suspension of such nano-sized particles in basematerial is known as nanofluid. There are various shapes of nanoparticles like cylindrical, spherical, blades, bricks etc. It has been observed that thermal conductance of base liquid highly depends upon nanoparticles shape. Better performance in terms of enhancing thermal conductance of the baseliquid is observed for cylindrical shape nanoparticles (nanotubes). Carbon nanotubes are cylindrical shape material with one (single-wall) or more (multi-wall) layers of graphene. There are extensive applications of CNTs in energy storages, microelectronics, coating and films, purification of drinking water, defense and sports materials etc. Initial analysis on nanofluid was performed by Choi and Eastman (1995). Applications of nanofluid comprises systems of drug delivery, refrigerant, solar collectors, solar cells and many more. Chemical reactions and melting phenomenon in flow of CNTs is presented by Hayat et al. (2018a). Khan et al. (2019) examined entropy production minimization in flow of Carreau nanomaterial. Chemical reactions, radiation and melting effect in flow of CNTs are studied by Hayat et al. (2018b). Heat generation and entropy production in MHD flow of nanomaterial is analyzed by Hosseini et al. (2019). Convection in flow of copper/water nanomaterial due to rotatory cone is examined by Dinarvand and Pop (2017). Melting heat, chemical reactions and radiation in flow of CNTs by a curved sheet in presented by Hayat et al. (2019a). Mahian et al. (2018) performed modern analysis in modeling and simulation of nanomaterial flow. Comparative analysis amongst nanomaterial, basefluid and hybrid nanomaterial is performed by Muhammad et al. (2020). Nowadays various experimental works has been performed by the researchers on dispersion of more than one nanoparticle in the same basefluid. This mixture of two or more nanoparticles and basefluid is referred as hybrid nanofluid. Hybrid nanofluid possesses better thermal features when compared with nanofluid. A review works on the development and applications of hybrid nanomaterial is performed by Sarkar et al. (2015). Some recent work on nanomaterial and hybrid nanomaterial can be seen in Hayat and Nadeem (2017), Huminic and Huminic (2019), Meribout (2019), Meribout et al. (2018, 2019), Xu et al. (2019a, b), Sajid and Ali (2018), Sun et al. (2019).

Flow due to the stretchable sheet is discussed extensively due to its wide range of technological as well as industrial applications. The quality of final produced material can be effected deeply by the heat transfer rate at the stretchable surface. In this domain, the initial study was performed by Crane (1970). Heat transport via melting phenomenon in flow Oldroyd-B material over a stretched sheet is studied by Hayat et al. (2018c). MHD and joule heating effect in ${\text{Fe}}_{{3}} {\text{O}}_{{4}}$-flow of nanomaterial is elaborate by Sajid et al. (2016). Hayat et al. (2019b) studied entropy production and activation energy in flow of Ree-Eyring nanomaterial over a stretching sheet. Blasious flow of nanomaterial using Buongiorno’s model is investigated by Naveed et al. (2016). Some recent analyses on flow over stretched surface can be seen in Singeetham and Puttanna (2019), Ali et al. (2017), Muhammad et al. (2019).

Literature survey indicates that in previously published works the researchers have investigated the flow of nanomaterial over a flat stretchable surface. Very little work is addressed yet regarding hybrid nanomaterial. Motivation behind the presented investigation is to study the flow of hybrid nanofluid by a curved stretched surface. CNTs (SWCNTs, MWCNTs) are dispersed in basefluid of gasoline oil. Characteristics of heat transportation are elaborated via thermal radiation and convection. Solutions are developed by the shooting method. Relative analysis for basefluid (gasoline oil), nanomaterial (SWCNTs) and hybrid nanomaterial (SWCNTs + MWCNTs) is emphasized.

Formulations

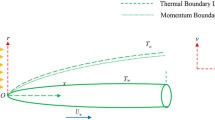
We have assumed flow of hybrid nanomaterial (SWCNTs + MWCNTs) by a curved stretching surface. Heat transport features are analyzed in presence of thermal radiation and convection. Nanofluid is prepared by adding SWCNTs (single-walled CNTs) in basefluid of gasoline oil while hybrid nanofluid is constructed by adding CNTs (single-walled and multiple-walled) in basefluid of gasoline oil. In Curvilinear coordinates system, $s{\text{ } - \text{ axis}}$ is taken along thecurved sheet while $r{\text{ } - \text{ axis}}$ normal to it. Single-wall CNTs are treated as first while multiple-wall CNTs are treated as second nanoparticles. We have assumed velocity V = [v(r, s), u(r, s), 0]. General forms of involved equations (continuity, momentum and energy) are (Muhammad et al. 2020):

$$ \frac{\partial \rho }{{\partial t}} + \nabla \cdot (\rho {\mathbf{V}}) = 0, $$

(1)

$$ \rho_{hnf} \left( {\frac{{\partial {\mathbf{V}}}}{\partial t} + ({\mathbf{V}} \cdot \nabla ){\mathbf{V}}} \right) = \nabla \cdot {{\varvec{\uptau}}} + \rho b, $$

(2)

$$ (\rho c_{p} )_{hnf} \left( {\frac{\partial T}{{\partial t}} + ({\mathbf{V}} \cdot \nabla )T} \right) = {\text{Tr}}({{\varvec{\uptau}}} \cdot {\mathbf{L}}) - \nabla \cdot {\mathbf{q}} + {\mathbf{q}}_{r} . $$

(3)

In aforementioned expressions, Eq. (1) is referred as continuity equation. The derivation of this equation is based on conservations law of mass. Equation (2) is called the momentum equation. Derivation of this equation is based on Newton’s second law. In this equation, left-hand side represents inertial forces while the first term on right-hand side is surface force and second term the body forces. Equation (3) is called the energy equation and its derivation is on the basis of the first law of thermodynamics. Here L.H.S represents total internal energy of the system while the first term on R.H.S is due to viscous dissipation, second for Fourier’s law of heat conduction and a third term for radiated heat flux. Here ${\mathbf{q}} = - k_{hnf} \nabla T$ represents heat flux. We are interested in studying the two-dimensional flow of an incompressible hybrid nanofluid in absence of thermal radiation and viscous dissipation. Thus after implementing aforementioned assumptions, the expressions are:

$$ (r + R)\frac{\partial v}{{\partial r}} + v + R\frac{\partial u}{{\partial s}} = 0, $$

(4)

$$ \frac{{u^{2} }}{r + R} = \frac{1}{{\rho_{hnf} }}\frac{\partial p}{{\partial r}}, $$

(5)

$$ \begin{aligned} v\frac{\partial u}{{\partial r}} + \frac{R}{r + R}u\frac{\partial u}{{\partial s}} + \frac{uv}{{r + R}} = & - \frac{1}{{\rho_{hnf} }}\frac{R}{r + R}\frac{\partial p}{{\partial s}} \\ & + \upsilon_{hnf} \left( {\frac{{\partial^{2} u}}{{\partial r^{2} }} + \frac{1}{r + R}\frac{\partial u}{{\partial r}} - \frac{u}{{(r + R)^{2} }}} \right), \\ \end{aligned} $$

(6)

$$ \begin{aligned} \left( {v\frac{\partial T}{{\partial r}} + \frac{R}{r + R}u\frac{\partial T}{{\partial s}}} \right) = & & \frac{{k_{hnf} }}{{(\rho c_{p} )_{hnf} }}\left( {\frac{1}{r + R}\frac{\partial T}{{\partial r}} + \frac{{\partial^{2} T}}{{\partial r^{2} }}} \right)\left( {1 + \frac{{16\sigma^{ * } T_{\infty } }}{{3k^{ * } }}} \right) \\ & + \frac{{Q^{ * } }}{{(\rho c_{p} )_{hnf} }}(T - T_{\infty } ), \\ \end{aligned} $$

(7)

with boundary conditions

$$ \begin{gathered} u = U_{w} (s) = U_{0} s,\,\;\;v = 0,\,\;\;\, - k_{hnf} \frac{\partial T}{{\partial r}} = \gamma_{0} (T_{f} - T){\text{ at }}r = 0, \hfill \\ u \to 0,\,\;\;T \to T_{\infty } \;\;{\text{as }}r \to \infty . \hfill \\ \end{gathered} $$

(8)

We choose the transformations:

$$ \begin{gathered} u = U_{0} sf^{\prime}(\eta ),\,\;\;v = - \frac{R}{r + R}\sqrt {\frac{{U_{0} \upsilon_{f} }}{2l}} f(\eta ),\,\;\;\eta = \sqrt {\frac{{U_{0} }}{{\upsilon_{f} }}} r, \hfill \\ \theta (\eta ) = \frac{{T - T_{\infty } }}{{T_{w} - T_{\infty } }},\,\;\;p = \rho_{f} U_{0}^{2} s^{2} P(\eta ). \hfill \\ \end{gathered} $$

(9)

Implementing these transformations on the above flow expressions, continuity equation is verified identically while other equations with boundary conditions become:

$$ P^{\prime}(\eta ) = A_{11} \frac{{f^{\prime 2} }}{\eta + \gamma }, $$

(10)

$$ \begin{aligned} \frac{2\gamma }{{\eta + \gamma }}P(\eta ) =\,\, & \frac{{(1 - \phi_{1} )^{2.5} (1 - \phi_{2} )^{2.5} \tfrac{\gamma }{\eta + \gamma }}}{{A_{11} }}\left( {ff^{\prime\prime} - f^{\prime 2} - \frac{\gamma }{\eta + \gamma }f^{\prime 2} + \frac{1}{\eta + \gamma }ff^{\prime}} \right) \\ & - \frac{{f^{\prime}}}{{(\eta + \gamma )^{2} }} + \frac{{f^{\prime\prime}}}{\eta + \gamma } + f^{\prime\prime\prime}, \\ \end{aligned} $$

(11)

$$ (1 + Rd)\frac{{k_{hnf} }}{{k_{f} }}\left( {\theta^{\prime\prime} + \frac{{\theta^{\prime}}}{\eta + \gamma }} \right) + B_{11} \mathop {\Pr }\limits \left( {\frac{\gamma }{\eta + \gamma }f\theta^{\prime} + \delta \theta } \right) = 0. $$

(12)

Elimination of $P(\eta )$ from Eqs. (10) and (11) yields

$$ \begin{gathered} \frac{{A_{11} }}{{\left( {1 - \phi_{1} } \right)^{2.5} \left( {1 - \phi_{2} } \right)^{2.5} }}\left( {f^{\prime \prime \prime \prime } + \frac{2}{\eta + \gamma }f^{\prime\prime\prime} + \frac{{f^{\prime}}}{{(\eta + \gamma )^{3} }} - \frac{1}{{(\eta + \gamma )^{2} }}f^{\prime\prime}} \right) \hfill \\ \qquad + \frac{\gamma }{\eta + \gamma }\left( {ff^{\prime\prime\prime} - f^{\prime}f^{\prime\prime} + \frac{{ff^{\prime\prime}}}{\eta + \gamma } - \frac{{f^{\prime 2} }}{\eta + \gamma } - \frac{{ff^{\prime}}}{{(\eta + \gamma )^{2} }}} \right) = 0, \hfill \\ \end{gathered} $$

(13)

with

$$ \begin{gathered} f^{\prime}(0) = 1,\,\;\;\theta^{\prime}(0) = \frac{{k_{f} }}{{k_{hnf} }}\beta (1 - \theta (0)),\,\;\;f(0) = 0, \hfill \\ f^{\prime}(\eta ) \to 0,\,\;\;f^{\prime\prime}(\eta ) \to 0,\, \, \theta (\eta ) \to 0{\text{ as }}\eta \to \infty , \hfill \\ \end{gathered} $$

(14)

$$ A_{11} = \frac{1}{{\left( {1 - \phi_{2} } \right)\,\left( {\left( {1 - \phi_{1} } \right) + \phi_{1} \tfrac{{\rho_{{s_{1} }} }}{{\rho_{f} }}} \right) + \phi_{2} \tfrac{{\rho_{{_{{s_{2} }} }} }}{{\rho_{f} }}}}, $$

(15)

$$ B_{11} = \left( {1 - \phi_{2} } \right)\,\left( {\left( {1 - \phi_{1} } \right) + \phi_{1} \frac{{(\rho c_{p} )_{{s_{1} }} }}{{(\rho c_{p} )_{f} }}} \right) + \phi_{2} \frac{{(\rho c_{p} )_{{s_{2} }} }}{{(\rho c_{p} )_{f} }}. $$

(16)

Physical parameters involved in flow field are defined by

$$ \begin{gathered} \gamma = \sqrt {\frac{{U_{0} }}{{\upsilon_{f} }}} R,\,\;\;\mathop {\Pr }\limits = \frac{{\upsilon_{f} }}{{\alpha_{f} }},\,\;\;\delta = \frac{{Q^{ * } }}{{U_{0} (\rho c_{p} )_{f} }}, \hfill \\ \beta = \frac{{\gamma_{0} }}{{k_{f} }}\sqrt {\frac{{\upsilon_{f} }}{{U_{0} }}} ,\,\;\;Rd = \frac{{16\sigma^{ * } T_{\infty }^{3} }}{{3k_{f} }}. \hfill \\ \end{gathered} $$

(17)

Expression for $C_{fs}$ (skin friction coefficient) and ${\text{Nu}}_{s}$ (Nusselt number)

Dimensionless expression of $C_{fs}$ and ${\text{Nu}}_{s}$ are

$$ C_{fs} = \frac{{(\tau_{w} )_{r = 0} }}{{\rho U_{w}^{2} }},\,\;\;{\text{Nu}}_{s} = \frac{{s(q_{w} )_{r = 0} }}{{k_{f} (T_{w} - T_{\infty } )}}, $$

(18)

$$ \tau_{w} = \mu_{hnf} \left( {\frac{\partial u}{{\partial r}} - \frac{u}{r + R}} \right),\,\;\;q_{w} = - k_{hnf} \frac{\partial T}{{\partial r}}. $$

(19)

Non-dimensional version is

$$ \sqrt {{\text{Re}}_{s} } C_{fs} = \frac{1}{{(1 - \phi_{1} )^{2.5} (1 - \phi_{2} )^{2.5} }}\left( {f^{\prime\prime}(0) - \frac{1}{\gamma }f(0)} \right), $$

(20)

$$ \frac{{{\text{Nu}}_{s} }}{{\sqrt {{\text{Re}}_{s} } }} = - \frac{{k_{hnf} }}{{k_{f} }}\theta^{\prime}(0). $$

(21)

Here $Re_{s} = \tfrac{{U_{w} (s)s}}{{\upsilon_{f} }}$ is the local Reynold number.

Himelton–Crosser model for hybrid nanofluid and nanofluid

Himelton–Crosser expressions for nanofluid and hybrid nanofluid are (Hayat and Nadeem 2017)

$$ \begin{gathered} \rho_{nf} = \left( {1 - \phi_{1} } \right)\,\rho_{f} + \phi_{1} \rho_{{s_{1} }} , \hfill \\ (\rho c_{p} )_{nf} = \left( {1 - \phi_{1} } \right)(\rho c_{p} )_{f} + \phi_{1} (\rho c_{p} )_{{s_{1} }} , \hfill \\ \frac{{\kappa_{nf} }}{{\kappa_{f} }} = \frac{{\kappa_{{s_{1} }} + (n - 1)\kappa_{f} - (n - 1)\phi_{1} (\kappa_{f} - \kappa_{{s_{1} }} )}}{{\kappa_{{_{{s_{1} }} }} + (n - 1)\kappa_{f} + \phi_{1} (\kappa_{f} - \kappa_{{s_{1} }} )}}, \hfill \\ \mu_{nf} = \frac{{\mu_{f} }}{{\left( {1 - \phi_{1} } \right)^{2.5} }},\,\;\upsilon_{nf} = \frac{{\mu_{nf} }}{{\rho_{nf} }}, \hfill \\ \end{gathered} $$

(22)

and

$$ \begin{gathered} \rho_{hnf} = \left( {1 - \phi_{2} } \right)\,\left( {\left( {1 - \phi_{1} } \right)\,\rho_{f} + \phi_{1} \rho_{{s_{1} }} } \right) + \phi_{2} \rho_{{s_{2} }} , \hfill \\ (\rho c_{p} )_{hnf} = \left( {1 - \phi_{2} } \right)\,\left( {\left( {1 - \phi_{1} } \right)(\rho c_{p} )_{f} + \phi_{1} (\rho c_{p} )_{{s_{1} }} } \right) + \phi_{{2(\rho c_{p} )s_{2} }} , \hfill \\ \frac{{\kappa_{hnf} }}{{\kappa_{nf} }} = \frac{{\kappa_{{s_{2} }} + (n - 1)\kappa_{nf} - (n - 1)\phi_{2} (\kappa_{nf} - \kappa_{{s_{2} }} )}}{{\kappa_{{_{{s_{2} }} }} + (n - 1)\kappa_{nf} + \phi_{2} (\kappa_{nf} - \kappa_{{s_{2} }} )}}, \hfill \\ \frac{{\kappa_{nf} }}{{\kappa_{f} }} = \frac{{\kappa_{{s_{1} }} + (n - 1)\kappa_{f} - (n - 1)\phi_{1} (\kappa_{f} - \kappa_{{s_{1} }} )}}{{\kappa_{{_{{s_{1} }} }} + (n - 1)\kappa_{f} + \phi_{1} (\kappa_{f} - \kappa_{{s_{1} }} )}}, \hfill \\ \mu_{hnf} = \frac{{\mu_{f} }}{{\left( {1 - \phi_{1} } \right)^{2.5} \left( {1 - \phi_{2} } \right)^{2.5} }},\,\;\upsilon_{hnf} = \frac{{\mu_{hnf} }}{{\rho_{hnf} }}. \hfill \\ \end{gathered} $$

(23)

In the above expressions, $n$ is known by shape parameter such that $n = 6$ represents cylindrical-shaped nanoparticles. Thus in our analysis, we have taken $n = 6$.

Method for solutions

The coupled non-linear ODEs are solved by shooting technique using with Runge–Kutta 4th-order algorithm (bvp4c: A matlab tool). This technique is applicable for first order ODEs. Thus we have adopted this method as $y_{0} = f,$

$$ y_{1} = f^{\prime}, $$

(24)

$$ y_{2} = f^{\prime\prime}, $$

(25)

$$ y_{3} = f^{\prime\prime\prime}, $$

(26)

$$ \begin{aligned} Y_{0} = y_{3}^{\prime } = & - A_{11} (1 - \phi_{1} )^{2.5} (1 - \phi_{2} )^{2.5} \frac{\gamma }{\eta + \gamma }\left( {y_{0} y_{3} - y_{1} y_{2} + \frac{{y_{0} y_{2} }}{\eta + \gamma } - \frac{{y_{1}^{2} }}{\eta + \gamma } - \frac{{y_{0} y_{1} }}{{(\eta + \gamma )^{2} }}} \right) \\ & - \frac{2\gamma }{{\eta + \gamma }}y_{3} - \frac{{y_{1} }}{{(\eta + \gamma )^{3} }} + \frac{{y_{2} }}{{(\eta + \gamma )^{2} }}, \\ \end{aligned} $$

(27)

$$ y_{4} = \theta , $$

(28)

$$ y_{5} = \theta^{\prime}, $$

(29)

$$ Y_{1} = y_{5}^{\prime } = - \frac{{B_{11} }}{1 + Rd}\frac{{k_{f} }}{{k_{hnf} }}\mathop {\Pr }\limits \left( {\frac{\gamma }{\eta + \gamma }y_{0} y_{5} + \delta y_{4} } \right) - \frac{{y_{5} }}{\eta + \gamma }, $$

(30)

with

$$ \begin{gathered} y_{1} (0) = 1,\,\;\;y_{5} (0) = \frac{{k_{f} }}{{k_{hnf} }}\beta (1 - y_{4} (0)),\,\;\;y_{0} (0) = 0, \hfill \\ y_{1} \to 0,\,\;\;y_{2} \to 0,\, \, y_{4} \to 0{\text{ as }}\eta \to \infty . \hfill \\ \end{gathered} $$

(31)

Analysis

Aim behind this analysis section is to perform or to elaborate relative study among basefluid (gasoline oil), nanomaterial (SWCNTs) and hybrid nanomaterial (SWCNTs + MWCNTs). $C_{fs}$ (Skin friction coefficient), $f^{\prime}(\eta )$ (velocity of fluid), $\theta (\eta )$ and ${\text{Nu}}_{s}$ (Nusselt number) are graphically visualized against higher estimations of $\beta ,\,\;\phi_{1} ,$ $\gamma ,$ $\phi_{2}$ and $Rd$. Such graphical analysis is performed as below:

1. a-graphs are plotted for relative analysis between nanomaterial (SWCNTs) and hybrid nanomaterial (SWCNTs + MWCNTs) corresponding to each physical parameter.

2. b-graphs are plotted for relative analysis among basefluid (gasoline oil), nanomaterial (SWCNTs) and hybrid nanomaterial (SWCNTs + MWCNTs).

Analysis of velocity ($f^{\prime}(\eta )$)

Flow ($f^{\prime}(\eta )$) against the larger estimation of $\phi_{1}$ during relative analysis of nanomaterial (SWCNTs) and hybrid nanomaterial (SWCNTs + MWCNTs) is presented in Fig. 1. Velocity $f^{\prime}(\eta )$ is maximized with increment in $\phi_{1}$. Also prominent impact is shown by a hybrid nanofluid. Volocity $f^{\prime}(\eta )$ due to larger values of $\gamma$ is sketched in Fig. 2. Direct relation between $f^{\prime}(\eta )$ and $\gamma$ is observed. Physically an increment in a radius of curved surface occurs with higher $\gamma$. Thus more fluid particles attain stretched surface velocity and thus $f^{\prime}(\eta )$ enhances. However, the prominent effect for hybrid nanofluid (SWCNTs + MWCNTs) is noticed. Figure 3 is plotted for relative analysis of nanomaterial (SWCNTs) and hybrid nanomaterial (SWCNTs + MWCNTs) during studying impact for higher $\phi_{2}$ on $f^{\prime}(\eta )$. Velocity $f^{\prime}(\eta )$ increases with larger $\phi_{2}$ and prominent impact is detected for hybrid nanofluid. Relative analysis for basefluid (gasoline oil), nanofluid (SWCNTs) and hybrid nanofluid (SWCNTs + MWCNTs) about $f^{\prime}(\eta )$ when $\phi_{1} =$ $\phi_{2} = 0.1$ is presented in Figs. 4, 5, 6, respectively. As expected the prominent behavior is noticed for hybrid nanofluid (SWCNTs + MWCNTs) followed by nanofluid (SWCNTs) and basefluid (gasoline oil), respectively.

Fig. 1

$f^{\prime}(\eta )$ vs $\phi_{1}$

Fig. 2

$f^{\prime}(\eta )$ vs $\gamma$

Fig. 3

$f^{\prime}(\eta )$ vs $\phi_{2}$

Fig. 4

$f^{\prime}(\eta )$ vs $\phi_{1}$ (compraison)

Fig. 5

$f^{\prime}(\eta )$ vs $\phi_{2}$ (compraison)

Fig. 6

$f^{\prime}(\eta )$ vs $\gamma$ (compraison)

Analysis for $\theta (\eta )$ (temperature)

Temperature of the fluid ($\theta (\eta )$) via higher estimation of $\phi_{1}$ is labeled in Fig. 7. Direct behavior is noticed for $\theta (\eta )$ via higher $\phi_{1}$. Physically an increment in $\phi_{1}$ intensifies thermophysical characteristics of basefluid due to which convective flow from heated surface towards cold fluid intensifies. Hence $\theta (\eta )$ increases. Furthermore, impact of hybrid nanofluid (SWCNTs + MWCNTs) is dominant. Figure 8 is plotted for variation in $\theta (\eta )$ against increment in $\gamma$. Temperature $\theta (\eta )$ enhances with enlargement in $\gamma$ and dominant behavior is noticed for nanofluid (SWCNTs). Variations in $\theta (\eta )$ due to higher estimation of $\beta$ is portrayed in Fig. 9.Temperature $\theta (\eta )$ directly varies with increasing values of $\beta$. Physically higher $\beta$ correspond to an increase in rate of heat transfer and thus $\theta (\eta )$ increases. Moreover, the impact of nanofluid (SWCNTs) is prominent when compared with hybrid nanofluid (SWCNTs + MWCNTs). Figure 10 is sketched for variations in $\theta (\eta )$ against higher $Rd$. Enlargement in $\theta (\eta )$ is observed for higher $Rd$. Physically higher $Rd$ leads to the production of more heat via radiation process and so $\theta (\eta )$ intensifies. Nanofluid (SWCNTs) shows prominent impacts as compared to hybrid nanofluid (SWCNTs + MWCNTs). Figure 11 is labeled for variations in $\theta (\eta )$ due to higher estimation of $\phi_{2}$. It is noticed that $\theta (\eta )$ intensifies with enlargement in $\phi_{2}$. Higher $\phi_{2}$ is associated with a more convective flow from the heated surface towards cold fluid above the surface. Thus $\theta (\eta )$ intensifies. Furthermore, the impact of hybrid nanomaterial (SWCNTs + MWCNTs) is dominant over nanofluid (SWCNTs). Temperature $\theta (\eta )$ against $\delta > 0$ (heat source parameter) and $\delta < 0$ (heat sink parameter) is plotted in Fig. 12. It is revealed that $\theta (\eta )$ intensifies for $\delta > 0$ while it reduces for $\delta < 0$. Further influence of nanofluid (SWCNTs) is more when compared with hybrid nanofluid (SWCNTs + MWCNTs). Figures 13, 14, 15, 16, 17, 18 presents relative study among basefluid (gasoline oil), nanomaterial (SWCNTs) and hybrid nanomaterial (SWCNTs + MWCNTs) during the analysis of $\theta (\eta )$ when $\phi_{1} = 0.01$ and $\gamma = \beta = \phi_{2} = Rd = \delta = 0.1$, respectively. It is dugout that performance of hybrid nanomaterial (SWCNTs + MWCNTs) is better which is followed by nanofluid (SWCNTs) and basefluid (gasoline oil) when $\phi_{1} = 0.01$ and $\phi_{2} = 0.1$ while the impact of basefluid (gasoline oil) is massive which is followed by nanofluid (SWCNTs) and hybrid nanofluid (SWCNTs + MWCNTs) when $\gamma = \beta = Rd = \delta = 0.1$.

Fig. 7

$\theta (\eta )$ vs $\phi_{1}$

Fig. 8

$\theta (\eta )$ vs $\gamma$

Fig. 9

$\theta (\eta )$ vs $\beta$

Fig. 10

$\theta (\eta )$ vs $Rd$

Fig. 11

$\theta (\eta )$ vs $\phi_{2}$

Fig. 12

$\theta (\eta )$ vs $\delta$

Fig. 13

$\theta (\eta )$ vs $\phi_{1}$ (comparison)

Fig. 14

$\theta (\eta )$ vs $\gamma$ (comparison)

Fig. 15

$\theta (\eta )$ vs $\beta$ (comparison)

Fig. 16

$\theta (\eta )$ vs $Rd$ (comparison)

Fig. 17

$\theta (\eta )$ vs $\phi_{2}$ (comparison)

Fig. 18

$\theta (\eta )$ vs $\delta$ (comparison)

Analysis of $C_{fs}$ (skin friction coefficient) and ${\text{Nu}}_{s}$ (Nusselt number)

$C_{fs \, }$ against higher estimations of $\phi_{1} ,$ $\phi_{2}$ and $\gamma$ is portrayed in Figs. 19, 20. It is founded that $C_{fs}$ enhances both $\phi_{1}$ and $\phi_{2}$ while it decays with higher $\gamma$. Further impact of hybrid nanomaterial (SWCNTs + MWCNTs) is more than nanomaterial (SWCNTs). Relative analysis of basefluid (gasoline oil), nanomaterial (SWCNTs) and hybrid nanomaterial (SWCNTs + MWCNTs) during computation of $C_{fs}$ when $\gamma = \phi_{1} = 0.1$ is performed in Figs. 21, 22. Better performance is seen for hybrid nanomaterial (SWCNTs + MWCNTs) followed by nanomaterial (SWCNTs) and basefluid (gasoline oil), respectively. However, for $\phi_{2} = 0.1$ the basefluid (gasoline oil) result is followed by nanofluid (SWCNTs) and hybrid nanofluid (SWCNTs). ${\text{Nu}}_{s}$ against higher $\gamma ,$ $\beta ,$ $\phi_{1}$ and $\phi_{2}$ is labeled in Figs. 23 and 24. It is found that $Nu_{s}$ intensifies with higher $\gamma ,$ $\beta ,$ $\phi_{1}$ and $\phi_{2}$. Moreover, the impact of hybrid nanomaterial (SWCNTs + MWCNTs) is more than nanomaterial (SWCNTs). Figures 25, 26 are sketched for the relative study of basefluid (gasoline oil), nanomaterial (SWCNTs) and hybrid nanofluid (SWCNTs + MWCNTs) during computation of ${\text{Nu}}_{s}$ when $\gamma = \phi_{1} = 0.1$. Efficient behavior is observed for hybrid nanomaterial (SWCNTs + MWCNTs) followed by nanomaterial (SWCNTs) and basefluid (gasoline oil). Nomenclature of involved physical parameters and expressions is presented in Abbreviations. Table 1 is constructed for thermal features of CNTs (SWCNTs + MWCNTs) and basefluid (gasoline oil).

Fig. 19

$C_{f}$ vs $\phi_{1}$ and $\phi_{2}$

Fig. 20

$C_{f}$ vs $\gamma$ and $\phi_{2}$

Fig. 21

$C_{f}$ vs $\gamma$ and $\phi_{2}$ (comparison)

Fig. 22

$C_{f}$ vs $\gamma$ and $\phi_{2}$ (comparison)

Fig. 23

${\text{Nu}}$ vs $\phi_{1}$ and $\phi_{2}$

Fig. 24

${\text{Nu}}$ vs $\gamma$ and $\beta$

Fig. 25

${\text{Nu}}$ vs $\phi_{1}$ and $\phi_{2}$ (comparison)

Fig. 26

${\text{Nu}}$ vs $\gamma$ and $\beta$ (comparison)

Table 1 Thermal features of CNTs (single-walled, multiple-walled) and basefluid (gasoline oil) (Crane 1970)

Final findings

In presented work, we have examined flow of hybrid nanofluid (SWCNTs, MWCNTs) by a curved stretching sheet. The vital findings are.

• Higher $f^{\prime}(\eta )$ (velocity of fluid) is noticed with increment in $\phi_{1} ,$ $\gamma$ and $\phi_{2}$.

• $\theta (\eta )$ (temperature of fluid) rises with increment in values of $\gamma ,$ $\beta$ and $Rd$ while opposite behavior of $\theta (\eta )$ is seen with increment in $\phi_{1}$ and $\phi_{2}$.

• Accurately $\theta (\eta )$ rises with heat source parameter ($\delta > 0$) while it decays with heat sink parameter ($\delta < 0$).

• $C_{f}$ (Skin friction coefficient) enlarges with increment in values of $\phi_{1}$ and $\phi_{2}$ while it reduces with $\gamma$.

• ${\text{Nu}}_{s}$ (Nusselt number) intensifies with increment in values of $\gamma ,$ $\beta ,$ $\phi_{1}$ and $\phi_{2}$.

• Impacts of hybrid nanomaterial (SWCNTs + MWCNTs) are more than nanomaterial (SWCNTs) and basefluid (gasoline oil).