Introduction

In recent days, the researchers seem to improve the aspects of heat transport rate due to its practical applications in industrial and engineering processes like atomic reactor coolants, nanocryosurgery, transportation, bio-therapeutic engineering, glass fiber innovations, magnetohydrodynamic power generators, automobile transformer, and geothermal power productivity. The base liquids like ethylene glycol, blood, oil, and water have inadequate thermal conductivity. To develop the performance of thermal conductivity of ordinary coolants, the insertion of nano-sized metal oxide and metals to ordinary liquids yields enhancement in heat transfer competence, energy productivity, and thermophysical qualities. Indeed, nanomaterials are homogeneous dispersion of solid particles and ordinary regular fluids and having extraordinary ability to boost thermal characteristics of normal liquids. The idea of such liquids was first originated by Choi (1995), but Masuda et al. (1993) termed such newly generated liquids as nanoliquids. Nanoliquid cannot exceed from 10 nm. Buongiorno (2006) discussed convective transport in nanoparticles and revealed that thermophoresis and Brownian factors yield improvement in thermal characteristics of carrier liquids. Farooq et al. (Farooq et al. 2016) modeled non-linear thermal radiative flow in MHD viscoelastic nanofluid to the region of stagnant point. Hayat et al. (2015, 2017a, b, c) reported nanoliquid dynamics considering various aspects. Sheikholeslami et al. (2017) established numerical solutions for Feimage-water nanomaterials considering convective heat transport and magnetic source. Sheikholeslami and Ganji (2015) considered MHD flow to establish the solutions for CuO–H2O nanoparticles and Al2O3. Impacts of stratification and magnetic field on Maxwell nanofluid along with motile gyrotactic microorganisms is outlined by Ijaz Khan et al. (2017). Sheikholeslami and Ganji (2017) utilized Marangoni convective transport subjected to magnetic field to examine the CuO–H2O nanofluid features. Shahzad et al. (2016) provided extensive study on the usefulness of magneto Oldroyd-B nanoliquids considering radiative cavity. Combined effects of thermal/pumping power utilizing nanoliquid subject to wavy medium are addressed by Akbarzadeh et al. (2016). Characteristics of Fe3O4-water nanomaterials to improve heat transport features comprising coulomb forces are modeled by Sheikholeslami (2017). Further fruitful attempts towards nanoliquids dynamics are elaborated in Acharya et al. (2016a, b, c, 2017, 2018, 2019), Das et al. (2016, 2018), Waqas et al. (2019), and Dogonchi et al. (2019).

The phenomena of non-linear thermal radiation have significant contribution on fluid flow, since it has diverse utilization in areas of mechanical and physical engineering. Especially, the non-linear thermal radiation has massive hold to improve heat transport features in space technology, polymers processing, atomic power plants, cooling of metals during manufacturing, and gas turbine combustors. Mushtaq et al. (2014) considered solar energy aspects in nanofluid flow for non-linear thermally radiative heat transfer analysis. Hayat et al. (2015) accounted slip effects for nanoliquids in the existence of uniform magnetic field and non-linearly thermal radiative flow. Shahzad et al. (2014) evaluated the series solutions by assuming the non-linear thermal radiation on three-dimensional Jeffery nanoliquid flow. Hussain et al. (2016) investigated non-linear thermally radiative mixed convection flow via stagnant point in a vertical stretchable cavity. Impacts of non-linear thermal radiative and magnetic field on upper convected Maxwell liquid by a convectively heated stretchable surface along with dust particles is executed by Krupalakshmi et al. (2016). Mahanthesh et al. (2016) explored three-dimensional flow of water-based nanoliquid via variable stretchable surface utilizing convective boundary condition. Khan et al. (2016) described the characteristics of non-linear thermal radiative flow in Burgers nanoliquid subject to new mass flux effect. Numerical solutions utilizing convective cylinder for non-linear thermal radiative flow are developed by Hayat et al. (2016). Further studies about non-linear radiated surface are given in Khan et al. (2017), Hayat et al. (2018), Dogonchi et al. (2019), Waqas et al. (2019), and Acharya et al. (2019).

Mass transfer mechanism exhibits when there is concentration difference of species in a mixture from a higher concentration locality to lower concentration locality. Furthermore, most of the reactant species must have acquired least quantity of energy to bend before undergoing a specified chemical reaction. This energy is known as activation energy. Activation energy and chemical reaction in the process of mass transport analysis are often greatly attracted the attention of researchers for instance nuclear reactor cooling, chemical processes in industry, hydrolytic constancy in drug discovery, food processing, thermal oil recovery, and in the combustion of a fuel. Bestman (1990) first derived the analytic solutions for natural convection flow in the existence of activation energy associated with chemical reaction on permeable surface utilizing perturbation approach. Makinde et al. (2011) numerically investigated activation energy along with chemical reaction in the binary mixture for unsteady natural convection heat transfer. Simultaneous features of exothermic/endothermic chemical reaction on MHD-free convection flow in the presence of modified Arrhenius activation energy are disclosed by Maleque et al. (2013). Awad et al. (2014) considered activation energy and chemical reaction to scrutinize the unsteady rotating liquid flow. Effectiveness of modified Arrhenius function along with binary chemical reaction on Maxwell liquid subject to rotating frame are addressed by Shafique et al. (2016). Abbas et al. (2016) derived numerical solutions for Casson liquid considering simultaneous characteristics of activation energy and chemical reaction in the region of stagnant point. They utilized spectral-collocation quasi-linearization approach to develop the solutions for ordinary differential system.

The goal here is to model and explore the impacts of activation energy on mixed convective magneto Eyring–Powell nanomaterials fluid flow subjected to non-linear radiation. Thermophoresis and Brownian motion aspects are comprised by nanoparticles. Moreover, concept of Joule heating, heat generation, and newly suggested mass flux boundary condition are also reported. Shooting technique (Waqas et al. 2016; Mustafa et al. 2017; Hayat et al. 2018; Irfan et al. 2019; Ali et al. 2019; Irfan and Khan 2019) is utilized to compute the differential equations. Here, the article has been designed in the succeeding manner. "Modeling" section contributes to mathematical modeling. "Results and discussion" section characterizes the detailed aspects of dimensionless velocity, temperature, and concentration profiles. "Conclusions" section reports the features of sundry variables for non-dimensionalized profiles by presenting graphs and tables.

Modeling

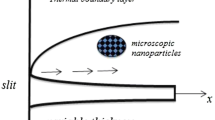
We intend to formulate the two-dimensional incompressible mixed convective Eyring–Powell nanomaterial flow towards moving surface. We consider magnetic effect with uniform strength \(B_{0}\) acting normal to the surface (see Fig. 1). Aspects of thermophoretic and Brownian diffusions are retained for nanofluid modeling. Heat transport analysis further comprises non-linear radiation and heat generation. A novel chemical reaction model considering activation energy characteristics is introduced. Furthermore, flux condition regarding nanoparticles is also imposed at the boundary. Keeping in view the aforestated assumptions, the boundary layer approximation governs the following expressions (Mustafa et al. 2017; Hayat et al. 2017):

$$\frac{\partial u}{\partial x} + \frac{\partial v}{\partial y} = 0,$$
(1)
$$\begin{aligned} u\frac{\partial u}{\partial x} + v\frac{\partial u}{\partial y} & = \left( {\nu + \frac{1}{{\rho_{f} \beta C^*}}} \right)\frac{{\partial^{2} u}}{{\partial y^{2} }} - \frac{1}{{2\rho_{f} \beta C^*}}\left( {\frac{\partial u}{\partial y}} \right)^{2} \frac{{\partial^{2} u}}{{\partial y^{2} }} - \frac{{\sigma^{*} B_{0}^{2} }}{{\rho_{f} }}u \\ & \quad + \frac{1}{{\rho_{f} }}\left[ {(1 - C_{\infty } )\rho_{f\infty } \beta (T - T_{\infty } ) - (\rho_{p} - \rho_{f\infty } )(C - C_{\infty } )} \right]g, \\ \end{aligned}$$
(2)
$$\begin{aligned} u\frac{\partial T}{\partial x} + v\frac{\partial T}{\partial y} & = \alpha \frac{{\partial^{2} T}}{{\partial y^{2} }} + \tau \left[ {D_{\text{B}} \frac{\partial C}{\partial y}\frac{\partial T}{\partial y} + \frac{{D_{\text{T}} }}{{T_{\infty } }}\left( {\frac{\partial T}{\partial y}} \right)^{2} } \right] \\ & \quad + \frac{{16\sigma^{ * * } }}{{3(\rho c)_{f} m^{ * } }}\frac{\partial }{\partial y}\left( {T^{3} \frac{\partial T}{\partial y}} \right) \\ & \quad + \frac{{\sigma^{*} B_{0}^{2} }}{{(\rho c)_{f} }}u^{2} + \frac{{Q_{0} }}{{(\rho c)_{f} }}\left( {T - T_{\infty } } \right), \\ \end{aligned}$$
(3)
$$\left. {u\frac{\partial C}{\partial x} + v\frac{\partial C}{\partial r} = D_{\text{B}} \frac{{\partial^{2} C}}{{\partial y^{2} }} + \frac{{D_{\text{T}} }}{{T_{\infty } }}\left( {\frac{{\partial^{2} T}}{{\partial y^{2} }}} \right) - k_{r}^{2} (C - C_{\infty } )\left( {\frac{T}{{T_{\infty } }}} \right)^{n} \exp \left( { - \frac{{E_{\text{a}} }}{\kappa T}} \right)} \right).$$
(4)
Fig. 1
figure 1

Physical configuration

Full size image

Here, \(\left( {x,y} \right)\) direction of velocity components are \(\left( {u,v} \right)\), \(\nu \left( { = \frac{\mu }{{\rho_{f} }}} \right)\) the kinematic velocity, \(\rho_{f}\) the density, \(\mu\) the dynamic viscosity, \(\left( {\beta ,C} \right)\) the material liquid parameters, \(\tau \left( { = \frac{{\left( {\rho c} \right)_{p} }}{{\left( {\rho c} \right)_{f} }}} \right)\) the ratio of heat capacity, \(\sigma^{*}\) the electrical conductivity of the fluid, \(\sigma^{**}\) the Stefan–Boltzmann constant, \(\left( {B_{0} ,m^{*} } \right)\) the (magnetic field strength, mean absorption coefficient), \(\alpha \left( { = \frac{k}{{\left( {\rho c} \right)_{f} }}} \right)\) the thermal diffusivity, \(k\) the thermal conductivity, \(\left( {D_{\text{B}} ,D_{\text{T}} } \right)\) the (Brownian, thermophoresis) diffusion coefficients, \(Q_{0}\) the heat generation/absorption parameter, \(\left( {T,C} \right)\) the (temperature, concentration), \(\left( {T_{\infty } ,C_{\infty } } \right)\) the ambient (temperature, concentration), \(k_{\text{r}}^{2}\) the reaction rate, \(n\) the fitted rate constant, \(\kappa\) the Boltzmann constant, and \(E_{\text{a}}\) the activation energy.

Boundary conditions

The aforementioned assumptions yield the boundary conditions in the following forms (Mahanthesh et al. 2016):

$$\begin{aligned} u = & U_{w} (x) = cx,\, \, v = 0,\, \, T = T_{w} ,\, \, D_{\text{B}} \frac{\partial C}{\partial y} + \frac{{D_{\text{T}} }}{{D_{\infty } }}\frac{\partial T}{\partial y}{\text{ at }}y = 0, \\ u \to & 0,\, \, T \to T_{\infty } ,\, \, C \to C_{\infty } {\text{ when }}y \to \infty , \\ \end{aligned}$$
(5)

where \(U_{w} (x) = cx\) illustrates the stretching velocity.

Transformations

We utilize

$$\begin{aligned} \xi = & y\sqrt {\frac{c}{\nu }} ,\, \, u = cxf^{{\prime }} (\xi ),\, \, v = - \sqrt {c\nu } f(\xi ),\, \\ \theta (\xi ) = & \frac{{T - T_{\infty } }}{{T_{w} -T_{\infty } }},\, \, \phi (\xi ) = \frac{{C - C_{\infty } }}{{C_{\infty } }}, \\ \end{aligned}$$
(6)

in which, \(\left( {f(\xi ),\;\theta (\xi ),\;\phi (\xi )} \right)\) are the dimensionless (temperature, velocity, and concentration). Equation (1) is satisfied identically for \(u = cxf^{\prime}(\xi )\) and \(v = - \sqrt {c\nu } f(\xi )\).

Transformed problems

Substituting expression (6) into the expressions (2)–(5), we get the differential systems as follows:

One has

$$\left( {1 + \varepsilon } \right)f^{\prime \prime \prime } + ff^{\prime \prime } - f^{\prime 2} - Mf^{\prime } + \lambda (\theta - {\text{Nr}}\phi ) - \varepsilon \delta f^{\prime \prime 2} f^{\prime \prime \prime } = 0,$$
(7)
$$\begin{aligned}&\left( {\left( {1 + \frac{4}{3}R\left( {1 + \left( {\theta_{w} - 1} \right)\theta } \right)^{3} \theta^{\prime } } \right)} \right)^{\prime } + \mathop {\Pr }\limits f\theta^{\prime} + \mathop {\Pr }\limits N_{b} \phi^{\prime } \theta^{\prime } \\& + \mathop {\Pr }\limits N_{t} \theta^{\prime 2} + \Pr {\text{Ec}}Mf^{\prime 2} + \Pr S\theta = 0, \end{aligned}$$
(8)
$$\phi^{\prime \prime } + Scf\phi^{\prime } + \frac{{N_{t} }}{{N_{b} }}\theta^{\prime \prime } - Sc\sigma \left( {1 + \delta_{1} \theta } \right)^{n} \exp \left( { - \frac{E}{{1 + \delta_{1} \theta }}} \right) = 0,$$
(9)
$$f\left( 0 \right) = 0,\, \, f^{{\prime }} \left( 0 \right) = 1,\, \, f^{{{\prime }}} \left( \infty \right) \to 0,\,$$
(10)
$$\theta \left( 0 \right) = 1,\, \, \theta \left( \infty \right) \to 0,$$
(11)
$${\text{Nb}}\phi^{\prime } \left( 0 \right) + {\text{Nt}}\theta^{\prime } \left( 0 \right) = 0, \, \phi \left( \infty \right) \to 0.$$
(12)

Here, prime \(\left( {^{\prime } } \right)\) denotes differentiation with respect to \(\xi ,\) \(\varepsilon \left( { = \frac{1}{\mu \beta C^*}} \right)\) and \(\delta \left( =\frac{c^{3}x^{2}}{2\left( C^{\ast }\right) ^{2}\nu }\right)\) the fluid parameters, \(M\left( { = \frac{{\sigma^{*} B_{0}^{2} }}{{\rho_{f} c}}} \right)\) the magnetic parameter, \({\text{Nr}}\) \(\left( { = \tfrac{{\left( {\rho_{p} - \rho_{f\infty } } \right)C_{\infty } }}{{\left( {1 - C_{\infty } } \right)\rho_{f\infty } \beta \left( {T_{w} - T_{\infty } } \right)}}} \right)\) the buoyancy ratio parameter, \(R = \tfrac{{4\sigma^{ * * } T_{\infty }^{3} }}{{{\text{km}}^{ * } }}\) the radiation parameter, \(\theta_{w} = \tfrac{{T_{w} }}{{T_{\infty } }}\) the temperature ratio parameter, \(\lambda \left( { = \tfrac{{g\beta \left( {1 - C_{\infty } } \right)\left( {T_{w} - T_{\infty } } \right)}}{{c^{2} x}} = \frac{{{\text{Gr}}_{x} }}{{Re_{x}^{2} }}} \right)\) the mixed convection parameter, \(\Pr \left( { = \tfrac{\nu }{\alpha }} \right)\) the Prandtl number, \({\text{Nt}} = \left( {\tfrac{{\tau D_{\text{T}} \left( {T_{w} - T_{\infty } } \right)}}{{\nu T_{\infty } }}} \right)\) the thermophoresis parameter, \({\text{Nb}} = \left( {\tfrac{{\tau D_{\text{B}} C_{\infty } }}{\nu }} \right)\) the Brownian motion parameter, \({\text{Ec}} = \frac{{c^{2} x^{2} }}{{c_{f} \left( {T_{w} - T_{\infty } } \right)}}\) the Eckert number, \({\text{S}} = \frac{{Q_{0} }}{{\left( {\rho c} \right)_{f} c}}\) the heat generation/absorption parameter, \({\text{Sc}} = \tfrac{\nu }{{D_{\text{B}} }}\) the Schmidt number, \(\sigma = \left( {\tfrac{{k_{\text{r}}^{2} }}{c}} \right)\) the dimensionless reaction rate, \(\delta_{1} = \frac{{T_{w} - T_{\infty } }}{{T_{\infty } }}\) the temperature difference parameter, and \(E = \tfrac{{E_{\text{a}} }}{{\kappa T_{\infty } }}\) the dimensionless activation energy.

Physical quantities

Surface drag force \((Cf_{x} )\) and heat transfer rate \(({\text{Nu}}_{x} )\) are expressed as follows:

$$Cf_{x} = \frac{{\tau_{w} }}{{\rho_{f} U_{w}^{2} }},\,$$
(13)
$${\text{Nu}}_{x} = \frac{{xq_{w} }}{{k (T_{w} - T_{\infty } )}},\,$$
(14)

where the wall shear stress \(\tau_{w}\) and the wall heat flux \(q_{w}\) are defined as follows:

$$\tau _{w}=\left( 1+\frac{1}{\beta C^{\ast }}\right) \frac{\partial u}{\partial y}-\frac{1}{6\beta \left( C^{\ast }\right) ^{3}}\left( \frac{\partial u}{\partial y}\right) ^{3}$$
(15)
$$q_{w} = \left[ { - k\frac{\partial T}{\partial y} - \frac{{16\sigma^{**} }}{{3m^{*} }}\left( {T^{3} \frac{\partial T}{\partial y}} \right)} \right]_{y = 0} .$$
(16)

Invoking Eqs. (15) and (16) in the Eqs. (13) and (14), we have the following:

$$Cf_{x} Re_{x}^{1/2} = \left( {1 + \varepsilon } \right)f^{\prime \prime } \left( 0 \right) - \frac{\delta \varepsilon }{3}\left( {f^{\prime \prime } \left( 0 \right)} \right)^{3} ,$$
(17)
$${\text{Nu}}_{x} Re_{x}^{ - 1/2} = - \left\{ {1 + \frac{4}{3}R\left( {1 + \left( {\theta_{w} - 1} \right)\theta \left( 0 \right)} \right)^{3} } \right\}\theta '\left( 0 \right),$$
(18)

where \(Re_{x} \left( = \frac{{xU_{w} }}{\nu } \right)\) signifies local Reynolds number.

Results and discussion

Here, the idea of shooting scheme is utilized for the computations of non-linear systems (7)–(9) subject to (10)–(12). Influences of magnetic parameter \(M\), material parameter \(\varepsilon\), thermal radiation parameter \(R\), Buoyancy ratio parameter \({\text{Nr}}\), temperature ratio parameter \(\theta_{w}\), heat generation parameter \(S\), thermophoresis parameter \({\text{Nt}}\), Brownian motion parameter \({\text{Nb}}\), mixed convection parameter \(\lambda\), Prandtl parameter \(\Pr\), Eckert number \({\text{Ec}}\), activation energy parameter \(E\), non-dimensionalized reaction rate \(\sigma\) and the Schmidt number \(Sc\) on velocity \(f'(\xi )\), temperature \(\theta (\xi )\), and concentration \(\phi (\xi )\) are highlighted in this section. Figures 1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12, 13, 14, 15, 16, 17 are plotted to elaborate the behavior of these variables.

Fig. 2
figure 2

\(\varepsilon\) against \(f^{{\prime }} \left( \xi \right)\)

Full size image
Fig. 3
figure 3

\(M\) against \(f^{{\prime }} \left( \xi \right)\)

Full size image
Fig. 4
figure 4

\(\lambda\) against \(f^{{\prime }} \left( \xi \right)\)

Full size image
Fig. 5
figure 5

\(Nr\) against \(f^{{\prime }} \left( \xi \right)\)

Full size image
Fig. 6
figure 6

\(R\) against \(\theta \left( \xi \right)\)

Full size image
Fig. 7
figure 7

\(\theta_{w}\) against \(\theta \left( \xi \right)\)

Full size image
Fig. 8
figure 8

\({\text{Nt}}\) against \(\theta \left( \xi \right)\)

Full size image
Fig. 9
figure 9

\(M\) against \(\theta \left( \xi \right)\)

Full size image
Fig. 10
figure 10

\(\Pr\) against \(\theta \left( \xi \right)\)

Full size image
Fig. 11
figure 11

\({\text{Ec}}\) against \(\theta \left( \xi \right)\)

Full size image
Fig. 12
figure 12

\(S\) against \(\theta \left( \xi \right)\)

Full size image
Fig. 13
figure 13

\({\text{Nt}}\) against \(\phi \left( \xi \right)\)

Full size image
Fig. 14
figure 14

\({\text{Nb}}\) against \(\phi \left( \xi \right)\)

Full size image

Characteristics of velocity

Feature of \(\varepsilon\), \(M,\) \(\lambda\) and \({\text{Nr}}\) via Figs. 2, 3, 4, and 5 is communicated in this subsection. Figure 2 portrays the impact of \(\varepsilon\) on \(f^{\prime } (\xi ).\) Here, \(f^{\prime } (\xi )\) improves for larger \(\varepsilon\) which enhances the thickness of associated boundary layer. Characteristic of \(f^{\prime } (\xi )\) for varying \(M\) is reported in Fig. 3. Increase in \(M\) decays \(f^{\prime } (\xi ).\) Hydromagnetic situation \(\left( {M \ne 0} \right)\) has lower fluid velocity in comparison to hydrodynamic flow \(\left( {M = 0} \right).\) Physically, increment in \(M\) yields greater Lorentz force. In other words, liquid velocity is reduced by greater Lorentz force. Figure 4 depicts the salient features of \(\lambda\) on \(f^{\prime } (\xi )\). Larger \(\lambda\) yields enhancement in liquid velocity. The impact of buoyancy ratio parameter \({\text{Nr}}\) against \(f^{\prime } (\xi )\) is discussed in Fig. 5. Clearly, greater \({\text{Nr}}\) is accountable for diminishment in \(f^{\prime } (\xi ).\)

Characteristics of temperature

Figures 6, 7, 8, 9, 10, 11, 12 report the salient characteristics of several variables on \(\theta (\xi ).\) Feature of \(R\) on \(\theta (\xi )\) is explored in Fig. 6. There is significant influence of thermal radiation on liquid temperature. Here thermal field expands when \(R\) is increased. Physically, it is due to fact that nanoliquids have higher radiative heat flux in the channel. Figure 7 demonstrates the effects of temperature ratio parameter \(\theta_{w} .\) Here, \(\theta (\xi )\) and associated thermal layer thickness improves via larger \(\theta_{w} .\) Feature of thermophoresis parameter \({\text{Nt}}\) on \(\theta (\xi )\) is investigated in Fig. 8. Here, \(\theta (\xi )\) rises for higher estimation of \({\text{Nt}}\). Actually, nano-scale particles pulled away from hottest region to coldest and enormous amount of nanoparticles moved from heated cavity which directly increases the liquid temperature. Attributes of \(M\) on \(\theta (\xi )\) are reported in Fig. 9. Larger estimation of \(M\) has stronger Lorentz force which results in the improvement of temperature of liquid flow. Figure 10 illustrates the features of \(\Pr\) on \(\theta (\xi ).\) Here, \(\theta (\xi )\) is decreasing for larger \(\Pr .\) There is inverse proportionality relation between \(\Pr\) and the thermal diffusivity. Thus, greater \(\Pr\) results decay in temperature field and significantly controls layer thickness. The curve of \({\text{Ec}}\) on \(\theta (\xi )\) is displayed in Fig. 11. Higher estimation of \({\text{Ec}}\) generates more heat which yields to higher temperature and improves related thermal layer thickness \(.\) Larger \({\text{Ec}}\) means the cooling of sheet, i.e., heat transfer from the sheet to fluid. It is due to fact that extreme collision of fluid particles reduces the viscous dissipation and produces more heat. Thus, increments in Eckert number cause increase in temperature of liquid. Figure 12 discloses the feature of heat absorption parameter \(S\) on \(\theta (\xi ).\) Rise in heat generation parameter \(S\) enhances fluid temperature and associated thickness of temperature layer.

Characteristics of concentration

Importance of \({\text{Nt}}\) and \({\text{Nb}}\) on \(\phi (\xi )\) is highlighted in Figs. 13 and 14. Here, we witnessed flipping behavior for concentration. For higher estimation, \({\text{Nt}}\) corresponds to enhancement in \(\phi (\xi )\), while opposite behavior is captured for \({\text{Nb}}\). Here, we noticed movement of nanoparticles from higher temperature to lower temperature which inflates nanoparticles concentration significantly for \({\text{Nt}}\). Furthermore, larger \({\text{Nb}}\) show decline in \(\phi (\xi ).\) Actually, Brownian motion improves the rate where nanoparticles collide in random direction with different velocities. Hence, large \({\text{Nb}}\) yields dwindling behavior in \(\phi (\xi ).\) Fig. 15 is plotted to show the features of \(E\) against \(\phi (\xi ).\) The nanoparticle concentration and associated layer thickness inflate via larger \(E\). Physically, increase in activation energy \(E_{\text{a}}\) and decrease in temperature decays modified Arrhenius function. Here, chemical reaction slows down which causes higher activation energy. Nanoparticle concentration upon Schmidt parameter \({\text{Sc}}\) is illustrated in Fig. 16. An increment in \({\text{Sc}}\) decreases the concentration \(\phi (\xi )\) and allied thickness layer. In fact, flipping behavior is noticed when \({\text{Sc}}\) is augmented. By definition, Schmidt number is inversely proportional to mass diffusivity. Thus, liquid concentration reduces via larger \({\text{Sc}}\) which is due to reduction in mass diffusivity. Figure 17 describes the variation of \(\phi (\xi )\) on chemical reaction parameter \(\sigma\). Increase in \(\sigma\) deaccelerates the nanoparticles volume fraction. Here, concentration gradient has inadequate impact of buoyancy forces, which causes the dwindling behavior of nanoparticle concentration.

Fig. 15
figure 15

\(E\) against \(\phi \left( \xi \right)\)

Full size image
Fig. 16
figure 16

\({\text{Sc}}\) against \(\phi \left( \xi \right)\)

Full size image
Fig. 17
figure 17

\(\sigma\) against \(\phi \left( \xi \right)\)

Full size image

Characteristics of surface drag force and heat transfer rate

This subsection demonstrates the features of \(\delta , \, \varepsilon , { }\lambda , { }M\,{\text{and}}\,{\text{Nr}}\) on surface drag force via Table 1. We observed that surface drag force reduces for larger \(\varepsilon ,\,M,\,{\text{and}}\,{\text{Nr}}\), whereas it improves via larger \(\delta \,{\text{and}}\,\lambda\). Table 2 elaborates the influence of several parameters on heat transfer rate. Clearly, heat transfer rate rises for increments in \(n\), \({\text{Nt}}\) and \(\sigma\), while decays for higher \(R\), \(\Pr\), \(\lambda\) \(E\), and \(\theta_{w}\). A comparison analysis featuring distinct \(M\) values is reported via Table 3. Here, decent agreement is found.

Table 1 Surface drag force \(Cf_{x} Re_{x}^{1/2}\) via different estimation of \(\delta , \, \varepsilon , { }\lambda ,\,M\,{\text{and}}\,{\text{Nr}}\) when \({\text{Ec}} = 0.4\), \(S = R = n = 0.3\), \(\theta_{w} = 0.2,\) \(Nb = Nt = 0.5, \, \Pr = 5.0, \, Sc = 0.7, \, \sigma { = }\delta_{ 1} = 1.0,{\text{ and }}E = 3.0\)
Full size table
Table 2 Heat transfer rate \({\text{Nu}}_{x} Re_{x}^{ - 1/2}\) via different estimation of \(\theta_{w} , \, E, \, \lambda , { }n , {\text{ Nt}}, \, R, \, \sigma {\text{ and }}\Pr\) when \({\text{Ec}} = 0.4, \, S = 0.3,{\text{ Nb}} = 0.5,{\text{ Sc}} = 0.7,{\text{ and }}\delta_{ 1} = 1.0\)
Full size table
Table 3 Comparison results for \(- f^{{{\prime \prime }}} \left( 0 \right)\) for distinct \(M\) when \(\delta = 0 = \varepsilon = \lambda = Nr\)
Full size table

Conclusions

Here, mixed convective Eyring–Powell nanoliquid flow subjected to magnetohydrodynamic (MHD) is examined. Energy distribution is analyzed by non-linear thermal radiation and Joule heating. Furthermore, Arrhenius activation energy and chemical reaction are also considered in concentration expression. The key findings noted from the whole analysis are as follows:

  • Liquid velocity has direct relation with material parameter \(\varepsilon\) and mixed convection parameter \(\lambda\).

  • Increments in buoyancy ratio parameter \({\text{Nr}}\) decrease the liquid velocity.

  • Larger magnetic parameter \(M\) deaccelerates the nanoliquid velocity, whereas temperature of nanoliquid enhances.

  • The features of Prandtl number \(\Pr\) and thermal radiation parameter \(R\) are observed opposite.

  • Higher estimation of Eckert number \({\text{Ec}}\) and heat absorption parameter \(S\) leads to higher temperature and improves heat transfer process.

  • The characteristics of nanoparticles concentration are similar for Brownian motion parameter \(N_{\text{b}}\) and dimensionless reaction rate \(\sigma .\)

  • Thermal layer and nanoparticle concentration enhance via higher magnitude of thermophoretic variable \({\text{Nt}}\).

  • Nanoparticle volume fraction is reduced via larger Schmidt number \(Sc\), while increases when activation energy \(E\) is incremented.

  • Surface drag force is decaying function of magnetic parameter \(M\) and material parameter \(\varepsilon\).

  • Heat transport rate boosts via larger thermophoretic variable \({\text{Nt}}\), whereas it reduces for radiation parameter \(R\) and Prandtl number \(\Pr\).

  • Vicous nanoliquid results can be retrieved when material parameters, i.e., \(\delta = 0 = \varepsilon\).