1 Introduction

The generalized theory of thermoelasticity is one of the modified versions of classical uncoupled and coupled theory of thermoelasticity. In order to remove the paradox of physical impossible phenomena of an infinite velocity of thermal signals in the classical coupled thermoelasticity, the generalized theory of thermoelasticity has been developed. The coupled theory of thermoelasticity is explained in [1]. In [2] generalized thermoelasticity theory involving one thermal relaxation time is studied. Lord–Shulman has been applied, in [3], to study the effect of dependence of the modulus of elasticity on the reference temperature in two-dimensional generalized thermoelasticity. Generalized electro-magneto-thermo-viscoelastic in the case of 2-D thermal shock problem in a finite conducting medium with one relaxation time was studied in [4]. Marin [5] explained an approach of a heat-flux-dependent theory for micropolar porous media. The deformation of a rotating two-temperature generalized magneto-thermoelastic medium with internal heat source due to hydrostatic initial stress was studied by Said [6]. The weak solutions in elasticity of dipolar bodies with stretch were studied by Marin and Stan [7]. In [8], two-temperature generalized magneto-thermoelastic medium, for dual-phase-lag model, under the effect of the gravity field and hydrostatic initial stress, is studied. Wave propagation in a magneto-micropolar thermoelastic medium with two temperatures for three-phase-lag model was studied by Said [9]. The origin of the linear theory of elastic materials with double porosity goes back to papers of Barenblatt et al. [10]. Wilson and Aifantis, in [11], discussed the theory of consolidation with double porosity. Khalili and Valliappan, in [12], used the theory of flow and deformation in double porous media. In [13], Masters et al. studied coupling temperature to a double-porosity model of deformable porous media. Berryman and Wang [14] investigated the elastic wave propagation and attenuation in a double-porosity dual-permeability medium. In [15], Khalili and Selvadurai studied the fully coupled constitutive model for thermo-hydro-mechanical analysis in elastic media with double porosity. Linear dynamics of double-porosity dual-permeability materials-I was discussed by Pride and Berryman [16]. Zhao and Chen [17] introduced the fully coupled dual-porosity model for anisotropic formations. Svanadze [18] studied the dynamical problems of the theory of elasticity for solids with double porosity. In [19], Ainouz investigated the homogenized double-porosity models for poro-elastic media with interfacial flow Barrier. Plane waves and boundary value problems in the theory of elasticity for solids with double porosity were studied by Svanadze [20]. Straughan [21] studied the stability and uniqueness in double-porosity elasticity. The so-called double-porosity model allows the body to have a double porous structure: macroporosity connected to pores in the body and a microporosity connected to fissures in the skeleton. Moreover, the generalized theory, with the help of Darcy’s law, is established to obtain the basic equations for elastic materials with double porosity involve the displacement vector field, a pressure associated with the pores, and a pressure associated with the fissures (see [17, 20, 21]). The materials with double porosity are of interest in geophysics [22, 23] and mechanics of bone [21]. The theory is established with the help of Darcy’s law. The basic equations for elastic materials with double porosity involve the displacement vector field, a pressure associated with the pores, and pressure associated with the issues [20,21,22,23,24,25,26]. Othman and Marin [27] studied the effect of thermal loading due to laser pulse on thermoelastic porous media under G-N theory. The plane waves in magneto-thermoelastic solids with voids and microtemperatures due to hall current and rotation were investigated by Othman et al. [28].

In the present paper, we have studied the equations of generalized thermoelastic material with double-porosity structure with one relaxation time. Effect of porosity and different times is shown graphically.

2 Formulation of the problem and basic equations

Consider a homogeneous thermoelastic half-space with double-porosity structure in the undeformed state at uniform temperature T0. It follows from the description of the problem that all the considered functions will depend upon (x, z, t). We thus obtain the displacement vector u of the form \(\varvec{u} = (u_{1} ,0,u_{3} )\). The field equations and constitutive relations for a homogeneous isotropic thermo-elastic solid with double-porosity structure in the absence of incremental body forces and heat source by L–S model are:

Stress–strain equation (see [29])

$$t_{ij} = \lambda e_{rr} \delta_{ij} + 2\mu e_{ij} + b\delta_{ij} \varPhi + d\delta_{ij} \varPsi - \beta \delta_{ij} (T - T_{0} ).$$
(1)

In case of isotropic solids, the constitutive equations for double porosity [29]

$$\sigma_{i} = \alpha \;\varPhi_{,i} + b_{1} \;\varPsi_{,i} .$$
(2)
$$\tau_{i} = b_{1} \;\varPhi_{,i} + \gamma \;\varPsi_{,i} .$$
(3)

The equation of motion in the absence of body force

$$t_{ij} = \rho \ddot{u}_{,i} .$$
(4)

Using (1) in (4), we get the equation of motion in the two dimensions

$$\mu \nabla^{2} u_{1} + (\lambda + \mu )\frac{\partial e}{\partial x} + b\frac{\partial \varPhi }{\partial x} + d\frac{\partial \varPsi }{\partial x} - \beta \frac{\partial T}{\partial x} = \rho \frac{{\partial^{2} u_{1} }}{{\partial t^{2} }},$$
(5)
$$\mu \nabla^{2} u_{3} + (\lambda + \mu )\frac{\partial e}{\partial z} + b\frac{\partial \varPhi }{\partial z} + d\frac{\partial \varPsi }{\partial z} - \beta \frac{\partial T}{\partial z} = \rho \frac{{\partial^{2} u_{3} }}{{\partial t^{2} }}.$$
(6)

Equilibrated stress equations of motion

$$\alpha \nabla^{2} \varPhi + b_{1} \nabla^{2} \varPsi - be - \alpha_{1} \varPhi - \alpha_{3} \varPsi + \gamma_{1} T = K_{1} \frac{{\partial^{2} \varPhi }}{{\partial t^{2} }},$$
(7)
$$b_{1} \nabla^{2} \varPhi + \gamma \nabla^{2} \varPsi - de - \alpha_{3} \varPhi - \alpha_{2} \varPsi + \gamma_{2} T = K_{2} \frac{{\partial^{2} \varPsi }}{{\partial t^{2} }}.$$
(8)

Equation of heat

$$K\nabla^{2} T = \left( {1 + \tau_{0} \frac{\partial }{\partial t}} \right)\left( {\rho C^{*} \frac{\partial T}{\partial t} + \beta T_{0} \frac{\partial e}{\partial t} + \gamma_{1} T_{0} \frac{\partial \varPhi }{\partial t} + \gamma_{2} T_{0} \frac{\partial \varPsi }{\partial t}} \right).$$
(9)

For the purpose of numerical evaluation, we introduce dimensionless variables

$$\begin{aligned} (x^{\prime } ,z^{\prime } ) = \frac{{\omega_{1} }}{{c_{1} }}(x,z),\quad (u_{1}^{\prime } ,u_{3}^{\prime } ) = \frac{{\omega_{1} }}{{c_{1} }}(u_{1} ,u_{3} ),\quad \{ \sigma_{1}^{\prime } ,\tau_{1}^{\prime } \} = \frac{{c_{1} }}{{\alpha \omega_{1} }}\{ \sigma_{1} ,\tau_{1} \} ,\quad (t^{\prime } ,\tau_{o}^{\prime } ) = \omega_{1} (t,\tau_{0} ), \hfill \\ [\varPhi^{\prime } ,\varPsi^{\prime } ] = \frac{{K_{1} \omega_{1}^{2} }}{{\alpha_{1} }}[\varPhi ,\varPsi ],\quad c_{1}^{2} = \frac{\lambda + 2\mu }{\rho },\quad \omega_{1} = \frac{{\rho c^{*} c_{1}^{2} }}{K},\quad \nabla^{2} = \frac{{\omega_{1}^{2} }}{{c_{1}^{2} }}\nabla^{\prime 2} ,\quad \gamma = (3\lambda + 2\mu )\alpha_{t} , \hfill \\ t_{ij}^{\prime } = \left( {\frac{1}{{\beta T_{0} }}} \right)t_{ij} ,\quad T^{\prime } = \frac{T}{{T_{0} }}. \hfill \\ \end{aligned}$$

Using the above dimensionless quantities, Eqs. (5)–(9) become:

$$\left( {\frac{\lambda + \mu }{{\rho c_{1}^{2} }}} \right)\frac{\partial e}{\partial x} + \left( {\frac{\mu }{{\rho c_{1}^{2} }}} \right)\nabla^{2} u_{1} + a_{1} \frac{\partial \varPhi }{\partial x} + a_{2} \frac{\partial \varPsi }{\partial x} - a_{3} \frac{\partial T}{\partial x} = \frac{{\partial^{2} u_{1} }}{{\partial t^{2} }},$$
(10)
$$\left( {\frac{\lambda + \mu }{{\rho c_{1}^{2} }}} \right)\frac{\partial e}{\partial z} + \left( {\frac{\mu }{{\rho c_{1}^{2} }}} \right)\nabla^{2} u_{3} + a_{1} \frac{\partial \varPhi }{\partial z} + a_{2} \frac{\partial \varPsi }{\partial z} - a_{3} \frac{\partial T}{\partial z} = \frac{{\partial^{2} u_{3} }}{{\partial t^{2} }},$$
(11)
$$a_{4} \nabla^{2} \varPhi + a_{5} \nabla^{2} \varPsi - a_{6} e - a_{7} \varPhi - a_{8} \varPsi + a_{9} T = \frac{{\partial^{2} \varPhi }}{{\partial t^{2} }},$$
(12)
$$a_{10} \nabla^{2} \varPhi + a_{11} \nabla^{2} \varPsi - a_{12} e - a_{13} \varPhi - a_{14} \varPsi + a_{15} T = \frac{{\partial^{2} \varPsi }}{{\partial t^{2} }},$$
(13)
$$a_{16} \nabla^{2} T = \left( {1 + \tau_{0} \frac{\partial }{\partial t}} \right)\left( {\frac{\partial T}{\partial t} + a_{17} \frac{\partial e}{\partial t} + a_{18} \frac{\partial \varPhi }{\partial t} + a_{19} \frac{\partial \varPsi }{\partial t}} \right).$$
(14)

where

$$\begin{aligned} a_{1} & = \frac{{b\alpha_{1} }}{{\rho c_{1}^{2} K_{1} \omega_{1}^{2} }},\quad a_{2} = \frac{{d\alpha_{1} }}{{\rho c_{1}^{2} K_{1} \omega_{1}^{2} }},\quad a_{3} = \frac{{\beta T_{0} }}{{\rho c_{1}^{2} }},\quad a_{4} = \frac{\alpha }{{K_{1} c_{1}^{2} }},\quad a_{5} = \frac{{b_{1} }}{{K_{1} c_{1}^{2} }},\quad a_{6} = \frac{b}{{\alpha_{1} }}, \\ a_{7} & = \frac{{\alpha_{1} }}{{K_{1} \omega_{1}^{2} }},\quad a_{8} = \frac{{\alpha_{3} }}{{K_{1} \omega_{1}^{2} }},\quad a_{9} = \frac{{\gamma_{1} T_{0} }}{{\alpha_{1} }},\quad a_{10} = \frac{{b_{1} }}{{c_{1}^{2} K_{2} }},\quad a_{11} = \frac{\gamma }{{c_{1}^{2} K_{2} }},\quad a_{12} = \frac{{dK_{1} }}{{\alpha_{1} K_{2} }}, \\ a_{13} & = \frac{{\alpha_{3} }}{{\omega_{1}^{2} K_{2} }},\quad a_{14} = \frac{{\alpha_{2} }}{{\omega_{1}^{2} K_{2} }},\quad a_{15} = \frac{{\gamma_{2} T_{0} K_{1} }}{{\alpha_{1} K_{2} }},\quad a_{16} = \frac{{K\omega_{1} }}{{\rho c^{*} c_{1}^{2} }},\quad a_{17} = \frac{\beta }{{\rho c^{*} }},\quad a_{18} = \frac{{\gamma_{1} \alpha_{1} }}{{\rho c^{*} K_{1} \omega_{1}^{2} }}, \\ a_{19} & = \frac{{\gamma_{2} \alpha_{1} }}{{\rho c^{*} K_{1} \omega_{1}^{2} }}. \\ \end{aligned}$$

Define displacement potentials ϕ1 and ψ1 that relate to displacement components u1 and u3 as,

$$u_{1} = \frac{{\partial \phi_{1} }}{\partial x} - \frac{{\partial \psi_{1} }}{\partial z},\quad u_{3} = \frac{{\partial \phi_{1} }}{\partial z} + \frac{{\partial \psi_{1} }}{\partial x}.$$
(15)

Using Eq. (15) in Eqs. (10)–(14), to obtain:

$$\nabla^{2} \phi_{1} + a_{1} \varPhi + a_{2} \varPsi - a_{3} T = \frac{{\partial^{2} \phi_{1} }}{{\partial t^{2} }},$$
(16)
$$\left( {\frac{\mu }{{\rho c_{1}^{2} }}} \right)\nabla^{2} \psi_{1} = \frac{{\partial^{2} \psi_{1} }}{{\partial t^{2} }},$$
(17)
$$a_{4} \nabla^{2} \varPhi + a_{5} \nabla^{2} \varPsi - a_{6} \nabla^{2} \phi_{1} - a_{7} \varPhi - a_{8} \varPsi + a_{9} T = \frac{{\partial^{2} \varPhi }}{{\partial t^{2} }},$$
(18)
$$a_{10} \nabla^{2} \varPhi + a_{11} \nabla^{2} \varPsi - a_{12} \nabla^{2} \phi_{1} - a_{13} \varPhi - a_{14} \varPsi + a_{15} T = \frac{{\partial^{2} \varPsi }}{{\partial t^{2} }},$$
(19)
$$a_{16} \nabla^{2} T = \left( {1 + \tau_{0} \frac{\partial }{\partial t}} \right)\left( {\frac{\partial T}{\partial t} + a_{17} \nabla^{2} \frac{{\partial \phi_{1} }}{\partial t} + a_{18} \frac{\partial \varPhi }{\partial t} + a_{19} \frac{\partial \varPsi }{\partial t}} \right).$$
(20)

Dimensionless variables of the stress components take the form,

$$\tau_{xx} = \left( {\frac{\lambda }{{\beta T_{0} }}} \right)e + \left( {\frac{2\mu }{{\beta T_{0} }}} \right)\frac{{\partial u_{1} }}{\partial x} - T + \left( {\frac{{b\alpha_{1} }}{{K_{1} \omega_{1}^{2} \beta T_{0} }}} \right)\varPhi + \left( {\frac{{d\alpha_{1} }}{{K_{1} \omega_{1}^{2} \beta T_{0} }}} \right)\varPsi ,$$
(21)
$$\tau_{zz} = \left( {\frac{\lambda }{{\beta T_{0} }}} \right)e + \left( {\frac{2\mu }{{\beta T_{0} }}} \right)\frac{{\partial u_{3} }}{\partial z} - T + \left( {\frac{{b\alpha_{1} }}{{K_{1} \omega_{1}^{2} \beta T_{0} }}} \right)\varPhi + \left( {\frac{{d\alpha_{1} }}{{K_{1} \omega_{1}^{2} \beta T_{0} }}} \right)\varPsi ,$$
(22)
$$\tau_{xz} = \left( {\frac{2\mu }{{\beta T_{0} }}} \right)e_{xz} .$$
(23)

3 Normal mode analysis

The solution of the considered physical variables can be decomposed in terms of normal mode as the following form

$$[u_{1} ,u_{3} ,e,T,\phi_{1} ,\psi_{1} ,\varPhi ,\psi ,t_{ij} ](x,z,t) = [u_{1}^{*} ,u_{3}^{*} ,e^{*} ,T^{*} ,\phi_{1}^{*} ,\psi_{1}^{*} ,\varPhi^{*} ,\psi^{*} ,t_{ij}^{*} ](z)\exp \;[i(\omega t + ax)].$$
(24)

where ω is the complex time constant (frequency), i is the imaginary unit, and a is the wave number in the x-direction.

Using (24) in Eqs. (16)–(20), we obtain

$$(D^{2} - n_{1} )\phi_{1}^{*} + a_{1} \varPhi^{*} + a_{2} \varPsi^{*} - a_{3} T^{*} = 0,$$
(25)
$$(D^{2} - m^{2} )\psi_{1}^{*} = 0,$$
(26)
$$(a_{4} D^{2} - n_{2} )\varPhi^{*} + (a_{5} D^{2} - n_{3} )\varPsi^{*} - (a_{6} D^{2} - n_{4} )\phi_{1}^{*} + a_{9} T^{*} = 0,$$
(27)
$$(a_{10} D^{2} - n_{5} )\varPhi^{*} + (a_{11} D^{2} - n_{6} )\varPsi^{*} - (a_{12} D^{2} - n_{7} )\phi_{1}^{*} + a_{15} T^{*} = 0,$$
(28)
$$(a_{16} D^{2} - n_{8} )T^{*} + n_{9} (D^{2} - a^{2} )\phi_{1}^{*} + n_{10} \varPhi^{*} + n_{11} \varPsi^{*} = 0.$$
(29)

where

$$\begin{aligned} D & = \frac{\partial }{\partial z},\quad m^{2} = a^{2} + \frac{{\omega^{2} \rho c_{1}^{2} }}{\mu },\quad n_{1} = a^{2} - \omega^{2} ,\quad n_{2} = a_{4} a^{2} + a_{7} - \omega^{2} ,\quad n_{3} = a_{5} a^{2} + a_{8} , \\ n_{4} & = a_{6} a^{2} ,\quad n_{5} = a_{10} a^{2} + a_{13} ,\quad n_{6} = a_{11} a^{2} + a_{14} - \omega^{2} ,\quad n_{7} = a_{12} a^{2} ,\quad n_{8} = a_{16} a^{2} + i\omega (1 + i\tau_{0} \omega ), \\ n_{9} & = - i\omega a_{17} (1 + i\tau_{0} \omega ),\quad n_{10} = - i\omega a_{18} (1 + i\tau_{0} \omega ),\quad n_{11} = - i\omega a_{19} (1 + i\tau_{0} \omega ). \\ \end{aligned}$$

Put the above Eqs. (25), (27), (28), (29) in the matrix, we find that the differential equation takes the form:

$$\text{[D}^{\text{8}} - \text{AD}^{\text{6}} \text{ + B}\,\text{D}^{\text{4}} - \text{C}\,\text{D}^{\text{2}} { + E]\{ }\phi_{\text{1}}^{\text{*}} {(z),\varPhi }^{\text{*}} {(z),\psi }^{\text{*}} {(z),\psi }_{\text{1}}^{\text{*}} \text{(z),T}^{\text{*}} {\} = 0}\text{.}$$
(30)

where

$$\begin{aligned} A & = \frac{{a_{11} (a_{4} n_{8} + a_{4} a_{16} n_{6} + a_{16} n_{2} - a_{1} a_{6} a_{16} + a_{4} a_{16} n_{1} - a_{4} a_{3} n_{9} ) + a_{16} (a_{10} a_{2} a_{6} - a_{10} a_{5} n_{1} - a_{10} n_{3} - a_{5} n_{5} - a_{4} a_{2} a_{12} ) + a_{5} (a_{1} a_{12} n_{16} + a_{10} a_{3} n_{9} )}}{{(a_{4} a_{11} a_{16} - a_{10} a_{5} a_{16} )}}, \\ B & = \frac{\begin{aligned} n_{8} (a_{4} n_{6} + a_{11} n_{2} - a_{10} n_{3} + n_{5} a_{5} + a_{1} a_{5} a_{12} - a_{1} a_{11} a_{6} ) + a_{16} (n_{5} n_{3} + n_{2} n_{6} + a_{1} a_{12} n_{3} + a_{1} a_{5} n_{7} - a_{1} a_{6} n_{6} - a_{1} a_{11} n_{4} ) \hfill \\ + n_{11} ( - a_{4} a_{15} + a_{10} a_{9} ) + n_{10} ( - a_{15} a_{5} + a_{11} a_{9} ) - a_{1} (a_{5} a_{15} n_{9} + a_{11} a_{9} n_{9} ) - a_{4} (a_{2} a_{12} n_{8} + a_{2} a_{16} n_{7} - a_{2} a_{15} n_{9} - a_{11} n_{1} n_{8} \hfill \\ - a_{3} a_{11} n_{9} n_{8} - a_{16} n_{1} n_{6} + a_{3} n_{6} n_{9} - a_{3} n_{11} a_{12} ) - n_{2} (a_{2} a_{16} a_{12} - a_{16} a_{11} n_{1} + a_{11} a_{3} n_{9} ) + n_{10} (a_{5} a_{3} a_{12} - a_{11} a_{3} a_{6} ) \hfill \\ + a_{10} (a_{2} a_{6} n_{8} + a_{2} a_{16} n_{4} - a_{2} a_{9} n_{9} - a_{5} n_{1} n_{8} + a_{5} a_{3} n_{9} n_{8} - a_{16} n_{1} n_{3} + a_{3} n_{9} n_{3} - a_{6} a_{3} n_{11} ) + n_{5} (a_{2} a_{6} a_{16} - a_{5} a_{16} n_{1} - a_{5} a_{3} n_{9} ) \hfill \\ \end{aligned} }{{a_{16} (a_{4} a_{11} - a_{10} a_{5} )}}, \\ C & = \frac{\begin{aligned} n_{2} (n_{6} n_{8} - n_{11} a_{15} ) + n_{10} (n_{3} n_{15} - a_{9} ( - a_{11} n_{1} + a_{9} + a_{2} a_{12} ) - a_{2} n_{2} (a_{12} n_{8} - a_{16} n_{7} - a_{15} n_{9} ) + a_{15} (a_{6} a_{2} + a_{5} n_{1} ) \hfill \\ - a_{3} (a_{5} n_{7} + a_{12} n_{3} + a_{11} n_{4} + a_{6} n_{6} )) + a_{1} n_{8} (a_{5} n_{7} + a_{12} n_{3} - a_{11} n_{4} - a_{6} n_{6} - a_{5} a_{15} n_{9} + a_{11} a_{9} n_{9} ) \hfill \\ + a_{1} (a_{16} n_{7} n_{3} - a_{15} n_{9} n_{3} - a_{16} n_{4} n_{6} + a_{9} n_{6} n_{9} + a_{6} n_{11} a_{6} - a_{9} n_{11} a_{12} ) - a_{4} n_{8} (a_{2} n_{7} + a_{2} a_{15} n_{9} + n_{6} \,n_{1} - a_{3} n_{6} n_{9} - a_{15} n_{11} n_{1} ) \hfill \\ + n_{8} ( + a_{3} n_{7} n_{11} + a_{11} n_{2} n_{1} - a_{3} a_{11} n_{2} n_{9} + a_{10} a_{2} n_{4} - a_{10} a_{2} a_{9} n_{9} - a_{5} n_{1} n_{5} - a_{3} a_{5} n_{5} n_{9} - a_{10} n_{3} n_{1} + a_{10} a_{3} n_{3} n_{9} + a_{2} a_{6} n_{5} ) \hfill \\ + n_{2} n_{6} (a_{16} n_{1} - 2a_{3} n_{9} ) + n_{11} (a_{3} a_{12} n_{2} + a_{10} (a_{9} n_{1} - a_{3} n_{4} )) + n_{5} (a_{16} a_{2} n_{4} - a_{2} a_{9} n_{9} - a_{16} n_{3} n_{1} - a_{3} n_{3} n_{9} + a_{3} a_{6} n_{11} \; + n_{3} n_{8} - n_{11} n_{9} ) \hfill \\ \end{aligned} }{{a_{16} (a_{4} a_{11} - a_{10} a_{5} )}}, \\ E & = \frac{\begin{aligned} - a_{1} ( - a_{9} n_{11} n_{7} - a_{15} n_{11} n_{4} - n_{8} (n_{3} n_{7} + a_{15} n_{9} n_{3} + n_{6} n_{4} \hfill \\ - a_{9} n_{9} n_{6} )) - n_{2} (n_{11} (a_{15} n_{1} + a_{3} n_{7} ) + n_{9} n_{8} (a_{2} a_{15} - a_{3} n_{6} )) \hfill \\ + n_{8} n_{5} (a_{2} n_{4} - n_{3} n_{1} - a_{2} a_{9} n_{9} + a_{3} n_{3} n_{9} ) + n_{11} n_{5} (a_{9} n_{1} -_{3} n_{4} ) \hfill \\ - n_{10} (a_{2} (a_{15} n_{4} + a_{9} n_{7} ) - n_{3} (a_{15} n_{1} + a_{3} n_{7} ) + n_{6} (a_{9} n_{1} - a_{3} n_{4} )) \hfill \\ \end{aligned} }{{a_{16} (a_{4} a_{11} - a_{10} a_{5} )}}. \\ \end{aligned}$$

The solution of Eq. (30) has the form

$$\varPhi^{*} = \sum\limits_{n = 1}^{4} {M_{n} } e^{{ - k_{n} z}} ,$$
(31)
$$\varPsi^{*} = \sum\limits_{n = 1}^{4} {H_{1n} M_{n} } e^{{ - {\kern 1pt} k_{n} z}} ,$$
(32)
$$\phi_{1}^{*} = \sum\limits_{n = 1}^{4} {H_{2n} M_{n} } e^{{ - k_{n} z}} ,$$
(33)
$$T^{*} = \sum\limits_{n = 1}^{4} {H_{3n} M_{n} } e^{{ - k_{n} z}} ,$$
(34)
$$\psi_{1}^{*} = M_{5} e^{ - mz} .$$
(35)

After substituting Eqs. (33)–(35) into (15), the displacements take the form:

$$u_{1} = \sum\limits_{i = 1}^{4} {iaH_{2n} M_{n} e^{{ - k_{n} z}} e^{i(\omega t + ax)} } + mM_{5} e^{ - mz} e^{i(\omega t + ax)} ,$$
(36)
$$u_{3} = \sum\limits_{i = 1}^{4} { - k_{n} H_{2n} M_{n} e^{{ - k_{n} z}} e^{i(\omega t + ax)} } + iaM_{5} e^{ - mz} e^{i(\omega t + ax)} .$$
(37)

In addition, substituting from Eqs. (34) and (36–37) into (21)–(23), the stress displacements become

$$\tau_{xx} = \sum\limits_{i = 1}^{4} {H_{4n} M_{n} e^{{ - k_{n} z}} e^{i(\omega t + ax)} } + H_{5} M_{5} e^{ - mz} e^{i(\omega t + ax)} ,$$
(38)
$$\tau_{zz} = \sum\limits_{i = 1}^{4} {H_{5n} M_{n} e^{{ - k_{n} z}} e^{i(\omega t + ax)} } - H_{5} M_{5} e^{ - mz} e^{i(\omega t + ax)} ,$$
(39)
$$\tau_{xz} = \sum\limits_{i = 1}^{4} {H_{6n} M_{n} e^{{ - k_{n} z}} e^{i(\omega t + ax)} - H_{7} M_{5} e^{ - mz} e^{i(\omega t + ax)} } .$$
(40)

Dimensionless variables for the components of σi, τi

$$\sigma_{3} = \eta_{1} \varPhi_{,z} + \eta_{2} \varPsi_{,z} ,$$
(41)
$$\tau_{3} = \eta_{3} \varPhi_{,z} + \eta_{4} \varPsi_{,z} .$$
(42)

where \(\eta_{1} = \frac{{\alpha_{1} }}{{k_{1} \omega_{1}^{2} }},\eta_{2} = \eta_{3} = \frac{{b_{1} \alpha_{1} }}{{\alpha k_{1} \omega_{1}^{2} }},\eta_{4} = \frac{{\gamma \alpha_{1} }}{{\alpha k_{1} \omega_{1}^{2} }}.\)

Moreover, substituting from Eqs. (31), (32) into (41) and (42), we get the solution of σ3 and τ3, as:

$$\sigma_{3} = \sum\limits_{n = 1}^{4} {H_{9n} M_{n} e^{{ - k_{n} z}} e^{i(\omega t + ax)} } ,$$
(43)
$$\tau_{3} = \sum\limits_{n = 1}^{4} {H_{8n} M_{n} e^{{ - k_{n} z}} e^{i(\omega t + ax)} } .$$
(44)

where

$$\begin{aligned} H_{1n} & = \frac{\begin{aligned} [a_{1} a_{9} + a_{3} (a_{4} k_{n}^{2} - n_{2} )][a_{15} n_{9} (k_{n}^{2} - a^{2} ) - (a_{12} k_{n}^{2} - n_{7} )(a_{16} k_{n}^{2} - n_{8} )] \hfill \\ - [a_{9} (k_{n}^{2} - n_{1} ) - a_{3} (a_{6} k_{n}^{2} - n_{4} )][n_{10} a_{15} + (a_{10} k_{n}^{2} - n_{5} )(a_{16} k_{n}^{2} - n_{8} )] \hfill \\ \end{aligned} }{\begin{aligned} [a_{9} (k_{n}^{2} - n_{1} ) - a_{3} (a_{6} k_{n}^{2} - n_{4} )][(a_{11} k_{n}^{2} - n_{6} )(a_{16} k_{n}^{2} - n_{8} ) + n_{11} a_{15} ] \hfill \\ - [a_{2} a_{9} + a_{3} (a_{5} k_{n}^{2} - n_{3} )][a_{15} n_{9} (k_{n}^{2} - a^{2} ) - (a_{12} k_{n}^{2} - n_{7} )(a_{16} k_{n}^{2} - n_{8} )] \hfill \\ \end{aligned} }, \\ H_{2n} & = \frac{{[n_{10} a_{15} + (a_{10} k_{n}^{2} - n_{5} )(a_{16} k_{n}^{2} - n_{8} )] + H_{1n} [(a_{11} k_{n}^{2} - n_{6} )(a_{16} k_{n}^{2} - n_{8} ) + n_{11} a_{15} ]}}{{[ - a_{15} n_{9} (k_{n}^{2} - a^{2} ) + (a_{12} k_{n}^{2} - n_{7} )(a_{16} k_{n}^{2} - n_{8} )]}}, \\ H_{3n} & = \frac{{[H_{2n} a_{9} (k_{n}^{2} - a^{2} ) + n_{10} + H_{1n} n_{11} ]}}{{(a_{16} k_{n}^{2} - n_{8} )}}. \\ H_{4n} & = \left( {\frac{\lambda }{{\beta T_{0} }}(k_{n}^{2} - a^{2} ) - \frac{{2\mu a^{2} }}{{\beta T_{0} }}} \right)H_{2n} - H_{3n} + \left( {\frac{{b\alpha_{1} }}{{k_{1} \omega_{1}^{2} \beta T_{0} }}} \right) + \left( {\frac{{d\alpha_{1} }}{{k_{1} \omega_{1}^{2} \beta T_{0} }}} \right)H_{1n} ,\quad H_{5} = \left( {\frac{2iam\mu }{{\beta T_{0} }}} \right) \\ H_{6n} & = \frac{{ - 2iak_{n} \mu }}{{\beta T_{0} }}H_{2n} ,\quad H_{7} = m^{2} + a^{2} ,\quad H_{8n} = - \eta_{3} k_{n} - \eta_{4} k_{n} H_{1n} ,\quad H_{9n} = - \eta_{1} k_{n} - \eta_{2} k_{n} H_{1n} . \\ \end{aligned}$$

4 Boundary conditions

We apply five boundary conditions for present problem at the plane surface z = 0.

$$\tau_{zz} = P_{1} e^{i(\omega t + ax)} ,$$
(45)
$$\tau_{xz} = 0,$$
(46)
$$\tau_{3} = 0,$$
(47)
$$\sigma_{3} = 0,$$
(48)
$$T = P_{2} e^{i(\omega t + ax)} .$$
(49)

Applying Eqs. (45–49) in (39), (40), (43), (44) and (34), we get

$$\sum\limits_{n = 1}^{4} {H_{5n} M_{n} - H_{5} M_{5} } = P_{1} ,$$
(50)
$$\sum\limits_{n = 1}^{4} {H_{6n} M_{n} } - H_{7} M_{5} = 0,$$
(51)
$$\sum\limits_{n = 1}^{4} {H_{8n} M_{n} } = 0,$$
(52)
$$\sum\limits_{n = 1}^{4} {H_{9n} M_{n} } = 0,$$
(53)
$$\sum\limits_{n = 1}^{4} {H_{3n} M_{n} } = P_{2} .$$
(54)

We can put Eqs. (50)–(54) in matrix and using MATLAB program to get \(M_{1} ,M_{2} , \ldots ..,M_{5}\),

$$\left( {\begin{array}{*{20}c} {M_{1} } \\ {M_{2} } \\ {M_{3} } \\ {M_{4} } \\ {M_{5} } \\ \end{array} } \right) = \left( {\begin{array}{*{20}c} {H_{51} } & {H_{52} } & {H_{53} } & {H_{54} } & { - H_{5} } \\ {H_{61} } & {H_{62} } & {H_{63} } & {H_{64} } & { - H_{7} } \\ {H_{81} } & {H_{82} } & {H_{83} } & {H_{84} } & 0 \\ {H_{91} } & {H_{92} } & {H_{93} } & {H_{94} } & 0 \\ {H_{31} } & {H_{32} } & {H_{33} } & {H_{34} } & 0 \\ \end{array} } \right)^{ - 1} \left( {\begin{array}{*{20}c} {P_{1} } \\ 0 \\ 0 \\ 0 \\ {P_{2} } \\ \end{array} } \right).$$
(55)

5 Special cases

Case (i) when we neglect the presence of double porosity, the problem turns into a generalized thermoelastic medium.

Case (ii) If τ0 = 0 in Eq. (9),the corresponding expressions for thermoelastic medium with double porosity in the context of the coupled theory of thermoelasticity were yielded.

6 Results and discussion

To discuss, numerically, the effect of double porosity, the copper is considered as the thermoelastic material for which we take the following values of the different physical constants as Othman et al. [30].

$$\begin{aligned} \lambda & = 7.7 \times 10^{10} \,{\text{N\,M}}^{ - 2} ,\quad \mu = 3.86 \times 10^{10} \,{\text{N\,m}}^{ - 2} ,\quad K = 3.86 \times 10^{3} \,{\text{N\,s}}^{ - 1} \,{\text{K}}^{ - 1} ,\quad \omega = 0.01, \\ \alpha_{t} & = 1.78 \times 10^{{{ - 5}}} \,\text{K}^{ - 1} ,\quad \rho = 8954\,\text{kg\,m}^{ - 3} ,\quad C^{*} = 383.1\;\text{J}\,\text{kg}^{ - 1} \,\text{K}^{ - 1} ,\quad T_{{0}} = 293\,\text{K,}\quad a = 1,\quad \tau_{0} = 0.7, \\ x & = 0.5,\quad \xi = - 1,\quad p_{1} = 1 \times 10^{ - 2} ,\quad p_{2} = 10 \times 10^{ - 2} . \\ \end{aligned}$$

Following Khalili [31], the double porous parameters are taken as

$$\begin{aligned} \alpha & = 1.3 \times 10^{ - 5} \,{\text{N}},\quad b_{1} = 0.12 \times 10^{ - 5} \,{\text{N}},\quad \gamma = 1.1 \times 10^{ - 5} \,{\text{N\,m}}^{ - 2} ,\quad \gamma_{1} = 0.16 \times 10^{5} \,{\text{N\,m}}^{ - 2} , \\ \gamma_{2} & = 0.219 \times 10^{5} \,{\text{N\,m}}^{ - 2} ,\quad d = 0.1 \times 10^{10} \,{\text{N\,m}}^{ - 2} ,\quad b = 0.9 \times 10^{10} \,{\text{N\,m}}^{ - 2} ,\quad K_{2} = 0.1546 \times 10^{ - 12} \,{\text{N\,m}}^{ - 2} , \\ K_{1} & = 0.1456 \times 10^{ - 12} \,{\text{N\,m}}^{ - 2} . \\ \end{aligned}$$

The numerical technique, outlined above, was used for the distribution of the real part of the temperature T, the displacement components u1, u3 the stress components τxx, τxz, τzz the components of double porosity σ and τ for the problem. All the variables are taken in non-dimensional form from the result.

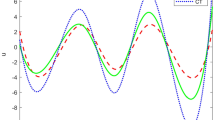
Figures 1 and 2 explain the comparison of the stress component τxx in the presence and absence of double porosity at two different times. We find that in Figs. 1 and 2 the stress τxx increases a small shift in the presence of double porosity and then decreases at two different values of time in the presence and absence of double porosity and take the form of the wave and try to return to zero. Figures 3 and 4 show the comparison of the stress component τzz in the presence and absence of double porosity at two different times. We find that in Fig. 3 the stress τzz increases to a maximum value at t = 1 and then decreases to a minimum value at t = 2 in the presence of double porosity and take the form of a wave and try to return to zero. Figure 4 explains that the stress τzz decreases to a minimum value in the absence of double porosity at two different times and then decays until it returns to zero. Figures 5 and 6 demonstrate the comparison of the stress component τxz in the presence and absence of double porosity at two different times. We find that in Fig. 5 the stress τxz decreases and then increases to a maximum value at t = 2 in the presence of double porosity and take the form of the wave and try to return to zero. Figure 6 shows that the stress τxz increases to a maximum value at z = 0.5 in the absence of double porosity at two different times and then decreases until it returns to zero. Figures 7 and 8 explain the comparison of the temperature T in the presence and absence of double porosity at two different times. We find that in Figs. 7 and 8 the temperature T decreases in the two cases (with and without double porosity) at two different times and then decays to zero. Figures 9 and 10 show the comparison of the displacement u1 in the presence and absence of double porosity at two different times. We find that in Fig. 9 the displacement u1 decreases at t = 0.5 more than at t = 1.5 and then decreases until it decays to zero in the positive direction of z, but in Fig. 10 the displacement u1 decreases to minimum value at t = 1.5 more than at t = 0.5 and takes the form of the wave until it decays to zero. Figures 11 and 12 illustrate the comparison of the displacement u3 in the presence and absence of double porosity at two different times. We find that in Fig. 11 the displacement u3 increases a small shift in the begging at t = 0.5 more than at t = 1.5 and then begins to decrease until it decays to zero, but in Fig. 12 the displacement u3 increases at t = 0.5 more than at t = 1.5 and then begins to decrease until it decays to zero. Figures 13 and 14 demonstrate the comparison of the equilibrated stresses σ and τ in the presence of double porosity at two different times. We find that in Figs. 13 and 14 the equilibrated stresses σ and τ increase with the increase in time to a minimum value at 1 and then begin to decrease and take the form of the wave and try to return to zero.

Fig. 1
figure 1

Distribution of the stress component τxx

Full size image
Fig. 2
figure 2

Distribution of the stress component τxx

Full size image
Fig. 3
figure 3

Distribution of the stress component τzz

Full size image
Fig. 4
figure 4

Distribution of the stress component τzz

Full size image
Fig. 5
figure 5

Distribution of the stress component τxz

Full size image
Fig. 6
figure 6

Distribution of the stress component τxz

Full size image
Fig. 7
figure 7

Distribution of the temperature T

Full size image
Fig. 8
figure 8

Distribution of the temperature T

Full size image
Fig. 9
figure 9

Distribution of the displacement u1

Full size image
Fig. 10
figure 10

Distribution of the displacement u1

Full size image
Fig. 11
figure 11

Distribution of the displacement u3

Full size image
Fig. 12
figure 12

Distribution of the displacement u3

Full size image
Fig. 13
figure 13

Distribution of the equilibrated stress τ

Full size image
Fig. 14
figure 14

Distribution of the equilibrated stress σ

Full size image

7 Conclusion

From the figures obtained by comparing the functions in the presence and absence of double porosity at two different times, important phenomena are observed:

Analytic solutions based upon normal mode analysis of the thermoelastic problem in solids have been developed, which used in the present article is applicable to a wide range of problems in hydrodynamics and thermoelasticity. There are significant differences in the presence and absence of double porosity under two different times.

All the physical quantities satisfy the boundary conditions. The value of all the physical quantities converges to zero, and all the functions are continuous. Though the problem is theoretical, it can provide useful information for experimental researchers working in the field of geophysics, earthquake engineering, along with seismologist working in the field of mining tremors and drilling into the crust of the earth. The numerical treatment of the general system of equations and conditions governing the phenomenon may be useful in getting rid of the limitations of the method of normal modes’ technique, and this task is in progress.