Campaigns, political mobility, and communication

Abstract

We study the interdependence between campaign contributions, the candidates’ positions, and electoral outcomes. In our model, a candidate who moves away from his firmly established position towards a more risky one generates costs for the voters. Campaign contributions allow the candidates to reduce these mobility costs. We show that if donations were prohibited, then a unique equilibrium regarding the position choices of candidates would exist. With unrestricted financing of political campaigns, two equilibria emerge, depending on whether a majority of interest groups runs to support the leftist or rightist candidate. Interest groups may finance candidates whose position is far away from their own ideal point. The equilibria generate a variety of new features of campaign games, and may help identify the objective functions of candidates empirically.

1 Introduction

Competitive political campaigns are still a very controversial issue. Discussions on this subject relate both to the influence of campaigns on political outcomes and to their impact on welfare.

In this paper we propose a model of political campaigns that allows to study the interdependence between campaign expenditures, candidates’ positions, and electoral outcomes. We focus on the following, often-observed political race. At the beginning of a political race for office, two candidates try to obtain campaign support from interest groups. They announce positions in a policy space that are more risky the more they differ from positions announced in the past. One candidate has a firmly established position on the left of the political spectrum and the same holds for the other candidate on the right side. Voters are risk-averse and the candidates will try to improve communication with them during campaigns in order to reduce location uncertainty. This, in turn, allows them to move their political positions towards the current median position. Fund-raising is a necessary condition for getting messages across, so candidates will attempt to obtain campaign contributions at the beginning of the political race to gain mobility within the political spectrum. Candidates maximize their vote shares.

We study the equilibria of this game and shed light on the role of political campaigns. The main—and novel—insight of our analysis is that with unrestricted financing of political campaigns, multiple equilibria and a run of interest groups emerge. Depending on the belief which candidate will win, a majority of interest groups runs to support the leftist or rightist candidate. As a consequence, even if the candidates’ initial positions and the ideal points of the interest groups are symmetrically distributed around the median, the political positions chosen in equilibrium will be asymmetric, and two asymmetric constellations will emerge.

Moreover, we demonstrate that donors may support a candidate whose position is not very close to their own ideal point in order to draw the position of the winning candidate towards their own ideal point. Suppose, for example, that the rightist candidate wins the election. Then, donors to the right of the winning rightist candidate give money to the leftist candidate, as this pushes the equilibrium position of the rightist candidate towards the right. Donors located around the median, however, will support the winning candidate. This counterintuitive result—in comparison with other theoretical results—could be used to draw inferences about whether candidates for public offices are more interested in policies or in winning elections. This will be developed in the next section.

Further, the candidates do not adopt the median position in the equilibria. However, campaigns lead to a partial convergence of positions towards the preferred position of the median voter, in comparison with the equilibrium without campaigns. Campaigns thus induce the winning position to move closer to the median ideal position.

Finally, our analysis may also enrich the incumbent/challenger discussion. A traditional argument suggests that incumbents are perceived with lower uncertainty than challengers, which implies a disadvantage for challengers if voters are risk-averse (see e.g. Bernhardt and Ingberman 1985). In our model, a risky challenger may defeat an incumbent if he is able to organize donors appropriately, because if donors believe that the challenger will win, a majority of donors will support him, thus confirming the donors’ expectations.

The paper is organized as follows: In the next section, we provide empirical examples and implications of our model. In Sect. 3, we review the literature. In Sect. 4, we outline the model. In Sect. 5, we characterize the equilibrium when campaigns are absent. In Sect. 6, we analyze the effects of campaigns. In Sect. 7, we examine the candidate and donor equilibria, and we illustrate the multiplicity by an example. Subsequently, we discuss extensions of the model and propose some final conclusions.

2 Empirical examples and implications

Our model and its results can be used in two different ways, to draw broader conclusions and to outline empirical implications.

Empirical examples

First, there are examples that groups support candidates from the other side of the political spectrum (see e.g. Gersbach and Liessem 2002). In the federal elections in Germany in 1994, for instance, employer associations gave almost 20 percent of their total campaign support funds to the left-wing Social Democrats.¹ These contribution patterns indicate that interest groups may not only support their close political friends, but also, in some cases, more distant parties. In this paper we offer an explanation for this behavior: contributing to a party on the other side of the political spectrum will induce that party to move closer to the ideal points of the donating interest groups.

Alternative explanations

We note that there might be other reasons why interest groups may contribute to a candidate whose platform is quite distant from their ideal point. We provide two examples. First, an interest group may contribute to the funding of such a candidate because he is the incumbent, and the interest group wants to gain his favor. Second, when a candidate is likely to win, an interest group may support his campaign to have access to him after the election. In the latter case, however, the interest groups may support a candidate by money and by voting because otherwise, the money spent may be useless. The explanation advanced in this paper may be particularly pertinent when interest groups support one candidate’s campaign with money, while voting for the opponent.

Indirect test of politicians’ objectives

The results of this paper are a set of testable propositions pertaining to the relationships among a set of endogenous variables (candidates’ policies, contribution decisions, amount of contributions, electoral outcomes, etc.) and a set of exogenous variables (incumbency advantage, distribution of voters and donors).

One of our predictions is that interest groups contribute to the candidate on the other side of the political spectrum. This counterintuitive prediction can be used to test the objectives of politicians indirectly. The logic is as follows.

There is sufficient empirical evidence that in most cases, ideological interest groups support the political party that is closer to their own ideology (see the earlier survey of Potters and Sloof 1996 and Stratmann 2005). Taking these empirical results as a guideline, we can compare the results in our paper with the results in Gersbach (1998). Specifically, we have assumed in the current paper that candidates only care about winning the election, and have no policy preferences.

Suppose instead that candidates do have policy preferences. As shown in Gersbach (1998), this produces a very different distribution of campaign expenditures across winners and losers—which is much more in line with the empirical evidence discussed in Potters and Sloof (1996)—, as only the interest groups of the center might switch between the candidates. Interest groups that have stronger ideological preferences always support the candidate whose platform is closest. The huge differences between the campaign patterns and the empirical evidence infer that the candidates have strong policy preferences, as this generates a campaign contribution pattern that is consistent with the empirical evidence mentioned above.

3 Relation to the literature

Three types of advertising have been proposed for political campaigns. The first type is directly informative advertising (see Austen-Smith 1987). Voters observe candidates’ positions with noise, and campaign expenditures reduce the variance of that noise. Building on this assumption, Gersbach (1998) has developed a model of campaigns in which the contributions help candidates to get elected because risk-averse voters prefer candidates with a more precise policy position. Informative campaigning in the sense of truthful revelation about policy platforms is important when redistributive policies are considered, as shown by Schultz (2007). He develops an interesting model in which parties target campaigns on groups where most votes are gained by informing about policies. As a consequence, targeted groups will become more informed, and benefit most from redistribution.

The second type is non-directly informative advertising (see e.g. Gerber 1996; Potters et al. 1997; Gersbach 2004, and Prat 2002). Each candidate is characterized by a non-policy dimension (valence) that lobbies can observe more precisely than voters. The amount of campaign money a candidate collects signals his valence to voters. Hence the role of campaign advertising is not to convey a direct message but to credibly “burn” campaign money.² Coate (2004a, 2004b) and Ashworth (2006) have further developed the signaling approach and assume that candidates send messages to voters. These costly messages may be equivalent to money burning but may also consist of verifiable information as regards the characteristics of candidates. This approach was generalized by Vanberg (2008) to two-dimensional candidate types.

The third type is persuasive advertising (see Baron 1994; McKelvey and Ordeshook 1987; Grossman and Helpman 1996, and Ortuno Ortin and Schultz 2005). Voters are either “informed”, “uninformed” or “impressionable”. The informed electorate votes according to the policies proposed by the different political parties (or candidates). Impressionable voters are, however, poorly informed as regards the policies of the different parties, and their vote is directly influenced by campaign spending.³

We assume that the candidates can use funds to increase the share of voters supporting them. This can be interpreted as informative advertising, where candidates use money to reduce (risk-averse) voters’ uncertainty about their policy positions. We will give a precise meaning of these approaches in Sect. 6. We allow that candidates’ ability to affect voting by campaign expenditures can differ. In contrast to Gersbach (1998), who focuses on candidates with policy preferences, we assume that candidates maximize their votes.

While we perform our analysis in the framework with risk-averse voters where campaigns reduce uncertainty, it is important that the same results could be obtained in a variant of the framework suggested by Baron (1994), where voters are either informed as regards the parties’ and candidates’ intentions or not, and advertising is persuasive. The closer the ideal point of an uninformed voter is to the historical position of the candidate, the more such a voter reacts to campaigns. Therefore, a higher amount of money enables candidates to increase the share of voters for a given position.

One of our central results is that interest group donations move the political outcome towards the median voter. The reason is that donors behave strategically. If a majority of interest groups expects that a candidate will win, the candidate obtains the majority of interest group donations, allowing him to move towards the center, confirming the expectations of the interest groups. This, in turn, makes the candidate attractive for a majority of voters, which confirms the assumptions of the interest groups. This insight is complementary to the work of Wittman (2007 and 2008). Wittman (2008), for instance, highlighted the importance of allowing uninformed voters to have counterstrategies when advertising is directed towards other voters. When those uninformed voters who do not receive targeted campaign advertising respond optimally, any negative effect of pressure groups and political advertising is mitigated, and the political outcome moves towards the median voter.

4 The model

Electoral processes exhibit many features, but often, they can be described in four stages, which include political advertising. The time pattern can be described as follows:

Stage 1: :

Candidates attempt to obtain campaign support from politically active groups. Donors spend their money to enhance the expected utilities arising for them from election.

Stage 2: :

In the political strategy space, candidates choose positions that will remain fixed during the entire electoral contest.⁴ The positions are determined so as to maximize the share of voters. The voters are only imperfectly aware of the position choice of the candidates.

Stage 3: :

Candidates use their financial support to reduce the voters’ uncertainty about their position.

Stage 4: :

Individuals cast their votes, and the electoral outcome is determined by majority voting.

In comparison to the model of Gersbach (1998), two basic assumptions are modified: (a) parties are vote-maximizing instead of policy-motivated; (b) the uncertainty on the location of the parties is not only decreasing in campaign spending, but also increasing in the distance to the parties’ well-known ideological positions. The properties of the equilibria differ significantly due to these assumptions.

Formally, we assume that voters view two candidates (or parties) b and c as being located somewhere on a unidimensional political space X=[−A,A] with A>0.

The single-peaked utility function of voter i is given by

$$ u_i(w)=d_i-(w-x_i)^2 $$

(1)

where d _i >0 represents the maximum utility obtainable by i and x _i his own most-preferred point on the policy space X. The variable w denotes the policies a candidate pursues in office as perceived by voters, and is either w _b or w _c . There is a continuum of voters represented by the continuous density function g(x _i ) and distribution function G(x _i ). The median voter is denoted by m and the ideal point of the median voter is normalized to x _m =0. No assumption is needed regarding the mean position of voters.

The position choices of the candidates in stage 2 are denoted by x _b and x _c . Voters perceive the announcements of positions by candidates as a noisy signal about their true position and hence about the policies a winning candidate would pursue in office. These signals are denoted by w _b and w _c , and differ, from the voters’ point of view, from the initially announced positions x _b and x _c by random variables z _b and z _c , w _b =x _b +z _b and w _c =x _c +z _c with $\mathbb{E}(z_{b}) = \mathbb{E}(z_{c}) = 0$, where $\mathbb{E}$ denotes the expectation operator.

We allow the variance of the signal to depend on the position of the candidate. Parties or candidates are often perceived via some form of ideological label. Accordingly, we assume that there exists one location for each candidate where he has an absolute advantage concerning the certainty of his position as perceived by voters. If candidates move away from their established position, they will progressively lose this advantage, and the voters will have much greater difficulty to predict what candidates will do in office.

We use V ^b and V ^c to denote the variances of w _b and w _c , respectively. The dependence on the effective position of the candidates is given by

$$\begin{aligned} &V^b=f_b+k_b\vert x_b- \hat{x}_b\vert, \\ &V^c=f_c+k_c\vert x_c-\hat{x}_c\vert, \\ &\quad f_b, f_c, k_b, k_c>0. \end{aligned}$$

(2)

$\hat{x}_{b}$ and $\hat{x}_{c}$ denote the most firmly-established position of the candidates, that is, the location they are perceived to occupy with the lowest uncertainty. The variables f _b and f _c represent irreducible uncertainty, which we will henceforth call “floor uncertainty”. k _b and k _c represent the mobility costs. Thus, if a candidate diverges from his established point, he will generate greater uncertainty the higher his mobility cost k _b or k _c is. Since voters are assumed to be risk-averse, this makes spatial movements costly to vote-maximizing candidates. We allow that the variables f _b ,f _c and k _b ,k _c differ across candidates.⁵

We assume that $\hat{x}_{b}< x_{m}=0< \hat{x}_{c}$. This implies that we have a leftist and a rightist candidate, as in most two-candidate elections. x _m is the ideal point of the median voter. Moreover, we focus on parameter constellations for which x _b <x _c will hold in equilibrium. We assume that x _b <x _c , momentarily.

As to the magnitudes of k _b and k _c , an important remark is in order. Suppose, for instance, that a conservative candidate moves to the right, starting from a position x _c . Then, it is a plausible requirement that the probability of the candidate’s being left to a position, say $\tilde{x}_{c}$, with $\tilde{x}_{c}\leq x_{c}$, has to decline. Otherwise, the probability that he is at the center, or even a left-wing politician, would increase. This puts an upper bound on k _b and k _c . If k _c is not too large, for instance, a move to the right, starting from a given level x _c , will lower the probability that the candidate’s position is to the left of $\tilde{x}_{c}$ with $\tilde{x}_{c} \leq x_{c}$. The precise value of this upper bound on k _c and k _b depends on the distribution of z _c and z _b .⁶

Throughout this paper, we will assume that k _b and k _c are below the upper bounds, such that, e.g., the probability that a conservative candidate is at the center declines when he moves to the right.

Given the position choices x _b and x _c and the associated signals w _b and w _c , voters derive the expected utility. The expected policies are x _b and x _c . From (1) we obtain

$$\begin{aligned} \begin{aligned} E \bigl[u_i(w_b) \bigr]&=d_i-(x_b-x_i)^2-V^b, \\ E \bigl[u_i(w_c) \bigr]&=d_i-(x_c-x_i)^2-V^c. \end{aligned} \end{aligned}$$

(3)

Voter i will prefer b to c if and only if E[u _i (w _b )]>E[u _i (w _c )], which is equivalent to

$$ x_i<\frac{x_c+x_b}{2}+\frac{V^c-V^b}{2(x_c-x_b)}. $$

(4)

Therefore, voter i will cast his vote for candidate b if and only if (4) holds.

5 Candidate equilibrium without campaigns

We now deduce the equilibrium without advertising, which is called a “candidate equilibrium”. The candidates maximize their votes. We define the position of the voter who is indifferent to the two candidates’ positions as

$$ x_i^{\mathrm{ind}}=\frac{x_c+x_b}{2}+\frac{V^c-V^b}{2(x_c-x_b)}. $$

(5)

All voters with $x_{i}<x_{i}^{\mathrm{ind}}$ will support candidate b and voters with $x_{i}>x_{i}^{\mathrm{ind}}$ cast their vote for candidate c. The voter $x_{i}=x_{i}^{\mathrm{ind}}$ is indifferent between the two candidates. As he has measure zero, his voting decision is irrelevant for the outcome. Vote-share maximization requires that the goals of the candidates are $\max x_{i}^{\mathrm{ind}}$ (candidate b) and $\min x_{i}^{\mathrm{ind}}$ (candidate c).

In order to derive a candidate equilibrium as a Nash equilibrium of the candidates’ platform choices, we assume interior solutions, i.e. the platform choices satisfy $\hat{x}_{b}<x_{b}$ and $\hat{x}_{c}>x_{c}$. Precise conditions for interior solutions will be given at the end of this section.

The first-order condition for the choice x _c , given some position x _b , requires that

$$ \frac{\partial x_i^{\mathrm{ind}}}{\partial x_c}=0. $$

(6)

By calculation of the corresponding first-order condition for candidate b, we obtain (see Appendix 1):⁷

Proposition 1

In a candidate equilibrium with interior solutions, candidates choose the following platforms:

$$\begin{aligned} x_c&=\frac{f_c-f_b+k_b\hat{x}_b+k_c\hat{x}_c+\frac{1}{4}(k_b+k_c)^2}{k_b+k_c}, \end{aligned}$$

(7)

$$\begin{aligned} x_b&=\frac{f_c-f_b+k_b\hat{x}_b+k_c\hat{x}_c-\frac{1}{4}(k_b+k_c)^2}{k_b+k_c} \end{aligned}$$

(8)

and

$$ x_c=\frac{1}{2}(k_c+k_b)+x_b. $$

(9)

Hence, mobility costs k _c and k _b are the only relevant parameters that cause candidates to adopt different platforms. We note that the candidates choose different positions despite the single-peakedness of the voters’ utility functions. This result is caused by the fact that there is an incentive to deviate from a common position, e.g. the median position. It is true that a spatial movement toward more extreme positions will attract fewer voters because of the distance effect. But by approaching his established position, a candidate reduces uncertainty and gains in reputation. This will outweigh the distance effect if the candidates are very close.

If the candidates quickly lose clarity by leaving established positions (i.e. if k _c and k _b are high), the candidates will be located very separately in equilibrium. If f _b =f _c , $\hat{x}_{b}=-\hat {x}_{c}$ and k _c =k _b , we will arrive at $x_{c}=\frac{1}{4}(k_{b}+k_{c})$ and $x_{b}=-\frac{1}{4}(k_{b}+k_{c})$, and thus, candidates are located symmetrically around the median. Moreover, we obtain:

Corollary 1

Suppose f _b =f _c , $\hat{x}_{b}=-\hat{x}_{c}$. Then

$$ \lim_{k_b\rightarrow0,k_c\rightarrow0}x_b=\lim_{k_b\rightarrow 0,k_c\rightarrow0}x_c=0. $$

Hence, for very small values of k _b and k _c and symmetric locations with identical floor uncertainties, we approach the classical median voter result.

Finally, we spell out the conditions under which this equilibrium holds. We have assumed interior solutions, i.e. $\hat{x}_{b} < x_{b}$ and $x_{c} < \hat{x}_{c}$. From (8), the condition $\hat {x}_{b}<x_{b}$ yields⁸

$$ f_c-f_b+k_c( \hat{x}_c-\hat{x}_b)-\frac{1}{4}(k_b+k_c)^2 >0. $$

(10)

Analogously, using (7), the condition $x_{c}<\hat{x}_{c}$ can be rewritten as

$$ f_c-f_b+k_b( \hat{x}_b-\hat{x}_c)+\frac{1}{4}(k_b+k_c)^2 < 0. $$

(11)

Next, we turn to the investigation of campaigns. We assume throughout this paper that $\hat{x}_{b} < x_{b}$ and $x_{c} < \hat{x}_{c}$. Essentially, this requires some minimal political polarization in comparison to mobility costs. That is, $\hat{x}_{c}-\hat{x}_{b}$ must be sufficiently large relative to k _b +k _c and |f _c −f _b |. We also recall that we have assumed that k _b and k _c are sufficiently small.

6 Campaigns and political outcomes

In our model, campaigns can reduce the variances V ^b and V ^c and thus the mobility costs of candidates. To characterize the contributions of donors, we first have to investigate how exogenous changes in mobility costs affect the candidate equilibrium. Accordingly, we focus on the political outcome arising from a reduction of mobility costs.⁹

We begin by examining how a reduction of k _c affects the candidate equilibrium. If candidate c can reduce the uncertainty surrounding his position, k _c will be lowered in the third stage. Thus, we obtain a new candidate equilibrium with the same characteristics as in (7), (8), and (9), but now featuring new parameters.

From the candidate equilibrium derived in the last section, we deduce in Appendix 2:

$$ \frac{\partial x_b}{\partial k_c}=\frac{k_b(\hat{x}_c-\hat {x}_b)-f_c+f_b-\frac{1}{4}(k_b+k_c)^2}{(k_b+k_c)^2}. $$

(12)

Using condition (11), (12) implies¹⁰

$$ \frac{\partial x_b}{\partial k_c} > 0. $$

(13)

From (9) we obtain

$$ \frac{\partial x_c}{\partial k_c}= \frac{1}{2}+\frac{\partial x_b}{\partial k_c}> 0. $$

(14)

Moreover, it will also be shown in Appendix 2 that

$$ \frac{\partial x^{\mathrm{ind}}}{\partial k_c} > 0. $$

(15)

Thus, if candidate c can reduce mobility costs, we will have a new candidate equilibrium in which c will be closer to the median because his increased mobility allows him to gain more voters by approaching the median voter position. In general, candidate b will then be forced to take a more extreme position.

Similarly, we will obtain symmetrical results if candidate b is able to inform the electorate more efficiently. Now we need to investigate the candidate equilibrium in the case of a reduction of k _b . Again, the formal details are to be found in Appendix 2:

$$ \frac{\partial x_c}{\partial k_b}=\frac{k_c(\hat{x}_b-\hat {x}_c)-f_c+f_b+\frac{1}{4}(k_b+k_c)^2}{(k_b+k_c)^2}. $$

(16)

Using condition (10), (16) implies

$$ \frac{\partial x_c}{\partial k_b} < 0. $$

(17)

Additionally, we obtain¹¹

$$ \frac{\partial x_b}{\partial k_b}=\frac{\partial x_c}{\partial k_b}- \frac{1}{2} < 0 \quad \text{and}\quad\frac{\partial x^{\mathrm{ind}}}{\partial k_b} < 0. $$

(18)

Hence, if candidate b can improve communication, his position will be drawn toward the center, and he will win more votes. Thus, every candidate has a strong incentive to reduce the uncertainty of his position as perceived by the voters.¹²

7 Donor and candidate equilibrium

7.1 The donor game

We next examine the incentives of donor groups to contribute in the first stage of the electoral game. We assume that there is a finite number N (N>2) of donor groups, with ideal points characterized by the preferred point of a typical group member. Let x _j (j=1,…, N) denote the corresponding ideal points. We assume that interest groups are ordered according to their ideal points, i.e. x ₁≤x ₂≤⋯≤x _N .

We denote by E _j interest group j’s budget for candidate support. We use E _jb (resp. E _jc ) to denote the support that candidate b (resp. c) receives from group j. Therefore, E _jb +E _jc =E _j . A donor will spend money on the candidate in order to minimize the distance between his ideal point and the political outcome.

The impact of campaigns can be characterized by two mobility cost or campaign functions that depend solely on the aggregate support levels received by each candidate:

$$ k_c \Bigl(\sum^N_{j=1} E_{jc} \Bigr) \quad \mbox{and}\quad k_b \Bigl(\sum^N_{j=1} E_{jb} \Bigr). $$

(19)

The first derivatives $k_{c}'$ and $k_{b}'$ are negative because the more campaign support a candidate receives, the more uncertainty he is able to reduce.

We assume that donors are fully informed as regards the candidates’ planned policies. Thus, they observe x _b and x _c . Accordingly, the donor group will support b if and only if the support of b leads to a political outcome that is closer to the preferred point than the one arising from support for candidate c.

7.2 The value of campaign contributions

We next determine the value of campaign contributions for an individual donor when he supports either candidate b or c. For this purpose, we consider four cases. First, we assume that candidate b wins the election with or without the contribution of a donor j, given the contributions of the other donors. The value of campaigns for an individual donor j in this case is denoted by ΔU _j (b)¹³ and calculated as the difference between the utility arising from support of b and the one arising from support of c, given the decision of the other donors. Thus,

$$\begin{aligned} \Delta U_j(b)&=u_j\bigl(x_b' \bigr)-u_j(x_b) \\ &=d_j-\bigl(x_b'-x_j \bigr)^2- \bigl(d_j-(x_b-x_j)^2 \bigr) \\ &=x_b^2-{x_b'}^2+2x_j \bigl(x_b'-x_b\bigr) \\ &=\bigl(x_b'-x_b\bigr) \bigl(-x_b-x_b'+2x_j\bigr). \end{aligned}$$

(20)

If donor j supports candidate b (resp. c), $x_{b}'$ (resp. x _b ) will be the political outcome. From the previous section, we know that $x_{b}'>x_{b}$. Thus, ΔU _j (b) is monotonically increasing with x _j , and ΔU _j (b) becomes zero for $x_{j}=\frac{x_{b}'+x_{b}}{2}$. Hence, we conclude that all donors with an ideal point greater than $\frac{x_{b}'+x_{b}}{2}$ will support candidate b in such a case.

Second, the situation is completely analogous if given the contributions of the other donors, candidate c wins the election with support (position $x_{c}'$) and without support (position x _c ) of donor j. The value of campaigns for donor j is then given by

$$\begin{aligned} \Delta U_j(c)&=u_j\bigl(x_c' \bigr)-u_j(x_c) \\ &=d_j-\bigl(x_c'-x_j \bigr)^2- \bigl(d_j-(x_c-x_j)^2 \bigr) \\ &=x_c^2-{x_c'}^2+2x_j \bigl(x_c'-x_c\bigr) \\ &=\bigl(x_c-x_c'\bigr) \bigl(x_c+x_c'-2x_j\bigr). \end{aligned}$$

(21)

From inequality (14) we know that $x_{c}'$ will be smaller than x _c . All donors with most-preferred points lower than $\frac {x_{c}'+x_{c}}{2}$ will select candidate c over b for campaign support.

The third and fourth cases concern scenarios where a single donor can affect the political outcome. These cases will be discussed later.

7.3 Existence of equilibria

We finally establish the existence of candidate and donor equilibria, which we call “CD-equilibria” in the remainder of the paper. Such an equilibrium is defined as follows.

Definition 1

A CD-equilibrium consists of positions {x _b ,x _c }, donor decisions $\{E_{jc}\}_{j=1}^{N}$ and $\{E_{jb}\}_{j=1}^{N}$, and voter decisions such that these strategies constitute a subgame perfect equilibrium of the four-stage game.

We will focus on two types of CD-equilibria. In the first CD-equilibrium, candidate b wins and in the second CD-equilibrium, candidate c wins.

7.3.1 Candidate b wins

We start with the circumstances in which candidate b wins the election. We define two critical candidate positions. It will turn out that they characterize the CD-equilibrium. We have

$$\begin{aligned} x_b^*&=\frac{f_c-f_b+k^*_b\hat{x}_b+k^*_c\hat{x}_c-\frac {(k^*_b+k^*_c)^2}{4}}{k^*_b+k^*_c}, \end{aligned}$$

(22)

$$\begin{aligned} x_c^*&=\frac{f_c-f_b+k^*_b\hat{x}_b+k^*_c\hat{x}_c+\frac {(k^*_b+k^*_c)^2}{4}}{k^*_b+k^*_c} \end{aligned}$$

(23)

with

$$ k_b^* := k_b^* \bigl(x_b^* \bigr) := k_b \biggl(\sum_{j\in\{j|x_j>x_b^*\} }E_j \biggr), \qquad k_c^* := k_c^* \bigl(x_b^* \bigr) := k_c \biggl(\sum_{j\in\{j|x_j<x_b^*\} }E_j \biggr). $$

(24)

Equations (24) define two values for $k_{b}^{*}$ and $k_{c}^{*}$ that are realized if all donors to the right of $x_{b}^{*}$ support candidate b and all donors to the left of $x_{b}^{*}$ support candidate c. Definitions (24), viewed as functions of $x_{b}^{*}$, define two step-functions $k_{b}^{*} (x^{*}_{b} )$ and $k_{c}^{*} (x^{*}_{b} )$, where $k_{b}^{*} (x^{*}_{b} )$ is monotonically increasing in $x^{*}_{b}$ while $k_{c}^{*} (x^{*}_{b} )$ is monotonically decreasing in $x^{*}_{b}$. We extend the step-functions $k_{b}^{*}(x_{b}^{*})$ and $k_{c}^{*}(x_{b}^{*})$ to correspondences by including the vertical connections between two steps.

Equation (22) (with $k_{b}^{*}(x_{b}^{*})$ and $k_{c}^{*}(x_{b}^{*})$ from (24)) defines an implicit function for the determination of $x_{b}^{*}$. We show that there exists a unique value $x_{b}^{*}$ that solves (22). We consider the left-hand and the right-hand side of (22) separately. The left-hand side of (22) is trivially strictly increasing with $x^{*}_{b}$, as it is equal to $x_{b}^{*}$. We next show that the right-hand side is monotonically decreasing with $x^{*}_{b}$. This follows from the properties of $k_{b}^{*}(x_{b}^{*})$ and $k_{c}^{*}(x_{b}^{*})$, and from the fact that the lower $k_{c}^{*}$ (or the higher $k_{b}^{*}$), the lower the right-hand side. Moreover, for $x^{*}_{b}=-A$, the left-hand side is smaller than the right-hand side, as all contributors support candidate b. For $x^{*}_{b}=0$, we assume that the right-hand side is smaller than the left-hand side.¹⁴ Then, the value $x^{*}_{b}$ that solves (22) exists and is uniquely determined. The arguments are similar for $x_{c}^{*}$.

We obtain two different cases for the intersection of the left-hand side of (22) with the right-hand side.

In the first case, $x_{b}^{*}$ is exactly the ideal point of a donor whose contributions are not included in the campaign functions k _b and k _c yet. As this donor is totally satisfied with the CD-equilibrium, we assume that he splits his contributions among the candidates to ensure that the CD-equilibrium is not disrupted by his contribution. In the second case, $x_{b}^{*}$ does not coincide with any ideal point of a donor. Then, by our definition of $k_{b}^{*}$ and $k_{c}^{*}$, every donor supports one candidate only.

$x_{b}^{*}$ and $x_{c}^{*}$ characterize a situation in which candidate b receives campaign contributions from all donors with an ideal point greater than $x_{b}^{*}$, whereas candidate c will only be supported by the rest of the donors.

We next establish

Proposition 2

Suppose that $x^{\mathrm{ind}}=\frac{x^{*}_{c}+x^{*}_{b}}{2} + \frac {V^{c*}-V^{b*}}{2(x^{*}_{c}-x^{*}_{b})} > 0$ and that x ^ind remains positive if one donor changes his contribution decision.¹⁵ Then $x^{*}_{b}$ and $x^{*}_{c}$ constitute a CD-equilibrium. Candidate b wins the election, and the political outcome is $x^{*}_{b}$.

The assumptions of Proposition 2 can be expressed by the exogenous parameters of the model. We provide a specific example in Sect. 7.4.

Proof of Proposition 2

For $x_{b}^{*}$ and $x_{c}^{*}$ to be equilibrium values, we have to show that no donor has an incentive to deviate. If a donor with $x_{j}<x_{b}^{*}$ changes his support to candidate b, candidate b still wins the election and the political outcome would be greater than $x_{b}^{*}$, and hence further away from the donors’ own preferred point. For the same reason, a donor with $x_{j}>x_{b}^{*}$ will not want to switch his support from b to c, as candidate b continues to win and would move further away from his preferred position. Therefore, given the contributions of the other donors, each donor will be worse off if he deviates. By construction, $\{x_{b}^{*},x_{c}^{*}\}$ is also a candidate equilibrium. Hence $x_{b}^{*}$ and $x_{c}^{*}$ constitute a CD-equilibrium. The political outcome is $x_{b}^{*}$. □

The intuition for the equilibrium behavior of donors runs as follows: Suppose donors expect the leftist candidate b to win the election. Then donors to the left of the winning leftist candidate will give money to the rightist candidate, as this pushes the equilibrium position of the leftist candidate towards the left. Donors located to the right of the winning position will support the winner, as this draws his position to the right.

7.3.2 Candidate c wins

In this section we construct a CD-equilibrium in which candidate c wins. We define

$$\begin{aligned} x_b^{**}&=\frac{f_c-f_b+k^{**}_b\hat{x}_b+k^{**}_c\hat{x}_c-\frac {(k^{**}_b+k^{**}_c)^2}{4}}{k^{**}_b+k^{**}_c}, \end{aligned}$$

(25)

$$\begin{aligned} x_c^{**}&=\frac{f_c-f_b+k^{**}_b\hat{x}_b+k^{**}_c\hat{x}_c+\frac {(k^{**}_b+k^{**}_c)^2}{4}}{k^{**}_b+k^{**}_c}, \\ k_b^{**} := k_b^{**} \bigl(x_c^{**}\bigr)&=k_b \biggl( \sum _{j\in\{j|x_j>x_c^{**}\} }E_j \biggr),\qquad k_c^{**}:=k_c^{**} \bigl(x_c^{**}\bigr)=k_c \biggl(\sum _{j\in\{ j|x_j<x_c^{**}\}}E_j \biggr). \end{aligned}$$

(26)

Again, as in the last subsection, the construction ensures that $x_{c}^{**}$ and $x_{b}^{**}$ exist and are unique. We obtain

Proposition 3

Suppose that $x^{\mathrm{ind}}=\frac{x^{**}_{c}+x^{**}_{b}}{2} + \frac {V^{c**}-V^{b**}}{2(x^{**}_{c}-x^{**}_{b})} < 0$ and that x ^ind remains negative if one donor changes his contribution decision. Then $x_{b}^{**}$ and $x_{c}^{**}$ constitute a CD-equilibrium. Candidate c wins the election and the political outcome is $x_{c}^{**}$.

The proof of Proposition 3 follows the same lines as the one of Proposition 2.

7.3.3 Summary

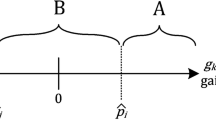
The characteristics of the equilibria are summarized in the following figure, which represents the donors’ ideal points, the median voter and the choices of candidates and donors in the CD-equilibria.

7.4 An example

We illustrate the multiplicity of equilibria by an example. Suppose the ideal points of voters are uniformly distributed on [−A,A]. The candidates’ established positions $\hat{x}^{b}$ and $\hat{x}^{c}$ are located symmetrically around the median voter x _m =0, with $\hat {x}_{b}=x_{m}-\Delta$ and $\hat{x}_{c}=x_{m}+\Delta$ for some Δ>0. Candidates are associated with the same floor level of uncertainty if they depart from their established position, i.e. f _b =f _c =f. Moreover, they have the same campaign functions:

$$\begin{aligned} k_c \Bigl( \sum E_{jc} \Bigr)&=\bar{k}-\lambda\sum E_{jc}, \end{aligned}$$

(27)

$$\begin{aligned} k_b \Bigl( \sum E_{jb} \Bigr)&=\bar{k}-\lambda\sum E_{jb}, \end{aligned}$$

(28)

with some parameter λ (λ>0). For simplicity, all contributors are located at the median position, i.e. x _j =x _m ,∀j∈{1,…,N}. The aggregate amount of campaign expenditures is denoted by $\bar{E}=\sum_{j=1}^{N} E_{j}$. We assume $\bar{k}-\lambda\bar {E}>0$. Then, we obtain

Proposition 4

There exist two CD-equilibria.

• (i) In one CD-equilibrium, all donors support candidate b. Candidate b wins and the platforms are

  $$\begin{aligned} x_b^*=\frac{\lambda\bar{E}\Delta-\frac{(2\bar{k}-\lambda\bar {E})^2}{4}}{2\bar{k}-\lambda\bar{E}}, \end{aligned}$$

  (29)
  $$\begin{aligned} x_c^*=\frac{\lambda\bar{E}\Delta+\frac{(2\bar{k}-\lambda\bar {E})^2}{4}}{2\bar{k}-\lambda\bar{E}}. \end{aligned}$$

  (30)

• (ii) In the other CD-equilibrium, all donors support candidate c. Candidate c wins and the platforms are

  $$\begin{aligned} x_b^{**}= \frac{-\lambda\bar{E}\Delta-\frac{(2\bar{k}-\lambda\bar {E})^2}{4}}{2\bar{k}-\lambda\bar{E}}, \end{aligned}$$

  (31)
  $$\begin{aligned} x_c^{**}= \frac{-\lambda\bar{E}\Delta+\frac{(2\bar{k}-\lambda\bar {E})^2}{4}}{2\bar{k}-\lambda\bar{E}}. \end{aligned}$$

  (32)

The example illustrates how two CD-equilibria emerge. Donors either run to the support of candidate b, ensuring that he wins, or they jointly secure the winning of candidate c. In both cases, the support decisions of donors are best responses.

7.5 Discussion of the assumptions and uniqueness

Before we consider further features of these equilibria, we shall first discuss the assumptions of Propositions 2 and 3 and the uniqueness issue. It is easy to demonstrate that under the assumptions of the last section, the derived equilibria are unique. Uniqueness is shown in two steps. Let us first consider the decisions of contributors. Suppose, for instance, a potential CD-equilibrium, say x _b and x _c , in which candidate b wins the election. If any donor with an ideal point lower than x _b supports candidate b, he can increase his utility by supporting c, which drives the political outcome toward his ideal point. Similarly, a donor with x _j >x _b can do no better than to support candidate b to reduce the distance between the political outcome and his preferred point.

Second, suppose there exist two equilibria in which candidate b wins, but the political platforms differ. Suppose the possible equilibrium positions of candidate b are $x_{b}^{*1}$ and $x_{b}^{*2}$, with $x_{b}^{*2}>x_{b}^{*1}$. Then, in the equilibrium with $x_{b}^{*2}$, candidate b would obtain a smaller share of contributors compared to $x_{b}^{*1}$, as he is supported by interest groups to the right. However, with $x_{b}^{*2}$, he is further away from his established position, which would require a larger share of contributors to reduce mobility. This is a contradiction and thus, there can exist only one equilibrium in which candidate b wins.

To sum up, under the assumptions of Propositions 2 and 3, the derived equilibria are unique.

Next, we discuss what happens if one assumption does not hold.

First, we have assumed that the positions $x_{b}^{*}$ and $x_{c}^{**}$ will gain a majority of voters, respectively. If this condition is not fulfilled, we will have only one CD-equilibrium. The reason is as follows: suppose, for instance, that candidate b gains no majority with $x_{b}^{*}$, as $x_{b}^{**}<x_{b}^{*}$ and $x_{c}^{**}<x_{c}^{*}$. Candidate c is certain of winning the election in the situation $(x_{b}^{**},x_{c}^{**})$, as he gains even more votes. Therefore we have at least one CD-equilibrium.

The second condition assumed in the last section states that given the constellation $(x_{b}^{*},x_{c}^{*})$ or $(x_{b}^{**},x_{c}^{**})$, no donor can change the political outcome by changing his decision. Suppose, e.g., that in a CD-equilibrium characterized by $x_{b}^{*}$ and $x_{c}^{*}$, a donor with $x_{j}>x_{c}^{*}$ can ensure that candidate c will win the election with his donations. Then, of course, he will select candidate c over b. Hence, in this case, $(x_{b}^{*}, x_{c}^{*})$ cannot be a candidate equilibrium. Thus, in general, if a donor is pivotal in a candidate equilibrium, he has an interest in changing the winning platform, and the equilibrium will not be a donor equilibrium. But again, if for instance, in $(x_{b}^{*},x_{c}^{*})$, the majority of voters in favor of candidate b is very small, which will enable one donor to change the political outcome, the CD-equilibrium with $(x_{b}^{**},x_{c}^{**})$, will in general imply a substantial majority for candidate c. So, as a rule in this case we again expect one CD-equilibrium to hold if we have enough donors.¹⁶

7.6 Implications

The derived CD-equilibria have some remarkable consequences. We now discuss several important features of the case when all assumptions hold and both CD-equilibria exist.

Both candidates have a chance of winning the election that depends on the realization of the CD-equilibrium. Moreover, some donor groups will support a candidate whose position is not closest to their own ideal point. In a CD-equilibrium with $(x_{b}^{*}, x_{c}^{*})$, on the other hand, donors with $x_{j}<x_{b}^{*}$ will support candidate c, whereas a donor with $x_{j}=x_{c}^{*}$ will contribute to funding of candidate b’s campaign. In any case, however, donors located around the median will support the winning candidate. If the median donor coincides with the median voter, the median donor will always contribute to the candidate whose position is closest to his.

Campaign support that increases the mobility of both candidates leads to a convergence of the candidates’ positions in the CD-equilibrium since

$$ x_c=\frac{k_c+k_b}{2}+x_b, $$

and k _c and k _b decrease due to advertising.¹⁷

This convergence does not end at the median or in equal locations, but the positions with campaigns are closer than those without campaigns.

Moreover, symmetrical political and support constellations yield asymmetrical outcomes. Suppose prospective campaign funds are symmetrically distributed around the median position, and $\hat{x}_{c}=-\hat{x}_{b}$, f _c =f _b , and k _c =k _b without campaigns. Then, in a CD-equilibrium, the candidates do not take up symmetrical positions. By contrast, in equilibrium, one candidate will attract the majority of donors and win despite the fact that both candidates are equally attractive at the outset.

A property of the equilibria is that small differences in candidate positions without campaigns do not destroy the incentives for donors to contribute, because a reduction of uncertainty affects the equilibrium positions independently of their distance. Political controversy is not a necessary condition for fundraising, which adds an important twist to the literature (e.g. Congleton 1989).

The increase of mobility by campaigns does not necessarily imply that voters perceive lower uncertainty in equilibrium. Let us consider a constellation in which candidate b is located in his established point $\hat{x}_{b}$ without campaigns and wins the election. In the CD-equilibrium in which b wins, voters will perceive higher uncertainty, since b is drawn toward the center, which is associated with higher uncertainty compared to the outcome without campaigns. Thus campaigns that reduce mobility costs can raise uncertainty in a CD-equilibrium.

It has been argued that consistent incumbents are perceived as a lottery with smaller variance than any challenger (e.g. Bernhardt and Ingberman 1985 and Anderson and Glomm 1992). This fact can be easily incorporated into our framework. Suppose candidate c is the incumbent. We assume that $\hat{x}_{c}=-\hat{x}_{b}$, f _c <f _b , and k _c <k _b without any campaign support. Then the incumbent will win the election without campaigns, since (5), (7), and (8) imply that $x_{i}^{\mathrm{ind}}<0=x_{m}$. But our model shows that despite this initial advantage, there may be a CD-equilibrium in which the challenger will win the election if he attracts the major part of the donors. This suggests another way of looking at incumbent/challenger competition, which is characterized by the difficulty of defeating the incumbent. If and only if the challenger is able to organize donor support much better than the incumbent, will he be able to defeat the incumbent. Hence, the electoral advantage for the incumbent can suddenly be outweighed by a new organization of donors by the challenger.

8 Concluding remarks

We have examined a simple model of campaigns in which contributors support candidates, who can then engage in more costly campaigning. We have argued that campaigns may induce a run by a number of interest groups to support one candidate.

The model presented in this paper can be extended in several directions. It could be complemented by other aspects of political campaigning, such as an exchange of money for services or for access to an officeholder. This would tend to strengthen the incentives of interest groups to support the winning candidate, and would reinforce the run phenomenon.

The results in this paper constitute a set of testable propositions pertaining to the relationships between a set of endogenous variables (candidates’ policies, contribution decisions, amount of contributions, electoral outcomes) and a set of exogenous variables (incumbency advantage, distribution of voters and donors). In particular, we have assumed that candidates only care about maximizing their votes. If we assumed that candidates also have policy preferences, this produces a very different distribution of campaign expenditures across winners and losers,¹⁸ which is much more in line with the empirical evidence discussed in Potters and Sloof (1996), as only interest groups that are in the center may switch between candidates. Comparing Gersbach (1998) and this paper thus constitutes a new indirect test of politicians’ objectives.

Appendices

Appendix 1

First we deduce the candidate equilibrium from (5):

$$ x_i^{\mathrm{ind}}=\frac{x_c+x_b}{2}+\frac{V^c-V^b}{2(x_c-x_b)}, $$

and from the candidate goals $\max x_{i}^{\mathrm{ind}}$ (candidate b) and $\min x_{i}^{\mathrm{ind}}$ (candidate c). Given some position x _b , the first-order condition for the choice x _c is given by

$$ \frac{\partial x_i^{\mathrm{ind}}}{\partial x_c}=\frac{1}{2} \biggl(1-\frac {f_c-f_b-k_cx_c+k_c\hat{x}_c-k_bx_b+k_b\hat{x}_b}{(x_c-x_b)^2}- \frac {k_c}{x_c-x_b} \biggr)=0. $$

Similarly, the first-order condition for x _b is

$$ \frac{\partial x_i^{\mathrm{ind}}}{\partial x_b}=\frac{1}{2} \biggl(1+\frac {f_c-f_b-k_cx_c+k_c\hat{x}_c-k_bx_b+k_b\hat{x}_b}{(x_c-x_b)^2}- \frac {k_b}{x_c-x_b} \biggr)=0. $$

By adding these two equations we obtain

$$ 1-\frac{k_c+k_b}{2(x_c-x_b)}=0, $$

which leads to

$$ x_c=\frac{1}{2}(k_c+k_b)+x_b. $$

Thus the candidates take different positions in equilibrium, depending on the mobility costs. We insert $x_{c}-x_{b}=\frac{1}{2}(k_{c}+k_{b})$ into the first first-order condition and obtain

$$ 1-\frac{f_c-f_b+k_c\frac{1}{2}(k_b+k_c)+k_c (\hat{x}_c-x_b-\frac {1}{2}(k_b+k_c) )-k_b(x_b-\hat{x}_b)}{\frac{1}{4}(k_c+k_b)^2}=0, $$

which implies

$$ \frac{1}{4}(k_c+k_b)^2=f_c-f_b-x_b(k_b+k_c)+k_c \hat{x}_c+k_b\hat{x}_b. $$

Thus we find that

$$ x_b=\frac{f_c-f_b+k_b\hat{x}_b+k_c\hat{x}_c-\frac{1}{4}(k_b+k_c)^2}{k_b+k_c}. $$

Because of $x_{c}=\frac{1}{2}(k_{c}+k_{b})+x_{b}$ we obtain

$$ x_c=\frac{f_c-f_b+k_b\hat{x}_b+k_c\hat{x}_c+\frac{1}{4}(k_b+k_c)^2}{k_b+k_c}. $$

Appendix 2

We calculate the derivative of x _b with respect to k _c :

$$\begin{aligned} \frac{\partial x_b}{\partial k_c}&=\frac{(k_b+k_c)\hat{x}_c-\frac {1}{2}(k_b+k_c)^2-f_c+f_b-k_b\hat{x}_b-k_c\hat{x}_c+\frac {1}{4}(k_b+k_c)^2}{(k_b+k_c)^2} \\ &=\frac{k_b(\hat{x}_c-\hat{x}_b)+f_b-f_c-\frac{1}{4}(k_b+k_c)^2}{(k_b+k_c)^2}. \end{aligned}$$

By using $\frac{\partial x_{c}}{\partial k_{c}}=\frac{1}{2}+\frac{\partial x_{b}}{\partial k_{c}}$, we derive

$$\begin{aligned} \frac{\partial x^{\mathrm{ind}}}{\partial k_c} =&\frac{\partial}{\partial k_c} \biggl(\frac{1}{2}(x_c+x_b)+ \frac{V^c-V^b}{2(x_c-x_b)} \biggr) \\ =&\frac{1}{2} \biggl\lbrace \frac{\partial x_b}{\partial k_c}+\frac {1}{2}+ \frac{\partial x_b}{\partial k_c}+\frac{\partial}{\partial k_c} \biggl(\frac{f_c+k_c(\hat{x}_c-x_c)-f_b-k_b(x_b-\hat {x}_b)}{x_c-x_b} \biggr) \biggr\rbrace \\ =&\frac{\partial x_b}{\partial k_c}+\frac{1}{4}+\frac{f_b-f_c+\frac {\partial x_b}{\partial k_c}(-k_c-k_b)(k_b+k_c)}{(k_b+k_c)^2} \\ &{}+\frac{\hat{x}_c(k_b)-k_b\hat{x}_b-\frac {1}{2}(k_c+k_b)^2}{(k_b+k_c)^2} \\ =&\frac{f_b-f_c+k_b(\hat{x}_c-\hat{x}_b)-\frac{1}{4}(k_c+k_b)^2}{(k_b+k_c)^2}. \end{aligned}$$

The last expression coincides exactly with $\frac{\partial x_{b}}{\partial k_{c}}$. Thus $\frac{\partial x^{\mathrm{ind}}}{\partial k_{c}}=\frac{\partial x_{b}}{\partial k_{c}} > 0$. Similarly, we obtain

$$\begin{aligned} \frac{\partial x_c}{\partial k_b}&=\frac{(k_b+k_c)\hat{x}_b+\frac {1}{2}(k_b+k_c)^2-f_c+f_b-k_b\hat{x}_b-k_c\hat{x}_c-\frac {1}{4}(k_b+k_c)^2}{(k_b+k_c)^2} \\ &=\frac{k_c(\hat{x}_b-\hat{x}_c)+f_b-f_c+\frac{1}{4}(k_b+k_c)^2}{(k_b+k_c)^2}, \\ \frac{\partial x^{\mathrm{ind}}}{\partial k_b}&=\frac{\partial x_c}{\partial k_b} < 0. \end{aligned}$$