1 Introduction

Nonlinear system theory finds wide-ranging applications in robotics (Aji et al. 2021), control systems (Alquran and Jaradat 2018), finance (Almutairi et al. 2021), machine learning (Gilpin et al. 2020), biomedical engineering (Shams et al. 2023), and environmental modeling (Schuwirth et al. 2019). Its use spans from designing stable controllers for robots to modeling complex behaviors in financial markets, powering neural networks for machine learning tasks, and understanding physiological and ecological systems. These practical applications highlight the versatility and significance of nonlinear system theory in diverse fields, contributing to advancements and insights in various domains. Because of the numerous practical uses of nonlinear systems, there has been a significant surge in interest among researchers in finding solutions for these types of equations. The exploration of nonlinear partial differential equations (NLPDEs) represents a fiercely competitive and demanding area of research. Scholars in this field are dedicated to comprehending the intricacies of equations with multiple variables and partial derivatives, moving away from the conventional linear framework. NLPDEs pose significant challenges, urging researchers to employ various analytical and computational tools. The pursuit of understanding these complex equations is driven by their wide-ranging implications across diverse scientific disciplines and technological applications (Abro et al. 2021; Malik et al. 2023; Abdelrahman and Alkhidhr 2020; Beck et al. 2019; Yan et al. 2021; Ali et al. 2023), making it a captivating and vital field of study.

The research makes a valuable contribution by exploring a diverse range of soliton solutions that encompass various wave forms. Solitons, being non-dispersive and self-sustaining wave packets that maintain their shape and speed as they propagate through a medium, hold immense importance in almost every scientific discipline. The significance of solitons lies in their widespread presence and influence across various fields of study. Solitons, fundamental and versatile entities, are crucial components in diverse physics disciplines, including nonlinear optics, condensed matter physics, and plasma physics. These solitary waves maintain their shape and coherence during propagation, setting them apart from conventional waves (Attia et al. 2023). Solitons play a pivotal role in high-speed data transmission through optical fibers (Andreeva and Potapov 2020), aid in understanding phenomena like superconductivity in condensed matter systems (Akbar et al. 2023), and contribute to advancements in plasma physics research (Deng et al. 2020). Moreover, their applications extend beyond fundamental physics, finding practical uses in various fields, such as modeling biological processes in medicine and studying nonlinear dynamics in neuronal systems (Takembo et al. 2019; Khodadadi et al. 2023; Ma and Li 2023). The significance and utility of solitons continue to drive innovation and research across a wide range of scientific domains. Investigating and defining different types of solitons, like bell-type solitons, lump solitons, combo-dark bright solitons, cuspons solitons, and rogue waves, presents exciting opportunities for advancing technology and gaining a valuable understanding of intricate systems’ dynamics. Such research holds the potential for significant technological advancements and valuable insights into the behavior of complex systems (Yang et al. 2019).

Numerous innovative techniques have been developed to ensure the accuracy and approximateness of NLPDE solutions. These methodologies enable us to conduct qualitative and quantitative analyses of these complex equations efficiently. Through the application of these distinct methods, we can obtain reliable solutions, advancing our understanding and practical utilization of NLPDEs in various fields such as physics, engineering, and computational science. Over the last few decades, various novel mathematical techniques have been introduced, each contributing significantly to the field. Among these ground-breaking methods are the exponential function method (Ahmad et al. 2023), the modified exp\((-\Phi (\eta ))\)-function method (Ahmad and Mustafa 2023), the Hirota’s direct method (Yang et al. 2022; Tariq et al. 2022), the improved F-expansion method (Tariq et al. 2023), the new extended hyperbolic function method (Tariq et al. 2023), the extended three soliton test method (Younis et al. 2021), the extended modified auxiliary equation mapping approach (Tariq et al. 2022a), the modified Kudryashov method (Li et al. 2021; Khater 2021a), the auxiliary equation method (Tariq and Seadawy 2019), the trigonometric-quantic-B-spline method (Khater and Lu 2021), the extended simplest equation method (Khater et al. 2021), the modified Khater method (Khater 2021b), generalized Riccati-expansion analytical scheme (Khater et al. 2021), Elkalla-expansion method (Khater and Ahmed 2021), the tanh-coth method (Rani et al. 2021), the generalized exponential rational function method (Kumar et al. 2020), the homotopy perturbation technique (He and El-Dib 2021), the trial equation method (Hu et al. 2021), the improved tanh method (Yokuş et al. 2022) and the sine-cosine method (Liang et al. 2022).

In 2017, Yang employed the Hirota’s bilinear forms to make a significant breakthrough in uncovering plentiful lump-type solutions for the JME (Yang and Ma 2017). In the year 2020, Hao-Nan Xu made a remarkable advancement in the study of multi-exponential wave solutions for the JME by utilizing the principle of the linear superposition (Xu et al. 2020). In 2021, Sachin Kumar achieved a noteworthy breakthrough in the investigation of closed-form invariant solutions for the JME through the application of the Lie symmetry method (Kumar et al. 2021).

In 2019, Dharmendra Kumar employed the bilinear neural network technique to investigate the GSWE (Kumar and Kumar 2019). The objective was to discover fresh periodic solitary wave solutions using this innovative approach. In the year 2020, Andronikos Paliathanasis utilized the Lie symmetries and singularity analysis to study the GSWE (Paliathanasis 2020). The primary aim was to unveil a variety of distinct soliton solutions using these methodologies. In 2021, Chaudry Masood Khalique utilized the Kudryashov’s approach to investigate the GSWE (Khalique and Plaatjie 2021). The main objective was to identify exact solutions and conserved vectors using this particular method. In 2022, Jian-Guo Liu employed the three-wave method to study the GSWE (Liu and Osman 2022). The primary focus was to discover various non-autonomous wave structure solutions using this particular approach. In 2019, Yuan Zhou utilized the Hirota direct method to investigate the HSIE (Zhou et al. 2019). The main objective was to identify lump and lump-soliton solutions using this specific method. In the year 2020, Si-Jia Chen employed a Backlund transformation to study the HSIE (Chen et al. 2020). The primary focus of the research was to discover exact solutions and analyze the interaction behavior of the equation. In 2021, Fan Yong-Yan successfully obtained new periodic wave solutions for the HSIE using the Hirota bilinear operator as a tool of investigation (Yong-Yan et al. 2021). In 2022, Fei Long accomplished the discovery of new interaction solutions for the HSIE by employing the Hirota direct method as a valuable tool of analysis (Long et al. 2022). In 2022, Zhen-ao Mou utilized the bilinear neural network method to discover analytical solutions for the HSIE. The reviewed literature provides valuable insights and lays the groundwork for further exploration in this research area.

The reason for considering these models in this study is because each of these equations holds distinctive importance in different branches of science and engineering. The JME is recognized for its relevance in describing soliton phenomena, which are unique wave-like behaviors observed in various physical systems. The GSWE finds applications in coastal dynamics, specifically in understanding the behavior of water waves in coastal regions. On the other hand, the HSIE is significant in the field of nonlinear optics, which deals with the behavior of light in nonlinear media. By exploring these equations, researchers can gain insights into various aspects of wave behavior, from solitons to water waves to optical phenomena. Each equation brings its own set of challenges and characteristics, making them intriguing subjects for study. Therefore, the decision to consider these three equations stems from their importance and relevance in distinct areas of science and engineering, offering valuable contributions to our understanding of various physical systems.

In this paper, a novel method called the EEM is introduced, enabling the direct discovery of traveling wave solutions to NLPDEs. The effectiveness and reliability of the EEM may vary based on factors such as equation complexity, the problem domain, and the underlying assumptions employed. This mathematical technique has been successfully applied across various scientific fields to obtain solutions for both nonlinear evolution equations (NLEEs) and NLPDEs. Researchers led by J. Ahmad utilized the EEM to investigate soliton solutions concerning the Caudrey-Dodd-Gibbon equation in 2022 (Rani et al. 2022). In 2023, Zulaikha Mustafa conducted research on the nonlinear resonant Schrodinger equation, employing the EEM (Ahmad and Mustafa 2023). The study involved the application of conformable derivatives and stability analysis in her investigation.

The research paper follows the subsequent structure: To begin with, it presents an introduction in Sect. 1. In Sect. 2, a summary of the EEM is provided. Moving on to Sect. 3, various structures of the soliton solutions of the BLMPE, the GSWE, and the HSIE are described. The obtained results are presented using graphs in Sect. 4. Finally, Sect. 5 contains the conclusion of the study.

2 Summary of method

By considering general NLPDEs, we are dealing with a class of PDEs that contain nonlinear terms.

$$\begin{aligned} \text {P}(\text {G},~\text {G}_{\text {t}},~\text {G}_{\text {x}},~\text {G}_{\text {y}},~\text {G}_{\text {tt}},~\text {G}_{\text {xt}},~\text {G}_{\text {xy}},~\text {G}_{\text {xx}},...)=0. \end{aligned}$$
(1)

The wave transformation for NLPDE can be written as

$$\begin{aligned} \text {G}(x, t)=\text {g}(\zeta ), ~~~ \zeta = -\sigma t+\kappa x+y \omega . \end{aligned}$$
(2)

The coefficient \(\sigma \) is associated with the time variable t in the transformed equation. It determines the rate at which the wave’s phase evolves with time. The coefficient \(\kappa \) is related to the spatial variable x in the transformation. It influences the wave’s propagation in space and represents the rate of change of phase with respect to the spatial coordinate. The coefficient \(\omega \) is associated with the variable y in the transformation. It often represents the angular frequency of the wave. Applying wave transformation

$$\begin{aligned} \text {Q}(\text {g}, \text {g}', \text {g}'', \text {g}''', \text {g}'''',...)=0. \end{aligned}$$
(3)

The solutions to Eq. (1) can be expressed using the EEM, where the symbol \('\) denotes the derivative with respect to \(\zeta \) (Ahmad et al. 2023).

$$\begin{aligned} \text {g}(\zeta )=\sum _{m=0}^{N} A_{m}(\exp (-\Phi (\zeta )))^m, \end{aligned}$$
(4)

where \(A_{m}\) are constants, \(A_{m}\ne 0\) and \(0\le m \le N\).

$$\begin{aligned} \Phi ^{'}(\zeta )= b \exp (\phi (\zeta ))+\exp (-\phi (\zeta ))+ a . \end{aligned}$$
(5)

The solutions of Eq. (5) can be obtained by taking the derivative with respect to \(\zeta \).


Cluster-i:

If \( b \ne 0\) and \( a ^2-4 b >0\), then

$$\begin{aligned} \Phi (\zeta )=\ln \left( \frac{-\sqrt{ a ^2-4 b }}{2 b } ~\text {tanh}~\left( \frac{\sqrt{ a ^2-4 b }}{2}(\zeta +F)\right) -\frac{ a }{2 b }\right) . \end{aligned}$$
(6)

Cluster-ii:

If \( b \ne 0\) and \( a ^2-4 b <0\), then

$$\begin{aligned} \Phi (\zeta )=\ln \left( \frac{\sqrt{ a ^2-4 b }}{2 b } ~\text {tan}~\left( \frac{\sqrt{ a ^2-4 b }}{2}(\zeta +F)\right) -\frac{ a }{2 b }\right) . \end{aligned}$$
(7)

Cluster-iii:

If \( b =0\), \( a \ne 0\) and \( a ^2-4 b >0\), then

$$\begin{aligned} \Phi (\zeta )=-\ln {\frac{ a }{\exp \left( a (\zeta +F)\right) -1}}. \end{aligned}$$
(8)

Cluster-iv:

If \( b \ne 0\), \( a \ne 0\) and \( a ^2-4 b =0\), then

$$\begin{aligned} \Phi (\zeta )=\ln {\frac{2( a (\zeta +H)+2)}{( a ^2(\zeta +F)}}. \end{aligned}$$
(9)

Cluster-v: If \( b =0\), \( a =0\) and \( a ^2-4 b =0\), then

$$\begin{aligned} \phi (\zeta )=\ln (\zeta +F). \end{aligned}$$
(10)

3 Extraction of soliton solutions

In this section, we will apply the JME, GSWE, and HSIE methods to implement the previously discussed methodology.

3.1 Jimbo-Miwa equation

This subsection aims to address the JME and find its solution (Yin et al. 2023; Yang and Ma 2017; Xu et al. 2020).

$$\begin{aligned} 2 \text {G}_{yt}+\text {G}_{xxxy}-3\text {G}_{xz}+3\text {G}_{xx}\text {G}_{y}+3\text {G}_{x}\text {G}_{xy}=0, \end{aligned}$$
(11)

where g is a function that depends on variables x, y, and t. Using the wave transformation of Eq. (2), we derive the resulting expression.

$$\begin{aligned} \kappa ^3 \omega g^{(3)}(\zeta )-3 \kappa ^2 \omega g'(\zeta )^2-\sigma \omega g'(\zeta )=0. \end{aligned}$$
(12)

Through the implementation of balancing techniques on Eq. (12) involving \(g^{(3)}\) and \((g')^2\), we achieve the following result.

$$\begin{aligned} m+3= & {} 2(m+1).\\ \Rightarrow ~ m= & {} 1. \end{aligned}$$

Setting m = 1 in Eq. (4) yields the following expression.

$$\begin{aligned} \text {g}(\zeta )=A_1 \exp (-\Phi (\zeta ))+A_o. \end{aligned}$$
(13)

By substituting Eqs. (5) and (13) into Eq. (12), we obtain the following result.

$$\begin{aligned} B_{-4}e^{-4\Phi (\zeta )}+B_{-3}e^{-3\Phi (\zeta )}+B_{-2}e^{-2\Phi (\zeta )}+B_{-1} e^{-\Phi (\zeta )}+B_o=0, \end{aligned}$$
(14)

where

$$\begin{aligned}{} & {} B_{-4}=-6 A_1 \kappa ^3 \omega -3 A_1^2 \kappa ^2 \omega ,\nonumber \\{} & {} \quad B_{-3}=-12 a A_1 \kappa ^3 \omega -6 a A_1^2 \kappa ^2 \omega ,\nonumber \\{} & {} \quad B_{-2}=-7 a ^2 A_1 \kappa ^3 \omega -3 a ^2 A_1^2 \kappa ^2 \omega -8 A_1 b \kappa ^3 \omega -6 A_1^2 b \kappa ^2 \omega +A_1 \sigma \omega ,\nonumber \\{} & {} \quad B_{-1}=- a ^3 A_1 \kappa ^3 \omega -8 a A_1 b \kappa ^3 \omega -6 a A_1^2 b \kappa ^2 \omega + a A_1 \sigma \omega ,\nonumber \\{} & {} \quad B_o=- a ^2 A_1 b \kappa ^3 \omega -2 A_1 b ^2 \kappa ^3 \omega -3 A_1^2 b ^2 \kappa ^2 \omega +A_1 b \sigma \omega .\nonumber \\{} & {} \quad {\left\{ \begin{array}{ll} B_{-4}=0, B_{-3}=0,\\ B_{-2}=0, B_{-1}=0, B_{o}=0. \end{array}\right. } \end{aligned}$$
(15)

Solving the system, the resulting outcome is as follows.

$$\begin{aligned} {\left\{ \begin{array}{ll} A_1=\frac{2 \root 3 \of {-1} \root 3 \of {\sigma }}{\root 3 \of { a ^2-4 b }},~\\ \kappa =-\frac{\root 3 \of {-1} \root 3 \of {\sigma }}{\root 3 \of { a ^2-4 b }}. \end{array}\right. } \end{aligned}$$
(16)

Through the utilization of Eqs. (5), (13), (12), and (16), the solutions for Eq. (11) are as follows:


Cluster-i:

$$\begin{aligned} G_{1}(x, y,t)=A_o-\frac{4 \root 3 \of {-1} b \root 3 \of {\sigma }}{\root 3 \of { a ^2-4 b } \left( \lambda -\sqrt{4 b - a ^2} \tan \left( \frac{1}{2} \sqrt{4 b - a ^2} \left( F-\sigma t-\frac{\root 3 \of {-1} \root 3 \of {\sigma } x}{\root 3 \of { a ^2-4 b }}+y \omega \right) \right) \right) }. \end{aligned}$$

Cluster-ii:

$$\begin{aligned} G_{2}(x, y,t)=A_o-\frac{4 \root 3 \of {-1} b \root 3 \of {\sigma }}{\root 3 \of { a ^2-4 b } \left( a -\sqrt{4 b - a ^2} \tan \left( \frac{1}{2} \sqrt{4 b - a ^2} \left( F-\sigma t-\frac{\root 3 \of {-1} \root 3 \of {\sigma } x}{\root 3 \of { a ^2-4 b }}+y \omega \right) \right) \right) }. \end{aligned}$$

Cluster-iii:

$$\begin{aligned} G_{3}(x, y,t)=A_o+\frac{2 \root 3 \of {-1} a \root 3 \of {\sigma }}{\root 3 \of { a ^2-4 b } \left( \exp \left( a \left( F-\sigma t-\frac{\root 3 \of {-1} \root 3 \of {\sigma } x}{\root 3 \of { a ^2-4 b }}+y \omega \right) \right) -1\right) }. \end{aligned}$$

Cluster-iv:

$$\begin{aligned} G_{4}(x, y,t)=A_o+\frac{4 \root 3 \of {-1} \root 3 \of {\sigma } \left( a +\frac{2}{F-\sigma t-\frac{\root 3 \of {-1} \root 3 \of {\sigma } x}{\root 3 \of { a ^2-4 b }}+y \omega }\right) }{ a ^2 \root 3 \of { a ^2-4 b }}. \end{aligned}$$

Cluster-v:

$$\begin{aligned} G_{5}(x, y,t)=A_o-\frac{2 \root 3 \of {-1} \root 3 \of {\sigma }}{\root 3 \of {-1} \root 3 \of {\sigma } x-\root 3 \of { a ^2-4 b } (F-\sigma t+y \omega )}. \end{aligned}$$

3.2 Generalized shallow water equation(GSWE)

This subsection aims to address the solution of the GSWE (Yin et al. 2023).

$$\begin{aligned} \text {G}_{yt}+\alpha \text {G}_{y}\text {G}_{xx}+2\alpha \text {G}_{x}\text {G}_{xy}+\beta \text {G}_{xy}+\gamma \text {G}_{xxxy}=0. \end{aligned}$$
(17)

In the given expression, \(\alpha \), \(\beta \), and \(\gamma \) represent constants, while g is a function that depends on variables x, y, and t. Using the wave transformation of Eq. (2), we derive the resulting expression.

$$\begin{aligned} \gamma \kappa ^3 \omega g^{(3)}(\eta )+\frac{3}{2} \alpha \kappa ^2 \omega g'(\eta )^2+\beta \kappa \omega g'(\eta )-\sigma \omega g'(\eta )=0. \end{aligned}$$
(18)

By employing balancing techniques, we arrive at the result m=1. By substituting Eqs. (5) and (13) into Eq. (18), we obtain the following result.

$$\begin{aligned} C_{-4}e^{-4\Phi (\eta )}+C_{-3}e^{-3\Phi (\eta )}+C_{-2}e^{-2\Phi (\eta )}+C_{-1} e^{-\Phi (\eta )}+C_o=0, \end{aligned}$$
(19)

where

$$\begin{aligned} C_{-4}= & {} \frac{3}{2} \alpha A_1^2 \kappa ^2 \omega -6 A_1 \gamma \kappa ^3 \omega ,\nonumber \\ C_{-3}= & {} 3 a \alpha A_1^2 \kappa ^2 \omega -12 a A_1 \gamma \kappa ^3 \omega ,\nonumber \\ C_{-2}= & {} \frac{3}{2} a ^2 \alpha A_1^2 \kappa ^2 \omega -7 a ^2 A_1 \gamma \kappa ^3 \omega +3 \alpha A_1^2 b \kappa ^2 \omega -8 A_1 b \gamma \kappa ^3 \omega -A_1 \beta \kappa \omega +A_1 \sigma \omega ,\nonumber \\ C_{-1}= & {} - a ^3 A_1 \gamma \kappa ^3 \omega +3 a \alpha A_1^2 b \kappa ^2 \omega -8 a A_1 b \gamma \kappa ^3 \omega - a A_1 \beta \kappa \omega + a A_1 \sigma \omega ,\nonumber \\ C_o= & {} - a ^2 A_1 b \gamma \kappa ^3 \omega +\frac{3}{2} \alpha A_1^2 b ^2 \kappa ^2 \omega -2 A_1 b ^2 \gamma \kappa ^3 \omega -A_1 b \beta \kappa \omega +A_1 b \sigma \omega .\nonumber \\ {\left\{ \begin{array}{ll} C_{-4}=0, C_{-3}=0,\\ C_{-2}=0, C_{-1}=0, C_{o}=0. \end{array}\right. } \end{aligned}$$
(20)

Solving the system, the resulting outcome is as follows.


Case 1:

$$\begin{aligned} {\left\{ \begin{array}{ll} A_1=\frac{4 \gamma \kappa }{\alpha },~\\ \sigma =\kappa \left( \gamma \kappa ^2 \left( a ^2-4 b \right) +\beta \right) . \end{array}\right. } \end{aligned}$$
(21)

Through the utilization of Eqs. (5), (13), (18), and (21), the solutions for Eq. (17) are as follows:

Cluster-i:

$$\begin{aligned} G_{6}(x, y, t)=A_o-\frac{8 b \gamma \kappa }{ a \alpha +\alpha \sqrt{ a ^2-4 b } \tanh \left( \frac{1}{2} \sqrt{ a ^2-4 b } \left( F-\kappa t \left( \gamma \kappa ^2 \left( a ^2-4 b \right) +\beta \right) +\kappa x+y \omega \right) \right) }. \end{aligned}$$

Cluster-ii:

$$\begin{aligned} G_{7}(x, y, t)=A_o-\frac{8 b \gamma \kappa }{ a \alpha -\alpha \sqrt{4 b - a ^2} \tan \left( \frac{1}{2} \sqrt{4 b - a ^2} \left( F-\kappa t \left( \gamma \kappa ^2 \left( a ^2-4 b \right) +\beta \right) +\kappa x+y \omega \right) \right) }. \end{aligned}$$

Cluster-iii:

$$\begin{aligned} G_{8}(x, y, t)=A_o+\frac{4 a \gamma \kappa }{\alpha \left( \exp \left( a \left( F-\kappa t \left( \gamma \kappa ^2 \left( a ^2-4 b \right) +\beta \right) +\kappa x+y \omega \right) \right) -1\right) }. \end{aligned}$$

Cluster iv:

$$\begin{aligned} G_{9}(x, y, t)=A_o+\frac{8 \gamma \kappa \left( a +\frac{2}{F-\kappa t \left( \gamma \kappa ^2 \left( a ^2-4 b \right) +\beta \right) +\kappa x+y \omega }\right) }{ a ^2 \alpha }. \end{aligned}$$

Cluster v:

$$\begin{aligned} G_{10}(x, y, t)=A_o+\frac{4 \gamma \kappa }{\alpha \left( F+\kappa \left( x-t \left( \gamma \kappa ^2 \left( a ^2-4 b \right) +\beta \right) \right) +y \omega \right) }. \end{aligned}$$

3.3 Hirota-Satsuma-Ito equation

This subsection focuses on the solution of the HSIE (Chen et al. 2023; Yong-Yan et al. 2021; Long et al. 2022).

$$\begin{aligned} \text {G}_{\text {xxxt}}+3 (\text {G}_{\text {x}}\text {G}_{\text {t}})_x+\text {G}_{\text {yt}}+\text {G}_{\text {xx}}=0, \end{aligned}$$
(22)

where g is a function that depends on variables x, y, and t. Using the wave transformation of Eq. (2), we derive the resulting expression.

$$\begin{aligned} \kappa ^3 (-\sigma ) g^{(3)}(\eta )-3 \kappa ^2 \sigma g'(\eta )^2+\kappa ^2 g'(\eta )-\sigma \omega g'(\eta )=0. \end{aligned}$$
(23)

By employing balancing techniques, we arrive at the result m=1. By substituting Eq. (5) and Eq. (13) into Eq. (23), we obtain the following result.

$$\begin{aligned} D_{-4}e^{-4\Phi (\eta )}+D_{-3}e^{-3\Phi (\eta )}+D_{-2}e^{-2\Phi (\eta )}+D_{-1} e^{-\Phi (\eta )}+D_o=0, \end{aligned}$$
(24)

where

$$\begin{aligned} D_{-4}= & {} 6 A_1 \kappa ^3 \sigma -3 A_1^2 \kappa ^2 \sigma ,\nonumber \\ D_{-3}= & {} 12 a A_1 \kappa ^3 \sigma -6 a A_1^2 \kappa ^2 \sigma ,\nonumber \\ D_{-2}= & {} 7 a ^2 A_1 \kappa ^3 \sigma -3 a ^2 A_1^2 \kappa ^2 \sigma +8 A_1 b \kappa ^3 \sigma -6 A_1^2 b \kappa ^2 \sigma -A_1 \kappa ^2+A_1 \sigma \omega ,\nonumber \\ D_{-1}= & {} a ^3 A_1 \kappa ^3 \sigma +8 a A_1 b \kappa ^3 \sigma -6 a A_1^2 b \kappa ^2 \sigma - a A_1 \kappa ^2+ a A_1 \sigma \omega ,\nonumber \\ D_o= & {} a ^2 A_1 b \kappa ^3 \sigma +2 A_1 b ^2 \kappa ^3 \sigma -3 A_1^2 b ^2 \kappa ^2 \sigma -A_1 b \kappa ^2+A_1 b \sigma \omega .\nonumber \\ {\left\{ \begin{array}{ll} D_{-4}=0, D_{-3}=0,\\ D_{-2}=0, D_{-1}=0, D_{0}=0. \end{array}\right. } \end{aligned}$$
(25)

Solving the system, the resulting outcome is as follows.


Case 1:

$$\begin{aligned} {\left\{ \begin{array}{ll} A_1=2 \kappa ,~\\ b =\frac{1}{4} \left( a ^2+\frac{\omega -\frac{\kappa ^2}{\sigma }}{\kappa ^3}\right) . \end{array}\right. } \end{aligned}$$
(26)

Through the utilization of Eqs. (5), (13), (23), and (26), the solutions for Eq. (22) are as follows:


Cluster i:

$$\begin{aligned} G_{11}(x, y, t)=\frac{- a ^2 \kappa ^3 \sigma +\kappa ^2-\sigma \omega }{\kappa ^2 \sigma \left( a +\sqrt{\frac{\kappa ^2-\sigma \omega }{\kappa ^3 \sigma }} \tanh \left( \frac{1}{2} \sqrt{\frac{\kappa ^2-\sigma \omega }{\kappa ^3 \sigma }} (F-\sigma t+\kappa x+y \omega )\right) \right) }. \end{aligned}$$

Cluster ii:

$$\begin{aligned} G_{12}(x, y, t)=A_o+\frac{- a ^2 \kappa ^3 \sigma +\kappa ^2-\sigma \omega }{ a \kappa ^2 \sigma -\sqrt{\kappa } \sigma \sqrt{\omega -\frac{\kappa ^2}{\sigma }} \tan \left( \frac{\sqrt{\omega -\frac{\kappa ^2}{\sigma }} (F-\sigma t+\kappa x+y \omega )}{2 \kappa ^{3/2}}\right) }. \end{aligned}$$

Cluster iii:

$$\begin{aligned} G_{13}(x, y, t)=A_o+\frac{2 a \kappa }{e^{ a (F-\sigma t+\kappa x+y \omega )}-1}. \end{aligned}$$

Cluster iv:

$$\begin{aligned} G_{14}(x, y, t)=A_o+\frac{4 \kappa \left( a +\frac{2}{F-\sigma t+\kappa x+y \omega }\right) }{ a ^2}. \end{aligned}$$

Cluster v:

$$\begin{aligned} G_{15}(x, y, t)=A_o+\frac{2 \kappa }{F-\sigma t+\kappa x+y \omega }. \end{aligned}$$

4 Graphical representation

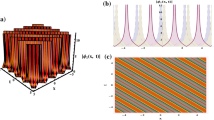
In this section, we present visually appealing representations of the exact solutions for three important equations: the JME, GSWE, and HSIE. These solutions are obtained using the EEM. It is essential to highlight that the soliton solutions’ specific shapes and characteristics may vary depending on the equation’s parameters and nonlinearities. Our results showcase the novelty of our findings, as they have not been previously reported in published studies. To illustrate the wave structures, we employ three-dimensional (3D), two-dimensional (2D), and their associated contour graphs. By adjusting the parameters in the equations, we can generate a diverse range of graphs, each representing different forms of the solution. The figures in our presentation beautifully display these obtained solutions in both 3D and 2D, providing a comprehensive visual understanding of the wave patterns. The contour graphs further enhance the clarity of the solutions, making it easier to grasp their intricate features. It is important to emphasize that the uniqueness of our method lies in the originality of the results, paving the way for new insights into the behavior of these equations. These results contribute to the advancement of research in this field, offering potential applications in various areas of science and engineering. Furthermore, the versatility of our methodology allows us to explore and understand the solutions’ characteristics with precision, providing valuable insights into the underlying dynamics of these important nonlinear equations. Our work stands as a significant contribution to the scientific community, presenting novel and intriguing solutions that were previously unknown and unexplored.

These illustrations offer valuable insights into the intricate characteristics and phenomena exhibited by waves in the presence of nonlinear environments. Figures 1 and 2 shows a special kind of wave called a bright wave solution or single-soliton solution. Unlike regular waves, this one has a strong bump in the middle and lower ripples around it. It happens because of how waves interact in certain situations where things aren’t straight and simple. Figures 3, 4, and 5 showcase a unique wave pattern called a periodic soliton solution or N-soliton solution. These patterns are remarkable because they keep their shape and speed as they move through a medium. These solitons are important in understanding nonlinear systems and have practical uses in various fields. Figures 6 and 7 illustrate a type of wave solution known as peakons. Peakons are distinctive because they consist of sharp peaks or discontinuities, which make them quite different from typical smooth waves. Figure 8 displays a mixed wave pattern consisting of both dark and bright solutions, or double-soliton solution. Dark waves have lower amplitudes, while bright waves have higher ones. This combination arises from complex interactions in nonlinear systems and holds significance in fields like optics. Figures 9, 10, 11, and 12 each illustrate a specific type of wave solution known as a singular solution. Singular solutions are notable for their distinct characteristics, often featuring abrupt changes or pronounced features. Figures 13 and 14 demonstrates a singular periodic solution or singular traveling solution. This type of solution is characterized by its unique periodic pattern combined with singular features. In other words, the wave pattern repeats itself in a periodic manner, but it also contains specific points or regions where it behaves in an exceptional or singular way. Figure 15 features a ’cuspons solution,’ a wave pattern with a sharp peak followed by a rapid decrease in amplitude. These specialized shapes arise from complex interactions in nonlinear systems and have applications in fields like fluid dynamics and optics.

Negative time within figures isn’t a direct reflection of physical time flowing in reverse. Instead, it often symbolizes a mathematical or theoretical tool, enabling researchers to explore complex scenarios. It aids in theoretical investigations by contemplating how physical systems would respond under time reversal. Additionally, negative time extends mathematical solutions beyond observed time frames, accommodating behaviors before initial moments and enhancing analytical insights. It can represent preparatory stages before events, incorporating setups occurring before the observed time span. Negative time also addresses mathematical symmetry or boundary conditions and facilitates the examination of unconventional conditions. Overall, it’s a valuable tool for comprehending intricate wave dynamics and nonlinear effects from diverse viewpoints, revealing hidden relationships and behaviors that might be obscured in positive time frames. In 2021, Serbay Duran et al. made a significant discovery in the field of wave dynamics. They successfully identified hyperbolic and trigonometric wave solutions within the framework of the shallow water wave system (Duran and Kaya 2021). Their achievement was facilitated by employing a modified expansion method as a key tool in their research methodology. In addition to their work on the shallow water wave system, they conducted research involving the Lonngren wave equation for the tunnel diodes (Duran 2021). Using the (1/G’)-expansion method, they investigated this equation and successfully identified hyperbolic-type traveling wave solutions. But what really makes my work stand out is the different solutions I found. Serbay Duran mainly looked at specific types of waves, like hyperbolic and trigonometric waves. But I went further. I checked out different types of solutions, and each had its own special qualities. Some were called cuspons, which are unique wave shapes. Others were peakons that acted in their own special ways. I even found something really interesting called singular soliton solutions, which are like exceptional wave behaviors. Finding all these different solutions helped us understand waves even more. It showed that waves can do many different things, making the whole wave world more complicated and fascinating. So, my work gives us a bigger and clearer view of how waves work and what makes them do what they do.

The solutions captured in the figures exhibit a range of interesting wave patterns. Among them are periodic solutions, which demonstrate repetitive oscillatory behavior (Bainov and Simeonov 2017). Singular solutions, on the other hand, display distinctive features where the wave amplitude becomes unbounded (Cachazo et al. 2020). The figures also contain examples of periodic singular solutions, which combine the characteristics of both periodicity and singularity (Andrade and Wei 2022). In addition to these, we have identified compacton solutions, which represent localized waves that maintain their shape as they travel (Iqbal and Naeem 2022). Bright and dark soliton solutions are also visualized, each exhibiting different types of nonlinear wave behavior (Raza and Arshed 2020). Cuspons, which are wave structures with both a cusp and a peak, are represented as well (Kassem and Rashed 2019). Lastly, the figures include hyperbolic soliton solutions, which possess hyperbolic-shaped waveforms (Rasool et al. 2023). The single-soliton solution, a self-reinforcing solitary wave that maintains its shape while propagating, has profound applications. In optical fibers, the nonlinear Schrödinger Equation’s single-soliton solution ensures efficient, distortion-free data transmission over long distances. Double-soliton solutions, describing interactions between two solitary waves, find utility in particle physics, aiding the understanding of particle interactions in theories like the sine-Gordon equation. N-soliton solutions, which extend interactions to multiple solitons, are significant in fields like plasma physics, aiding the comprehension of soliton collisions and dynamics. Meanwhile, singular traveling solutions, which involve solutions with singularities, have implications in oceanography, contributing insights into rogue wave formation and turbulent flows (Khater and Alabdali 2021). In summary, these soliton-based solutions cater to a wide range of applications, from enhancing communication to unraveling the mysteries of complex physical phenomena. The variety of these depicted solutions highlights the richness and complexity of nonlinear wave dynamics in different media. Our work contributes to a better understanding of these phenomena and provides valuable insights into the physical behavior of waves in nonlinear systems. The clear and detailed visualizations presented in the figures offer a unique and insightful perspective, contributing to the originality of our study, and these findings pave the way for further research and applications in various scientific and engineering domains.

Fig. 1
figure 1

Graphical interpretation of \(u_{1}(x, y, t)\) with different parametric values \(A_o=2,~ b =0.5,~\sigma =1.5,~y=1.9,~\omega =1.8,~F=1.9,~ \text {and} ~ a =0.5\)

Full size image
Fig. 2
figure 2

Graphical interpretation of \(u_{10}(x, y, t)\) with different parametric values \(\alpha =2.25,~\gamma =3.9,~\kappa =3.15,~\beta =0.8,~A_o=0.2,~ b =0,~\sigma =0.4,~y=2.3,~\omega =1.99,~F=3.78,~\text {and}~ a =0\)

Full size image
Fig. 3
figure 3

Graphical interpretation of \(u_{2}(x, y, t)\) with different parametric values \(A_o=2.5,~ b =1.5,~\sigma =2.5,~y=2.9,~\omega =2.8,~F=0.9,~ \text {and} ~ a =1\)

Full size image
Fig. 4
figure 4

Graphical interpretation of \(u_{6}(x, y, t)\) with different parametric values \(\alpha =0.5,~\gamma =0.9,~\kappa =3.5,~\beta =0.8,~A_o=2,~ b =0.5,~\sigma =2.4,~y=4,~\omega =0.99,~F=0.78,~\text {and}~ a =0.5\)

Full size image
Fig. 5
figure 5

Graphical interpretation of \(u_{11}(x, y, t)\) with different parametric values \(\kappa =0.3,~A_o=1.5,~ b =0.5,~\sigma =0.5,~y=3.4,~\omega =3.5,~F=3.9,~\text {and}~ a =0.5\)

Full size image
Fig. 6
figure 6

Graphical interpretation of \(u_{3}(x, y, t)\) with different parametric values \(A_o=2.4,~ b =0,~\sigma =2.6,~y=2.9,~\omega =2.9,~F=3.5,~ \text {and} ~ a =1.4\)

Full size image
Fig. 7
figure 7

Graphical interpretation of \(u_{4}(x, y, t)\) with different parametric values \(A_o=1.5,~ b =0.6,~\sigma =2.4,~y=1.9,~\omega =1.8,~F=0.5,~ \text {and} ~ a =0.8\)

Full size image
Fig. 8
figure 8

Graphical interpretation of \(u_{5}(x, y, t)\) with different parametric values \(A_o=2.5,~ b =0,~\sigma =2.4,~y=2.9,~\omega =2.8,~F=2.5,~ \text {and}~ a =0\)

Full size image
Fig. 9
figure 9

Graphical interpretation of \(u_{8}(x, y, t)\) with different parametric values \(\alpha =1.25,~\gamma =1.9,~\kappa =1.15,~\beta =0.8,~A_o=0.2,~ b =0,~\sigma =0.4,~y=2.3,~\omega =0.99,~F=2.78,~ \text {and}~ a =2.4\)

Full size image
Fig. 10
figure 10

Graphical interpretation of \(u_{9}(x, y, t)\) with different parametric values \(\alpha =2.25,~\gamma =3.9,~\kappa =3.15,~\beta =0.8,~A_o=0.2,~ b =0,~\sigma =0.4,~y=2.3,~\omega =1.99,~F=3.78,~\text {and}~ a =1\)

Full size image
Fig. 11
figure 11

Graphical interpretation of \(u_{14}(x, y, t)\) with different parametric values \(\kappa =3.7,~A_o=1.67,~ b =1,~\sigma =2.4,~y=0.67,~\omega =1.79,~F=1.78,~\text {and}~ a =2\)

Full size image
Fig. 12
figure 12

Graphical interpretation of \(u_{15}(x, y, t)\) with different parametric values \(A_o=0.5,~\sigma =0.44,~y=0.89,~\omega =0.38,~F=0.55,~ \text {and}~\kappa =2.65\)

Full size image
Fig. 13
figure 13

Graphical interpretation of \(u_{7}(x, y, t)\) with different parametric values \(\alpha =1.25,~\gamma =1.9,~\kappa =1.15,~\beta =0.8,~A_o=0.2,~ b =1.5,~\sigma =0.4,~y=2.3,~\omega =0.99,~F=0.78,~\text {and}~ a =1\)

Full size image
Fig. 14
figure 14

Graphical interpretation of \(u_{12}(x, y, t)\) with different parametric values \(\kappa =0.2,~A_o=3.5,~ b =1.5,~\sigma =0.25,~y=1.39,~\omega =3.88,~F=1.79,~ \text {and}~ a =1\)

Full size image
Fig. 15
figure 15

Graphical interpretation of \(u_{13}(x, y, t)\) with different parametric values \(\kappa =2.1,~A_o=3.4,~ b =0,~\sigma =2,~y=3.9,~\omega =3.69,~F=2.75,~\text {and}~ a =1.4\)

Full size image

5 Conclusion

The \(\exp (-\Phi (\eta ))\)-expansion method (EEM) has been effectively employed in this research paper to investigate the aforementioned models. Through the application of this innovative method, the study obtained numerous solutions represented by hyperbolic and exponential functions. In the realm of mathematics, the EEM proves to be a valuable tool for effectively researching NLPDEs. The exact soliton solutions derived from this research hold tremendous significance for researchers and mathematicians, given their practical applications in engineering. Notably, solitons play a crucial role in understanding water waves, rogue waves, and tsunamis. Moreover, in the field of optics, optical solitons manifest as localized intensity peaks or waveforms that can propagate through fibers without spreading out or deforming (Khater et al. 2021). The implications of this research extend beyond mathematics and engineering. Solitary wave models resulting from these findings are instrumental in comprehending and predicting the behavior of immense waves in oceans and coastal areas, thus contributing to the development of effective coastal protection measures and structures.

As a result, these solutions hold relevance across various academic disciplines, particularly in the realm of fluid dynamics. The precision of the study is significantly enhanced through a blend of computational efforts and graphical representations. Notably, the calculated solutions presented in this research surpass those of prior studies, thereby imparting valuable insights to the scientific community without compromising authenticity. The advancements in the JME may unveil novel symmetries, conservation laws, and exact solutions, establishing connections with other systems in both classical and quantum domains. Exploring the equation in higher dimensions will unveil deeper mathematical intricacies and enhanced physical implications. Within fluid dynamics, the GSWE holds promise for refining predictive models involving friction, viscosity, and non-uniform topography, thereby benefiting hazard management for phenomena such as tsunamis, storm surges, and coastal erosion. Further investigations into the HSIE have the potential to catalyze innovative applications in science and engineering. Pursuing these research directions will fuel future technological breakthroughs and enrich our comprehension of the natural world.

Our research endeavors have led to unique discoveries that not only offer a diverse range of solutions but also unveil novel aspects of wave behavior previously unknown. Our study stands apart from existing research, presenting fresh perspectives on the intricacies of wave dynamics. Through thoughtful adjustments in our calculations, we have brought to light untold narratives of waves, showcasing distinctive behaviors like periodic soliton solutions and singular phenomena. These revelations can be likened to new pieces of a puzzle, contributing to a deeper comprehension of how waves manifest under varied conditions. The significance of our work lies in its innovative nature. Our research is not solely about providing answers; it is a journey of posing new questions that evoke curiosity and excitement for further exploration. Reflecting on our research journey, we recognize that the equations governing waves offer a multitude of possibilities. The discoveries we have made extend beyond the boundaries of our immediate field, potentially influencing diverse areas of study. Each newfound concept is like a wave, carrying with it fresh insights into the workings of these phenomena. As we embark on this new chapter of exploration, we remain poised to delve deeper. Moving forward, the solutions we’ve uncovered will serve as guiding beacons, motivating us to delve into the realms of knowledge that lie ahead.

In terms of future directions, this study lays the foundation for several potential avenues of research. First and foremost, the exploration of soliton solutions can be extended to encompass multi-dimensional systems, offering a deeper understanding of their behavior across different dimensions. Additionally, investigating the interactions between distinct soliton solutions, either within the same equation or in different equations, could provide insights into their complex dynamics. To ensure the practical applicability of these solutions, a thorough stability analysis should be conducted, assessing their robustness under various perturbations and conditions. Collaborations with experts from fields such as oceanography, optics, and communication systems could uncover novel applications and guide the integration of these solutions into real-world technologies. Moreover, considering a broader range of nonlinear equations and assessing the generalizability of the discovered soliton solutions would contribute to a more comprehensive understanding of their significance. Utilizing numerical simulations and experimental setups could offer additional validation and insights into the behavior of these solutions. Finally, exploring how these solutions can be integrated into emerging mathematical frameworks or theories could yield new mathematical insights and connections. Pursuing these future research avenues promises to further enhance our understanding of soliton solutions and their potential applications, driving advancements in the field of nonlinear wave dynamics.