1 Introduction

During the latest decades, integrable systems have received intensive researches with many integrable models, such as the Korteweg–de Vries (KdV) equation, the nonlocal modified Korteweg–de Vries (mKdV) equation, nonlinear derivative Schördinger equation, Kadomtsev–Petviashvili equation, Camassa–Holm equation, nonlocal sine-Gordon equation, the fifth-order integrable equation with time dispersion and other integrable systems as well [1]. These newly discovered integrable models have a variety of applications in many phenomena such as pulses propagation in optical communications, plasma physics, wave propagations, fluid mechanics, condensed matter, electro-magnetics and many more. Several theoretical approaches have been applied to describe the physics of solitons for integrable models. Exact solutions of nonlinear models and integrable systems have become hot issues, and many promising findings have potential applications in various branches of physics and engineering [2,3,4,5,6].

In Refs. [7, 8], Wazwaz firstly proposed a new fifth-order nonlinear integrable equation, which reads

$$\begin{aligned} u_{ttt}-u_{txxxx}-4(u_xu_t)_{xx}-4(u_xu_{xt})_x=0, \end{aligned}$$
(1)

or equivalently,

$$\begin{aligned} u_{ttt}-u_{txxxx}-12u_{xt}u_{xx}-8u_{x}u_{xxt}-4u_tu_{xxx}=0, \end{aligned}$$
(2)

which is the first type of integrable equations that involves the third-order time-dispersion term \(u_{ttt}\). Note that its three-soliton solution has been reported in Ref. [7], where the dispersion relations \(c_i\) and the phase shifts \(a_{ij}\) are given as

$$\begin{aligned} c_i= & {} \pm k_i^2,\,\,\,i=1,2,3,\nonumber \\&a_{ij}\,=\,\dfrac{(k_i-k_j)^2}{k_i^2+k_j^2},\,\,\,\,1\le i<j \le 3. \end{aligned}$$
(3)

The fifth-order equation (1) with temporal dispersion has potentials applications and attracted much attention of scholars, and its various exact solutions have been constructed by using the Painlevé analysis, Lie symmetry analysis and several direct algebraic methods [7,8,9,10].

Like the well-known KdV and mKdV equations, most nonlinear integrable models have first-order partial derivative term with respect to time variable t; in other words, these equations always include one term \(u_t\). However, other kind of nonlinear integrable models with a second-order partial derivative term \(u_{tt}\), such as the Klein–Gordon equation, Boussinesq equation and bidirectional Kaup–Kupershmidt equation, always admits bidirectional soliton solutions and can be used to simulate shallow water waves moving in both directions.

Generally speaking, a first-order partial derivative term \(u_t\) was included in nonlinear models for unidirectional optical pulses and temporal propagation. Unlike the competing spatially nonlinear models, temporal propagation may be beneficial in various physical contexts due to its preservations of causality [11,12,13]. Delayed systems have been widely used to describe optical systems, map lattices or communication networks, where dispersion effects may appear naturally.

In Ref. [14], the third-order time dispersion for delayed systems was investigated by studying the model

$$\begin{aligned} u_x=u\ln \eta -2 u_t-\frac{2}{3}u_{ttt}, \end{aligned}$$
(4)

which can be used to analyze the dispersive response quantitatively and attributes to understand the significant importance of third-order dispersion effects, where \(\eta \) represents the reflectivity. One of the most striking findings, as pointed out by authors in [14], is that their exact analytical results are highly consistent with some experimental observations. The third-order dispersion may lead to the creation of satellites on one edge of the pulse which induces a new form of pulse instability.

Based on the previous work in Ref. [15], we study a generalized fifth-order equation with temporal and spatial dispersion

$$\begin{aligned}&u_{ttt}\,-\,\lambda _1\,u_{txxxx}\,-\,\lambda _2\,u_{xxt}\,-\,\lambda _3\,(u_{x}u_t)_{xx}\nonumber \\&-\lambda _4\,(u_{x}u_{xt})_x\,=\,0. \end{aligned}$$
(5)

where \(\lambda _i\)\((i=1,2,3,4)\) are real nonzero parameters, u is a real differentiable function of the scaled spatial coordinates x, y and temporal coordinate t, and the subscripts denote the partial derivatives. Higher-order nonlinear and dispersive effects have been found to be important in various physical applications [16], and equation (5) can describe the evolution of steeper waves of shorter wavelength better than the well-known third-order KdV equation does. Note that Eq. 1 is a subcase of (5) with \(\lambda _1=1\), \(\lambda _2=0\), \(\lambda _3=\lambda _4=4\).

The rest of this paper is organized as follows: First of all, the integrability test indicates that this generalized fifth-order equation is Painlevé integrable for particular choice of parameters. In Sect. 3, we apply the simplified form of Hirota bilinear method to derive the multiple soliton solutions with bidirectional propagating properties. In Sect. 4, we present an improved projective Riccati expansion method to derive new travelling wave solutions. In Sect. 5, several novel periodic soliton interaction solutions are constructed via a direct method. Finally, Sect. 6 formulates the conclusions.

2 Integrability test

The Painlevé analysis method provides an efficient tool to investigate nonlinear models [17], which can verify whether a given equation is integrable or not. Furthermore, other integrable properties can also be obtained as by-products, such as the bilinear equations, bilinear Bäcklund transformation, Darboux transformation, Lax pairs as well as various types of exact solutions [18,19,20,21].

According to the standard WTC method, equation (5) is Painlevé integrable if it has the solution

$$\begin{aligned} u(x,t)\,=\,\sum \limits _{k=0}^{\infty }\,u_k\,f^{k+\alpha } \end{aligned}$$
(6)

with four arbitrary functions among \(u_k\) in addition to the singular manifold f(x, t). Furthermore, the leading exponent \(\alpha \) should be a negative integer.

The first step of the integrability test is the leading order analysis. It is easily seen that the values of \(u_0\) and \(\alpha \) only depend on the first term in (6). Thus, one may consider the ansatz \(u \sim u_0 f^{\alpha }\). Substituting the ansatz into (5) and balancing the most dominant terms lead to \(\alpha =-1\) and \(u_0=12\lambda _1 f_x/(2\lambda _3+\lambda _4)\).

Next, one can find the resonant points by substituting the truncated expansion \(u=u_0\,f^{-1}+u_k\,f^{k-1}\) into (5). The five resonances are found to lie in the positions \(k=-1\), 1, 4, 5 and 6.

For Eq. (5), one should further verify the compatibility conditions at all nonnegative resonant points \(k=1\), 4, 5 and 6. The maximum value of resonant points is 6; thus, the series (6) is truncated as

$$\begin{aligned} u(x,t)\,=\,\sum \limits _{k=0}^{6}\,u_k\,f^{k-1}. \end{aligned}$$
(7)

To simplify the calculations, we adopt the Kruskal’s ansatz for the singular manifold, \(f=x+\psi (t)\), with \(\psi \) being arbitrary function of t. Inserting (7) into (5) and gathering the coefficients of f with the same degree, we have

$$\begin{aligned} u_2= & {} \dfrac{(\psi ^{\prime })^3\,+\,\lambda _3\,u_1^{\prime }\,-\,\lambda _2\psi ^{\prime }}{(2\lambda _3+\lambda _4)\,\psi ^{\prime }},\\ u_3= & {} [(8\lambda _3+\lambda _4)\psi ^{\prime \prime }(\psi ^{\prime })^3+\lambda _3(\lambda _4-\lambda _3)\psi ^{\prime \prime } u_1^{\prime }\\&+\,\lambda _3(\lambda _3-\lambda _4)\psi ^{\prime }u_1^{\prime \prime }]/[2(2\lambda _3+\lambda _4)^2]. \end{aligned}$$

Together the values of \(u_0\), \(u_2\) and \(u_3\), the compatibility condition at \(k=4\) is calculated as

$$\begin{aligned}&\lambda _1\lambda _3\,(\lambda _3-\lambda _4)\,[4\psi ^{\prime \prime \prime }(\psi ^{\prime })^4 +\lambda _4\psi ^{\prime \prime \prime }\psi ^{\prime }u_1^{\prime }\nonumber \\&\quad -\,\lambda _4(\psi ^{\prime })^2u_1^{\prime \prime \prime } +3\lambda _4\psi ^{\prime }\psi ^{\prime \prime }u_1^{\prime \prime } -3\lambda _4(\psi ^{\prime \prime })^2u_1^{\prime }]=0.\nonumber \\ \end{aligned}$$
(8)

Since \(\lambda _1 \ne 0\), it follows from (8) that the compatibility condition at \(k=4\) holds for the following two cases:

Case A:  \(\lambda _3=0\).

In this case, substituting the truncated expansion (7) into (5), we have

$$\begin{aligned} u_0\,=\,\dfrac{12\lambda _1}{\lambda _4},u_2\,=\,\dfrac{(\psi ^{\prime })^2-\lambda _2}{\lambda _4},u_3\,=\,\dfrac{\psi ^{\prime \prime }}{2\lambda _4}, \end{aligned}$$

and the coefficients \(u_1\), \(u_4\), \(u_5\) in (7) are arbitrary functions of t. However, the compatibility condition at \(k=6\) is obtained as

$$\begin{aligned}&24\lambda _1\lambda _4u_5^{\prime }\,-\,10\psi ^{\prime }(\psi ^{\prime \prime })^2 \,-\,5(\psi ^{\prime })^2\psi ^{\prime \prime \prime }\nonumber \\&\quad \,+\,\lambda _4u_1^{\prime \prime \prime }\,+\,\lambda _2 \psi ^{\prime \prime \prime }\,=\,0.\nonumber \\ \end{aligned}$$
(9)

Due to the arbitrariness of \(\psi \), \(u_1\) and \(u_5\), the condition (9) is not satisfied identically. Thus, (5) fails the integrability test in this case.

Case B:  \(\lambda _4=\lambda _3\).

In a similar way, one can get the coefficients of (7) as follows:

$$\begin{aligned} u_0\,=\,\dfrac{4\lambda _1}{\lambda _3},u_2\,=\,\dfrac{(\psi ^{\prime })^3+\lambda _3 u_1^{\prime }-\lambda _2\psi ^{\prime }}{3\lambda _3\psi ^{\prime }},u_3\,=\,\dfrac{\psi ^{\prime \prime }}{2\lambda _3},\\ \end{aligned}$$

and the coefficients \(u_1\), \(u_4\), \(u_5\), \(u_6\) are arbitrary functions, which implies that all resonant conditions are satisfied identically; thus, it is concluded that equation (5) passes the integrability test and it has integrable in sense of Painlevé.

From the above analysis, we derive an integrable fifth-order equation with temporal and spatial dispersion:

$$\begin{aligned}&u_{ttt}\,-\,\lambda _1\,u_{txxxx}\,-\,\lambda _2\,u_{xxt}\,-\,\lambda _3\,(u_{x}u_t)_{xx}\nonumber \\&\quad -\lambda _3\,(u_{x}u_{xt})_x\,=\,0. \end{aligned}$$
(10)

Note that Eq. (1) is a subcase of (10) if taking \(\lambda _1=1\), \(\lambda _2=0\) and \(\lambda _3=4\).

3 Bidirectional soliton solutions

The integrability of Eq. (10) predicts that it can be solvable by several classical methods. The Hirota bilinear method is one of the most efficient methods to seek for various exact solutions of nonlinear models [22,23,24,25], such as multiple soliton solutions, quasi-periodic solutions, rogue wave solutions, decay mode solutions and rational solutions of various types.

The simplified Hirota method proposed by Hereman and Nuseir [26] has some advantages, which can not only avoid the problem of “intermediate expression swell,” but also does not need to transform nonlinear equations into bilinear representation [27, 28]. Bidirectional solitons can simulate ocean waves phenomenon well and have potential applications in various branches of physics [29,30,31,32,33,34].

Here, we apply the simplified Hirota method to derive bidirectional soliton solutions of equation (10). Without loss of generality, we set \(\lambda _1=\lambda _2=1\) and \(\lambda _3=4\) in the following sections. The Painlevé–Bäcklund transformation of Eq. (10) reads

$$\begin{aligned} u(x,t)\,=\,(\ln f(x,t))_x\,+\,w_1, \end{aligned}$$
(11)

with \(w_1\) being the seed solution of (10), and we may take \(w_1=0\). Substituting (11) into (10), then integrating it once with respect to x and taking the arbitrary function of integration as zero, we have

$$\begin{aligned}&f^2(f_{ttt} -f_{xxt}-f_{xxxxt})+ f(4f_xf_{xxxt}-3f_tf_{tt}\nonumber \\&\quad +\,2 f_{xt}f_{x}- 2f_{xx}f_{xxt} + f_{xx}f_t +f_{xxxx}f_t)\nonumber \\&\quad -\,2\,(2f_x^2 f_{xxt}-2f_{x}f_{xt}f_{xx}+2f_{x}f_{t}f_{xxx}\nonumber \\&\quad +f_{x}^2f_t -f_{xx}^2f_{t}-f_t^3)\,=\,0. \end{aligned}$$
(12)

Following the “step-by-step” principle [24], equation (12) can be rewritten as

$$\begin{aligned} f^2 L \cdot f + f N_1 (f,f) + N_2(f,f,f)\,=\,0, \end{aligned}$$
(13)

where linear differential operator L, and nonlinear differential operators \(N_1\) and \(N_2\) are defined by

$$\begin{aligned} L \cdot= & {} \dfrac{\partial ^3 \cdot }{\partial t^3} - \dfrac{\partial ^3 \cdot }{\partial x^2\partial t}- \dfrac{\partial ^5 \cdot }{\partial x^4\partial t},\\N_1(v,w)\,=\,&4v_xw_{xxxt}-3v_t w_{tt}+2v_{xt}w_{x}-2v_{xx}w_{xxt}\\&\quad +\,v_{xx}w_t + v_{xxxx}w_t,\\N_2(v,w,g)\,=\,&-4v_xw_xg_{xxt}-2v_xw_xg_t +4v_x w_{xt}g_{xx}\\&\quad -\,4v_xw_tg_{xxx}+2v_{xx}w_{xx}g_{t}+2 v_t w_tg_t,\\ \end{aligned}$$

with v, w, g being auxiliary functions.

The quasi-solution f of (13) may be supposed as

$$\begin{aligned} f\,=\,1 + \delta f_1 + \delta ^2 f_2 + \cdots + \delta ^r f_r + \cdots ,\,\,r\,\in \mathbb {N}, \end{aligned}$$
(14)

with \(f_r\) being unknown functions, and \(\delta \) serves as a book-keeping parameter. Inserting (14) into equation (13) and comparing the coefficient of power of \(\delta \), we have the recursion formulae for \(f_r\) as follows:

$$\begin{aligned} L \cdot f_1= & {} 0,\nonumber \\ L \cdot f_2= & {} - N_1(f_1,f_1),\nonumber \\ L \cdot f_3= & {} - (2 f_1 L\cdot f_2 \,+\,f_1 N_1(f_1,f_1)\nonumber \\&\quad +\,N_1(f_2,f_1)+N_1(f_1,f_2)+N_2(f_1,f_1,f_1)), \cdots \, \end{aligned}$$
(15)

Solving these recursion equations, one can construct the multiple soliton solutions of Eq. (10).

3.1 One-soliton solution

In the expression (14), we may suppose

$$\begin{aligned} f_1\,=\,\mathrm{e}^{\theta },\,\,\,\,\,\theta \,=\,k_1\,x\,+c_1\,t, \end{aligned}$$
(16)

where \(k_1, c_1\) are constants to be determined. Substituting (16) into the first equation of (15) leads to

$$\begin{aligned} c_1\,=\,\pm k_1\,\sqrt{1\,+\,k_1^2},\,\,\mathrm{or}\,\,c_1\,=\,0. \end{aligned}$$
(17)

Note that the case with \(c_1=0\) can be associated with a steady waves regime. In this work, we only focus on the case with nonzero wave speed. For the case with \(c_1=\pm k_1\sqrt{1\,+\,k_1^2}\), the second equation of (15) becomes

$$\begin{aligned} L \cdot f_2\,=\,0. \end{aligned}$$
(18)

Together with the boundary condition

$$\begin{aligned} \lim \limits _{|x|\rightarrow +\infty }\,f_2(x,t)\,=\,0, \end{aligned}$$

it follows from (18) that \(f_2=0\).

Making use of (15)–(17), it is found that the series (14) can be truncated at \(r=2\). Through the transformation (11), the one-soliton solution is obtained as

$$\begin{aligned} u_1=\dfrac{\delta k_1 \mathrm{e}^{k_1 x + \epsilon \,k_1 \sqrt{1+k_1^2} t}}{1\,+\,\delta \,\mathrm{e}^{k_1 x + \epsilon \,k_1 \sqrt{1+k_1^2} t}}, \end{aligned}$$
(19)

where \(\epsilon =\pm 1\), and \(k_1\) and \(\delta \) are arbitrary constants. The solution (19) is just the same as the result reported in Ref. [15].

3.2 Two-soliton solution

To construct the two-soliton solutions, we may suppose

$$\begin{aligned} f_1\,=\,\mathrm{e}^{\theta _1}\,+\,\mathrm{e}^{\theta _2},\,\,\,\,\theta _j\,=\,k_j\,x\,+c_j\,t,\,\,j=1,2, \end{aligned}$$
(20)

where \(k_j, c_j(j=1,2)\) are constants to be determined. Inserting (20) into the first equation of (15) yields two different solutions:

$$\begin{aligned} \mathrm{(i)}\,\,c_1\,=\,\epsilon k_1 \sqrt{1+k_1^2},c_2\,=\,\epsilon k_2 \sqrt{1+k_2^2}, \end{aligned}$$
(21)
$$\begin{aligned} \mathrm{(ii)}\,\, c_1\,=\,\epsilon k_1 \sqrt{1+k_1^2},c_2\,=\,-\epsilon k_2 \sqrt{1+k_2^2}, \end{aligned}$$
(22)

where \(\epsilon =\pm 1\).

For the first dispersion relation (21), substituting (20) with (21) into the second equation of (15) yields

$$\begin{aligned} L \cdot f_2= & {} -2k_1k_2(k_1-k_2)[\sqrt{1+k_1^2}(1+k_1k_2\\&+2k_1^2+k_2^2)-\,\sqrt{1+k_2^2}(1+k_1k_2+k_1^2+2k_2^2)]\mathrm{e}^{\theta _1+\theta _2},\\ \end{aligned}$$

from which we get

$$\begin{aligned} f_2\,=\,a_{12}\,\mathrm{e}^{\theta _1+\theta _2}, \end{aligned}$$
(23)

where

$$\begin{aligned} a_{12}= & {} \Big [\sqrt{1+k_1^2}(1+2k_1^2+k_1k_2+k_2^2)(k_1-k_2)\nonumber \\&\quad -\,\sqrt{1+k_2^2}(1+k_1^2+k_1k_2+2k_2^2)(k_1-k_2)\Big ]\nonumber \\&\qquad /[\sqrt{1+k_1^2}(2k_1^3+3k_1^2k_2+2k_1k_2^2-k_2^3\nonumber \\&\quad +\,k_1-k_2)-\sqrt{1+k_2^2}\,(-2k_2^3-3k_1k_2^2\nonumber \\&\quad -\,2k_1^2k_2+k_1^3+k_1-k_2)\,]. \end{aligned}$$
(24)

Together with (20), (21) and (23), it is found that \(f_r =0\) when \(r \ge 3\). Therefore, the series (14) is truncated at \(r=3\). Through the transformation (11), we obtain two-soliton solution with the form

$$\begin{aligned} u_2= & {} (\ln f)_x,\nonumber \\ f= & {} 1 + \delta \,\mathrm{e}^{k_1 x +\epsilon \, k_1\sqrt{1+k_1^2} t} + \delta \,\mathrm{e}^{k_2 x + \epsilon \,k_2 \sqrt{1+k_2^2} t}\nonumber \\&\quad +\, \delta ^2 a_{12} \mathrm{e}^{(k_1+k_2) x + \epsilon \,(k_1\sqrt{1+k_1^2}+k_2\sqrt{1+k_2^2}) t}, \end{aligned}$$
(25)

where \(\epsilon =\pm 1\), \(a_{12}\) is given by (24), and \(k_1, k_2\) and \(\delta \) are arbitrary constants.

Next, we consider the second dispersion relation (22). Utilizing the procedure as before, from (11) and (20), we obtain another two-soliton solution:

$$\begin{aligned} u_3= & {} (\ln f)_x,\nonumber \\ f= & {} 1+ \delta \mathrm{e}^{k_1 x+\epsilon \,k_1\sqrt{1+k_1^2} t}+ \delta \mathrm{e}^{k_2 x-\epsilon k_2\sqrt{1+k_2^2} t}\nonumber \\&+ \delta ^2 a_{12} \mathrm{e}^{(k_1+k_2) x + \epsilon \,(k_1\sqrt{1+k_1^2}- k_2\sqrt{1+k_2^2}) t}, \end{aligned}$$
(26)

where \(k_1, k_2, \delta \) are arbitrary constants, \(\epsilon =\pm 1\), and \(a_{12}\) is given by

$$\begin{aligned} a_{12}= & {} [\sqrt{1+k_1^2}(1+2k_1^2+k_1k_2+k_2^2)(k_1-k_2)\nonumber \\&+\,\sqrt{1+k_2^2}(1+k_1^2+k_1k_2+2k_2^2)(k_1-k_2)]\nonumber \\&\quad /[\sqrt{1+k_1^2}(2k_1^3+3k_1^2k_2+2k_1k_2^2-k_2^3\nonumber \\&+\,k_1-k_2)+\sqrt{1+k_2^2}\,(-2k_2^3-3k_1k_2^2\nonumber \\&-\,2k_1^2k_2+k_1^3+k_1-k_2)\,]. \end{aligned}$$
(27)

To illustrate the collisions between two solitons more clearly, we analyze the potentials of \(u_2\) and \(u_3\), namely \(u_{2,x}\) and \(u_{3,x}\). In Fig. 1a, two left-running solitons undergo an “elastic collision,” and the tall one moves faster and overtakes the small one, and each soliton still remains its shape, velocity and amplitude after interactions. Figure 1b depicts another overtaking collisions between two right-running solitons. Figure 1c describes the head-on collision between two solitons. The tall one is right going, and the small one is left going. After interactions, they will come back to their original profile and move in the opposite x-direction.

Fig. 1
figure 1

(Color online) The plots of two-soliton solution. a The overtaking collision of two left-going solitons given by (25) with \(k_1=1.0\), \(k_2=1.5\) and \(\delta =\epsilon =1\). b The overtaking collision of two right-going solitons given by (25) with \(k_1=1.0\), \(k_2=1.5\)\(\delta =-\epsilon =1\). c The head-on collision of two solitons given by (26) with \(k_1=1.4\), \(k_2=0.9\) and \(\delta =-\epsilon =1\)

Full size image

3.3 Three-soliton solution

Non-integrable systems may possess one-soliton and two-soliton solutions at most but not higher multi-solitons. According to the conjecture on integrability, as pointed out by Hietarinta [23], nonlinear evolution equations that arise from various branches of physics are integrable if they admit three-soliton solutions. Repeating the similar calculations as in Sect. 3.2, we obtain two types of three-soliton solutions. For the sake of conciseness, the detailed computation is omitted here.

Case A

$$\begin{aligned} U= & {} u_{4,x}=(\ln f)_{xx},\nonumber \\ f= & {} 1+\delta (\mathrm{e}^{\theta _1}+\mathrm{e}^{\theta _2}+\mathrm{e}^{\theta _3})+\delta ^2(a_{12}\mathrm{e}^{\theta _1+\theta _2} +a_{13}\mathrm{e}^{\theta _1+\theta _3})\nonumber \\&\quad + \delta ^2 a_{23}\mathrm{e}^{\theta _2+\theta _3} + \delta ^3 a_{12}a_{13}a_{23}\mathrm{e}^{\theta _1+\theta _2+\theta _3},\nonumber \\&\quad \theta _i\,=\,k_i x+\epsilon k_i\sqrt{1+k_i^2} t,\,\,i=1,2,3, \end{aligned}$$
(28)

where \(k_1, k_2, k_3\) and \(\delta \) are arbitrary constants, \(\epsilon =\pm 1\). The phase shifts \(a_{12}\), \(a_{13}\) and \(a_{23}\) are given by

$$\begin{aligned} a_{ij}= & {} [\sqrt{1+k_i^2}(1+2k_i^2+k_ik_j+k_j^2)(k_i-k_j)\nonumber \\&\quad \,-\,\sqrt{1+k_j^2}(1+k_i^2+k_ik_j+2k_j^2)(k_i-k_j)]\nonumber \\&\qquad /[\sqrt{1+k_i^2}(2k_i^3+3k_i^2k_j+2k_ik_j^2-k_j^3\nonumber \\&\quad +k_i-k_j)-\,\sqrt{1+k_j^2}\,(k_i^3 + k_i - k_j\nonumber \\&\quad -2k_j^3-3k_ik_j^2 -2k_i^2k_j)\,],\,\, 1 \le i<j\le 3. \end{aligned}$$
(29)

Figure 2 describes the overtaking collisions between three right-going solitons given by (28). The largest-amplitude soliton moves fastest and overtakes another two solitons (Fig. 2b–e). As can be seen in Fig. 2c, the soliton with the smallest amplitude has been swallowed and these three solitons evolve into two-hump bright soliton. As shown in Fig. 2f, three solitons come back to their original wave shapes and velocities after interactions and move in same x-axis. There is no energy exchange between three solitons after the collision, which means that the collision is completely elastic. Additionally, if choosing appropriate parameter values for \(k_1\), \(k_2\) and \(k_3\) and \(\epsilon =1\), one can obtain another type of overtaking collision between three left-going solitons.

Fig. 2
figure 2

Color online) Overtaking collision of three right-going solitons given by (28) with \(k_1=1.1\), \(k_2=1.6\), \(k_3=2.0\), \(\delta =-\epsilon =1\). a\(t=-18\), b \(t=-13.5\), c \(t=-1\), d \(t=4\), e \(t=12\), f the evolution plot of three solitons

Full size image

Case B

$$\begin{aligned} U= & {} u_{5,x}\,=\,(\ln f)_{xx},\nonumber \\ f= & {} 1+\delta (\mathrm{e}^{\theta _1}+\mathrm{e}^{\theta _2}+\mathrm{e}^{\theta _3})+ \delta ^2(a_{12}\mathrm{e}^{\theta _1+\theta _2} +a_{13}\mathrm{e}^{\theta _1+\theta _3})\nonumber \\&+ \,\delta ^2 a_{23}\mathrm{e}^{\theta _2+\theta _3} +\delta ^3 a_{12}a_{13}a_{23}\mathrm{e}^{\theta _1+\theta _2+\theta _3},\nonumber \\&\theta _i\,=\,k_i x+\epsilon k_i\sqrt{1+k_i^2} t,\,\,i=1,2,\nonumber \\&\theta _3\,=\,k_3 x-\epsilon k_3\sqrt{1+k_3^2} t, \end{aligned}$$
(30)

where \(k_1, k_2, k_3, \delta \) are arbitrary constants, \(\epsilon =\pm 1\), \(a_{12}\) is given by (24), and the phase shifts \(a_{13}\) and \(a_{23}\) are given by

$$\begin{aligned} a_{i3}&= [\sqrt{1+k_i^2}(1+2k_i^2+k_ik_3+k_3^2)(k_i-k_3)\nonumber \\&+\,\sqrt{1+k_3^2}(1+k_i^2+k_ik_3+2k_3^2)(k_i-k_3)]\nonumber \\& /[\sqrt{1+k_i^2}(2k_i^3+3k_i^2k_3+2k_ik_3^2-k_3^3\nonumber \\&+\,k_i-k_3)\,+\,\sqrt{1+k_2^2}\,(k_i^3 + k_i - k_3\nonumber \\&-\,2k_3^3-3k_ik_3^2-2k_i^2k_3 )\,],\,i=1,2. \end{aligned}$$
(31)

Figure 3 depicts the head-on collision between three solitons given by (30). As shown in Fig. 3, the largest amplitude soliton is right going and the other two are left going. Note that there also exists overtaking collision between two left-going solitons, and the soliton with larger amplitude moves faster than the smaller one (Fig. 3a–e). These three solitons still remain their original wave shapes and velocities after head-on interactions.

Fig. 3
figure 3

Color online) Head-on collision of three solitons given by (30) with \(k_1=0.9\), \(k_2=1.2\), \(k_3=1.4\), \(\delta =\epsilon =1\). a \(t=-10\), b \(t=-3\), c \(t=0\), d \(t=2.5\), e \(t=10\), f the evolution plot of three solitons

Full size image

Equation (10) seems to be slightly different from Eq. (1), where we only added one more third-order dispersion term. The soliton structure of Eq. 1 is very simple, and its phase shift is given by Eq. (3), while the soliton structure of equation (10) is more complicated, and the phase shift is rather tedious. The bidirectional two-soliton solutions and three-soliton solutions (25), (26), (28) and (30) are firstly reported here. In Ref. [15], the values of the phase shift were calculated with proper selections of parameters, and its explicit expression has not been presented.

In a similar manner, one can obtain four- and five-soliton solutions of (10). The interactions between N solitons (\(N \ge 4\)) are more abundant than those of three solitons. Bidirectional solitons are physical meaningful for water waves and the evolvement of waves group caused by topographical changes. A variety of nonlinear models in \(2+1\) and \(3+1\) dimensions admit bidirectional soliton solutions, but they are rare in \(1+1\) dimensions.

4 Abundant travelling wave solutions

The travelling wave solutions for nonlinear models play an important role to understand complex nonlinear phenomena. For example, the wave phenomena observed in fluid dynamics, plasma and elastic media are often modelled by the bell-shaped sech solutions and the kink-shaped tanh solutions. Up to now, a number of methods have been established and developed by many scholars, such as the tanh-function method [35], the sine–cosine method [36], the unified algebraic method [37], the Jacobian elliptic function expansion method [38, 39] and the \(\frac{G^{\prime }}{G}\)-expansion method [40].

In this section, we present an extended projective Riccati expansion method for constructing novel travelling wave solutions. The key idea of this method is to make use of the close relations between the special functions and the coupled Riccati system. Our main contribution is to give two types of exact solutions of a coupled Riccati system involving several arbitrary parameters, which are more general than the results given in Refs. [41,42,43,44]. Abundant travelling wave solutions of (10) are derived in a systematic way.

4.1 Analysis of the coupled Riccati equation

We consider the coupled Riccati system:

$$\begin{aligned} f^{\prime }= & {} p\,f(\xi )\,g(\xi ),\nonumber \\ g^{\prime }= & {} q\,+\,p\,g^2(\xi )\,-\,r\,f(\xi ), \end{aligned}$$
(32)

where p and q are nonzero constants, and r is an arbitrary constant.

Through the transformation

$$\begin{aligned} f\,=\,\dfrac{1}{w},g\,=\,-\dfrac{w^{\prime }}{p\,w}, \end{aligned}$$

the system (32) can be reduced to a second-order ordinary differential equation (ODE), which reads

$$\begin{aligned} w^{\prime \prime }\,+\,p\,q\,w\,-\,pr\,=\,0. \end{aligned}$$
(33)

With the aid of symbolic computation, we derive two new types of explicit solutions to Eq. (32). If \(pq<0\), it admits the combined solitary wave solution

$$\begin{aligned} f= & {} \dfrac{q}{a_1\,q\,\sinh (\sqrt{-pq}\xi )+a_2\,q\,\cosh (\sqrt{-pq}\xi )+r},\nonumber \\ g= & {} -\dfrac{q\,\sqrt{-pq}\,[a_2\sinh (\sqrt{-pq}\xi )+a_1\cosh (\sqrt{-pq}\xi )]}{p\,[a_1q\sinh (\sqrt{-pq}\xi )+a_2q\cosh (\sqrt{-pq}\xi )+r]}, \end{aligned}$$
(34)

where \(a_1\), \(a_2\) are two arbitrary constants, and the relation between f and g is obtained as

$$\begin{aligned} g^2\,=\,-\dfrac{q}{p}\,+\,\dfrac{2r}{p}\,f\,-\,\dfrac{a_1^2q^2-a_2^2q^2+r^2}{pq}\,f^2. \end{aligned}$$
(35)

If \(pq>0\), Eq. (32) has the combined trigonometric function solutions

$$\begin{aligned} f= & {} \dfrac{q}{a_1\,q\,\sin (\sqrt{pq}\xi )+a_2\,q\,\cos (\sqrt{pq}\xi )+r},\nonumber \\ g= & {} \dfrac{q\,\sqrt{pq}\,[a_2\sin (\sqrt{pq}\xi )-a_1\cos (\sqrt{pq}\xi )]}{p\,[a_1\,q\,\sin (\sqrt{pq}\xi )+a_2\,q\,\cos (\sqrt{pq}\xi )+r]}, \end{aligned}$$
(36)

where \(a_1\), \(a_2\) are two arbitrary constants, and the relation between f and g is given by

$$\begin{aligned} g^2\,=\,-\dfrac{q}{p}\,+\,\dfrac{2r}{p}\,f\,+\,\dfrac{a_1^2q^2+a_2^2q^2-r^2}{pq}\,f^2. \end{aligned}$$
(37)

Remark 1

If \(p=-1\), \(q=1\), equation (32) is reduced to the coupled Riccati system studied in Refs. [41, 42], where the obtained solution is the special case of (34) with \(p=-1\), \(q=1\) and \(a_1=0\).

Remark 2

When \(p=-1\), \(q=1\), \(r=0\), equation (32) is reduced to the coupled Riccati system studied by Yao et al. [43], where the reported solution is another particular case of (34).

Remark 3

Fu et al. also considered the coupled system (32) to construct exact solutions of nonlinear models. The solitary wave solutions and trigonometric function solutions given in [44] are the subcases of (34) and (36) with proper selections of \(a_1\) and \(a_2\).

4.2 The projective Riccati expansion method

For a given nonlinear evolution equation, say, in two variables,

$$\begin{aligned} G(u, u_x, u_t, u_{xx}, u_{xt}, u_{tt}, u_{xxx}, \cdots )\,=\,0, \end{aligned}$$
(38)

where the subscripts denote partial derivatives, and G is a polynomial in unknown function u(x, t) and its derivatives, and the method is consisted of four steps.

Step 1:  Through the transformation

$$\begin{aligned} \xi \,=\,k\,x\,+\,\nu \,t \end{aligned}$$
(39)

with k and \(\nu \) being the wave number and wave speed, equation (38) is changed into an ordinary differential equation

$$\begin{aligned} H(u, u^{\prime }, u^{\prime \prime }, u^{\prime \prime \prime }, \cdots )\,=\,0, \end{aligned}$$
(40)

Step 2:  The solutions of (40) can be expressed as

$$\begin{aligned} u\,=\,\sum \limits _{i=0}^{m}\,A_i\,f^i\,+\,\sum \limits _{j=1}^{m}\,B_j\,f^{j-1}\,g, \end{aligned}$$
(41)

where f and g satisfy the coupled Riccati system (32). In (41), the value of integer m can be determined by balancing the nonlinear term and the linear derivative term with the highest order, and the coefficients \(A_i\) and \(B_j\) are undetermined constants.

Step 3:  We first insert the ansatz (41) into (40). Next, the derivatives of f and g in the obtained ordinary differential equation can be expressed as the polynomials of f ad g by employing (32). Subsequently, any power of g higher than one can be eliminated via the relation (35) or (37). And finally setting the coefficients of the terms with the same power of f and g to zero, we obtain a nonlinear algebraic system (NAS) with respect to the unknown parameters k, \(\nu \), p, q, r, \(a_1\), \(a_2\), \(A_i(i=0,\cdots ,m)\), \(B_j(j=1,\cdots ,m)\).

Step 4:  Putting each solution of the NAS into (41) and making use of the solutions (34) and (36), some new solutions of Eq. (38) can be constructed systematically.

4.3 New travelling wave solutions of Eq. (10)

Applying the transformation (39) to (10), we get

$$\begin{aligned} (\nu ^2-k^2)u^{\prime \prime \prime }-k^4u^{\prime \prime \prime \prime \prime } -12k^3(u^{\prime \prime })^2-12k^3u^{\prime }u^{\prime \prime \prime }=0. \end{aligned}$$
(42)

Under the following boundary conditions

$$\begin{aligned} \lim \limits _{|\xi |\rightarrow +\infty }\,u^{\prime }(\xi )= & {} \lim \limits _{|\xi |\rightarrow +\infty }\,u^{\prime \prime }(\xi )\,=\,\lim \limits _{|\xi |\rightarrow +\infty }\,u^{\prime \prime \prime }(\xi )\\= & {} \lim \limits _{|\xi |\rightarrow +\infty }\,u^{\prime \prime \prime \prime }(\xi )\,=0, \end{aligned}$$

integrating (42) with respect to \(\xi \) twice and setting the two integration constants as zero, we have

$$\begin{aligned} (\nu ^2-k^2)\,u^{\prime }\,-\,k^4\,u^{\prime \prime \prime } \,-\,6k^3\,(u^{\prime })^2\,=\,0. \end{aligned}$$
(43)

According to the above method, the solution of (43) can be expressed as

$$\begin{aligned} u\,=\,A_0\,+\,A_1 f\,+\,B_1\,g, \end{aligned}$$
(44)

where \(A_1B_1 \ne 0\), and f and g satisfy (32). In order to derive the solitary wave solutions and periodic wave solutions in terms of trigonometric functions, two different cases should be further investigated.

Case A

Substituting (44) into (43), together with (32) and the relation (35), collecting all the terms with the same power of \(f^{i}(i=1,2,3,4), f^{j}\,g(j=1,2,3)\) and setting the coefficients to zero, we have

$$\begin{aligned}&f^4:\,\,{k}^{3}\,( a_1^2{q}^{2}-a_2^2{q}^{2}+{r}^{2})(a_1^2kp{q}^{2}B_1-a_2^2kp{q}^{2}B_{{1}}\\&\qquad +\,{{a_1^2}}{q}^{2}B_1^{2}-{{a_2^2}}{q}^{2}B_1^{2} + kp{r}^{2}B_{{1}}-pq A_1^{2}+{r}^{2}B_1^{2})\,=\,0,\\&f^3g:\,\,k^3pqA_1(a_1^2q^2-a_2^2q^2+r^2)(kp+2B_1)\,=\,0,\\&f^3:\,{k}^{3}qr\,(a_1^{2}kp{q}^{2}B_1-a_2^{2}kp{q }^{2}B_1+a_1^{2}{q}^{2}B_1^{2}-a_2^{2}{q}^{2}B_1^{2}\\&\qquad +\,kp{r}^{2}B_1-pq A_1^{2}+{r}^{2}B_1^{2})\,=\,0,\\&f^2g:\,\,k^3pq r A_1\,(kp\,+\,2B_1)\,=\,0,\\ \end{aligned}$$
$$\begin{aligned}&f^2:\,\,q\,( 4\,a_1^{2}{k}^{4}p{q}^{3}B_{{1}}-4\,a_2^{2}{k} ^{4}p{q}^{3}B_{{1}}+{\nu }^{2}{r}^{2}B_{{1}}-{k}^{2}{r}^{2}B_{{1}}\\&\qquad -\,6\,{k}^{3}p{q}^{2}A_1^{2}+6\,{k}^{3}q{r}^{2}B_1^{2} -a_1^{2}{k}^{2}{q}^{2}B_{{1}}+7\,{k}^{4}pq{r}^{2}B_{{1}}\\&\qquad +\,a_1^{2}{\nu }^{2}{q}^{2}B_{{1}}+a_2^{2}{k}^{2}{q}^ {2}B_{{1}}-a_2^{2}{\nu }^{2}{q}^{2}B_{{1}})\,=\,0,\\&f g:\,\,p\,q^2\,A_1\,(k^4pq\,-\,k^2\,+\,\nu ^2)\,=\,0,\\&f:\,\,q^2 r B_1\,(k^4pq\,-\,k^2\,+\,\nu ^2)\,=\,0. \end{aligned}$$

Solving the above algebraic system with respect to all parameters, we get three sets of solutions:

$$\begin{aligned} \mathrm{(I)}\,\, A_1= & {} r=0,B_1=-k\,p,\nonumber \\ \nu= & {} \pm k\,\sqrt{1-4\,k^2\,pq}. \end{aligned}$$
(45)
$$\begin{aligned} \mathrm{(II)}\,\, A_1= & {} 0,B_1=-k\,p,\nu =\epsilon _1\,k\,\sqrt{1-k^2\,pq},\nonumber \\ a_2= & {} \epsilon _2\,a_1,\epsilon _1=\pm 1, \epsilon _2=\pm 1. \end{aligned}$$
(46)
$$\begin{aligned} \mathrm{(III)}\,\,A_1= & {} \dfrac{k \epsilon _1}{2}\,\sqrt{-\dfrac{p(a_1^2q^2-a_2^2q^2+r^2)}{q}},\nonumber \\ B_1= & {} -\dfrac{k\,p}{2},\nu =k\epsilon _2\,\sqrt{1-k^2\,pq},\nonumber \\ \epsilon _1= & {} \pm 1, \epsilon _2=\pm 1. \end{aligned}$$
(47)

Combining the above results, we can derive three types of solitary wave solutions for (10).

Type 1 If \(pq<0\), from (34), (44) and (45), we have

$$\begin{aligned} u_6= & {} A_0\,+\,\nonumber \\&\quad \dfrac{k\,\sqrt{-pq}\,[a_2\,\sinh (\sqrt{-pq}\xi )+a_1\,\cosh (\sqrt{-pq}\xi )]}{a_1\,\sinh (\sqrt{-pq}\xi )+a_2\,\cosh (\sqrt{-pq}\xi )},\nonumber \\ \xi= & {} k\,(x \pm \,\sqrt{1-4k^2\,pq}\,t), \end{aligned}$$
(48)

where \(A_0, k, a_1, a_2\) are arbitrary constants.

Type 2 If \(pq<0\), from (34), (44) and (46), we have

$$\begin{aligned} u_7= & {} A_0\,+\,\nonumber \\&\dfrac{ka_1q\sqrt{-pq}\,[\epsilon _2 \sinh (\sqrt{-pq}\xi )+\cosh (\sqrt{-pq}\xi )]}{a_1q\sinh (\sqrt{-pq}\xi )+\epsilon _2 a_1\,q\,\cosh (\sqrt{-pq}\xi )+r},\nonumber \\ \xi= & {} k\,(x+\epsilon _1\,\sqrt{1-k^2\,pq}\,t),\,\epsilon _1=\pm 1, \epsilon _2=\pm 1, \end{aligned}$$
(49)

where \(A_0, k, a_1, r\) are arbitrary constants.


Type 3 If \(pq<0\), from (34), (44) and (47), we have

$$\begin{aligned} u_8= & {} A_0\,\,\nonumber \\&+\,\dfrac{k \epsilon _1\sqrt{-pq(a_1^2q^2-a_2^2q^2+r^2)}}{2[a_1q\sinh (\sqrt{-pq}\xi )+a_2q\cosh (\sqrt{-pq}\xi )+r]}\nonumber \\&+\,\dfrac{k\,q\sqrt{-pq}\,[a_2\sinh (\sqrt{-pq}\xi )+a_1\cosh (\sqrt{-pq}\xi )]}{2[a_1q\sinh (\sqrt{-pq}\xi )+a_2q\cosh (\sqrt{-pq}\xi )+r]},\nonumber \\ \xi= & {} k\,(x+\epsilon _2\,\sqrt{1-k^2\,pq}\,t),\,\epsilon _1=\pm 1, \epsilon _2=\pm 1, \end{aligned}$$
(50)

where \(A_0, k, a_1, a_2, r\) are arbitrary constants.

Case B

Substituting (44) into (43), together with (32) and the relation (37), collecting all the terms with the same power of \(f^{i}(i=1,2,3,4), f^{j}\,g(j=1,2,3)\) and setting the coefficients to zero, we get

$$\begin{aligned}&f^4:\,\,{k}^{3}\,( a_1^2{q}^{2}+a_2^2{q}^{2}-{r}^{2})(a_1^2kp{q}^{2}B_1+a_2^2kp{q}^{2}B_{{1}}\\&\qquad +\,{{a_1^2}}{q}^{2}B_1^{2}+{{a_2^2}}{q}^{2}B_1^{2} -kp{r}^{2}B_{{1}}+pq A_1^{2}-{r}^{2}B_1^{2})\,=\,0,\\& f^3g:\,\,k^3pqA_1(a_1^2q^2+a_2^2q^2-r^2)(kp+2B_1)\,=\,0,\\& f^3:\,{k}^{3}qr\,(a_1^{2}kp{q}^{2}B_1+a_2^{2}kp{q }^{2}B_1+a_1^{2}{q}^{2}B_1^{2}+a_2^{2}{q}^{2}B_1^{2}\\&\qquad -\,kp{r}^{2}B_1+pq A_1^{2}-{r}^{2}B_1^{2})\,=\,0,\\&\quad f^2g:\,\,k^3pq r A_1\,(kp\,+\,2B_1)\,=\,0,\\& f^2:\,\,q\,( 4\,a_1^{2}{k}^{4}p{q}^{3}B_{{1}}+4\,a_2^{2}{k} ^{4}p{q}^{3}B_{{1}}-{\nu }^{2}{r}^{2}B_{{1}}+{k}^{2}{r}^{2}B_{{1}}\\&\qquad +\,6\,{k}^{3}p{q}^{2}A_1^{2}-6\,{k}^{3}q{r}^{2}B_1^{2}-a_1^{2}{k}^{2}{q}^{2}B _{{1}}-7\,{k}^{4}pq{r}^{2}B_{{1}}\\&\qquad -\,a_1^{2}{\nu }^{2}{q}^{2}B_{{1}}-a_2^{2}{k}^{2}{q}^ {2}B_{{1}}+a_2^{2}{\nu }^{2}{q}^{2}B_{{1}})\,=\,0,\\& f g:\,\,p\,q^2\,A_1\,(k^4pq\,-\,k^2\,+\,\nu ^2)\,=\,0,\\& f:\,\,q^2 r B_1\,(k^4pq\,-\,k^2\,+\,\nu ^2)\,=\,0.\\ \end{aligned}$$

Solving the above system with respect to all parameters leads to the following two solutions:

$$\begin{aligned} \mathrm{(I)}\,\,A_1= & {} \dfrac{k \epsilon _1}{2}\,\sqrt{\dfrac{p(a_1^2q^2+a_2^2q^2-r^2)}{q}},\nonumber \\ B_1= & {} -\dfrac{k\,p}{2},\nu =k\epsilon _2\,\sqrt{1-k^2\,pq},\nonumber \\ \epsilon _1= & {} \pm 1, \epsilon _2=\pm 1. \end{aligned}$$
(51)
$$\begin{aligned} \mathrm{(II)}\,\,A_1= & {} r=0,B_1=-k\,p,\nonumber \\ \nu= & {} \pm k\,\sqrt{1-4\,k^2\,pq}. \end{aligned}$$
(52)

Combining the above results, we can derive two types of trigonometric function periodic solutions for (10).

Type 1 If \(pq>0\), from (44), (51) and (36), we get

$$\begin{aligned} u_9= & {} A_0\,\,\nonumber \\&+ \dfrac{k \epsilon _1\sqrt{pq(a_1^2q^2+a_2^2q^2-r^2)}}{2[a_1\,q\,\sin (\sqrt{pq}\xi )+a_2\,q\,\cos (\sqrt{pq}\xi )+r]}\nonumber \\&\,-\,\dfrac{k\,q\sqrt{pq}\,[a_2\,\sin (\sqrt{pq}\xi )-a_1\,\cos (\sqrt{pq}\xi )]}{2[a_1\,q\,\sin (\sqrt{pq}\xi )+a_2\,q\,\cos (\sqrt{pq}\xi )+r]},\nonumber \\ \xi= & {} k\,(x+\epsilon _2\,\sqrt{1-k^2\,pq}\,t),\,\epsilon _1=\pm 1, \epsilon _2=\pm 1, \end{aligned}$$
(53)

where \(A_0, k, a_1, a_2, r\) are arbitrary constants.

Type 2 If \(pq>0\), from (44), (52) and (36), we have

$$\begin{aligned} u_{10}= & {} A_0\,\,\nonumber \\&- \dfrac{k\,\sqrt{pq}\,[a_2\,\sin (\sqrt{pq}\xi )-a_1\,\cos (\sqrt{pq}\xi )]}{a_1\,\sin (\sqrt{pq}\xi )+a_2\,\cos (\sqrt{pq}\xi )},\nonumber \\ \xi= & {} k\,(x +\epsilon _1\,\sqrt{1-4k^2\,pq}\,t), \end{aligned}$$
(54)

where \(\epsilon _1=\pm 1\), \(A_0,k,a_1,a_2\) are arbitrary constants.

The solutions (48), (49), (50), (53), (54) are firstly reported here. By selecting appropriate parameters, their plots are shown in Figs. 4 and 5, respectively.

Fig. 4
figure 4

The plots of solitary wave solutions.  a The solution (48) with \(q=-p=a_1=\epsilon _1=-\epsilon _2=1\), \(k=0.8\), \(r=3\) and \(t=0.2\), \(A_0\)=0. b The solution (49) with \(q=-p=a_1=\epsilon _1=1\), \(k=0.4\), \(a_2=4\) and \(t=0.5\), \(A_0\)=0. c The solution (50) with \(q=-p=k=\epsilon _1=\epsilon _2=1\), \(a_1=-0.5\), \(a_2=-5\), \(r=10\), \(t=0.2\), \(A_0\)=0

Full size image
Fig. 5
figure 5

The plots of trigonometric function periodic solutions.  a The solution (53) with \(p=q=\epsilon _1=-\epsilon _2=1\), \(a_1=0.5\), \(a_2=2\), \(k=0.8\), \(r=1.9\), \(t=0.5\), \(A_0=0\). b The solution (53) with \(p=q=\epsilon _1=\epsilon _2=1\), \(a_1=-0.5\), \(a_2=1.2\), \(k=0.8\), \(r=0.3\), \(t=0.5\), \(A_0\)=0. c The solution (54) with \(p=q=a_1=\epsilon _1=1\), \(a_2=2\), \(k=0.4\), \(t=0.5\), \(A_0\)=0

Full size image

5 Periodic solitary wave solutions

In the past years, the study about interaction solutions has become a hot research issue and has already got good results [45,46,47,48,49,50,51,52,53,54]. In order to find the interaction solutions which can show some interesting physical phenomena, such as the fermionic quantum plasma between soliton and periodic waves [54], we aim at constructing some new interaction solutions between periodic waves and solitary waves using the direct method. Therefore, the solutions of (12) are supposed as

$$\begin{aligned} f= & {} a_1\,\mathrm{e}^{\theta _1}\,+\,a_2\,\mathrm{e}^{-\theta _1}\,+\, a_3\,{\sin (\theta _2)},\nonumber \\ \theta _1= & {} k_1\,x\,+\,c_1\,t,\,\,\, \theta _2\,=\,k_2\,x\,+\,c_2\,t, \end{aligned}$$
(55)

where \(a_1a_2 \ne 0\), and \(a_1\), \(a_2\), \(a_3\), \(k_1\), \(k_2\), \(c_1\), \(c_2\) are real parameters to be determined later.

Inserting (55) into (12) and equating the coefficients of \(\mathrm{e}^{\theta _1}\sin (\theta _2)\cos (\theta _2)\), \(\mathrm{e}^{-\theta _1}\sin (\theta _2)\cos (\theta _2)\), \(\mathrm{e}^{\theta _1}\sin ^2(\theta _2)\), \(\mathrm{e}^{-\theta _1}\sin ^2(\theta _2)\), \(\mathrm{e}^{2\theta _1}\sin (\theta _2)\), \(\mathrm{e}^{-2\theta _1}\sin (\theta _2)\), \(\mathrm{e}^{2\theta _1}\cos (\theta _2)\), \(\mathrm{e}^{-2\theta _1}\cos (\theta _2)\), \(\mathrm{e}^{\theta _1}\), \(\mathrm{e}^{-\theta _1}\) and \(\cos (\theta _2)\), we have an algebraic system consisting of 11 equations (see “Appendix A”). With the aid of computer algebraic software, we obtain two types of solutions:

$$\begin{aligned} \mathrm{(I)}\,a_1= & {} \Lambda _1,\,a_2\,=\,a_2,\,a_3=a_3,\nonumber \\ c_2= & {} \dfrac{\epsilon _1}{2}\sqrt{2(6k_1^2k_2^2-k_1^4-k_2^4 -k_1^2+k_2^2+\epsilon _2\Delta )},\nonumber \\ \Delta= & {} (k_1^8+4k_1^6k_2^2+6k_1^4k_2^4+4k_1^2k_2^6 +k_2^8+2k_1^6 \nonumber \\&+\,2k_1^4k_2^2-2k_1^2k_2^4-2k_2^6+k_1^4+2k_1^2k_2^2+k_2^4)^{1/2}, \end{aligned}$$
(56)

where \(\epsilon _1=\pm 1\), \(\epsilon _2=\pm 1\), the expression for \(\Lambda _1\) is cumbersome and thus given in “Appendix B,” and \(c_1\) is given by

$$\begin{aligned}&3c_{{2}}c_1^{2} + 4\,(k_{{1}}k_2^{3}-4k_1^{3}k_{{2}}-2k_{{1}}k_{{2}})\,c_1 + (6\,k_1^{2}k_2^{2}\nonumber \\&\quad -k_1^{4}-k_2^{4}-k_1^{2}+k_2^{2})\,c_2-c_2^{3}\,=0. \end{aligned}$$
(57)
$$\begin{aligned} \mathrm{(II)}\,a_1= & {} a_1,\,a_2=a_2,\,a_3=0,\,k_1=k_1,\nonumber \\ c_1= & {} \pm k_1\sqrt{1+4k_1^2}. \end{aligned}$$
(58)

From (56), together with (11) and (55), the periodic solitary wave solution is obtained as

$$\begin{aligned} u_{11}=\,& {} [\Lambda _1\,k_1\,\mathrm{e}^{(k_1 x+c_1t)}\,-\,a_2\,k_1\,\mathrm{e}^{-(k_1 x+c_1t)}\nonumber \\&+\,a_3\,k_2\,\cos (k_2 x+c_2t)]/[\Lambda _1 \mathrm{e}^{(k_1 x+c_1t)}\nonumber \\&\,+\,a_2\,\mathrm{e}^{-(k_1 x+c_1t)}\,+\,a_3\,\sin (k_2 x+c_2t)], \end{aligned}$$
(59)

where \(a_2\), \(a_3\), \(k_1\), \(k_2\) are arbitrary constants, \(c_2\) is given in (56) and \(\Lambda _1\) is given in “Appendix B.”

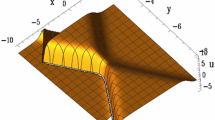
From (57), it is obvious that \(\Lambda _1\), \(c_1\) and \(c_2\) depend on the parameters \(k_1\) and \(k_2\). Additionally, \(a_2\) and \(a_3\) are arbitrary constants. Different choices of arbitrary parameters lead to different interactions between periodic waves and solitary waves. The three-dimensional plots and contour plots of (59) are shown in Fig. 6. It can be clearly seen that the interaction solution (59) is periodic in the space and time.

Fig. 6
figure 6

(Color online) The plots of interaction solution given by (59) with \(\epsilon _1=\epsilon _2=1\).  a \(a_2=0.9\), \(a_3=1.0\), \(k_1=-2\), \(k_2=2.5\), b \(a_2=3\), \(a_3=-1.5\), \(k_1=0.8\), \(k_2=2\), c \(a_2=1\), \(a_3=0.3\), \(k_1=0.2\), \(k_2=0.8\), d the contour plot of a, e the contour plot of b, f the contour plot of c

Full size image

If \(a_2=\Lambda _1\), the solution (59) can be expressed as

$$\begin{aligned} u_{11}^1\,=\,\dfrac{2\Lambda _1\,k_1\,\sinh (k_1 x+c_1t)\,+\,a_3\,k_2\,\cos (k_2 x+c_2t)}{2\Lambda _1\cosh (k_1 x+c_1t)\,+\,a_3\,\sin (k_2 x+c_2t)}. \end{aligned}$$

Taking \(a_2=-\Lambda _1\), the solution (59) becomes

$$\begin{aligned} u_{11}^{2}\,=\,\dfrac{2\Lambda _1\,k_1\,\cosh (k_1 x+c_1t)\,+\,a_3\,k_2\,\cos (k_2 x+c_2t)}{2\Lambda _1\sinh (k_1 x+c_1t)\,+\,a_3\,\sin (k_2 x+c_2t)}. \end{aligned}$$

From (11), (55) and (58), we get

$$\begin{aligned} u_{12}\,=\,\dfrac{a_1\,k_1\,\mathrm{e}^{(k_1 x \pm k_1\sqrt{1+4k_1^2}\,t)}\,-\,a_2\,k_1\,\mathrm{e}^{-(k_1 x \pm k_1\sqrt{1+4k_1^2}\,t)}}{a_1 \mathrm{e}^{(k_1 x \pm k_1\sqrt{1+4k_1^2}\,t)}\,+\,a_2\,\mathrm{e}^{-(k_1 x \pm k_1\sqrt{1+4k_1^2}\,t)}}, \end{aligned}$$

where \(a_1\), \(a_2\), \(k_1\) are arbitrary constants.

Remark 4

The solutions of (12) may be supposed as

$$\begin{aligned} f= & {} a_1\mathrm{e}^{\theta _1}+a_2\mathrm{e}^{-\theta _1}+ a_3{\cos (\theta _2)},\\ f= & {} a_1\mathrm{e}^{\theta _1}+a_2\mathrm{e}^{-\theta _1}+ a_3{\sin (\theta _2)}+a_4\,{\cosh (\theta _3)},\\ f= & {} a_1\mathrm{e}^{\theta _1}+a_2\mathrm{e}^{-\theta _1}+ a_3{\cos (\theta _2)}+a_4{\sinh (\theta _3)}, \end{aligned}$$

where \(\theta _i=k_i x+c_i t,\,(i=1,2,3)\). Performing the similar analysis, one can find other interesting multiple wave interaction solutions.

6 Conclusions

In this paper, we investigated a generalized fifth-order nonlinear equation with temporal and spatial dispersion. Following the standard WTC method, this model has been proven to be integrable in the sense of Painlevé for particular choice of parameters.

Searching for explicit exact solutions of nonlinear evolution equations is one of the significant problems in nonlinear science. For the integrable fifth-order nonlinear equation (10), several types of exact solutions have been presented via three different methods. We expect these new solutions helping us to understand the wave propagation processes in fluid mechanics for nonlinear equations with higher-order temporal and spatial dispersion.

The existence of multiple soliton solutions can further prove its integrability. In order to reduce the calculation complexity, we adopted the simplified Hirota method to derive the one-, two- and three-soliton solutions. This method does not need to transform the original nonlinear model into bilinear equations. Unlike most nonlinear evolution equations in 1+1 dimensions, this fifth-order nonlinear equation can describe shallow water waves moving in both directions. The evolution analysis for two-soliton and three-soliton solutions illustrates that both overtaking- and head-on collisions between multiple solitons are completely elastic.

Furthermore, the fifth-order nonlinear equation also possesses some interesting exact solutions, and we presented an extended projective Riccati expansion method and derived abundant travelling wave solutions systematically. This method can be applied to many other nonlinear evolution equations.

By virtue of the truncated Painlevé expansion, we also obtained some new types of interactions solutions between periodic waves and solitary waves. Using this direct method to obtain multiple waves interaction solutions, there always exists the problem of “intermediate expression swell.” It is an interesting research issue to propose a more efficient method for constructing multiple waves interaction solutions.

As future work, we can explore the higher dimensional extension of this fifth-order equation with third-order temporal and spatial dispersion. Meanwhile, other interesting properties, such as its infinite symmetries, bilinear Bäcklund transformation, Lax pair as well as more explicit solutions with physical interest, are also important issues to study in the future.