1 Introduction

There are two types of breathers, i.e., the Kuznetsov–Ma breathers (KMBs) [1, 2] and Akhmediev breathers (ABs) [3, 4]. The ABs are periodic in space and localized in time. On the contrary, the KMBs are periodic in time and localized in space. Taking the limit of these two solutions, we obtain the Peregrine soliton (PS) solution [5], which is regarded as a prototypical rogue wave (RW) profile in a series of experimental fields [6,7,8]. RWs, which are originally used to explain the extreme wave events in deep oceans [9], have recently been the subject of investigations in a wide range of complex nonlinear models with different mechanisms and physical backgrounds [10,11,12,13,14,15,16]. It appears from nowhere and disappears without a trace [17]. RW has a peak amplitude generally more than twice the significant wave height. In various models for describing RWs, the nonlinear Schrödinger (NLS) equation with the rational solution is considered to be the most accepted one. However, it has some restrictions in many physical backgrounds.

Various factors such as the variation in the lattice parameters of the fiber medium and variation of the fiber geometry lead to some nonuniformities in the fiber. Therefore, the effects including the fiber gain or loss, self-phase modulation (SPM) and variable dispersion are produced [18]. In order to describe the effects of nonlinear effects on the propagation of optical pulses, we often consider the variable-coefficient nonlinear Schrödinger (vc-NLS) equation and other types of variable-coefficient models [19, 20]. Such models, which offer more realistic description than their constant coefficient counterpart [21,22,23,24,25,26], have obtained extensive attention owing to the potential applications in dispersion management [27] and pulse compression [28]. Moreover, recent publications have shown that the RWs in nonautonomous systems provide certain novel characteristics such as the nonlinear tunneling effect, recurrence, annihilation and sustainment [29,30,31,32], to name a few. Besides, the studies of the control and manipulation of the RWs in the vc-NLS equations are useful to manage them experimentally in inhomogeneous optical fibers [32, 33].

It is well known that the standard NLS equation can be used to describe the propagation of a picsecond optical pulse. However, the higher-order effects such as the third-order dispersion (TOD), higher-order nonlinearities and self-steepening (SS) are indispensable for describing the propagation of ultrashort pulses [34,35,36,37,38,39]. These effects may contribute to certain novel properties for the wave propagation behaviors [36, 40, 41]. Compared with the standard NLS equation, the higher-order NLS ones characterize the nonlinear wave phenomena more accurately in reality [36, 37]. Additionally, a series of studies have shown that the higher-order effects account for the state transition between the breathers (or RWs) and other nonlinear waves on a continuous wave background [37, 42,43,44,45,46,47,48,49]. These transitions do not exist in the standard NLS equation. For instance, Akhmediev et al. have indicated that a breather solution can be converted into a soliton one in the third- and fifth-order equations [50, 51]. Wang et al. have discovered that the breathers can be transformed into various nonlinear waves in the fourth-order NLS equation [44]. Moreover, such state transitions have also been found in some higher-order coupled systems, i.e., the Hirota–Maxwell–Bloch (HMB) system [42], NLS-MB system [43] and coupled Hirota equation [47].

Currently, it is the most acceptable concept that the RW appears as a result of MI [52]. Interestingly, with certain higher-order perturbation terms such as the TOD and delayed nonlinear response term, the MI growth rate shows a non-uniform distribution characteristic in the low perturbation frequency region. In particular, it opens up a stability region as the background frequency changes [37, 47]. In addition, using the RW eigenvalue, one can find that the modulation stability (MS) condition is consistent with the state tranisition condition, which converts RWs into solitons on constant backgrounds [44,45,46].

In this paper, we study the vc-NLS equation with the fourth-order [53,54,55,56],

$$\begin{aligned}&iu_{t}+\alpha (t) u_{xx}+\beta (t)u|u|^2\nonumber \\&\qquad +\gamma _{1}(t) u_{xxxx}+\gamma _{2}(t)|u|^2 u_{xx}\nonumber \\&\qquad +\gamma _{3}(t)u^2 u^{*}_{xx}+\gamma _{4}(t)u^{*}u^{2}_{x}\nonumber \\&\qquad +\gamma _{5}(t)|u_{x}|^2 u+\gamma _{6}(t)|u|^4=0,\, \end{aligned}$$
(1.1)

where x is the propagation variable, t is the transverse variable, u(x, t) represents the coherent amplitude in Glaubers coherent-state representation for the Heisenberg ferromagnetic spin chain or the probability amplitude of the excitation in the protein molecular chain[53], \(\alpha (t)\), \(\beta (t)\), \(\gamma _{i}(t)\) (i =1, 2, ..., 6) are all the real functions of t. Equation (1.1) can be used to describe an inhomogeneous one-dimensional continuum anisotropic Heisenberg ferromagnetic spin chain or alpha helical protein [53,54,55]. In order to ensure the integrability of Eq. (1.1), all of these functions satisfy the linear relations, i.e., \(\gamma _{2}(t)=4\kappa \gamma _{1}(t)\), \(\gamma _{3}(t)=\kappa \gamma _{1}(t)\), \(\gamma _{4}(t)=3\kappa \gamma _{1}(t)\), \(\gamma _{5}(t)=2\kappa \gamma _{1}(t)\), \(\gamma _{6}(t)=\frac{3}{2}\kappa \gamma _{1}(t)\), \(\beta (t)=\kappa \alpha (t)\). The coefficients \(\alpha (t)\) and \(\gamma _{1}(t)\) describe the GVD and FOD effects, respectively. The terms proportional to \(\alpha (t)\) and \(\gamma _{1}(t)\) represent the elementary spin excitations related to the lowest order of continuum approximation and octupole–dipole interaction, respectively [53]. Yang et al. have discussed the dynamics of soliton solution for Eq. (1.1) via the bilinear method [53]. They have shown the interactions between a bound state and a single soliton [53]. In addition, they have found that the RWs can be divided into many similar components when the variable coefficients are the polynomial functions, while the RWs can be divided into many different components when the variable coefficients are the hyperbolic secant functions [54]. Xie et al. have found that the directions of two solitons change and the elastic interactions occur when the variable coefficients are functions [55]. Su et al. have reported the influences of the GVD, TOD, FOD and gain or loss coefficient on the propagation and interaction of the nonautonomous breathers and RWs for Eq. (1.1) [56]. To the best of our knowledge, the dynamics of nonautonomous multi-peak solitons and MI characteristic for Eq. (1.1) have not been reported in the existing papers, which are the main results of this paper.

The arrangement of this paper is as follows: In Sect. 2, we will discuss the dynamics of nonautonomous multi-peak soliton for Eq. (1.1). The state transition condition between nonautonomous breathers and nonautonomous multi-peak solitons will be given. Further, the characteristics of multi-peak solitons will be studied. The relation between the MI and state transition condition will be revealed in Sect. 3. Finally, Sect. 4 will give the conclusions of this paper.

2 The dynamics of the nonautonomous multi-peak soliton

In this section, by using the Darboux transformation (DT), the expression of the first-order nonautonomous breather solution of Eq. (1.1) can be given by

$$\begin{aligned} q_{B}^{[1]}=\bigg (c+8\,\beta _{1}\,\frac{G_{B}^{[1]}+i\,H_{B}^{[1]}}{D_{B}^{[1]}}\bigg )\,e^{i\,\rho },\, \end{aligned}$$
(2.1)

with

$$\begin{aligned} \rho =&\,rx+s(t), \\ s(t)=&\,\int ((-r^2+c^2\,\kappa )\alpha (t)+\frac{1}{2}(2\,r^4-12\,r^2\,c^2\,\kappa \\&+\,3\,c^4\,\kappa ^2)\gamma _{1}(t)){\text {d}}t, \\ G_{B}^{[1]}=&\,k_{1}\,k_{2}\,\chi _{1}\,\cos (t\,V_{H}+x\,\omega _{R})\\&+\,c\,(2+\kappa )\,M\,\cosh (tV_{T}+x\,\omega _{I})\\&+\,c\,(-2+\kappa )\,M\,\sinh (t\,V_{T}+x\,\omega _{I}), \\ H^{[1]}_{B}=&\,c\,(-2+\kappa )\,N\,\cosh (t\,V_{T}+x\,\omega _{I})\\&+\,k_{1}\,k_{2}\,\chi _{2}\,\sin (t\,V_{H}+x\,\omega _{R})\\&+\,c\,(2+\kappa )\,N\,\sinh (t\,V_{T}+x\,\omega _{I}), \\ D_{B}^{[1]}=&\,8\,\kappa \,c\,k_{1}\,k_{2}\,M\,\cos (tV_{H}+x\omega _{R})\\&+\,(2+\kappa )\,\chi _{1}\,\cosh (t\,V_{T}+x\,\omega _{I})\\&+\,(-2+\kappa )\,\chi _{1}\,\sinh (t\,V_{T}+x\,\omega _{I}), \\ \omega =&\,2\,\sqrt{\frac{\kappa }{2}\,c^2+\left( \frac{r}{2}+\lambda \right) ^2}=(\omega _{R}+i\omega _{I}),\\ h=&\,[x+(\alpha (t)(2\,\lambda -r)+\gamma _{1}(t)(r^3-2\,r^2\,\lambda \\&+\,2\,c^2\,\kappa \,\lambda -8\,\lambda ^3-3\,c^2\,\kappa \, r+4\,\lambda ^2\,r))t]\frac{\omega }{2}\\ =&\,[x+(h_{R}+ih_{I})t]\frac{\omega }{2}, \\ \chi _{1}=&\,r^2+h_{I}^2-2\,r\,h_{R}+h_{R}^2+4\,r\,\alpha _{1}-4\,h_{R}\,\alpha _{1}\\&+\,4\,\alpha _{1}^2-4\,h_{I}\,\beta _{1}+4\beta _{1}^2+2\,c^2\,\kappa , \\ \chi _{2}=&\,r^2+h_{I}^2-2\,r\,h_{R}+h_{R}^2+4\,r\,\alpha _{1}-4\,h_{R}\,\alpha _{1}\\&+\,4\,\alpha _{1}^2-4\,h_{I}\,\beta _{1}+4\beta _{1}^2-2\,c^2\,\kappa ,\,\\ k_{1}=&\,1, \quad k_{2}=-1,\, \\ M=&\,h_{I}-2\,\beta _{1},\, \quad N=r-h_{R}+2\alpha _{1},\,\\ V_{T}=&\,2(h_{R}\,\omega _{I}+h_{I}\,\omega _{R}),\\ V_{H}=&\,2(h_{R}\,\omega _{R}-h_{I}\,\omega _{I}). \end{aligned}$$

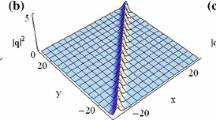
We note that Eq. (2.1) contains two variable coefficients, i.e., the GVD coefficient \(\alpha (t)\) and FOD coefficient \(\gamma _{1}(t)\), which can be flexibly manipulated according to different physical backgrounds. For example, Fig. 1 displays the periodic accelerating and decelerating motions of the first-order nonautonomous breather with \(\alpha (t)=1\), \(\gamma _{1}(t)=\cos (t)\). Further, from Eq. (2.1), we can calculate two significant physical quantities, namely the phase velocity \(V_\mathrm{p}=h_{R}+\frac{h_{I}\,\omega _{R}}{\omega _{I}}\) and group velocity \(V_\mathrm{g}=h_{R}-\frac{h_{I}\,\omega _{I}}{\omega _{R}}\). Generally speaking, Eq. (2.1) describes the dynamics of nonautonomous breather when \(V_\mathrm{p}\ne V_\mathrm{g}\) (or \(h_{I}\ne 0\)). In this case, the expression (2.1) contains both hyperbolic functions and trigonometric functions. However, if the phase velocity and group velocity have same value, i.e., \(V_\mathrm{p}= V_\mathrm{g}\) (or \(h_{I}=0\)), the state transition between nonautonomous breather and nonautonomous multi-peak soliton can be achieved. From Eq. (2.1), one can find that the phase velocity and group velocity can be controlled by initial wave number r. Therefore, we can get some special values of r by solving \(V_\mathrm{p}= V_\mathrm{g}\). Figure 2 describes the locus of \(V_\mathrm{p}\) and \(V_\mathrm{g}\). When \(r=2.32337\) and \(r=-1.12337\), we have \(V_\mathrm{p}= V_\mathrm{g}\), shown by the wine red dots in Fig. 2. Additionally, it should be pointed out that the case \(V_\mathrm{p}= V_\mathrm{g}\) is equivalent to

$$\begin{aligned} \frac{V_{T}}{\omega _{I}}=\frac{V_{H}}{\omega _{R}}, \end{aligned}$$
(2.2)

i.e.,

$$\begin{aligned} \frac{\alpha (t)}{\gamma _{1}(t)}=\frac{1}{r^2-4r\alpha _{1}+12\alpha ^{2}_{1}-4\beta ^{2}_{1}-c^2\kappa }. \end{aligned}$$
(2.3)

Equation. (2.2) [or Eq. (2.3)] implies the extrema of trigonometric and hyperbolic functions in the solution Eq. (2.1) is located along the same straight lines in the (x, t) plane, which leads to the transformation of the breather into a continuous soliton. Moreover, we should point out that GVD and FOD coefficients need to meet a directly proportional relationship to achieve the state transition. Otherwise, Eq. (2.3) has no solution with respect to r. This is different from the constant coefficient case in Ref. [44].

Fig. 1
figure 1

First-order nonautonomous breather with \(r=0.3\), \(c=0.9\), \(\gamma _{1}(t)=\cos (t)\), \(\alpha (t)=1\), \(\alpha _{1}=-0.15\), \(\beta _{1}=1\), \(\kappa =2\)

Full size image
Fig. 2
figure 2

Locus of phase velocity and group velocity with \(c=1\), \(\gamma _{1}(t)=4\), \(\alpha (t)=1\), \(\alpha _{1}=0.3\), \(\beta _{1}=-0.6\), \(\kappa =2\)

Full size image

In order to exhibit the dynamical properties of Eq. (1.1), we discuss the effects of the FOD coefficient \(\gamma _{1}(t)\) and GVD coefficient \(\alpha (t)\) on the nonautonomous multi-peak soliton.

Firstly, we consider the effects of higher-order terms on the velocity of the multi-peak soliton. From Eq. (2.1), we can see that the group velocity contains the FOD coefficient. Selecting two different values of \(\gamma _{1}(t)\) [\(\gamma _{1}(t)=0.58\) and \(\gamma _{1}(t)=0.32\)] will lead to different values of the group velocity \(V_\mathrm{g}\). As shown in Fig. 3a, b, the nonautonomous multi-peak soliton have negative velocity (\(h_{R}<0\) ) and positive velocity (\(h_{R}>0\)), respectively. In other words, the higher-order terms have the effect on the direction of the nonautonomous multi-peak soliton. Additionally, the similar influence of higher-order effects on the velocity of other nonlinear structures such as the standard solitons, breathers and RWs, have also been found in different higher-order NLS models [57,58,59].

Fig. 3
figure 3

Effects of FOD term on the velocity of nonautonomous multi-peak soliton with \(\gamma _{1}(t)\), a \(\gamma _{1}(t)=0.58\), b \(\gamma _{1}(t)=0.32\). Other parameters are \(c=1\), \(\alpha _{1}=0.7\), \(\beta _{1}=-0.3\), \(\alpha (t)=1\), \(\kappa =2\)

Full size image
Fig. 4
figure 4

Effects of FOD term on the localization of nonautonomous multi-peak solitons with \(\gamma _{1}(t)\), a \(\gamma _{1}(t)=0.029\), b \(\gamma _{1}(t)=0.089\), c \(\gamma _{1}(t)=0.298\). Other parameters are \(c=1\), \(\alpha _{1}=0.5\), \(\beta _{1}=-0.4\), \(\alpha (t)=1\), \(\kappa =2\)

Full size image

Secondly, we study the effects of higher-order terms on the localization of the multi-peak soliton. Fixing the value of \(\alpha (t)\), we change the value of \(\gamma _{1}(t)\). By choosing \(\gamma _{1}(t)=0.029\) and \(\gamma _{1}(t)=0.089\), Fig. 4a, b, respectively, shows a strong localization and a weak localization of the multi-peak solitons along the x-direction. This reflects the second significant effect of the FOD term on the multi-peak soliton, in addition to the velocity. However, the effects of FOD term have no obvious effects on the peak number and amplitude of the multi-peak soliton. In particular, the localization of the wave vanishes completely with \(\gamma _{1}(t)=0.298\). In this case, the multi-peak soliton is transformed into a periodic wave with vanishing localization, which is displayed in Fig. 4c. Correspondingly, the exact expression of the periodic wave reads as

$$\begin{aligned} q_\mathrm{p}^{[1]}=\bigg (c+8\,\beta _{1}\,\frac{G_\mathrm{p}^{[1]}+i\,H_\mathrm{p}^{[1]}}{D_\mathrm{p}^{[1]}}\bigg )\,e^{i\,\rho },\, \end{aligned}$$
(2.4)

with

$$\begin{aligned} G_\mathrm{p}^{[1]}=&\,k_{1}\,k_{2}\,\chi _{11}\,\cos (\omega _{R}(x+2\,t\,h_{R}))+c\,(2+\kappa )\,M_{1}, \\ H^{[1]}_\mathrm{p}=&\,c\,(-2+\kappa )\,N_{1}+k_{1}\,k_{2}\,\chi _{12}\,\sin (\omega _{R}(x+2\,t\,h_{R})),\\ D_\mathrm{p}^{[1]}=&\,8\,\kappa \,c\,k_{1}\,k_{2}\,M_{1}\,\cos (\omega _{R}(x+2\,t\,h_{R}))+(2+\kappa )\,\chi _{11},\,\\ \chi _{11}=&\,r^2-2\,r\,h_{R}+h_{R}^2+4\,r\,\alpha _{1}-4\,h_{R}\,\alpha _{1}+4\,\alpha _{1}^2\\&+\,4\beta _{1}^2+2\,c^2\,\kappa ,\,\\ \chi _{12}=&\,r^2-2\,r\,h_{R}+h_{R}^2+4\,r\,\alpha _{1}-4\,h_{R}\,\alpha _{1}+4\,\alpha _{1}^2\\&+\,4\beta _{1}^2-2\,c^2\,\kappa ,\,\\ M_{1}=&\,-2\,\beta _{1},\, N_{1}=r-h_{R}+2\alpha _{1}. \end{aligned}$$

Thirdly, we investigate the effects of GVD term on the multi-peak soliton. Unlike the previous discussions, we adjust the value of \(\alpha (t)\) while fixing the value of \(\alpha (t)\). As depicted in Fig. 5, we find that increasing the values of \(\alpha (t)\) leads to a stronger localization and a samller oscillation period for the multi-peak soliton. Moreover, the GVD coefficient \(\alpha (t)\) can change the number of peaks of the soliton. We observe that the wave described by the dashed purple curve [\(\alpha (t)=3.47\)] has nine humps, while the wave described by the solid blue curve [\(\alpha (t)=4.45\)] has fifteen humps. In other words, as the value of \(\alpha (t)\) increases, the number of peaks of soliton increases. However, the amplitude of the main peak remains unchangeable. This suggests that the GVD coefficient \(\alpha (t)\) not only affects the localization of the soliton, but also controls its peak number.

Fig. 5
figure 5

Effects of GVD term on the peak number of nonautonomous multi-peak solitons with \(\gamma _{1}(t)=0.29\), \(c=1\), \(\alpha _{1}=0.5\), \(\beta _{1}=-0.4\), \(\kappa =2\)

Full size image
Fig. 6
figure 6

a Compression effect of nonautonomous multi-peak solitons with \(r=0.5\), \(c=1\), \(\sigma _{1}=-4.328\), \(\sigma _{2}=1\), \(\alpha _{1}=0.504\), \(\beta _{1}=-0.36\), \(\kappa =2\), \(\varepsilon =0.1\). b Periodic variable motion of multi-peak solitons with \(r=0.5\), \(c=1\), \(\sigma _{1}=-2.157\), \(\sigma _{2}=1\), \(\alpha _{1}=0.48\), \(\beta _{1}=-0.36\), \(\kappa =2\), \(\varepsilon =0.1\)

Full size image

Fourthly, we consider an exponential fiber system, letting \(\gamma _{1}(t)\) and \(\alpha (t)\) as two linearly related functions. For instance,

$$\begin{aligned} \gamma _{1}(t)=\sigma _{1}\exp (\varepsilon t),\quad \quad \quad \alpha (t)=\sigma _{2}\exp (\varepsilon t). \end{aligned}$$
(2.5)

where the variable parameters \(\sigma _{1}\) and \(\sigma _{2}\) are connected with the FOD and GVD effects, the parameter \(\varepsilon \) is a constant. In Fig. 6a, we observe that the velocity and width of the multi-peak soliton change during the propagation. The case \(\varepsilon <0\) causes the multi-peak soliton to be compressed, whereas \(\varepsilon >0\) results in the multi-peak soliton to be amplified.

Finally, we consider a soliton management system, which is similar to that of Ref. [60], i.e., the periodic distributed system

$$\begin{aligned} \gamma _{1}(t)=\sigma _{1}\cos (\varepsilon t),\quad \quad \quad \alpha (t)=\sigma _{2}\cos (\varepsilon t). \end{aligned}$$
(2.6)

where trigonometric functions are physically relevant because they provide opposite (positive and negative) dispersion and nonlinearity with alternating regions. As shown in Fig. 6b, the multi-peak soliton is periodic acceleration and deceleration in the propagation.

From the above analysis, we can find that when the GVD coefficient \(\alpha (t)\) and FOD coefficient \(\gamma _{1}(t)\) satisfy the condition (2.3), the nonautonomous breather can be transformed into nonautonomous multi-peak soliton in a one-dimensional continuum anisotropic Heisenberg ferromagnetic spin chain or alpha helical protein. In addition, we find that the lowest order of continuum approximation and octupole–dipole interaction in a Heisenberg ferromagnetic spin chain do not influence the amplitude of the soliton, but they influence the velocity, localization and peak number of the nonautonomous multi-peak soliton, respectively. For the detailed discussions of relation between the interactions between/among the two and three solitons and the ferromagnetism, one can refer to [53, 61].

3 MI characteristics

In this section, we reveal the explicit relation between the state transition and MI characteristic for Eq. (1.1) by linear stability analysis. The plane-wave solution of Eq. (1.1) is presented as

$$\begin{aligned} {u}(x,t)=c\,e^{i\,(r\,x+s(t))}, \end{aligned}$$
(3.1)

where c and r are two real parameters. The perturbation solution can be expressed as

$$\begin{aligned} {u}(x,t)=(c+\epsilon \,{\widehat{u}}(x,t))\,e^{i\,(r\,x+s(t))}, \end{aligned}$$
(3.2)

where \({\widehat{u}}(x,t)\) is the small-amplitude perturbation [62]. Substituting Eq. (3.2) into Eq. (1.1) yields the evolution equation for the perturbation \({\widehat{u}}(x,t)\) as

$$\begin{aligned}&\kappa \,c^{2}\,\alpha (t)\,{\widehat{u}}(x,t)-6\kappa \,r^{2}\,c^{2}\,\gamma _{1}(t){\widehat{u}}(x,t)\nonumber \\&\quad +\,3\kappa ^2\,c^{4}\,\gamma _{1}(t){\widehat{u}}(x,t)\nonumber \\&\quad +\,\kappa \,c^{2}\,\alpha (t){\widehat{u}}^{*}(x,t)-6\kappa \,c^{2}\,r^{2}\,\gamma _{1}(t){\widehat{u}}^{*}(x,t)\nonumber \\&\quad +\,3\kappa ^2\,c^{4}\,\gamma _{1}(t){\widehat{u}}^{*}(x,t)\nonumber \\&\quad +\,i\,{\widehat{u}}^{(0,1)}(x,t)+2\,i\,r\,\alpha (t){\widehat{u}}^{(1,0)}(x,t)\nonumber \\&\quad -\,4\,i\,r^{3}\,\gamma _{1}(t){\widehat{u}}^{(1,0)}(x,t)\nonumber \\&\quad +\,12\kappa \,i\,r\,c^{2}\,\gamma _{1}(t)\,{\widehat{u}}^{(1,0)}(x,t)+\alpha (t){\widehat{u}}^{(2,0)}(x,t)\nonumber \\&\quad -\,6\,r^{2}\,\gamma _{1}(t){\widehat{u}}^{(2,0)}(x,t)+4\kappa \,c^{2}\,\gamma _{1}(t){\widehat{u}}^{(2,0)}(x,t)\nonumber \\&\quad +\,\kappa \,c^{2}\,\gamma _{1}(t){\widehat{u}}^{*(2,0)}(x,t)+4\,i\,r\,\gamma _{1}(t){\widehat{u}}^{(3,0)}(x,t)\nonumber \\&\quad +\,\gamma _{1}(t)\,{\widehat{u}}^{(4,0)}(x,t)=0. \end{aligned}$$
(3.3)

Noting that the linearity of Eq. (3.3) with respect to \(\widehat{u}(x,t)\), we introduce

$$\begin{aligned} {\widehat{u}}(x,t)={\text {U}}1\,e^{i\,(Q\,x-\varpi (t))}+{\text {V}}1\,e^{-i\,(Q\,x-\varpi ^{*}\,(t))}, \end{aligned}$$
(3.4)

which is characterized by the frequency \(\varpi (t)\) and wave number Q. Using Eq. (3.4) into Eq. (3.3) gives a linear homogeneous system of equations for U1 and V1:

$$\begin{aligned} \begin{aligned}&-\,2\,r\,Q\,{\text {U}}1\,\alpha (t)-Q^2\,{\text {U}}1\,\alpha (t)+\kappa \,c^{2}\,{\text {U}}1\alpha (t)\\&\quad +\kappa \,c^{2}\,{\text {V}}1\,\alpha (t)+4\,r^{3}\,Q\,{\text {U}}1\gamma _{1}(t)+6\,r^{2}\,Q^{2}\,{\text {U}}1\gamma _{1}(t)\\&\quad -6\,\kappa \,r^{2}\,c^{2}\,{\text {U}}1\gamma _{1}(t)-12\,\kappa \,\,r\,c^{2}\,Q\,\,{\text {U}}1\gamma _{1}(t)\\&\quad -4\,\kappa \,c^{2}\,Q^{2}\,\,{\text {U}}1\gamma _{1}(t)-6\,\kappa \,r^{2}\,c^{2}\,{\text {V}}1\gamma _{1}(t)\\&\quad -\kappa \,c^{2}\,Q^{2}\,{\text {V}}1\gamma _{1}(t)+3\,\kappa ^{2}\,c^{4}\,{\text {U}}1\gamma _{1}(t)\\&\quad +3\,\kappa ^{2}\,c^{4}\,{\text {V}}1\gamma _{1}(t)+{\text {U}}1\,\varpi '(t)=0, \end{aligned} \end{aligned}$$
(3.5)
$$\begin{aligned} \begin{aligned}&2\,r\,Q\,{\text {V}}1\,\alpha (t)-Q^2\,{\text {V}}1\,\alpha (t)+\kappa \,c^{2}\,{\text {U}}1\alpha (t)\\&\quad +\,\kappa \,c^{2}\,{\text {V}}1\,\alpha (t)-4\,r^{3}\,Q\,{\text {V}}1\gamma _{1}(t)+6\,r^{2}\,Q^{2}\,{\text {V}}1\gamma _{1}(t)\\&\quad -\,6\,\kappa \,r^{2}\,c^{2}\,{\text {V}}1\gamma _{1}(t)+12\,\kappa \,\,r\,c^{2}\,Q\,\,{\text {V}}1\gamma _{1}(t)\\&\quad -\,4\,\kappa \,c^{2}\,Q^{2}\,\,{\text {V}}1\gamma _{1}(t)-6\,\kappa \,r^{2}\,c^{2}\,{\text {U}}1\gamma _{1}(t)\\&\quad -\,\kappa \,c^{2}\,Q^{2}\,{\text {U}}1\gamma _{1}(t)+3\,\kappa ^{2}\,c^{4}\,{\text {U}}1\gamma _{1}(t)\\&\quad +\,3\,\kappa ^{2}\,c^{4}\,{\text {V}}1\gamma _{1}(t)-{\text {V}}1\,\varpi '(t)=0. \end{aligned} \end{aligned}$$
(3.6)

From the determinant of the coefficient matrix of Eqs. (3.5)–(3.6), the dispersion relation for the linearized disturbance can be determined as

$$\begin{aligned} {\varpi }^{2}(t)+J_{1}\,\varpi (t)+J_{0}=0, \end{aligned}$$
(3.7)

with

$$\begin{aligned} J_{1}=&\,-4 \,r \, Q\, \left( 4\, r^2\, \gamma _{1}(t) +3\,\kappa \, c^2 \gamma _{1}(t)+Z\right) , \\ J_{0}=&\,Q^{2}(\alpha (t)^{2}(4\,r^{2}-Q^{2}+2\,\kappa \,c^{2}\,)\\&+\,4(-4\,r^{4}+\kappa \,c^{2}(-2\,Q^{2}+3\,\kappa \,c^{2}))\alpha (t)\gamma _{1}(t)\\&+\,(16\,r^{6}-12\,r^{4}(3\,Q^{2}+2\,\kappa \,c^{2})\\&+\,24\,\kappa \,r^{2}\,c^{2}(2\,Q^{2}+3\,\kappa \,c^{2})\\&+\,3\,\kappa ^{2}\,c^{4}(-5\,Q^{2}+6\,\kappa \,c^{2}))\gamma _{1}(t)^{2}),\\ Z=&\,\alpha (t) -6\,r^2 \gamma _{1}(t) +3\,\kappa \,c^2 \,\gamma _{1}(t) . \end{aligned}$$

Solving the above equation, we have

$$\begin{aligned} \varpi (t)= & {} \, 2 \,r \, Q (4\, r^2\, \gamma _{1}(t) +3\,\kappa \, c^2 \gamma _{1}(t)+Z)\nonumber \\&\pm \,Q\sqrt{\, Z (Q ^2 (2\,\kappa \,c^2\, \gamma _{1}(t) +Z)-2\,\kappa \, Z \,c^2)},\,\nonumber \\ \end{aligned}$$
(3.8)

In this case, the frequency \( \varpi (t) \) becomes complex and the disturbance will grow with time exponentially if and only if \( Q^2<Q_{c}^2=\frac{2\,\kappa \, Z \,c^2}{2\,\kappa \,c^2\, \gamma _{1}(t) +Z} \), and the growth rate of the instability is given by

$$\begin{aligned} \varGamma =|Q| \sqrt{\, Z \left( -Q ^2 \left( 2\,\kappa \,c^2\, \gamma _{1}(t) +Z\right) +2\,\kappa \, Z \,c^2\right) }. \end{aligned}$$
(3.9)

To obtain the maximum growth rate of the instability, we take the derivative of Eq. (3.9) with respect to Q, and set it to zero. Then, we obtain

$$\begin{aligned} Q_{\max }=\pm \sqrt{\frac{\kappa \,c^2\, Z}{2\,\kappa \,c^2\, \gamma _{1}(t)+Z }}, \end{aligned}$$
(3.10)

and the following maximum growth rate of the instability:

$$\begin{aligned} \varGamma _{\max }=\frac{\kappa \,c^2\, \left| Z\right| }{\sqrt{2\,\kappa \,c^2\, \gamma _{1}(t)/Z+1 }}. \end{aligned}$$
(3.11)

From Fig. 7, we can see that the distribution characteristic of MI gain is impacted by the FOD coefficient in the region \(-\sqrt{2\,\kappa }\,c<Q<\sqrt{2\,\kappa }\,c\), and it has two symmetric modulation stability (MS) region where the MI growth rate is vanishing in the low perturbation frequency region. The MS regions are characterized by the two dashed orange lines in Fig. 7. Moreover, the expression of the MS regions is given by

$$\begin{aligned} r=r_{s}=\pm \sqrt{\frac{\alpha (t)}{6\,\gamma _{1}(t)}+\frac{\kappa c^{2}}{2}}, \end{aligned}$$
(3.12)

i.e.,

$$\begin{aligned} \gamma _{1}(t)=\frac{\alpha (t)}{6\,r^2-3\,\kappa \,c^{2}}. \end{aligned}$$
(3.13)

We note that the MS condition (3.13) requires a special coefficient relationship where \(\gamma _{1}(t)\) is proportional to \(\alpha (t)\). This is consistent with the soliton management system described by Eqs. (2.5) and (2.6). Further, using the RW eigenvalue \(\lambda _{0}=-\frac{r}{2}+i\frac{\sqrt{2\kappa }}{2}\,c\), we find the state transition (2.3) is consistent with the MS region condition (3.13). Our results show that the state transition between the RWs and multi-peak solitons can exist in the MS region with low frequency perturbation.

Fig. 7
figure 7

Characteristics of MI growth rate \(\varpi \) on (Q, r) plane with c=0.5, \(\kappa =2\), \(\alpha (t)=1\), \(\gamma _{1}(t)=0.02\). Here the dashed orange lines represent the stability region in the perturbation frequency region \(-\sqrt{2\,\kappa }\,c<Q<\sqrt{2\,\kappa }\,c,\) which is presented as  \(r=r_{s}=\pm \sqrt{\frac{\alpha (t)}{6\,\gamma _{1}(t)}+\frac{\kappa c^{2}}{2}}\). (Color figure online)

Full size image

4 Conclusions

We have presented the first-order nonautonomous breather solution for Eq. (1.1) and the state transition between nonautonomous breather and nonautonomous multi-peak soliton. We have discussed the effects of higher-order terms on the nonautonomous multi-peak soliton, including the velocity, localization, peak number and width. We have also shown that the state transition condition is consistent with the MS region condition when the RW eigenvalue is taken.