Highly efficient continuous bistable nonlinear energy sink composed of a cantilever beam with partial constrained layer damping

Abstract

This paper focuses on the transient nonlinear dynamics and targeted energy transfer (TET) of a Bernoulli–Euler beam coupled to a continuous bistable nonlinear energy sink (NES). This NES comprises a cantilever beam with the partial constrained layer damping (PCLD) and an end mass controlled by a nonlinear magnetostatic interaction force. The theoretical model of the nonlinear system is built based on the Lagrange equations and assumed-modes expansion method. A new parameter system damping ratio is proposed to evaluate the TET efficiencies. Impact experiments are carried out to verify the theoretical model and mechanisms. The results show that the bistable NES can achieve high and strongly robust TET efficiencies under broad-range impacts. The shear modulus of the viscoelastic layer, the length of the PCLD and the end mass have significant influences on TET efficiencies. Analyses of the TET mechanisms in the bistable NES show the following: steady transition of the stable state is an important reason for maintaining high TET efficiencies; nonlinear beatings can occur in high-frequency, fundamental and long-period subharmonic branches; and resonance captures featuring fundamental and subharmonic also help achieve rapid energy dissipation.

1 Introduction

Vibration has large influences on the function, noise control and reliability of a mechanical system. Passive vibration control techniques have been chosen as a primary method in many facilities because of the advantages of high efficiency and lack of power consumption. The efficiencies of the traditional linear vibration absorbers or tuned mass dampers (TMDs) are restricted by their narrowband and poor robustness [1, 2]. A nonlinear approach for vibration suppression was considered to enable maintain high performance with a broader bandwidth under transient excitations. The passive nonlinear targeted energy transfer (TET) technique utilizes nonlinear mode localization and internal resonance to irreversibly transfer transient vibration energy from the primary system to a nonlinear energy sink (NES) that eventually dissipates the energy in the NES [3]. The robustness of an NES arises from the absence of the preferential linear natural frequency [4]. Research has been extensively investigated the phenomena and mechanisms of NES [5,6,7]. Fundamental TET, subharmonic TET and TET initiated by nonlinear beating are the main mechanisms, and nonlinear beating along the high-frequency special branches represents the most efficient mechanism of energy dissipation [8]. Presently, several types of NESs have been proposed and studied, such as oscillating dissipative attachments with essentially strong nonlinear stiffness [9,10,11,12], rotational elements [13, 14], vibro-impact NES [15, 16] and magnet-based NES [17]. Experiments have been carried out to verify the performance of NESs [18]. However, a traditional NES has a critical energy threshold in the transient regime in order to initiate the nonlinear beating [2, 8, 18], resulting in only having high TET efficiency under moderate transient impacts. Recently, a bistable NES [19, 20] was proposed to breakthrough the limit of the input energy threshold to maintain high performance of shock mitigation under a broad range of input energy. To explore the transient dynamics in a linear system coupled to a lightweight bistable mass, Romeo et al. [21] carried out a numerical study and Manevitch et al. [22] presented an analytical study with the complexification-averaging method [23]. Both studies focused on the impulsively excited linear oscillator (LO) to the bistable NES. The results show that, along with the main regime of 1:1 resonance capture, the superharmonic 1:3 resonance capture regime can also be realized and thus results in a strong energy exchange between the LO and bistable NES. However, the TET mechanisms and the nonlinear dynamics of the bistable NES need more research and experimental validations.

At present, a NES consists of a discrete single-degree-of-freedom (SDOF) nonlinear oscillator and a viscous damper. The transient dynamic behaviors of the SDOF NES coupled to a LO have been widely investigated. The only performance index of absorbers considered is the percentage of instantaneous total energy dissipated by the NES. However, this index percentage cannot reflect the decay rate of vibration energy that is an important parameter in practice. Recently, Farid and Gendelman [24] used the relative amount of the energy left in the system after a given time as an alternative index. Moreover, research on the NES composed of continuous structures is minimal. The bistable continuous structures were employed as vibration energy harvesters (VEHs) to achieve good performance under low-frequency excitations [25]. These VEHs usually consist of a cantilever beam attached with piezoelectric generators. Most of the relevant literature modeled the bistable VEH as a SDOF oscillator by considering the first-order modal of the beam and considered the primary structures as rigid bodies [25,26,27,28,29]. However, these modeling methods are not proper for broad-range and high-frequency vibrations and cannot reveal the deformation of primary structures.

From the review above, it is important and interesting to study the nonlinear dynamics of a continuous elastic structure coupled to a continuous bistable NES. This paper focuses on the transient nonlinear dynamics and the TET efficiency of a continuous bistable energy sink coupled to an elastic beam. The NES consists of a cantilever beam attached with partial constrained layer damping (PCLD) and a magnetostatic field that produces an interaction force as the nonlinear restoring force. Based on the Lagrange equations and the assumed-modes expansion method, the motion differential equations are established to study the dynamic behaviors with numerical solutions. Besides the commonly used parameter percentage of total energy dissipated by NES, another parameter system damping ratio is defined to evaluate the TET efficiency. Impact experiments on the structure with different restoring forces are carried out to validate the theoretical model and the bistable transition behaviors. Then, the influences of different PCLD and structure parameters on TET efficiencies are investigated. Finally, this paper analyzes and summarizes the TET mechanisms of the bistable NES. There are five sections in this paper. The second section presents the process to establish the theoretical model and the analysis method. Impact experiments and comparisons between the experimental and theoretical results are described in Sect. 3. The efficiencies and mechanisms of the bistable NES are analyzed in Sect. 4 based on the numerical methods. Finally, we make conclusions in Sect. 5.

2 Modeling and derivation

2.1 Model description

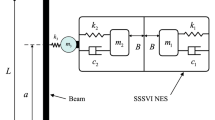
The related systems are illustrated in Fig. 1. An NES is coupled to a primary Bernoulli–Euler beam (P-beam). Boundary conditions of the P-beam can be defined by its modal functions. The absorber consists of a small cantilever beam (S-beam) attached with PCLD and an end mass $m_\mathrm{t}$. The magnetostatic interaction force of the permanent magnets provides the nonlinear restoring force for the S-beam. There are two types of configurations: repulsive force and attractive force. Their modeling methods are identical; thus, only the attractive force NES is taken into account in the experiments and analyses. The S-beam is fixed to the P-beam at point $\hbox {O}_{1}$ with a rigid strut whose height is $L_\mathrm{c}$. The other strut is fixed at point $\hbox {O}_{2}$ to support the magnets. For attractive force NES, the two smaller magnets on point $\hbox {O}_{2}$ are symmetrical to the undeformed S-beam. By modulating the magnetic forces, the absorber can behave as a monostable NES or a bistable NES. It is a linear absorber if the interaction force is zero. The masses of the struts are $m_{p1}$ and $m_{p2}$, respectively. Other parameters are labeled in Fig. 1.

Fig. 1

The model of a monostable/bistable energy sink coupled to an elastic beam

2.2 Magnetostatic interaction forces of permanent magnets

Traditionally, dipole–dipole magnetostatic interaction equations were used by most of the authors to incorporate the magnetic coupling induced into the dynamics of the energy harvesters [25,26,27,28]. However, the dipole–dipole formulation restricts the application within the sphere of its corresponding assumptions. Vokoun et al. [30, 31] proposed the equations for magnetostatic forces between cylindrical permanent magnets. Avvari [32] used these equations to generate multi-stable states. With parameters labeled in Fig. 2b, the potential energy is [31, 32]

$$\begin{aligned}&E\left( D,y\right) =\varepsilon \mu _0 M_1 M_2 \pi R_2 R_1^2 \nonumber \\&\quad \cdot \int _0^\infty {J_0 \left( \frac{yq}{R_1 }\right) } \frac{J_1 \left( {R_2 q}/{R_1}\right) }{q^{2}}J_1 \left( q\right) U\left( D,q\right) {\text{ d }}q\nonumber \\ \end{aligned}$$

(1)

where $\varepsilon =-1(\varepsilon =1)$ denotes the attractive (repulsive) force, $\mu _{0}$ is the vacuum permeability $\mu _{0}=4\pi \times 10^{-7}\,\hbox {N}\, \hbox {A}^{-2}, R_{i}$ denotes the radiuses of the cylinders, $R_{2}\le R_{1}$ is assumed, $M_{1}$ and $M_{2}$ are the saturation magnetizations, and $J_{0}(\cdot )$ and $J_{1}(\cdot )$ are the zero-order and first-order Bessel functions, respectively. U(D, q) is expressed as [32]

$$\begin{aligned} U\left( D,q\right)= & {} \hbox {e}^{-{q\left( d_1 +d_2 +D\right) }/{R_1 }}+\hbox {e}^{-{qD}/{R_1}} \\&-\,\hbox {e}^{-{q\left( D+d_1 \right) }/{R_1}} \\&-\,\hbox {e}^{-{q\left( D+d_2 \right) }/{R_1}} \end{aligned}$$

${\hbox {d}J_0 (x)}/{\hbox {d}x}\,{=}\,-J_1 (x)$ and the interaction forces are obtained by $F_y (D,y)\,{=}\,-{\partial E}/{\partial y}$. Therefore, for the NES of the attractive force configuration, the total force in the transverse direction is

$$\begin{aligned}&F_y \left( D,\Delta \right) =\mu _0 M_1 M_2 \pi R_1 R_2 \nonumber \\&\quad \cdot \int _0^\infty {\left[ {J_1 \left( {\frac{\left( d/2-\Delta \right) q}{R_1}} \right) -J_1 \left( {\frac{\left( d/2+\Delta \right) q}{R_1}} \right) } \right] } \nonumber \\&\quad \, \cdot \frac{J_1 \left( {R_2 q}/{R_1 }\right) }{q}J_1 \left( q\right) U\left( D,q\right) {\text{ d }}q \end{aligned}$$

(2)

As analyzed in Fig. 3, $\Delta$ denotes the displacement of the end magnet on a cantilever beam relative to its undeformed point. Although $J_0 (\cdot )$ and $J_1 (\cdot )$ cannot be accurately expanded with few low-order terms of the Taylor series $(\hbox {order}\,{<}\,5)$, we can still accurately fit the force $\Delta$ curves described by (2) with a function $F_{y}(D, \Delta ) = \varepsilon (\beta _{1}\Delta +\beta _{2}\Delta ^{3})+O(\Delta ^{5})$. Thus,

$$\begin{aligned} F_y \left( D,\Delta \right) \approx \beta _1 \Delta +\beta _2 \Delta ^{3} \end{aligned}$$

(3)

One can use the polynomial fitting method to obtain parameters $\beta _{1}$ and $\beta _{2}$. It can be deduced from equation (2) that the stiffness of $\beta _1$ and $\beta _2$ are correlative and that $\beta _1 >0,\beta _2 <0$.

Fig. 2

Magnetostatic interaction in a dipole–dipole configuration and b cylinder–cylinder configuration

Fig. 3

Interaction forces generated by repulsive and attractive forces

2.3 Motion equations for NES coupled to an elastic beam

In engineering, the effectiveness of vibration control also depends on the damping configurations. Viscoelastic damping is widely adopted in continuous structures [33]. Constrained layer dampers (CLDs) were proposed by Edward and Kerwin [34]. The dissipation mechanisms of CLD mainly arise from the shear deformation of the viscoelastic layer [33]. PCLD was proposed by Plunkett and Lee [35] to increase structural damping. Many researchers used the genetic algorithm method [36], moving asymptotes [37] and evolutionary structural optimization [38] to optimize the parameters of CLDs and PCLDs. This paper adopted unilateral PCLD to realize the damping of NES. The analytical calculations of CLD and PCLD are based on modal strain energy approaches proposed by Mead et al. [39, 40].

The basic assumptions for the analytical model are (1) the presence of shear strain in the base and constrained layers; the rotary inertia of all layers is negligible; (2) the presence of only shear stress but no normal stress in the viscoelastic layer; (3) the strain is small compared to the structure for both linear and nonlinear NES; (4) the slipping does not occurs at the interfaces between layers and the deformations at the interfaces are continuous; (5) the plane transverse to the middle layer cross section remains planar during bending; and (6) the transverse displacements of all layers are identical. Based on these assumptions, the deformation patterns of the base beam, viscoelastic layer and constrained layer are illustrated in Fig. 4.

Fig. 4

Deformation pattern of three layers in PCLD treatment

The geometry functions of displacements are

$$\begin{aligned} u_c =u_v +{h_v \left( \gamma -\theta \right) }/2-{h_c \theta }/2 \nonumber \\ u_b =u_v -{h_v \left( \gamma -\theta \right) }/2+{h_b \theta }/2 \end{aligned}$$

(4)

where subscripts ‘b’, ‘v’ and ‘c’ denote the base beam of the absorber, the viscoelastic layer and the constrained layer, respectively; $u_b, u_v$ and $u_c$ are longitudinal displacements (in x direction) of middle planes; $w_{r}$ is the transverse displacement of the base beam relative to point $\hbox {O}_{1}$ in Fig. 1, and angle $\theta ={\partial w_r}/{\partial x}$; $\gamma$ denotes the angle of the transverse plane of viscoelastic layer relative to its transverse direction; and $h_{\mathrm{b}}, h_{\mathrm{v}}$, and $h_{\mathrm{c}}$ represent the heights of the corresponding layers. Solving Eq. (4) obtains

$$\begin{aligned} u_v= & {} \frac{u_b +u_c }{2}+\frac{h_c -h_b }{4}\frac{\partial w_r }{\partial x} \nonumber \\ \gamma= & {} \frac{1}{h_v }\left[ {u_c -u_b +h_t \frac{\partial w_r }{\partial x}} \right] ,h_t =\frac{h_c +h_b +2h_v }{2} \end{aligned}$$

(5)

Assuming that the longitudinal displacement of the P-beam is negligible, the P-beam has a transverse displacement y(x, t) only. Because the strut connecting the P-beam and the S-beam is rigid, the displacements and angles for the two beams should be identical at point $\hbox {O}_{1}$. Assuming the displacement at point $\hbox {O}_{1}$ is $\varphi (t)=y(L_{0}, t)$, the absolute displacement of the S-beam is

$$\begin{aligned} w\left( x,t\right) =w_r +\varphi +x\sin \theta _0 \approx w_r +\varphi +x\frac{\partial y\left( L_0, t\right) }{\partial x} \end{aligned}$$

(6)

where x is the distance between the point on the S-beam and its root $\hbox {O}_{1}$. For the P-beam, a further assumption is made that additional mass $m_{pi}$ are scattered at positions $L_{xi}$.

When the absorber is coupled to the P-beam, the kinetic energy of the entire system is

$$\begin{aligned} T=T_p +T_b +T_v +T_c +T_m \end{aligned}$$

(7)

The subscripts ‘p’ and ‘m’ denote the P-beam and the end mass $m_t$, respectively. The expressions for $T_{p}, T_{b}, T_{v}, T_{c}$ and $T_{m}$ are shown in Appendix.

The potential energy of the system includes two parts: the strain energy of structures and the magnetostatic potential energy generated by force $F_y (D,\Delta )$ in Eq. (3). The strain potential energy of the P-beam, the base beam of absorber and the constrained layer are

$$\begin{aligned} V_p= & {} \frac{1}{2}\int _0^L {E_p I_p \left( {\frac{\partial ^{2}y\left( x,t\right) }{\partial x^{2}}} \right) ^{2}} {\text{ d }}x \nonumber \\ V_b= & {} \frac{1}{2}\int _0^{L_b} {\left[ {E_b A_b \left( {\frac{\partial u_b }{\partial x}} \right) ^{2}+E_b I_b \left( {\frac{\partial ^{2}w_r }{\partial x^{2}}} \right) ^{2}} \right] } {\text{ d }}x \nonumber \\ V_c= & {} \frac{1}{2}\int _{x_1 }^{x_2} {\left[ {E_c A_c \left( {\frac{\partial u_c }{\partial x}} \right) ^{2}+E_c I_c \left( {\frac{\partial ^{2}w_r }{\partial x^{2}}} \right) ^{2}} \right] } {\text{ d }}x\nonumber \\ \end{aligned}$$

(8)

where $I_s, I_b$ and $I_c$ represent the moment of inertia across the neutral surface of P-beam, the S-beam and the constrained layer, respectively, and they are $I_{b(c)} =bh_{b(c)}^3 /12$, where b is the width. The shear strain energy of the viscoelastic layer is

$$\begin{aligned} V_v =\frac{1}{2}\int _{x_1 }^{x_2} {G_v A_v \gamma ^{2}} {\text{ d }}x \end{aligned}$$

(9)

In Eqs. (8) and (9), $E_{p(b,c)}$ are elastic moduli and $G_v$ is the shear modulus. These moduli are expressed as complex formats $E_{p(b,c)} =\bar{{E}}_{p(b,c)} (1+i\eta _{p(b,c)}), G_v =\bar{{G}}_v (1+i\eta _v), i^{2}=-1$, where $\eta _{p(b,c,v)}$ are loss factors.

Fig. 5

Relationships between displacements

The magnetostatic potential energy depends on the relative motion of magnets. As shown in Fig. 5, the end point of the undeformed S-beam falls at position $\hbox {O}_{3}$. $\hbox {O}_{4}$ marks this point on the P-beam. After being deformed, $\hbox {O}_{3}$, moves to ${O}_{3}^{\prime \prime }$, while $\hbox {O}_{4}$ moves to ${O}_{4}^{\prime }$, with a generated relative displacement $\Delta \,{=}\,|{O}_{3}^{\prime \prime } {O}_{4}^{\prime }|$. Seriously, $|{O}_{3}^{\prime \prime } {O}_{4}^{\prime } |$is not a transverse displacement. In practice, the distance D in Fig. 2b has a small alteration, and $|{O}_{3}^{\prime \prime } {O}_{4}^{\prime } |$ can be used to approximate this alteration. According to Eq. (6), $\Delta$ is expressed as

$$\begin{aligned} \Delta= & {} w\left( L_b, t\right) -y\left( L_m, t\right) =w_r \left( L_b, t\right) +y\left( L_0, t\right) \nonumber \\&+\, L_b \frac{\partial y\left( L_0, t\right) }{\partial x}-y\left( L_m, t\right) \end{aligned}$$

(10)

Defining $y_\Delta (t)\,{=}\,y(L_0, t)-y(L_m, t)$, then the magnetostatic potential energy generated by force $F_y (D,\Delta )$ is

$$\begin{aligned} V_m= & {} V_{m0} -\frac{1}{2}\beta _1 \left[ {w_r \left( L_b, t\right) +L_b \frac{\partial y\left( L_0, t\right) }{\partial x}+y_\Delta \left( t\right) } \right] ^{2} \nonumber \\&-\,\frac{1}{4}\beta _2 \left[ {w_r \left( L_b, t\right) +L_b \frac{\partial y\left( L_0,t\right) }{\partial x}+y_\Delta \left( t\right) } \right] ^{4}\nonumber \\ \end{aligned}$$

(11)

in which $V_{m0} \,{=}\,E(D,0)$ is the potential energy when the system is undeformed. Combining Eq. (5), the total energy is

$$\begin{aligned} V=V_p +V_b +V_v +V_c +V_m +V_{st0} \end{aligned}$$

(12)

where $V_{st0}$ is an artificial parameter added in the equation that makes the total potential energy zero when NES is positioned at its stable equilibrium at rest. One can obtain $V_{\mathrm{st0}}$ by making $V(t\rightarrow \infty )=0$. For a certain absorber, $V_{st0}$ is a unique constant. If there is only one stable equilibrium, $V_{st0} \equiv 0$.

This paper adopts the assumed-modes expansion method to investigate the nonlinear dynamics of monostable/ bistable NES. With the separation of variables, displacements are assumed to be

$$\begin{aligned}&y\left( x,t\right) =\sum _i^{n_s} {Y_i \left( x\right) \phi _i \left( t\right) } ={\varvec{\upphi }}^{T}{} \mathbf{Y} \nonumber \\&w_r \left( x,t\right) =\sum _{i=1}^{n_w} {W_{ri} \left( x\right) \eta _i \left( t\right) } ={{\varvec{\upeta }}}^{T}{} \mathbf{W}_r \nonumber \\&u_b \left( x,t\right) =\sum _{i=1}^{n_b} {U_{bi} \left( x\right) \xi _i \left( t\right) } ={{\varvec{\upxi }}}^{T}{} \mathbf{U}_b \nonumber \\&u_c \left( x,t\right) =\sum _{i=1}^{n_c} {U_{ci} \left( x\right) \alpha _i \left( t\right) } ={{\varvec{\upalpha }}}^{T}{} \mathbf{U}_c \end{aligned}$$

(13)

in which $Y_i (x), W_{ri} (x), U_{bi} (x)$ and $U_{ci} (x)$ are modal shape functions, and $\phi _i (t), \eta _i (t), \xi _i (t)$ and $\alpha _i (t)$ are the corresponding time functions of displacements. Then, letting $Y_{\Delta i} =Y_i (L_0 )-Y_i (L_m)$, one obtains $y_\Delta (t)=\sum _i^{n_s} {Y_{\Delta i} \phi _i(t)}$.

Assuming that the distributed transverse loads $f_{p}(x, t)$ are applied on the P-beam and that $f_{n}(x, t)$ is applied on the S-beam, the generalized forces on the P-beam and S-beam can be calculated using

$$\begin{aligned} \mathbf{F}_p \left( t\right)= & {} \int _0^L {f_p \left( x,t\right) \mathbf{Y}\left( x\right) } {\text{ d }}x \nonumber \\ \mathbf{F}_n \left( t\right)= & {} \int _0^{L_b} {f_n \left( x,t\right) \mathbf{W}_r \left( x\right) } {\text{ d }}x \end{aligned}$$

(14)

Subscript ‘n’ represents the NES or linear absorber. Usually, the external loads applied on the S-beam are zero.

The motion equations of the system can be set up with the Lagrange equations

$$\begin{aligned} \frac{{\text{ d }}}{{\text{ d }}t}\left( {\frac{\partial T}{\partial {\dot{q}}_i}} \right) -\frac{\partial T}{\partial q_i }+\frac{\partial V}{\partial q_i }=F_i, \quad i=1,2,\ldots \end{aligned}$$

(15)

in which $q_i$ denotes the generalized modal coordinates in (13) and $F_i$ represents the generalized force in (14). Substituting Eq. (13) into Eqs. (7) and (12) gives the total kinetic energy and potential energy. Then, we can calculate the motion differential function of the nonlinear system

$$\begin{aligned}&\mathbf{M}_{\mathrm{e}} {\ddot{\mathbf{p}}}\left( t\right) +\mathbf{K}_{\mathrm{e}} \mathbf{p}\left( t\right) \nonumber \\&\quad -\, \beta _2 \left[ {w_r \left( L_b, t\right) +L_b \frac{\partial y\left( L_0, t\right) }{\partial x}+y_\Delta \left( t\right) } \right] ^{3}{} \mathbf{P}={} \mathbf{F}_{\mathrm{e}} \end{aligned}$$

(16)

$$\begin{aligned}&\begin{array}{cccc} \mathbf{p}\left( t\right) =\Big [{\varvec{\upphi }}^\mathrm{T}&{{\varvec{\upeta }}}^\mathrm{T}&{{\varvec{\upxi }}}^\mathrm{T}&{{\varvec{\upalpha }}}^\mathrm{T}\Big ]^\mathrm{T} \end{array}\nonumber \\&\mathbf{F}_{\mathrm{e}} =\left[ {{ \begin{array}{cccc} {\mathbf{F}_p \left( t\right) ^\mathrm{T}}&{} {\mathbf{F}_n \left( t\right) ^\mathrm{T}}&{} 0&{} 0 \\ \end{array}}} \right] ^\mathrm{T} \end{aligned}$$

(17)

In Eq. (16), $\mathbf{M}_{\mathrm{e}}$ and $\mathbf{K}_{\mathrm{e}}$ are the generalized mass and complex stiffness matrices, respectively. $\mathbf{F}_{\mathrm{e}}$ is the generalized excitation force vector. Subscript ‘e’ represents the ‘entire’ system. $\mathbf{p}(t)$ is the time function array of the displacement. $\mathbf{P}$ is an array that represents the influence of nonlinear elements.

Both $\mathbf{M}_{\mathrm{e}}$ and $\mathbf{K}_{\mathrm{e}}$ are symmetrical block matrices, as listed in Appendix. $\mathbf{M}_{c\phi \phi }$ represents the coupling effect. The linear part of the magnetic force is exhibited in the matrices $\mathbf{K}_{\phi \eta }$ and $\mathbf{K}_{\eta \eta }$. If the bistable NES was not coupled to an elastic beam but was fixed on a rigid plane, all the terms relevant to y(x, t) in the equations above are zero. In this situation, the motion differential equation for the standalone NES can be written as

$$\begin{aligned}&\mathbf{M}_{\mathrm{n}} {\ddot{\mathbf{q}}}\left( t\right) +\mathbf{K}_{\mathrm{n}} \mathbf{q}\left( t\right) -\beta _2 w_r^3 \left( L_b, t\right) \mathbf{P}_{\mathrm{n}} =\mathbf{F}_{\mathrm{n}} \left( t\right) \nonumber \\&\mathbf{q}\left( t\right) =\left[ {{\varvec{\upeta }}}^\mathrm{T}\quad {{\varvec{\upxi }}}^\mathrm{T}\quad {{\varvec{\upalpha }}}^\mathrm{T}\right] ^\mathrm{T} \end{aligned}$$

(18)

$\mathbf{M}_{\mathrm{n}}$ and $\mathbf{K}_{\mathrm{n}}$ are also presented in Appendix.

2.4 Modal shape functions

Modal shape functions can be determined by boundary conditions. The P-beam is considered to be a cantilever beam whose modal shape functions are

$$\begin{aligned} Y_i (x)= & {} \cos g_i x-\cosh g_i x \nonumber \\&+\, \frac{\sin g_i L-\sinh g_i L}{\cos g_i L+\cosh g_i L}(\sin g_i x-\sinh g_i x)\nonumber \\ \end{aligned}$$

(19)

with the eigenfunction $\cos gL\cosh gL\,{=}\,-1$. And $i=1,2\ldots n_p$. Other beams under different boundary conditions can be considered by choosing proper modal shape functions. For the S-beam in the absorber, the modal shape function of the transverse relative motion has the same form as (19) except the length is $L_b$. Its modal shape function of longitudinal motion is

$$\begin{aligned} U_b (x)=\sin \frac{(2i-1)\pi x}{2L_b },\quad i=1,2\ldots n_b \end{aligned}$$

(20)

The constrained layer is considered as a fee-free rod; therefore,

$$\begin{aligned} U_c (x)=\cos \frac{(i-1)\pi x}{x_2 -x_1 },\quad i=1,2\ldots n_c \end{aligned}$$

(21)

2.5 Damping transfer and definitions of TET efficiencies

Based on Eq. (16), for the linear system $\beta _1 \,{=}\,\beta _2\,{=}\,0$, the steady response formula under an excitation $\mathbf{F}_{\mathrm{e}}={\bar{{\mathbf{F}}}}_{\mathrm{e}} e^{i\omega t}$ is $\left[ {\mathbf{K}_\mathbf{e} -\omega ^{2}{} \mathbf{M}_{\mathrm{e}}} \right] {\bar{{\mathbf{p}}}}={\bar{{\mathbf{F}}}}_{\mathrm{e}}$. Then

$$\begin{aligned} {\bar{{\mathbf{p}}}}=\left[ {\mathbf{K}_\mathbf{e} -\omega ^{2}{} \mathbf{M}_{\mathrm{e}}} \right] ^{-1}{\bar{{\mathbf{F}}}}_{\mathrm{e}} \end{aligned}$$

(22)

substituting (22) into (13) provides the analytical solution for the steady response of the linear system. By comparing the analytical results with the linear finite element (FE) results, one can determine the proper DOF numbers for this research: being $n_\mathrm{p}\,{=}\,5, n_\mathrm{w}\,{=}\,6, n_\mathrm{b}\,{=}\,n_\mathrm{c}=2$. This part of the content is presented in Supplementary material. The results also validate the accuracy of Eq. (22).

However, for the nonlinear NES that $\beta _1 \,{\ne }\,0,\beta _2 \,{\ne }\,0$, the solutions for both transient and steady responses become difficult because the DOF numbers are much larger than that of the 2DOF discrete oscillators. Numerical methods are adopted to solve the responses from the ordinary differential equations in (16) and (18). Because the imaginary part in the matrix $\mathbf{K}_\mathbf{e}$ makes the numerical integration become non-convergent, we transform the structure damping model into a viscous damping model with an equivalent method. Decomposing the stiffness matrices into real and imaginary parts,

$$\begin{aligned} \mathbf{K}_{\mathrm{e}} =\mathbf{K}_{\mathrm{er}} +i\cdot \mathbf{K}_{\mathrm{eI}}, \quad \mathbf{K}_{\mathrm{n}} =\mathbf{K}_{\mathrm{nr}} +i\cdot \mathbf{K}_{\mathrm{nI}} \end{aligned}$$

(23)

The imaginary part represents the damping effect. Under 1:1 resonance, one obtains

$$\begin{aligned} i\cdot \mathbf{K}_{\mathrm{eI}} \mathbf{p}(t)=\frac{1}{\omega }\mathbf{K}_{\mathrm{eI}} {\dot{\mathbf{p}}}(t) \end{aligned}$$

(24)

Although the equivalent method (24) is under 1:1 resonance, it is also a valid approximation in other regimes. Under transient impact excitations, the system responses are mainly about the first-order natural frequency though higher-order modal responses still exist. Thus, one can replace the $\omega$ in Eq. (24) with the first natural frequency $\omega _{s1}$ of the P-beam. Defining the total damping matrix as $\mathbf{C}_{\mathrm{e}} \,{=}\,{\mathbf{K}_{\mathrm{eI}}}/{\omega _{s1}}$, then the motion equation can be expressed as

$$\begin{aligned}&\mathbf{M}_{\mathrm{e}} {\ddot{\mathbf{p}}}(t)+\mathbf{C}_{\mathrm{e}} {\dot{\mathbf{p}}}(t)+\mathbf{K}_{\mathrm{er}} \mathbf{p}(t) \nonumber \\&\quad -\,\beta _2 \left[ {w_r (L_b, t)+L_b \frac{\partial y(L_0, t)}{\partial x}+y_\Delta (t)} \right] ^{3}{} \mathbf{P}=\mathbf{F}_{\mathrm{e}}\nonumber \\ \end{aligned}$$

(25)

A similar treatment to Eq. (18) obtains

$$\begin{aligned} \mathbf{M}_{\mathrm{n}} {\ddot{\mathbf{q}}}(t)+{} \mathbf{C}_{\mathrm{n}} {\dot{\mathbf{q}}}(t)+{} \mathbf{K}_{\mathrm{nr}} \mathbf{q}(t)-\beta _2 w_r^3 (L_b, t)\mathbf{P}_{\mathrm{n}} ={} \mathbf{F}_{\mathrm{n}} (t)\nonumber \\ \end{aligned}$$

(26)

Thereafter, the response of the nonlinear system can be accurately solved with the solver ode23t in MATLAB.

Simplifying expressions in (7) and (12), we obtain

$$\begin{aligned} T= & {} \frac{1}{2}{\dot{\mathbf{p}}}(t)^\mathrm{T}{} \mathbf{M}_{\mathrm{e}} {\dot{\mathbf{p}}}(t) \nonumber \\ V= & {} \frac{1}{2}{} \mathbf{p}(t)^\mathrm{T}{} \mathbf{K}_{\mathrm{er}} \mathbf{p}(t)-\frac{1}{4}\beta _2 \Delta ^{4}+V_{st0} \end{aligned}$$

(27)

The energy dissipated by the whole structure $E_{ed}$ and by absorber $E_{nd}$ in the time interval $(t_{0}, t)$ is

$$\begin{aligned} E_{ed} (t)= & {} \int _{t_0 }^t {{\dot{\mathbf{p}}}(\tau )^\mathrm{T}{} \mathbf{C}_{\mathrm{e}} {\dot{\mathbf{p}}}(\tau )} {\text{ d }}\tau \nonumber \\ E_{nd} (t)= & {} \int _{t_0 }^t {{\dot{\mathbf{q}}}(\tau )^\mathrm{T}{} \mathbf{C}_{\mathrm{n}} {\dot{\mathbf{q}}}(\tau )} {\text{ d }}\tau \end{aligned}$$

(28)

Therefore, the percentage of total impulse energy dissipated by the absorber (or energy dissipation percentage) can be defined as

$$\begin{aligned} r_{dn} ={E_{nd}}/{(E_{ed}}+V_{st0} )\times 100\% \end{aligned}$$

(29)

However, $r_{dn}$ cannot reflect the decay rate of vibration energy that is also an important parameter in practice.

As is well understood, the response of a 1DOF linear oscillator under impact excitation has the form $y(t)=\bar{{A}}\hbox {e}^{-\lambda \omega _n t}\sin (\omega _d t+\theta )$ and $\omega _d =\omega _n \sqrt{1-\lambda ^{2}}$ [41], where $\omega _n$ is the natural frequency and $\lambda$ is the critical damping ratio. For a continuous system, the amplitude follows the same rule. With an analogical method, the total energy of the NES system can be estimated as

$$\begin{aligned} E_{\mathrm{e}} (t)=T+V\approx \bar{{A}}_E \hbox {e}^{-2\lambda \omega _{s1} t}\sin (2\omega _d t+\theta ) \end{aligned}$$

(30)

where $\omega _{s1}$ is the first natural frequency. The term $\hbox {e}^{-2\lambda \omega _{s1} t}$ plays the major role in amplitude decay in Eq. (30). Actually, the total energy can be always fitted with an exponential expression $E_{\mathrm{e}} (t)\,{\approx }\, \bar{{A}}_E \hbox {e}^{-2\lambda \omega _{s1} t}$. Therefore, one can define $\lambda$ as the damping ratio of the entire system that represents the decay rate of the impact energy. Then, the system damping ratio can be accurately evaluated when the responses of the system are calculated. In engineering, not only a large energy dissipation percentage but also high damping ratios are needed to protect the primary system.

2.6 Analysis of stable equilibriums

The stiffness of the absorber will increase after the S-beam is attached with the PCLD and becomes nonlinear when the magnetostatic force is applied at the end of the S-beam. The first-order nonlinear stiffness determines the number of stable equilibriums of the NES.

Fig. 6

Testing scheme and experiment setups

Firstly, the nonlinear NES coupled to a rigid body is considered. Assuming that a constant force $\bar{{f}}(x)$ is applied on point $L_{x}$, then $\mathbf{F}_n (t)\,{=}\,f_n \cdot \mathbf{s}(L_x)$, where $f_n (x,t)\,{=}\,\bar{{f}}(x)\delta (x-L_x)$, and the array $\mathbf{s}(L_x) \,{=}\, [W_{r1}(L_{x}){\ldots }W_{{rn}_w} (L_{x}) 0{\ldots }0]^\mathrm{T}$. By setting ${\ddot{\mathbf{q}}}(t)=0$ in Eq. (26), one obtains ${\bar{{\mathbf{q}}}}=\bar{{f}}\cdot \mathbf{K}_{\mathrm{nr}}^{-1}\mathbf{s}(L_x)$. Therefore, the deflection at point $L_{x}$ is $\bar{{w}}_r =\bar{{f}}\cdot \mathbf{s}^\mathrm{T}{} \mathbf{K}_{\mathrm{nr}}^{-1}{} \mathbf{s}$. The first-order stiffness near the center point is

$$\begin{aligned} {\tilde{k}}_1 =\frac{\bar{{f}}}{\bar{{w}}_r }=\frac{1}{\mathbf{s}^\mathrm{T}{} \mathbf{K}_{\mathrm{nr}}^{-1}{} \mathbf{s}} \end{aligned}$$

(31)

In practice, the NES is fixed to an elastic deformable structure. In this situation, the first-order stiffness $k_1$ of NES is smaller than ${\tilde{k}}_1$. With array P and the same approaches above, the equation is given by

$$\begin{aligned} k_1 =\frac{\bar{{F}}}{\bar{{w}}}=\frac{1}{\mathbf{P}^\mathrm{T}\mathbf{K}_{\mathrm{er}}^{-1}{} \mathbf{P}} \end{aligned}$$

(32)

For a linear structure $\beta _{1}\,{=}\,\beta _{2}\,{=}\,0$, one obtains the stiffness of the linear absorber $k_0$ with Eq. (32), $k_0\,{=}\,k_1 |_{\beta _1 =0}$. For the pure cantilever beam without PCLD, its static stiffness is $k_{p0}\,{=}\,3EI/L^{3}$. Because PCLD increases the stiffness, $k_{p0}$ is smaller than $k_{0}$, and a larger difference would occur for longer PCLD.

For nonlinear structures, the total restoring force for NES is $F_r (t)\,{=}\,k_1 \Delta -\beta _2 \Delta ^{3}$ where $k_1 \,{=}\,k_0 -\beta _1$. Therefore, with specified parameters $k_0$ and $\beta _1$, it is convenient to determine the stable state of NES based on$\;k_1$. As stated before, $\beta _{1}\,{>}\,0$. If $\beta _1 \,{\le }\, k_0, k_1 \,{\ge }\, 0$, although the magnetostatic force has a negative stiffness, the NES has single stable equilibrium that leads to a monostable NES. However, if $\beta _1 \,{>}\,k_0, \;\;k_1 \,{<}\,0$, there will be three equilibriums on the occasion a bistable NES is obtained: the center unstable point and the other two stable equilibriums.

3 Experiments and comparisons

3.1 Experiment setups and parameters

The laser vibrometer Polytec-PSV-500 is used to measure the transient responses of the system. The testing scheme and experiment setup are illustrated in Fig. 6. Three vibrometers are employed to measure the vibration velocities at the three points, A, B and C, simultaneously. Point A is positioned at the end of the P-beam, point B is next to the endpoint of the S-beam, and point C is fixing point $\hbox {O}_{1}$. The distance between B and C is 0.07 m. The modal hammer-2302 with a rubber head is used to impact the P-beam at point A. The hammer’s sensitivity is 2.5 mV/N.

Table 1 Experiment parameters

The experiment parameters are listed in Table 1. Other parameters are as follows: the complex modulus of the viscoelastic layer $G_\mathrm{v}\,{=}\,3(1+0.1\hbox {i})\hbox { MPa}$; the position of the fixing point $L_{0}\,{=}\,{\vert }\hbox {OO}_{1}{\vert }\,{=}\,0.19\hbox { m}$, as shown in Fig. 1; and the position of PCLD $x_{1}\,{=}\,0, x_{2}\,{=}\,0.03\hbox { m}$, as shown in Fig. 3. The masses and positions of the two struts are $m_{p1}=0.005\hbox { kg}$ and $L_{x1}=L_{0}$ and $m_{p2}\,{=}\,0.016\hbox { kg}$ and $L_{{x2}}\,{=}\,L_\mathrm{0}+L_{\mathrm{n}}$. With these parameters, we can calculate the stiffness of the linear absorber ${\tilde{k}}_0 \,{=}\,454.47 \hbox { N/m}$ and $k_0 \,{=}\,453.26 \hbox { N/m}$. The end mass of the absorber is $m_{t}=0.0156\hbox { kg}$, $R_{1}\,{=}\,0.0075\hbox { m}, R_{2}\,{=}\,0.005\hbox { m}, d_{1}\,{=}\,0.012\hbox { m}, d_{2}\,=\,0.004\hbox { m}$ and $d\,{=}\,0.03\hbox { m}$, as illustrated in Fig. 3. The saturation magnetizations are $M_{1}=M_{2}\,{=}\,12.6\times 10^{5}\hbox { A/m}$.

3.2 Transient response of the cantilever beam coupled to a bistable NES

Before moving on to the nonlinear system containing bistable NES, three impact experiments were carried out on the pure P-beam, the P-beam coupled to a linear absorber and the P-beam coupled to a traditional monostable NES (Test-1 in Table 2), respectively. These experiments were conducted to verify the theoretical model for a linear and monostable NES under complex inputs and also to validate these parameters in part 3.1. Because the tested hammer impact forces contain a ‘double hit,’ the test forces were directly used as the input signals in the theoretical simulations to achieve a better comparison between the tests and theoretical results. These results are presented in Supplementary Materials.

With the same $k_0$, the modulation of distance D generates different nonlinear dynamic properties. Additional parameters of the tests are provided in Table 2. The first test is monostable NES, and the second and the third tests are bistable NESs with different first-order stiffness $k_{1}$. The fourth-order complex Gaussian wavelets are adopted in wavelet transforms (WTs).

Table 2 Additional experimental parameters for nonlinear restoring force

Fig. 7

Transient response of the system in Test-2. a Response at point A; b response at point B; c phase portraits of point B

Fig. 8

Wavelet transforms of the response displacements in Test-2. a Theoretical results and b experimental results

Figures 7 and 8 illustrate the transient responses of the system in Test-2 under the impact amplitude of 40 N including the ‘double hit’ effects (as seen in the small iconography in Fig. 7b. As shown in Fig. 7a, b, the experimental and theoretical results are consistent in the initial stage; in the consequent motion, although they have differences in certain time ranges because of the frequency difference, the oscillating trends in the time domain and WTs are the same. A high-order response appears in the continuous NES, but it is not the major component. As seen in the theoretical phase diagram of point B, the distance between the two symmetrical stable equilibriums is 8.4 mm. However, the un-centering installation errors in the experiments cause the experimental phase diagram of point B to be asymmetrical. The bistable NES generates two jumps from one stable equilibrium to another one with a subharmonic frequency. This paper defines this phenomenon as the stable state transition. Combining the results of WTs, the high-amplitude transition generates a 1:2 subharmonic nonlinear beating (during 0–0.3s) that results in highly efficient TET with a simultaneously rapid dissipation of energy. After the transition, motions of bistable NES are captured by one stable equilibrium. Additionally, vibrations in the primary system are largely weakened, and the 2:3 subharmonic resonance capture takes places to transfer energy to NES continuously. The subharmonic nonlinear beating observed in experiments is different from the high-frequency nonlinear beating occurring in traditional monostable NES.

In Test-3, both ${\vert }k_{1}{\vert }$ and ${\vert }\beta _{2}{\vert }$ are increased, but the wave of the impact force is similar to that of Test-2. As shown in Fig. 9a–c and Fig. 10a, b, the theoretical results exactly depict the vibration attenuation laws in this experiment. In (a) (b) (c) Figure 9c, the un-centering error still exists in the measurements. In this case, the distance between the two stable equilibriums increases to 13.8 mm, while the energy needed for the absorber to transition from one stable state to another one also increases. Therefore, the bistable NES in Test-3 only generates one transition under a similar impact. In the WTs plots, obvious components lower than 10 Hz during 0–0.2 s caused by the nonzero mean value rather than the vibration are observed. After a transient 1:1 resonance capture, energy localized in NES increases enough to drive the NES to transition and simultaneously bring a strong nonlinear beating following the 2:3 subharmonic orbit. During 0.3–1 s, continuous nonlinear beatings take place following the 1:1 resonance orbit. These nonlinear beatings generate highly efficient TET to suppress the vibration of the primary structure.

The analyses above indicate that the theoretical model established for continuous NES coupled to the elastic beam can accurately describe the dynamics of a linear system, monostable NES and bistable NES under complex impacts.

Fig. 9

Transient response of the system in Test-3. a Response at point A; b response at point B; c phase portraits of point B

Fig. 10

Wavelet transforms of the response displacements in Test-3. a Theoretical results and b experimental results

4 TET efficiency and mechanisms

In this section, the TET efficiencies and TET mechanisms of bistable NES are discussed based on the theoretical model. Analysis of a large number of impact force signals generated by a hammer revealed that the width of a pulse is approximately 0.004 s. Therefore, the half sinusoidal pulses with a constant width of 0.004 s are used to simulate the impact forces on the P-beam.

4.1 TET efficiency of different absorbers

The TET efficiencies $r_{dn}$ and $\lambda$ of the linear absorber are independent on the input pulse. By modulating the elastic modulus $E_{\mathrm{b}}$ of the S-beam, TET efficiencies with different stiffness $k_{\mathrm{s0}}$ are illustrated in Fig. 11. The linear absorber is sensitive to the structure parameters, leading to poor robustness. Moreover, the parameter $E_\mathrm{b} \,{=}\,70\hbox { GPa}$ in the experiments in Sect. 3 is demonstrated to be the optimal choice for a linear structure ($r_{dn} \,{=}\,66\%, \lambda \,{=}\,0.016)$ attached with a certain PCLD.

Fig. 11

TET efficiencies of linear absorbers with different stiffness $k_\mathrm{s0}$

To explore the TET efficiency of an NES, a group of simulations are performed, as listed in Table 3. The structure parameters in Group 1 are identical with the parameters in the experiments. In the following contents, the numbers are labeled Gi-S-Nj and Gi-Bi-Nj. ‘Gi’ denotes the $i\hbox {th}$ Group, ‘S-N’ denotes the traditional monostable NES, and ‘Bi-N’ represents the bistable NES.

Table 3 Parameters of restoring forces in Group 1

For bistable NES, there are two cases with opposite force directions, as shown in Fig. 12a. Taking G1-Bi-N2 as an example, Fig. 12b expatiates on the influence of force directions on the TET efficiencies. Both cases exhibit the sensitivity to the amplitudes of pulses, and TET efficiencies follow the same variation rule as a whole. When 1$<\bar{{F}}\le 5\hbox {N}$, case II has higher $r_{dn}$ and $\lambda$ because the force in this direction excites a more efficient TET mechanism. When $\bar{{F}}>65\hbox {N}$, the variation trends of $r_{dn}$ and $\lambda$ are different; $\lambda$ decreases while $r_{dn}$ maintains a high value as the pulse amplitude increases. The mean value of the two cases is calculated to evaluate the TET efficiencies of bistable NES in the following analyses.

Fig. 12

TET efficiencies of bistable NES in two cases. a Two directions of excitation force and b TET efficiency

TET efficiencies of different NESs in Group 1 are illustrated in Fig. 13. Under an extremely high-energy input ($\bar{{F}}>100\hbox { N}$), deformations of the P-beam and S-beam would exceed the size and linearity limits, which is not considered in this paper. Because of the weak robustness of the linear absorber, 85% of the optimal values ($r_{dn}=56\%, \lambda =0.0136$) are also described. As shown in Fig. 13a, there is a critical threshold of impact amplitude, approximately 60 N, for highly efficient TET for monostable NESs. Under lower impacts, their efficiencies almost maintain constants and are much lower than the linear absorbers. In contrast, bistable NESs do not have the input threshold and can exceed the optimal efficiencies of the linear absorber in a wide impact range through modulating parameters. Moreover, the capacities for vibration attenuation of bistable NESs can be much better than monostable NESs under the middle-to-low amplitudes of impacts. The changing rules of TET efficiencies prove that the variation of $r_{dn}$ is not consistent with that of $\lambda$ under higher-energy input again.

Fig. 13

TET efficiencies of different absorbers in Group 1. a Monostable NES and b bistable NES

Fig. 14

TET efficiencies of absorbers in Group 2

4.2 Influences of structure parameters on TET efficiencies of bistable NES

We can interpret bistable NESs in terms of the influences of parameters on TET efficiencies of linear absorbers. The influences of $G_\mathrm{v}$ and $h_\mathrm{v}$ on the TET efficiencies of linear absorbers are shown in Supplementary Material. From the results, a set of proper parameter $G_\mathrm{v} =6(1+0.1\hbox {i})\hbox { MPa}$ and $h_\mathrm{v}=0.25\hbox { mm}$ (suboptimal values) are adopted for the simulations of the second group of bistable NESs. In this case, $k_{0}=489.16\hbox { N/m}, r_{dn} =74.73\%, \lambda =0.0339$ for linear absorber and $d_{2}=6\hbox { mm}$ for nonlinear ones. The restoring force parameters in Group 2 are listed in Table 4 in Appendix.

The TET efficiencies of the five absorbers are illustrated in Fig. 14. Compared with Group 1, the TET efficiencies of bistable NESs get enhanced with the increase in the shear modulus of the viscoelastic layer and the damping ratios are enhanced much more. Efficiencies of the bistable NES are similar with the optimal linear absorber but are much better than the 85% of the optimal value. Although the stiffness changed remarkably, the efficiencies of the bistable NES remain steady with different impact amplitudes, indicating that an optimized NES has strong robustness.

With the parameters of G2-Bi-N2, influences of the length of PCLD with $x_{1}=0$ are investigated in Group 3. Its parameters are listed in Table 5 in Appendix. The results in Fig. 15 indicate that the PCLD length has important influence. Increasing the length will gain an additional energy dissipation percentage. However, when the length exceeds 30 mm, $r_{dn}$ remains close to the upper limit of 80% although the PCLD length increases greatly. However, continuously increasing the length still significantly improves $\lambda$ under small- and high-amplitude impacts. Moreover, modulating the nonlinear stiffness will improve the damping ratio further.

Fig. 15

TET efficiencies of bistable NESs in Group 3

With the parameters of G2-Bi-N2, the influences of the end mass on TET efficiencies of bistable NES are shown in Fig. 16. The total mass of primary structures is $m_{pt}=m_{p}+m_{p1}+m_{p2}=204.6\hbox { g}$. The mass ratio is defined as $\varepsilon =m_{t}/m_{pt}\times 100\%$. Similar to the monostable NES, there is also a critical threshold for the small end mass continuous bistable NES (see the region $\bar{{F}}<40\hbox {N}$ and $\varepsilon <4\%$). Near $\varepsilon =5\%, r_{dn}$ reaches its optimal value, and there is a peak-value interval of $40<\bar{{F}}<70\hbox {N}$ for $\lambda$. This interval is to the right of the lowly efficient interval in Group 3, indicating that modulating the end mass can further optimize the bistable NES. However, the influences of the end mass are not monotonous; both $r_{dn}$ and $\lambda$ will decrease when $\varepsilon$ increases continuously, and $\lambda$ decreases faster.

Fig. 16

Influences of end mass on TET efficiencies of bistable NES

In brief, the shear modulus of the viscoelastic layer, the PCLD length and the end mass of NES have important influences on the TET efficiencies of bistable NESs, and the system damping ratio is more easily affected.

4.3 TET mechanisms of bistable NES

Because of the strong nonlinearity, the TET mechanisms of bistable NES are much more complicated than that of the monostable NES. In recent publications [21, 22], the main regimes considered in the discrete bistable oscillator are 1:1 and 1:3 resonance captures. Romeo et al. [20] found that the two main mechanisms in a discrete bistable NES are aperiodic (chaotic) cross-well oscillations and in-well nonlinear beats. In this section, nonlinear beating, fundamental and subharmonic resonance capture, and transitions of stable state are analyzed with examples in Group 1.

Under middle and higher-amplitude pulses, TET efficiencies of the bistable NES in Group 1 are steady. In the contents below, the relative displacement of NES denotes the point on the S-beam to be $x=L_{\mathrm{b}}$. The WTs of displacements and the frequency energy plot (FEP) of the relative displacement under $\bar{{F}}=80\hbox { N}$ are illustrated in Fig. 17. Smn and Umn (m and n are integers) are frequency orbits where the main frequency of the NES is $\omega =m\omega _{s0}/ n, \omega _{s0}=60\pi$. This paper decomposes S11 into S11+ and S11- but does not decompose other orbits as [3] did.

Fig. 17

WTs and FEP of G1-Bi-N2 under 80N. a Displacements; b WTs of the displacements; c FEP

As shown in Fig. 17b, TET is initiated by high-frequency nonlinear beating following special orbits U65 to U32. Impact energy is transferred from low-frequency modes to high-frequency modes and is localized in NES. Therefore, the bitable NES gets a highly efficient TET. During 0.1–0.35 s, the mechanisms transform from the fundamental resonance capture along S11+ to subharmonic resonance capture along S23. Then, NES escapes from S23 and generates a steady 1:3 subharmonic resonance capture until there is no enough energy localized in NES to support its transition between two stable equilibriums. During the subharmonic resonance capture, the low-amplitude high-frequency vibration of the primary system drives the bistable NES to generate a high-amplitude low-frequency (HALP) response. Simultaneously, the high strain energy will dissipate the impact energy. The two stable equilibriums attract the bistable NES generating steady transitions with higher amplitudes than the monostable ones.

When the impact energy decreases, the frequency of nonlinear beating is also reduced from high-frequency branches to 1:1 orbits. However, when the pulse amplitudes are lower than the critical threshold of monostable NES, the TET mechanism of monostable NES is 1:1 resonance capture along the orbit S11-, and nonlinear beatings disappear [3]. In contrast, bistable NES can still generate subharmonic responses because of the stable state transition. However, if the motions are captured by one stable state, the mechanisms will change.

Fig. 18

Displacements and WTs of G1-Bi-N2 under 41 N. a Displacements and b WTs of displacements

Taking $\bar{{F}}=41\hbox {N}$ as an example, results are shown in Fig. 18. In the initial stage before 0.1 s, energy transfer is initiated by a transient 1:1 fundamental resonance capture rather than the nonlinear beating. However, during 0.1–0.4 s, the bistable NES behaves as a HALP subharmonic response following S23 to S12; this is not the resonance capture but a long period of subharmonic nonlinear beating with the primary structure, making the energy irreversibly localized in bistable NES. Subsequently, another beating occurs along branch S13. The frequency of monostable NES monotonously decreases with energy. However, for a bistable NES, when the motions are captured by single stable equilibrium after 0.6 s, the responses of NES transfer from orbit S13 to S12, implying that the resonant frequency increases but the energy decreases. Figure 17c also illustrates this abnormal non-monotonic phenomenon.

Fig. 19

WTs of G1-Bi-N2 under case II $\hbox {F}\,{=}\,5\hbox { N}$ (case I $\hbox {F}\,{=}\,-5\hbox { N}$ is presented in Supplementary material)

Under small impacts, the NES motions are captured by single stable equilibrium. This situation is identical with that of the energy in NES attenuates from the transition to being captured by a stable equilibrium as shown above. Taking $\hbox {F}\,{=}\,5\hbox {N}$ as an example, it generates a highly efficient long period of 1:2 subharmonic nonlinear beating, as illustrated in Fig. 19. Because the impact direction has influence on the TET efficiency in this case, $\hbox {F}\,{=}\,-5\hbox {N}$ results in lower efficiencies because the bistable NES under $\hbox {F}\,{=}\,-5\hbox {N}$ only generates a lowly efficient 1:1 resonance capture along orbit S11-, as shown in Fig. S7 See Supplementary Material. Under micro-impacts ($\hbox {F}\le 1\hbox { N}$), bistable NES behaves with similar dynamics with the linear absorber that generates a 1:1 beating along S11-, as shown in Fig. S8 See Supplementary Material. Therefore, different from traditional monostable NES, the orbit S11- is not always a lowly efficient orbit for TET. Furthermore, a 1:1 resonance under low energy may not always exist. It depends on the parameters of NES and impact forces.

The work has analyzed the steady transition behaviors. However, for non-optimized bistable NES, because of the sensitivities to impact force, an unstable transition may appear. As shown in Fig. 20, before 0.5 s, there is 1:1 resonance, but the NES oscillates between a stable equilibrium and the unstable equilibrium. This is the unstable transition. Therefore, under a moderate-to-high impact, if unstable transition occurs in the initial stage of TET, the vibration attenuation efficient will be low. Subharmonic steady transition appears after 0.5 s, and then, the vibration of the primary beam is rapidly suppressed.

Fig. 20

Displacements and phase diagram of G1-Bi-N2 for $\hbox {F}\,{=}\,-43\hbox { N}$

Based on the simulations above, the TET mechanisms of bistable NES are classified below.

5 Conclusions

The investigated continuous system consists of a Bernoulli–Euler beam coupled to a continuous bistable NES. The NES comprises a cantilever beam with PCLD and an end mass controlled by a nonlinear magnetostatic interaction force. Both monostable and bistable NES can be achieved by modulating the nonlinear restoring force. This paper focuses on the transient nonlinear dynamics and TET efficiencies.

• (1) The motion differential equations of the system are established based on the Lagrange equations and assumed-modes expansion method. In addition to the energy dissipation percentage, a new index system damping ratio is proposed for evaluating TET efficiencies. The respective mean values of the two efficiency parameters in two opposite impact directions are calculated. Changing rules of the two parameters are not consistent, especially under large amplitude impacts.

• (2) Impact experiments on the linear absorber, monostable NES and bistable NES are gradually carried out to verify the theoretical model. The transition of the stable state, resonance capture and subharmonic nonlinear beating are observed in experiments. There are also high-order responses.

• (3) The monostable NES has to breakthrough a critical energy threshold to generate highly efficient TET. Bistable NES can achieve highly efficient and strongly robust TET under broad-range impacts. Efficiencies of bistable NESs are similar with the corresponding optimized linear absorber but are much better than the 85% of the optimal value. The impact directions influence the local but not the global properties of TET efficiencies. The shear modulus of the viscoelastic layer, the length of PCLD and the end mass have great influences on the TET efficiencies of bistable NESs, and the system damping ratio is more easily affected.

• (4) TET mechanisms in bistable NES are complex; nonlinear beating that achieves highly efficient irreversible TET can occur in high-frequency, fundamental and long-period subharmonic orbits. Resonance captures featuring fundamental and subharmonic also help achieve rapid energy dissipation. The steady transition of the stable state is an important reason for maintaining high efficiencies. However, an unstable transition may result in low capacity for vibration attenuation. Furthermore, NES frequencies would not decrease monotonously with decreasing energy when motions are captured by a stable equilibrium.

We perform the conclusions about the mechanisms based on abundant numerical results (partially presented in this paper). An analytical proof would also be necessary to prove the results made in this study.

Appendix

The kinetic energies of different parts can be expressed as

$$\begin{aligned}&T_p =\frac{1}{2}\int _0^L {\left[ \rho _p A_p +\sum _i {m_{pi} \delta \left( x-L_{xi} \right) } \right] \left( {\frac{\partial y\left( x,t\right) }{\partial t}} \right) ^{2}{\text{ d }}x}\\&T_b =\frac{1}{2}\int _0^{L_b} {\rho _b A_b \left[ {\left( {\frac{\partial w_r }{\partial t}+{\dot{\varphi }}+x\frac{\partial ^{2}y\left( L_0, t\right) }{\partial x\partial t}} \right) ^{2}+\left( {\frac{\partial u_b }{\partial t}} \right) ^{2}} \right] {\text{ d }}x}\\&T_v +T_c =\frac{1}{2}\left( \rho _v A_v +\rho _c A_c \right) \\&\qquad \quad \qquad \cdot \int _{x_1 }^{x_2} {\left( {\frac{\partial w_r }{\partial t}+{\dot{\varphi }}+x\frac{\partial ^{2}y\left( L_0, t\right) }{\partial x\partial t}} \right) ^{2}{\text{ d }}x} \\&\qquad \quad \qquad +\, \frac{1}{2}\int _{x_1 }^{x_2} {\left( {\rho _v A_v \left( {\frac{\partial u_v }{\partial t}} \right) ^{2}+\rho _c A_c \left( {\frac{\partial u_c }{\partial t}} \right) ^{2}} \right) {\text{ d }}x} \\&T_m =\frac{1}{2}m_t \left( {\frac{\partial w_r \left( L_b, t\right) }{\partial t}+{\dot{\varphi }}+L_b \frac{\partial ^{2}y\left( L_0, t\right) }{\partial x\partial t}} \right) ^{2} \\&\qquad \quad +\,\frac{1}{2}m_t \left( {\frac{\partial u_b \left( L_b, t\right) }{\partial t}} \right) ^{2}+\frac{1}{2}J_t \left( {\frac{\partial ^{2}w_r \left( L_b, t\right) }{\partial x\partial t}} \right) ^{2} \\ \end{aligned}$$

where $J_t$ is the end mass’s moment of inertia relative to the axis oz in Fig. 2b, $J_t ={m_t R_1^2 }/4+{m_t d_1^2 }/{12}$.

Table 4 Parameters of restoring forces in Group 2

Table 5 Parameters for Group 3

The elements in the generalized mass matrix and generalized complex stiffness matrix are listed below.

$$\begin{aligned}&\mathbf{M}_{\mathrm{e}} =\left[ {{ \begin{array}{ccccc} {\mathbf{M}_{\phi \phi } +\mathbf{M}_{c\phi \phi }}&{} {\mathbf{M}_{\phi \eta }}&{} 0&{} 0 \\ {\mathbf{M}_{\phi \eta }^\mathrm{T}}&{} {\mathbf{M}_{\eta \eta }}&{} {\mathbf{M}_{\eta \xi }}&{} {\mathbf{M}_{\eta \alpha }} \\ 0&{} {\mathbf{M}_{\eta \xi }^\mathrm{T}}&{} {\mathbf{M}_{\xi \xi }}&{} {\mathbf{M}_{\xi \alpha }} \\ 0&{} {\mathbf{M}_{\eta \alpha }^\mathrm{T}}&{} {\mathbf{M}_{\xi \alpha }^\mathrm{T}}&{} {\mathbf{M}_{\alpha \alpha }} \\ \end{array}}} \right] \\&\mathbf{K}_{\mathrm{e}} =\left[ {{ \begin{array}{ccccc} {\mathbf{K}_{\phi \phi }}&{} {\mathbf{K}_{\phi \eta }}&{} 0&{} 0 \\ {\mathbf{K}_{\phi \eta }^\mathrm{T}}&{} {\mathbf{K}_{\eta \eta }}&{} {\mathbf{K}_{\eta \xi }}&{} {\mathbf{K}_{\eta \alpha }} \\ 0&{} {\mathbf{K}_{\eta \xi }^\mathrm{T}}&{} {\mathbf{K}_{\xi \xi }}&{} {\mathbf{K}_{\xi \alpha }} \\ 0&{} {\mathbf{K}_{\eta \alpha }^\mathrm{T}}&{} {\mathbf{K}_{\xi \alpha }^\mathrm{T}}&{} {\mathbf{K}_{\alpha \alpha }} \\ \end{array}}} \right] \end{aligned}$$

$$\begin{aligned}&M_{\phi \phi , ij} =\int _0^L {\rho _p A_p Y_i Y_j} {\text{ d }}x+\sum _k {m_{pk} Y_i (L_k )Y_j (L_k )}, \\&\quad i,j=1,2\ldots n_p \\&M_{c\phi \phi , ij} =\int _0^{L_b} {\rho _b A_b [Y_i (L_0 )+xY_i^{\prime }(L_0 )]} \\&\quad \cdot \, [Y_j (L_0 )+xY_j^{\prime }(L_0 )]dx+(\rho _c A_c +\rho _v A_v ) \\&\quad \cdot \int _{x_1 }^{x_2} {[Y_i (L_0 )+xY_i^{\prime }(L_0 )][Y_j (L_0 )+xY_j^{\prime }(L_0 )]} {\text{ d }}x \\&\quad +\, m_t [Y_i (L_0 )+L_b Y_i^{\prime }(L_0 )][Y_j (L_0 )+L_b Y_j^{\prime }(L_0 )], \\&\quad i,j=1,2\ldots n_p \\ \end{aligned}$$

$$\begin{aligned}&\begin{array}{l} M_{\phi \eta , ij} =\displaystyle \int _0^{L_b} {\rho _b A_b [Y_i (L_0 )+xY_i^{\prime }(L_0 )]W_{rj} (x)} {\text{ d }}x \\ \quad +\displaystyle \int _{x_1 }^{x_2} {(\rho _v A_v +\rho _c A_c )[Y_i (L_0 )+\,xY_i^{\prime }(L_0 )]W_{rj} (x){\text{ d }}x} \\ \quad +\, m_t [Y_i (L_0 )+L_b Y_i^{\prime }(L_0 )]W_{rj} (L_b ), \\ \quad i=1,2\ldots n_p, j=1,2\ldots n_w \\ \end{array}\\&M_{\eta \eta , ij} =\int _0^{L_b} {\rho _b A_b W_{ri} W_{rj} {\text{ d }}x}\\&\quad +\int _{x_1 }^{x_2} {(\rho _v A_v +\rho _c A_c )W_{ri} W_{rj} {\text{ d }}x} \\&\quad \cdot \int _{x_1 }^{x_2} {\rho _v A_v \frac{(h_c -h_b )^{2}}{16}{W}_{ri}^{\prime } {W}_{rj}^{\prime } {\text{ d }}x} \\&\quad +\, m_t W_{ri} (L_b )W_{rj} (L_b )+J_t {W}_{ri}^{\prime } (L_b ){W}_{rj}^{\prime } (L_b ),\; \\&\quad i,j=1,2\ldots n_w \\&M_{\eta \xi , ij} =\frac{1}{8}\int _{x_1 }^{x_2} {\rho _v A_v (h_c -h_b ){W}_{ri}^{\prime } U_{bj} {\text{ d }}x}, \\&\quad i=1,2\ldots n_w, j=1,2\ldots n_b \\&M_{\eta \alpha , ij} =\frac{1}{8}\int _{x_1 }^{x_2} {\rho _v A_v (h_c -h_b ){W}_{ri}^{\prime } U_{cj} {\text{ d }}x,\quad } \\&\quad i=1,2\ldots n_w, j=1,2\ldots n_c \\&M_{\xi \xi , ij} =\int _0^{L_b} {\rho _b A_b U_{bi} U_{bj} {\text{ d }}x} \\&\quad +\, \frac{1}{4}\int _{x_1 }^{x_2} {\rho _v A_v U_{bi} U_{bj} {\text{ d }}x} +m_t U_{bi} (L_b )U_{bj} (L_b ), \\&\quad i,j=1,2\ldots n_b \\&M_{\xi \alpha , ij} =\frac{1}{4}\int _{x_1 }^{x_2} {\rho _v A_v U_{bi} U_{cj} {\text{ d }}x}, \\&\quad i=1,2\ldots n_b, j=1,2\ldots n_c \\ \end{aligned}$$

$$\begin{aligned}&M_{\alpha \alpha , ij} =\int _{x_1 }^{x_2} {\left( \rho _c A_c +\frac{1}{4}\rho _v A_v \right) U_{ci} U_{cj} {\text{ d }}x}, \\&\quad i,j=1,2\ldots n_c K_{\phi \phi , ij} =\int _0^L {E_p I_p Y_i^{\prime \prime }Y_j^{\prime \prime }} {\text{ d }}x\\&\quad -\,\beta _1 \left( Y_{\Delta i} +L_b {Y}_{i}^{\prime } \right) \left( Y_{\Delta j} +L_b {Y}_{j}^{\prime } \right) ,\; \\&\quad i,j=1,2\ldots n_p \\&K_{\phi \eta , ij} =-\beta _1 \left( Y_{\Delta i} +L_b {Y}_{i}^{\prime } \right) W_{rj} (L_b ), \\&\quad i=1,2\ldots n_p, j=1,2\ldots n_w \\&K_{\eta \eta , ij} =\int _0^{L_b} {E_b I_b {W}_{ri}^{\prime \prime } {W}_{rj}^{\prime \prime }} {\text{ d }}x+\int _{x_1 }^{x_2} {E_c I_c {W}_{ri}^{\prime \prime } {W}_{rj}^{\prime \prime }} {\text{ d }}x \\&\quad +\int _{x_1 }^{x_2} {\frac{G_v A_v }{h_v^2 }h_t^2 {W}_{ri}^{\prime } {W}_{rj}^{\prime }} {\text{ d }}x-\beta _1 W_{ri} (L_b )W_{rj} (L_b ),\; \\&\quad i,j=1,2\ldots n_w \\&K_{\eta \xi , ij} =-\int _{x_1 }^{x_2} {\frac{G_v A_v }{h_v^2 }h_t {W}_{ri}^{\prime } U_{bj}} {\text{ d }}x, \\&\quad i=1,2\ldots n_w, j=1,2\ldots n_b \\&K_{\eta \alpha , ij} =\int _{x_1 }^{x_2} {\frac{G_v A_v }{h_v^2 }h_t {W}_{ri}^{\prime } U_{cj}} {\text{ d }}x,\\&\quad i=1,2\ldots n_w, j=1,2\ldots n_c \\&K_{\xi \xi , ij} =\int _0^{L_b} {E_b A_b {U}_{bi}^{\prime } {U}_{bj}^{\prime }} {\text{ d }}x+\int _{x_1 }^{x_2} {\frac{G_v A_v }{h_v^2 }U_{bi} U_{bj}} {\text{ d }}x,\; \\&\quad i,j=1,2\ldots n_b \\&K_{\xi \alpha , ij} =-\int _{x_1 }^{x_2} {\frac{G_v A_v }{h_v^2 }U_{bi} U_{cj}} {\text{ d }}x, \\&\quad i=1,2\ldots n_b, j=1,2\ldots n_c \\&K_{\alpha \alpha , ij} =\int _{x_1 }^{x_2} {E_c A_c {U}_{ci}^{\prime } {U}_{cj}^{\prime }} {\text{ d }}x+\int _{x_1 }^{x_2} {\frac{G_v A_v }{h_v^2 }U_{ci} U_{cj}} {\text{ d }}x, \\&\quad i,j=1,2\ldots n_c \end{aligned}$$

$$\begin{aligned}&P_i =\left\{ {\begin{array}{l} (Y_{\Delta i} +L_b {Y}_i^{\prime } )\quad \quad \quad for\;\;\;i=1,2\ldots n_p \\ W_{ri} (L_b )\quad \;for\;\;\;i=n_p +1,\ldots n_p +n_w \\ 0\quad \quad others \\ \end{array}} \right. \\&\mathbf{M}_{\mathrm{n}} =\left[ {{ \begin{array}{ccc} {\mathbf{M}_{\eta \eta }}&{} {\mathbf{M}_{\eta \xi }}&{} {\mathbf{M}_{\eta \alpha }} \\ {\mathbf{M}_{\eta \xi }^\mathrm{T}}&{} {\mathbf{M}_{\xi \xi }}&{} {\mathbf{M}_{\xi \alpha }} \\ {\mathbf{M}_{\eta \alpha }^\mathrm{T}}&{} {\mathbf{M}_{\xi \alpha }^\mathrm{T}}&{} {\mathbf{M}_{\alpha \alpha }} \\ \end{array}}} \right] , \\&\mathbf{K}_{\mathrm{n}} =\left[ {{ \begin{array}{ccc} {\mathbf{K}_{\eta \eta }}&{} {\mathbf{K}_{\eta \xi }}&{} {\mathbf{K}_{\eta \alpha }} \\ {\mathbf{K}_{\eta \xi }^\mathrm{T}}&{} {\mathbf{K}_{\xi \xi }}&{} {\mathbf{K}_{\xi \alpha }} \\ {\mathbf{K}_{\eta \alpha }^\mathrm{T}}&{} {\mathbf{K}_{\xi \alpha }^\mathrm{T}}&{} {\mathbf{K}_{\alpha \alpha }} \\ \end{array}}} \right] \end{aligned}$$