Introduction

Research on teaching practices related to mathematics has focused on identifying types of knowledge or beliefs that affect teaching practice (e.g., Hill et al. 2008a; Thompson 1984; Tzur 2010) or explaining how changes in teacher knowledge or beliefs relate to effective teaching (e.g., Leikin and Zazkis 2010; Liljedahl et al. 2007). Researchers have also attended to mathematical knowledge or beliefs teachers use in practice (e.g., Askew et al. 1997; Borko et al. 1992; Ma 1999; Sullivan 2008; Simon 1993; Thompson 2015) and found that some knowledge and beliefs have positively influenced teachers’ enactment of effective practices (Ball et al. 2001; Hill et al. 2005). Teachers’ enactment of instructional practices is also affected by their diagnoses of students’ learning behaviors during a lesson, with the diagnoses informing teachers’ subsequent instructional decisions (Klug et al. 2013). This study builds on the body of research about teacher characteristics that affect instructional decisions for effective teaching, by specifying two aspects of teacher’s knowledge and beliefs that influence effective teaching practices.

As we observed teachers plan lessons and use a task with their students, we noticed teachers struggling to identify and differentiate between the knowledge students bring to the classroom and the knowledge students were to learn. Teacher difficulties appeared to be related to how they conceived students’ prior knowledge and how they conceived the potential of mathematical tasks for new learning. We also noticed that the teachers tended to put all the ideas to solve a given task on the same level. This implies how challenging it is for teachers to transform a mathematical task into an effective mathematics lesson.

Teaching for understanding relies on teachers’ understanding of students’ mathematical thinking and effective classroom implementation of mathematical tasks (e.g., Carpenter et al. 1996, 1999; Stein et al. 2007; Watson and Ohtani 2015). Among many aspects of these two characteristics, this study investigates teacher conceptions of students’ prior knowledge and teacher conceptions of mathematical tasks with the following questions:

  1. 1.

    In what ways do teachers conceive students’ prior knowledge and how do their conceptions shape teaching?

  2. 2.

    In what ways do teachers conceive the potential of a task for helping students develop new knowledge and how do these conceptions shape teaching?

  3. 3.

    How does the relationship between teacher conceptions of prior knowledge and the mathematical potential of a task shape their teaching?

Background and conceptual framework

Research has shown that teaching practices are significantly influenced by teachers’ beliefs related to mathematics, teaching, and learning (Askew et al. 1997; Calderhead 1996; Clark and Peterson 1986; Cohen 1990; Polly et al. 2013, 2014; Swan 2006; Thompson 1984, 1992). In contrast, research linking teaching practices to teachers’ mathematical knowledge has been problematic (Askew 2008). In some cases, researchers found effective instruction despite teachers’ lack of mathematical knowledge (Askew and Brown 1997; Bennett and Carre 1993; Brown et al. 1998), while Hill et al. (2008b) illustrated cases of strong mathematical knowledge with both high-quality and low-quality instructions. Consequently, this study furthers the research on teaching practices by investigating a combination of teachers’ mathematical knowledge and beliefs, defined as teachers’ conceptions (Philipp 2007; Thompson 1992).

Two major characteristics underscore effective mathematics teaching: teachers’ understanding of students’ mathematical thinking and teachers’ abilities to implement mathematical tasks while maintaining the cognitive demand (e.g., Carpenter et al. 1996; Stein et al. 2007). The present study is grounded in research on characteristics of effective mathematics teaching that pursues conceptual understanding. In light of this, we highlight two aspects of teacher conceptions: teacher conceptions of their students’ prior knowledge (PK) and teacher conceptions of the potential of a task for learning (TP). We also investigate how these conceptions affect instructional decisions. Teachers’ conception of PK is a combination of their knowledge of students’ mathematical thinking and their beliefs about learning mathematics as it relates to previously learned knowledge. Teachers’ conception of TP is a combination of teachers’ knowledge of tasks and their beliefs about teaching mathematics as it relates to the mathematics involved in tasks.

Teachers’ conceptions of students’ prior knowledge

Researchers have described the relationship between prior knowledge and new knowledge in some cases as affordances (e.g., Bishop et al. 2014; Steffe 2002) and in other cases as obstacles (Brousseau 1997). Schwartz et al. (2007) further contrasted the role of prior knowledge in learning by describing it in terms of commensurable versus incommensurable. When prior knowledge and new knowledge are commensurable, new knowledge can be explained in the same rational system that supports the prior knowledge. Incommensurability arises when learning new knowledge requires learners to change their understanding of the system that was satisfactory for prior knowledge. These contrasting perspectives on the relationships between prior knowledge and new knowledge suggest that there may be various ways in relating them when it comes to teaching.

Since new knowledge is built on prior knowledge, instruction should be designed to make contact with prior knowledge. But Schwartz et al. (2007) expressed concern about the effectiveness of instruction when students’ prior knowledge is incommensurable for the new knowledge. Gibson (1986) also questioned what might happen if students did not perceive their prior knowledge as affordances. These concerns are important for teachers because how teachers conceive students’ prior knowledge affects the focus of their instruction (Schwartz et al. 2007). Teachers who conceive prior knowledge as commensurables would be likely to provide learning resources that support using prior knowledge, whereas teachers who conceive prior knowledge as incommensurables would focus on helping students develop earlier forms of knowledge so that students are prepared to learn the mathematics targeted. Heinz et al. (2000) reported on a teacher whose conception of prior knowledge led her to focus on whether her students had the specific knowledge that she saw as necessary for the next step in her trajectory but not on the nature of the knowledge that they had. This implies that studying teachers’ conception of PK could help us understand their practice.

Teachers’ conception of PK is related to their beliefs about students and how they learn (Askew et al. 1997). For example, teachers who believe students need to be ready before they can learn mathematical ideas (discovery beliefs) would be likely to take students’ prior knowledge as ready to use; teachers who believe students’ strategies should be refined through instruction (connectionist beliefs) would be likely to take students’ prior knowledge as being in progress. Since teachers’ knowledge of students includes their ability to recognize the preconceptions and background knowledge that students bring to classrooms (NBPTS 2001), teachers’ conception of PK overlaps their knowledge of students. Furthermore, teachers’ conception of PK is related to knowledge of content and students (KCS), defined as teachers’ understanding of students’ learning (Hill et al. 2008). The constituents of KCS are not yet well understood, but teachers may develop important aspects of KCS working with students (Hill et al. 2008). In this study, we see teachers’ conception of PK as the knowledge that teachers use and develop while teaching. Thus, studying teachers’ conception of PK may shed light on an important aspect of teacher knowledge that affects teachers’ instructional decisions.

Consistent with the assumption that “fundamental change in teaching requires growth in teachers’ conceptions of mathematics and learning” (Simon and Schifter 1991, p. 312), we assume that teachers’ conceptions of PK can allow us to explain why teachers have often maintained traditional perspectives of mathematics teaching while adopting reform-oriented strategies in practice (e.g., Cohen and Ball 1990; Hiebert and Stigler 2000; Peterson 1990; Simon and Schifter 1993). In addition, we agree that teachers develop their own theories of learning as a base for instructional decision (Simon and Schifter 1991). We expect this study of teachers’ conceptions of PK to contribute to such theory building by clarifying how teachers may listen to students’ ideas and understandings in order to build models of their development. Thus, in this study we consider two possible teachers’ conceptions of PK to better understand their instructional decisions: (1) conceiving students’ prior knowledge as to-be-developed or (2) conceiving students’ prior knowledge as no-need-to-be-developed.

Teachers’ conceptions of the potential of tasks for new knowledge

One aspect of mathematical knowledge for teaching is teachers’ ability to notice new knowledge embedded in a mathematical task (Hill et al. 2008). Recognizing this mathematical potential is also specialized content knowledge (Sullivan 2008) in that it requires teachers to appraise and modify tasks for their learning goals. In a study on tasks for teachers, Sullivan (2008) asked teachers “If you developed a lesson based on this idea, what mathematics would you hope that students would learn?” (p. 5). He found that some teachers had difficulty articulating what they saw as the content for student learning. Our observation of the participating teachers was consistent with Sullivan’s findings.

Researchers have investigated reasons for the tendency of teachers to reduce students’ high-level engagement in tasks (e.g., Charalambous 2008; Desforges and Cockburn 1987; Tzur 2008). In particular, Henningsen and Stein (1997) identified classroom-based factors that contributed to the decline of tasks and found three patterns of decline: using procedures without connection to concepts and understanding, unsystematic exploration, and no mathematical activity. Removing mathematical challenges from the task was one of the significant factors that influenced decline. The removal of mathematical challenges relates to teachers’ goals for using the task and to their beliefs about the potential of the task for student learning. For example, if teachers intend to use the task to help students develop new knowledge, they would be less likely to allow the cognitive demand to decline. Likewise, if teachers believe students must learn mathematical concepts before using them, they would not see the potential of a task for developing new knowledge. This would likely lead to instruction that revisits what has been taught when students encounter challenges.

In order to learn by engaging in a task, students should experience a transformation in what they attend to mathematically. Realizing how complex teacher knowledge must be to make such learning possible, Watson and Mason (2007) argued that first the tasks must be real to teachers in the context of teaching. We see tasks being real to teachers when they become aware of mathematical tasks as central to students’ learning (NCTM 1991). This awareness is related to how teachers conceive the potential of a task for developing new knowledge, a conception that is critical to their instructional decisions. For instance, if a teacher identifies the mathematics in a given task as having no potential for new knowledge, she would be likely to use the task as an exercise to which students can directly apply what they already learned. But when the teacher conceives the given task as having potential for new knowledge, she would be likely to help students learn new knowledge. In this study, we identify and explicate two teacher conceptions of a task in terms of its mathematical potential: (1) a task as involving potential for students to learn new knowledge or (2) a task as involving no potential for developing new knowledge. Teachers with the second conception may reason that the task has no potential for students to develop new knowledge because they see previously learned procedures as transferable or as generalizable.

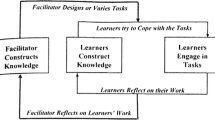
We recognize that in their teaching practices, teachers draw on their conceptions of prior knowledge and new knowledge at the same time. This allows us to examine combinations of those two conceptions. For example, when the teacher conceives prior knowledge as to-be-developed and the mathematics involved in the task as having potential for new knowledge, she is likely to provide an opportunity for students to transform their prior knowledge in a new context. Distinct types of teaching can be observed as a result of the combinations of conception of prior knowledge and conception of a task for its mathematical potential (Fig. 1).

Fig. 1
figure 1

Teachers’ conceptions and teaching practice

Full size image

Based on the above discussion, this study attempts to specify teacher conceptions that influence the enactment of effective teaching. In particular, we investigate teachers’ conceptions of students’ prior knowledge and of the potential of tasks for knowledge development and use them as a framework to interpret instructional practice.

Methods

Setting and participants

This study occurred in the first year of a 3-year professional development project in the northwest USA. Fifty-four teachers participated in the project from seven school districts, including rural, urban, and suburban districts. The primary purpose of the project was to improve Algebra I teachers’ knowledge of and ability to teach the Common Core State Standards (CCSSI 2010). In particular, the project was designed to help teachers develop teaching which builds on the logic of the students and the logic of the mathematics (Ball et al. 2008; Sztajn et al. 2012; Wilson et al. 2014). The participating teachers attended six one-day workshops and a 3-day summer institute each year. Mr. Devlon and Ms. Anderson (all names are pseudonyms) taught Algebra I at the high school, and Mr. Ames taught Algebra I at the middle school that feeds into the high school. In the USA, where tracking is common, students in the 8th grade taking algebra learn the same content as students in the 9th grade. Mr. Devlon had been teaching for 9 years, Ms. Anderson for 23 years, and Mr. Ames for 5 years.

Data collection and analysis

Data come from four related activities: a workshop session in which teachers worked together on three related tasks, a professional learning community (PLC) meeting in their school in which teachers planned a two-day lesson, observations of three teachers each teaching the two-day lesson, and a workshop session in which the PLC described a learning trajectory related to the task. Table 1 summarizes project activities, data collected, and methods of analyses.

Table 1 Teacher activities, data collection, and data analysis
Full size table

In the March workshop, teachers worked in groups to solve and discuss the Intersections tasks (“ Appendix 1: Intersections tasks” Section), with each group working on one of the three tasks. Groups wrote descriptions of the mathematical ideas and procedures they identified as (1) most significant to solve the task and (2) supporting the most significant ideas and procedures. All work was collected and analyzed for themes. Whole-group discussion followed the small-group work. All discussions were audiotaped, transcribed, and analyzed for themes. Initial discussions and coding among researchers focused on data that could be used to characterize teachers’ conceptions of students’ prior knowledge (PK), and their conceptions of the problematic notions of mathematical tasks (PN). We wrote analytic memos describing our interpretations of teachers’ conceptions based on the data. Each PLC also wrote a purpose statement for a lesson using the tasks. We analyzed these statements by describing our interpretation of them, writing analytic memos, and coding them using initial codes.

After the workshop, teachers worked in their PLCs to plan the lessons and then taught them. The first two authors attended the lesson-planning meeting of Mr. Devlon, Ms. Anderson, Mr. Ames, and three of their colleagues. This PLC planned a two-day lesson which used Task 1, Task 2, and Task 0, which they created as a warm-up task. We audiotaped the discussion and collected artifacts, which included the worksheets they created to supplement the lesson, the warm-up, and homework assignments. Task 1 asks whether a line passing through the vertex of the given parabola with slope 5 intersects the parabola again at another point. Task 2 asks which lines passing through (−2, −1) intersect the given parabola again at another point. Task 0 is the same as Task 1 except the vertex is (0, 0) instead of (−2, −1). Note that the Intersections tasks involve contrasting cases, which Schwartz et al. (2007) considered critical in preparing students to learn new knowledge. Contrasting cases in these tasks include constant rate of change versus changing rate of change, algebraic representation versus graphical representation, and a single equation versus a system of equations.

The first author observed and audiotaped each 2-day lesson of Mr. Devlon, Ms. Anderson, and Mr. Ames. All audiotapes were transcribed. Initially, we employed open coding to conceptualize and categorize teacher moves and wrote memos (Charmaz 2002; Strauss and Corbin 1990) recording our thoughts, interpretations, and questions about teacher moves. The categories were refined as we compared and contrasted them within the teachers as well as across the teachers. We independently coded transcripts, discussed discrepancies, and clarified each code. Through this process, we described and defined three categories of PK and three categories of PN (“Appendix 2” Section). The reader should keep in mind that the analyses of data were our interpretations of the teachers’ perspectives, which were refined and developed over the time before, during, and after the use of the Intersections tasks. We adopted Simon and Tzur’s (1999) research methodology of “explaining the teachers’ perspective from the researchers’ perspectives” (p. 254), which allowed us to show empirically how teachers’ conceptions shaped their teaching.

After initial passes through the data, we created tables of data for each lesson that organized the exchanges between the teacher and students, relating the exchanges to the teacher’s use of students’ prior knowledge and the teacher’s focus on the mathematical potential of the tasks. The tables allowed us to examine the excerpts, discussing what the teachers and students could mean. The tables also allowed us to examine data coded for PK and PN in relation to each other. As we advanced our analyses, the initial codes about PK and PN were further refined. Table 2 shows the refined descriptions of PK, and Table 3 shows new codes of TP (potential of a task) developed from PN.

Table 2 Teachers’ conceptions of students’ prior knowledge
Full size table
Table 3 Teachers’ conceptions of the potential of task
Full size table

In the April workshop, after they taught the lesson, PLCs created a learning trajectory to identify the prior knowledge of students, which included the concepts, procedures, and mathematical practices that could be used when they solved the Intersections tasks. PLCs’ discussions during this activity were audiotaped. While these data are not described in this report, it helped us better understand how the teachers conceived students’ prior knowledge, ways they could help students advance that knowledge, and their conceptions of the task potential. The first author continued to observe all three teachers’ classroom teaching for 3 years as a requirement of the professional development project and found teachers’ teaching of other topics was consistent with the findings of this study. Finally, it is important to note that all three teachers had participated in the group and PLC discussions about the task during the March workshop and that they planned the two lessons together. The teachers had discussed possible difficulties they would encounter when teaching and difficulties their students would encounter when learning. However, we observed three different types of teaching.

Findings

In this section, we present (1) what mathematical notions emerged during the workshop, (2) what the teachers identified as students’ prior knowledge related to the Intersections tasks and how they used the identified students’ prior knowledge, and (3) whether or not teachers identified new knowledge when using the Intersections tasks and how they dealt with the identified knowledge (new or not) for their students’ learning.

Two mathematical notions emerged

During the March workshop, we observed both algebraic and geometric approaches. The teachers with algebraic approaches solved the tasks by finding the equations of a line and the given parabola. The group of teachers who used a geometric approach reasoned about the tasks by focusing on rates of change of the graphs. The whole-group discussion was facilitated with a focus on the geometric approach as we took the following teachers’ concerns into consideration: (1) after drawing a line with slope 5 to solve Task 1, students may claim that the line is parallel to the right most part of the given parabola (Fig. 2); and (2) it may be challenging to convince students that any non-vertical line passing through the vertex of the given parabola intersects the parabola again except when the slope is zero. The discussion centered around the notion of rate of change: “I expected it to cross a second time by knowing the behaviors of the linear slope to the ‘slope’ of quadratics” and “Idea of ‘slope’ along a parabola—changing constantly rate of change.” The teachers who solved the tasks algebraically also noticed connections between algebraic and geometric meanings of the intersecting points: “Create a table for points to get the same values at intersections” and “If they intersect they will have another common (x, y) pair.”

Fig. 2
figure 2

A line with a slope of 5 passing through the vertex of a parabola

Full size image

The notion of solution As they solve one-variable equations, students develop the meaning of a solution as a value of the variable that makes the equation true. In the transition from solving one-variable equations to solving systems of equations, students should extend the meaning of solution from a single value to a pair of values as a solution of an equation in two variables and finally, as a common solution to two equations in a system. Solving a system of equations graphically requires understanding that the coordinates of any point on the graph of a function satisfy its algebraic representation, the equation. Such connection is challenging to students, and they tend to perceive the graph of a function “as a means to support the algebraic-solution methods rather than as a means to a solution in and of itself” (Knuth 2000, p. 506).

The notion of rate of change When asked to graph a linear function with a given slope, students in the USA usually recall the phrase “rise over run,” where “rise” means a change in y and “run” a change in x, and use the phrase to find another point so they can draw the line. This implies that students are likely to attend to the change of each quantity instead of coordinating the change in one quantity with the change in the other quantity. The ability to coordinate changes in x- and y-values of a line is critical in seeing the slope as an attribute of the line, which is taking rate of change as a quantity (Thompson 1994). For students to use rate of change as a tool to describe and differentiate among types of graphs, their conception of rate of change based on the slope of a line should be advanced toward a mathematical object that can be observed and compared among types of graphs. With the advanced understanding of rate of change, the slope of a line becomes an object using the notion of covariation and the shape of a parabola is determined by the changes in the rate of change. Rate of change becomes a conceptual tool to investigate a parabola and further understand different types of graphs (e.g., Carlson and Oehrtman 2005).

Teachers’ conceptions of students’ prior knowledge

Our participating teachers’ conceptions and uses of students’ prior knowledge differed in how teachers defined the scope and depth of the mathematics students would bring to the classroom. In a sense that teachers’ conception of PK is their own construct and reflects their knowledge of mathematics and of students, analyzing teachers’ use of students’ prior knowledge while teaching the lessons informed us of their combined knowledge of mathematics and students in practice.

Prior knowledge as a concept to develop

Mr. Ames demonstrated a conception of PK as a concept to progress. While his students solved Intersections Task 0, he elicited their prior knowledge of solutions of one-variable equations and different representations of functions. His teaching used these constructs to develop students’ understanding of the intersection of two graphs as a solution of a system. Rather than consider the new idea as simply an aggregation of their prior knowledge, he considered the prior knowledge incommensurable.

We explain this category of teacher conception in terms of how Mr. Ames defined the scope and depth of the identified prior knowledge in his practice. First, the scope of prior knowledge “solution” for student learning: Mr. Ames expanded the equation context where students would bring the meaning of solution to the system of equations context via a function context. In particular, the term function was deliberately chosen to provide an opportunity for his students to make connections between functions and equations using various representations of functions. Second, the depth of prior knowledge of “solution” for student learning: students started with the meaning of solution as a value that makes an equation true and developed the meaning of solution as an ordered pair that makes the equation of a function true. This allowed students to understand the graph of a function as a set of solutions and deepened the meaning of the intersection of graphs as a common solution of the equations of the graphs. Note that according to the described depth of the notion of solution, the intersection of graphs is more than a graphical representation of an algebraic solution of a system of equations.

Mr. Ames posed the Intersections task by asking students for different representations of a function. Using the tabular, algebraic, and graphical representations suggested by students, he helped students extend their understanding of solution developed in the equation context to the function context. Students demonstrated their understanding of the graph of a function as a set of solutions of the function in equation form and furthered their understanding toward the algebraic meaning of the intersection of graphs. In support of this, he said, “if I drew this [a point] a little bit better, we could see that that 0.1 and 0.5 [the coordinate of (0.1, 0.5)] is a possible solution to this equation, and so that line allows us to represent the infinite number of solutions for this equation [y = 5x]. So when we take a look back here, what does that intersection mean?” One student responded, “a possible solution to both of them [equations in the given system of equations].” Mr. Ames’ practice evidences that students’ prior knowledge made meaningful connections with upcoming concepts. He focused on the progression of the identified prior knowledge so that it became critical to solve the given mathematical task.

Prior knowledge as the knowledge to develop while remaining at the previous level

Ms. Anderson demonstrated her conception of PK as a concept to progress. Before the lesson, she explained to the observer her plan to help students progress their prior knowledge to find the function rule of a given parabola by having them notice a connection between factored form in solving an equation and x-intercepts of a parabola. She identified the procedure of solving a quadratic equation by factoring as the prior knowledge students could bring to the classroom.

Given a parabola, Ms. Anderson had students find x = a and x = b, the x-intercepts of the graph, and write y = < quadratic expression in x > in factored form (x−a)(x−b). In the midst of guiding students to writing the factored form, she had students reverse the procedure of solving a quadratic equation by factoring and see how the reversed process is related to finding the function rule of the given parabola: “You’re going to use what you know about solving quadratic equations by factoring to work backward to find the function rule for this graph.” Finding a function rule from zeroes is not simply the reverse of solving quadratic equations by factoring but requires reverses of thought processes and procedures. However, when many students wrote the equation (x−a)(x−b) = 0 as a function rule she simply asked the students to replace the zero with y without highlighting the meaning of doing so.

Ms. Anderson constrained the scope and depth of the meaning of a quadratic equation in the system of equations context. She attempted to use students’ prior knowledge meaningfully in a new context but instead had the students practice the reversed procedure of solving a quadratic equation exactly the same way as they did previously. In contrast, she could have developed the meaning of (x−a)(x−b) = 0 in the new context of systems by helping students see it as a system of equations consisting of y = 0 and y = < quadratic expression in x >. This would afford students an opportunity to make a connection between the task of finding intersections and the task of solving a one-variable equation.

The prior knowledge remained at the previous context without any progression. Such constrained scope and depth of prior knowledge limited students’ opportunity to make conceptual connections between the equation context and the system of equations context. As demonstrated, her way of using prior knowledge reflects a conception of prior knowledge as to-be-developed in a constrained manner.

Prior knowledge as an entity

Teachers’ conception of PK as an entity was demonstrated in Mr. Devlon’s way of using students’ prior knowledge. By entity, we mean that prior knowledge was based on a dichotomy, existing as complete or not existing. Mr. Devlon identified the following as his students’ prior knowledge: knowing the term solution, being able to graph linear and quadratic functions, and being able to solve quadratic equations by factoring. He seemed to consider students’ prior knowledge as commensurables in that the identified prior knowledge is sufficient to solve the task. This implies that there is nothing new for students to develop from the prior knowledge in the new context of a system of equations.

We explain this category of teacher conception of PK in terms of how Mr. Devlon defined the scope and depth of the identified prior knowledge in practice. The scope of prior knowledge “solution” for student learning: Mr. Devlon used the term solution to signify an answer to a question and did not encourage further meaning, suggesting that there was no need to further develop the meaning across different contexts. When solving the system of equations y = −2x and y = x2−4x graphically, some students circled the intersecting points of the graphs after having drawn the line and the parabola. The dialogue below shows a student’s way of perceiving the intersecting point. “The” in line 8 indicates that S2 used the term solution as a name to designate to particular objects. The “The” also allows us to infer that S2 used the word common to refer to a perceptual observation of the points that cross rather than a conceptual meaning of common in “common solution of the equations of given graphs.” Mr. Devlon confined the meaning of solution and reinforced students’ view of solution as a physical object, the intersecting point of the graphs. Therefore, the given new context of a system of equations was not used to expand the scope of the notion of solution.

Dialogue Points that cross are solutions (T stands for Mr. Devlon; S1 and S2 stand for different students.)

  1. 1.

    T: Why are you circling points, what’s so special about those [intersections of a line and a parabola]?

  2. 2.

    S1: Umm.

  3. 3.

    T: [S2], go for it.

  4. 4.

    S2: That’s what they have in common, the two.

  5. 5.

    T: Those are the points that they have in common.

  6. 6.

    S2: The ones that cross.

  7. 7.

    T: That’s where they cross, right, what do we call those points?

  8. 8.

    S2: The solution.

  9. 9.

    T: The solutions, absolutely right, those are the solutions to this system of equations.

For the depth of prior knowledge “solution” for student learning, Mr. Devlon checked students’ understanding of solution by having them solve a quadratic equation by factoring. He did not engage students in transforming the meaning of solution they developed while solving a quadratic equation into the new context of finding the equation of a given parabola and further solving a system of equations. Mr. Devlon’s practice evidences that students’ prior knowledge was not used to make conceptual connections with upcoming concepts; he rather focused on checking and aggregating the prior knowledge which he took as sufficient to solve the task.

Teachers’ conceptions of the potential of task

Whether teachers notice the potential of a task to be used to help students develop new knowledge or not may reflect their own knowledge of mathematics and the mathematics of their students. We found two different conceptions of the potential of task evidenced in practice.

Potential of task for new knowledge

Mr. Ames demonstrated a conception of the potential of a task to develop new knowledge. This conception reflects his view of a task as involving a mathematical concept to develop. He identified two concepts as the potential of the Intersections Task 0: conceiving the parabola in terms of rate of change and seeing the parabola as a set of solutions of its quadratic function. His lessons involved using the task to provide opportunities for students to develop the identified potential.

We explain this category of teacher conception in terms of the characteristics of the potential evidenced in practice. During the class discussion about whether the graph of y = 5x intersects a given parabola again, students paid attention to the shape of the parabola without bringing specific prior knowledge, such as “this [the parabola] keeps going out and this [the right most part of the parabola] does stay.” Mr. Ames invited his students to reason about the shape of the parabola while reflecting on what they did to draw a line, plotting points, and examining the relationship among the points. He asked students what the graph of a given line actually shows. When a student answered it with a slope of 5, he asked the student what it means and the student responded, “Up five and over one, up five and over one.” Mr. Ames called on another student who said, “It represents a steady growth.” He then asked students, “What does that parabola look like?” as he encouraged them to examine table values they created for the line and parabola. He developed the previously learned concept of rate of change for a line as a tool to describe the parabola and further compare the line and the parabola. He also advanced the conception of solution from the algebraic context to the graphical context, which was critical to justify the intersection of graphs as a common solution of the equations in the system.

The identified potential of the task was also relevant to students’ future learning. Mr. Ames did not revert to focusing on the previously learned context when implementing the task. When students were asked to find intersections of the graphs of y = x2 and y = 5x, one student wrote x2 + 5x = 0, x(x + 5) = 0, and x = −5 applying the substitution method wrongly and leaving out another solution x = 0. Mr. Ames had the student refer to the graphs to re-examine his solution and consider the meaning of the solution. Rather than use the instance solely to have the student correctly use the substitution method, Mr. Ames had the student work on the graphical meaning of solution in the newly posed context of a system of equations.

No potential of task for new knowledge

Mr. Devlon and Ms. Anderson demonstrated a conception of the potential of a task as “no potential of task for new knowledge.” This conception reflects the view of a task as useful merely for exercising skills without expecting the development of new knowledge. The teachers’ goal appeared to be to equip students with the skills that would be sufficient to solve the problem.

Mr. Devlon reinforced students’ reliance on their observations without asking them to justify the observations using mathematical ideas. For example, when asking which lines passing through the origin intersect the graph of y = x2 again at another point, he had students manipulate their pencils to imitate lines over the curves, which were drawn roughly by hand. Indeed, when one student raised the issue of rate of change in trying to reason about the relationship between a line and the parabola, Mr. Devlon did not bring the issue to the whole class even though the notion of rate of change could be used to explore attributes of the graphs that may affect the location or number of intersections. He did not seem to find anything new for students engaging in the task. The purpose of using the task for Mr. Devlon seemed to be to find answers rather than provide an opportunity for students to learn new knowledge.

Mr. Devlon treated meanings new to students as if they had already learned them. He asked students, “Find the solutions of a given parabola” when helping them find the x-intercepts so they could write the factored form of a function rule. He used the term solutions, a term students had learned in the equation context, for the new concept of solution of a parabola, which is a set of coordinates that satisfy the function rule. We may attribute his erroneous use of mathematical terms to his conception of the task as useful only for already learned concepts. He did not seem to consider that the term solution would involve a deep connection among x-intercepts of a parabola, zeros of the quadratic function, and solution of a parabola. Ms. Anderson also brought into one incidence all the related mathematical terms that students had yet to develop in the new context of system of equations. She seemed to want to restore everything that might be problematic for students about the relationship between quadratic equations and quadratic functions or the relationship between the algebraic representation and graphs of quadratic functions.

That they saw no potential of the task for new knowledge caused Mr. Devlon and Ms. Anderson to have their students leave the task unfinished. Even though students were supposed to solve a system of equations, the teachers permitted students to stop working when they found the function rule of a given parabola. They seemed to assume that solving a system of a linear equation and a quadratic equation required the same mathematical skill as solving a quadratic equation even though the system was provided graphically. They dealt with the newly posed geometric context of a system as if it were the quadratic equation context.

Structuring and interpretation

In this section, we structure our findings to explain how teachers’ conceptions of PK may interact with teachers’ conceptions of TP in terms of new knowledge. We also illustrate how the interaction may shape teaching.

Promoting prior knowledge for new knowledge

Mr. Ames elicited students’ prior knowledge to promote the potential of the task for new knowledge. Instead of treating students’ prior knowledge as the complete knowledge needed to solve the task, he used students’ prior knowledge to open up something problematic for students. This helped him focus on a progression or reorganization of the concepts needed to solve the task. For example, when posing a system of equations, he did not take it for granted that students would understand the meaning of solution, especially the meaning of solution in the graph of a system of equations. He put students into a problematic situation as he moved from the equation context to the function context and further to the system of equations context. This strategy of dealing with students’ prior knowledge enabled Mr. Ames to view the mathematical concepts identified as prior knowledge in a progressive manner rather than a regressive manner. He identified the potential of the task in a new context and provided students with opportunities to reflect on their prior knowledge along a continuum and advance the knowledge.

Mr. Ames demonstrated conceptions of PK as a concept to progress and TP as having potential for new knowledge. Table 4 summarizes the characteristics of teaching that may be shaped by those two conceptions.

Table 4 Teachers’ conception of prior and new knowledge and teaching (1)
Full size table

Reducing new knowledge to the context where prior knowledge was identified

Ms. Anderson and Mr. Devlon reduced the potential of a task from the system of equations context to the equation or function context. This reduction led them to find something problematic for students in the context where prior knowledge was identified instead of in the newly posed context. It also resulted in suppressing the concepts that could have been advanced by engaging in the task. For example, both Ms. Anderson and Mr. Devlon treated a system of equations as a single equation problem. The focus of their instruction was restoring students’ prior knowledge identified as sufficient to solve a given task. However, their teaching strategies were dissimilar due to the difference in their conceptions of PK.

Ms. Anderson dealt with students’ prior knowledge as a concept to develop. But the identified concept was constrained to the previous context rather than developed in the new context. This limited the potential of a task for student learning. Given a system of linear and quadratic equations graphically, Ms. Anderson provided opportunities for students to deepen the concept of slope as the rate of change and develop the ability to write the equation of a given parabola by reversing the procedure to find the x-intercepts of a quadratic. However, she did not have students use the deepened understanding of the prior knowledge for the system of equations context. We identify Ms. Anderson’s teaching strategy as reviewing, which means teaching a previously learned concept in a new context but setting the expectation in the previous context. Using the strategy of reviewing, teachers may repeat the way they taught the concept the first time or find a new way to help increase conceptual understanding of the concept but with limited expectations.

Mr. Devlon dealt with students’ prior knowledge as an entity. This also limited the potential of a task for new learning. Unlike Ms. Anderson, he did not provide an opportunity for students to make connections between a factored equation and a function in factored form in graphical contexts. He had students recall previously learned mathematics such as solving a quadratic equation by factoring and use it to find the function rule of the given parabola using x-intercepts. We identify Mr. Devlon’s teaching strategy as recalling. The critical difference between the teaching strategies demonstrated by Ms. Anderson and Mr. Devlon is whether they take a developmental perspective of students’ prior knowledge.

Ms. Anderson and Mr. Devlon presented the same conception of TP as having no potential for new knowledge. But they differed in conceiving students’ prior knowledge: for Ms. Anderson, prior knowledge was a concept to develop but constrained by the prior context, and for Mr. Devlon, prior knowledge was an entity. Table 5 summarizes the characteristics of teaching that may be shaped by the combined conceptions presented by Ms. Anderson and Mr. Devlon.

Table 5 Teachers’ conceptions of prior and new knowledge and teaching (2)
Full size table

In sum, one teacher practiced a “progressing” teaching strategy and treated students’ prior knowledge in a progressive manner rather than a regressive manner. He had students reconsider their prior knowledge in the new context and noticed the potential of the task for new knowledge. Accordingly, he provided students with opportunities to advance their knowledge. In contrast, teachers who practiced “recalling” or “reviewing” teaching strategies treated the potential of tasks in a regressive manner, not providing opportunities for students’ prior knowledge to advance in the new context.

Discussion

Our participating teachers presented three distinct conceptions of PK: PK as (1) a concept to progress, (2) a concept to progress but constrained in the previous context, and (3) an entity. They also presented two distinct conceptions of TP: (1) having potential for developing new knowledge and (2) having no potential for new knowledge. Since a conception of PK as a concept to progress but constrained in the previous context appears to be affected by teachers’ conception of the task potential for new knowledge, we combined it with the conception of PK as a concept to progress as one category labeled To-be-developed (see Table 6).

Table 6 Types of teaching by prior knowledge and potential of task
Full size table

In the previous section, we described three types of teaching except Type B in Table 6, using different combinations of teachers’ conceptions of PK and TP. Mr. Devlon’s teaching strategy, recalling, demonstrates Type A; Ms. Anderson’s teaching strategy, reviewing, demonstrates Type C; and Mr. Ames’ teaching strategy, progressing, demonstrates Type D. We envision Type B when the teacher notices the potential of a task for student learning and identifies students’ prior knowledge as full-fledged knowledge that can be used without transformation. The focus of instruction is on aggregating knowledge as much as needed until the given task is solved. The critical difference between Type B and Type D is that Type D requires transformation of prior knowledge, whereas Type B does not.

The findings in this study relate to prior research on the relationship among teachers’ beliefs, knowledge, and enacted teaching practices. Askew et al. (1997) found that teachers’ beliefs and knowledge about the nature of numeracy, how children learn to become numerate, and how best to teach children to become numerate were associated with their teaching approaches. They differentiated three orientations of teachers—connectionist, transmission, and discovery. Teachers who had different orientations differed in their interactions with children and in their understandings of how to use and apply what children had learned. Building on Askew et al.’s three orientations of teachers, Swan (2006) and Polly et al. (2013, 2014) provided quantitative evidence about the relationship between teachers’ beliefs and practices. The results of this present study are consistent with the previous studies, but we have elaborated the relationship with a focus on teachers’ conceptions of PK and TP.

Teachers’ conception of PK is related to knowledge of content and students (Hill et al. 2008) in that we examined the conception while teachers attended to both the specific content and how students thought about and learned the particular content. Similarly, teachers’ conception of a task as having the potential for developing new knowledge relates to pedagogical task knowledge (Liljedahl et al. 2007) in that the conception is about using mathematical tasks to access mathematical concepts and orchestrating classroom dynamics to achieve the goal. The combination of PK as to-be-developed and TP as having potential for new knowledge leads to the “progressing” type of teaching. This type is aligned with connectionist-oriented practices in that connectionist-oriented teachers expect differentiated learning experiences among students in the classroom and believe that students benefit from tasks for which they did not have the skills immediately available.

Teachers’ beliefs and knowledge about students are important in helping students learn with understanding (Fennema et al. 1996; Franke et al. 2007). Likewise, teachers’ beliefs and knowledge about mathematical tasks are important in students’ learning as well as in the transformation of teaching practices (Henningsen and Stein 1997; Liljedahl et al. 2007; Swan 2007). The present study took these important components of teachers’ beliefs and knowledge into consideration and further specified a way that teachers can be helped through the development of their conceptions of PK and TP.

We note that conceptions of PK and conceptions of TP may provide specific information for teachers to make instructional decisions in that the conceptions are forms of ongoing diagnoses with the aim to allow for didactic action before, during, and after instruction, which is critical in improving diagnostic competencies (Klug et al. 2013). This notion of PK and TP as a tool for teachers’ diagnostic activity allows us to use them as criteria that shape teaching: Criterion (1) whether teachers conceive what students bring as knowledge ready to use or knowledge to be developed; Criterion (2) whether teachers conceive the task as involving something new for students to learn or the task as merely providing an opportunity for students to review or recall knowledge and skills.

Tzur (2010) also suggested two criteria to describe types of teaching: psychology (passive stance vs. active stance toward learning) and epistemology (learner-independent stance vs. learner-dependent stance toward knowing mathematics). He argued that an active stance on learning emerged differently according to whether it was combined with a learner-independent stance on mathematics or with a learner-dependent stance. This explains why even though teachers seemingly do the same thing on the surface, like letting students actively share their ideas, their teaching may markedly differ at students’ conceptual level. The present study also provides clues about why teachers with a developmental perspective of students’ mathematics end up doing reviews. In order to use a task in ways that allow students’ knowledge to progress rather than stay at their current level, teachers must conceive the potential for new knowledge in the task. We found that to teach for coherence, teachers must conceive students’ prior knowledge as the knowledge to be developed and the task as having potential for developing new knowledge.

Findings of this present study have implications for both professional development and teacher education programs. Previous research has found that a lack of alignment between students’ prior knowledge and tasks inhibited teachers’ abilities to implement high-level tasks (Bennett and Desforges 1988; Doyle 1988; Hiebert and Carpenter 1992). Our findings suggest that activities provided in professional development and teacher preparation programs may be able to help teachers conceive students’ prior knowledge as to-be-developed and tasks as having the potential for developing new knowledge. These conceptions could help teachers overcome such alignment issues and set a boundary of instructional decisions to be made when they incorporate the mathematics of students and new knowledge to develop. The suggested activities will reinforce teachers’ learning in practice to see the potential of the mathematics that students bring and the potential of the mathematics embedded in a given task.

This present study was limited in examining the stability of teachers’ conceptions of PK and TP across mathematical topics and across grade levels. We also have not investigated whether our PK-TP framework in relation to type of teaching practice is generalizable to a larger population of teachers, but we believe this could be a productive next step for research. Since this is the first study we conducted with this framework, the investigation of the stability and the generalizability to other topics and other grade levels can be a question for further research.