Auctioning Versus Grandfathering in Cap-and-Trade Systems with Market Power and Incomplete Information

Abstract

We compare auctioning and grandfathering as allocation mechanisms of emission permits when there is a secondary market with market power and firms have private information on their own abatement technologies. Based on real-life cases such as the EU ETS, we consider a multi-unit, multi-bid uniform auction. At the auction, each firm anticipates its role in the secondary market, either as a leader or a follower. This role affects each firms’ valuation of the permits (which are not common across firms) as well as their bidding strategies and it precludes the auction from generating a cost-effective allocation of permits, as it occurs in simpler auction models. Auctioning tends to be more cost-effective than grandfathering when the firms’ abatement cost functions are sufficiently different from one another, especially if the follower has lower abatement costs than the leader and the dispersion of the marginal costs is large enough.

1 Introduction

Most economists tend to favour auctioning of emission permits in cap-and-trade (CAP) systems, while business tends to prefer a grandfathering scheme, as noted for example by Hepburn et al. (2006). Until very recently, auctioning has been the exception rather than the rule, while grandfathering has been by far the most widespread method used to distribute emission permits. Nevertheless, the situation is changing and may change even more dramatically in the coming future. Thus, it seems relevant to wonder to what extent moving from a grandfathering scheme to auctioning may deliver any improvements in efficiency. The aim of this paper is to compare both mechanisms and, more specifically, to test whether auctioning can outperform grandfathering in terms of cost-effectiveness in a framework of incomplete information and market power (with a leader and a follower) in the permit market.

The world’s first $\hbox {CO}_{2}$ CAP system to require the widespread use of auctions was the Regional Greenhouse Gas Initiative (RGGI). This programme, which began in 2009, includes 9 northeastern US states and covers $\hbox {CO}_{2}$ emissions from electricity generators. As noted by Burtraw et al. (2009), the RGGI proposal represents a substantial break with the past since, instead of giving the permits away for free, the RGGI states decided to auction close to 90  % of their permit budgets. As another important example, the role of auctioning is becoming increasingly relevant for the European Union Emission Trading System (EU ETS), considered to be the largest environmental market in the world. The 2008 revised European Emission Trading Directive established as a fundamental change for the third trading period, starting in 2013, the mandate that auctioning of allowances is to be the default method for allocating allowances. From 2013 onwards, all allowances not allocated for free must be auctioned and auctioning will progressively replace grandfathering as the main method in all EU-ETS sectors, except aviation. Roughly half of the allowances are expected to be auctioned.¹ The arguments posed by the European Commission (EC) to support the introduction of auctions in the third period are that auctioning “best ensures the efficiency, transparency and simplicity of the system, creates the greatest incentives for investment in a low-carbon economy and eliminates windfall profits”.²

The aim of this paper is to provide some insight into the properties of auctioning versus grandfathering. Specifically, we test the EC’s argument supporting the introduction of auctioning on the grounds of efficiency. The efficiency of an environmental policy requires: (a) enforcing the “right” (efficient) amount of emissions, and (b) distributing such emissions among emitters in a cost-effective way. We disregard the first issue by assuming that the amount of permits is exogenously decided to focus on the second dimension, i.e., the right way of distributing permits among polluting firms. Summing up, our central research question is: Can auctioning distribute permits in a more cost-effective way than grandfathering?

We present a simple model in which two firms receive an initial allocation of emission permits (either by means of grandfathering or auctioning) and then interact in a secondary market.³ To simplify the exposition, we abstract from output production. The main elements of our model are market power in the secondary market and incomplete information in the auction. Market power is modelled by assuming that one of the firms in the secondary market acts as a leader and the other, as a follower. As is usual in auction theory, we model incomplete information by assuming that the agents have random and privately observed types. In our framework, these types determine the relative position of the abatement–cost functions.

In this setting, we compare auctioning and grandfathering in terms of cost-effectiveness. An argument to support the hypothesis that an auction can be more cost-effective than grandfathering is that the auction could work in such a way so as to reduce the chances of the leader exerting its market power. To test the validity of this argument, we focus on a case in which both the leader and the follower act non-strategically in the auction even though they are capable of anticipating the role of each firm in the secondary market. Under these circumstances, the auction has the effect of making the firms more symmetric in a certain sense.

Our main results fall into two categories. The first has to do with the predictive ability of the theory, including the existence and uniqueness of the equilibrium under the auction. Under the technical requirements we impose, existence and uniqueness is always guaranteed and we are able to compute the closed-form of the equilibrium.

In the second category, we obtain some normative results by comparing auctioning and grandfathering in terms of cost-effectiveness. We do so in three distributional scenarios. In the first, the firms’ types are iid, which amounts to assuming that, in expected terms, they are equally efficient in terms of abatement costs. In the other two cases, we consider positive correlation between the types. In the second scenario, the leader enjoys an abatement technology that is more efficient than the follower’s with probability one, while the opposite happens in the third scenario.

In all the cases we study, the equilibrium of the auction is never cost-effective, because the leader will either over-bid or under-bid to place it in the most advantageous position for the secondary market. In general, grandfathering is not cost-effective either, except by chance. We also conclude that, if the firms’ abatement costs are sufficiently different from one another, the firm with the higher cost is likely to bid more aggressively and get all the permits in the auction, and this situation tends to generate more cost-effective results than grandfathering. If, on the contrary, the firms are similar enough to each other, permits are shared among both firms in the auction, but the follower is generally under-assigned and the leader is over-assigned with respect to the cost-effective allocation. In general terms, under auctioning, the leader tends to be over-assigned as compared to cost-effective allocation in expected terms. Moreover, the auction generally does worse than grandfathering in terms of expected cost when the types are independently distributed or the leader’s marginal cost is ex-ante lower than the follower’s. Contrarily, if the follower has access to a more efficient abatement technology and, additionally, the variability of the types is large in terms of the cap, then it is the auction that outperforms grandfathering, since the allocation generated by the auction is closer to the cost-effective one than that resulting from grandfathering.

As a general policy implication, we conclude that the mere fact of auctioning permits instead of allocating them by means of grandfathering is not a panacea, at least in terms of cost-effectiveness. As a matter of fact, in two of the three cases considered auctioning results in higher total costs than grandfathering, while in the third case the comparison is inconclusive as it depends on the parameter values.

Our paper builds on two strands of literature. The first strand, pioneered by Hahn (1984), focuses on CAP systems under market power.⁴ It is well known that, under perfect competition and complete information, the initial allocation of permits only matters for the distribution of the gains from trade, but it is irrelevant for the final allocation and hence for cost-effectiveness (see Montgomery 1972). However, as first noted by Hahn (1984), if there is market power in the permit market, the initial distribution of permits does matter for the sake of cost-effectiveness. In fact, the cost-effective allocation will be reached only when, in the initial allocation, the leader receives exactly the amount of permits that it would receive under perfectly competitive pricing. So, on the grounds of cost-effectiveness, the EC’s argument for auctioning only makes sense under the implicit recognition that there is not a perfect market for permits, since, otherwise, the allocation method would be irrelevant for cost-effectiveness.

Sturn (2008) notes that whether market power actually constitutes a problem in an emission trading system has to be analyzed for each market separately. Market power is probably not one of the main issues in the EU ETS since the number of facilities involved is very large, but it may constitute a serious problem for future international emissions trading system within the framework of the Kyoto Protocol and for some regional emissions markets. Montero (2009) and Muller et al. (2002) stress that market power issues are more likely to show up in markets where countries—rather than individual entities—are the relevant players, as is the case of the Kyoto Protocol. On the other hand, it can be argued that, even in a CAP system with a large number of participants (such as the EU ETS), it is not unrealistic to assume that the market is not perfectly competitive, as there may be information asymmetries, collusive behavior, interaction with other markets and other imperfections. For example, Ehrhart et al. (2008) claim that “loopholes in EU emissions trading law foster tacit collusion that impacts oligopolistic product markets” (p. 347). Specifically, they state that “permits might be diverted from their intended use as a vehicle for tacit collusion in two basically different manners: by increasing permit prices or by coordinating the firms’ emissions” (pp. 357–358). Hinterman (2011) focuses on the power sector and considers a case in which there is market power in both the power and the permit market. In an application to the EU ETS, he concludes that the largest electricity producers in Germany, the UK and the Nordpool market might have found it profitable to manipulate the permit price upwards and he claims that this could explain the elevated allowance price level during the first 18 months of the EU ETS. For each individual market, the higher or lower severity of these failures will determine how imperfect the market is and therefore the room for efficiency improvements.

Besides market power, we consider an additional element to make the comparison between grandfathering and auctioning non-trivial, which is incomplete information at the time of making the initial allocation. Apart from theoretical interest, there are two main arguments for considering incomplete information. The first argument is that it is required to make the introduction of auctioning meaningful. In fact, if information were complete, and more specifically, if the environmental planner could observe or make a perfect forecast of firms’ abatement costs, he could compute the cost-effective solution and implement it.⁵ Under those conditions, there would be no room for further improvements when introducing auctioning, since grandfathering would readily provide the minimum-cost allocation. The second argument is that, in real life, information does not actually appear to be complete. For example, Ellerman et al. (2010) claim that, during the first period of the EU ETS, “the task of setting a cap that was at or close to business-as-usual (BAU) emissions was made enormously more difficult by poor data. The problem was that no member state government had a good idea of the exact emissions within the ETS sectors ... and the data problem was even worse in the new member states of the eastern Europe”. Moreover, “the problems created by poor data were not limited to cap-setting; they extended into the allocation of allowances to installations, which required installation-level emissions data ...Not surprisingly, since allocations to these installations depended on the data submitted, industrial firms were forthcoming, although there has always been a suspicion that the intended use of the data imparted an upward bias to these data” (pp. 37–38).⁶ The large variability of the price of permits during the first years of the EU ETS (see e.g. Ellerman et al. 2010, Chapter 5) is also consistent with the belief that the market was not working under complete information. Based on these arguments, we assume that firms have private information about their technology and the effects of external events on their BAU emissions.

The second strand of literature refers to auction theory. This literature typically focuses on optimal bidding strategies, on how auctioned goods are allocated to bidders and what revenue the auctioneer can expect to get from the auction. Specifically, we are concerned about multi-unit multi-bid auctions, in which more than one unit is being auctioned (multi-unit) and bidders can bid for more than one unit⁷ (multi-bid). Typically, theoretical papers on multi-unit multi-bid auctions do not model the secondary market and assume common value; that is, the value of each auctioned unit is constant both across units and across bidders (see, for example, Wang and Zender 2002 or Alvarez and Mazón 2012). More general settings, such as the one presented in Castro and Riascos (2009), do not fully characterize equilibrium as we do, but only optimal responses. Under common value, efficiency is not a relevant question, since any allocation of units across bidders is efficient.

In our model, the common-value assumption fails to hold for two reasons. First, bidders—polluting firms—at the time of bidding are aware that there will be a leader and a follower in the secondary market, and each firm anticipates its own role and the rival’s, either as a price setter or taker. This heterogeneity across firms implies that the value attached to permits is not common to them.⁸ Second, we realistically assume that each firm’s marginal abatement cost is not constant. Therefore, the value of one additional permit is not constant either across firms or across units for a single firm. Thus, the efficiency of the auction allocation turns out to be a relevant question in our setting, as efficiency results that holds true for simpler auctions do not trivially extend to this setting.⁹

To the best of our knowledge, the question of how cost-effective an auction is compared to grandfathering (under market power) has not been addressed at a theoretical level, although some related informal discussions and experimental studies have been conducted (see Ledyard and Szakaly-Moore 1994; Godby 1999, 2000; Muller et al. 2002). In a theoretical paper, Antelo and Bru (2009) compare auctioning and grandfathering in a permit market with a dominant firm when the government is concerned both about cost-effectiveness and public revenue, but one of our main building blocks, incomplete information, is absent in their work, which implies that cost-effectiveness is not a relevant issue. Montero (2009) also uses a deterministic approach and, as a consequence, both Antelo and Bru (2009) and Montero (2009) conclude that, in a setting in which the auction is followed by a secondary market, it is optimal for the leader not to take part in the auction and acquire all the permits in the secondary market. The rationale underlying this somewhat counterintuitive result is that, for the leader, it is optimal not to bid in order to keep the price low and then buy the permits in the market at a lower price than the competitive one by acting as a monopsonist. We conclude instead that, under incomplete information, it is not necessarily optimal for the leader to refrain from participating in the auction (although it is so in some specific cases). Actually, in some equilibria it is just the opposite: the leader captures all the permits in the auction and sells part of them in the secondary market acting as a monopolist.

The article is organized as follows. In Sect. 2 we present the main elements of the model, the technical assumptions and the structure of the secondary market. Section 3 shows how we model grandfathering. In Sect. 4 we analyze the auction and compare the results with those under grandfathering in terms of cost-effectiveness. Section 5 presents some conclusions, as well as some discussion and ideas for future research.

2 The Model

2.1 Basic Setting

There are two risk-neutral polluting firms, labelled as $i\in \{F,L\}$, that are subject to a CAP system. $\bar{Q}$ emission permits are issued and distributed between the firms by some allocation procedure (either auctioning or grandfathering). The number of permits is taken as given and we only care about the distribution of those permits between firms. Each firm $i$ receives an initial allocation of permits, denoted as $q_{i0}$, with $q_{L0}+q_{F0}=\bar{Q}$, and then the permits are traded in a secondary market in which $L$ acts as a leader and $F$ as a follower.¹⁰ Since in real-world examples the number of permits to be assigned is typically very large we take it to be a continuous variable as a reasonable approximation.¹¹

To meet environmental legal requirements, each firm has two options: first, to use permits and, second, to do some abatement effort to reduce its emissions. For the sake of analytical tractability, we assume that the cost of the latter option is given by a quadratic total abatement cost function ($TC$), which entails a linear marginal abatement cost function ($MC$):¹²

$$\begin{aligned} TC _{i}\left( e_{i}\right)&= K_{i}-\alpha _{i}e_{i}+\frac{\beta }{2}e_{i}^{2},\nonumber \\ MC _{i}\left( e_{i}\right)&= - TC _{i}^{\prime }=\alpha _{i}-\beta e_{i} ,\quad \text {with }K_{i}, \alpha _{i}\text {, }\beta \ge 0, \end{aligned}$$

(1)

where $e_{i}$ represents the effective emissions of firm $i$ and $K_{i}$ is a fixed-cost term, whereas $\alpha _{i}$ and $\beta$ represent the intercept and the slope of $MC _{i}$ respectively. We allow for heterogeneity across firms by making the intercept firm-specific.¹³ Moreover, we introduce uncertainty in the model by assuming that $\alpha _{i}$ is a random variable that, as is usual in games of incomplete information, we refer to as the type of firm $i$.¹⁴ This assumption accounts for the fact that the abatement cost of a firm depends on a number of uncertain factors. Some of those factors are general, such as the weather, the level of economic activity or energy prices, but others might be firm-specific. Moreover, even if the general factors have a similar effect across firms, it is likely that the effect is not exactly the same due to specific firms’ characteristics (activity sector, size, technology, internal organization, etc.) and firms will typically have more accurate information than their rivals about the effects of those shocks on their own results. We capture this notion by assuming that firms have private information. Specifically, at the beginning of the game, each firm observes only its own type and the distribution of types is common knowledge.

After the initial allocation is made, the types are publicly revealed. Then the firms engage in the secondary market and trade allowances with complete information. To assume that the types are known in the secondary market but not in the auction amounts to the fact that transactions in the secondary market are far more frequent than auctions and hence it is realistic to assume that the agents handle more information in the secondary market.¹⁵ Summing up, the timing of the game is the following:

• 1. Each player $i$ observes its own type (realization of $\alpha _{i}$)

• 2. The planner initially allocates the permits. We consider two separate cases:

  • Option 1: Grandfathering (for free and based on BAU emissions)

  • Option 2: Auctioning $\left\{ \begin{array}{ll} 2.1. &{}\hbox {The players simultaneously submit their bids} \\ 2.2.&{}\hbox {The planner collects the bids and sets the opt-out price} \\ 2.3.&{} \hbox {The bidders pay the price and get the permits} \end{array} \right.$

• 3. The types are revealed to everyone

• 4. Secondary market $\left\{ \begin{array}{ll} 4.1.&{}\hbox {The leader sets the price} \\ 4.2.&{} \hbox {The follower decides its demand} \\ 4.3.&{}\hbox {The market clears} \end{array} \right.$

We assume that, without the CAP system, the firms would emit laissez-faire or BAU emissions, $e_{i}^{ BAU }$, i.e., the amount of emissions that would minimize $TC _{i}$. By making the marginal abatement cost equal to zero, we get¹⁶

$$\begin{aligned} e_{i}^{ BAU }=\frac{\alpha _{i}}{\beta }. \end{aligned}$$

(2)

For the sake of comparison, we compute the cost-effective allocation $\left( e_{L}^{CE}\text {, }e_{F}^{CE}\right)$, which follows from equating marginal abatement costs across firms and imposing $e_{L}+e_{F}=\bar{Q}$:

$$\begin{aligned} e_{L}^{CE}=\frac{\alpha _{L}-\alpha _{F}\,+\beta \bar{Q}}{2\beta }, \quad e_{F}^{CE}=\frac{\alpha _{F}-\alpha _{L}+\beta \bar{Q}}{2\beta }, \end{aligned}$$

(3)

where $CE$ stands for “cost-effective”. Note that, when both firms are identical ($\alpha _{F}=\alpha _{L}$), it is optimal that each firm gets $\frac{\bar{Q}}{2}$ permits, whereas if $\alpha _{i}>\alpha _{j}$, it is optimal that firm $i$ receives more permits than $j$.

Regarding the distribution of types, consider the $\alpha _{F}\alpha _{L}$ plane, in which $\alpha :=(\alpha _{F},\alpha _{L})$ represents an arbitrary point. On this plane, the support of the pair $\alpha$ is contained in a bounded square, denoted by $\Omega$, with the lower-left and upper-right corners denoted as $(\theta , \theta )$ and $\left( \theta +\sigma ,\theta +\sigma \right)$, respectively, where $\theta$, $\sigma >0$. Formally, $\Omega :=[\theta ,\theta +\sigma ]\times [\theta ,\theta +\sigma ]$, where $\theta$ captures the size and $\sigma$ accounts for (maximum) dispersion in the types. For technical reasons, we include the following assumption:

Assumption 1

The parameters of the model satisfy the following condition:

$$\begin{aligned} \frac{3}{2}\theta \ge \beta \bar{Q}\ge \sigma . \end{aligned}$$

(4)

Below we prove that Assumption 1 ensures that the solution in the secondary market is interior in the sense that, in equilibrium, each firm demands a quantity of permits that is non-negative and is smaller than its BAU emissions. To guarantee that this is true, we need, first, that the dispersion of the types’ distribution is not too large with respect to the bottom value and, second, that the total amount of permits, $\bar{Q}$, is not too large or too small to prevent, on the one hand, that it is profitable for any firm to demand more permits than the BAU emissions and, on the other, that the permits are so scarce that any firm prefers to keep a negative amount of them. As we show below, although (4) ensures an interior solution in the secondary market, it allows for corner solutions in the auction.

In the analysis of the auction, in order to get some additional insight into the properties of the equilibrium, we sometimes need to assume a specific form of the conditional expectation of the rival’s type and the joint distribution of the types. Thus, we also introduce the following assumption, which is used for some of the results.

Assumption 2

The conditional expectation of the rival’s type conditional on one’s own type is a linear function, denoting its coefficients as follows:

$$\begin{aligned} E\{\alpha _{i}\mid \alpha _{j}\}=\mu _{j}+\lambda _{j}\alpha _{j}, \end{aligned}$$

(5)

where $\{i, j\}$ is an arbitrary enumeration of $\{L,F\}$. Moreover, the distribution of $\left( \alpha _{F},\alpha _{L}\right)$ is uniform. Particularly, we consider three different cases that are consistent with (5):

• Case 1 (“Independent types”): uniform distribution on the whole square $\Omega$. In this case, we have

  $$\begin{aligned} \mu _{L}=\mu _{F}=\theta +\frac{\sigma }{2},\quad \lambda _{F}=\lambda _{L}=0. \end{aligned}$$

  (6)

• Case 2 (“$L$-ex ante efficient”): uniform distribution on the lower diagonal triangle of $\Omega$, i.e., uniform on all points satisfying $\alpha _{L}\le \alpha _{F}$. Therefore,

  $$\begin{aligned} \mu _{F}=\frac{\theta }{2},\quad \mu _{L}=\frac{\theta +\sigma }{2},\quad \lambda _{F}=\lambda _{L}=\frac{1}{2}. \end{aligned}$$

  (7)

• Case 3 (“$F$-ex ante efficient”): uniform distribution on the upper diagonal triangle of $\Omega$, i.e., uniform on all points satisfying $\alpha _{F}\le \alpha _{L}$. Thus,

  $$\begin{aligned} \mu _{F}=\frac{\theta +\sigma }{2},\quad \mu _{L}=\frac{\theta }{2},\quad \lambda _{F}=\lambda _{L}=\frac{1}{2}. \end{aligned}$$

  (8)

In case 1, the types are iid (which implies that the expected value of $\alpha _{i}$ is the same for both firms), whereas the types are positively correlated in cases 2 and 3. In case 2, we say that $L$ is ex-ante (more) efficient (than $F$) in the sense that the abatement cost function of $L$ is below that of $F$ with probability one (w.p.1). In case 3, $\alpha _{F}\le \alpha _{L}$ holds w.p.1 and we say that $F$ is ex-ante efficient. The implication of $\alpha _{i}\ge \alpha _{j}$ is that the marginal abatement cost function of firm $i$ is higher than that of firm $j$ , i.e., $MC _{i}(e) \ge MC _{j}(e)$ for any given value of $e$. The existence of cases 1, 2 or 3 may be due to technological reasons in the sense that $\alpha _{i}\ge \alpha _{j}$ means that firm $j$ has access to a more efficient abatement technology than $i$ does. Based on this interpretation, we choose the labels for cases 2 and 3 (“$L/F$ efficient”). This is not the only interpretation, though. For example, firm $i$’s $MC$ function could be higher simply for a matter of size: it produces more output and, hence, it is more costly for it to cut down emissions. However, since we are not dealing with the output market, but only with emissions, the former interpretation fits more naturally in our setting. The notation is summarized in “Appendix 1”.

2.2 The Secondary Market

Denote as $q_{i1}$ the amount of permits that firm $i$ holds after trading in the secondary market, which has to coincide with its realized emissions, $e_{i}$. Therefore, the amount of permits sold (if $q_{i0}>q_{i1}$) or bought (if $q_{i1}>q_{i0}$) in the secondary market by firm $i$ is $\left| q_{i0}-q_{i1}\right|$. The aim of both firms is to maximize their profits, considering both the revenue or the expenses due to permits trading and abatement costs, i.e.,

$$\begin{aligned} \Pi _{i}\left( q_{i1},p_{1},\varvec{\alpha }\right) :=p_{1}\left( q_{i0}-q_{i1}\right) - TC _{i}\left( q_{i1}\right) =p_{1}\left( q_{i0}-q_{i1}\right) -\int _{q_{i1}}^{e_{i}^{ BAU }} MC _{i}\left( e\right) de \end{aligned}$$

(9)

where $p_{1}$ is the price of permits in the secondary market. The follower chooses its net demand while taking $p_{1}$ as given whereas the leader takes into account the effect of its own behavior as well as the follower’s on the market price. We restrict ourselves to interior solutions in the secondary market, i.e., we require both $q_{L1}$ and $q_{F1}$ to be positive and lower than $\bar{Q}$ in equilibrium. Besides its tractability, assuming that the post-market holdings of permits are positive for every firm is fairly realistic. Moreover, to avoid meaningless solutions, we require $q_{i1}\le e_{i}^{ BAU }$ for all $i$, i.e., no firm will hold more permits than their BAU emissions and, hence, some abatement effort is required in equilibrium. Proposition 1 shows that the fulfilment of these requirements in equilibrium is guaranteed under Assumption 1.

Proposition 1

If Assumption 1 holds, the number of permits held by each firm in secondary-market equilibrium is given by

$$\begin{aligned} q_{L1}^{*}&= \frac{\alpha _{L}-\alpha _{F}}{3\beta }+\frac{\bar{Q}+q_{L0}}{ 3}, \end{aligned}$$

(10)

$$\begin{aligned} q_{F1}^{*}&= \frac{\alpha _{F}-\alpha _{L}+\beta \left( 2\bar{Q} -q_{L0}\right) }{3\beta }, \end{aligned}$$

(11)

and it is guaranteed that, for any initial allocation, $q_{i1}\in [0,\min \{\bar{Q},e_{i}^{ BAU }\}]$ holds w.p.1 for $i\in \{F,L\}$. The resulting profits are given by

$$\begin{aligned} \pi _{L}\left( q_{L0},{\varvec{\alpha }}\right)&:= \Pi _{i}\left( q_{i1}^{*},p_{1}^{*},\varvec{\alpha }\right) =\Theta _{L}+ \frac{\alpha _{L}+2\alpha _{F}-2\beta \bar{Q}}{3}q_{L0}+\frac{\beta }{6} q_{L0}^{2}, \end{aligned}$$

(12)

$$\begin{aligned} \pi _{F}\left( q_{F0},{\varvec{\alpha }}\right)&:= \Pi _{i}\left( q_{i1}^{*},p_{1}^{*},\varvec{\alpha }\right) =\Theta _{F}+ \frac{2\alpha _{L}+7\alpha _{F}-2\beta \bar{Q}}{9}q_{F0}-\frac{5\beta }{18} q_{F0}^{2}, \end{aligned}$$

(13)

where $\Theta _{L}$ and $\Theta _{F}$ are two terms that depend on the parameters of the model as well as the types but are independent of the initial allocation. $\square$

In Proposition 1, $\pi _{i}$ refers to the profit function of firm $i$ once the secondary market equilibrium is taken into account. From the results in Proposition 1, it is important to underline the fact that the equilibrium reached in the secondary market depends on the initial allocation. Moreover, as first noted by Hahn (1984), the only case in which the secondary market renders the cost-effective solution is that in which the leader initially receives exactly the amount of permits that corresponds to the cost-effective allocation. If this is the case, the secondary market is superfluous in the sense that no transaction will be made. In any other event, the role of the secondary market will be relevant. In fact, using (3) in (10), it is easy to verify that, if $q_{L0}<e_{L}^{CE}$, then $e_{L}^{CE}>q_{L1}>q_{L0}$. Symmetrically, if $q_{L0}>e_{L}^{CE}$, then $e_{L}^{CE}<q_{L1}<q_{L0}$. In other words, if the leader initially receives less permits than in the cost-effective allocation, it will act as a monopsonist in the secondary market and will buy less permits than what would be cost-effective. If, on the contrary, it receives more permits than $e_{L}^{CE}$, it will act as a monopolist and will sell less permits than required to reach cost-effectiveness.

Summing up, the initial allocation of permits is crucial in determining the equilibrium of the secondary market and its cost-effectiveness. The secondary market is found to be relevant insofar as the initial allocation is different from the cost-effective one. We claim that this is generally the case. Under grandfathering, if the planner had perfect information (in our framework, if he could observe the realizations of $\alpha _{F}$ and $\alpha _{L}$ from the outset), then it would be possible to compute the cost-minimizing solution and, by allocating the right amount of permits to each firm, cost-effectiveness would be achieved with certainty and the secondary market would be redundant. However, if the planner does not have perfect information about the firms’ technology and the effect of random shocks on each firms’ result (i.e., he acts under incomplete information), in general, he cannot allocate the cost-effective amount to each firm. Under auctioning, as discussed in the Introduction, the resulting allocation in a multi-unit, multi-bid auction with private value, is not necessarily efficient, which in our framework means that it is not cost-effective. This is consistent with our findings below.

For the analysis of the auction it is also important to note that, according to Proposition 1, $\pi _{F}$ is strictly concave in $q_{F0}$ (as one typically expects from a profit function), while $\pi _{L}$ is strictly convex in $q_{L0}$. The intuition for the shape of $L$’s profit function has to do with its ability to exert market power in the secondary market (by acting either as a monopolist or a monopsonist). As discussed previously, market power cannot be exerted when the initial allocation is the cost-effective one and the leader enjoys the highest market power when it gets all the permits (acting as a monopolist) or no permits at all (acting as a monopsonist). In Corollary 1 we show that total cost can be expressed as a function of the amount of permits initially assigned to the follower.

Corollary 1

The aggregate abatement cost can be written as the following quadratic function of $q_{F0}:$

$$\begin{aligned} TC \left( q_{F0},\varvec{{\alpha }}\right) := TC _{L}+ TC _{F}=\Theta + \frac{\alpha _{L}-\alpha _{F}-\beta \bar{Q}}{9}q_{F0}+\frac{\beta }{9} q_{F0}^{2}, \end{aligned}$$

(14)

where $\Theta$ is a term that depends on the parameters and the types, but not on the initial allocation. $\square$

We include $\varvec{\alpha }$ as an argument of $TC$ in (14) to emphasize its dependence on the types. Seeing as we focus on cost-effectiveness, $TC$ will be our comparison criterion to assess grandfathering and auctioning as alternative allocation mechanisms. From (14) it can be seen that calculating expected total cost under grandfathering is rather simple as $q_{F0}$ is exogenously given (decided by the planner) and therefore $\alpha _{L}$ and $\alpha _{F}$ are the only stochastic variables. The calculation is much more complex under auctioning however, as $q_{F0}$ comes out as an equilibrium result determined by the firms’ strategies, which are in turn driven by the realized types.

An implication of Corollary 1 is that the total cost entailed by an allocation system can be assessed simply by checking how close the initial allocation is to the cost-effective one. In the special case $\alpha _{L}=\alpha _{F}$ it is straightforward to conclude, once again, that the cost-minimizing distribution involves $q_{L0}=q_{F0}=\frac{\bar{Q}}{2}$. It is interesting to note that, although abatement cost depends on the final holdings of permits, $\left( q_{F1},q_{L1}\right)$, (14) only depends on the initial allocation, $\left( q_{F0},q_{L0}\right)$, as the secondary-market effect is implicitly incorporated. Actually, all the information we need about the secondary market is contained in (12), (13) and (14).¹⁷ For notational simplicity, we define the following monotone linear transformation of $TC$:

$$\begin{aligned} h(\delta ,{\varvec{\alpha }}):=\frac{9}{\bar{Q}}\left[ TC \left( q_{F0},{\varvec{\alpha }}\right) -\Theta \right] =\delta ^{2}\beta \bar{Q}+(\alpha _{L}-\alpha _{F}-\beta \bar{Q})\delta , \end{aligned}$$

(15)

which is a function of $\delta :=q_{F0}/\bar{Q}$, i.e., the proportion of permits initially assigned to $F$. For the sake of simplicity, and with a slight abuse of terminology, sometimes we shall sometimes refer to $h$ as “cost”, though it is actually a monotone linear transformation of it. This is innocuous given that we are using costs only to determine the relative position of auctioning and grandfathering, i.e., to ascertain which allocation mechanism is more cost-effective, and the relative position is not altered by a monotone transformation.¹⁸

3 Grandfathering

Grandfathering is usually applied by allocating permits based on observed past emissions as a proxy for BAU emissions. The idea is to take what firms would do if they were not subject to the CAP system as a benchmark. In practice, however, past emissions data are not always available or, even if the data exist, they might not be reliable enough, as it was the case, for example, in the first stage of the EU ETS (see the quotation from Ellerman et al. (2010) in the Introduction). To capture this idea in our model, we assume that, under grandfathering, permits are allocated based on BAU emissions, $e_{i}^{ BAU }=\frac{\alpha _{i}}{\beta }$ —see (2). However, as the planner cannot perfectly observe this value, he uses the best prediction of it, which is its expected value, $E\left\{ e_{i}^{ BAU }\right\} =\frac{E\left\{ \alpha _{i}\right\} }{\beta }$.

Therefore, each firm $i$ receives an initial endowment of permits given by a fraction of $\bar{Q}$ proportional to $E\left\{ e_{i}^{ BAU }\right\}$. Specifically, the follower receives $q_{F0}^{G}=\delta ^{G}\bar{Q}$ and the leader receives $q_{L0}^{G}=\left( 1-\delta ^{G}\right) \bar{Q}$, where $G$ stands for grandfathering and

$$\begin{aligned} \delta ^{G}:=\frac{E\left\{ \alpha _{F}\right\} }{E\left\{ \alpha _{L}\right\} +E\left\{ \alpha _{F}\right\} }. \end{aligned}$$

(16)

As a particularly simple case, consider that $E\left\{ \alpha _{L}\right\} =E\left\{ \alpha _{F}\right\}$, which implies $\delta ^{G}=\frac{1}{2}$. Using (15) and taking expected values, we obtain the total cost under grandfathering and its (unconditional) expected value:

$$\begin{aligned} h(\delta ^{G},\varvec{\alpha })=\frac{2\left( \alpha _{L}-\alpha _{F}\right) -\beta \bar{Q}}{4}, \quad E\{h(\delta ^{G},\varvec{\alpha })\}=-\frac{\beta \bar{Q}}{4}. \end{aligned}$$

(17)

4 Auctioning

Let us now assume that the permits are auctioned. Based on the observed features of real auctions of permits, we assume a uniform auction format, which means that all the awarded units pay the stop-out price.¹⁹ Moreover, we assume that both $L$ and $F$ act non-strategically in the auction (i.e., both act as price-takers). There are two main reasons to assume that firms are non-strategic in the auction. The first reason is simplicity, since strategic behavior is much more complex to tackle, particularly in a multi-unit multi-bid setting with private value. The second reason is that we intentionally intend to explore a setting that seems a-priori favorable for the auction by setting both firms on an equal footing. In fact, if the leader could act strategically, it would probably take advantage of this capacity to position itself at an even more advantageous position to exert its market power, which would tend to generate a stronger distortion.²⁰

Seeing as there are multiple units to be auctioned and every bidder can request more than one unit, we are faced with a multi-unit multi-bid auction. As usual in auction theory, a strategy for firm $i$ is a mapping from the support of firm $i$’s type into the set of feasible bids. Specifically, in our model, a strategy for firm $i$ is a demand function of the form $q_{i}^{b}(\alpha _{i},p_{0})$, where $b$ stands for “bid” and the required amount, $q_{i}^{b}$, depends on the auction price, $p_{0}$, and firm $i$’s type, but not on the rival’s type. As the total amount of auctioned permits is $\bar{Q}$, we further assume that the demanded quantity submitted by the bidders must lie in $[0,\bar{Q}]$, i.e., firms are not individually allowed to submit a demanded quantity which is either negative or larger than $\bar{Q}$ at any price. Let us denote as $v_{i}\left( q_{i0},\alpha _{i}\right) :=E\{\pi _{i}\left( q_{i0},{\varvec{ \alpha }}\right) \mid \alpha _{i},q_{i0}\}$ the value function of firm $i$ at the auction, i.e., the expected value that $i$ assigns to get $q_{i0}$ permits in the auction conditional on its own type, where $\pi _{i}$ is given by (12) or (13).²¹ Since the value function depends on $i$’s beliefs regarding its rival’s type, its bid optimal also depends on such beliefs. Hence, $i$’s best strategy is defined as

$$\begin{aligned} q_{i}^{b*}(\alpha _{i},p_{0})\underset{q_{i0}\in \left[ 0,\bar{Q}\right] }{:=\arg \max }\{v_{i}\left( q_{i0},\alpha _{i}\right) -p_{0}q_{i0}\}, \end{aligned}$$

(18)

i.e., for each firm, the optimal bid is the demand function that maximizes its value function net of the cost incurred to get $q_{i0}$ permits in the auction. An equilibrium in the auction is a pair of best strategies, $q_{F}^{b*},$ $q_{L}^{b*}$, and a stop-out price $p_{0}^{*}$, defined as $p_{0}^{*}:=max\{p_{0}\mid q_{F}^{b*}(\alpha _{F},p_{0})\, +\, q_{L}^{b*}(\alpha _{L},p_{0})\ge \overline{Q}\}$ for all $(\alpha _{F},\alpha _{L})$. This (standard) definition states that there is no a higher price than $p_{0}^{*}$ such that all the permits are awarded while both firms follow their best strategies. The latter inequality allows for excess demand at the stop-out price, (which, as we show below, occurs at some equilibria). If so, a standard pro-rata formula is used, i.e., each player $i$ receives a proportion $\frac{q_{i}^{b}}{q_{i}^{b}+q_{j}^{b}}$ of the permits. As the auction is uniform, all the awarded units pay $p_{0}^{*}$. Proposition 2 characterizes the optimal bidding functions.

Proposition 2

If Assumption 1 holds, there exist three threshold values, $p_{Fu}$, $p_{Fd}$ and $p_{L}$, where $p_{Fu}>$ $p_{Fd}$, such that the optimal bidding functions of $F$ and $L$ are given, respectively, by

$$\begin{aligned} q_{F}^{b*}(\alpha _{F},p_{0})&= \left\{ \begin{array}{l@{\quad }l} 0 &{} \text {if}\quad p_{0}\ge p_{Fu}, \\ \frac{2E\{\alpha _{L}\mid \alpha _{F}\}+7\alpha _{F}-2\beta \bar{Q}-9p_{0}}{5\beta } &{} \text {if}\quad p_{Fd}<p_{0}<p_{Fu}, \\ \bar{Q} &{} \text {if}\quad p_{0}\le p_{Fd}, \end{array} \right. \end{aligned}$$

(19)

$$\begin{aligned} q_{L}^{b*}(\alpha _{L},p_{0})&= \left\{ \begin{array}{l@{\quad }l} 0 &{} \text {if}\quad p_{0}>p_{L}, \\ \bar{Q} &{} \text {if}\quad p_{0}\le p_{L}. \end{array} \right. \end{aligned}$$

(20)

$\square$

By evaluating $q_{F}^{b*}\left( \alpha _{F},p_{0}\right)$ in $p_{Fd}$ and $p_{Fu}$ [see expression (28) in the proof of Proposition 2], it is straightforward to show that the follower’s optimal bidding function is continuous in the auction price, $p_{0}$. Note that $F$ demands no permits when $p_{0}$ is high enough, it demands the maximum amount, $\bar{Q}$, when $p_{0}$ is low enough, and it demands something in between for intermediate values of $p_{0}$. Also note that $q_{F}^{b*}$ is decreasing in the price, as expected. Moreover, it is increasing both in $\alpha _{F}$ and the expected value of $\alpha _{L}$. Given that $\alpha _{F}$ shifts the follower’s abatement cost up, it is reasonable to expect that the higher $\alpha _{F}$, the more $F$ is willing to pay for the permits. Regarding $\alpha _{L}$, although this parameter affects $L$’s, and not $F$’s cost, the follower forecasts that a higher value of $\alpha _{L}$ will make the permits more valuable in the secondary market, which would also make it more willing to pay in the auction. Finally, $q_{F}^{b*}$ depends negatively on the total amount of issued permits, $\bar{Q}$, as the greater the number of permits issued, the easier it will be to obtain cheaper permits in the secondary market.

As for the leader’s strategy, given that $v_{L}$ is strictly convex in $q_{L0}$, its problem only has corner solutions and therefore its optimal bidding function is discontinuous: $L$ will demand all the permits if the price is high enough and none otherwise. The interpretation of this result is that it is optimal for the leader to position itself in the best possible situation to exert its market power in the secondary market (by acting either as a monopolist or a monopsonist). As discussed previously, such market power cannot be exerted when the initial allocation is the cost-effective one. Therefore, the leader is interested in placing itself as far as possible from the cost-effective allocation. This can be done either by demanding too few or too many permits. It is optimal to do the former if the price is high enough and to do the latter if the price is low enough.

Proposition 3

If Assumption 1 holds, the auction has a unique equilibrium that belongs to one of the following three types:

$$\begin{aligned}&type\,1{:} \quad q_{L0}^{*}(\alpha _{L},p_{0}^{*})=0\,and\,q_{F0}^{*}(\alpha _{F},p_{0}^{*})=\bar{Q},\,with\,p_{0}^{*}=p_{Fd}, \\&type\,2{:} \quad q_{L0}^{*}(\alpha _{L},p_{0}^{*})=\bar{Q}\,and\, q_{F0}^{*}(\alpha _{F},p_{0}^{*})=0,\,with\,p_{0}^{*}=p_{L}, \\&type\,3{:} \quad q_{L0}^{*}(\alpha _{L},p_{0}^{*})=\bar{Q}\,and\, q_{F0}^{*}(\alpha _{F},p_{0}^{*})\in \left[ 0,\bar{Q}\right] ,\,with\,p_{0}^{*}=p_{L}. \end{aligned}$$

Type-1 equilibrium occurs iff $\xi <-1$, type-2 equilibrium occurs iff $\xi >1$, and type-3 equilibrium occurs iff $-1\le \xi \le 1$, where

$$\begin{aligned} \xi :=\frac{6\alpha _{L}-14\alpha _{F}+12E\{\alpha _{F}\mid \alpha _{L}\}-4E\{\alpha _{L}\mid \alpha _{F}\}}{5\beta \bar{Q}}. \end{aligned}$$

(21)

$\square$

According to Proposition 3, the interaction of the bidders gives rise to three different types of equilibria, the occurrence of which is governed by the ratio $\xi$. Notice that $\xi$ weights $L$’s incentives to bid against $F$’s. In our model, $L$ has two reasons to bid aggressively. It does so if (i) it has a high abatement cost (high $\alpha _{L}$), and/or (ii) it believes—conditional on its own type—that its rival has a high cost (high $E\{\alpha _{F}\mid \alpha _{L}\}$). The first reason encourages $L$ to increase its bid in order to save on abatement costs, whereas the second has to do with the expected value of permits in the secondary market. If $L$ expects that $F$ has a high valuation of the permits, it also anticipates the possibility to make a larger profit by selling permits in the secondary market at a high price. Proposition 3 shows that when $L$’s incentives to bid aggressively are sufficiently stronger than $F$’s reasons to do so—or equivalently, when $\xi$ is large enough—type-2 equilibrium occurs and thus $L$ is awarded all the permits. An analogous reasoning applies for small enough values of $\xi$: $F$’s incentives being stronger than $L$’s tends to generate type-1 equilibrium, in which $F$ gets all the permits. When both firm’s incentives are similar enough, type-3 equilibrium tends to arise and the permits are shared between both firms. The denominator of $\xi$ indicates that those equilibria in which a single firm gets all the permits are more unlikely as the total amount of permits gets larger.

Note also that Proposition 3 does not require imposing any assumption regarding the form of the conditional expectations involved (the proposition uses Assumption 1 but not Assumption 2). Different forms imply different results regarding the impact of changes in the bidder’s own type. Consider the three cases introduced in Assumption 2. In case 1, the expected value of the rival’s type is constant and, thus, a change in the bidder’s own type will reinforce effect (i) and leave (ii) unchanged. In cases 2 and 3, the expectation depends positively on the own type (which we consider to be the most realistic situation) and hence both effects are the same sign. In all three cases, an increase in the own type unambiguously leads to a more aggressive bidding. However one could also consider (at least theoretically) a case in which the types are negatively correlated and hence conditional expectation depends negatively on the own type. Then, a higher value of one’s own type would mean, on the one hand, that one’s own abatement costs are higher, but also that one’s rival’s costs (and hence, its valuation of the permits) are expected to be lower, and it is not obvious that one’s optimal response would be to bid more aggressively or not.²²

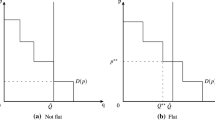
Figure 1 displays the aggregate demand for permits in the auction, given by $q_{F}^{b}\,+\,q_{L}^{b}$. The figure shows that different values of $\xi$ result in different relative positions for the threshold values $p_{Fu\text {, }}$ $p_{Fd}$ and $p_{L}$. The equilibrium point is identified with a circle. Recall that the opt-out price is defined as the highest price at which all the permits are awarded. In the left panel of the figure, we have the $\xi <-1$ case, in which $p_{L}<p_{Fd}$, which implies that, at price $p_{Fd}$, $F$ demands all the permits and $L$ demands zero. Thus, in equilibrium, $F$ receives all $\bar{Q}$ permits, i.e., $\delta ^{A}=1$, where $A$ stands for “auction”. The central panel illustrates the $\xi >1$ case, in which $p_{L}>p_{Fu}$. The equilibrium involves $p_{0}=p_{L}$, at which $F$ demands zero, $L$ demands $\bar{Q}$ and hence $\delta ^{A}=0$. Finally, the right panel illustrates the $-1\le \xi \le 1$ case, when $p_{Fd}\le p_{L}\le p_{Lu}$. The equilibrium involves $p_{0}=p_{L}$, at which the leader demands $\bar{Q}$ and the follower demands a positive quantity lower than $\bar{Q}$. The third case implies that there is excess demand and rationing is required, with $\delta ^{A}\in \left[ 0,\frac{1}{2} \right]$.

Fig. 1

Types of equilibria in the auction depending on the value of $\xi$. Each panel shows the aggregate demand for permits (resulting from the aggregation of $q_{F}^{b}$ and $q_{L}^{b}$). The supply is rigid at $\bar{Q}$. The equilibrium point is identified by a circle. When all $\bar{Q}$ permits are awarded for more than one price, the relevant one is the highest

Note that type-1 equilibrium resembles Antelo and Bru’s (2009) Proposition 1, which states that, in a complete-information model, it is always optimal for the leader to bid zero in the auction (see also Montero 2009). We show that, under incomplete information, this is a possible result that arises if type-1 equilibrium prevails, but it could be the other way around. In fact, in the other two types of equilibrium, it is optimal for $L$ to bid $\bar{Q}$. Moreover, under type-2 equilibrium, the leader obtains all the permits.

In the rest of this section we analyze each of the three cases considered in Assumption 2 separately.

4.1 Independent Types (Case 1)

We now analyze the likelihood of the three types of equilibria and the comparison with grandfathering when the types are independently distributed, as stated in the first case of Assumption 2. We still restrict ourselves to the case in which the secondary market equilibrium is interior and both firms exert a positive abatement effort, i.e., we assume that (4) holds. The following notation is used below. Consider the square $\Omega$, which contains the support of the pair $(\alpha _{F},\alpha _{L})$. Let us define the diagonal of $\Omega$ as the set $D:=\{(\alpha _{F},\alpha _{L})\ /\ \alpha _{F}=\alpha _{L}=\theta +a\sigma ,\;a\in [0,1]\}$. In other words, the diagonal is the set of feasible realizations of the types such that both firms’ costs functions are identical. Additionally, let $\kappa :=\frac{\beta \bar{Q}}{\sigma }$. Notice that $\kappa$ is a ratio that depends positively on a measure of the market size given by the total amount of permits ($\bar{Q}$) weighted by the impact of each permit on the marginal abatement cost ($\beta$) and depends negatively on the variability of the types ($\sigma$). Note also that (4) implies $\kappa \ge 1$. Let us denote as $\Omega _{l}$ the region in which type-$l$ equilibrium takes place, where $l\in \{1,2,3\}$. All the results displayed up to now relied only on Assumption 1. To compute the probability of the equilibria, we now require some distributional information and hence we also need to apply Assumption 2.

Proposition 4

If Assumption 1 holds and the firms’ types are mutually independent (i.e., case 1 in Assumption 2 holds), then i) in $D$ there are only type-3 equilibria; ii) given a realization of $\varvec{\alpha }$, if it gives rise to type-1 equilibrium, there will be also type-1 equilibrium for any convex combination of $\varvec{\alpha }$ and $\left( \theta +\sigma ,\theta \right)$; iii) if $\varvec{\alpha }$ gives rise to type-2 equilibrium, there will be also type-2 equilibrium for any convex combination of $\varvec{\alpha }$ and $\left( \theta ,\theta +\sigma \right)$; and iv) type-1 and type-2 equilibria are equiprobable, with

$$\begin{aligned} \Pr (\Omega _{1})=\Pr (\Omega _{2})=\left\{ \begin{array}{l@{\quad }l} \frac{25}{42}\left( \frac{2-k}{2}\right) ^{2} &{} if\,\kappa <2 \\ 0 &{} otherwise; \end{array} \ \right. \, \end{aligned}$$

(22)

(v) conditional on $\Omega _{1}$ and $\Omega _{2}$, the auction entails lower expected costs than grandfathering, i.e.,

$$\begin{aligned} E\{h(\delta ^{A},{\varvec{\alpha }})\mid \Omega _{1}\}<E\{h(\delta ^{G},{\varvec{\alpha }})\mid \Omega _{1}\} ,\quad E\{h(\delta ^{A},{\varvec{\alpha }})\mid \Omega _{2}\}<E\{h(\delta ^{G},{\varvec{\alpha }})\mid \Omega _{2}\}. \end{aligned}$$

$\square$

As a general conclusion, Proposition 4 states that type-3 equilibria are much more likely than type-1 and type-2. There is always a positive probability of type-3 equilibrium arising, which can be computed as $\Pr (\Omega _{3})=1-2\Pr (\Omega _{1})$ and it is the only possible equilibrium on the diagonal. Moreover, if $\kappa \ge 2$ holds, $Pr(\Omega _{3})=1$. If, on the contrary, $\kappa <2$, all three types of equilibrium can arise with positive probability, although type-3 equilibrium is always the most likely one. In fact, by taking $\kappa =1$ in (22), which is the lowest value consistent with (4), i.e., the most favorable for type-1 and type-2 equilibria, we still get $Pr(\Omega _{3})>0.7$. To understand why type-3 is the most likely equilibrium, notice that the other two types require that one of the firms bids zero and the other bids $\bar{Q}$, which in turn requires one of the firms valuing the permits much more than the other one. However, the maximum asymmetry in the firm’s valuation, which is determined by $\sigma$, cannot be too large, seeing as $\sigma$ it is bounded by Assumption 1 to ensure interior solution in the secondary market. Notice also that the probability of type-1 and type-2 equilibria depends negatively on $\kappa$. Given that $\kappa$ increases with the market size, it is rather natural that a higher $\kappa$ makes it less likely that a single firm can obtain all the permits.

Another consequence of Proposition 4 is related to the location of each type of equilibrium in the feasible region of types. As can be seen in Fig. 2, whenever $\kappa <2$ holds, the realizations under which type-1 equilibria occur are located close to the bottom right corner (when $\alpha _{F}$ is well above $\alpha _{L}$) and type-2 equilibria close to the top left corner (when $\alpha _{L}$ is well above $\alpha _{F}$).

Fig. 2

Equilibria for independent types (case 1) when $\kappa <2$. Type-1, -2 and -3 equilibria arise in the green, yellow and blue regions, respectively. (Color figure online)

Finally, the proposition also states that, in expected terms, the auction outperforms grandfathering in the ranges where type-1 and type-2 equilibria arise. To understand this result, it is important to notice that, although $\delta ^{G}$ does not depend on the realization of the types (specifically, under independent types, $\delta ^{G}=\frac{1}{2}$), to make a fair comparison between grandfathering and auctioning, we need to evaluate the expected value of $h\left( \delta ^{G},{\varvec{\alpha }}\right)$ conditional on the set of realizations of $\alpha$ such that each equilibrium takes place. Recall that type-1 (type-2) equilibrium tends to arise when $\alpha _{F}$ is well above $\alpha _{L}$ ($\alpha _{L}$ is well above $\alpha _{F}$). In this case, $F$’s ($L$’s) marginal abatement cost is higher enough than $L$’s ($F$’s) and, therefore, a situation in which $F$ ($L$) holds all the permits is likely to be closer to the cost-effective allocation than a situation in which both firms receive $\frac{\bar{Q}}{2}$.

By direct comparison between the possible allocations resulting from the auction and the cost-effective allocation given in (3), it is obvious that, under type-1 equilibrium, the leader will receive less permits in the auction than what would be cost-effective (and will therefore act as a monopsonist in the secondary market) w.p.1 and, under type-2 equilibrium, it will receive more permits than in the cost-effective allocation (and will hence be a monopolist in the secondary market) w.p.1. Corollary 2 shows that, in the case in which type-3 equilibrium holds w.p.1 (i.e., $\kappa \ge 2$), the leader becomes over-assigned with respect to the cost-effective allocation w.p.1 (and hence will act again as a monopolist).

Corollary 2

Under Assumption 1 and independent types (case 1 in Assumption 2), if $\kappa \ge 2$, $L$ gets over-assigned in the auction with respect to its cost-effective allocation of permits w.p.1. $\square$

As discussed previously, under type-1 and type-2 equilibria, auctioning is more cost-effective than grandfathering in expected terms. To make a general assessment of auctioning relative to grandfathering, we need to evaluate costs under all three possible types of equilibria, weighted by their respective probabilities. Unfortunately, under type-3 equilibrium no general analytical statements can be made due to the complex analytical form of expected cost.²³ Hence, we now proceed with some numerical analysis.

We evaluate total abatement costs under auctioning and grandfathering for each combination of parameter values, $(\theta ,\beta \bar{Q},\sigma )$.²⁴ For each parameter combination, we generate 1,000 random realizations of the pair of types.²⁵ For each realization, we compute the corresponding equilibrium at the auction and the associated realized costs under auctioning and grandfathering. We first check how different realizations of the types determine the relative cost of both allocation methods. We then average across realizations to obtain an estimation of the expected cost of each system.

We exhaustively explore the parameter space defined by (4). A first result is that variations in $\theta$ are irrelevant for the cost comparison insofar as it is large enough to ensure that the set defined by (4) is non-empty. The reason is that modifying the value of $\theta$ is a change of origin that equally affects both firms’ marginal costs, but not the difference between them. Once the value of $\theta$ is fixed, the set of parameter values consistent with (4) is bounded, which eases the exploration. Accordingly, we simply present the results for a specific value, $\theta =10$, and explore a grid of values of the pair $(\beta \bar{Q},\sigma )$ such that (4) is respected.

Figure 3 shows an illustration of the relative performance of auctioning and grandfathering in terms of cost for different realizations of the pair of types, given a specific combination of the parameters. The upper (resp. lower) panel displays the combinations such that auctioning renders lower (higher) cost than grandfathering. Two major insights can be gained from this figure.

Fig. 3

Realized Costs with $(\sigma ,\beta \bar{Q})=(6,8)$ and $\theta =10$. Each dot corresponds to a realization of $\varvec{\alpha }$ and is displayed in the upper (resp. lower) panel if, for that realization, the total cost under auctioning is smaller (higher) than that under grandfathering. In the upper panel, the colors range from yellow to red, where yellow means both costs are roughly similar to each other and red means a larger difference from one to another. In the lower panel, the colors range from yellow to blue, where yellow once again means similar costs and blue means a larger difference. (Color figure online)

First, those cases in which the difference between the marginal cost of both firms is large enough tend to favour auctioning while, if the firms’ marginal costs are similar enough, grandfathering is less costly than the auction. To interpret this result, it is useful to identify two effects that can be labelled as the “information effect” and “market power effect”. Regarding the former, note that via the bids, the auction allocation incorporates more information on the realized types (which are privately observed), whereas grandfathering only uses expected values. This effect is likely to favour the auction. As for the latter effect, the auction gives the leader the opportunity to bid so as to position itself in a more advantageous position to exert its market power more aggressively in the secondary market, which tends to aggravate market distortion and result in higher costs when the permits are auctioned. When the types are very close to each other, the cost-effective allocation involves allocating similar amounts of permits to both firms (at the limit, if $\alpha _{L}=\alpha _{F}$, the optimal allocation requires $\delta =\frac{1}{2}$) and so, grandfathering is likely to generate an allocation that is very close to the first best (recall that grandfathering renders a constant value $\delta ^{G}=\frac{1}{2}$). In this case, the lack of information on the realized types is not a major problem because the expected value is a good enough proxy and hence the market power effect dominates. The opposite happens when the marginal costs are very different from each other. In this case, the mean is a poor proxy for the realized values and the auction is likely to perform better than grandfathering, because the potential gain of using more precise information can be higher than the loss due to market power distortion.

Second, provided that the types are sufficiently different, when it is the leader’s marginal cost which is above the follower’s ($\alpha _{L}>\alpha _{F}$), the range under which auctioning beats grandfathering is larger than in the opposite case ($\alpha _{F}>\alpha _{L}$). In other words, the chances of the auction rendering a good result in terms of costs are better when the firm with the lower marginal abatement costs is not the same as the one that has market power. The intuition of this result is that, if $L$ enjoys a cost advantage, this would reinforce its leadership, which it would use to bias the market result for its own profit and this distortion would weaken the beneficial information effect. The situation is more balanced when the follower can counterbalance its disadvantageous position by having lower costs than the leader.

We now move on to compare expected costs, computed as the average across realizations. Our numerical analysis reveals that, for all the parameter values satisfying (4), grandfathering outperforms the auction in expected terms. Given that Proposition 4 states that, in type-1 and type-2 equilibria auctioning outperforms grandfathering, it has to be the case that grandfathering outperforms auctioning on average in type-3 equilibria, which is confirmed by our numerical results. Moreover, recall that the probability of type-1 and type-2 equilibria is always smaller than that of type-3 equilibrium. As we have discussed in Sect. 2.2, the fact that auctioning entails higher costs than grandfathering means that, on average, the former renders allocations that are further away from the cost-effective distribution than the latter. This fact is illustrated in Fig. 4, which depicts these distances for each realization of the pair of types under the same combination of parameter values considered in Fig. 3. In this example, the vast majority of the equilibria are type-3, and the basic message of the figure is to confirm the theoretical result (see Corollary 2) that, in the auction, $L$ gets over-assigned (or, equivalently, $F$ under-assigned) with respect to the cost-effective allocation whenever type-3 equilibria occur.

Fig. 4

Allocations in terms of $\delta :=\frac{q_{F0}}{\bar{Q}}$, with independent types and $(\sigma ,\beta \bar{Q})=(6,8)$ and $\theta =10$. The green points represent auction versus cost-effective allocations for each realization of $\varvec{\alpha }$, thus the closer these points are to the diagonal (red line), the more cost-effective the auction allocation is. The blue line represents the grandfathering allocation (which is constant and equal to $1/2$). Grandfathering is cost-effective if $\delta ^{CE}=\frac{1}{2 }$ (which corresponds to $\alpha _{L}=\alpha _{F}$) and $L$ gets over-assigned (resp. under-assigned) if $\delta ^{CE}>\frac{1}{2}$ ($\delta ^{CE}<\frac{1}{2}$). In the vast majority of realizations, $\delta ^{A}<\delta ^{CE}$, i.e., auctioning allocates more permits to the leader than what is cost-effective. (Color figure online)

In terms of sensitivity analysis, our results suggest that the difference between auction and grandfathering increases with $\kappa$. In short, a small variability of the types (as measured by $\sigma$ in terms of the market size) with respect to the amount of permits tends to favour grandfathering over the auction. The reason is that a small variability implies that the average will be a good proxy for the actual value of the types and hence the grandfathering allocation is likely to be very close to the cost-effective one.

4.2 Non-independent Types (Cases 2 and 3)

We now study cases 2 and 3 in Assumption 2. In both of these cases, the types are positively correlated. This is a reasonable event to consider, as it might well be the case that different firms are affected similarly by external shocks. For example, it is commonly believed that, in the first years of the EU ETS, the cap was too mild in the sense that too many permits were distributed. The evolution of the energy markets and the weather led to an excess of permits or, in other words, meeting environmental requirements turned out to be easier than expected for most facilities. This is not to say that the effect of these conditioning factors were exactly the same for all firms, as there were clear asymmetries among them, but it seems safe to state that these effects worked roughly in the same direction. Propositions 5 and 6 contain our main analytical findings for these cases.

Proposition 5

Under Assumption (1), if $\alpha _{L}\le \alpha _{F}$ holds w.p.1 (case 2 in Assumption 2) then i) type-1 equilibria occur with positive probability if and only if $\kappa <2$ and do not occur for any $\varvec{\alpha }$ in $D$; ii) if type-1 equilibrium occurs for some pair $\varvec{\alpha }$, type-1 equilibrium also occurs for any convex combination of $\varvec{\alpha }$ and $\left( \theta , \theta +\sigma \right)$; iii) type-2 equilibria occur with positive probability if and only if $\kappa < \frac{6}{5}$; iv) if type-2 equilibrium occurs for some pair $\varvec{\alpha }$, type-2 equilibrium also occurs for any convex combination of $\varvec{\alpha }$ and $\left( \theta , \theta \right)$, and v) type-3 equilibria always occur with positive probability. $\square$

Proposition 6

Under Assumption (1), if $\alpha _{F}\le \alpha _{L}$ holds w.p.1 (case 3 in Assumption 2) then i) type-1 equilibria occur with positive probability if and only if $\kappa <\frac{6}{5}$; ii) if type-1 equilibrium occurs for some $\varvec{\alpha }$, type-1 equilibrium also occurs for any convex combination of $\varvec{\alpha }$ and $(\theta +\sigma ,\theta +\sigma )$; iii) type-2 equilibria occur with positive probability if and only if $\kappa <2$, and do not occur for any $\varvec{\alpha }$ in $D$; iv) if type-2 equilibrium occurs for some pair $\varvec{\alpha }$, type-2 equilibrium also occurs for any convex combination of $\varvec{\alpha }$ and $\left( \theta , \theta +\sigma \right)$, and v) type-3 equilibria always occur with positive probability. $\square$

Let us first consider case 2 ($L$ efficient). The regions that lead to the different equilibria are shown in Fig. 5 panel a. The main message of this figure (together with Proposition 5) is that the range for type-2 equilibrium to emerge shrinks and could even disappear. In fact, if $\frac{6}{5}<\kappa <2$, then there will be type-1 and type-3 equilibria with positive probability, but not type-2 ($\Pr \left( \Omega _{2}\right) =0$). The reason is that $L$ being more efficient ($\alpha _{L}\le \alpha _{F}$) with certainty, it is more unlikely to value the permits so high that it bids strong enough to get all the permits in equilibrium (which happens in type-2 equilibrium). However, it is noticeable that even in this case, if the market size is small enough, there is still a positive probability that, in equilibrium, the leader obtains all the permits.

Fig. 5

Equilibrium configuration for cases 2 and 3 when $\kappa$ takes a value to enable all three types of equilibria. Type-1, -2 and -3 equilibria arise in the green, yellow and blue regions, respectively. (Color figure online)

Regarding case 3 ($F$ efficient). Figure 5 panel b shows the relative position of the realizations of the types leading to each possible equilibrium. In this case, it is the follower who has a more efficient abatement technology and it is more likely to be in less need for permits than the leader. Thus, the range for type-1 equilibrium (in which $F$ holds all the permits) shrinks and could even disappear although, once again, if the market size is small enough, such an event can arise with positive probability.

We now proceed to evaluate the relative cost of auctioning and grandfathering in cases 2 and 3. The more complex structure of these cases prevent us from obtaining analytical results and so we proceed with numerical analysis. Case 2 renders qualitatively identical results to case 1. In short, although there are realizations of the types that result in lower costs under auctioning than under grandfathering, the latter always outperforms the former in expected terms.

Let us now consider case 3. Figure 6 shows that, unlike the two previous cases, there are parameter values for which the expected cost under auctioning is smaller than under grandfathering. Those points are shown in green in the graph. The implication of this result is that, as discussed above, it is favorable for the auction that the agent endowed with market power is not the one with the lowest, but the highest cost, as any cost advantage would make it more capable to exploit its market power. Another message we can get from this analysis is that auctioning tends to do better, as compared to grandfathering, when the variability of types, as measured by $\sigma$, is large enough in terms of $\beta \bar{Q}$, which is in line with the analysis carried out for the independent-types case.

Fig. 6

Expected costs under auctioning ($A$) and grandfathering ($G$) in case 3. For each realization, we compute the expected cost under auctioning ($A$) and grandfathering ($G$) and average across 1,000 random realizations. A red (green) dot indicates that $G$ ($A$) entails a lower expected cost. In cases 1 and 2, all the dots would be red. (Color figure online)

Figure 7 shows a case in which the auction leads to lower expected cost. For ease of comparison with the case of independent types, we take as an example $(\sigma ,\beta \bar{Q})=(6,8)$ and $\theta =10$ (the same parameter values as those used in Figs. 3, 4). As in the case with independent types, $L$ is still over-assigned in the auction with respect to the cost-minimizing allocation (represented by the red line), but now the allocations resulting from the auction (represented by green dots) are, on average, closer to being cost-effective than those resulting from grandfathering (the blue line).

Fig. 7

Allocations for type-3 equilibria in case 3 with $(\sigma ,\beta \bar{Q})=(6,8)$ and $\theta =10$. The interpretation is the same as in Fig. 4. (Color figure online)

5 Conclusions, Discussion and Future Research

This paper compares the two most common allocation mechanisms of emission permits, auctioning and grandfathering, under two central assumptions: the existence of a secondary market with market power and the presence of incomplete information at the auction. On the one hand, we claim that the presence of these two elements is essential for the comparison between both methods to be non-trivial. On the other hand, it is rather realistic to assume that both elements are present to some extent in important real-life examples such as the Kyoto Protocol or the EU ETS.

One reason to be interested in this comparison is that there seems to be an increasing interest in auctioning as an alternative to grandfathering in order to allocate emission permits (the EU ETS being a notable example). This paper offers some insights into what we can really expect the auction to do in a relatively adverse scenario: market power and incomplete information. Within the framework of a simple model, we study optimal bidding strategies as well as equilibria and characterize the conditions under which auctioning does better or worse than grandfathering in terms of aggregate abatement cost.

The advantage of auctioning versus grandfathering is due essentially to the fact that, by means of bids, the auction allocation incorporates information that is only privately observable and hence could not be used by the planner to implement a centralized allocation. On the other hand, the auction gives the leader the possibility of bidding in order to increase its chances to exert market power in the secondary market and thus distort the equilibrium in its own benefit. The final balance depends on the relative strength of these two forces.

The auction tends to be more cost-effective than grandfathering when the firms are very asymmetric in terms of cost, especially if the firm with cost advantage is not the same one that enjoys market power. In this case, the additional information used by the auction tips the scales in its favour. In expected terms, the chances for the auction to outperform grandfathering increase when the follower’s costs are well below the leader’s and the variability of the types is large enough in terms of the number of permits to be distributed.

The aim of this paper is to provide some general insights about cap-and trade systems and not about one specific market such as the EU ETS (in which, moreover, it is not that clear that market power is one of the main issues). Nevertheless, for the sake of motivation, it has been useful for us to take as a starting point the EC’s argument in support of auctioning on the grounds of efficiency (although we have focused on the milder criterion of cost-effectiveness by taking the cap as given). Although our analysis is strongly focused on cost-effectiveness, it can also provide some clues about the plausibility of the EC’s other arguments in support of auctioning: transparency, simplicity, more incentives for abatement investments and the elimination windfall profits.

The transparency argument makes sense given that auctioning automatically incorporates more information than grandfathering, since the bids contain privately observed data that will not normally be accessible to the environmental authorities. Moreover, auctioning has the advantage of treating facilities more evenly whereas, in the two first phases of the EU ETS, grandfathering was applied through the so-called National Allocation Plans, which have inevitably introduced some across-countries and across-facilities asymmetries in permit allocation.

Regarding simplicity, it is by no means obvious that auctioning is simpler than grandfathering. From the planner’s point of view, auctioning incorporates a clearer market-based dimension to the allocation procedure, which frees the environmental authorities to some extent from the responsibility of making direct allocations. On the other hand, however, it entails some additional complexities in the setup of the system, such as choosing the design of the auction (e.g., uniform or discriminatory), creating auction platforms or deciding about the destiny of revenue. From the firms’ point of view, it requires more frequent interaction between the participants and the authorities and probably some additional training to be able to bid properly.

Concerning the introduction of incentives for abatement investments, according to our results this will probably be truer for firms without market power, which will typically bear higher costs and will hence have stronger incentives to improve their abatement technologies. Finally, the elimination of windfall profits is supported, to some extent, by the mere fact that firms will have to pay for the permits and, therefore, it is no longer the case that operators charge their customers the cost of allowances they have received for free. Nevertheless, the fact that allowances are not free of charge does not fully eliminate the possibility of windfall profits in the form of speculative operations. We conclude that, in most cases, the allocation resulting from an auction will be further away from being cost-effective than that resulting from grandfathering. This implies that marginal abatement costs will be more different across facilities, hence creating room for windfall profits. What can probably be argued is that windfall profits will be smaller on average and concentrated in fewer hands, specifically in the hands of those firms that enjoy some market power. Summing up, our analysis seems to support the EC’s arguments of transparency and more incentives for abatement investments, but not those of simplicity and the elimination of windfall profits. Obviously, these thoughts must be taken as a preliminary and incomplete informal discussion, not as a fully-fledged analysis, which is left for future work.

There are several interesting issues that have not been addressed in this paper and offer possible lines for future work. First, we have not addressed the links between the permit market and the output market. Some authors have shown that, even if the permit market is perfectly competitive, cost-effectiveness is typically not achieved if the product market is not perfectly competitive. For example, Ehrhart et al. (2008) show that collusion to manipulate prices in the product market may occur even if the firms are price takers in the permit market. In fact, under some conditions, a permit price increase leads to higher profits due to a decrease in output quantities, which in turn increases the output price. In the industrial organization literature, this strategy is generally known as “raising rivals’ costs”. Ehrhart et al. (2008) argue that in EU ETS, even if there is no explicit market power in the permit market, there are loopholes in the trading law that allow collusive behavior among firms to push the price of permits upwards. Although this movement has the direct effect of raising one’s own cost, as it also raises the rival’s, both firms could benefit by restricting the quantity of output and increasing its price. An explicit study of the connections between abatement and production would entail additional complexities such as the type of competition that would prevail in the output market.

Second, we do not consider the possibility that firms hold less permits than needed and hence we are implicitly assuming perfect enforcement. Another interesting line of research is to consider imperfect enforcement and consider the optimal design of the enforcement policy, as it is done, for example, in Malik (1990), Stranlund and Dhanda (1999) and Macho-Stadler (2008). In this respect, it would also be interesting to analyze the possibility that the regulator could progressively learn about the specific characteristic of the firms (which would progressively alleviate information incompleteness) and determine the optimal environmental policy within such a framework.

Appendices

Appendix 1: Notations

Fundamentals
$\bar{Q}$	Total amount of permits to be shared, exogenous
$i,j\in \left\{ F,L\right\}$	Firms: $F$ and $L$ denote follower and leader, respectively
$MC _{i}\left( e\right) =\alpha _{i}-\beta e$	Marginal abatement cost of firm $i$, where $e$ is the emission level
$\alpha _{i}$, $\varvec{\alpha }=\left( \alpha _{F},\alpha _{L}\right)$	Firms’ types, random. The support of $\varvec{\alpha }$ belongs to $\Omega := \left[ \theta ,\theta +\sigma \right] ^{2}$
$D$	Diagonal of $\Omega$, i.e., $D:=\left\{ \left( \alpha , \alpha \right) \in \Omega \right\}$
$\kappa :=\frac{\beta \bar{Q}}{\sigma }$	Ratio market size to across-firm heterogeneity
$E\{\alpha _{i}\mid \alpha _{j}\}=\mu _{j}+\lambda _{j}\alpha _{j}$	Expectation of $\alpha _{i}$ conditional on $\alpha _{j}$, assumed linear with coeffs. $\mu _{j},\lambda _{j}$
$e_{i}^{ BAU }$, $e_{i}^{CE}$	Business as usual and cost-effective emissions of $i$, respectively
$\xi ,\Theta ,\Theta _{L},\Theta _{F}$	Auxiliary coefficients
Secondary market
$q_{i1},$ $p_{1}$	Post-market holdings of permits for firm $i$ and market price, respectively
$\Pi _{i}\left( q_{i1},p_{1},\varvec{\alpha }\right)$	Realized profit of firm $i$
$\pi _{i}\left( q_{i0},\varvec{\alpha }\right) :=\Pi _{i}\left( q_{i1}^{*},p_{1}^{*},\varvec{\alpha }\right)$	Realized profit of firm $i$ (under eq. in the secondary market) (the asterisk denotes equilibrium value)
$v_{i}\left( q_{i0},\alpha _{i}\right)$	Value function for firm $i$ (valuation of initially getting $q_{i0}$ permits)
Primary market (auctioning or grandfathering)
$q_{i0}$	Initial allocation of permits for firm $i$ (under grand. or auctioning)
$p_{0}$	Price of permits at the auction
$\delta :=\frac{q_{F0}}{\bar{Q}}$	$F^{\prime }$s share of permits ($\delta ^{A}$, $\delta ^{G}$ under auctioning or grandf., respectively)
$h\left( \delta ,\varvec{\alpha }\right)$	Monotone transformation of total abatement cost (incorporates secondary-market behavior)
$q_{i}^{b}\left( \alpha _{i},p_{0}\right)$	Firm $i$’s bid (mapping from support of $\alpha _{i}$ to set of demand funct.)
$\Omega _{l},$ $l=1,2,3$	Set of types’ values such that type-$l$ equilibrium occurs
$\left( p_{L},p_{Fu},p_{Fd}\right)$	Auxiliary variables to define equilibrium strategies at the auction

Appendix 2: Proofs

1.1 Proof of Proposition 1

Let us initially assume that the solution is interior (which we check below). The optimal behavior of the follower is derived by maximizing (9) for $i=F$ while taking $p_{1}$ as given. From the first-order condition (FOC), we get the follower’s net demand for permits:

$$\begin{aligned} q_{F1}^{d}\left( p_{1}\right) =\frac{1}{\beta }\left( \alpha _{F}-p_{1}\right) , \end{aligned}$$

(23)

where $d$ stands for “demand”. The leader’s problem is to maximize (9) for $i=L$ including the market clearance condition, $q_{L1}+q_{F1}=\bar{Q}$, and the follower’s demand, (23), as constraints. The (interior) solution to this problem is given by (10). Using the market clearing condition and (23), we get the equilibrium price in the secondary market,

$$\begin{aligned} p_{1}^{*}=\frac{\alpha _{L}+2\alpha _{F}-\beta \left( 2\bar{Q} -q_{L0}\right) }{3}, \end{aligned}$$

(24)

and using (24) in (23) we obtain the equilibrium number of permits for the follower, given by (11). Using (10), (11) and (24) in the expressions for $F$’s and $L$’s profits and rearranging, we get (12) and (13), where $\Theta _{L}$ and $\Theta _{F}$ include the terms that do not depend on the initial allocation.

To show that the solution satisfies $q_{i1}\in [0,\min \{\bar{Q} ,e_{i}^{ BAU }\}]$ w.p.1, note first that, by construction, $0\le q_{F1}\le \bar{Q}$ ensures $0\le q_{L1}\le \bar{Q}$ and using (23) we have that $0\le q_{F1}\le \bar{Q}\iff \alpha _{F}\ge p_{1}\ge \alpha _{F}-\beta \bar{Q}$, which, using (24), can be written as $\frac{\alpha _{F}-\alpha _{L}}{\beta } +2\bar{Q}\ge q_{L0}\ge \frac{(\alpha _{F}-\alpha _{L})}{\beta }-\bar{Q}$. As $q_{L0}\in [0,\bar{Q}]$, the previous inequalities are ensured for any initial allocation iff $\frac{\alpha _{F}-\alpha _{L}}{\beta }+2\bar{Q} \ge \bar{Q}$ and$\ 0\ge \frac{(\alpha _{F}-\alpha _{L})}{\beta }-\bar{Q}$ hold at the same time. The intersection of these inequalities is: $\bar{Q} \ge \frac{1}{\beta }|\alpha _{L}-\alpha _{F}|$, which is guaranteed if

$$\begin{aligned} \beta \bar{Q}\ge \sigma \end{aligned}$$

(25)

as the maximum value of $|\alpha _{L}-\alpha _{F}|$ is $\sigma$.

Firm $i$ makes a strictly positive abatement effort iff $q_{i1}\le e_{i}^{ BAU }=\frac{\alpha _{i}}{\beta }$. If we particularize this inequality for $i=F$, using (23), we have $p_{1}\ge 0$ , which, using (24), can be written as $\alpha _{L}+2\alpha _{F}\ge \beta (2\bar{Q}-q_{L0})$. As $q_{L0}\in [0,\bar{Q}]$ and the minimum value for each type is $\theta$, the latter equality is guaranteed under (4). For $i=L$, using (10), $q_{L1}\le \frac{\alpha _{L}}{\beta }$ can be written as

$$\begin{aligned} 2\alpha _{L}+\alpha _{F}\ge \beta (\bar{Q}+q_{L0}). \end{aligned}$$

(26)

The most adverse values for the fulfilment of (26) are $\alpha _{L}=\alpha _{F}=\theta$, $q_{L0}= \bar{Q}$. To ensure that (26) holds w.p.1, we plug these values to conclude $\theta \ge \frac{2}{3}\beta \bar{Q}$. Combining this inequality with (25), we get (4), which holds by assumption.

1.2 Proof of Corollary 1

Total cost can be computed by inserting (10) and (11) in (1).²⁶ Substituting $q_{L0}$ by $\bar{Q} -q_{F0}$ and rearranging, we get (14), where $\Theta$ includes the terms that do not depend on the initial allocation.

1.3 Proof of Proposition 2

Using (13), $v_{F}\left( q_{F0},\alpha _{F}\right)$ can be written as

$$\begin{aligned} v_{F}\left( q_{F0},\alpha _{F}\right) =E\left\{ \Theta _{F}\mid \alpha _{F}\right\} +\frac{1}{9}\left( 2E\{\alpha _{L}\mid \alpha _{F}\}+7\alpha _{F}-2\beta \bar{Q}\right) q_{F0}-\frac{5}{18}\beta q_{F0}^{2}. \end{aligned}$$

As $v_{F}$ is strictly concave in $q_{F0}$ for every $\alpha _{F}$, an interior solution to $F$’s problem (if it exists) follows easily from the first-order condition, which can be solved to get

$$\begin{aligned} q_{F}^{b}=\tau _{F}\left( q_{F0},\alpha _{F}\right) :=\frac{2E\{\alpha _{L}\mid \alpha _{F}\}+7\alpha _{F}-2\beta \bar{Q}-9p_{0}}{5\beta }, \end{aligned}$$

(27)

where, for simplicity of exposition, we have denoted as $\tau _{F}\left( q_{F0},\alpha _{F}\right)$, the right-hand side of the previous equality. Since the submitted demand must lie in $[0,\bar{Q}]$, and given the concavity of $v_{F}$ on $q_{F0}$, we conclude that $q_{F}^{b}=0$ and $q_{F}^{b}=\bar{Q}$ whenever $\tau _{F}\left( p_{0},\alpha _{F}\right) \le 0$ and $\tau _{F}\left( p_{0},\alpha _{F}\right) \ge \bar{Q}$, respectively. Note also that $\tau _{F}\left( p_{0},\alpha _{F}\right)$ is strictly decreasing in $p_{0}$ for every $\alpha _{F}$, hence $p_{Fu}$ and $p_{Fd}$ are defined by $\tau _{F}(p_{Fu},\alpha _{F})=0$ and $\tau _{F}(p_{Fd},\alpha _{F})=\bar{Q}$, respectively. Thus, we have

$$\begin{aligned} p_{Fu}=\frac{2E\{\alpha _{L}\mid \alpha _{F}\}+7\alpha _{F}-2\beta \bar{Q}}{9 }\ ,\quad p_{Fd}=\frac{2E\{\alpha _{L}\mid \alpha _{F}\}+7\alpha _{F}-7\beta \bar{Q}}{9}. \end{aligned}$$

(28)

Let us now consider $L$’s problem, which is $max_{q_{L0}}\{v_{L}\left( q_{L0},\alpha _{L}\right) -p_{0}q_{L0}\}$ where

$$\begin{aligned} v_{L}\left( q_{L0},\alpha _{L}\right)&= E\{\pi _{L}\left( q_{L0},\varvec{ \varvec{\alpha }}\right) \mid \alpha _{L},q_{L0}\}=E\left( \Theta _{L}\mid \alpha _{L}\right) \\&+\,\frac{\alpha _{L}+2E\{\alpha _{F}\mid \alpha _{L}\}-2\beta \bar{Q}}{3}q_{L0}\,+\,\frac{\beta }{6}q_{L0}^{2}. \end{aligned}$$

Clearly $v_{L}\left( q_{L0},\alpha _{L}\right)$ is strictly convex in $q_{L0}$ for every $\alpha _{L}$, and therefore the leader’s problem only has corner solutions, i.e., $q_{L}^{b}\in \{0,\bar{Q}\}$ with $q_{L}^{b}=\bar{Q} \iff v_{L}\left( \bar{Q},\alpha _{L}\right) \ge v_{L}\left( 0,\alpha _{L}\right)$. Given that $v_{L}\left( 0,\alpha _{L}\right) =E\left\{ \Theta _{L}\mid \alpha _{L}\right\}$, $L$ chooses $q_{L0}=\bar{Q}$ whenever $v_{L}\left( \bar{Q},\alpha _{L}\right) \ge E\left\{ \Theta _{L}\mid \alpha _{L}\right\} +p_{0}\bar{Q}$ holds. Let us denote as $p_{L}$ the maximum value of $p_{0}$ such that this inequality holds, i.e., $p_{L}=\frac{ v_{L}\left( \bar{Q},\alpha _{L}\right) -E\left\{ \Theta _{L}\mid \alpha _{L}\right\} }{\bar{Q}}$. Using the expression for $v_{L}$ we have that

$$\begin{aligned} p_{L}:=\frac{\alpha _{L}+2E\{\alpha _{F}\mid \alpha _{L}\}}{3}\,-\,\frac{1}{2} \beta \bar{Q}. \end{aligned}$$

(29)

1.4 Proof of Proposition 3

Given that the support of $\alpha _{L}$ and $\alpha _{F}$ is $\left[ \theta ,\theta +\sigma \right]$, from (29) we know $p_{L}>\theta -\frac{1}{2 }\beta \bar{Q}>0$, where the latter inequality follows from (4). Thus, there exists at least one positive price ($p_{L}$) at which the demanded quantity is not smaller than supply, which guarantees the existence of an equilibrium in the auction. Uniqueness follows from the fact that each firm’s demand is non-increasing in $p_{0}$ for every possible pair $\left( \alpha _{F},\alpha _{L}\right)$. Seeing as $p_{Fu}>p_{Fd}$ and $p_{L}>0$ hold w.p.1, there are just three possible cases.

• First case: $p_{L}<p_{Fd}$. In this case, the stop-out price cannot be $p_{0}>p_{Fd}$ because there would be excess supply (i.e., some units would not be awarded). As all the permits would be awarded for any $p_{0}\le p_{Fd}$, the opt-out price must be $p_{Fd}$. At this price, $F$ bids $\bar{Q}$, $L$ bids zero and hence $F$ gets all the permits and $L$ gets nothing. This corresponds to type-1 equilibrium.

• Second case: $p_{Fu}<p_{L}$. In this case, for any $p_{0}>p_{L}$ there would be excess supply and all the permits would be awarded for any $p_{0}\le p_{L}$. Therefore, the opt-out price must be $p_{L}$. At this price, $L$ bids $\bar{Q}$ and $F$ bids zero. Thus, $L$ gets all the permits, which corresponds to type-2 equilibrium.

• The third case is $p_{Fd}\le p_{L}\le p_{Fu}$, which corresponds to type-3 equilibrium, in which permits are shared between $F$ and $L$. The equilibrium price is $p_{L}$, at which $F$ and $L$ demand $\tau _{F}(p_{L},\alpha _{F})\in \left[ 0,\bar{Q}\right]$ and $\bar{Q}$, respectively, and rationing is required. Using (28) and (29), straightforward algebra leads to $p_{L}<p_{Fd}\iff \xi <-1$, $p_{Fu}<p_{L}\iff \xi >1$ and $p_{Fd}\le p_{L}\le p_{Fu}\iff -1\le \xi \le 1$.

1.5 Proof of Proposition 4

We split the proof into the following parts:

Part i)

Under independent types, using (5), (6), (21) and the definition of $\kappa$, the conditions given in Proposition 3 for type-1 and type-2 equilibria collapse to

$$\begin{aligned} \text {type 1:}&{\qquad }\xi <-1\Leftrightarrow 3\alpha _{L}-7\alpha _{F}+4\theta +2\sigma +\frac{5\sigma \kappa }{2}<0, \end{aligned}$$

(30)

$$\begin{aligned} \text {type 2:}&{\qquad }\xi >1\Leftrightarrow 7\alpha _{F}-3\alpha _{L}-4\theta -2\sigma +\frac{5\sigma \kappa }{2}<0. \end{aligned}$$

(31)

For any pair in $D$, (30) and (31) become, respectively

$$\begin{aligned} 5\kappa <8a-4, \quad 5\kappa <4-8a, \end{aligned}$$

none of which, under (4), holds for any admissible values of $\kappa$ and $a$. Thus, we only have type-3 equilibria in $D$, which proves the first statement in the proposition.

Parts ii) and iii)

The results in these parts straightforwardly follow from the fact that conditions (30) and (31) define two convex sets, that $\left( \alpha _{F},\alpha _{L}\right) =\left( \theta +\sigma ,\theta \right)$, is the most favorable pair to satisfy (30) and that $\left( \alpha _{F},\alpha _{L}\right) =\left( \theta ,\theta +\sigma \right)$, is the most favorable pair to satisfy (31).

Part iv)

To compute the probabilities of type-1 and type-2 equilibria, notice first that the pair $\left( \alpha _{F},\alpha _{L}\right) =\left( \theta +\sigma ,\theta \right)$, which is the most favorable pair to satisfy (30), satisfies it if and only if $\kappa <2$ holds. Similarly, the pair $\varvec{\alpha }=\left( \theta ,\theta +\sigma \right)$ , which is the most favorable pair to satisfy (31), satisfies it if and only if $\kappa <2$ holds. Contrarily, if $\kappa \ge 2$ holds, (30) and (31) never hold and hence $\Pr (\Omega _{1})=\Pr (\Omega _{2})=0$.

Let us now consider the $\kappa <2$ case. Under the uniform distribution assumption, the probability of type-$i$ equilibria is proportional to the area of $\Omega _{i}$ (for $i=1,2$).

Regarding $\Omega _{1}$, as shown in Fig. 2, this area is a triangle in the bottom right corner of $\Omega$. To compute the size of this triangle, we first compute the infimum value for $\alpha _{F}$ in $\Omega _{1}$, which can be obtained by taking $\alpha _{L}=\theta$ in (30) and solving for $\alpha _{F}$ to obtain

$$\begin{aligned} \alpha _{F}^{Inf}:=\theta +n_{F}\sigma , \quad \text { where }\quad n_{F}:= \frac{4+5\kappa }{14}. \end{aligned}$$

Similarly, the supremum value for $\alpha _{L}$ in $\Omega _{1}$ is obtained by taking $\alpha _{F}=\theta +\sigma$ in (30) and solving to obtain

$$\begin{aligned} \alpha _{L}^{Sup}:=\theta +\frac{5\left( 2-\kappa \right) }{6}\sigma . \end{aligned}$$

Using these values, we can obtain the area of $\Omega _{1}$:

$$\begin{aligned} A(\Omega _{1}):=\frac{1}{2}\left( \theta +\sigma -\alpha _{F}^{Inf}\right) \left( \alpha _{L}^{Sup}-\theta \right) =\frac{25\sigma ^{2}}{42}\left( 1-\frac{\kappa }{2} \right) ^{2} \end{aligned}$$

and bearing in mind that the size of the square $\Omega$ is equal to $\sigma ^{2}$, the probability of type-1 equilibrium is $\Pr (\Omega _{1})= \frac{1}{\sigma ^{2}}A(\Omega _{1})$.

Regarding $\Omega _{2}$, as shown in Fig. 2, this region is a triangle in the top left corner of $\Omega$. Proceeding as above, from (30) we can compute the infimum value of $\alpha _{L}$ and the supremum value of $\alpha _{F}$ within $\Omega _{2}$:

$$\begin{aligned} \alpha _{L}^{Inf}=\theta +\frac{5\kappa -4}{6}\sigma \text {, }\qquad \alpha _{F}^{Sup}=\theta +\frac{5\left( \kappa -2\right) }{14}\sigma . \end{aligned}$$

Using the relevant expressions, it is straightforward to check that $\theta +\sigma -\alpha _{F}^{Inf}=\alpha _{F}^{Sup}-\theta$ and $\alpha _{L}^{Sup}-\theta =\theta +\sigma -\alpha _{L}^{Inf}$, i.e., $\Omega _{2}$ is simply a $180 {{}^o}$-rotation of $\Omega _{1}$ and, therefore, both areas are identical, which, jointly with the uniform distribution assumption, implies $\Pr (\Omega _{1})=\Pr (\Omega _{2})$.

Part v)

Notice that, if both types are independent, under grandfathering we always have

$$\begin{aligned} \delta ^{G}=\frac{q_{F0}^{G}}{\bar{Q}}=\frac{E\{e_{F}^{*}\}}{ E\{e_{F}^{*}\}+E\{e_{L}^{*}\}}=\frac{1}{2}. \end{aligned}$$

Let us first consider type-1 equilibria. In this case we know that the follower gets all the permits and, therefore, $\delta ^{A}=1$. Using $\kappa \sigma =\beta \bar{Q}$ and the values of $\delta ^{G}$ and $\delta ^{A}$ in (15), we have that

$$\begin{aligned}&E\{h(\delta ^{G},{\varvec{\alpha }}) \mid \Omega _{1}\}=E\{h(1/2,{\varvec{\alpha }})\mid \Omega _{1}\}=\frac{1}{2} E\{\alpha _{L}-\alpha _{F}\mid \Omega _{1}\}-\frac{\kappa \sigma }{4},\\&E\{h(\delta ^{A},{\varvec{\alpha }}) \mid \Omega _{1}\}=E\{h(1, {\varvec{\alpha }})\mid \Omega _{1}\}=E\{\alpha _{L}-\alpha _{F}\mid \Omega _{1}\}, \end{aligned}$$

and direct comparison reveals that

$$\begin{aligned} E\{h(\delta ^{A},{\varvec{\alpha }})\mid \Omega _{1}\}<E\{h(\delta ^{G},{\varvec{\alpha }})\mid \Omega _{1}\}\iff E\{\alpha _{L}-\alpha _{F}\mid \Omega _{1}\}<-\frac{\kappa \sigma }{2}. \end{aligned}$$

(32)

Therefore, to determine the relative expected costs we need to compute $E\left\{ \alpha _{L}-\alpha _{F}\mid \Omega _{1}\right\}$. The first step is to obtain the conditional distributions of $\alpha _{F}\mid \Omega _{1}$ and $\alpha _{L}\mid \Omega _{1}$. As regards $\alpha _{F}\mid \Omega _{1}$, note that the relevant support is the interval $[\alpha _{F}^{Inf},\theta +\sigma ]$. For any arbitrary value of $\alpha _{F}$ in that interval, say $\alpha$, according to (30) we know that $\alpha _{L}$ ranges (within $\Omega _{1}$) from $\theta$ to $\hat{\alpha }_{L}(\alpha ):= \frac{7\alpha -4\theta }{3}-\frac{\left( 4+5\kappa \right) \sigma }{6}$. Thus, the joint probability of the events “$\alpha _{F}=\alpha$” and “type-1 equilibrium occurring” is²⁷

$$\begin{aligned} \Pr (\alpha _{F}=\alpha \cap \Omega _{1})=\frac{1}{\sigma ^{2}}\int _{\theta }^{\hat{\alpha }_{L}(\alpha )}dz=\frac{1}{\sigma ^{2}}\left( \hat{\alpha } _{L}(\alpha )-\theta \right) . \end{aligned}$$

We now apply Bayes’ rule to obtain the conditional density

$$\begin{aligned} \Pr (\alpha _{F}=\alpha \mid \Omega _{1})=\frac{\Pr (\alpha _{F}=\alpha \cap \Omega _{1})}{\Pr (\Omega _{1})}=7k_{0}^{F}\left( \alpha -\theta -n_{F}\sigma \right) \end{aligned}$$

where $k_{0}^{F}=\left( 3A(\Omega _{1})\right) ^{-1}$. The corresponding conditional expectation is

$$\begin{aligned} E\{\alpha _{F}\mid \Omega _{1}\}=\int _{\alpha _{F}^{Inf}}^{\theta +\sigma }\alpha \Pr (\alpha _{F}=\alpha \mid \Omega _{1})d\alpha =7k_{0}^{F}\int _{\alpha _{F}^{Inf}}^{\theta +\sigma }\left\{ \alpha ^{2}- \left[ \theta +n_{F}\sigma \right] \alpha \right\} d\alpha \end{aligned}$$

which, after some straight-forward algebra, results in

$$\begin{aligned} E\{\alpha _{F}\mid \Omega _{1}\}=\theta +\left( \frac{5\kappa +32}{42} \right) \sigma . \end{aligned}$$

(33)

We proceed analogously with $\alpha _{L}\mid \Omega _{1}$. Its support is $[\theta ,\alpha _{L}^{Sup}]$. For any arbitrary $\alpha$ in that interval, from (30) we conclude that $\alpha _{F}$ ranges (within $\Omega _{1}$) from $\hat{\alpha }_{F}(\alpha ):=\frac{3}{7}\alpha + \frac{4}{7}\theta +\frac{1}{7}\left( 2+\frac{5}{2}\kappa \right) \sigma$ to $\theta +\sigma$.

The joint probability of the events “$\alpha _{L}=\alpha$” and “type-1 equilibrium occurring” is

$$\begin{aligned} \Pr (\alpha _{L}=\alpha \cap \Omega _{1})=\frac{1}{\sigma ^{2}}\int _{\hat{ \alpha }_{F}(\alpha )}^{\theta +\sigma }dz=\frac{1}{\sigma ^{2}}\left( \theta +\sigma -\hat{\alpha }_{F}(\alpha )\right) \end{aligned}$$

and using Bayes’ rule,

$$\begin{aligned} \Pr (\alpha _{L}=\alpha \mid \Omega _{1})=k_{0}^{L}\left( \theta -\alpha + \frac{5}{3}\left( 1-\frac{1}{2}\kappa \right) \sigma \right) \end{aligned}$$

where $k_{0}^{L}=3\left( 7\sigma ^{2}\Pr (\Omega _{1})\right) ^{-1}$. The corresponding conditional expectation is

$$\begin{aligned} E\{\alpha _{L}\mid \Omega _{1}\}=\int _{\theta }^{\alpha _{L}^{Sup}}\alpha \Pr (\alpha _{L}=\alpha \mid \Omega _{1})d\alpha =\theta +\frac{5\left( 2-\kappa \right) }{18}\sigma . \end{aligned}$$

(34)

Using (33) and (34), we have that

$$\begin{aligned} E\{\alpha _{L}-\alpha _{F}\mid \Omega _{1}\}=-\frac{13+25\kappa }{63}\sigma \end{aligned}$$

(35)

and using (35) in (32) we conclude

$$\begin{aligned} E\{h(\delta ^{A},\varvec{{\alpha }})\mid \Omega _{1}\}\le E\{h(\delta ^{G},\varvec{{\alpha }})\mid \Omega _{1}\}\iff \kappa \le 2 \end{aligned}$$

where the latter inequality is trivially true if $\Omega _{1}$ is nonempty (which requires $\kappa <2$).

Under type-2 equilibrium, we know that the leader obtains all the permits and, therefore, $\delta ^{A}=0$. Using this value and $\delta ^{G}=\frac{1}{2 }$ in (15), we have that

$$\begin{aligned}&E\{h(\delta ^{A},{\varvec{\alpha }}) \mid \Omega _{2}\}=E\{h(0,{\varvec{\alpha }})\mid \Omega _{2}\}=0, \\&E\{h(\delta ^{G},{\varvec{\alpha }}) \mid \Omega _{2}\}=E\{h(1/2,{\varvec{\alpha }})\mid \Omega _{2}\}=\frac{1}{2} E\{\alpha _{L}-\alpha _{F}\mid \Omega _{2}\}-\frac{1}{4}\kappa \sigma \end{aligned}$$

and comparing both expressions,

$$\begin{aligned} E\{h(\delta ^{A},{\varvec{\alpha }})\mid \Omega _{2}\}<E\{h(\delta ^{G},{\varvec{\alpha }})\mid \Omega _{2}\}\Leftrightarrow E\{\alpha _{L}-\alpha _{F}\mid \Omega _{2}\}>\frac{ \kappa \sigma }{2}. \end{aligned}$$

(36)

To compute $E\{\alpha _{L}-\alpha _{F}\mid \Omega _{2}\}$, we can proceed as we did for equilibrium one (obtaining the conditional distributions and then computing the conditional expectations of $\alpha _{L}$ and $\alpha _{F}$ ). However, it is possible to exploit the rotational symmetry property between areas $\Omega _{1}$ and $\Omega _{2}$ (see part iii) above) to show ²⁸

$$\begin{aligned} E\{\alpha _{L}-\alpha _{F}\mid \Omega _{2}\}=-E\{\alpha _{L}-\alpha _{F}\mid \Omega _{1}\}=\frac{7+31\kappa }{63}\sigma \end{aligned}$$

and using this value in (36), we conclude that

$$\begin{aligned} E\{h(\delta ^{A},{\varvec{\alpha }})\mid \Omega _{2}\}<E\{h(\delta ^{G},{\varvec{\alpha }})\mid \Omega _{2}\}\Leftrightarrow E\{\alpha _{L}-\alpha _{F}\mid \Omega _{2}\}>\frac{ \kappa \sigma }{2}\Leftrightarrow \kappa >-14 \end{aligned}$$

and the last inequality is trivially true because $\kappa$ is positive by construction.

1.6 Proof of Corollary 2

Our strategy to prove the corollary is to show that, provided that $\kappa \ge 2$ holds, for any arbitrary realization $\varvec{\alpha }:=(\alpha _{F},\alpha _{L})$, we have $\delta ^{A}<\delta ^{CE}=\frac{\alpha _{F}\,-\,\alpha _{L}\,+\,\beta \bar{Q}}{2\beta \bar{Q}}$, where the last equality comes directly from (3).

Under type-3 equilibrium, the auction allocation is given by $\delta ^{A}:= \frac{\tau _{F}\left( q_{F0},\alpha _{F}\right) }{\tau _{F}\left( q_{F0},\alpha _{F}\right) \,+\,\overline{Q}}$, where $\tau _{F}\left( q_{F0},\alpha _{F}\right)$ is defined in (27). Using this expression for $\delta ^{A}$, we have that

$$\begin{aligned} \delta ^{A}<\delta ^{CE}\iff \beta \tau _{F}\left( q_{F0},\alpha _{F}\right) \le \frac{\delta ^{CE}}{1-\delta ^{CE}}\beta \bar{Q}. \end{aligned}$$

(37)

We now evaluate $\tau _{F}\left( q_{F0},\alpha _{F}\right)$ in the equilibrium price $p_{0}^{*}$, which, under type-3 equilibrium is equal to $p_{L}$, where $p_{L}$ is given by (29). Using the expression for $\delta ^{CE}$ together with (5) and, using (6), (37) can be written as

$$\begin{aligned} \delta ^{A}<\delta ^{CE}\iff \frac{7\alpha _{F}-3\alpha _{L}-4\theta -2\sigma }{5}<\frac{3(\alpha _{F}-\alpha _{L})+\beta \overline{Q}}{2(\beta \overline{Q}+\alpha _{L}-\alpha _{F})}\beta \overline{Q}. \end{aligned}$$

Notice that we can write $\alpha _{i}=\theta +r_{i}\sigma$ for $i\in \{F,L\}$, where $r_{i}$ is uniformly distributed in $[0,1]$, and there is a one-to-one mapping between $\alpha _{i}$ and $r_{i}$. Additionally, we use the definition of $\kappa$ to write $\beta \overline{Q}=\kappa \sigma$. Making these substitutions in the latter inequality, we get rid of $\theta$ and $\sigma$ to obtain

$$\begin{aligned} \delta ^{A}<\delta ^{CE}\iff 7r_{F}-3r_{L}-2<\frac{3(r_{F}-r_{L})+\kappa }{ \kappa -(r_{F}-r_{L})}\cdot \frac{5}{2}\kappa . \end{aligned}$$

(38)

To check that this inequality holds for any pair $(\alpha _{F},\alpha _{L})$ or, equivalently, for any pair $(r_{F},r_{L})$, we distinguish between two cases. Let us first consider first $r_{F}\ge r_{L}$. Notice that $7r_{F}-3r_{L}-2\le 3(r_{F}-r_{L})+2$ and that

$$\begin{aligned} \frac{3(r_{F}-r_{L})+\kappa }{\kappa -(r_{F}-r_{L})}\ge \frac{ 3(r_{F}-r_{L})+2}{\kappa -(r_{F}-r_{L})} \end{aligned}$$

where we have used $\kappa \ge 2$. Thus, a sufficient condition for (38) to hold is

$$\begin{aligned} 3(r_{F}-r_{L})+2<\frac{3(r_{F}-r_{L})+2}{\kappa -(r_{F}-r_{L})}\frac{5}{2} \kappa . \end{aligned}$$

(39)

If $r_{F}\ge r_{L}$, the left-hand side of (39) is positive and we can divide both sides by $3(r_{F}-r_{L})+2$ and rearrange to get $\frac{3}{2}\kappa >r_{L}-r_{F}$, which trivially holds when $\kappa \ge 2$ and $r_{F}\ge r_{L}$.

Let us now consider $r_{F}<r_{L}$. Notice that the right-hand side in (38) increases with $\kappa$, so it suffices to prove the inequality for $\kappa =2$. Introducing this value of $\kappa$ and rearranging, we have that

$$\begin{aligned} -7r_{F}^{2}-3r_{L}^{2}+r_{F}+7r_{L}+10r_{F}r_{L}-14<0. \end{aligned}$$

(40)

A sufficient condition for (40) to hold is that both of the following inequalities hold:

$$\begin{aligned} r_{F}+7r_{L}-8&\le 0 \end{aligned}$$

(41)

$$\begin{aligned} -7r_{F}^{2}-3r_{L}^{2}+10r_{F}r_{L}-6&< 0 \end{aligned}$$

(42)

given that, if both (41) and (42) hold, (40) follows from the sum of them both. Clearly, (41) holds as $r_{F}$, $r_{L}\in [0,1]$. To prove ( 42), we define $s:=\frac{r_{F}}{r_{L}}$, where $s\in [0,1]$ and rewrite (42) as $(-7s^{2}-3+10s)r_{L}^{2}-6<0$. For any $r_{L}$, the expression inside the parenthesis attains its maximum at $s=5/7$. For this value of $s$, the latter inequality becomes $\frac{4}{7}r_{L}^{2}-6<0$, which clearly holds true for any $r_{L}\in [0,1]$.

1.7 Proof of Proposition 5

As shown in Proposition 3, the condition for type-1 and type-2 equilibria to arise are, respectively, $\xi <-1$ and $\xi >1$. Using (21), (5), (7) and the definition of $\xi$ we obtain, respectively:

$$\begin{aligned} \text {type 1:}&{\qquad }2\alpha _{L}-\frac{8}{3}\alpha _{F}+\frac{2}{3} \theta +\sigma +\frac{5}{6}\beta \bar{Q}<0, \end{aligned}$$

(43)

$$\begin{aligned} \text {type 2:}&{\qquad }-2\alpha _{L}+\frac{8}{3}\alpha _{F}-\frac{2}{3 }\theta -\sigma +\frac{5}{6}\beta \bar{Q}<0. \end{aligned}$$

(44)

As for type-1 equilibria, particularizing (43) in the diagonal, we have $5\kappa \le 4a-6$, which clearly does not hold for any admissible value of $a$. In addition, the most favorable pair of realizations for type-1 equilibrium is $\alpha _{F}=\theta +\sigma$ and $\alpha _{L}=\theta$, under which the condition $\xi <-1$ becomes $\kappa <2$. Thus, there is a positive probability of obtaining type-1 equilibrium if and only if this latter inequality holds and, if it holds, the pair $\left( \alpha _{F}=\theta +\sigma \text {, }\alpha _{L}=\theta \right)$ leads to type-1 equilibrium. This proves part (i), whereas part (ii) follows from the convexity of the half-space defined by (43) whenever it is nonempty.

Notice that any pair $\varvec{\alpha }$ can be written as $\left( \theta +r_{F}\sigma ,\theta +r_{L}\sigma \right)$, with $r_{F}$, $r_{L}\in \left[ 0,1\right]$. Moreover, as $\alpha _{L}\le \alpha _{F}$, we must have $r_{L}\le r_{F}$. Using this notation, (44) becomes

$$\begin{aligned} \left( \frac{8}{3}r_{F}-2r_{L}-1\right) \sigma +\frac{5}{6}\beta \bar{Q}\le 0. \end{aligned}$$

(45)

To identify the feasible value of $\varvec{\alpha }$ that is more favorable for type-2 equilibrium, we minimize the left-hand side of (45) subject to $0\le r_{L}\le r_{F}\le 1$ ²⁹ and obtain $(r_{F},r_{L})=(0,0)$, or, equivalently, $\varvec{\alpha }=(\theta ,\theta )$. Under this value, (45) becomes $\kappa \le \frac{6}{5}$. This proves (iii), whereas part (iv) follows from the convexity of the half-space defined by (44) whenever it is nonempty. To prove part (v) it suffices to note that, under (4), at $\varvec{\alpha }=(\theta +\sigma ,\theta +\sigma )$ we have $\xi =\frac{2\sigma }{5\beta \bar{Q}}$, which belongs to $\left( -1,1\right)$ under (4) and, by continuity, this is true for some non-empty interval around $\left( \theta +\sigma ,\theta +\sigma \right)$.

1.8 Proof of Proposition 6

In case 3, using (5) and (8) we conclude that the condition for type-1 and type-2 equilibria to occur are given by

$$\begin{aligned} \text {type 1:}&{\qquad }2\alpha _{L}-\frac{8}{3}\alpha _{F}+\frac{2}{3} \theta -\frac{1}{3}\sigma +\frac{5}{6}\beta \bar{Q}<0, \end{aligned}$$

(46)

$$\begin{aligned} \text {type 2:}&{\qquad }-2\alpha _{L}+\frac{8}{3}\alpha _{F}-\frac{2}{3 }\theta +\frac{1}{3}\sigma +\frac{5}{6}\beta \bar{Q}<0. \end{aligned}$$

(47)

Consider (46). We use the same notation as in the proof of proposition 5 to write any pair $\varvec{\alpha }$ as $(\theta +r_{L}\sigma ,\theta +r_{F}\sigma )$ with $0\le r_{F}\le r_{L}\le 1$. Using this notation, (46) becomes

$$\begin{aligned} \left( 2r_{L}-\frac{8}{3}r_{F}-\frac{1}{3}\right) \sigma +\frac{5}{6}\beta \bar{Q}<\sigma . \end{aligned}$$

(48)

For (46), the most favorable feasible value is $(r_{F},r_{L})=(1,1)$,³⁰ or, equivalently, $\varvec{\alpha }=(\theta +\sigma ,\theta +\sigma )$. Under this value, (48) becomes $\ \kappa \le \frac{6}{ 5}$. This proves (i), whereas part (ii) follows from the convexity of the half-space defined by (46) whenever it is nonempty. Now consider (47). Using the previous notation, it can be written as

$$\begin{aligned} \left( -2r_{L}+\frac{8}{3}r_{F}+\frac{1}{3}\right) \sigma +\frac{5}{6}\beta \bar{Q}<\sigma . \end{aligned}$$

(49)

Notice that any $\varvec{\alpha }$ in $D$ is characterized by $r_{F}=r_{L}=r$ with $r\in [0,1]$, and (49) fails to hold for any of these values. In addition, the most favorable value of the pair $(r_{F},r_{L})$ for (49) within $0\le r_{F}\le r_{L}\le 1$ is $(r_{F},r_{L})=(0,1)$ or, equivalently, $\varvec{\alpha } =(\theta ,\theta +\sigma )$, under which (49) becomes $\kappa <2$. This proves (iii), whereas part (iv) follows from the convexity of the half-space defined by (47) whenever it is nonempty. To prove part (v) it suffices to note that, at $\varvec{\alpha }=(\theta ,\theta )$, we have $\xi =\frac{2\sigma }{5\beta \bar{Q}}$, which belongs to $\left( -1,1\right)$ under (4) and, by continuity, this is true for some non-empty interval around $\left( \theta ,\theta \right)$.