1 Introduction

There cannot be any general solution of general relativistic (GR) gravitational collapse equations due to complexity of the ten coupled nonlinear partial differential equations, an unknown evolution of the equation of state of the collapsing fluid and the associated complex radiation transport properties. At best, there could be particular solutions depending on various simplifications and assumptions made for particular cases. In fact, in view of such difficulties, there cannot be any general solution of the gravitational collapse problem even in the much simpler Newtonian gravity. This is true even if one assumes spherical symmetry. Given such difficulties, to make a significant headway, it is natural to assume the collapsing fluid to be “dust” having no pressure at all: p=0. Further, if the dust is assumed to be homogeneous, one obtains an exact solution for GR collapse (Oppenheimer and Snyder 1939) and apparently this solution corresponds to formation of a finite-mass black hole (BH). However, the no-pressure assumption is obviously most unrealistic, and therefore we may say that the final state of GR collapse is still unknown.

It is, however, known that when non-geodesic body forces are considered, GR collapse need not be monotonic. For example, even for a non radiative adiabatic collapse, Bondi (1969) found a gravitational bounce due to pressure gradient forces and in general, a pressure gradient indeed opposes the collapse (Mitra 2010). In reality, a gravitational collapse is always nonadiabatic (Mitra 2006a) and effects of dissipation and radiation pressure may lead to formation of radiation pressure supported hot stars (Mitra 2006b; Mitra and Glendenning 2010). In fact, one of us has shown earlier that radiative GR collapse may lead to formation of modestly compact non-singular massive hot objects (Pant et al. 2010; Pant and Tewari 2010). These objects are similar to radiation pressure supported hot super-massive stars, first conceived by Hoyle and Fowler (1963). Once the collapsing object dips below its photon sphere, both matter and radiation quanta tend to move in closed orbits in a fashion (Mitra and Glendenning 2010) somewhat like an Einstein cluster (Einstein 1939), and this would create huge tangential stresses. Such a tangential pressure could be an additional source of support against gravitational contraction (Pinheiro and Chan 2008, 2011). In an interesting study involving the role of tangential pressure (Pinheiro and Chan 2008) mentioned that “As we have shown the black hole is never formed because the apparent horizon formation condition is never satisfied. This could be interpreted as the formation of a naked singularity. However, this is not the case because the star radiates all its mass before it reaches the singularity at r=0 and t=0. Not even a marginally naked singularity is formed for the same reason, since in this case the apparent horizon should coincide with the singularity at r=0 and t=0. The pressure of the star, at the beginning of the collapse, is isotropic, but due to the presence of shear, the pressure becomes more and more anisotropic. The star radiates all its mass during the collapse and this explains why the apparent horizon never forms”. Further most of the astrophysical plasma is endowed with a frozen magnetic field whose strength increases as 1/r 2 during the gravitational contraction. And non-geodesic effects due to the magnetic field could play a very important role in the continued gravitational collapse. In fact, long back, it was claimed that (Ardavan and Partovi 1977). “It is shown that gravitationally collapsed bounded systems which are too massive to be supported by their pressure may be held in equilibrium by self induced magnetic stresses. Some physical implications of the derived solutions—none of which contain a singularity or an event horizon—have also been discussed.”

We may also recall a recent work on this line by Tsagas (2006): “We also show that the relativistic coupling between magnetism and geometry, together with the tension properties of the field, lead to a magneto curvature stress that opposes the collapse. This tension stress grows stronger with increasing curvature distortion, which means that it could potentially dominate over the gravitational pull of the matter. If this happens, a converging family of non-geodesic world lines can be prevented from focusing without violating the standard energy conditions.” For a charged fluid, even if one would ignore magnetic and radiation pressure, the electrostatic repulsion itself may halt collapse (Pinheiro 2011). Thus, after formulation of the Einstein-Maxwell field equations, the relativists have been proposing different models of GR compact charged objects. The presence of a charge as a component of counterbalancing the gravitational force is the subject matter of the present paper. However, here we shall consider only non-singular static solutions which will indicate the formation of such compact objects following GR collapse.

2 Static charged relativistic fluid spheres

It is well known that the presence of some charge may avert the gravitational collapse by counterbalancing the gravitational attraction by electric repulsion in addition to the presence of a pressure gradient (Felice et al. 1995; Ivanov 2002). Felice et al. (1995) proposed a model of a charged perfect fluid and concluded that the inclusion of charge hinders the growth of space-time curvature which has a great role in avoiding a catastrophic collapse. In this context Bonnor (1965) also pointed out that a dust distribution of arbitrarily large mass and small radius can remain in equilibrium against the pull of gravity by a repulsive force produced by a small amount of charge. Thus it is desirable to study the implications of Einstein-Maxwell field equations with reference to a prediction of gravitational collapse. For this purpose, charged fluid ball models are required. The external field of such a ball must be the Reissner–Nordstrom solution.

3 Einstein-Maxwell equation for charged fluid distribution

Let us consider a spherical symmetric metric in curvature coordinates

$$ ds^{2} = - e^{\lambda }dr^{2} - r^{2}\bigl(d\theta^{2} + \sin^{2}\theta d \phi^{2}\bigr) + e^{\nu }dt^{2} $$
(1)

where the functions λ(r) and ν(r) satisfy the Einstein-Maxwell equations

(2)

where ρ, p, ν′, F ij denote energy density, fluid pressure, velocity vector and skew-symmetric electromagnetic field tensor respectively.

In view of the metric (1), the field equation (2) gives

(3)
(4)
(5)

where prime (′) denotes the differentiation with respect to r and q(r) represents the total charge contained within the sphere of radius r.

4 Conditions for well-behaved solutions

For a well-behaved nature of the solution in curvature coordinates, the following conditions should be satisfied, in augmentation of the Delgaty and Lake (1998) and Pant (2010) conditions:

  1. (i)

    The solution should be free from physical and geometric singularities, i.e., should yield finite and positive values of the central pressure, central density and nonzero positive values of e λ and e ν.

  2. (ii)

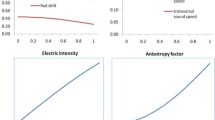
    The solution should have positive and monotonically decreasing expressions for pressure and density (p and ρ) as r increases. There should be a positive value pressure-density ratio, smaller than 1 (the weak energy condition) and smaller than 1/3 (the strong energy condition) throughout the star, which should be monotonically decreasing as well.

  3. (iii)

    The casualty condition (dp/dρ)1/2<1 i.e., the velocity of sound should be less then that of light throughout the model. In addition, the velocity of sound should decrease towards the surface, i.e., (d/dr)(dp/dρ)<0, or d 2 p/dρ 2>0 for 0≤r≤r b , i.e., the velocity of sound increases with increasing density. In this context it is worth mentioning that the equation of state in an ultra-high distribution (Pant et al. 2011) has the property that the speed of sound decreases outwards.

  4. (iv)

    p/ρ≤dp/dρ everywhere within the ball as

    $$\gamma = \frac{d\ln p}{d\ln \rho} = \frac{\rho dp}{pd\rho} \Rightarrow\frac{dp}{d\rho} = \gamma\frac{p}{\rho} $$

    and for realistic matter γ≥1.

  5. (v)

    The red shift z should be positive, finite and monotonically decreasing with increasing r.

  6. (vi)

    The electric intensity E is positive and monotonically increases from the center to the boundary, and at the center the electric intensity is zero. Under these conditions, one has to assume the gravitational potential and electric field intensity in such a way that the field equation can be integrated and the solution should be well-behaved. Keeping that in mind, several authors have obtained a parametric class of exact solutions: Pant et al. (2011), Maurya and Gupta (2011) and Pant (2011). These solutions are well-behaved with some positive values of the charge parameter K and completely describe the interior of a super dense astrophysical object with charged matter. Further, its mass can be maximized by assuming the surface density ρ b =2×1014 g/cm3.

5 New class of solution

Now let us assume

$$ e^{v} = B\bigl(1 + c{}_{1}r^{2} \bigr)^{4} $$
(6)

which is the same as that of the metric obtained by Durgapal (1982).

Putting (6) into (3)–(5), we have

(7)
(8)

and Y satisfying the equation

$$ \frac{dY}{dx} + \frac{7x^{2} - 2x - 1}{x(x + 1)(5x + 1)}Y = \frac{x + 1}{x(5x + 1)} \biggl( \frac{2c_{1}q^{2}}{x} - 1 \biggr) $$
(9)

where x=c 1 r 2, e −λ=Y.

In order to solve the differential equation (9) we consider the electric intensity E of the following form:

$$ \frac{E^{2}}{c_{1}} = \frac{c_{1}q^{2}}{x^{2}} = \frac{K}{2}x(x + 1) (5x + 1)^{3/5} $$
(10)

where K is a positive constant. The electric intensity is so assumed that the model is physically significant and well behaved, i.e. E remains regular and positive throughout the sphere. In addition, E vanishes at the centre of the star. Thus we have

$$ K \ge 0,\qquad c_{1} \ge 0 $$
(11)

In view of (10), the differential equation (9) yields the following solution

(12)

where A is an arbitrary constant of integration.

Substituting (6), (9) and (12) into (7) and (8) we get the following expressions for pressure and energy density

(13)
(14)

6 Properties of the new class of solution

(15)
(16)

For p 0 and ρ 0 must be positive and \(\frac{p_{0}}{\rho_{0}} \le 1\), we have

(17)

Differentiating (13) and (14) w.r.t. to x, we get

(18)
(19)

Dividing the above two equations we get,

(20)

where

(21)
(22)
$$\biggl( \frac{1}{c^{2}}\frac{d\rho}{d\rho} \biggr)_{x = 0} = \frac{ - 23k - 280A + 2080}{5(21k - 280A + 400)} \le 1 $$

for all values of k and A satisfied by (17).

The expression for gravitational red shift (z) is given by

$$ z = \frac{(1 + x)^{ - 2}}{\sqrt{B}} - 1 $$
(23)

The central value of gravitational red shift to be non zero positive finite, we have

$$0 < \sqrt{B} < 1 $$

Differentiating (23) w.r.t. x, we get

$$ \biggl( \frac{dz}{dx} \biggr)_{x = 0} = \frac{ - 2}{\sqrt{B}} < 0 $$
(24)

The expression of the right side of (24) is negative, thus the gravitational red shift is maximum at the centre and monotonically decreasing.

Differentiating equation (10) w.r.t. x, we get

(25)
(26)

The expression of right hand side of (26) is positive, the electric intensity is minimum at the centre and monotonically increasing for all values of k>0. Also at the centre it is zero.

7 Boundary conditions

The solutions so obtained are to be matched over the boundary with the Reissner-Nordstrom metric:

(27)

which requires the continuity of eλ, eυ and q across the boundary r=r b .

(28)
(29)
(30)
(31)

The condition (31) can be utilized to compute the value of the arbitrary constant A as follows.

On setting \(x_{r = r_{b}} = X = c_{1}r_{b}^{2}\) (r b being the radius of the charged sphere), pressure at p(r=r b )=0 gives

(32)
$$ \frac{GM}{c^{2}} = \frac{r_{b}}{2}.\frac{X[KX(X + 1)(5X + 1)^{8/5} +8]}{(9X + 1)} $$
(33)

In view of (6), (28) and (29) we also get

$$ B = \frac{ - KX^{2}(X + 1)(5X + 1)^{3/5} + 2}{2(X + 1)^{3}(9X + 1)} $$
(34)

The surface density is given by

(35)

where b 3=207X 3+172X 2+51X+6. Here,

$$ \frac{e^{2}}{r_{b}^{2}} = \frac{KX^{2}(X + 1)(5X + 1)^{3/5}}{2} $$
(36)

The central red shift z x=0 is given by

$$ z_{x = 0} =\frac{1}{\sqrt{B}} - 1 $$
(37)

and the red shift at the surface is given by

$$ z_{x = X} = \frac{(1 + X)^{ - 2}}{\sqrt{B}} - 1 $$
(38)

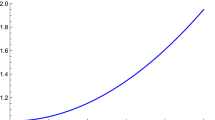
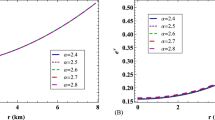
The graphs of K vice-versa r b and K vice-versa mass of star are given in Figs. 1 and 2.

Fig. 1
figure 1

K vice-versa r b

Full size image
Fig. 2
figure 2

K vice-versa mass

Full size image

In view of Tables 1, 2 and 3, it has been observed that all the physical parameters (\(p,\rho,\frac{p}{\rho c^{2}},\frac{dp}{d\rho}\) and E) are positive at the centre and within the limit of realistic equation of state (EOS) and well behaved conditions and \(\frac{p}{\rho} < \frac{dp}{d\rho}\) everywhere within the star, therefore, adiabatic constant is always greater than 1. For all values of K satisfying the inequalities 0≤K≤2.2 with X=0.235. However, corresponding to any values of K>2.2 be nature of adiabatic sound speed is imaginary in the vicinity of the boundary. We now present here a model of super dense star based on the particular solution discussed above by assuming surface density; ρ b =2×1014 g/cm3. Corresponding to K=0 with X=0.235, the resulting well behaved model has the mass M=4.04M Θ with radius 19.53 km. It has been observed that under well behaved conditions, this solution gives us the mass and radius of super dense object within the range of neutron star. Corresponding to K=1.5 with X=0.235, the resulting well behaved model has the mass M=4.43M Θ with radius 18.04 km and corresponding to K=2.2 with X=0.235, the resulting well behaved model has the mass M=4.56M Θ with radius 17.30 km. It has been observed that under well behaved conditions, this solution gives us the mass and radius of super dense object within the range of neutron star. From the graphs (Figs. 1 and 2), it has been observed that with the increase of charge, the mass of the star increases while radius of the star decreases.

Table 1 The march of pressure, density, pressure–density ratio, square of adiabatic sound speed and electric field intensity within the ball corresponding to K=0 with X=0.235
Full size table
Table 2 The march of pressure, density, pressure–density ratio, square of adiabatic sound speed and electric field intensity within the ball corresponding to K=1.5 with X=0.235
Full size table
Table 3 The march of pressure, density, pressure–density ratio, square of adiabatic sound speed and electric field intensity within the ball corresponding to K=2.2 with X=0.235
Full size table

8 Slowly rotating structures and their application to the crab pulsar

For slowly rotating structures like the crab (a rotation velocity about 188 rad/s) and vela pulsars (about 70 rad/s), one can calculate the moment of inertia in the first order approximation which appears in the Lense Thirring frame-dragging effect. However, in the present case of an exact solution, it is useful to apply an approximate, but very precise, empirical formula based on numerical results obtained for a large number of theoretical equations of state (EOSs) of dense nuclear matter. For the type of solution considered in the present study, the formula yields in the following form (Bejger and Haensel 2002; Pant and Faruqi 2012).

$$ I = (2/5) (1 + y)MR^{2} $$
(39)

where y is defined as

$$ y = (M/R)/M_{\varTheta} $$
(40)

With the help of (39), we can calculate the moment of inertia, for various super dense objects as shown in Table 4. These values of masses and moment of inertia agree quite well with those of the masses and the moment of inertia calculated for the crab pulsars.

Table 4 By assuming the surface density ρ b =2×1014 g/cm3, the variation of maximum neutron star mass, radius r b , central red shift z x=0, surface red shift z x=X and moment of inertia with different values of K at X=0.235
Full size table

9 Conclusion

After the depletion of nuclear fuel the cores of massive stars must start contracting, the pressure and internal energy do persist, and because of negative specific heat associated with bulk gravity, a star may actually become hotter during collapse. In general, there are always various agents like pressure gradient, heat flow, radiation pressure, tangential pressure and magnetic stresses which tend to resist the continued gravitation collapse. Further, in a charged fluid, the electrostatic repulsion alone may resist the collapse. Accordingly, here we studied a model of a charged massive relativistic star which is not only non singular but is endowed with all physically appealing features. By setting the charge to zero, we applied it to uncharged and discreetly relativistic compact objects. A good matching of our results for crab pulsars shows the sturdiness of our model. Moreover, convincingly we can say that the more massive star can have stability due to presence of charge as obvious from the graphs.