1 Introduction

In contrast to the classical theory of elasticity, micropolar theory considers additional rotational degrees of freedom of the material particles, which are considered as small rigid bodies. Interactions between adjoined particles occur in terms of the classical force stress tensor and the micropolar stress tensor which originate due to the rotation of particles. As a result, the micropolar theory of elasticity considers the length-scale effect, which is thought to be microstructure-dependent. Considering the microstructure of a material is very important in modeling devices and structures made of heterogeneous material especially at micro- and nano-scale, for example MEMS and NEMS, see Altenbach and Eremeyev [1], McFarland and Colton [51], Waseem et al. [63] and Yoder et al. [66] and in biomechanics for bone modeling, see Eremeyev et al. [28, 29] and Fatemi et al. [33]. Since the publication of the Cosserat brother’s landmark book [22], there is a lot more books and papers dedicated to various aspects of the theory of micropolar continua and its applications. Among many other, the noteworthy books of Eringen [32] and Nowacki [52] as well as the recently published book of Eremeyev et al. [27] are mentioned here. For more information between others, one can also refer to our previous publications Carrera and Zozulya [18, 19].

Many structures in airspace, civil and mechanical engineering, micro-electronics, etc., can be considered as beams, plates and shells (see for example Reddy [55] for references). Therefore, the development of the plate theories based on the micropolar theory of elasticity is relevant and important task for the engineering community. The first micropolar models of rods, plates and shells were developed by the Cosserat brothers in [22], but it was only after the publication of the seminal paper by Ericksen and Truesdell [30] the generalized micropolar models of rods, plates and shells are further developed and extensively discussed in the literature. A review and critical analysis related to the micropolar theories of rods, plates and shells can be found in Altenbach et al. [2], Carrera and Zozulya [18, 19], Kovvali and Hodges [43]. We would like also to mention some works related to micropolar plates, which were useful for this paper’s preparation: Altenbach and Eremeyev [2], Altenbach et al. [3] Ambartsumian [4, 5], Ansari et al. [7, 8], Eringen [31], Jemielita [41], Karttunen et al. [42], Kvasov and Steinberg [45], Sargsyan [58], Shaw [59], Steinberg [60], Steinberg and Kvasov [61]. Also, in our previous papers Carrera and Zozulya [18, 19] one can find more details of the higher order theory of micropolar beams and plates based on the CUF.

The CUF approach for the development of the higher order theories of beams, rods, plates and shells has been presented in detail for the first time in Carrera [12] and then was further developed and published in numerous publications. For more information, one can refer to the review papers Carrera [13, 14] and the book Carrera et al. [15]. Navier’s closed-form solution for the case of simply supported plates and shells based on CUF has been developed in Carrera et al. [16, 17]. Roughly speaking, the CUF consists in the expansion of the field equations in the series of functions of the coordinates of the cross section. In the case of plates and shells, usually it is expansion of the 3D equations of elasticity in the series of functions of the thickness coordinate. In general, these functions can be arbitrary, but final equation becomes simpler if they are polynomials, especially orthogonal polynomials. The Legendre polynomials have been widely used for the higher order theories of beams, rods, plates and shells, among others see Vekua [62] and Zozulya [67]. For the development of the higher order micropolar theory of beams, rods, plates and shells, the Legendre polynomials have been used in Zozulya [68,69,70,71,72].

Constitutive equations of micropolar elasticity contain six coefficients that define the mechanical properties of material. In order to theory be effective and applicable in practice, one have to know these coefficients. There are at least two approaches to their determination: theoretical (from first principles, via homogenization, numerical calculation, etc.) and experimental (testing of specimens of real materials in laboratories).

Theoretical approach to micropolar elastic moduli definition can be generally divided into homogenization and numerical calculation based on discrete molecular models. In Bigoni and Drugan [11], closed-form formulae for Cosserat moduli via homogenization of a dilute suspension of elastic spherical inclusions embedded in an isotropic elastic matrix is derived for the case that the inclusions are less stiff than the matrix. Dos Reis and Ganghoffer [24, 25] applied asymptotic homogenization of periodic beam and articulated bars lattices for the homogenized micropolar moduli definition. The homogenized behavior of the tetragonal and hexagonal lattices is determined in terms of homogenized micropolar moduli. Goda et al. [37,38,39] applied discrete asymptotic homogenization for definition of micropolar moduli for textile and bone. In Diebels [26], a theory of porous media is applied to micropolar mixture models. Askar [9] suggests calculating the micropolar material moduli using the theory of molecular crystals dynamics. This work could be considered as the first successful attempt for determining some of the micropolar material coefficients from the first principles. Askar and Cakmak [10] proposed a 2D model composed of orientable points, joined by extensible and flexible rods to explain the foundations of the micropolar continuum and determinate the micropolar elastic moduli of the equivalent micropolar continuum. Chiroiu and Munteanu [20] used a nonlinear wave theory to construct an inverse approach to estimate the micropolar elastic moduli of a micropolar plate. Based on the interrelations between natural frequencies and the elastic properties of the material they show that it is possible to reconstruct in a unique manner the unknown micropolar elastic moduli. In Chung and Waas [21], expressions for the characterization of circular cell honeycombs as micropolar elastic solids using a combination of non-dimensional analysis and numerical analysis are obtained. Closed-form expressions for the four in-plane micropolar compliances are derived in terms of the cell size, cell thickness and the linear elastic properties of the cell’s wall material. In Kumar [44], linear elastic micropolar constants are obtained using an energy approach for square, equilateral triangular, mixed triangle and diamond cell topologies. In Liebenstein and Zaiser [50], a two-scale model of disordered cellular materials using a beam network model is presented and material parameters for regular honeycomb structures is described. A review of the lattice model application in micromechanics of materials has been done in Ostoja-Starzewski [53].

As mentioned in Hassanpour and Heppler [40], Gauthier and Jahsman [34,35,36] were the first who tried to determinate all six micropolar moduli. Based on theoretical consideration, they tested a circular cylinder and rectangular plate made of composite of epoxy matrix and distributed rigid aluminum shot applying torsional and pure bending deformation, respectively. They performed both static and dynamic tests to determine all six micropolar elastic moduli of the material. The static test was not successful Gauthier and Jahsman citer35, whereas the dynamic test was quite successful and as a result they determined four (out of six) micropolar elastic moduli Gauthier and Jahsman [36]. See also Gauthier [34]. We would like to mention that based on the results from Gauthier and Jahsman [36] all six micropolar elastic moduli is reported in Shaw [59]. The experimental approach suggested by Gauthier and Jahsman was further developed and extensively used by Lakes and coauthors to determine the micropolar elastic moduli of different materials such as bone Lakes [47], Park and Lakes [54] and Yang and Lakes [65], polymeric foams Lakes [46, 47] and Rueger and Lakes [56, 57], cell polymethacrylimide foams of three different grades (i.e., WF51, WF110, WF300) Anderson and Lakes [6]. A review of experimental research and more experimental data can be found in Hassanpour and Heppler [40] and Lakes [48, 49].

In this paper, based on the 2D higher order models of micropolar elastic plates developed using a principle of virtual displacements Navier’s closed-form solutions for the case of the simply supported plate based on CUF is presented. Numerical calculations of the displacements and rotation as well as classical force and micropolar couple stress tensors have been done, and the results of calculation are presented in the form of tables and graphs. The obtained results can be useful for modeling of thin-walled structures in macro-, micro- and nano-scales by taking into account micropolar couple stress and rotation effects. The proposed models can also be efficient in MEMS and NEMS modeling as well as in computer simulation.

2 Mathematical formulation of the boundary-value problem

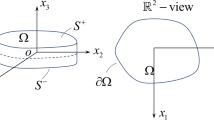
Here, we present a closed-form solution for higher order elastic micropolar plates, which are developed in Carrera and Zozulya [19] based on CUF. We first introduce here basic notations and equations which are presented in detail in Carrera and Zozulya [19]. Let us consider an elastic body in a 3D Euclidian space, which occupies the domain\(V=\Omega \times [r-h,h]\), where \(\Omega \) is the middle plane of the plate, 2h is the thickness of the plate. The adopted system of coordinates is Cartesian, where axises xandy coincide with the middle plane of the plate and z is directed orthogonally to the middle plane.

In micropolar theory, it is assumed that the body consists of interconnected particles in the form of small rigid bodies. In this case, each particle possesses six degrees of freedom and the position of the particle during deformation is determined by the displacements \({\mathbf{u}}(x,y,z)\)and rotation \({\varvec{\upomega }}(x,y,z)\) vectors as functions of their coordinates. The internal forces (the interaction between adjacent elements) in a micropolar continuum are defined in terms of classical force stress tensor \({\varvec{\upsigma }}\varvec{(x,y,z)}\) and a micropolar couple stress tensor \({\varvec{\upmu }}\varvec{(x,y,z)}\). The micropolar deformations are fully described by the asymmetric strain \({\varvec{\upvarepsilon }}\varvec{(x,y,z)}\) and torsion \({\varvec{\upchi }}\varvec{(x,y,z)}\) tensors.

In the same way as in Carrera and Zozulya [18, 19], here we introduce vector notations and represent the above functions that define the stress–strain state of the micropolar media in vector form. The classical force stress and strain as well as micropolar couple stress and twist tensors are presented as nine component vectors, as it is presented bellow

$$\begin{aligned} {\varvec{\upsigma }}= & {} \left| {\sigma _{xx} ,\sigma _{yy} ,\sigma _{zz} ,\sigma _{xy} ,\sigma _{yx} ,\sigma _{xz} ,\sigma _{zx} ,\sigma _{yz} ,\sigma _{zy} } \right| ^{T},\nonumber \\ \quad {\varvec{\upvarepsilon }}= & {} \left| {\varepsilon _{xx} ,\varepsilon _{yy} ,\varepsilon _{zz} ,\varepsilon _{xy} ,\varepsilon _{yx} ,\varepsilon _{xz} ,\varepsilon _{zx} ,\varepsilon _{yz} ,\varepsilon _{zy} } \right| ^{T}, \nonumber \\ {\varvec{\upmu }}= & {} \left| {\mu _{xx} ,\mu _{yy} ,\mu _{zz} ,\mu _{xy} ,\mu _{yx} ,\mu _{xz} ,\mu _{zx} ,\mu _{yz} ,\mu _{zy} } \right| ^{T},\nonumber \\ \quad {\varvec{\upchi }}= & {} \left| {\chi _{xx} ,\chi _{yy} ,\chi _{zz} ,\chi _{xy} ,\chi _{yx} ,\chi _{xz} ,\chi _{zx} ,\chi _{yz} ,\chi _{zy} } \right| ^{T}, \end{aligned}$$
(2.1)

The displacements and rotations are presented as three component vectors in the following form

$$\begin{aligned} \begin{array}{l} {\mathbf{u}}(x,y,z)=\left| {u_{x} (x,y,z),u_{y} (x,y,z),u_{z} (x,y,z)} \right| ^{T}, \\ {\varvec{\upomega }}(x,y,z)=\left| {\omega _{x} (x,y,z),\omega _{y} (x,y,z),\omega _{z} (x,y,z)} \right| ^{T} \end{array} \end{aligned}$$
(2.2)

In the equations, superscript T means transpose vector.

Kinematic relations in micropolar elasticity connect vectors of displacements and rotation with the strain and torsion vectors (2.2) by the following equations

$$\begin{aligned} {\varvec{\upvarepsilon }}={\mathbf{D}}\cdot {\mathbf{u}}+{\mathbf{D}}^{c}\cdot {\varvec{\upomega }},\quad {\varvec{\upchi }}={\mathbf{D}}\cdot {\varvec{\upomega }} \end{aligned}$$
(2.3)

where \({\mathbf{D}}\) and \({\mathbf{D}}^{c}\) are matrix operators of the form

$$\begin{aligned} {\mathbf{D}}^{T}=\left| {{ \begin{array}{*{20}c} {\frac{\partial }{\partial x}} &{} 0 &{} 0 &{} 0 &{} {\frac{\partial }{\partial y}} &{} 0 &{} {\frac{\partial }{\partial z}} &{} 0 &{} 0 \\ 0 &{} {\frac{\partial }{\partial y}} &{} 0 &{} {\frac{\partial }{\partial x}} &{} 0 &{} 0 &{} 0 &{} 0 &{} {\frac{\partial }{\partial z}} \\ 0 &{} 0 &{} {\frac{\partial }{\partial z}} &{} 0 &{} 0 &{} {\frac{\partial }{\partial x}} &{} 0 &{} {\frac{\partial }{\partial y}} &{} 0 \\ \end{array} }} \right| ,\quad {\mathbf{D}}^{c,T}=\left| {{\begin{array}{*{20}c} 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} 1 &{} {-1} \\ 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} {-1} &{} 1 &{} 0 &{} 0 \\ 0 &{} 0 &{} 0 &{} 1 &{} {-1} &{} 0 &{} 0 &{} 0 &{} 0 \\ \end{array} }} \right| , \end{aligned}$$
(2.4)

Constitutive relations are presented here by introducing the function of density of a potential energy. In the case of linear orthotropic micropolar media, it can be presented in the following general form

$$\begin{aligned} 2W({\varvec{\upvarepsilon }},{\varvec{\upchi }})={\varvec{\upvarepsilon }}^{T}\cdot {\mathbf{C}}\cdot {\varvec{\upvarepsilon }}+{\varvec{\upchi }}^{T}\cdot {\mathbf{A}}\cdot {\varvec{\upchi }} \end{aligned}$$
(2.5)

where classical and micropolar moduli of elasticity for linear orthotropic micropolar media are presented as \(9\times 9\) matrices of the form

$$\begin{aligned} {\mathbf{C}}= & {} \left| {{\begin{array}{lllllllll} {C_{11} } &{} {C_{12} } &{} {C_{13} } &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 \\ {C_{12} } &{} {C_{22} } &{} {C_{23} } &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 \\ {C_{13} } &{} {C_{23} } &{} {C_{33} } &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 \\ 0 &{} 0 &{} 0 &{} {C_{44} } &{} {C_{44}^{T} } &{} 0 &{} 0 &{} 0 &{} 0 \\ 0 &{} 0 &{} 0 &{} {C_{44}^{T} } &{} {C_{44} } &{} 0 &{} 0 &{} 0 &{} 0 \\ 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} {C_{55} } &{} {C_{55}^{T} } &{} 0 &{} 0\\ 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} {C_{55}^{T} } &{} {C_{55} } &{} 0 &{} 0 \\ 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} {C_{66} } &{} {C_{66}^{T} }\\ 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} {C_{66}^{T} } &{} {C_{66} } \\ \end{array} }} \right| ,\nonumber \\ {\mathbf{A}}= & {} \left| {{\begin{array}{lllllllll} {A_{11} } &{} {A_{12} } &{} {A_{13} } &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 \\ {A_{12} } &{} {A_{22} } &{} {A_{23} } &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 \\ {A_{13} } &{} {A_{23} } &{} {A_{33} } &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 \\ 0 &{} 0 &{} 0 &{} {A_{44} } &{} {A_{44}^{T} } &{} 0 &{} 0 &{} 0 &{} 0 \\ 0 &{} 0 &{} 0 &{} {A_{44}^{T} } &{} {A_{44} } &{} 0 &{} 0 &{} 0 &{} 0 \\ 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} {A_{55} } &{} {A_{55}^{T} } &{} 0 &{} 0 \\ 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} {A_{55}^{T} } &{} {A_{55} } &{} 0 &{} 0 \\ 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} {A_{66} } &{} {A_{66}^{T} } \\ 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} {A_{66}^{T} } &{} {A_{66} } \\ \end{array} }} \right| \end{aligned}$$
(2.6)

where \(C_{ij} ,\;i,j=1,2,3\) are the classical and \(C_{ii} ,\;C_{ii}^{T} ,\;i=4,5,6\) are the micropolar and also \(A_{ij}\,,\, i,j=1,2,3\) and \(A_{ii} ,\;A_{ii}^{T} ,\;i=4,5,6\) are the micropolar moduli of elasticity

In the case of isotropic materials, there are many notations for the micropolar elastic modulus (for references see Hassanpour and Heppler [40], Jemielita [41], etc.). Here, we use the constitutive relations for the micropolar linear elasticity introduced by Nowacki [52]. The corresponding classical and micropolar moduli of elasticity in this case have the form

$$\begin{aligned} C_{11}= & {} C_{22} =C_{33} =\lambda +2\mu ,\quad C_{12} =C_{13} =C_{23} =\lambda ,\nonumber \\ \quad C_{44}= & {} C_{55} =C_{66} =\mu +\alpha ,\quad C_{44}^{T} =C_{55}^{T} =C_{66}^{T} =\mu -\alpha , \nonumber \\ A_{11}= & {} A_{22} =A_{33} =\beta +2\gamma \quad A_{12} =A_{13} =A_{23} =\beta ,\nonumber \\ A_{44}= & {} A_{55} =A_{66} =\gamma +\varepsilon ,\quad A_{44}^{T} =A_{55}^{T} =A_{66}^{T} =\gamma -\varepsilon \end{aligned}$$
(2.7)

where \(\lambda \) and\(\mu \)are Lamé constants of classical elasticity, \(\alpha \), \(\beta \), \(\gamma \) and \(\varepsilon \)are additional micropolar elastic constants.

When taking derivative of the function of density of a potential energy (2.5) with respect to strain \({\varvec{\upvarepsilon }}\) and torsion \({\varvec{\upchi }}\) tensors and substituting in the obtained result the kinematic relations (2.3), the classical stress and micropolar couple stress vectors can be presented in one of the following forms

$$\begin{aligned} {\varvec{\upsigma }}=\frac{\partial W}{\partial {\varvec{\upvarepsilon }}}={\mathbf{C}}\cdot {\varvec{\upvarepsilon }}={\mathbf{C}}\cdot ({\mathbf{D}}\cdot {\mathbf{u}}+{\mathbf{D}}^{c}\cdot {\varvec{\upomega }}),\quad {\varvec{\upmu }}=\frac{\partial W}{\partial {\varvec{\upchi }}}={\mathbf{A}}\cdot {\varvec{\upchi }}={\mathbf{A}}\cdot {\mathbf{D}}_{u} \cdot {\varvec{\upomega }} \end{aligned}$$
(2.8)

Following the CUF, the displacements and the rotation field that are functions of the three Cartesian coordinates (x, y, z) are represented as series of functions of the coordinate z directed orthogonally to the middle plane in the form

$$\begin{aligned} {\mathbf{u}}(x,y,z)={\mathbf{F}}_{u,\tau } (z)\cdot {\mathbf{u}}_{\tau } (x,y),\quad {\varvec{\upomega }}(x,y,z)={\mathbf{F}}_{\omega ,\tau } (z)\cdot {\varvec{\upomega }}_{\tau } (x,y),\quad \tau =1,2,\;\ldots \;,M \end{aligned}$$
(2.9)

Where the basic functions of the thickness coordinates

$$\begin{aligned} \begin{array}{l} {\mathbf{F}}_{{\mathbf{u}},\tau } (z)=\left| {{\begin{array}{*{20}c} {F_{u_{x} ,\tau } (z)} &{} 0 &{} 0 \\ 0 &{} {F_{u_{y} ,\tau } (z)} &{} 0 \\ 0 &{} 0 &{} {F_{u_{z} ,\tau } (z)} \\ \end{array} }} \right| ,\quad {\mathbf{u}}_{\tau } (x,y)=\left| {{\begin{array}{*{20}c} {u_{x,\tau } (x,y)} \\ {u_{y,\tau } (x,y)} \\ {u_{z,\tau } (x,y)} \\ \end{array} }} \right| , \\ {\mathbf{F}}_{{\varvec{\upomega }},\tau } (z)=\left| {{\begin{array}{*{20}c} {F_{\omega _{x} ,\tau } (z)} &{} 0 &{} 0 \\ 0 &{} {F_{\omega _{y} ,\tau } (z)} &{} 0 \\ 0 &{} 0 &{} {F_{\omega _{z} ,\tau } (z)} \\ \end{array} }} \right| ,\quad {\varvec{\upomega }}_{\tau } (x,y)=\left| {{\begin{array}{*{20}c} {\omega _{x,\tau } (x,y)} \\ {\omega _{y,\tau } (x,y)} \\ {\omega _{z,\tau } (x,y)} \\ \end{array} }} \right| \\ \end{array} \end{aligned}$$
(2.10)

In (2.9) according to the Einstein notation, the repeated subscript \(\tau \) indicates summation. The first subscript in the base functions indicates a component of the displacements or rotations vectors, and the second one indicates the number of series component. In general, the choice of the number M and functions \({\mathbf{F}}_{{\mathbf{u}},\tau } (z)\)and \({\mathbf{F}}_{{\varvec{\upomega }},\tau } (z)\)is arbitrary, that is, different base functions of any order can be taken into account to model the kinematic field of the plate along its thickness. Usually number M corresponds to the order of the plate theory. The final equation becomes simpler if functions \({\mathbf{F}}_{{\mathbf{u}},\tau } \)and \({\mathbf{F}}_{{\varvec{\upomega }},\tau } \) are polynomials, especially orthogonal polynomials. Coefficients of the expansions \({\mathbf{u}}_{\tau } (x,y)\) and \({\varvec{\upomega }}_{\tau } (x,y)\) are functions of the coordinates x and y, which coincided with the middle plane of the plate. The first subscript in the base functions \({\mathbf{F}}_{{\mathbf{u}},\tau } \)and \({\mathbf{F}}_{{\varvec{\upomega }},\tau } \)indicates the component of the displacements or rotations vectors, the second one indicates the serial number of the function in the expansion.

Applying matrix differential operators (2.4) to the displacements and rotation presented by Eq. (2.9), one can obtain the strain and torsion vectors in the form

$$\begin{aligned} {\varvec{\upvarepsilon }}={\mathbf{D}}_{{\mathbf{u}},\tau } \cdot {\mathbf{u}}_{\tau } +{\mathbf{D}}_{\omega ,\tau }^{T} \cdot {\varvec{\upomega }}_{\tau } ,\quad {\varvec{\upchi }}={\mathbf{D}}_{{\varvec{\upomega }},\tau } \cdot {\varvec{\upomega }}_{\tau } \end{aligned}$$
(2.11)

where \({\mathbf{D}}_{{\mathbf{u}},\tau } \), \({\mathbf{D}}_{{\varvec{\upomega }},\tau } \)and \({\mathbf{D}}_{\omega ,\tau }^{T} \) are matrix operators obtained by substituting expansion (2.9) to the matrix operators (2.4). Their explicit form is presented in the Carrera and Zozulya [19].

By substituting kinematic relations (2.11) into generalized Hooke’s law (2.8), the classical force stress and the micropolar couple stress vectors can now be presented in the form

$$\begin{aligned} {\varvec{\upsigma }}={\mathbf{C}}\cdot \left( {{\mathbf{D}}_{u,\tau } \cdot {\mathbf{u}}_{\tau } +{\mathbf{D}}_{\tau }^{\omega } \cdot {\varvec{\upomega }}_{\tau } } \right) ,\quad {\varvec{\upmu }}={\mathbf{A}}\cdot {\mathbf{D}}_{\omega ,\tau } \cdot {\varvec{\upomega }}_{\tau } \end{aligned}$$
(2.12)

Originally the CUF approach for the development of the higher order theories of plates and shells was based on the principle of virtual displacements (PVD), (see Washizu [64]), which in the general case of 3D linear micropolar elasticity can be presented in the form

$$\begin{aligned} \delta \int \limits _V {W({\varvec{\upvarepsilon }},{\varvec{\upchi }})\hbox {d}V} =\delta L_{ext} ({\mathbf{b}}_{u} ,{\mathbf{b}}_{\omega } ,{\mathbf{p}}_{u} ,{\mathbf{p}}_{\omega } ) \end{aligned}$$
(2.13)

Here, \(W({\varvec{\upvarepsilon }},{\varvec{\upchi }})\)is a density of potential energy of the micropolar body, \(L_{ext} ({\mathbf{b}}_{u} ,{\mathbf{b}}_{\omega } ,{\mathbf{p}}_{u} ,{\mathbf{p}}_{\omega } )\)is a work of the external volume \({\mathbf{b}}_{u} ,{\mathbf{b}}_{\omega } ,\) and \({\mathbf{p}}_{u} ,{\mathbf{p}}_{\omega } \) surface load.

By substituting the expressions for the strain and torsion vectors presented by Eq. (2.11), the classical force stress and the micropolar couple stress vectors presented by Eqs. (2.12) into (2.13) we will get a variation of the density of potential energy in the form

$$\begin{aligned} \delta W= & {} ({\mathbf{D}}_{u,\tau }^{T} \cdot {\mathbf{F}}_{u,\tau } (z)\cdot \delta {\mathbf{u}}_{\tau } (x,y)\nonumber \\&+\,{\mathbf{D}}_{\tau }^{\omega ,T} \cdot {\mathbf{F}}_{\omega ,\tau } (z)\cdot \delta {\varvec{\upomega }}_{\tau } (x,y))\cdot {\mathbf{C}}\cdot ({\mathbf{D}}_{u,s} \cdot {\mathbf{F}}_{u,s} (z)\cdot {\mathbf{u}}_{s} (x,y)+ \nonumber \\&+\,{\mathbf{D}}_{s}^{\omega } \cdot {\mathbf{F}}_{\omega .s} (z)\cdot {\varvec{\upomega }}_{s} (x,y))+({\mathbf{D}}_{u,\tau }^{T} \cdot {\mathbf{F}}_{\omega ,\tau } (z)\cdot \delta {\varvec{\upomega }}_{\tau } (x,y))\cdot {\mathbf{A}}\cdot {\mathbf{D}}_{u,s} \cdot {\mathbf{F}}_{\omega ,s} (z)\cdot {\varvec{\upomega }}_{s} (x,y) \end{aligned}$$
(2.14)

The volume forces and momentum loads are the function of the Cartesian coordinates \({\mathbf{b}}_{u} (x,y,z)\) and \({\mathbf{b}}_{\omega } (x,y,z)\). They also can be represented as a series of functions of the thickness coordinate z in the form

$$\begin{aligned} {\mathbf{b}}_{u} (x,y,z)={\mathbf{F}}_{u,\tau } (z)\cdot {\mathbf{b}}_{u,\tau } (x,y),\quad {\mathbf{b}}_{\omega } (x,y,z)={\mathbf{F}}_{\omega ,\tau } (z)\cdot {\mathbf{b}}_{\omega ,\tau } (x,y),\quad \tau =1,2,\;\ldots \;,M \end{aligned}$$
(2.15)

The surface load is the function of only the plate middle plane coordinatesx and y, and the thickness coordinate z has specific values that correspond to points on the surfaces \(z=-h\) and \(z=h\).

Let us consider a variation of the work of the external volume and surface load in the case of micropolar media. Taking into account (2.14) and (2.15), the variation of the work of the external volume and surface load in the case of micropolar media has the form

$$\begin{aligned}&\delta L_{ext} ({\mathbf{b}}_{u} ,{\mathbf{b}}_{\omega } ,{\mathbf{p}}_{u} ,{\mathbf{p}}_{\omega } )\nonumber \\&\quad =\int \limits _V {({\mathbf{F}}_{u,\tau } (z)\cdot \delta {\mathbf{u}}_{\tau } (x,y)\cdot {\mathbf{F}}_{u,s} (z)\cdot {\mathbf{b}}_{u,s} (x,y)+{\mathbf{F}}_{\omega ,\tau } (z)\cdot \delta {\varvec{\upomega }}_{\tau } (x,y)\cdot {\mathbf{F}}_{\omega ,s} (z)\cdot {\mathbf{b}}_{\omega ,s} (x,y))\hbox {d}V} + \nonumber \\&\qquad +\,\int \limits _{\partial V} {{\mathbf{F}}_{u,\tau } (z)\cdot \delta {\mathbf{u}}_{\tau } (x,y)\cdot {\mathbf{p}}_{u} (x,y)+{\mathbf{F}}_{\omega ,\tau } (z)\cdot \delta {\varvec{\upomega }}_{\tau } (x,y)\cdot {\mathbf{p}}_{\omega } (x,y))\hbox {d}S} \end{aligned}$$
(2.16)

Here, the integrals over volume have been transformed into the integrals over surface by using the matrix analogy of the Gauss–Ostrogradsky divergence theorem in the form

$$\begin{aligned}&\int \limits _V {\mathbf{D}}_{u,\tau }^{y} \cdot ({\mathbf{C}}\cdot ({\mathbf{D}}_{u,s} \cdot {\mathbf{F}}_{u,s} (z)\cdot {\mathbf{u}}_{s} (x,y)+{\mathbf{D}}_{\omega ,s} \cdot {\mathbf{F}}_{\omega .s} (z)\cdot {\varvec{\upomega }}_{s} (x,y))\cdot \delta {\mathbf{u}}_{\tau } (x,y)\hbox {d}V \nonumber \\&\quad =\int \limits _{\partial V} {\mathbf{D}}_{n,\tau }^{u,T} \cdot ({\mathbf{C}}\cdot ({\mathbf{D}}_{u,s} \cdot {\mathbf{F}}_{u,s} (z)\cdot {\mathbf{u}}_{s} (x,y)+{\mathbf{D}}_{\omega ,s} \cdot {\mathbf{F}}_{\omega .s} (z)\cdot {\varvec{\upomega }}_{s} (x,y))\cdot \delta {\mathbf{u}}_{\tau } (x,y)\hbox {d}S , \nonumber \\&\int \limits _V {{\mathbf{D}}_{\omega ,\tau }^{y} \cdot ({\mathbf{A}}\cdot {\mathbf{D}}_{\omega ,s} \cdot {\mathbf{F}}_{\omega ,s} (z)\cdot {\varvec{\upomega }}_{s} (x,y))\cdot \delta {\varvec{\upomega }}_{\tau } (x,y)\hbox {d}V}\nonumber \\&\quad =\int \limits _{\partial V} {{\mathbf{D}}_{n,\tau }^{\omega ,T} \cdot ({\mathbf{A}}\cdot {\mathbf{D}}_{\omega ,s} \cdot {\mathbf{F}}_{\omega ,s} (z)\cdot {\varvec{\upomega }}_{s} (x,y))\cdot \delta {\varvec{\upomega }}_{\tau } (x,y)\hbox {d}S} \end{aligned}$$
(2.17)

where \({\mathbf{D}}_{a,\tau }^{T} ={\mathbf{D}}_{a,\tau }^{y,T} +{\mathbf{D}}_{a,\tau }^{C,T} \) are matrix operators of the form

$$\begin{aligned} {\mathbf{D}}_{a,\tau }^{y,T} =\left| {{\begin{array}{*{20}c} {F_{a_{x} ,\tau } (z)\frac{\partial }{\partial x}} &{} 0 &{} 0 &{} 0 &{} {F_{a_{x} ,\tau } (z)\frac{\partial }{\partial y}} &{} 0 &{} 0 &{} 0 &{} 0 \\ 0 &{} {F_{a_{y} ,\tau } (z)\frac{\partial }{\partial y}} &{} 0 &{} {F_{a_{y} ,\tau } (z)\frac{\partial }{\partial x}} &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 \\ 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} {F_{a_{z} ,\tau } (z)\frac{\partial }{\partial x}} &{} 0 &{} {F_{a_{z} ,\tau } (z)\frac{\partial }{\partial y}} &{} 0 \\ \end{array} }} \right| , \end{aligned}$$
(2.18)
$$\begin{aligned} {\mathbf{D}}_{a,\tau }^{C,T} =\left| {{\begin{array}{*{20}c} 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} {\frac{\partial F_{a_{x} ,\tau } (z)}{\partial z}} &{} 0 &{} 0 \\ 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} {\frac{\partial F_{a_{y} ,\tau } (z)}{\partial z}} \\ 0 &{} 0 &{} {\frac{\partial F_{a_{z} ,\tau } (z)}{\partial z}} &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 \\ \end{array} }} \right| \end{aligned}$$
(2.19)

and \({\mathbf{D}}_{n,\tau }^{a,T} \) is the matrix analogy of the vector normal to the boundary of the form

$$\begin{aligned} {\mathbf{D}}_{n,\tau }^{a,T} =\left| {{\begin{array}{*{20}c} {n_{x} F_{a_{x} ,\tau } (z)} &{} 0 &{} 0 &{} 0 &{} {n_{y} F_{a_{x} ,\tau } (z)} &{} 0 &{} 0 &{} 0 &{} 0 \\ 0 &{} {n_{y} F_{a_{y} ,\tau } (z)} &{} 0 &{} {n_{x} F_{a_{y} ,\tau } (z)} &{} 0 &{} 0 &{} 0 &{} 0 &{} 0\\ 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} {n_{x} F_{a_{z} ,\tau } (z)} &{} 0 &{} {n_{y} F_{a_{z} ,\tau } (z)} &{} 0 \\ \end{array} }} \right| \end{aligned}$$
(2.20)

In fact, Eq. (2.17) represent equations of equilibrium and natural boundary conditions for displacements and rotations of the micropolar elastic higher order plate in the integral form obtained using the CUF.

The integrals over volume and surface in (2.17) have the form

$$\begin{aligned} \int \limits _V {(\cdot )\hbox {d}V} =\int \limits _\Omega {\int \limits _{-h}^h {\left( \cdot \right) \hbox {d}z} \hbox {d}\Omega } ,{}\quad \int \limits _{\partial V} {(\cdot )\hbox {d}V} =\int \limits _{\partial \Omega } {\int \limits _{-h}^h {\left( \cdot \right) \hbox {d}z} \hbox {d}S} \end{aligned}$$
(2.21)

When taking into account this decomposition and integrating these equations over the plate’s thickness, as well as considering that variations \(\delta {\mathbf{u}}_{\tau }\) and \(\delta {\varvec{\upomega }}_{\tau }\) depend only on variables x and y, the differential equations for displacements and rotations of the micropolar elastic higher order plates can be presented in the matrix form

$$\begin{aligned} {\mathbf{L}}_{M}^{G} \cdot {\mathbf{u}}_{M}^{G} ={\mathbf{b}}_{M}^{G} \end{aligned}$$
(2.22)

where global matrix operator \({\mathbf{L}}_{n}^{G}\), vectors of unknown functions \({\mathbf{u}}_{M}^{G} \) and right hand \({\mathbf{b}}_{M}^{G} \) side have the form

$$\begin{aligned} {\mathbf{L}}_{M}^{G} =\left| {{\begin{array}{*{20}c} {{\mathbf{L}}_{1,1}^{loc} } &{} \cdots &{} {{\mathbf{L}}_{1,M}^{loc} } \\ \vdots &{} \ddots &{} \vdots \\ {{\mathbf{L}}_{M,1}^{loc} } &{} \cdots &{} {{\mathbf{L}}_{M,M}^{loc} } \\ \end{array} }} \right| ,\quad {\mathbf{u}}_{M}^{G} =\left| {{\begin{array}{*{20}c} {{\mathbf{u}}_{1}^{loc} } \\ \vdots \\ {{\mathbf{u}}_{M}^{loc} } \\ \end{array} }} \right| ,\quad {\mathbf{b}}_{M}^{G} =\left| {{\begin{array}{*{20}c} {{\mathbf{b}}_{1}^{loc} } \\ \vdots \\ {{\mathbf{b}}_{M}^{loc} } \\ \end{array} }} \right| \end{aligned}$$
(2.23)

Matrices \({\mathbf{L}}_{\tau ,s}^{loc} \)are the fundamental nucleus of the differential equations of equilibrium for the higher order micropolar elastic plates. The explicit form of \({\mathbf{L}}_{\tau ,s}^{loc} \) as well as of the vectors of local unknown functions \({\mathbf{u}}_{s}^{loc} \) and local expression for external body and surface loads \({\mathbf{b}}_{s}^{loc} \)and also expressions for natural boundary conditions have been presented in Carrera and Zozulya [19].

Essential boundary conditions for the micropolar elastic higher order plates can be presented in the matrix form

$$\begin{aligned} \left. {{\mathbf{B}}_{M}^{E,G} \cdot {\mathbf{u}}_{M}^{G} } \right| _{0}^{L} ={\mathbf{u}}_{M}^{0,G} \end{aligned}$$
(2.24)

where global matrix operator \({\mathbf{B}}_{M}^{E,G} \), vectors of the right-hand side have the form

$$\begin{aligned} {\mathbf{B}}_{M}^{E,G} =\left| {{\begin{array}{*{20}c} {{\mathbf{I}}} &{} \cdots &{} 0 \\ \vdots &{} \ddots &{} \vdots \\ 0 &{} \cdots &{} {{\mathbf{I}}} \\ \end{array} }} \right| ,\quad {\mathbf{u}}_{M}^{0,G} =\left| {{\begin{array}{*{20}c} {{\mathbf{u}}_{1}^{0,loc} } \\ \vdots \\ {{\mathbf{u}}_{M}^{0,loc} } \\ \end{array} }} \right| \end{aligned}$$
(2.25)

Here, \({\mathbf{I}}\)is the identity matrix and therefore global matrix operator \({\mathbf{B}}_{M}^{E,G} \) is the identity matrix operator.

In this section, the system of differential equations as well as natural and essential boundary conditions for the 2D linear micropolar theory of the elastic plate within the framework of the CUF is considered in detail. These equations will be used in the next sections for the development of the close form solution for the higher order micropolar 2D plate theories.

3 Navier’s close form solution for higher order micropolar elastic plate

Boundary-value problems for the system of equations and boundary conditions presented in Sect. 1 are generally solved numerically, for example by using finite element methods Carrera et al. [15]. In some special cases, they can be solved analytically. In this section, we develop Navier’s close form solution for higher order micropolar plates, which is based on the CUF.

Let us present displacements and rotation fields as a Fourier series expansion in the form

$$\begin{aligned} u_{x} (x,y,z)= & {} F_{u_{x} ,\tau } (z)\sum \limits _{n,m=0}^\infty {U_{u_{x} ,\tau }^{n.m} \cos \left( {\frac{n\pi }{L_{1} }x} \right) \sin \left( {\frac{m\pi }{L_{2} }y} \right) } ,\nonumber \\ \quad \omega _{x} (x,y,z)= & {} F_{\omega _{x} ,\tau } (z)\sum \limits _{n,m=0}^\infty {U_{\omega _{x} ,\tau }^{n,m} \sin \left( {\frac{n\pi }{L_{1} }x} \right) \cos \left( {\frac{m\pi }{L_{2} }y} \right) } , \nonumber \\ u_{y} (x,y,z)= & {} F_{u_{y} ,\tau } (z)\sum \limits _{n,m=0}^\infty {U_{u_{y} ,\tau }^{n,m} \sin \left( {\frac{n\pi }{L_{1} }x} \right) \cos \left( {\frac{m\pi }{L_{2} }y} \right) } ,\nonumber \\ \quad \omega _{y} (x,y,z)= & {} F_{\omega _{y} ,\tau } (z)\sum \limits _{n,m=0}^\infty {U_{\omega _{y} ,\tau }^{n,m} \cos \left( {\frac{n\pi }{L_{1} }x} \right) \sin \left( {\frac{m\pi }{L_{2} }y} \right) } , \nonumber \\ u_{z} (x,y,z)= & {} F_{u_{z} ,\tau } (z)\sum \limits _{n,m=0}^\infty {U_{u_{z} ,\tau }^{n,m} \sin \left( {\frac{n\pi }{L_{1} }x} \right) \sin \left( {\frac{m\pi }{L_{2} }y} \right) } ,\nonumber \\ \quad \omega _{z} (x,y,z)= & {} F_{\omega _{z} ,\tau } (z)\sum \limits _{n,m=0}^\infty {U_{\omega _{z} ,\tau }^{n,m} \cos \left( {\frac{n\pi }{L_{1} }x} \right) \cos \left( {\frac{m\pi }{L_{2} }y} \right) } , \end{aligned}$$
(3.1)

where \(U_{u_{x} ,\tau }^{n} ,\;\ldots \;,U_{\omega _{z} ,\tau }^{n} \) are coefficients of the Fourier series expansion of the functions \(u_{x,\tau } (y),\;\ldots \;,\omega _{z,\tau } (y)\)of the form

$$\begin{aligned} U_{u_{x} ,\tau }^{n,m}= & {} \frac{4}{L_{1} L_{2} }\int \limits _0^{L_{1} } {\cos \left( {\frac{n\pi }{L_{1} }x} \right) \int \limits _0^{L_{2} } {u_{x,\tau } (x,y)\sin \left( {\frac{m\pi }{L_{2} }y} \right) \hbox {d}y} \hbox {d}x} ,\;\ldots \;,U_{\omega _{z} ,\tau }^{n,m}\nonumber \\= & {} \frac{4}{L_{1} L_{2} }\int \limits _0^{L_{1} } {\cos \left( {\frac{n\pi }{L_{1} }x} \right) \int \limits _0^{L_{2} } {\omega _{z,\tau } (x,y)\cos \left( {\frac{m\pi }{L_{2} }y} \right) \hbox {d}y} \hbox {d}x} \end{aligned}$$
(3.2)

Components of the vector of external load will be presented here as a Fourier series expansion in the form

$$\begin{aligned} \begin{array}{l} {\tilde{b}}_{u_{x} ,\tau } (x,y)=\sum \limits _{n,m=0}^\infty {B_{u_{x} ,\tau }^{n.m} \cos \left( {\frac{n\pi }{L_{1} }x} \right) \sin \left( {\frac{m\pi }{L_{2} }y} \right) } ,\quad {\tilde{b}}_{\omega _{x} ,\tau } (x,y)=\sum \limits _{n,m=0}^\infty {B_{\omega _{x} ,\tau }^{n,m} \sin \left( {\frac{n\pi }{L_{1} }x} \right) \cos \left( {\frac{m\pi }{L_{2} }y} \right) } , \\ {\tilde{b}}_{u_{y} ,\tau } (x,y)=\sum \limits _{n,m=0}^\infty {B_{u_{y} ,\tau }^{n,m} \sin \left( {\frac{n\pi }{L_{1} }x} \right) \cos \left( {\frac{m\pi }{L_{2} }y} \right) } ,\quad {\tilde{b}}_{\omega _{y} ,\tau } (x,y)=\sum \limits _{n,m=0}^\infty {B_{\omega _{y} ,\tau }^{n,m} \cos \left( {\frac{n\pi }{L_{1} }x} \right) \sin \left( {\frac{m\pi }{L_{2} }y} \right) } , \\ {\tilde{b}}_{u_{z} ,\tau } (x,y)=\sum \limits _{n,m=0}^\infty {B_{u_{z} ,\tau }^{n,m} \sin \left( {\frac{n\pi }{L_{1} }x} \right) \sin \left( {\frac{m\pi }{L_{2} }y} \right) } ,\quad {\tilde{b}}_{\omega _{z} ,\tau } (x,y)=\sum \limits _{n,m=0}^\infty {B_{\omega _{z} ,\tau }^{n,m} \cos \left( {\frac{n\pi }{L_{1} }x} \right) \cos \left( {\frac{m\pi }{L_{2} }y} \right) } , \\ \end{array} \end{aligned}$$
(3.3)

where \(B_{u_{x} ,\tau }^{n,m} ,\;\ldots \;,B_{\omega _{z} ,\tau }^{n,m} \) are coefficients of the Fourier series expansion of the functions \({\tilde{b}}_{u_{x} ,\tau } (x,y),\;\ldots \;,{\tilde{b}}_{\omega _{z} ,\tau } (x,y)\), they have the form

$$\begin{aligned} B_{u_{x} ,\tau }^{n,m}= & {} \frac{4}{L_{1} L_{2} }\int \limits _0^{L_{1} } {\cos \left( {\frac{n\pi }{L_{1} }x} \right) \int \limits _0^{L_{2} } {{\tilde{b}}_{u_{x} ,\tau } (x,y)\sin \left( {\frac{m\pi }{L_{2} }y} \right) \hbox {d}y} \hbox {d}x} ,\;\cdots \;,B_{\omega _{z} ,\tau }^{n}\nonumber \\= & {} \frac{4}{L_{1} L_{2} }\int \limits _0^{L_{1} } {\cos \left( {\frac{n\pi }{L_{1} }x} \right) \int \limits _0^{L_{2} } {{\tilde{b}}_{\omega _{z} ,\tau } (x,y)\cos \left( {\frac{m\pi }{L_{2} }y} \right) \hbox {d}y} \hbox {d}x} \end{aligned}$$
(3.4)

Substitution of the representations (3.1) for the displacements and rotation fields into (2.22) for any n and m give us a system of linear algebraic equations for the Fourier series expansion coefficients in the form

$$\begin{aligned} {\mathbf{K}}_{n,m,M}^{G} \cdot {\mathbf{U}}_{n,m,M}^{G} ={\mathbf{B}}_{n,m,M}^{G} \end{aligned}$$
(3.5)

where global matrix operator \({\mathbf{K}}_{n,m,M}^{G} \), vectors of unknown coefficients \({\mathbf{U}}_{n,m,M}^{G} \) and right-hand side \({\mathbf{B}}_{n,m,M}^{G} \) have the form

$$\begin{aligned} {\mathbf{K}}_{n,m,M}^{G} =\left| {{\begin{array}{*{20}c} {{\mathbf{K}}_{1,1}^{n,m,loc} } &{} \cdots &{} {{\mathbf{K}}_{1,M}^{n,m,loc} } \\ \vdots &{} \ddots &{} \vdots \\ {{\mathbf{K}}_{M,1}^{n,m,loc} } &{} \cdots &{} {{\mathbf{K}}_{M,M}^{n,m,loc} } \\ \end{array} }} \right| ,\quad {\mathbf{U}}_{n,m,M}^{G} =\left| {{\begin{array}{*{20}c} {{\mathbf{U}}_{1}^{n,m,loc} } \\ \vdots \\ {{\mathbf{U}}_{M}^{n,m,loc} } \\ \end{array} }} \right| ,\quad {\mathbf{B}}_{n,m,M}^{G} =\left| {{\begin{array}{*{20}c} {{\mathbf{B}}_{1}^{n,m,loc} } \\ \vdots \\ {{\mathbf{B}}_{M}^{n,m,loc} } \\ \end{array} }} \right| \end{aligned}$$
(3.6)

Matrices \({\mathbf{K}}_{\tau ,s}^{loc} \)are the fundamental nucleus of the algebraic equations for the Navier’s solution for the higher order micropolar elastic beams. These matrices and vectors of local unknown functions \({\mathbf{U}}_{s}^{loc} \) and local expression for external body forces \({\mathbf{B}}_{s}^{loc} \)have the form

$$\begin{aligned}&{\mathbf{K}}_{\tau ,s}^{n,m,loc} =\left| {{\begin{array}{*{20}c} {K^{n,m,\tau ,s}_{u_{x} ,u_{x} } } &{} {K^{n,m,\tau ,s}_{u_{x} ,u_{y} } } &{} {K^{n,m,\tau ,s}_{u_{x} ,u_{z} } } &{} {K^{n,m,\tau ,s}_{u_{x} ,\omega _{x} } } &{} {K^{n,m,\tau ,s}_{u_{x} ,\omega _{y} } } &{} {K^{n,m,\tau ,s}_{u_{x} ,\omega _{z} } } \\ {K^{n,m,\tau ,s}_{u_{y} ,u_{x} } } &{} {K^{n,m,\tau ,s}_{u_{y} ,u_{y} } } &{} {K^{n,m,\tau ,s}_{u_{y} ,u_{z} } } &{} {K^{n,m,\tau ,s}_{u_{y} ,\omega _{x} } } &{} {K^{n,m,\tau ,s}_{u_{y} ,\omega _{y} } } &{} {K^{n,m,\tau ,s}_{u_{y} ,\omega _{z} } } \\ {K^{n,m,\tau ,s}_{u_{z} ,u_{x} } } &{} {K^{n,m,\tau ,s}_{u_{z} ,u_{y} } } &{} {K^{n,m,\tau ,s}_{u_{z} ,u_{z} } } &{} {K^{n,m,\tau ,s}_{u_{z} ,\omega _{x} } } &{} {K^{n,m,\tau ,s}_{u_{z} ,\omega _{y} } } &{} {K^{n,m,\tau ,s}_{u_{z} ,\omega _{z} } } \\ {K^{n,m,\tau ,s}_{\omega _{x} ,u_{x} } } &{} {K^{n,m,\tau ,s}_{\omega _{x} ,u_{y} } } &{} {K^{n,m,\tau ,s}_{\omega _{x} ,u_{z} } } &{} {K^{n,m,\tau ,s}_{\omega _{x} ,\omega _{x} } } &{} {K^{n,m,\tau ,s}_{\omega _{x} ,\omega _{y} } } &{} {K^{n,m,\tau ,s}_{\omega _{x} ,\omega _{z} } } \\ {K^{n,m,\tau ,s}_{\omega _{y} ,u_{x} } } &{} {K^{n,m,\tau ,s}_{\omega _{y} ,u_{y} } } &{} {K^{n,m,\tau ,s}_{\omega _{x} ,u_{z} } } &{} {K^{n,m,\tau ,s}_{\omega _{y} ,\omega _{x} } } &{} {K^{n,m,\tau ,s}_{\omega _{y} ,\omega _{y} } } &{} {K^{n,m,\tau ,s}_{\omega _{y} ,\omega _{z} } } \\ {K^{n,m,\tau ,s}_{\omega _{z} ,u_{x} } } &{} {K^{n,m,\tau ,s}_{\omega _{z} ,u_{y} } } &{} {K^{n,m,\tau ,s}_{\omega _{z} ,u_{z} } } &{} {K^{n,m,\tau ,s}_{\omega _{z} ,\omega _{x} } } &{} {K^{n,m,\tau ,s}_{\omega _{z} ,\omega _{y} } } &{} {K^{n,m,,\tau ,s}_{\omega _{z} ,\omega _{z} } } \\ \end{array} }} \right| ,\;{\mathbf{U}}_{s}^{n,loc} =\left| {{\begin{array}{*{20}c} {U_{u_{x} ,s}^{n,m} } \\ {U_{u_{y} ,s}^{n,m} } \\ {U_{u_{z} ,s}^{n,m} } \\ {U_{\omega _{x} ,s}^{n,m} } \\ {U_{\omega _{y} ,s}^{n,m} } \\ {U_{\omega _{z} ,s}^{n,m} } \\ \end{array} }} \right| ,\nonumber \\&\;{\mathbf{B}}_{s}^{n,loc} =\left| {{\begin{array}{*{20}c} {B_{u_{x} ,\tau }^{n,m} } \\ {B_{u_{y} ,\tau }^{n,m} } \\ {B_{u_{z} ,\tau }^{n,m} } \\ {B_{\omega _{x} ,\tau }^{n,m} } \\ {B_{\omega _{y} ,\tau }^{n,m} } \\ {B_{\omega _{z} ,\tau }^{n,m} } \\ \end{array} }} \right| \end{aligned}$$
(3.7)

Analytical expressions for coefficients of the fundamental nucleus matrixes \({\mathbf{K}}_{\tau ,s}^{n,m,loc} \) are presented in “Appendix.”

Using the equations presented here, one can find a analytical solution of the boundary-value problem for the higher order micropolar plate with homogeneous essential boundary conditions in the form of a Fourier series expansion (3.1). In the next sections, we specify the form of Eq. (3.5) and coefficients presented in “Appendix” for some relatively simple completely linear expansion cases (CLEC). The theories based on shear deformation and Kirchhoff–Love hypothesis can be considered as special case of the CLEC. For the CLEC, we present exactly expressions for corresponding differential equations and solve also some specific problems.

4 Linear expansion case for micropolar elastic plate

Here, we consider the particular case of the CLEC of the micropolar model of plates in detail. In this case, the displacement and rotation vectors are presented in the form

$$\begin{aligned} {\mathbf{u}}(x,y,z)={\mathbf{F}}_{{\mathbf{u}},1} (z)\cdot {\mathbf{u}}_{1} (x,y)+{\mathbf{F}}_{{\mathbf{u}},2} (z)\cdot {\mathbf{u}}_{2} (x,y),\quad {\varvec{\upomega }}(x,y,z)={\mathbf{F}}_{{\varvec{\upomega }},1} (z)\cdot {\varvec{\upomega }}_{1} (x,y)+{\mathbf{F}}_{{\varvec{\upomega }},2} (z)\cdot {\varvec{\upomega }}_{2} (x,y),\nonumber \\ \end{aligned}$$
(4.1)

where

$$\begin{aligned} \begin{array}{l} {\mathbf{F}}_{{\mathbf{u}},1} =\left| {{\begin{array}{*{20}c} 1 &{} 0 &{} 0 \\ 0 &{} 1 &{} 0 \\ 0 &{} 0 &{} 1 \\ \end{array} }} \right| ,\quad {}{}{\mathbf{u}}_{1} =\left| {{\begin{array}{*{20}c} {u_{x,1} } \\ {u_{y,1} } \\ {u_{z,1} } \\ \end{array} }} \right| ,\quad {}{}{\mathbf{F}}_{{\mathbf{u}},2} =\left| {{\begin{array}{*{20}c} z &{} 0 &{} 0 \\ 0 &{} z &{} 0 \\ 0 &{} 0 &{} z \\ \end{array} }} \right| ,\quad {}{}{}{\mathbf{u}}_{2} =\left| {{\begin{array}{*{20}c} {u_{x,2} } \\ {u_{y,2} } \\ {u_{z,2} } \\ \end{array} }} \right| , \\ {\mathbf{F}}_{{\varvec{\upomega }},1} =\left| {{\begin{array}{*{20}c} 1 &{} 0 &{} 0 \\ 0 &{} 1 &{} 0 \\ 0 &{} 0 &{} 1 \\ \end{array} }} \right| ,\quad {\varvec{\upomega }}_{1} =\left| {{\begin{array}{*{20}c} {\omega _{x,1} } \\ {\omega _{y,1} } \\ {\omega _{z,1} } \\ \end{array} }} \right| ,\quad {\mathbf{F}}_{{\varvec{\upomega }},2} =\left| {{\begin{array}{*{20}c} z &{} 0 &{} 0 \\ 0 &{} z &{} 0 \\ 0 &{} 0 &{} z \\ \end{array} }} \right| ,\quad {\varvec{\upomega }}_{2} =\left| {{\begin{array}{*{20}c} {\omega _{x,2} } \\ {\omega _{y,2} } \\ {\omega _{z,2} } \\ \end{array} }} \right| , \\ \end{array} \end{aligned}$$
(4.2)

In this case, vector of displacements \({\mathbf{u}}(x,y,z)\) as well as vector of rotations \({\varvec{\upomega }}(x,y,z)\) are represented by two vector-valued coefficients; Taylor expansion\({\mathbf{u}}_{1} (x,y)\), \({\mathbf{u}}_{2} (x,y)\) and \({\varvec{\upomega }}_{1} (x,y)\), \({\varvec{\upomega }}_{2} (x,y)\), all of which have three components. In total, we have twelve functions that describe the displacement and rotation fields in the micropolar plates. These functions are related by differential equations (2.22) together with boundary conditions of the form (2.24) for the case \(M=2\). In this case, integrals of the functions of the thickness coordinates in Eqs. (3.6)–(3.7) can be easily calculated analytically, and they only have values 0,2h and \({2h^{3}}/{3}\). By substituting these values in (3.5), we obtain the exact expressions for matrix operators \({\mathbf{L}}_{M}^{G} \)(2.23) of the system of differential equations. The obtained system of differential equations is the general one, it takes into account all variables represented in the expansion (4.1) and together with boundary conditions it can be used for analysis of the stress–strain state of the micropolar plates under arbitrary loading.

Analysis of the obtained differential equations shows that there are two groups of equations and corresponding unknown functions, which can be considered separately. Using series representations (3.1), these differential equations can be transformed in the corresponding algebraic equations. Let us consider each group of the equations in detail.

The first group of independent variables are \(U_{u_{z} ,1}^{n,m} ,U_{\omega _{x} ,1}^{n,m} ,\;U_{\omega _{y} ,1}^{n,m} ,U_{u_{x} ,2}^{n,m} ,U_{u_{y} ,2}^{n,m} ,U_{\omega _{z} ,2}^{n,m} \), and the displacements here are related to the bending mode of deformation in the z direction perpendicular to the plane x, y, which is represented by functions \(U_{u_{z} ,1}^{n,m} ,U_{u_{x} ,2}^{n,m} ,U_{u_{y} ,2}^{n,m} \) and rotations which are related to the twisting mode along x,y and z directions, which are represented by functions \(U_{\omega _{x} ,1}^{n,m} ,\;U_{\omega _{y} ,1}^{n,m} ,U_{\omega _{z} ,2}^{n,m} \). In this case, the system of differential equations (2.22) has the following form

$$\begin{aligned} {\mathbf{K}}_{n,m,2}^{G} =\left| {{\begin{array}{*{20}c} {K^{n,m,1,1}_{u_{z} ,u_{z} } } &{} {K^{n,m,1,1}_{u_{z} ,\omega _{x} } } &{} {K^{n,m,1,1}_{u_{z} ,\omega _{y} } } &{} {K^{n,m,1,2}_{u_{z} ,u_{x} } } &{} {K^{n,m,1,2}_{u_{z} ,u_{y} } } &{} {K^{n,m,1,2}_{u_{z} ,\omega _{z} } } \\ {K^{n,m,1,1}_{\omega _{x} ,u_{z} } } &{} {K^{n,m,1,1}_{\omega _{x} ,\omega _{x} } } &{} {K^{n,m,1,1}_{\omega _{x} ,\omega _{y} } } &{} {K^{n,m,1,2}_{\omega _{x} ,u_{x} } } &{} {K^{n,m,1,2}_{\omega _{x} ,u_{y} } } &{} {K^{n,m,1,2}_{\omega _{x} ,\omega _{z} } } \\ {K^{n,m,1,1}_{\omega _{y} ,u_{z} } } &{} {K^{n,m,1,1}_{\omega _{y} ,\omega _{x} } } &{} {K^{n,m,1,1}_{\omega _{y} ,\omega _{y} } } &{} {K^{n,m,1,2}_{\omega _{y} ,u_{x} } } &{} {K^{n,m,1,2}_{\omega _{y} ,u_{y} } } &{} {K^{n,m,1,2}_{\omega _{y} ,\omega _{z} } } \\ {K^{n,m,2,1}_{u_{x} ,u_{z} } } &{} {K^{n,m,2,1}_{u_{x} ,\omega _{x} } } &{} {K^{n,m,2,1}_{u_{x} ,\omega _{y} } } &{} {K^{n,m,2,2}_{u_{x} ,u_{x} } } &{} {K^{n,m,2,2}_{u_{x} ,u_{y} } } &{} {K^{n,m,2,2}_{u_{x} ,\omega _{z} } } \\ {K^{n,m,2,1}_{u_{y} ,u_{z} } } &{} {K^{n,m,2,1}_{u_{y} ,\omega _{x} } } &{} {K^{n,m,2,1}_{u_{y} ,\omega _{y} } } &{} {K^{n,m,2,2}_{u_{y} ,u_{x} } } &{} {K^{n,m,2,2}_{u_{y} ,u_{y} } } &{} {K^{n,m,2,2}_{u_{y} ,\omega _{z} } } \\ {K^{n,m,2,1}_{\omega _{z} ,u_{z} } } &{} {K^{n,m,2,1}_{\omega _{z} ,\omega _{x} } } &{} {K^{n,m,2,1}_{\omega _{z} ,\omega _{y} } } &{} {K^{n,m,2,2}_{\omega _{z} ,u_{x} } } &{} {K^{n,m,2,2}_{\omega _{z} ,u_{y} } } &{} {K^{n,m,2,2}_{\omega _{z} ,\omega _{z} } } \\ \end{array} }} \right| ,{\mathbf{U}}_{n,m,2}^{G} =\left| {{\begin{array}{*{20}c} {U_{u_{z} ,1}^{n,m} } \\ {U_{\omega _{x} ,1}^{n,m} } \\ {U_{\omega _{y} ,1}^{n,m} } \\ {U_{u_{x} ,2}^{n,m} } \\ {U_{u_{y} ,2}^{n,m} } \\ {U_{\omega _{z} ,2}^{n,m} } \\ \end{array} }} \right| ,\;{\mathbf{B}}_{n,m,2}^{G} =\left| {{\begin{array}{*{20}c} {B_{u_{z} ,1}^{n,m} } \\ {B_{\omega _{x} ,1}^{n,m} } \\ {B_{\omega _{y} ,1}^{n,m} } \\ {B_{u_{x} ,2}^{n,m} } \\ {B_{u_{y} ,2}^{n,m} } \\ {B_{\omega _{z} ,2}^{n,m} } \\ \end{array} }} \right| \nonumber \\ \end{aligned}$$
(4.3)

Analytical expressions for coefficients of the fundamental matrix \({\mathbf{K}}_{n,m,2}^{G} \)can be obtained from corresponding coefficients of the fundamental nucleus matrixes \({\mathbf{K}}_{\tau ,s}^{n,m,loc} \) presented in “Appendix.” Essential boundary conditions have the form similar to (2.24) with taking into account that the vector of independent variables has the form (4.3).

The second group of variables are \(U_{u_{x} ,1}^{n,m} ,U_{u_{y} ,1}^{n,m} ,\;U_{\omega _{z} ,1}^{n,m} ,U_{u_{z} ,2}^{n,m} ,U_{\omega _{x} ,2}^{n,m} ,U_{\omega _{y} ,2}^{n,m} \), and displacements here are related to the tension–compression mode of deformation in the plane x, y, which is represented by functions \(U_{u_{x} ,1}^{n,m} ,U_{u_{y} ,1}^{n,m} ,\;U_{u_{z} ,2}^{n,m} \) and rotations which are related to the stretching mode along x,y and z directions, which are represented by functions \(U_{\omega _{z} ,1}^{n,m} ,U_{\omega _{x} ,2}^{n,m} ,U_{\omega _{y} ,2}^{n,m} \). In this case, the system of differential equations (2.22) has the following form

$$\begin{aligned} {\mathbf{K}}_{n,m,2}^{G} =\left| {{\begin{array}{*{20}c} {K^{n,m,1,1}_{u_{x} ,u_{x} } } &{} {K^{n,m,1,1}_{u_{x} ,u_{y} } } &{} {K^{n,m,1,1}_{u_{x} ,\omega _{z} } } &{} {K^{n,m,1,2}_{u_{x} ,u_{z} } } &{} {K^{n,m,1,2}_{u_{x} ,\omega _{x} } } &{} {K^{n,m,1,2}_{u_{x} ,\omega _{y} } } \\ {K^{n,m,1,1}_{u_{y} ,u_{x} } } &{} {K^{n,m,1,1}_{u_{y} ,u_{y} } } &{} {K^{n,m,1,1}_{u_{y} ,\omega _{z} } } &{} {K^{n,m,1,2}_{u_{y} ,u_{z} } } &{} {K^{n,m,1,2}_{u_{y} ,\omega _{x} } } &{} {K^{n,m,1,2}_{u_{y} ,\omega _{y} } } \\ {K^{n,m,1,1}_{\omega _{z} ,u_{x} } } &{} {K^{n,m,1,1}_{\omega _{z} ,u_{y} } } &{} {K^{n,m,1,1}_{\omega _{z} ,\omega _{z} } } &{} {K^{n,m,1,2}_{\omega _{z} ,u_{z} } } &{} {K^{n,m,1,2}_{\omega _{z} ,\omega _{x} } } &{} {K^{n,m,1,2}_{\omega _{z} ,\omega _{y} } }\\ {K^{n,m,1,1}_{u_{z} ,u_{x} } } &{} {K^{n,m,1,1}_{u_{z} ,u_{y} } } &{} {K^{n,m,1,1}_{u_{z} ,\omega _{z} } } &{} {K^{n,m,1,2}_{u_{z} ,u_{z} } } &{} {K^{n,m,1,2}_{u_{z} ,\omega _{x} } } &{} {K^{n,m,1,2}_{u_{z} ,\omega _{y} } } \\ {K^{n,m,1,1}_{\omega _{x} ,u_{x} } } &{} {K^{n,m,1,1}_{\omega _{x} ,u_{y} } } &{} {K^{n,m,1,1}_{\omega _{x} ,\omega _{z} } } &{} {K^{n,m,1,2}_{\omega _{x} ,u_{z} } } &{} {K^{n,m,1,2}_{\omega _{x} ,\omega _{x} } } &{} {K^{n,m,1,2}_{\omega _{x} ,\omega _{y} } }\\ {K^{n,m,1,1}_{\omega _{y} ,u_{x} } } &{} {K^{n,m,1,1}_{\omega _{y} ,u_{y} } } &{} {K^{n,m,1,1}_{\omega _{y} ,\omega _{z} } } &{} {K^{n,m,1,2}_{\omega _{y} ,u_{z} } } &{} {K^{n,m,1,2}_{\omega _{y} ,\omega _{x} } } &{} {K^{n,m,2,2}_{\omega _{y} ,\omega _{z} } }\\ \end{array} }} \right| ,{\mathbf{U}}_{n,m,2}^{G} =\left| {{\begin{array}{*{20}c} {U_{u_{x} ,1}^{n,m} } \\ {U_{u_{y} ,1}^{n,m} } \\ {U_{\omega _{z} ,1}^{n,m} } \\ {U_{u_{z} ,2}^{n,m} } \\ {U_{\omega _{x} ,2}^{n,m} } \\ {U_{\omega _{y} ,2}^{n,m} } \\ \end{array} }} \right| ,\;{\mathbf{B}}_{n,m,2}^{G} =\left| {{\begin{array}{*{20}c} {B_{u_{z} ,1}^{n,m} } \\ {B_{u_{y} ,1}^{n,m} } \\ {B_{\omega _{z} ,1}^{n,m} } \\ {B_{u_{z} ,2}^{n,m} } \\ {B_{\omega _{x} ,2}^{n,m} } \\ {B_{\omega _{y} ,2}^{n,m} } \\ \end{array} }} \right| \nonumber \\ \end{aligned}$$
(4.4)

Analytical expressions for coefficients of the fundamental matrix \({\mathbf{K}}_{n,m,2}^{G}\) can be obtained from corresponding coefficients of the fundamental nucleus matrixes \({\mathbf{K}}_{\tau ,s}^{n,m,loc}\) presented in “Appendix.” Essential boundary conditions have the form similar to (2.24) with taking into account that the vector of independent variables has the form (4.4).

In the case of isotropic material, Eqs. (4.3) and (4.4) are simplified. In Nowacki’s notations (2.7), full form of the differential equations of equilibrium in form of displacement and rotation for bending and tension–compression modes has been presented in “Appendix.” Essential boundary conditions in the case of the isotropic material have the same form as for considered above case of orthotropic material.

5 Models of micropolar plates based on Mindlin’s hypothesis

Theories of micropolar plates, which are based on the shear deformation Mindlin’s hypothesis, can be obtained as a special case of the linear expansion theory developed in the previous section.

According to the shear deformation kinematic assumptions and based on the CUF, the displacement field of the micropolar plate can be presented in the form

$$\begin{aligned} \begin{array}{l} u_{x} (x,y,z)=u_{x,1} (x,y)+zu_{x,2} (x,y), \\ u_{y} (x,y,z)=u_{y,1} (x,y)+zu_{x,2} (x,y), \\ u_{z} (x,y,z)=u_{z,1} (x,y), \\ \end{array} \end{aligned}$$
(5.1)

Following Eringen [24], Altenbach and Eremeyev [2] and Carrera and Zozulya [19] and based on the analysis of Eqs. (4.3) and (4.4) of the linear micropolar plates model, the rotation field will be presented in the form

$$\begin{aligned} \omega _{x} (x,y,z)=\omega _{x,1} (x,y),\quad \omega _{y} (x,y,z)=\omega _{y,1} (x,y),\quad \omega _{z} (x,y,z)=\omega _{z,1} (x,y), \end{aligned}$$
(5.2)

In this case, basic functions of the thickness coordinate (2.10) have the form

$$\begin{aligned} {\mathbf{F}}_{{\mathbf{u}},1} =\left| {{\begin{array}{*{20}c} 1 &{} 0 &{} 0 \\ 0 &{} 1 &{} 0 \\ 0 &{} 0 &{} 1 \\ \end{array} }} \right| ,\quad {\mathbf{F}}_{{\mathbf{u}},2} =\left| {{\begin{array}{*{20}c} z &{} 0 &{} 0 \\ 0 &{} z &{} 0 \\ 0 &{} 0 &{} 0 \\ \end{array} }} \right| ,\;\quad {\mathbf{F}}_{{\varvec{\upomega }},1} =\left| {{\begin{array}{*{20}c} 1 &{} 0 &{} 0 \\ 0 &{} 1 &{} 0 \\ 0 &{} 0 &{} 1 \\ \end{array} }} \right| ,\; \end{aligned}$$
(5.3)

In this case, we have five unknown functions \(u_{x,1} (x,y),u_{y,1} (x,y),u_{z,1} (x,y),u_{x,2} (x,y),u_{x,2} (x,y),\)for the displacement vector \({\mathbf{u}}(x,y,z)\), and three unknown functions \(\omega _{x,1} (x,y),\omega _{y,1} (x,y),\omega _{z,1} (x,y)\) for the rotation vector \({\varvec{\upomega }}(x,y,z)\), in total we have eight functions that describe the displacement and rotation fields in the micropolar plate. These functions are related by the system of differential equations, which can be obtained from the corresponding equations for the linear theory by just deleting functions that are omitted in the theory based on the shear deformation hypothesis.

Analysis of the corresponding equations shows that in the same way as in the linear theory case there are two groups of equations along with their corresponding variables that can be considered separately, independently of other equations of the full system. Let us consider the equations of each group separately.

The first group of variables are \(U_{u_{z} ,1}^{n,m} ,U_{\omega _{x} ,1}^{n,m} ,\;U_{\omega _{y} ,1}^{n,m} ,U_{u_{x} ,2}^{n,m} ,U_{u_{y} ,2}^{n,m} \), the displacements here are related to the bending mode of deformation in the z direction, and the rotations are related to the twisting along directions x and y. Twisting along direction z is not presented in this group of equations; therefore, the system of differential equations in form of displacement and rotation has the following form

$$\begin{aligned} {\mathbf{K}}_{n,m,2}^{G} =\left| {{\begin{array}{*{20}c} {K^{n,m,1,1}_{u_{z} ,u_{z} } } &{} {K^{n,m,1,1}_{u_{z} ,\omega _{x} } } &{} {K^{n,m,1,1}_{u_{z} ,\omega _{y} } } &{} {K^{n,m,1,2}_{u_{z} ,u_{x} } } &{} {K^{n,m,1,2}_{u_{z} ,u_{y} } } \\ {K^{n,m,1,1}_{\omega _{x} ,u_{z} } } &{} {K^{n,m,1,1}_{\omega _{x} ,\omega _{x} } } &{} {K^{n,m,1,1}_{\omega _{x} ,\omega _{y} } } &{} {K^{n,m,1,2}_{\omega _{x} ,u_{x} } } &{} {K^{n,m,1,2}_{\omega _{x} ,u_{y} } } \\ {K^{n,m,1,1}_{\omega _{y} ,u_{z} } } &{} {K^{n,m,1,1}_{\omega _{y} ,\omega _{x} } } &{} {K^{n,m,1,1}_{\omega _{y} ,\omega _{y} } } &{} {K^{n,m,1,2}_{\omega _{y} ,u_{x} } } &{} {K^{n,m,1,2}_{\omega _{y} ,u_{y} } } \\ {K^{n,m,2,1}_{u_{x} ,u_{z} } } &{} {K^{n,m,2,1}_{u_{x} ,\omega _{x} } } &{} {K^{n,m,2,1}_{u_{x} ,\omega _{y} } } &{} {K^{n,m,2,2}_{u_{x} ,u_{x} } } &{} {K^{n,m,2,2}_{u_{x} ,u_{y} } } \\ {K^{n,m,2,1}_{u_{y} ,u_{z} } } &{} {K^{n,m,2,1}_{u_{y} ,\omega _{x} } } &{} {K^{n,m,2,1}_{u_{y} ,\omega _{y} } } &{} {K^{n,m,2,2}_{u_{y} ,u_{x} } } &{} {K^{n,m,2,2}_{u_{y} ,u_{y} } } \\ \end{array} }} \right| ,{\mathbf{U}}_{n,m,2}^{G} =\left| {{\begin{array}{*{20}c} {U_{u_{z} ,1}^{n,m} } \\ {U_{\omega _{x} ,1}^{n,m} } \\ {U_{\omega _{y} ,1}^{n,m} } \\ {U_{u_{x} ,2}^{n,m} } \\ {U_{u_{y} ,2}^{n,m} } \\ \end{array} }} \right| ,\;{\mathbf{B}}_{n,m,2}^{G} =\left| {{\begin{array}{*{20}c} {B_{u_{z} ,1}^{n,m} } \\ {B_{\omega _{x} ,1}^{n,m} } \\ {B_{\omega _{y} ,1}^{n,m} } \\ {B_{u_{x} ,2}^{n,m} } \\ {B_{u_{y} ,2}^{n,m} } \\ \end{array} }} \right| \end{aligned}$$
(5.4)

Analytical expressions for coefficients of the fundamental matrix \({\mathbf{K}}_{n,m,2}^{G} \)can be obtained from corresponding coefficients of the fundamental nucleus matrixes \({\mathbf{K}}_{\tau ,s}^{n,m,loc} \) presented in “Appendix.” Essential boundary conditions have the form similar to (2.24) with taking into account that the vector of independent variables has the form (5.4).

The second group of variables are \(U_{u_{x} ,1}^{n,m} ,U_{u_{y} ,1}^{n,m} ,\;U_{\omega _{z} ,1}^{n,m} \), the displacements here are related to the tension–compression mode of deformation in the plane x, y and the rotations are related to the twisting mode along direction z. The twisting along directions x and y is not presented in this system of equations and therefore, has the following form

$$\begin{aligned} {\mathbf{K}}_{n,m,2}^{G} =\left| {{\begin{array}{*{20}c} {K^{n,m,1,1}_{u_{x} ,u_{x} } } &{} {K^{n,m,1,1}_{u_{x} ,u_{y} } } &{} {K^{n,m,1,1}_{u_{x} ,\omega _{z} } } \\ {K^{n,m,1,1}_{u_{y} ,u_{x} } } &{} {K^{n,m,1,1}_{u_{y} ,u_{y} } } &{} {K^{n,m,1,1}_{u_{y} ,\omega _{z} } } \\ {K^{n,m,1,1}_{\omega _{z} ,u_{x} } } &{} {K^{n,m,1,1}_{\omega _{z} ,u_{y} } } &{} {K^{n,m,1,1}_{\omega _{z} ,\omega _{z} } } \\ \end{array} }} \right| ,{\mathbf{U}}_{n,m,2}^{G} =\left| {{\begin{array}{*{20}c} {U_{u_{x} ,1}^{n,m} } \\ {U_{u_{y} ,1}^{n,m} } \\ {U_{\omega _{z} ,1}^{n,m} } \\ \end{array} }} \right| ,\;{\mathbf{B}}_{n,m,2}^{G} =\left| {{\begin{array}{*{20}c} {B_{u_{x} ,1}^{n,m} } \\ {B_{u_{y} ,1}^{n,m} } \\ {B_{\omega _{z} ,1}^{n,m} } \\ \end{array} }} \right| \end{aligned}$$
(5.5)

Analytical expressions for coefficients of the fundamental matrix \({\mathbf{K}}_{n,m,2}^{G} \)can be obtained from corresponding coefficients of the fundamental nucleus matrixes \({\mathbf{K}}_{\tau ,s}^{n,m,loc} \) presented in “Appendix.” Essential boundary conditions have the form similar to (2.24) with taking into account that the vector of independent variables has the form (5.5).

In the case of isotropic material, Eqs. (5.4) and (5.5) are simplified. In Nowacki’s notations (2.7), full form of the differential equations of equilibrium in form of displacement and rotation for bending and tension–compression modes have been presented in “Appendix.” Essential boundary conditions in the case of the isotropic material have the same form as for considered above case of orthotropic material.

6 Some numerical results and discussion

In this section, we provide the results of the evaluation of the presented higher order models of the micropolar elastic plates and consider examples of the numerical calculation of the displacements, rotation, as well as classical and micropolar stress tensors. Because experimental data for mechanical characteristics of the classical and micropolar properties of materials is available only for isotropic cases, only isotropic materials will be considered here. As it was mentioned in Carrera and Zozulya [19], different authors use different notations for field functions and for classical and micropolar material constants. Therefore, usually it is a complicated task to analyze and compare the results obtained by different authors. In Cowin [23], Hassanpour and Heppler [40] and Jemielita [41] relations between classical and micropolar constants used by different authors are presented. The most frequently used are notations introduced by Nowacki [52] and Eringen [32]. In spite of many disadvantages, Eringen’s notations critically analyzed in Hassanpour and Heppler [40] are used in numerous theoretical (see Altenbach and Eremeyev [2], Ansari, et al., [7, 8], Eremeyev, at all, [28, 29], Kovvali and Hodges [43], etc.), and experimental (see Gauthier [34], Gauthier and Jahsman [35, 35], Lakes [46, 47, 49], Park and Lakes [54], Yang and Lakes [65], etc.) works. That is way in this work we use Nowacki’s notations. In Table 1, we present the relation between Nowacki’s notations used here and Eringen’s notations, as well as for the notations used in the review paper by Hassanpour and Heppler [40], where summarized information about experimental data of classical and micropolar constants which is available in the literature. In Table 1, constants in Eringen’s notations supplied with upper index E and constants in Hassanpour and Heppler’s notations with upper index H as well as constants in notations used here are presented without upper index.

Table 1 Comparison of notations for material constants
Full size table

Another problem which also complicates the situation is the following, in the works related to the experimental tests of materials, and classical and micropolar mechanical propertied definition are used so-called engineering constants. The relations between engineering constants and constants used for the constitutive law formulation in Eringen’s notations are presented in Gauthier [34], Lakes [47, 49], etc. For convenience, we present the relations between engineering constants and constants used for the constitutive law formulation in Nowacki’s notations here

$$\begin{aligned} \begin{array}{l} E=\frac{\mu (3\lambda +2\mu )}{\lambda +\mu },G=\mu ,\nu =\frac{\lambda }{2(\lambda +\mu )}, \\ l_{t}^{2} =\frac{\gamma }{\mu },l_{b}^{2} =\frac{\gamma +\varepsilon }{4\mu },N^{2}=\frac{\alpha }{\mu +\alpha },\psi =\frac{2\gamma }{\beta +2\gamma } \\ \end{array} \end{aligned}$$
(6.1)

where E, G and \(\nu \) are Young’s and shear modules and Poisson ratio, \(l_{b} \) and \(l_{t} \) are characteristic lengths for bending and torsion, respectively, Nis a coupling number, \(\psi \)is a polar ratio.

Now, from the relations (6.1) one can find all of the material constants used for the constitutive relations formulation (2.8) in Nowacki’s notations (2.7). They have the following form

$$\begin{aligned} \begin{array}{l} \lambda =\frac{2G\nu }{1-2\nu },\mu =G,\alpha =\frac{GN^{2}}{(N^{2}-1)}, \\ \beta =\frac{2(\alpha +\mu )(\psi -1)l_{1}^{2} }{\psi },\gamma =\mu l_{t}^{2} ,\varepsilon =\mu (4l_{b}^{2} -l_{t}^{2} ), \\ \end{array} \end{aligned}$$
(6.2)

For convenience, we translate the experimental data for the classical and micropolar elastic moduli for different materials available in the literature to Nowacki’s notations using relations (6.2) and present in Table 2.

Table 2 Experimental data for classical and micropolar moduli of some materials in Nowacki’s notations
Full size table

The information presented in Table 2 was gathered from the references presented in the parentheses below. We also use the following abbreviations: HB - human bone (Lakes [47, 48], Park and Lakes [54], Yang and Lakes [65]), FPSF-PSF foam (Lakes [46, 48]), FDP-dense polyurethane foam (Lakes [47, 48]), FP-polyurethane foam (Lakes [46, 48]), FS-syntactic foam (Lakes [46, 48]), WF300, WF110 and WF51-polymethacrylimide foam of the corresponding grade (Anderson and Lakes [6]), AEC-aluminum–epoxy composite (Gauthier [34], Gauthier and Jahsman [35, 36]). We have to mention that most of the data presented in Table 2 can be found in the papers Hassanpour and Heppler [40] and Lakes [48], but in a different form and different notations.

In order to validate the proposed higher order model of the micropolar plates, we (following Kvasov and Steinberg [45] and Steinberg and Kvasov [61]) consider the bending of the square micropolar plate of thickness\(2h=0.1 \text{ m }\), made of polyurethane foam, subjected to the mechanical loading applied in the perpendicular direction to the top surface of the plate. The values of the polyurethane foam elastic and micropolar elastic moduli are presented in Table 2, line four. The distribution of the applied mechanical loading is sinusoidal and has the form

$$\begin{aligned} {\tilde{b}}_{u_{z} ,1} (x,y)=B_{u_{z} ,1}^{1,1} \sin \left( {\frac{\pi }{L}x} \right) \sin \left( {\frac{\pi }{L}y} \right) \end{aligned}$$
(6.3)

Here, \(L_{1} =L_{2} =L\) is the length of the plate side, \(B_{u_{z} ,1}^{1,1} \) is an amplitude of the mechanical loading.

For the numerical calculation, we use Navier’s close form solutions presented for higher order theories by Eqs. (3.1)–(3.5) and for CLEC presented by Eq. (4.3). Amplitude of the mechanical loading is taken \(B_{u_{z} ,1}^{1,1} =10^{6}\;(\hbox {N}/{\hbox {m}^{2}})\) for the cases \(2h/L=1/ 5,\dots ,1/15\) and \(B_{u_{z} ,1}^{1,1} =10^{5}\;(\hbox {N} /{\hbox {m}^{2}})\) for the case \(2h/L=1/20, \ldots ,1/30\). The results of the displacements and the rotations calculation for the orders \(M=1,\dots ,4\) models are presented in Table 3. Unfortunately, we cannot compare the obtained results with the ones reported in Kvasov and Steinberg [45] and Steinberg and Kvasov [61] because in those papers calculations were done for the wrong dimension of the micropolar moduli. For the right dimension of the classical and micropolar moduli refer to Table 2 (also see papers Carrera and Zozulya [19], Hassanpour and Heppler [40], Eremeyev et al. [28, 29], Kovvali and Hodges [43], Shaw [59], etc.).

Table 3 Displacements and rotation of the free supported plate for the theories of order \(M=1,\ldots ,4\)
Full size table
Fig. 1
figure 1

Components \(u_{x} \) and \(u_{z} \) of the displacement vector of the micropolar plate in x direction

Full size image
Fig. 2
figure 2

Components \(u_{x} \) and \(u_{z} \) of the displacement vector of the micropolar plate in x and y directions

Full size image
Fig. 3
figure 3

Components \(\omega _{x} \) and \(\omega _{y} \) of the rotation vector of the micropolar plate in x and y directions, respectively

Full size image
Fig. 4
figure 4

Components \(\omega _{x} \) and \(\omega _{y} \) of the rotation vector of the micropolar plate in x and y directions

Full size image
Fig. 5
figure 5

Components \(\sigma _{xx} \) and \(\sigma _{zz} \) of the force stress tensor of the micropolar plate in y direction

Full size image
Fig. 6
figure 6

Components \(\sigma _{xx} \) and \(\sigma _{zz} \) of the force stress tensor of the micropolar plate in x and y directions

Full size image
Fig. 7
figure 7

Components \(\sigma _{xx} \) and \(\sigma _{zz} \) of the force stress tensor of the micropolar plate in y and z directions

Full size image

In order to illustrate theory developed above of the higher order micropolar elastic beam using CUF, we consider the micropolar elastic plate with \(2h/L=1/ {10}\) subjected to the mechanical loading applied to the upper surfaces of the plate. Distribution of the applied mechanical loading has the form

$$\begin{aligned} \begin{array}{l} {\tilde{b}}_{u_{x} ,1} (x,y)=B_{u_{x} ,1}^{1,1} \cos \left( {\frac{\pi }{L}x} \right) \sin \left( {\frac{\pi }{L}y} \right) , \\ {\tilde{b}}_{u_{y} ,1} (x,y)=B_{u_{y} ,1}^{1,1} \sin \left( {\frac{\pi }{L}x} \right) \cos \left( {\frac{\pi }{L}y} \right) , \\ {\tilde{b}}_{u_{z} ,1} (x,y)=B_{u_{z} ,1}^{1,1} \sin \left( {\frac{\pi }{L}x} \right) \sin \left( {\frac{\pi }{L}y} \right) . \\ \end{array} \end{aligned}$$
(6.4)

Analysis of the data presented in Table 3 as well as Figs. 1 and 3 shows that for theories of order \(M=2\) and higher order the displacements and the rotations have almost the same values. Therefore, rest the calculations have been done for the case \(M=2\). The results of calculations of the displacement and rotation field are presented in Figs. 1, 2, 3 and 4. Components of the classical force and couple stress tensors are presented in Figs. 5, 6, 7, 8, 9, 10, 11, 12, 13, 14, 15 and 16. For the displacement and rotation field, we present line graphs of their distribution along the line \(y=L/2\) and surface graphs of their distribution along plane x, y. For the classical force and couple stress tensors beside linear and surface graphs mentioned above, we also present contour graphs of their distribution along the thickness.

Fig. 8
figure 8

Components \(\sigma _{xy} \) and \(\sigma _{xz} \) of the force stress tensor of the micropolar plate in y direction

Full size image
Fig. 9
figure 9

Components \(\sigma _{xy} \) and \(\sigma _{xz} \) of the force stress tensor of the micropolar plate in x and y directions

Full size image
Fig. 10
figure 10

Components \(\sigma _{xy}\) and \(\sigma _{xz} \) of the force stress tensor of the micropolar plate in y and z directions

Full size image
Fig. 11
figure 11

Components \(\mu _{xx}\) and \(\mu _{yy} \) of the couple stress tensor of the micropolar plate in y direction

Full size image
Fig. 12
figure 12

Components \(\mu _{xx}\) and \(\mu _{yy} \) of the couple stress tensor of the micropolar plate in x and y directions

Full size image
Fig. 13
figure 13

Components \(\mu _{xx}\) and \(\mu _{yy}\) of the couple stress tensor of the micropolar plate in y and z directions

Full size image
Fig. 14
figure 14

Components \(\mu _{xy} \) and \(\mu _{xz}\) of the couple stress tensor of the micropolar plate in y direction

Full size image
Fig. 15
figure 15

Components \(\mu _{xy} \) and \(\mu _{xz} \) of the couple stress tensor of the micropolar plate in x and y directions

Full size image
Fig. 16
figure 16

Components \(\mu _{xy} \) and \(\mu _{xz} \) of the couple stress tensor of the micropolar plate in y and z directions

Full size image

The data presented in Table 3 and in Figs. 5, 6, 7, 8, 9, 10, 11, 12, 13, 14, 15 and 16 give qualitative and quantitative information about the behavior of the displacements, rotation as well as forced and couple stress. Analysis of the equations of micropolar theory of plates based on the Kirchhoff hypothesis presented in Altenbach and Eremeyev [2], Carrera and Zozulya [19] and Eringen [31] shows that equations for rotational fields can be solved independently of displacement fields. It means that in this case the consistent theory of micropolar plates cannot be developed. The result presented in Table 3 and in Figs. 1 and 3 for theories of the first-order \(M=1\) coincides with one obtained using the micropolar theory of plated based on Mindlin’s hypothesis presented in Sect. 5. It can be used as a benchmark example for the finite element analysis of micropolar plates.

7 Conclusion

In this paper, we apply Navier’s method of the variables separation for higher-order theories for micropolar plates based on the CUF approach. In this publication, the 2D micropolar plate theory is developed from general 3D equations of linear micropolar elasticity using the principle of virtual displacements and the CUF approach. The obtained equations have been used here for the development of the close form Navier’s solution and computation of the displacements, rotations as well as classical force and micropolar couple stress tensors.

A complete 2D system of the differential equations for the higher order theory of micropolar elastic plates is presented here. Carrera and Zozulya [19] have been presented in details theoretical results and complete system of the equations for micropolar plates based on the CUF approach. Whereas in this paper, Navier’s closed-form solution for the case of the simply supported plate has been developed for the in general case of higher order theory of micropolar plates based on CUF. For the case of The CLEC approximation theory, elements of the matrix operators that are presented in the Navier’s closed-form solution are presented in the explicate form. For the verification and application of the theories of the higher order micropolar plates based on CUF that have been developed here, numerical examples have been considered and the influence of the rotation field on the stress–strain fields has been analyzed.

Theoretical and experimental methods to determinate the classical and micropolar elastic moduli of different materials have been analyzed, and available experimental data have been presented in Nowacki’s notations.

The obtained equations can be used for stress–strain calculation as well as for modeling thin-walled structures in macro-, micro- and nano-scales by taking into account micropolar couple stress and rotation effects. The proposed models can especially be efficient in MEMS and NEMS modeling as well as computer simulation.