An outline of the non-linear viscoelastic behaviour of wheat flour dough in shear

Abstract

Creep and creep recovery, stress relaxation and small- and large-amplitude oscillatory shear experiments have been used to study the steady-state flow behaviour and the transient viscoelastic response of wheat flour dough in shear over large ranges of time, stress and strain. The results are discussed with reference to the limited body of reliable literature data. Dough does display a linear viscoelastic domain. The complex character of its non-linear viscoelastic properties is essentially due to the extremely low shear rate limit of the initial Newtonian plateau and to the onset of time-dependent flow behaviour above a certain strain threshold, which explain qualitatively the discrepancies observed in certain cases on a part of the range of the rheological variables explored, despite global self-consistency of the results. Comparison of gluten and dough linear viscoelastic properties shows that dough cannot be viewed simply as a concentrated suspension of starch granules in the hydrated viscoelastic gluten matrix.

Introduction

It has been recognised for long that the rheological characteristics of wheat flour dough are involved all along the breadmaking process and that they control, to a large extent, the quality of the end products. Dough rheology has been therefore the subject of much work. But it is a recurrent topic because most of the investigations have been based on an altogether empirical experimental approach, or on rheological data that are non-empirical in essence but are handled and analysed in an empirical way. An impressive body of practical knowledge, very useful for wheat breeders and for processing industries, has emerged from these studies. However, the empirical approach does not yield a quantitative rheological description, which could be related to the structure of the material through a physical model. Moreover, the predictive power of the empirical approach, based essentially on statistical correlations between more or less arbitrarily chosen parameters, taken as descriptors of the rheological properties and composition data or quality indices, is quite limited. Often, the method even proves misleading.

On the other hand, the renewed interest in the matter, the larger availability of performing rheological instruments and a better apprehension of rheological methods in the field of cereal science aroused more fundamental studies on dough rheology. A recent review (Dobraszczyk and Morgenstern 2003) contains an impressive list, and yet, it is not exhaustive. As stressed in this review, dough processing and fermentation involve elongational deformations besides shear. Uniaxial and biaxial deformations are applied to dough in many empirical testing instruments and procedures. However, reliable true rheological measurements are much more difficult to obtain, to analyse and to interpret in these modes of deformation than in shear, whereas, actually, many aspects of dough shear behaviour remain ill characterised and ill understood. Most of the published work is based on low-shear dynamic measurements. The rheological information obtained is thus limited to the linear domain, and moreover to the restricted time scale corresponding to the frequency range of rotational rheometers, limited in practice to 0.001–100 rad/s at best. The time scale of the viscoelastic response of the material should dictate the time scale of the rheological experiments; in the case of dough, steady state requires several hours to be established, as it will be shown later. Therefore, we cannot get even an outline of dough linear viscoelastic behaviour in this way, while, on the other hand, the importance of characterising the non-linear behaviour, because dough is submitted to large strains and shear rates during processing, is well recognised (Dobraszczyk and Morgenstern 2003). Transient regime methods (stress relaxation and retardation tests) allow us, in principle, to extend the experimental window as far as required in the longer-times direction for the steady state to be reached, and these methods can be used beyond the linear viscoelastic limit as simply as within the limit. Nonetheless, these advantages have not been fully exploited in the case of dough. In many studies, shear creep and recovery (Bloksma 1962; Glucklich and Shelef 1962; Campos et al. 1997; Wang and Sun 2002; Pedersen et al. 2004) or shear stress relaxation (Ramkumar et al. 1996; Rao et al. 2000, 2001; Li et al. 2003) were carried out over time scales that were, by far, too short (a few minutes), precluding in fact any characterisation and analysis of the rheological behaviour. Anyway, in general, this was not the purpose of this kind of work, where rheological measurements are used in an empirical way, for instance, to compare different wheat varieties or to examine possible correlations with technological characteristics. Comparatively few papers report results performed over longer time scales. In most of them, the linear or low-shear behaviour only (Edwards et al. 2001; Phan-Thien and Safari-Ardi 1998; Safar-Ardi and Phan-Thien 1998; Uthayakumaran et al. 2002), or one arbitrarily chosen high value of the relaxation strain (Keentok et al. 2002), was considered. The retardation experiments of Smith and Tschoegl (1970) in simple tension were also performed in the linear viscoelastic domain. The non-linear retardation (Hibberd and Parker 1979; Lefebvre et al. 2004) or stress relaxation (Bohlin and Carlson 1981) behaviours of dough in shear have actually been little-studied, and only over quite limited ranges of stress or of strain.

Therefore, there is a need for more systematic investigation of dough rheology in shear, especially as regards the non-linear viscoelasticity and the steady-state properties of this material. The purpose of this paper is to delineate the rheological behaviour of flour-and-water dough in shear over large time, stress, shear rate and strain ranges. The general approach is based on the systematic and reasoned use of classical rheological tests. We have also applied a less conventional method, the large amplitude oscillatory shear test; this technique is attracting increasing attention in the field of complex materials characterisation, but, to our knowledge, it has been applied to dough in only one paper (Phan-Thien et al. 2000). At the expense of some simplification, the approach we follow allows quantitative, although still partial, analysis of the steady state and of the transient viscoelastic non-linear responses of dough to be made. However, it is extremely time-consuming due to the very long viscoelastic response of dough. For this reason, the study that is reported in this paper is limited to one example of standard dough.

Materials and methods

Material

Because of the large quantities of material needed to carry out the work, a commercial flour lot (“CNS flour”; 10.5% protein, 0.556% ash, db; Chopin Alveograph characteristics: W=194 J, P/L=0.56), with good baking performance according to French standards, was used. Dough was prepared at 46.1% hydration (total water/dough w/w) by mixing flour with distilled water to maximum consistency in a 2-g Mixograph (88 rpm) at 20°C.

Rheometers

Two rotational controlled stress rheometers were used with cone-plate geometries: AR 2000 (TA Instruments) with a 2-cm-diameter and 4°-angle cone, and SR 2000 (Rheometrics) with a 2.5-cm-diameter and 5.7°-angle cone. A few experiments were performed with the first instrument using a 4-cm, 4° cone. The AR 2000 rheometer works with a very quick feedback loop allowing satisfactory performance of stress relaxation tests.

All experiments were done at 20°C. Immediately after mixing, dough was transferred to the measuring system of the rheometer. After the gap was set, the samples were covered with fluid silicon oil to avoid drying, and they were left to rest for 1 h before starting the rheological tests. Dough has limited microbial and biochemical stability; moreover, it exhibits long-lasting viscoelastic memory effects, thixotropy and shear-induced irreversible changes, as will be shown later. Therefore, a new dough preparation was used for each rheological experiment. Reproducibility of dough preparation can be evaluated from the data of the initial frequency sweep that was systematically performed in every test sequence (see below); the values of G′ and G″, measured at 0.95 rad/s with the AR2000 rheometer on 60 different dough preparations, displayed a coefficient of variation of 15%.

Rheological experiments: small-amplitude oscillations and transient regime tests

The standard rheological experiment (AR 2000 instrument) consisted in a frequency sweep followed by a transient regime test. The frequency sweep was performed at 0.1% strain amplitude over the 0.06–100 rad/s frequency range. As will be discussed later, at this strain amplitude the viscoelastic behaviour of dough in dynamic measurements is practically linear. The transient test was either a retardation test (creep and creep recovery), or a stress relaxation test. In the first case, creep stress σ _c was varied in the 0.3–350 Pa range, and was applied during a time S=3 h unless otherwise stated, whereas creep recovery was recorded during 12 h. In the second case, strain values γ _o, in the range 10⁻³≤γ _o≤10, were applied and kept constant for 20 h; the time t _o necessary to reach the fixed γ _o value was ∼10⁻² s.

Rheological experiments: large-amplitude oscillations

Large-amplitude oscillation experiments (LAOS) were performed with the SR 2000 rheometer at two frequency values: 0.01 and 0.1 rad/s. The sample was submitted to a series of sinusoidal stress cycles and the resulting strain was recorded; the amplitude σ _o of the stress was varied in the 5–450 Pa range. Preliminary test chaining up to 18 cycles having shown that the strain signal stabilises after eight to nine cycles, a sequence of nine cycles was routinely applied and the stress and strain signals of the ninth cycle were analysed. The number of sampling points was 128 per cycle.

In response to the stress

$$\sigma {\left( t \right)} = \sigma _{{\text{o}}} \cos {\left( {ω _{x} t + ϕ _{\sigma } } \right)}$$

(1)

the strain will be in the linear domain of the viscoelastic behaviour:

$$\gamma {\left( t \right)} = \gamma _{{\text{o}}} \cos {\left( {ω _{x} t + ϕ _{\gamma } } \right)}$$

(2a)

But, beyond the linearity limit, the strain signal will contain higher harmonics and it becomes:

$$\gamma {\left( t \right)} = {\sum\limits_{k = 1}^K {\gamma _{{{\text{o,}}\,k}} \cos {\left( {kω _{x} t + ϕ _{{\gamma ,\,k}} } \right)}} }$$

(2b)

In the Fourier series (Eq. 2b), γ _{o, k} and ϕ _{γ, k} represent the amplitude and the phase of the kth harmonic, respectively, and depend on σ _o. For isotropic liquids, normally, only odd harmonics should be present, so that k=1,3,5,…K; all the lost work is in the first harmonic (Giacomin and Dealy 1993). The components of the complex modulus relative to the kth harmonic are then (Heymann et al. 2002):

$$G\prime _{k} {\left( {ω _{x} ,\sigma _{{\text{o}}} } \right)} = \frac{{\sigma _{{\text{o}}} }} {{\gamma _{{{\text{o,}}\,k}} }}\cos {\left( {\delta _{k} } \right)}$$

(3a)

$$G\prime \prime _{k} {\left( {\omega _{x} ,\sigma _{{\text{o}}} } \right)} = \frac{{\sigma _{{\text{o}}} }}{{\gamma _{{{\text{o,}}\,k}} }}\sin {\left( {\delta _{k} } \right)}$$

(3b)

$$\delta _{k} = ϕ _{{\gamma ,\,k}} - kϕ _{\sigma } $$

(3c)

In our experimental range of stress amplitude, it was not necessary to consider harmonics beyond k=5 to fit the strain signal.

Steady state behaviour of dough

An advantage of the creep test in the study of viscoelastic liquids is that, in principle, it allows straightforward separation of the non-recoverable contribution to the total strain, due to flow, from the recoverable one, which corresponds to the reversible viscoelastic response, provided the test is carried on during a time S long enough for the steady state to be reached. When steady state is reached, the strain γ(t) and the compliance J(t) become linear functions of time. From the slope of these functions the steady state shear rate ${\mathop \gamma \limits^. }$ and viscosity η are obtained; extrapolation to t=0 of the linear terminal zone of the compliance gives the steady state compliance ${\left( {{\text{J}}^{{\text{o}}}_{e} } \right)}_{{\text{c}}} ,$ which is a measure of the elastic deformation during steady flow. The contribution of flow to creep compliance is t/η, and the (visco)elastic contribution is given by the creep recoverable compliance $J_{{\text{c}}} {\left( t \right)} = J{\left( t \right)} - \frac{t}{\eta }$ J _c(t) is a monotonously increasing function of time which reaches the plateau value ${\left( {{\text{J}}^{{\text{o}}}_{{\text{e}}} } \right)}_{{\text{c}}}$ when the terminal region is entered. This very simple analysis of creep curves, classical for linear viscoelastic behaviour (Ferry 1980), remains valid in the case of non-linear behaviour, provided that the non-Newtonian viscosity does not depend on time (i.e., does not vary during the test), but is a function of stress or shear rate only.

Flow behaviour

A few examples of dough creep curves, obtained for S=3 h and σ _c values in the middle and the upper part of the explored range of creep stress are given in Fig. 1a. The recoverable compliances $J_{{\text{r}}} {\left( t \right)} = J{\left( S \right)} - J{\left( t \right)}{\left( {t > S} \right)}$ obtained from the corresponding recovery curves are plotted against t−S in Fig. 1b. Figure 1a shows that steady state flow seems to be reached after t>∼4,000 s, t>∼9,000 s and t>∼10,000 s creep for σ _c=128 Pa, 200 Pa and 280 Pa, respectively. However, whereas for σ _c=128 Pa the creep curve has the “normal” shape, with a slope that decreases steadily until the linear terminal region is reached, the curves obtained for 200 Pa and 280 Pa show an upturn before the apparent steady state region is attained (Fig. 1a). Such a shape, while the corresponding curves of J _r(t−S) keep to the usual form (Fig. 1b), results from the onset of time-dependent viscosity when σ _c≥∼200 Pa. This is demonstrated by increasing time S. For creep stress values below ∼200 Pa, the viscosity obtained from the apparent linear terminal region of the creep curves does not depend on S (if we allow for experimental uncertainties), as shown in Fig. 2 for σ _c=5 Pa and σ _c=128 Pa; true steady state regime is thus reached when S=3 h. For σ _c=200 Pa, the apparent viscosity decreases very slightly when S is increased from 3 to 5 h, and at higher creep stress values its decrease becomes spectacular (Fig. 2). Time-dependent flow behaviour starts apparently when strain reaches a value ∼5 during creep.

Fig. 1

A few examples of CNS dough creep (a) and creep recovery (b) curves, showing the onset of time-dependent flow behaviour when σ _c≥∼200 Pa. The dotted lines in a represent the linear fit of the terminal region of the curves. See text for experimental details. In b, time scale starts from the beginning of recovery

Fig. 2

Effect of creep duration S on the apparent steady state shear viscosity of CNS dough determined from the slope of the terminal part of the creep curves

We have not studied in more details the time-dependence of dough viscosity. Let us, nevertheless, suppose that, when creep has been carried out for long enough time S, steady state flow is eventually reached or approached whatever the stress, and let us then plot creep viscosity η against creep stress (Fig. 3a) or against the value of the shear rate in the terminal region of the creep curves (Fig. 3b).

Fig. 3

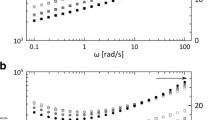
Steady state flow curves of CNS dough determined from creep results. a Steady state shear viscosity plotted against creep stress. 1 onset of non-Newtonian behaviour; 2 onset of time-dependent behaviour; 3 Newtonian viscosity determined from stress relaxation data in the linear domain [Eq. 5; see text]. b Steady state viscosity plotted against shear rate. The continuous line represents the fit of Eq. 4. 3 as shown in a

The η(σ _c) curve can be divided into three regions (Fig. 3a): A plateau extends up to σ _c∼40 Pa, with η≈6.6×10⁶ Pa s. In the range ∼40<σ _c<∼200 Pa, η decreases mildly as stress increases; we have not observed time-dependence in this region. At higher stress values, in the range where we observed viscosity to be time-dependent, the decrease of viscosity becomes very steep and η drops eventually to values of the order of 10³ Pa·s. The transition from the second to the third region of the η(σ _c) curve is very abrupt, reflecting probably some shear-induced structural change in the system, to which the onset of time-dependent flow is related.

On the contrary, η shows a smooth variation with the shear rate, of the classical shear-thinning type (Fig. 3b). One observes an initial viscosity plateau extending up to ${\mathop \gamma \limits^. }$≈6·10⁻⁶ s⁻¹, followed by a gradual decrease of η and then by a power law region. Actually, the curve can be fitted accurately with the simplified Cross equation:

$$\eta = \frac{{\eta _{{\text{o}}} }} {{{\left[ {1 + {\left( {{{\mathop \gamma \limits^. }} \mathord{\left/ {\vphantom {{{\mathop \gamma \limits^. }} {{\mathop \gamma \limits^. }_{{\text{c}}} }}} \right. \kern-\nulldelimiterspace} {{\mathop \gamma \limits^. }_{{\text{c}}} }} \right)}^{n} } \right]}}}$$

(4)

The fit gives, for the initial Newtonian viscosity plateau, η _o=6.4·10⁶ Pa·s, for the critical shear rate, ${\mathop \gamma \limits^. }_{{\text{c}}}$=2.9·10⁻⁵ s⁻¹, and for the Cross exponent, n=0.93. The value of the exponent is very high, but it is significantly less than one.In Fig. 3a,b are shown for comparison the Newtonian viscosity values of dough obtained from the stress relaxation modulus function G(t) in the linear range of deformation (stress relaxation results will be examined later), using the classical relation (Ferry 1980):

$$\eta _{{\text{o}}} = {\int_{ - \infty }^{ + \infty } {tG{\left( t \right)}{\text{d}}\ln {\left( t \right)}} }$$

(5)

Considering the experimental scatter and the truncation approximation in the numerical integration of Eq. 5, the agreement between the results obtained from creep and from relaxation is fairly good. We can thus consider the initial viscosity plateau in Fig. 3a,b as a true Newtonian plateau. The existence of an actual linear domain of dough shear behaviour will be confirmed hereafter.

Creep test is better suited than the classical viscosimetric test for the study of the viscosity of viscoelastic materials displaying very high viscosities, extremely low shear rate limits of the Newtonian plateau and requiring very long time for steady-state flow to be established. Moreover, we observed that, in step shear rate experiments, dough samples very soon fractured or rolled out the rheometer gap when the shear rate exceeded 6.10⁻² s⁻¹, whereas no steady state response could be reached at lower shear rates. These experimental problems, already reported in the literature (Bagley et al. 1998; Phan-Thien et al. 1997), make it impossible to determine directly the flow curve of dough. Different authors (for example Phan-Thien et al. 1997; Dus and Kokini 1990) have published shear flow curves $\eta {\left( {{\mathop \gamma \limits^. }} \right)}$ obtained by viscosimetric tests, but they were not necessarily obtained in steady-state flow conditions. Besides, only the power law region is visible within the shear-rate range covered. The magnitudes of the different rheological characteristics of dough are susceptible to depend actually on flour composition, on the nature of gluten proteins and on dough hydration to a relatively large extent. The effect of the first two of these factors on dough viscosity is illustrated by the results of the creep experiments of Edwards et al. (2001). The tests were carried on for a time long enough (10⁴ s) for the steady state to be at least approached; the Newtonian viscosities of doughs from five common wheat varieties ranged between 3.5×10⁶ and 7.6×10⁶ Pa s, encompassing the value we found for CNS flour dough. Thus, the range of variation of η _o for doughs prepared from different flours seems to be rather narrow. Such comparisons of rheological data obtained on different wheat cultivars are often obscured by the fact that dough hydration is adjusted to the “Farinograph optimum water absorption”, meaning that doughs were prepared at different moisture contents adjusted to get similar empirical consistency values. In a previous study (Lefebvre et al. 2004) we found that η _o of doughs, prepared at the same hydration from flours of four experimental wheat lines differing in glutenin subunits compositions, varied in the 3.1×10⁶–7.1×10⁶ Pa s range only. The retardation test was also used by Pedersen et al. (2004) to compare biscuit doughs from different wheat cultivars; however, creep and recovery times were extremely short (300 s); besides, biscuit doughs are very different and much more complex than bread doughs.The time-dependent character of dough flow behaviour has been generally overlooked. Time dependent flow behaviour of dough could be observed on Bloksma’s creep curves (Bloksma 1962) that showed an inflection with upward curvature when σ _c∼100 Pa. Berland and Launay (1995) addressed specifically the shear softening and thixotropic properties of dough. However, this was through the study, at a fixed frequency, of the recovery of G′, measured in the linear domain, after dynamic shearing at different strain amplitudes beyond the linearity limit. Thus, the effect of shear on flow viscosity was not separated from its effect on the elastic contribution; when considering the frequency used, the latter was certainly dominant. They observed that shear softening became irreversible above ∼15% strain amplitude. Long before, Hibberd and Wallace (1966) and Smith et al. (1970) followed a similar experimental procedure to very briefly examine dough thixptropic behaviour.

Steady state compliance

Normally, the steady state compliance ${\left( {J^{{\text{o}}}_{e} } \right)}_{{\text{c}}}$ can be readily determined from creep results, but this is obviously no longer possible when flow behaviour becomes time dependent. On the other hand, for σ _c≥200 Pa, we still observe a plateau compliance ${\left( {J^{{\text{o}}}_{e} } \right)}_{{\text{r}}}$ of the recoverable compliance in creep recovery at long recovery times (Fig. 1b). Whereas ${\left( {J^{{\text{o}}}_{e} } \right)}_{{\text{c}}} = {\left( {J^{{\text{o}}}_{e} } \right)}_{{\text{r}}}$ in the linear domain, the equality does not hold when the behaviour becomes non-linear. For want of a better solution, we have plotted in Fig. 4 ${\left( {J^{{\text{o}}}_{e} } \right)}_{{\text{r}}}$ against σ _c, together with ${\left( {J^{{\text{o}}}_{e} } \right)}_{{\text{c}}}$ when the latter was obtainable.

Fig. 4

Dependence on creep stress of the steady state shear compliance of CNS dough, determined from creep and from creep recovery results. 1 mean $J^{{\text{o}}}_{e}$ value determined from stress relaxation data in the linear domain [Eq. 6; see text]. Dotted lines are guides for the eyes

Within experimental uncertainty, ${\left( {J^{{\text{o}}}_{e} } \right)}_{{\text{c}}} = {\left( {J^{{\text{o}}}_{e} } \right)}_{{\text{r}}}$ and remains constant up to σ _c∼60 Pa (Fig. 4); moreover (Fig. 4), the value of this initial plateau is in good agreement with the value of the steady state compliance calculated from stress relaxation data in the linear domain according to the following equation (Ferry 1980):

$$J^{{\text{o}}}_{e} = \frac{1} {{\eta ^{2}_{{\text{o}}} }}{\int_{ - \infty }^{ + \infty } {t^{2} \,G{\left( t \right)}{\text{d}}\ln {\left( t \right)}} }$$

(6)

In the range 60 Pa<σ _c<200 Pa, non-linearity shows up: ${\left( {J^{{\text{o}}}_{e} } \right)}_{{\text{c}}}$ increases with σ _c and seems to differ now significantly, although slightly, from ${\left( {J^{o}_{e} } \right)}_{r}$ However, departure from linearity remains limited in this creep stress range. For σ _c≥200 Pa, ${\left( {J^{{\text{o}}}_{e} } \right)}_{{\text{c}}}$ cannot be determined, but we see that ${\left( {J^{{\text{o}}}_{e} } \right)}_{{\text{r}}}$ tends to decrease as the stress increases; the amplitude of this variation remains rather small up to the highest creep stress we applied in our experiments.

Reliable literature data on the elastic compliance of dough in general, and on the stress dependence of $J^{{\text{o}}}_{e}$ in particular, are indeed extremely scarce. The results of Smith and Tschoegl (1970) were obtained in simple tension for a 45.8% hydrated dough; the behaviour seemed to be linear over the extremely narrow stress range explored (184–328 Pa). They obtained a steady state tensile compliance of 2.7×10⁻⁴ m²/N; this would correspond to $J^{{\text{o}}}_{e} = 8.1 \cdot 10^{{ - 4}} {{\rm\text{m}}^{2} } \mathord{\left/ {\vphantom {{{\text{m}}^{2} } {\text{N}}}} \right. \kern-\nulldelimiterspace} {\rm\text{N}}$ in shear, a value lower than our $J^{{\text{o}}}_{e}$ values in the linear domain (1.1−1.7×10⁻³ m²/N). However, the steady shear viscosity calculated from their data would be ∼4.10⁹ Pa s, a value that seems unrealistically high, indicating that steady state was probably not reached in their experiments. In the work of Hibberd and Parker (1979), creep duration was, by far, too short (S=250 s), so that the flow-dominated region was not even approached. From the results of Edwards et al. (2001), $J^{{\text{o}}}_{e}$ values ca. 2×10⁻³ m²/N² in the linear domain can be estimated for their common wheat doughs at 42–46% hydration. The linear steady state compliances we obtained previously (Lefebvre et al. 2004) for the doughs of four experimental wheat lines studied at the same hydration ranged between 1.3×10⁻³ and 2.7×10⁻³ m²/N.

The transient viscoelastic response of dough in retardation tests

For low values of the creep stress, the curves of the two recoverable compliance functions J _c(t) and J _r(t−S) are actually superimposed over the complete time range, confirming that a linear domain of dough viscoelasticity does exist. An example (σ _c=8 Pa) is given in Fig. 5a. This superposition is lost at larger creep stress values; the linearity limit in stress of the viscoelastic response seems thus located between 8 and 16 Pa, corresponding to a maximum creep strain comprised between 3 and 6%, and to a creep terminal strain rate comprised between 1.6×10⁻⁶ and 3.3×10⁻⁶ s⁻¹. While this shear rate linearity limit seems in agreement with that observed for the steady state viscosity (Fig. 3b), the stress linearity limit is substantially lower than that which could be deduced from the ${\left( {J^{{\text{o}}}_{e} } \right)}_{{\text{c}}} {\text{vs }}\kern1pt\sigma _{{\text{c}}}$ curve (Fig. 4). The reason is that, in the beginning of the non-linear region, J _r(t−S)>J _c(t), but the two recoverable compliance functions get closer at large time values (Fig. 5b).

Fig. 5

Comparison of the creep compliance J(t) function, the recoverable compliance function $J_{{\text{c}}} {\left( t \right)} = J{\left( t \right)} - \frac{t} {\eta }$ calculated from creep data and the recoverable compliance function J _r(t−S) measured in creep recovery for CNS dough. a Creep stress 8 Pa; b creep stress 64 Pa

Whether the viscoelastic response is linear or not, it is always possible to fit J _r with a generalised Kelvin–Voigt model:

$$J_{{\text{r}}} {\left( t \right)} = {\sum\limits_{i = 1}^N {J_{i} } }{\left[ {1 - \exp {\left( {{ - t} \mathord{\left/ {\vphantom {{ - t} {\tau _{i} }}} \right. \kern-\nulldelimiterspace} {\tau _{i} }} \right)}} \right]}$$

(7)

We have fitted Eq. 7 to the recoverable compliance data obtained in creep recovery, using Kaschta’s method (Kaschta and Schwarzl 1994) with N=8 (two spectral lines per logarithmic decade of time). In the linear domain, the ordinary storage and loss compliance functions are obtained from the discrete retardation spectrum [J _i , τ _i ] as (Ferry 1980):

$$J\prime {\left( ω \right)} = {\sum\limits_{i = 1}^N {\frac{{J_{i} }} {{1 + {\left( {ω τ _{i} } \right)}^{2} }}} }$$

(8a)

$$J″{\left( ω \right)} = \frac{1} {{ω \eta _{{\text{o}}} }} + {\sum\limits_{i = 1}^N {\frac{{J_{i} {\left( {ω τ _{i} } \right)}}} {{1 + {\left( {ω τ _{i} } \right)}^{2} }}} }$$

(8b)

where ω is the angular frequency. The storage [G′(ω)] and loss [G″(ω)] moduli are readily calculated from J′(ω) and J″(ω). As shown in Fig. 6 for σ _c=4 Pa, G′(ω) and G″(ω) obtained from J _r(t), measured during recovery after creep under low stress values, match satisfactorily the low strain amplitude dynamic data recorded before the retardation test. This confirms the existence of a linear domain of dough rheological behaviour. The composite mechanical spectrum of Fig. 6 demonstrates that the linear viscoelasticity plateau of CNS dough is remarkably wide because it extends down to ∼3×10⁻⁴ rad/s, whereas its upper frequency limit is located above 100 rad/s, beyond the experimental range. The linear viscoelasticity composite mechanical spectrum of dough will be discussed in more detail later.

Fig. 6

Linear mechanical spectrum of CNS dough combining dynamic measurements (symbols) with the data obtained by converting creep recovery results (lines) (see text). Recovery followed application of 4 Pa creep stress during 3 h. Steady state Newtonian viscosity was calculated from the slope of the terminal region of the creep curve. Filled symbols and continuous line: G′. Empty symbols and dotted line: G″

In Eq. 7 we have omitted the glassy compliance term (“instantaneous compliance”). Transient oscillations, due to the coupling of the intertia of the moving part of the stress-controlled rheometer with the viscoelasticity of the sample, are visible at the beginning of the creep and of the recovery curves, so that the compliance data recorded on dough at times shorter than at least 0.1 s are to be discarded. Therefore, the glassy zone of the behaviour is totally out of reach of the measurements, and the “instantaneous compliance” values sometimes reported (Edwards et al. 2001) are illusory.

The transient viscoelastic response of dough in stress relaxation tests

Figure 7 gives a few examples of the stress relaxation curves of dough, obtained for different values of the imposed strain γ _o. The exploitable time range encompasses visibly the viscoelastic plateau region and the beginning of the terminal region of the stress relaxation modulus G(t, γ _o). The curves have similar shapes, but, indeed, they cannot be superimposed by shifting. Thus, dough relaxation does not exhibit time–strain factorability.

Fig. 7

Examples of CNS dough stress relaxation curves obtained at different values of shear strain γ _o: 0.002 (a), 0.02 (b), 0.2 (c) and 2 (d)

Let us consider the isochronous stress relaxation modulus curves G(γ _o, t _x ) as a function of γ _o for different values t _x of the relaxation time, a few examples of which are shown in Fig. 8. An equation proposed by Vinogradov and Malkin (1980), with a theoretical basis but used here in an empirical way, fits satisfactorily the data for γ _o<6 (Fig. 8):

$$G{\left( {t,\,\gamma _{{\text{o}}} } \right)} = \frac{{G{\left( t \right)}_{{{\text\rm {lin}}}} }} {{1 + {\left( {{2\gamma _{{\text{o}}} } \mathord{\left/ {\vphantom {{2\gamma _{{\text{o}}} } {t_{{\text{o}}} ^{{1/2}} }}} \right. \kern-\nulldelimiterspace} {t_{{\text{o}}} ^{{1/2}} }} \right)}^{a} }} \approx \frac{{G{\left( t \right)}_{{{\text\rm {lin}}}} }} {{1 + {\left( {20\gamma _{{\text{o}}} } \right)}^{a} }}$$

(9)

Fig. 8

Isochronous stress modulus curves: dependence of the stress relaxation modulus on strain at t=1, 100, 1,000 and 10,030 s (from top to bottom). The lines represent the fit of Eq. 9 for γ _o<6

In Eq. 9, G(t)_lin is the linear relaxation modulus and the exponent a is an empirical parameter which measures the deviation from the linear behaviour. The variations with relaxation time t of both parameters are represented in Fig. 9. One observes good agreement between G(t)_lin on one hand, and G(t) recorded in the experiments with the lowest values of γ _o on the other hand. The variation of exponent a with time is bell-shaped, with a maximum located at t∼25 s.

Fig. 9

Variation with the relaxation time of the parameters G _lin (a) and a (b) of Eq. 9. The filled symbols represent the values of the parameters. In a, the relaxation modulus recorded at γ _o=4×10⁻³ has been plotted for comparison. In b, the dotted line is a guide for the eyes

Equation 9 gives the linear behaviour as the asymptotic limit of the modulus for γ _o→0. However, if we allow for the experimental error, G(t) curves are practically identical for γ _o≤6×10⁻³; it is impossible to decide whether there is a definite strain limit for linear behaviour in stress relaxation, or whether linearity is only asymptotically reached.

The relaxation modulus function G(t) can be fitted by a generalised Maxwell model:

$$G{\left( t \right)} = {\sum\limits_{i = 1}^{N\prime } {G_{i} \exp {\left( {{ - t} \mathord{\left/ {\vphantom {{ - t} {\lambda _{i} }}} \right. \kern-\nulldelimiterspace} {\lambda _{i} }} \right)}} }$$

(10)

The fit was done using the same method as for J _r(t), with N′=9 logarithmically equidistant relaxation times λ _i (two per decade). Considering that linear behaviour is approached, if not exactly verified, when γ _o≤6×10⁻³, G′(ω) and G″(ω) are readily obtained from the discrete relaxation spectrum [G _i , λ _i ] determined at low strain values (Ferry 1980). In the region where the two frequency domains overlap, the results are not in bad agreement with the data obtained directly from dynamic measurements previously to the stress relaxation test (Fig. 10), although the match is less good than that obtained when the recoverable compliance function was converted (Fig. 6). Moreover, the composite mechanical spectra of Figs. 6 and 10 are very close each other, demonstrating the consistency of the results in the linear domain.

Fig. 10

Linear mechanical spectrum of CNS dough combining dynamic measurements (symbols) with the data obtained by converting stress relaxation results recorded at 0.002 strain value (lines) (see text). Filled symbols and continuous line: G′. Empty symbols and interrupted line: G″

In Fig. 8, the points relative to γ _o>6 lie visibly somewhat aside the curves described by Eq. 9. This departure results probably from a drastic structural change in dough related to the onset of flow time-dependence discussed above and to the existence of a critical shear strain in the 5–10 range, repeatedly pointed out by step shear rate experiments (Keentok et al. 2002; Phan-Thien et al. 1997; Amemiya and Menjivar 1992; Larsson et al. 2000) and that we observed around γ∼10 (results not shown).

Studies of the effect of strain on stress relaxation of dough are very few. Safar-Ardi and Phan-Thien (1998) carried out stress relaxation experiments in the 10⁻³−0.3 strain range, but the scope of these experiments was just to show, by visual comparison of relaxation curves, that differences between flours types, quite small at low strain, were amplified at larger strain values. Bohlin and Carlson (1981) studied dough relaxation in the 0.1−1 strain range, which already starts far from the linearity limit; non-linearity was quantified by the isochronous relaxation modulus vs γ _o curve for t=0.3 s; the results were very similar to ours in the same strain range. They observed time–strain separability up to γ _o=0.4. Time–strain separability was alleged by Ramkumar et al. (1996) and frequency–strain amplitude separability was also assumed by Hibberd and Parker (1975a) in their study of dough dynamic viscoelastic behaviour. This is not necessarily in opposition with our negative conclusion on this point. The reason for the apparent contradiction is certainly that these authors considered only very restricted ranges of time (frequency) and/or strain.

The response of dough to harmonic excitation

Small-amplitude oscillations: the problem of linearity

The validity of classical “dynamic” measurements is limited to the linear viscoelasticity domain. By common consent, the linearity limit for doughs in the dynamic mode, within the practical frequency range (grossly, 0.01–100 rad/s), corresponds to strain amplitudes close to 0.1%. This order of magnitude of the linearity limit, determined as the strain amplitude above which the proportionality of the stress amplitude or the constancy of G′ and G″ are lost when the sample is submitted to strain or stress amplitude ramps at a fixed frequency, is amply documented. However, old but very careful studies on the amplitude dependence of the storage and loss moduli (Smith et al. 1970; Hibberd and Parker 1975a; Navickis et al. 1982) indicate that dough behaves non-linearly even at the lowest strain amplitudes used (from ∼2×10⁻⁴ to ∼2.5×10⁻³). More recently, Phan-Thien et al. (1997) gave a linearity limit around 10⁻³ strain amplitude; however, inspection of their results shows in fact that below this limit one observes a slight dependence of G′, of η′, and of the phase angle δ on strain amplitude. We observed, at ω=1 rad/s (results not shown), clear departure from linear behaviour from the lowest strain amplitude we used (γ _o∼2×10⁻³). The reason is perhaps that the strain (or stress) amplitude sweep procedure does not insure that steady–steady state conditions are established in the sample (Smith et al. 1970), especially at the beginning of the test. On the other hand, at the lowest strain amplitude we used the shear rate amplitude ${\mathop \gamma \limits^. }_{{\text{o}}} = ω \gamma _{{\text{o}}}$ is already 2×10⁻³ s⁻¹, far beyond the limit of the Newtonian plateau of the flow curve (Fig. 3b). Therefore, although fairly small in the usual frequency range, the contribution of non-Newtonian viscosity (which affects both G′ and G″) could be responsible for dough non-linear behaviour in harmonic regime at low strain amplitudes. Nevertheless, departure from linearity remains negligible in practice up to γ _o∼10⁻³ (Hibberd and Parker 1975a).

Large-amplitude oscillations

Lissajous plots (Fig. 11) illustrate that the range of stress amplitude we covered in LAOS experiments, starting from the linear domain, goes far into the non-linear domain of dough viscoelastic behaviour. To allow the results to be plotted on the same scales, the stress and the strain have been normalised by their maximal values σ _o and γ _max, respectively. Whereas for σ _o<∼50 Pa, the cycles are actually ellipses and are practically superimposed, showing that up to this stress amplitude, the behaviour is linear at the frequency of the experiments, larger stress amplitudes result in an increasing departure from the elliptical shape, soon becoming very striking, and the cycles do not superimpose any longer. Phan-Thien et al. (2000), operating at ∼6 rad/s and at lower strain amplitudes, observed still larger distortions from the elliptical shape at lower strain amplitudes; however, they used plate–plate geometry, in which the sample is not uniformly sheared.

Fig. 11

Response of CNS dough to LAOS. Normalised Lissajous plots at ω=0.01 rad/s; shear stress amplitudes: 16 Pa (a), 30 Pa (b), 100 Pa (c), 200 Pa (d) and 300 Pa (e). The resulting strain maxima were 0.0087, 0.02, 0.43, 2.85 and 12.3, respectively

The simple harmonic analysis procedure outlined in the “Material and methods” section allows a quantitative analysis of LAOS results. Figure 12 shows the variation of the amplitudes of the first, third and fifth harmonics of the strain signal as a function of stress amplitude for ω=0.1 rad/s (similar results were obtained at ω=0.01 rad/s). At low stress amplitudes, higher harmonics are not measurable; γ ₃ becomes measurable when σ _o>∼50 Pa (γ ₁>∼0.03), and γ ₅ when σ _o reaches 100 Pa (γ ₁≈0.13); but their contributions remain modest, not exceeding ∼8 and ∼2%, respectively, of the maximum strain. The stress amplitude threshold values for non-linear behaviour in harmonic excitation are consistent with the stress limits of the linear domain we observed for steady state behaviour.

Fig. 12

Response of CNS dough to LAOS (ω=0.1 rad/s). Dependence of the amplitude of the strain harmonic overtones on the shear stress amplitude

Two interesting facts emerge from Fig. 12:

• 1. In the low stress amplitude range 30≤σ _o≤60 Pa (corresponding to 10⁻³≤γ ₁≤0.032), albeit the response signal does not differ significantly from the sinusoid (absence of measurable higher harmonics contribution), γ ₁ does not increase linearly with σ _o but according to a power law (exponent 1.34). There is therefore discordance between the two criteria for linear behaviour in dynamic mode. The fact was already noticed by Smith et al. (1970) and by Hibberd and Parker (1975b); they reported that the response signal remained sinusoidal up to ∼4×10⁻³ (Smith et al. 1970) and even to considerably larger strain amplitudes (Hibberd and Parker 1975b) (we converted to strain amplitudes the peak-to-peak displacement values given these papers), whereas G′ and G″ decreased definitely as strain amplitude increased in this range. However, in both papers, the signals were not analysed. Hibberd and Parker (1975b) put forward that this curious behaviour results from dough thixotropic properties. Indeed, as already pointed out, in dynamic measurements, even at very small strain amplitudes, shear rates are relatively high.

• 2. At the highest stress amplitudes studied, γ ₃ and γ ₅ tend to level off, so that both γ ₃/γ ₁ and γ ₅/γ ₁ exhibit a maximum in the σ _o=200–300 Pa region, corresponding to ∼1 strain amplitude. Such maxima have been recently observed in LAOS studies on concentrated non-colloidal monodisperse suspensions (Heymann et al. 2002) and on suspensions of fibrillar assemblies of ovalbumin (Veerman et al. 2005). In the second case, the authors attributed the decrease of γ ₃/γ ₁ that they observed above 0.25 strain to shear-induced breakup of protein clusters. Such an explanation does not seem relevant in the first case, but the authors did not give a structural interpretation of the existence of γ ₃/γ ₁ and γ ₅/γ ₁ maxima.

Let us now take into account the information contained in the phases of the stress and of the strain signals, which is readily obtained by harmonic analysis but not by usual fast Fourier transform procedures. The storage (G′₁) and loss (G″₁) moduli, relative to the first harmonic at the different stress amplitudes studied, can be calculated using Eqs. 3a, 3b and 3c. Figure 13 shows the results for ω=0.1 rad/s. We see that above σ _o∼50 Pa, G′₁ decreases more steeply than G″₁ as σ _o increases and becomes eventually smaller. It is interesting to notice that the crossover occurs at about σ _o=250 Pa, i.e. at about the same stress amplitude as the maximum of γ ₃/γ ₁; Heymann et al. (2002) made the same observation on their system.

Fig. 13

Comparison of CNS dough non-linear behaviour in LAOS and in stress relaxation tests. The storage, G′₁ (filled circles), and loss, G″₁ (empty circles), moduli relative to the first harmonic (fundamental) in LAOS at ω=0.1 rad/s are plotted against the stress amplitude. The pseudo storage and loss moduli, Γ′₁ (filled triangles) and Γ″₁ (empty triangles), at ω=0.1 rad/s are obtained from the discrete relaxation spectra determined from stress relaxation data at different strain values, and they are plotted against the stress at relaxation time t=10 s (see text)

In Fig. 13, we have also plotted, against the stress measured after 10 s relaxation, the “pseudo moduli” $\Gamma \prime {\left( {\omega ,\,\sigma _{{\text{o}}} } \right)} = {\sum\limits_1^9 {G_{i} } }\frac{{\omega ^{2} \lambda _{i} ^{2} }}{{{\left( {1 + \omega ^{2} \lambda _{i} ^{2} } \right)}}}$and $\Gamma \prime{\prime}{\left( {ω ,\,\sigma _{{\text{o}}} } \right)0} = {\sum\limits_1^9 {G_{i} } }\frac{{ω \lambda _{i} }} {{{\left( {1 + ω ^{2} \lambda _{i} ^{2} } \right)}}}$ calculated with ω=0.1 rad/s using the (G _i, λ _i ) data obtained by fitting Eq. 10 to the relaxation curves recorded at the different γ _o values. The values of the “pseudo moduli” match those of G′₁ and G″₁ remarkably well, except at stress values larger than 200–250 Pa. The discrepancy observed at high stress values, i.e. in conditions where flow behaviour becomes strongly time-dependent, is probably due to the very different mechanical histories undergone by dough in LAOS and in stress relaxation experiments.

General discussion and concluding comments

We have shown that by combining the different classical rheological tests it is possible to get a self-consistent set of data which amounts to a general description of the rheological behaviour of dough in shear over large time, stress and strain ranges. Although the work we report here has been performed on only one commercial flour, we think that the results are representative of the broad lines of dough rheological behaviour, at least in the usual ranges of protein and water contents. Indeed, as discussed above, they converge, even quantitatively, with the piecemeal literature data published on doughs prepared in different conditions with flours from variable origins.

The complexity of dough rheological behaviour is due principally to the viscous component. Non-Newtonian behaviour begins at extremely low shear rates. Besides, viscosity becomes time-dependent when total strain exceeds some threshold value in the 5–10 range, related to a major structural change in the system. Nevertheless, both the vicous component and the elastic component of dough viscoelasticity do exhibit a linear domain, as shown clearly by the results of the retardation test and their agreement with stress relaxation data at low strain amplitude. Because, even at the lowest strain amplitudes studied, the sample is submitted to comparatively large shear rate values during part of its mechanical history, a true linear domain is not observable in dynamic measurements. Our results do not give any evidence for the existence of a yield stress, in opposition with the interpretation that Smith et al. (1970) gave of their dynamic measurements results, but in agreement with the conclusion of Hibberd and Parker (1975a, 1979). In more recent papers, the question of the possible existence of a yield stress is not examined as such, but the rheological model of Phan-Thien et al. (1997, 2000) contains an equilibrium relaxation modulus term; the authors consider dough as a solid and the gluten matrix as a permanent crosslinked network. Such a view, which the same research group seems to have implicitly abandoned later (Uthayakumaran et al. 2002), is not tenable. The results reported here do show that dough has a liquid-like behaviour, in agreement with the conclusion of Smith and Tschoegl (1970). We found it to be the same for gluten (Lefebvre et al. 2003).

The linearity limit of gluten in the dynamic regime is generally found to be ca. 10% strain amplitude (Bohlin and Carlson 1981; Lefebvre et al. 2003; Wang and Kokini 1995; Khatkar et al. 1995), although smaller values have been reported occasionally (Uthayakumaran et al. 2002). The presence of starch granules in dough is certainly responsible for the low linearity limit of dough as compared to gluten, as indeed expected if we consider starch granules as playing the role of an inert filler in the viscoelastic gluten matrix of dough. At first analysis, this is in agreement with the increase in storage modulus (Uthayakumaran et al. 2002; Smith et al. 1970; Larsson et al. 2000; Hibberd 1970; Khatkar and Schofield 2002) and in relaxation modulus at short times (Uthayakumaran et al. 2002; Matsumoto 1979) observed when starch content was increased in “synthetic doughs” prepared from gluten and starch mixtures or by adding starch or gluten to a “natural” flour. Several authors have credited starch with an “active” participation in dough viscoelasticity to explain their intricate results on synthetic flours containing different proportions of gluten, starch and water. For example, Hibberd (1970) and Petrofsky and Hoseney (1995) put forward protein/starch interactions. Some authors suggested that starch granules, at least in some conditions, form a three-dimensional structure in dough, which would be responsible for dough thixotropy in addition to its highly non-linear behaviour (Smith and Tschoegl 1970; Smith et al. 1970), or even altogether for its viscoelasticity below 300% shear strain (Amemiya and Menjivar 1992). It is really impossible to follow these authors. Wheat starch granules (5–40 μm) are not colloidal particles; they are not subject to Brownian motion and they cannot flocculate or build particle gels through colloidal interactions. In the case of large (and, besides, non-charged) particles such as starch granules, hydrodynamic interactions dominate by far over particle–particle forces and their magnitude increases with the viscosity of the matrix.

Actually, the synthetic dough approach, besides being limited by the fact that the range of compositions of the gluten/starch/water system yielding a material comparable to dough is extremely limited, leads to results the interpretation of which is far from being straightforward. In practice it is impossible to change the content of the system in one of the constituents without changing the contents in the others. In this respect, a very difficult problem is that of free water; dough contains an amount of water in excess over what is needed to hydrate gluten and starch. The importance of free water, and more generally that of the distribution of water between the phases in dough, as to the understanding of its rheology has, indeed, been stressed (Smith et al. 1970; Larsson et al. 2000), but the point is generally overlooked. Moreover, it has been recognised that gluten and starch blends are unable to duplicate the rheological properties of doughs. This was suggested to be the consequence of the importance of the minor components of flour in determining these properties (Uthayakumaran et al. 2002). Indeed, we have shown recently (Rouillé et al. 2005) that removing the water-extractable constituents results, in particular, in a large increase of the Newtonian viscosity of dough together with an important shift of limit of the Newtonian plateau in the low-shear-rates direction. On the other hand, the comparison of “natural” doughs with different compositions to shed some light on the question of starch contribution is inevitably obfuscated by differences in gluten protein “quality”.

Surprisingly, to our knowledge, the rheological behaviour of doughs has been rarely compared to that of the glutens extracted from them. At short relaxation times the relaxation modulus (Uthayakumaran et al. 2002; Bohlin and Carlson 1981) or the storage modulus (Georgopoulos et al. 2004) of dough was reported to be larger than that of gluten, but the difference decreased as strain increased (Uthayakumaran et al. 2002; Bohlin and Carlson 1981). In addition, this difference seems to decrease as relaxation time increases in the 0.1–1,000 s range (Uthayakumaran et al. 2002), an indication that the presence of starch granules affects fast relaxation processes to a greater extent than slow relaxation processes, and moreover, the difference reduces as dough hydration increases (Georgopoulos et al. 2004).

To get a better insight into the effect of starch granules at different time scales, we have compared the linear viscoelastic behaviour of CNS dough to that of the corresponding gluten on the basis of the results of the retardation test and of the dynamic measurements. Gluten was studied using a similar procedure to that followed for dough, except that strain amplitude was 1% in dynamic measurements, and that the stress (5 Pa) was applied during 10 h and creep recovery was monitored during 30 h. In Fig. 14, the composite mechanical spectra of gluten and of dough are plotted in terms of the storage compliance J′(ω) and of the recoverable loss compliance J″_r(ω)=J″(ω)−1/η _oω, so that the comparison between dough and gluten is not obscured by the contribution of flow, which differs considerably because η _o is much larger in the case of gluten, as shown later. For ω<10⁻³ rad/s, the mechanical spectra are very close to each other. But when ω>10⁻² rad/s, J′(ω) and J″_r(ω) become larger for gluten than for dough, meaning that dough is more viscoelastic than gluten at the small time scale (t<100 s)—in agreement with literature results (Uthayakumaran et al. 2002; Georgopoulos et al. 2004). However, the difference observed is actually not so large. Moreover, both mechanical spectra display a bimodal shape (much more clearly in the case of gluten), with the distributions of the fast and the slow retardation processes located more or less in the same frequency regions. We have not studied the non-linear viscoelastic behaviour of CNS gluten. Nevertheless, we have shown previously (Lefebrve et al. 2003) that gluten was extremely shear thinning and displayed time-dependent viscosity above a certain creep stress value, whereas the increase of the steady-state compliance with stress was very limited. Thus, the linear and the non-linear rheological properties of gluten and of dough display qualitatively the same general features in shear, and the differences seem to be quantitatively rather limited in the linear viscoelastic domain.

Fig. 14

Comparison of CNS dough linear viscoelastic behaviour (interrupted lines and triangles) with that of its gluten (thin lines and circles). The storage compliance J′(ω) (thick lines and filled symbols) and the recoverable loss compliance $J_{{\text{r}}} {\left( \omega \right)} = J{\left( \omega \right)} - \frac{1} {{\omega \eta _{{\text{o}}} }}$ (interrupted lines and empty symbols) were obtained by dynamic measurements (symbols) and by conversion of creep recovery data (lines) (see text)

The presence of a filler is expected to increase the viscosity of the system, the higher the volume fraction of the filler, and the effect would be larger for an “active” filler than for an inert one. However, we found that the Newtonian viscosity of CNS gluten was ∼10⁸ Pa s, more than ten times that of the dough. This is in line with the observation that in step shear rate experiments, as well as in elongation, glutens displayed larger viscosities than the corresponding doughs (Uthayakumaran et al. 2002). Starch seems to act on viscosity as a diluent rather than as a filler, in the opposite direction of its effect on viscoelasticity! Thus, the simple model of dough as a concentrated dispersion of starch granules within a gluten phase (Phan-Thien et al. 1997, 2000) is not relevant. The problem is probably connected with the status of water in dough and with the role of the minor components such as pentosans; questions, perhaps related, that we have already raised.