1 Introduction

The dynamical problem of magneto-thermoelasticity has received much attention in the literature during the past decade. In recent years the theory of magneto-thermo-elasticity which deals with the interactions among strain, temperature and electromagnetic fields has drawn the attention of many researchers because of its extensive uses in diverse field, such as geophysics for understanding the effect of the Earth's magnetic field on seismic waves, damping of acoustic waves in a magnetic field, emissions of electromagnetic radiations from nuclear devices, development of a highly sensitive superconducting magnetometer, electrical power engineering, optics etc. The thermal stress problem in a finite circular cylinder has attracted the attention of numerous investigators [1–3]. Stress functions method of plane stress thermoelastic problem in a multiply connected region of variable thickness, has been investigated by Sugano [4]. The rotation of non-homogeneous composite infinite cylinder was investigated by El-Nagaar, et al. [5]. Abd-Alla, et al. [6] have investigated the thermal stress in an infinite circular cylinder of orthotropic material. El-Naggar, et al. [7] studied the thermal stresses in a rotating non-homogeneous orthotropic hollow cylinder. Janele, et al. [8] studied the finite amplitude spherically symmetric wave propagation in a compressible hyperelastic solid. Knopoff [9] and Nowacki [10] adressed these types of problems at the begining. Kaliski [11] investigated the wave equations of thermo-electric-magneto-elasticity. Suhbi [12] studied magneto-thermo-viscoelastic interactions in a body having cylindrical geometry. Mukhopadhyay and Roychoudhurj [13] discussed magneto-thermo-elastic interactions in an infinite isotropic elastic cylinder subjected to a periodic loading.

In the present paper, we have investigated the generation of stress, temperature and magnetic field in an infinite isotropic elastic cylinder placed in a constant primary magnetic field. The governing equations for the non-homogeneous in an isotropic elastic solid are obtained in conservation form. These equations are solved using a numerical method which uses relation from the characteristics theory of finite difference scheme. This scheme is easier to implement than the method of characteristic discussed by Haddow and Mioduchowski [14, 15]. Numerical results are presented for the variation of temperature, displacement and stresses with the time t and through the thickness of the cylinder. The effects of inhomogeneity and the magnetic field are very pronounced.

2 Formulation of the problem

Let us consider an infinite isotropic elastic solid cylinder with internal radius a and external radius b. (r, θ, z) are taken as the cylindrical coordinates with z-axis as the axis of the cylinder. The cylinder is placed in a constant primary magnetic field H O , acting in the direction of the z-axis. Assuming the medium to be non-ferromagnetic and ferroelectric and ignoring the Thompson effect, the simplified Maxwell's equations of electro-dynamics for perfectly conducting elastic medium are:

$$\nabla \times \vec h = \vec j,$$
(2.1a)
$$\nabla \times \vec E = - \mu {{\partial \vec h} \over {\partial t^{\prime}}},$$
(2.1b)
$$\nabla \cdot \vec h = 0$$
(2.1c)
$$\nabla \cdot \vec E = 0$$
(2.1d)
$$\vec E = - \mu \left({{{\partial \vec u} \over {\partial t^{\prime}}} \times \vec H} \right)$$
(2.1e)
$$\vec h = \nabla \times (\vec u \times \vec H),$$
(2.1f)

where \({\vec H}\) is the magnetic field, \({\vec E}\) is the electric field, \({\vec j}\) is the current density, \({\vec u}\) is the mechanical displacement, and \({\vec h}\) is the perturbed magnetic,

$$\vec H = \overrightarrow {H_o} + \vec h.$$

The corresponding equations for the adjoining free space are

$$\nabla \times \overrightarrow {h^o} = \varepsilon ^o {{\partial \overrightarrow {E^o}} \over {\partial t^{\prime}}},$$
(2.2a)
$$\nabla \times \overrightarrow {E^o} = - \mu ^o {{\partial \overrightarrow {h^o}} \over {\partial t^{\prime}}},$$
(2.2b)
$$\nabla \cdot \overrightarrow {h^o } = 0$$
(2.2c)
$$\nabla \cdot \overrightarrow {E^o } = 0$$
(2.2d)

where superscript o refers to values for the free space. The stress equations of motion in the absence of body forces are:

$$\sigma _{ij,j} + \tau _{ij,j} = \rho \ddot u_i ,$$
(2.3)

where Maxwell's electro-magnetic stress tensor τ ij is given by

$$\tau _{ij} = \mu (h_i H_j + h_j H_i - h_k H_k \delta _{ij})$$
(2.4)

and the mechanical stress tensor σ ij is given by

$$\sigma _{ij} = \lambda \Delta \delta _{ij} + 2\mu^{\prime}e_{ij} - \gamma T^{\prime}\delta _{ij} ,$$
(2.5)
$$e_{ij} = {1 \over 2}(u_{i,j} + u_{j,i}),$$
$$ \matrix{ {e_{ii} = \Delta ,} \hfill & {i,j = 1,2,3} \hfill \cr } . $$

Considering radial vibrations of the medium, the only non-zero displacement is u r = u(r, t′), so that

$$e_{rr} = {{\partial u} \over {\partial r}},\quad e_{\theta \theta } = {u \over r},\quad e_{zz} = 0.$$
(2.6)

The field components in the medium and in the contacting free space are then obtained from equations (2.1), (2.2) and (2.4) as:

$$\vec E = - \mu \left[{0, - H_o {{\partial u} \over {\partial t^\prime}},0} \right],$$
(2.7a)
$$\vec j = \left[{0, - {{\partial h_z } \over {\partial r}},0} \right],$$
(2.7b)
$$\vec h = \left[{0,0, - H_o \left({{{\partial u} \over {\partial r}} + {u \over r}} \right)} \right],$$
(2.7c)
$$\overrightarrow {E^o } = \left[{0,E_2^o ,0} \right],$$
(2.7d)
$$\overrightarrow {h^o } = \left[{0,0,h_z^o } \right].$$
(2.7e)

The stress equations of motion (2.3) then reduce to

$${{\partial \sigma _{rr} } \over {\partial r}} + {1 \over r}(\sigma _{rr} - \sigma _{\theta \theta }) + {{\partial \tau _{rr} } \over {\partial r}} = \rho {{\partial ^2 u} \over {\partial t^{\prime 2}}}$$
(2.8)

where

$$\sigma _{rr} = \lambda \left({{{\partial u} \over {\partial r}} + {u \over r}} \right) + 2\mu^{\prime}{{\partial u} \over {\partial r}} - \gamma T^{\prime},$$
(2.9a)
$$\sigma _{\theta \theta } = \lambda \left({{{\partial u} \over {\partial r}} + {u \over r}} \right) + 2\mu^{\prime}{u \over r} - \gamma T^{\prime},$$
(2.9b)
$$\tau _{rr} = \mu H_o^2 \left({{{\partial u} \over {\partial r}} + {u \over r}} \right),$$
(2.9c)

where u is the component of displacement in the radial direction, e ij are the strain components, T′ is the absolute temperature, λ and μ′ are Lame's constants, γ = (3λ+ 2μ′)α t , α t is the coefficient of linear thermal expansian, μ is the magnetic permeability and t′ is the time. The heat conduction equation in the presence of heat sources can be written in the following form

$${{\partial ^2 T^{\prime}} \over {\partial r^2 }} + {1 \over r}{{\partial T^{\prime}} \over {\partial r}} - {1 \over {k_1 }}{{\partial T^{\prime}} \over {\partial t^{\prime}}} = - {Q \over {k_2 }},$$
(2.10)

where k 1 and k 2 are the thermal diffusivity and thermal conductivity respectively, Q is the intensity of applied heat source.

The elastic constants λ and μ′, magnetic permeability μ and density ρ are taken as a power functions of the radial coordinate.

We characterize the non-homogeneity of the material by

$$\lambda = Lr^{2m} \quad \mu ^{\prime} = v^{\prime}r^{2m} \quad \mu = vr^{2m} \quad \rho = \rho _o r^{2m} ,$$
(2.11)

where L, v′, v and ρ o are constants (the values of λ, μ′, μ and ρ in homogeneous matter) and m is a rational number. Substituting from equations (2.11) into equations (2.9) we obtain the stress-displacement relations are

$$\sigma _{rr} = r^{2m} \left[{L\left({{{\partial u} \over {\partial r}} + {u \over r}} \right) + 2v^{\prime}\left({{{\partial u} \over {\partial r}}} \right) - \gamma ^{\prime}T^{\prime}} \right],$$
(2.12a)
$$\sigma _{\theta \theta } = r^{2m} \left[{L\left({{{\partial u} \over {\partial r}} + {u \over r}} \right) + 2v^{\prime}\left({{u \over r}} \right) - \gamma^{\prime}T^{\prime}} \right],$$
(2.12b)
$$\tau _{rr} = r^{2m} vH_o^2 \left({{{\partial u} \over {\partial r}} + {u \over r}} \right)$$
(2.12c)

where γ′ = (3L + 2v′)

Using (2.12) we have from (2.8) the displacement formulation of the equation of motion;

$${{\partial ^2 u} \over {\partial r^2 }} + {{\alpha _1 } \over r}{{\partial u} \over {\partial r}} - \alpha _2 {u \over {r^2 }} - {{\gamma^{\prime}} \over \eta }\left({{{2m} \over r}T^{\prime} + {{\partial T^{\prime}} \over {\partial r}}} \right) = {{\rho _o } \over \eta }{{\partial ^2 u} \over {\partial t^{\prime 2} }}.$$
(2.13)

It is convenient to introduce the following non-dimensionalization scheme

$$b(U,R) = (u,r),$$
$$t^{\prime} = {b \over v}t,$$
$$T = {{T^\prime} \over {T_o }}.$$
(2.14)

where T o is a reference temperature and v is the dimensionless velocity. In terms of these non-dimensional variables, equations (2.10) and (2.13) can be rewritten in more convenient form as.

$${{\partial ^2 T} \over {\partial R^2 }} + {1 \over R}{{\partial T} \over {\partial R}} + \alpha {Q \over {k_2 }} = \alpha ^\prime{{\partial T} \over {\partial t}},$$
(2.15)
$${{\partial ^2 U} \over {\partial R^2 }} + {{\alpha _1 } \over R}{{\partial U} \over {\partial R}} - \alpha _2 {U \over {R^2 }} - \alpha _3 \left({{{2m} \over R}T + {{\partial T} \over {\partial R}}} \right) = \alpha _4 {{\partial ^2 U} \over {\partial t^2 }}.$$
(2.16)

where

$$\alpha = {{b^2 } \over {T_o }},\quad \alpha ^{\prime} = {{vb} \over {k_1 }},\quad \alpha _1 = 2m + 1,\quad \alpha _2 = 1 - {{2m(L + vH_o^2)} \over \eta },$$
$$\alpha _3 = {{\gamma^{\prime}T_o } \over \eta },\quad \alpha _4 = {{\rho _o v^2 } \over \eta },\quad \eta = L + 2v^{\prime} + vH_o^2 .$$

The stress components induced by the temperature T are related to displacement component U by

$$\sigma _{RR} = (bR)^{2m} \left[{L\left({{{\partial U} \over {\partial R}} + {U \over R}} \right) + 2v^\prime\left({{{\partial U} \over {\partial R}}} \right) - \gamma ^\prime T_o T} \right],$$
(2.17)
$$\sigma _{\theta \theta } = (bR)^{2m} \left[{L\left({{{\partial U} \over {\partial R}} + {U \over R}} \right) + 2v^\prime\left({{U \over R}} \right) - \gamma ^\prime T_o T} \right],$$
(2.18)

Assume that the intensity of the applied heat source is taken to be in the following form

$$Q = {{\varepsilon t\exp (- \beta R)} \over R},$$
(2.19)

where β being a nonnegative constant, t is the time and ε a constant.

From preceding description, the initial condition may be expressed as

$$at\ t = 0\quad T = 0,\quad U = {{\partial U} \over {\partial t}} = 0.$$
(2.20)

The boundary condition may be expressed as

$$at\ R = {a \over b}\quad T = 0,\quad U = 0,$$
(2.21a)
$$at\ R = 1\quad {{\partial T} \over {\partial R}} = 0,\quad U = 0$$
(2.21b)

3 Numerical Scheme

A finite difference scheme which is a modification of MacCormack's scheme is described by wachtman, et al. [16]. Where it is used to obtain solutions to problem of thermal stress emanating from cylindrical cavity in a bounded medium. This scheme is a forwared-backwared predictor corrector scheme. We take the finite difference grids with spatial intervals h in the direction R and k as the time step, and use the subscripts i and n to denote the ith discrete points in the R direction and the nth discrete time. A mesh is defined by

$$ \matrix{ {R_i = a_o + ih,} \hfill & {i = 0,1,2,3, \ldots ,j - 1} \hfill \cr } $$
$$t^n = kn,\quad n = 0,1,2,3, \ldots ,k\ being\ the\ time\ step,$$

where \(a_o = {a \over b}\) .

The functions T(R,t), U(R,t) and Q(R,t) may be at any nodal location

$$T(R_i ,t^n) = T_i^n ,$$
$$U(R_i ,t^n) = U_i^n $$
$$Q(R_i ,t_i^n) = Q_i^n .$$

Thus the heat conduction equation (2.15) may be expressed in the finite difference as follows:

$$\eqalign{T_i^{n + 1} = T_i^n + {\rho \over a}&\left[T_{i + 1}^n - 2T_i^n + T_{i - 1}^n + \left({h \over {{a \over b} + h}} \right)\left({T_{i + 1}^n - T_i^n } \right) \right. \cr & \quad \left. + {{\delta kn} \over {{a \over b} + h}}\exp \left({- \beta \left({{a \over b} + h} \right)} \right) \right] } $$
(3.1)

Also, the equation of motion (2.16) may be expressed in the finite difference as follows

$$\eqalign{U_i^{n + 1} = 2U_i^n - U_i^{n - 1} + {{\rho _1 } \over {\alpha _4 }} & \left[{U_{i + 1}^n - 2U_i^n + U_{i - 1}^n + {{\alpha _1 h\left({U_{i + 1}^n - U_i^n } \right)} \over {{a \over b} + ih}}} \right. \cr & \left. {\quad - \alpha _2 \left({{h \over {{a \over b} + ih}}} \right)^2 U_i^n - \alpha _3 h\left({T_{i + 1}^n - T_i^n + {{2mh} \over {{a \over b} + ih}}T_i^n } \right)} \right] } $$
(3.2)

where

$$\rho = {k \over {h^2 }},\quad \delta = {{h^2 \alpha \varepsilon } \over {k_2 }}\quad and\quad \rho _1 = \left({{k \over h}} \right)^2 $$

4 Numerical results and discussion

For computational work, we take cooper as the example, for which the material constants at T o = 27°C are as follows:

$$\eqalign{& L = 1.387 \times 10^{12}\,dyne/cm^2 ,\quad v^\prime = 0.448 \times 10^{12} \,dyne/cm^2 , \cr & \varepsilon = 0.25,\quad \beta = 0.12 \cr & \rho = 8.93\,g/cm^3 ,\quad k_1 = 1.14\,cm^2 /s,\quad k_2 = 0.918\,cal/s^\circ C\,cm } $$
$$H_o = 1000.000\,Oersted,\quad \alpha _t = 1.67 \times 10^{- 8} /^\circ C,\quad v = 0.5\,gauss/Oersted $$
(4.1)

Results are presented for cylinder with

$$a = 0.1,\quad b = 1.0.$$

To study the non-homogeneous case, we assume that m = 0.5 and for the homogeneous case we assume that m=0.0. We represented the numerical results graphically.

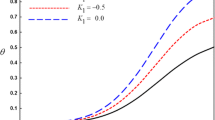
Figure 1 shows the temperature variation for various non-dimensional time t. It is noticed that the temperature increases with the increasing of R in all the contexts of all three modes and satisfied the boundary conditions.

Fig. 1.
figure 1

Temperature distribution at different times

Full size image

Figures 2 and 3 show the radial displacement along the radial direction R at various dimensionless t. From these figures the radial displacement U decreases and it starts to increase at the value R=0.4 for the non-homogeneous case and homogeneous case. It is noticed that the displacement component decreases with the increase of t under the effect of the magnetic field.

Fig. 2.
figure 2

Radial displacement distribution (m=0.5, ν=0.5)

Full size image
Fig. 3.
figure 3

Radial displacement distribution (m=0, ν=0.5)

Full size image

Figures 4, 5, 6 and 7 show the radial stress δ RR and tangential stress δθθ along the radial direction R at various times t. Also, they show the influence of the non-homogeneity of the material constants and the magnetic field on the stresses δ RR and δθθ. It will be observed from those graphs that the radial stress δ RR and tangential stresses δθθ decrease with the increase of R for the non homogeneous case and homogeneous case. It is noticed that they decrease with the increase of t.

Fig. 4.
figure 4

Radial stress distribution (m=0, ν=0.5)

Full size image
Fig. 5.
figure 5

Radial stress distribution (m=0, ν=0.5)

Full size image
Fig. 6.
figure 6

Tangential stress distribution (m=0.5, ν=0.5)

Full size image
Fig. 7.
figure 7

Tangential stress distribution (m=0, ν=0.5)

Full size image

The variation of stresses and displacement δ RR , δθθ and U are due to the effect of inertia and magnetic field. Also, the influence of the non-homogeneity on displacement and stresses is very pronounced.

5 Conclusions

Some interesting conclusions can be drawn from the analysis presented here. The material is elastic and has an inhomogeneity in the direction perpendicular to the boundary for the cylinder. A finite difference predictor-corrector scheme using a relation from characteristic theory at the inner and outer radii is used to obtain solutions for the non-homogeneous infinite cylinder. Compared with the homogeneous case the inhomogeneities in which Lame's constants increase and decrease with the distance measured from the boundary have respectively amplifying and attenuating effects on both the stress and displacement. The results are specific for the example considered, but other examples may have different trends because of the dependence of the results on the mechanical and thermal constants of the material.