Keywords

Introduction

While rightly deflected after the Indian Ocean tsunami of 2004, the focus of tsunami researchers in the last decade has been largely on understanding the waves generated by submarine and subaerial landslides. Despite this effort, the wave-generating potential of a landslide and its associated risk to the coastline are difficult to assess with precision; the obvious reason for this deficiency is the complexity of the process. The deadly tsunami of Papua New Guinea (PNG) spurred much of this recent work, and has greatly assisted researchers in their understanding of the waves created by underwater landslides. Studies into the source of PNG have been widespread (e.g., Sweet et al. 1999; Tappin et al. 1999, 2001; Geist 2000; Heinrich et al. 2000; Synolakis et al. 2002). Hydrodynamic investigations of PNG are also numerous, with some looking at the differences between landslide and seismic dislocation (e.g., Satake and Tanioka 2003), while others focus on the physical properties of the PNG waves (e.g., Lynett et al. 2003). This paper will provide a review of recent work on landslide tsunamis, broadly divided into the categories of generation, open ocean propagation, and inundation.

Tsunami Generation by Landslide

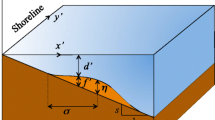
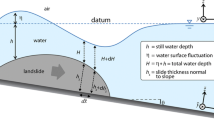
The properties of the disturbed free water surface above the landslide — the near-field condition — are a function of the time history of the landslide, which is then a function of typically unknown, or very difficult to quantify a-priori, properties of the seabed. The simplest description of a landslide source is solid body motion, where it is assumed that the slide or slump progresses down a constant slope as a single coherent mass. This approach has been employed by Watts (2000) both experimentally and with an empirical, curve-fitting approach applied to derive a characteristic tsunami amplitude. Numerically, solid body motion has been used within high-order accurate numerical models (e.g., Grilli and Watts 1999; Lynett and Liu 2002), in order to develop a more precise relationship between the slide and the tsunami.

From geological evidence, it is clear that large landslides degenerate into or exist primarily as debris flows and turbidity currents. One of the initial numerical modeling attempts at debris flow and tsunami generation/interaction was that of Jiang and LeBlond (1992, 1993, 1994). In this series of papers, both viscous and Bingham flow models were presented for submarine slides. Recent applications of the Jiang and LeBlond model have been widespread (e.g., Fine et al. 2001; Bornhold et al. 2003), and investigations have indicated that the choice of model, whether solid, viscous, or Bingham, has O(1) effects on the generated tsunami height (Fine et al. 2003). An example of how the slide properties affect the generated wave is shown in Fig. 1. In the top row of this figure, termed case A, a dense, viscous fluid flows into a body of water, driven only by the force of gravity. The viscous fluid develops a half-arrowhead type shape upon entry as it interacts with the initially still water, and pushes a large pulse of water offshore. In the lower plot of this figure, case B, an identical initial slide volume is used, but a thin top layer of less dense fluid is placed on the dense fluid. As the slide interacts with the water, naturally these two dense fluid layers behave differently, as well as interact with each other. Interestingly, the front of the lower density fluid of case B moves as a faster rate as compared to Case A, but the generated wave is 15% less in height. This example helps to elucidate that precise modeling of the wave generation by geophysical events is very difficult.

Fig. 1
figure 1_55

In the top row are shown three spatial snapshots of a single fluid flowing into water. In the second row are snapshots, taken at the same time, of a two-fluid mass flowing into water. The two-fluid mass is meant to very roughly represent a turbidity current above a debris flow

Full size image

Nearly all of the applications of these mudflow models to wave generation take a shallow water approach. Unless the slide is in extremely shallow water, essentially subaerial, it is known that the nonlinear shallow water wave (NLSW) equations do not correctly capture the interaction between sliding mass and wave generation (Lynett and Liu 2002). This aspect becomes particularly troubling as one inspects the evolution of a mudflow downslope. The formation of a bore-type front face occurs (e.g., Jiang and LeBlond 1994), which has a relatively large slope. For these cases, the NLSW will greatly over-predict the wave height, due to the fact that generation of surface waves by a traveling steep-sided body is an intermediate to deep water problem (Lynett and Liu 2002).

Open Ocean Propagation of Landslide Tsunami

Landslide tsunamis, especially those created by large slides, can be characterized as nonlinear waves (wave height is a non-negligible fraction of the water depth) in the near field, either linear or nonlinear during open ocean propagation, and then usually nonlinear in the nearshore. Additionally, landslide waves, depending on the horizontal length scale of the slide, can often be described as dispersive, meaning that the various important frequency components that compose the waveform travel at different speeds. This effect will cause the wave energy to spread lengthwise in the direction of propagation, and can cause the maximum energy flux reaching the shoreline to diminish. Due to this relative complexity of a landslide wave, it can be difficult to model correctly.

There are two main classes of governing equations employed to predict the evolution of water waves and the associated fluid flow: depth-integrated and fully 3D. Depth-integrated equations are those which, typically by way of an assumption of the length scale ratio, can be vertically integrated to yield an explicit equation form. The net effect of this integration is to remove the vertical dimension from the equations, leaving a 2D equation where once was a 3D equation. Elimination of the vertical dimension yields an exponential reduction in the required computational time to solve, and thus allows one to simulate phenomenon over very large spatial scales.

Focusing on tsunami research, the depth-integrated category has historically included only NLSW models. Earthquake generated tsunamis, with their very long wavelengths, are ideally matched with NLSW for transoceanic propagation. Models such as Titov and Synolakis (1995) and Liu et al. (1995) have been shown to be accurate throughout the lifeline of a seismic tsunami, and are in widespread use today. However, when examining the tsunamis generated by submarine mass failures, the NLSW can lead to errors in the wave shape and arrival time of the wave (Lynett et al. 2003). The length scale of a submarine failure tends to be much less that that of an earthquake, and thus the wavelength of the created tsunami is shorter. To correctly simulate the shorter wave phenomenon, one needs equations with excellent shallow to intermediate water properties, such as the Boussinesq equations. While the Boussinesq model too has accuracy limitations on how deep (or short) the landslide can be (Lynett and Liu 2002), it is able to simulate the majority of tsunami generating landslides.

Shallow Water Evolution

As the tsunami approaches the beach and turbulence effects may become important, depth-integrated models are able to provide only a coarse prediction. NLSW and Boussinesq models are by definition invicsid, and so require special treatment to capture the physical dissipation processes, such as breaking and bottom friction. Examples are the numerical dissipation in NLSW models (e.g., Liu et al. 1995) or the ad-hoc breaking models utilized in Boussinesq models (e.g., Kennedy et al. 2000). Additionally, wave interaction with complex, spatially variable structures is difficult, due to the mild slope assumptions inherent in long wave models. In order to simulate nearshore dissipation and complex wave-structure interaction with confidence, the use of a fully 3D model is required. These models tend to require substantial computer processing time, and their use for large-scale tsunami problems is currently limited.

An interesting physical phenomenon that occurs to some long waves in shallow water, and are especially relevant to landslide generated waves, is fission. Long wave fission is most commonly discussed in the literature via a solitary wave propagating over an abrupt change in depth, such as a step (e.g., Madsen and Mei 1969; Johson 1972; Seabra-Santos et al. 1987; Losada et al. 1989; Goring and Raichlen 1992; Liu and Cheng 2001). In these cases, there is a deep water segment of the seafloor profile, where a solitary wave initially exists. In this depth, the solitary wave is of permanent form. As the solitary wave passes over the change in depth, into shallower water, the leading wave energy will try to re-discover a balance between nonlinearity and dispersion; the solitary wave. Since this new solitary wave will be a different shape and contain a lower level of mass, by conservation there must be some trailing disturbance to account for the deficient. This trailing disturbance will take the form of a rank-ordered train of solitons. Note, however, that discussion of fission in this sense is not particularly relevant to “real” tsunami modeling, where the offshore wave approaching the shelf break rarely resembles a solitary wave solution (Tadepalli and Synolakis 1996). However, the offshore wave does not need to specifically be a solitary wave for this process to occur.

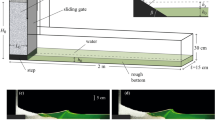
In numerous eyewitness accounts and videos recorded of the 2004 Indian Ocean tsunami, there is evidence of the tsunami approaching the coastline as a series of short period (on the order of 1 min and less) breaking fronts, or bores (e.g., Ioualalen et al. 2007). These short period waves may be the result of fission processes of a steep tsunami front propagating across a wide shelf of shallow depth. Along the steep front of a very long period wave, nonlinearity will be very important. There will be a large amount of energy in high-frequency components with wavelengths similar the horizontal length of the tsunami front (on the order of 1 km). As the wave continues to shoal, the high-frequency locked waves may eventually become free waves, and will take the form of very short waves “riding” the main wave pulse. This situation is akin to an undular bore in a moving reference frame. The newly freed waves, in the nonlinear and shallow environment, will attempt to reach an equilibrium state, where frequency dispersion and nonlinearity are balanced. Thus, the fission waves will appear as solitary waves, or more generally, cnoidal waves. This fact provides some guidance as to the wavelength of these fission waves; they can be approximately calculated via solitary wave theory using the tsunami height and depth of the shelf. For example, on a shelf with depth of 30 m and an incident tsunami height of 5 m, fission waves with a wavelength of approximately 240 m and period of 13 s would be generated. In recent work looking at landslide tsunamis along the eastern U.S.A coast, where there exists a wide shallow shelf, this fission process has been investigated (Geist et al. 2009). Figure 2 gives a few numerical simulation snapshots, and shows where the fission occurs, and the eventually impact on the waveform. This simulation, run with the dispersive equations, generated fission waves with lengths in the range of 100–200 m, and required a grid size of 5 m to attain numerically convergent results. In this example, the steep fission waves break offshore, and have little impact on the maximum runup. It is noted that landslide waves may be more likely to exhibit these fission features, due to the fact that the nonlinearity of a landslide wave may be large, even as it first approaches a shelf break.

Fig. 2
figure 2_55

Example of tsunami fission. Simulation results are from Geist et al (2009) for a landslide-generated tsunami off the east coast of the U.S.A. The top plot shows the beach profile and six free surface profiles at different times. The lower subplots are zoom-in's of those six profiles, with the times given in the individual plot titles. The red marks visible in the lowest plots indicate regions where the wave is breaking

Full size image

Inundation

In order to simulate the flooding of dry land by a tsunami, a numerical model must be capable of allowing the shoreline to move in time. Here, the shoreline is defined as the spatial location where the solid bottom transitions from submerged to dry, and is a function of the two horizontal spatial coordinates and time. Numerical models generally require some type of special consideration and treatment to accurately include these moving boundaries; the logic and implementation behind this treatment is called a moving shoreline, or runup, algorithm.

For typical tsunami propagation models, it is possible to divide runup algorithms into two main approaches: those on a fixed grid and those on a Lagrangian or transformed domain. Note that there is no explicit difference between modeling the inundation of a seismic tsunami or a landslide tsunami, although the differences in period between the two phenomena can lead to preferences of one runup scheme over another. Both runup algorithm approaches have their advantages and disadvantages; currently fixed grid methods are found more commonly in operational-level models (e.g., Titov and Synolakis 1998), likely due in large part to their conceptual simplicity. A review of these two classes of models will be given in this section, followed by a review of the standard analytical, experimental, and field benchmarks used to validate the runup models. For additional information, the reader is directed to the comprehensive review given in Pedersen (2006).

With a fixed grid method, the spatial locations of the numerical grid points or control volumes are determined at the start of a simulation, and do not change shape or location throughout the simulation duration. These methods can be classified into extrapolation, stair-step, auxiliary shoreline point, and permeable beach techniques. The extrapolation method has its roots in Sielecki and Wurtele (1970), with extensions by Hibberd and Peregrine (1979); Kowalik and Murty (1993); and Lynett et al. (2002). The basic idea behind this method is that the shoreline location can be extrapolated using the nearest wet points, such that its position is not required to be locked onto a fixed grid point; it can move freely to any location.

Stair-step moving shoreline methods, one of the more common approaches found in tsunami models (e.g., Liu et al. 1994), reconstruct the naturally continuous beach profile into a series of constant elevation segments connected through vertical transitions. In essence, across a single cell width, the bottom elevation is taken as the average value. A cell transitions from a dry cell to a wet cell when the water elevation in a neighboring cell exceeds the bottom elevation, and transitions from wet to dry when the local total water depth falls below some small threshold value. These methods are particularly useful in finite volume and C-grid (Arakawa and Lamb 1977) type approaches (e.g., Liu et al. 1995; LeVeque and George 2004), but can be difficult to implement in centered difference models, particularly high-order models or those sensitive to fluid discontinuities, where the “shock” of opening and closing entire cells can lead to numerical noise.

Auxiliary shoreline point methods require dynamic re-gridding very near the shoreline, such that the last wet point is always located immediately at the shoreline. Obviously, this method requires a numerical scheme that can readily accommodate non-uniform and changing node locations. There is some relation to the extrapolation methods discussed above; the moving shoreline point must be assigned some velocity, and it is extrapolated from the neighboring wet points. However, it is fundamentally different in that the shoreline point is explicitly included in the fluid domain. Thus, it would be expected that the governing conservation equations near the shoreline are more precisely satisfied here, although still dependent on the appropriateness of the extrapolation. One such method can be found in Titov and Synolakis (1995), and has been successfully applied in NSLW equation models.

Alternative to fixed grid methods is the Lagrangian approach. Here, the fluid domain is descritized into particles, or columns of fluid in depth-integrated models, that are transported following the total fluid derivative. There are no fixed spatial grid locations; the columns move freely in space and time and thus these techniques require numerical flexibility, in terms of utilizing constantly changing space and time steps. The Lagrangian approach can be described as both the more physically consistent and mathematical elegant method of describing shoreline motion. The shoreline “particle” is included in the physical formulation just as any other point in the domain (i.e., no extrapolations are necessary), and thus the shoreline position accuracy will be compromised only by the overarching physical approximation (e.g., long wave approximation) and the numerical solution scheme (e.g., second-order time integration). The cost for this accuracy is a mathematical system that can be more difficult and tedious to solve numerically, typically requiring domain transformations, mappings, and/or re-griddings. Lagrangian methods have been used successfully in finite difference and finite element nonlinear shallow water (NLSW) and Boussinesq equation models (e.g., Pedersen and Gjevik 1983; Gopalakrishnan and Tung 1983; Petera and Nasshei 1996; Zelt 1991; Ozkan-Haller and Kirby 1997; Birknes and Pedersen 2006).

Conclusions

As noted in a state-of-the-science paper looking at landslide tsunami hazards (Bardet et al. 2003), the physical understanding of this hazard is poor, and “there is an immediate need for research” such that we can be reasonably prepared for devastating events like PNG. This is, of course, no simple task. Extreme geophysical events are, by definition, complex and involve multi-scale processes, and landslide tsunamis are no exception. The waves are generated at a multi-phase rock-soil-water interface, where the various constituents can be moving at high speeds, with slip surfaces dictated by very local geotechnical properties. The resulting free surface waves can be both nonlinear and dispersive, implying that only a general wave model, without substantial approximation, can be used to properly model the propagation of the disturbance.