Keywords

These keywords were added by machine and not by the authors. This process is experimental and the keywords may be updated as the learning algorithm improves.

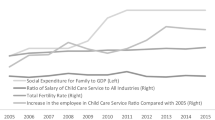
Population growth is one of factors to affect the economic growth. Such growth affects not only economic growth but also labour supply. Many developed countries face the population declining. For example, Japan is one of the most declining country where the number of population is estimated about 128 million at 2010 and will be estimated around 99 million at 2046. (National Institute of Population and Social Security Research of Japan (2013).)Footnote 1 Total fertility rate of Japan had been 3.5 at 1950 but was 1.43 at 2014 (Cabinet office of Japan) as in Fig. 12.1.Footnote 2 Both population and total fertility rate will make the demographic change and affect the labour supply, tax revenues and social security systems. Then, these changes indirectly influence economic growth more.

Fig. 12.1
figure 1

Total fertility rate (Source: Declining birthrate white paper of 2015 Japan (Cabinet office of Japan))

Full size image

According to Lapan and Enders [21] and Cabinet Office of Japan [6], the reasons to decrease the fertility are summarised as follows. First, the number of people who gets married later in life and not get married increase. Second, there are cost to raise children. Such costs include the opportunity cost for labour supply instead of caring for children.

Whether parents have the children or not depends on not only the utility from having children but also the raising cost including decreasing wage income (opportunity cost ) because the raising children makes parents work less. When such decreasing income is one of reason to decrease fertility, government provides public support for children to compensate for such opportunity cost. For example, we have public day care centre which public sector raise children while parents go to work. More public day care centre makes parents work more and gives them the incentive to have more children.

Zhang and Zhang [37], Wigger [34] and others analyse how public policy affects economic growth through fertility change in a model with parents’ raising time. Zhang and Zhang [37] examine the relationships between the incentive to have children and social security in the endogenous growth model and reveal that increasing tax for social security worsens economic growth. Similar to Zhang and Zhang [37], Wigger [34] examines public pension and fertility when children give gifts to parents at the retirement. However, in these models, public policy which decreases the opportunity cost to raising children is not discussed.

For such opportunity cost, government can give public subsidy for children to parents. Government compensates parents for the opportunity cost (decreasing wage income due to the raising). Apps and Rees [1] explain how taxation and public child support influence the inverse relationship between female labour supply and fertility. Groezen, Leers and Meijdam [17], Zhang and Zhang [40], and Hirazawa and Yakita [19] examine the relationships between public pension and public subsidy for children in a model with introducing the number of children into the utility function. However, these literatures do not take consideration that public supports compensate for the opportunity cost and do not discuss how such policy affect economic growth and fertility.

The purpose of first half of this chapter is to examine how allocation between public support for children and public subsidy for children affects fertility and economic growth in a model with the decision on having children and opportunity cost for raising children.Footnote 3 In the literature, public subsidy is provided by cash and public support is in-kind . There are discussions on cash versus in-kind (Blackorby and Donaldson [5], Munro [23], and Gahvari [12]). However, based on the income redistribution policy, they discussed cash versus in-kind. Blackorby and Donaldson [5] examine the Pareto efficiency between them when government has the imperfect information. Munro [23] and Gahvari [12] examine the effects of them on social security through labour-leisure choice. Furthermore, although these models are static framework and few discussion in the dynamic framework, our model is developed in the dynamic framework.

1 Public Support for Children and Public Subsidy for Children

Based on the overlapping generations model developed by Samuelson [30] and Diamond [9], we suppose that identical and perfect foresight consumers, firms and government are in the one good closed economy. For one period, there are three generations; the young generation, the working generation and the retirement generation. The government provides public support for children and public subsidy for children. Population of working generation at the initial period is normalised to one but the growth rate of population is determined endogenously.

1.1 Consumers

The consumers live for three period: the young period, the working period and the retirement period. At the young period, consumers are raised by the parents and do not do any decision makings. At the working period, they allocate one unit time between raising children and working. The wage income expends for consumption, saving and the wage income tax.Footnote 4 In this model, we assume away the bequest. At the retirement period, they do the consumption financed by saving and that interest.

We call the working generation at period t as generation t. The utility for representative consumer of generation t depends on the consumptions at the working period and the retirement period and the number of children.Footnote 5 We specialise the utility for representative consumer of generation t as

$$\displaystyle{ U^{t} = U\left (c_{ t}^{t},\ c_{ t+1}^{t},\ n_{ t+1}\right ) =\ln c_{t}^{t} +\beta \ln c_{ t+1}^{t} +\epsilon \ln \left (1 + n_{ t+1}\right ), }$$
(12.1)

where c t t and c t+1 t are consumption at the working period and the retirement period of generation t, respectively, and n t+1 is the number of children, β is the subjective discount factor, and ε is the parameter which shows the preference level to children. For the simplicity, we assume that parents get the utility from not n t+1 but \(1 + n_{t+1}\).Footnote 6

When the population of working generation at period t is shown by N t , population at period t + 1 is \(N_{t+1} = (1 + n_{t+1})N_{t}\).

We assume that the raising cost is equal to the raising time at period t, h t and h t depends on the number of children and public support for children. That is,

$$\displaystyle{h_{t} = h_{t}(n_{t+1},G_{p,t}),}$$

where G p, t is public support for children per generation t (parents of generation t + 1) at period t.Footnote 7

The budget constraint for the working period of generation t is

$$\displaystyle{ c_{t}^{t} + S_{ t} = \left (1-\tau \right )\left [1 - h_{t}(n_{t+1},G_{p,t})\right ]W_{t} + (1 + n_{t+1})G_{s,t}, }$$
(12.2)

and that of the retirement period of generation t is

$$\displaystyle{ c_{t+1}^{t} = \left (1 + r_{ t+1}\right )S_{t}, }$$
(12.3)

where S t is saving at period t, τ is the wage income tax rate, W t is the wage rate per time at period t, G s, t is the public subsidy for one child at period t. A representative agent of generation t chooses consumption at the working period, saving and the number of children to maximise his utility (12.1) subject to budget constraints (12.2) and (12.3). For simplicity, we suppose that the perfect foresight consumer knows the amount of public subsidy and he (she) does the decision making based on budget constraints including the amount.Footnote 8

1.2 Firms

Firms produce the output with physical capital and the efficiency labour .Footnote 9 The production technology is assumed by the constant return to scale and the aggregative production function at period t is shown by

$$\displaystyle{ Y _{t} = F(K_{t},A_{t}L_{t}) = A_{t}L_{t}f(k_{t}), }$$
(12.4)

where Y t , K t , L t are the aggregative output, the aggregative physical capital and the aggregative labour, respectively at period t.Footnote 10 When k t is the capital per efficiency unit at period t, \(k_{t} \equiv \frac{K_{t}} {A_{t}L_{t}}\).Footnote 11

Following Romer [29] and Grossman and Yanagawa [18], the labour productivity at period t, A t is

$$\displaystyle{ A_{t} = \frac{\frac{K_{t}} {L_{t}} } {a}, }$$
(12.5)

where a is the positive parameter.Footnote 12

The profit maximisation behaviour makes us show the factor prices as

$$\displaystyle{ r_{t} = f'(k_{t}), }$$
(12.6)

and

$$\displaystyle{ W_{t} = A_{t}\left [f(k_{t}) - k_{t}f'(k_{t})\right ]. }$$
(12.7)

Therefore, the wage rate per efficiency unit is

$$\displaystyle{ w_{t} \equiv \frac{W_{t}} {A_{t}}. }$$
(12.8)

1.3 Government

Government collects the wage income tax to finance public support for children and public subsidy for children . The government budget constraint per capita is

$$\displaystyle{ \tau \left [1 - h_{t}(n_{t+1},G_{p,t})\right ]W_{t} = (1 + n_{t+1})G_{s,t} + G_{p,t}. }$$
(12.9)

When Δ is the allocation rate to public support for children of total tax revenue at period t, public support for children is shown by

$$\displaystyle{ G_{p,t} =\varDelta \tau \left [1 - h_{t}(n_{t+1},G_{p,t})\right ]W_{t}, }$$
(12.10)

and public subsidy for children is

$$\displaystyle{ (1 + n_{t+1})G_{s,t} = (1-\varDelta )\tau \left [1 - h_{t}(n_{t+1},G_{p,t})\right ]W_{t}. }$$
(12.11)

Hereinafter, τ and Δ are assumed constant for simplicity.

1.4 Economic Growth Rate

Market Equilibrium

In this model, as we suppose that the agents supply labour to firms inelastically, when the capital market is cleared, the markets are in equilibrium. The equilibrium condition of capital market is

$$\displaystyle{ K_{t+1} = S_{t}N_{t}. }$$
(12.12)

The Number of Children

The technical reason makes us assume that the raising time depends on the number of children and the allocation rate between public support for children and public subsidy for children.Footnote 13 h t (n t+1, G p, t ) is rewritten by

$$\displaystyle{ h_{t}\left (n_{t+1},\varDelta \right ) =\eta \varDelta ^{-(1+\theta )}(1 + n_{ t+1}),\quad 0 <\eta < 1,\theta > 0. }$$
(12.13)

Based on these assumptions, from (12.13) and (12.11), the lifetime budget constraint for representative agents of generation t is shown by

$$\displaystyle{ \left (1-\varDelta \tau \right )\left [1 -\eta \varDelta ^{-(1+\theta )}(1 + n_{ t+1})\right ]W_{t} = c_{t}^{t} + \frac{c_{t+1}^{t}} {1 + r_{t+1}}. }$$
(12.14)

Therefore, the first order conditions show the number of children for representative consumer of generation t as

$$\displaystyle{ 1 + n_{t+1} = \frac{\epsilon } {(1 +\beta +\epsilon )\left (\eta \varDelta ^{-(1+\theta )}\right )}. }$$
(12.15)

The number of children, (12.15) depends on the allocation rate Δ and the constant parameters. As the identical consumers are supposed, n t+1 is the growth rate of population from generation t to generation t + 1. When the saving rate is s t , s t is shown by

$$\displaystyle{ s_{t} = \frac{S_{t}} {(1-\tau )\left [1 -\eta \varDelta ^{-(1+\theta )}(1 + n_{t+1})\right ]W_{t} + (1 + n_{t+1})G_{s,t}}. }$$
(12.16)

With (12.11) and the first order conditions of consumer’s optimal behaviour, the saving is

$$\displaystyle{S_{t} = \frac{(1-\tau \varDelta )\beta W_{t}} {1 +\beta +\epsilon } > 0,}$$

and s t is

$$\displaystyle{ s_{t} = \frac{\beta } {1+\beta }. }$$
(12.17)

Economic Growth Rate

We define the economic growth rate at the steady state as

$$\displaystyle{ 1 +\gamma ^{y} \equiv \frac{\frac{Y _{t+1}} {N_{t+1}} } { \frac{Y _{t}} {N_{t}}}. }$$
(12.18)

Using the labour productivity, (12.5), the wage rate per efficiency unit, (12.8), the equilibrium condition of capital market, (12.12) and the definition of saving rate, (12.17), the economic growth rate is rewritten by

$$\displaystyle{ 1 +\gamma ^{y} = \frac{s_{t}(1-\tau \varDelta )w_{t}} {(1 + n_{t+1})a}. }$$
(12.19)

The derivation of this economic growth rate is examined at Appendix 1. Therefore, in this model, the economic growth rate in steady state grows at the constant rate.Footnote 14

1.5 Policy Effects

In this subsection, at the constant tax rate, we examine the effects of allocation between public support for children and public subsidy for children on economic growth rate .

From (12.15), at the constant tax rate, the effects of changing allocation from public subsidy for children to public support for children on fertility is

$$\displaystyle{ \frac{dn_{t+1}} {d\varDelta } = \frac{\epsilon (1 +\beta +\epsilon )\eta (1+\theta )\varDelta ^{-(1+\theta )-1}} {[(1 +\beta +\epsilon )\eta \varDelta ^{-(1+\theta )}]^{2}} > 0. }$$
(12.20)

Decreasing public subsidy for children and increasing public support for children enhances the fertility. Increasing such support can make consumers supply labour more and get more wage income. Such income gives consumers the incentive to have more children and enhances the fertility rate. This depends on the log utility function, (12.1) and the assumption of raising time because the marginal substitution of consumptions at each period is constant in the log utility function. We note \(\frac{dn_{t+1}} {d\tau } = 0\) from (12.15) because we assume the log utility function.

Next, we consider how the allocation change affects the economic growth rate. Differentiation economic growth rate, (12.19) with respect to Δ makes

$$\displaystyle{ \frac{d(1 +\gamma ^{y})} {d\varDelta } = \frac{-s_{t}w_{t}\tau (1 + n_{t+1})a - (1-\tau \varDelta )s_{t}aw_{t}\frac{dn_{t+1}} {d\varDelta } } {[(1 + n_{t+1})a]^{2}} < 0. }$$
(12.21)

Changing allocation from public subsidy for children to public support for children decreases public subsidy and saving. Such changing increases public support and saving through wage income and raising cost. The former effect is shown by the first term of right hand side of numerator on (12.21). The latter effect is by the second term of right hand side of numerator on (12.21). In this model, because the negative effect of public subsidy and saving on economic growth rate dominates that of public support and saving, increasing public support for children declines the economic growth rate.Footnote 15

We note that, from (12.19),

$$\displaystyle{\frac{d(1 +\gamma ^{y})} {d\tau } = \frac{-s_{t}\varDelta w_{t}} {(1 + n_{t+1})a} < 0.}$$

Increasing wage tax rate decreases the economic growth rate through decreasing disposable income and saving.

1.6 Welfare Effect

In this subsection, we examine welfare effect s of allocation change at the constant tax rate.

Using (12.11) and (12.13), the optimal plans for representative agent of generation t are

$$\displaystyle{ c_{t}^{t} = \frac{\left (1-\varDelta \tau \right )W_{t}} {\left (1 +\beta +\epsilon \right )}, }$$
(12.22)

and

$$\displaystyle{ c_{t+1}^{t} = \frac{\left (1 + r_{t+1}\right )\beta \left (1-\varDelta \tau \right )W_{t}} {\left (1 +\beta +\epsilon \right )}. }$$
(12.23)

Based on these optimal plans, as a social welfare function , we define the indirect utility function for the representative agent of generation t,

$$\displaystyle\begin{array}{rcl} V ^{t} =\ln \frac{\left (1-\varDelta \tau \right )W_{t}} {\left (1 +\beta +\epsilon \right )} +\beta \ln \frac{\left (1 + f'\left (a\right )\right )\beta \left (1-\varDelta \tau \right )W_{t}} {\left (1 +\beta +\epsilon \right )} +\epsilon \ln \frac{\epsilon } {(1 +\beta +\epsilon )\left (\eta \varDelta ^{-(1+\theta )}\right )}.& &{}\end{array}$$
(12.24)

At period t, we show the welfare effect of changing allocation from public subsidy for children to public support for children by

$$\displaystyle{ \frac{dV ^{t}} {d\varDelta } = \frac{-\tau \left (1+\beta \right )} {\left (1-\varDelta \tau \right )} + \left (1+\beta \right )\frac{\frac{dW_{t}} {d\varDelta } } {W_{t}} +\epsilon \left (1+\theta \right )\frac{1} {\varDelta }. }$$
(12.25)

Furthermore, given K t and L t , when

$$\displaystyle{ \frac{\tau \left (1+\beta \right )} {\left (1-\varDelta \tau \right )} =\epsilon \left (1+\theta \right )\frac{1} {\varDelta } + \left (1+\beta \right )\frac{\frac{dW_{t}} {d\varDelta } } {W_{t}}, }$$
(12.26)

social welfare is maximised at period t. The left hand side of (12.26) shows the negative welfare effect of increasing public support for children and decreasing public subsidy for children and consumption. The first term of right hand side of (12.26) shows the welfare effect of increasing public support for children and the second term of that is the effects of increasing public support for children and wage income through working time.

On the contrary to th effects of these policy on economic growth rate, based on (12.26), allocation change makes social welfare maximise at the constant tax. In the steady state, increasing public support for children decreases the economic growth rate but can enhances the social welfare. Whether these policies are favourable or not depends the objective of government policy.

2 Public Education and Social Security

As discussed above, declining fertility affects labour force. Having children enhances parents’ utility but generates child care cost and the cost of education for these children. The former cost includes parents’ own opportunity cost, which means that in raising children, parents sacrifice working time and income. The latter shows the material sacrifices made by parents. These costs are a disincentive to have children, causing fertility to decline and contributing to the aging of society. For labour force problems, government policies provide public support for children and public subsidy for children and promote human capital accumulation. Public policies should take into consideration the incentives to have children.

Beginning with Becker and Barro [3], it is well known that social security may affect the demand for children. As discussed before, Zhang and Zhang [37] introduce parents’ child care time into the model to examine the connection between the disincentive to have children and social security in an endogenous growth model. They show that a higher social security tax rate tends to be detrimental to economic growth and welfare. Groezen, Leers and Meijdam [17], Zhang and Zhang [40] and Hirazawa and Yakita [19] analyse public pensions and child support in a model with endogenous fertility. Cremer, Gahvari and Pestieau [7] discuss the design of pension schemes when fertility is endogenous and parents differ in the ability to raise children. However, they do not take into account educational cost.

Both education and social security can be viewed as mechanisms of intergenerational transfers . Becker and Tomes [4] and Gale and Scholz [13] show that these altruistically motivated transfers have a significant effect on the growth process via their impact on human capital accumulation. Kaganovich and Zilcha [20] examine the effects of the public funding of social security and education on economic growth without assuming population growth. They find that the shifting of tax revenues from social security benefits to education can improve welfare. Similar to Kaganovich and Zilcha [20], Pecchenino and Utendorf [27] assume that working people both educate their dependent children and pay into pay-as-you-go social security . In their view, social security crowds out education and reduces economic growth and social welfare. Pecchenino and Pollard [26] indicate that if the quality of the education system is sufficiently high, increasing the education tax and subsequently lowering the social security tax rate enhance growth and welfare. Glomm and Kaganovich [14] study how the allocation of government expenditures between public education and pay-as-you-go social security affects human capital distribution in an economy with heterogeneous agents. They show that increased spending in public education may lead to higher inequality. In all their models, population growth is assumed to be exogenous and child care time is not considered. Glomm and Kaganovich [15] examine, with the introduction of child care time into a model, how the relationship between economic growth and inequality depends on the levels of funding public education and social security. However, they do not discuss the effects of such public policies on fertility.

In this model, as in Kaganovich and Zilcha [20], parents derive their utility from the human capital of their children, investing in their children’s education at considerable material sacrifice . The number of children parents have depends on their investment in education. Glomm and Ravikumar [16] find that public education reduces income inequality more quickly than private education , but private education yields higher per capita income unless the initial income inequality is sufficiently large. In their analysis, any spillovers of these decisions on fertility are abstracted. Introducing child care time into the model without social security, de la Croix and Doepke [8] examine the relationship between private investment in education, public education and parents’ fertility decision making. They find that while private schooling leads to higher growth when there is little inequality in human capital endowments across families, public schooling can dominate when inequality is sufficiently high. As social security is abstracted in all their models, it is not well understood how the relationship between incentives to have children and social security impacts individual fertility decisions. Zhang and Zhang [38] examine the effects of unfunded social security with bequests, fertility and human capital by considering a mix of earnings-dependent and universal social security benefits. They show that social security is more likely to promote growth by reducing fertility and increasing human capital investment if its benefits are more dependent on individuals’ own earnings. Yew and Zhang [33] discuss the optimal scale of social security in a dynastic family model with human capital externalities, fertility and endogenous growth. In these all models, public education is not introduced.

In the second half of this chapter, we introduce the education cost into the pay-as-you-go social security model to examine the effects of public education and social security on fertility.Footnote 16 We suppose that individuals have the disincentive to have children while paying the education cost as a material sacrifice and cutting their working hours while raising children as an opportunity cost. Furthermore, for the compensation of children costs, parents cut their saving for the retirement and they need more social security benefits.

Additionally, to clarify how income tax affects fertility decision, we examine the effects of different types of income taxes on fertility. First, we discuss the effects of capital income tax on fertility. If a capital income tax is available as a source of revenue to finance education and social security and this makes the old not only beneficiaries but also contributors to the fiscal system, capital income tax might affect the fertility decision.Footnote 17 We then examine the effect of capital income tax on fertility. Second, when government budget constraint is decoupled and there are dedicated taxes for both social security and for public investment in education, we can consider the effect of education tax on fertility while keeping the social security tax constant and that of social security tax with a constant education tax. These discussions make it much clearer how parents’ decision on children depends on public investment in education and/or social security benefits.

2.1 Model

We consider an overlapping generations economy , where is comprised of identical three-period lived agents, perfectly competitive firms, and a government.

Consumers

Agents in the first period of their lives, the young, are raised by their parents and combine inputs provided by their parents and government to develop their human capital. Agents in the second period of their lives, the working, inelastically supply their effective labour to firms. They have children and divide their one unit of time between raising their children and working and their after-tax income among current consumption, saving for consumption when retirement, and private investment in education. Agents in the final period of their lives, the retirement, consume their social security benefits and their accumulated savings. We assume no bequests. The working generation at period t is called generation t. Following Omori [25], the preference of a representative agent of generation t is

$$\displaystyle{ U\left (c_{t}^{t},\;c_{ t+1}^{t},\;n_{ t+1},\;h_{t+1}\right ) =\ln c_{t}^{t}\; +\;\beta \ln c_{ t+1}^{t}\; +\;\delta \ln \; n_{ t+1}h_{t+1}, }$$
(12.27)

where c t t and c t+1 t are the consumption of generation t during the working period and the retirement period, respectively, n t+1 is the number of children for generation t, h t+1 is the human capital at t + 1 and β and δ are the positive parameter.Footnote 18

Let N t be the total working population at period t, and we have \(N_{t+1} = \left (1 + n_{t+1}\right )N_{t}\). The human capital of each agent in generation t + 1 is a function of the private investment in education made by parents, e t , as the material sacrifice, the public investment in education made by the government, E t , and the parents’ child caring time per child, \(\varLambda\), as the opportunity cost.Footnote 19 We note that we can enjoy the economies of scale in education when we privately educate our children. Taking into consideration such insight, we assume the input of private education as

$$\displaystyle{ \epsilon _{t} = \frac{e_{t}} {n_{t+1}^{\psi }}, }$$
(12.28)

where ψ ≥ 0. Then, the human capital of each agent in generation t + 1 is given by

$$\displaystyle{ h_{t+1} =\epsilon _{ t}^{\eta }E_{ t}^{1-\eta }\varLambda ^{\gamma }, }$$
(12.29)

where γ > 0 and 0 < η < 1.

The budget constraints of a representative agent of generation t in working and the retirement period are given, respectively, by

$$\displaystyle{ \left (1 -\tau _{w}\right )\,\left [1 - n_{t+1}\varLambda \right ]\,w_{t}h_{t} = c_{t}^{t} + e_{ t} + s_{t}, }$$
(12.30)

and

$$\displaystyle{ \left (1 + r_{t+1}\right )s_{t} + T_{t+1} = c_{t+1}^{t}, }$$
(12.31)

where τ w is the wage income tax rate , w t the wage rate at t, s t his(her)savings, r t+1 the interest rate at t + 1, and T t+1 the social security benefits at t + 1.Footnote 20

Given the wage rate, the interest rate, the human capital, the wage income tax rate, and the child care time per child, a representative agent chooses c t t, c t+1 t, n t+1, and e t to maximise his utility, (12.27), subject to the budget constraints, (12.30) and (12.31).

From the first-order conditions, we can show the optimal plans as

$$\displaystyle\begin{array}{rcl} n_{t+1}& =& \frac{\delta \left (1-\eta \psi \right )} {\left (1 +\beta +\delta \left (1 +\eta \left (1-\psi \right )\right )\right )\varLambda } \\ & & \quad + \frac{\delta \left (1-\eta \psi \right )T_{t+1}} {\left (1 -\tau _{w}\right )\varLambda w_{t}h_{t}\left (1 + r_{t+1}\right )\left (1 +\beta +\delta \left (1 +\eta \left (1-\psi \right )\right )\right )},{}\end{array}$$
(12.32)
$$\displaystyle{ e_{t} = \frac{\left (\left (1 -\tau _{w}\right )w_{t}h_{t} + \frac{T_{t+1}} {\left (1+r_{t+1}\right )}\right )\delta \eta } {\left (1 +\beta +\delta \left (1 +\eta \left (1-\psi \right )\right )\right )}, }$$
(12.33)

and

$$\displaystyle{ s_{t} = \frac{\beta \left (1 -\tau _{w}\right )w_{t}h_{t}} {\left (1 +\beta +\delta \left (1 +\eta \left (1-\psi \right )\right )\right )} - \frac{\left (1 +\delta \left (1 +\eta \left (1-\psi \right )\right )\right )T_{t+1}} {\left (1 + r_{t+1}\right )\left (1 +\beta +\delta \left (1 +\eta \left (1-\psi \right )\right )\right )}. }$$
(12.34)

We assume \(\beta \left (1 + r_{t+1}\right ) \geq \frac{\left (1+\delta \left (1+\eta \left (1-\psi \right )\right )\right )T_{t+1}} {\left (1-\tau _{w}\right )w_{t}h_{t}}\) for the non-negative saving.

Firms

Firms behave perfect competitively to maximise their profit and produce output with capital and effective labour. The aggregate production function is expressed as

$$\displaystyle{Y _{t} = AK_{t}^{\alpha }H_{ t}^{1-\alpha },}$$

where Y t is the aggregate output at period t, A is a time-independent productivity scalar, K t the aggregate capital at period t, H t the aggregate effective labour at period t, \(H_{t} = h_{t}\left (1 - n_{t+1}\varLambda \right )N_{t}\), and 0 < α < 1. The production technology exhibits a constant return to scale, and the marginal productivity of each input is positive and decreasing. We define \(y_{t} \equiv \frac{Y _{t}} {N_{t}}\) and \(k_{t} \equiv \frac{K_{t}} {N_{t}}\). The output per the generation t can be rewritten by

$$\displaystyle{ y_{t} = Ak_{t}^{\alpha }\left [h_{ t}\left (1 - n_{t+1}\varLambda \right )\right ]^{1-\alpha }. }$$
(12.35)

From the first-order conditions for profit maximisation, factor prices are derived as follows,

$$\displaystyle{ w_{t} = \left (1-\alpha \right )Ak_{t}^{\alpha }\left [h_{ t}\left (1 - n_{t+1}\varLambda \right )\right ]^{-\alpha } = \left (1-\alpha \right ) \frac{y_{t}} {\left [h_{t}\left (1 - n_{t+1}\varLambda \right )\right ]}, }$$
(12.36)

and

$$\displaystyle{ 1 + r_{t} =\alpha Ak_{t}^{\alpha -1}\left [h_{ t}\left (1 - n_{t+1}\varLambda \right )\right ]^{1-\alpha } =\alpha \frac{y_{t}} {k_{t}}. }$$
(12.37)

Government

The government is assumed to behave under a balanced budget regime. Tax revenues are collected to finance public investment in education and social security benefits in this period. The government budget constraint in period t is

$$\displaystyle{\tau _{w}\left [1 - n_{t+1}\varLambda \right ]w_{t}h_{t}N_{t} = E_{t}N_{t} + T_{t}N_{t-1}.}$$

Let us further define a parameter Δ to stand for the fraction of government revenue devoted to public investment in education (0 < Δ < 1). This investment in education in period t is

$$\displaystyle{ E_{t} =\varDelta \tau _{w}\left [1 - n_{t+1}\varLambda \right ]w_{t}h_{t} =\varDelta \tau _{w}\left (1-\alpha \right )y_{t}, }$$
(12.38)

and the social security benefits at t are expressed by

$$\displaystyle{ T_{t} = \left (1 + n_{t}\right )\left (1-\varDelta \right )\tau _{w}\left [1 - n_{t+1}\varLambda \right ]w_{t}h_{t} = \left (1 + n_{t}\right )\left (1-\varDelta \right )\tau _{w}\left (1-\alpha \right )y_{t}. }$$
(12.39)

In the following discussions, the government predetermines the sequences of τ w and Δ for simplicity.

2.2 Number of Children

The good market clears when the capital market clears. The equilibrium condition of capital market can be written per capita as

$$\displaystyle{ \left (1 + n_{t+1}\right )k_{t+1} = s_{t}. }$$
(12.40)

Using (12.34)–(12.39), (12.40) can be rewritten as

$$\displaystyle\begin{array}{rcl} & & (1 + n_{t+1})k_{t+1} = \frac{\left (1-\alpha \right )} {\left (1 +\beta +\delta (1 +\eta (1-\psi ))\right )} \\ & & \quad \times \left [ \frac{\beta \left (1 -\tau _{w}\right )y_{t}} {\left (1 - n_{t+1}\varLambda \right )} -\alpha ^{-1}\left (1 +\delta (1 +\eta (1-\psi ))\right )\left (1-\varDelta \right )\tau _{ w}\left (1 + n_{t+1}\right )k_{t+1}\right ].{}\end{array}$$
(12.41)

In equilibrium, substituting (12.36), (12.37), (12.39), and (12.41) into the optimal plan for the number of children, (12.32), we can obtain the optimal number of children in the steady state as

$$\displaystyle\begin{array}{rcl} n_{t+1}& =& \frac{\delta \left (1-\eta \psi \right )} {\left (1 +\beta +\delta \left (1 +\eta \left (1-\psi \right )\right )\right )\varLambda } \\ & & \quad + \frac{\delta \left (1-\eta \psi \right )} {\left (1 +\beta +\delta \left (1 +\eta \left (1-\psi \right )\right )\right )\varLambda } \\ & & \times \frac{\left (1-\varDelta \right )\tau _{w}\left (1-\alpha \right )\beta } {\left [\alpha \left (1 +\beta +\delta \left (1 +\eta \left (1-\psi \right )\right )\right ) + \left (1 +\delta \left (1 +\eta \left (1-\psi \right )\right )\right )\left (1-\alpha \right )\left (1-\varDelta \right )\tau _{w}\right ]}.{}\end{array}$$
(12.42)

As τ w and Δ are assumed to be predetermined, n t+1 is the time-invariant variable in the equilibrium.Footnote 21 The first term on the right-hand side in (12.42) shows the substitute effect of having children and the second term indicates the income effect of social security benefits on the number of children. We note that the first term does not depend on any public policy parameters.

2.3 Economic Growth Rate

In this model, the steady state is in the path that the ratio of human capital, (h) and physical capital,(k) is constant. That is,

$$\displaystyle{ \frac{h_{t+1}} {k_{t+1}} = \frac{h_{t}} {k_{t}} =\omega. }$$
(12.43)

The economic growth rate in the steady state is

$$\displaystyle{1+\gamma = \frac{k_{t+1}} {k_{t}}.}$$

Using (12.28)–(12.29) and (12.33)–(12.42), we can show the economic growth rate at the steady state as, in the from of logarithm function,

$$\displaystyle\begin{array}{rcl} & & \ln \left (1+\gamma \right ) =\ln \left (\frac{k_{t+1}} {k_{t}} \right ) =\ln \frac{1} { \dfrac{\left (1 - n_{t+1}\varLambda \right )} {\beta \left (1 -\tau _{w}\right )\left (1-\alpha \right )}} \\ & & +\ln \left [\frac{1} {\alpha } \left (1 +\delta \left (1 +\eta \left (1-\psi \right )\right )\right )\left (1 + n_{t+1}\right )\left (1-\varDelta \right )\tau _{w}\left (1-\alpha \right )\right. \\ & & \qquad \qquad + \left.\left (1 +\beta +\delta \left (1 +\eta \left (1-\psi \right )\right )\right )\right ] \\ & & +\ln \left (1 +\beta +\delta \left (1 +\eta \left (1-\psi \right )\right )\right ) +\ln \left (1 - n_{t+1}\varLambda \right ) + \left (1-\alpha \right )\ln \omega,{}\end{array}$$
(12.44)

where

$$\displaystyle\begin{array}{rcl} & & \ln \omega =\ln \left (\frac{h_{t+1}} {k_{t+1}}\right ) =\eta \ln \frac{\delta \eta } {\left (1 +\beta +\delta \left (1 +\eta \left (1-\psi \right )\right )\right )} \\ & & +\eta \ln \bigg [ \frac{1} {\beta \left (1-\alpha \right )}\Big[\frac{1} {\alpha } \left (1 +\delta \left (1 +\eta \left (1-\psi \right )\right )\right )\left (1 - n_{t+1}\right )\left (1-\varDelta \right )\tau _{w}\left (1-\alpha \right ) \\ & & \qquad \qquad + \left (1 +\beta +\delta \left (1 +\eta \left (1-\psi \right )\right )\right )\Big]\bigg ] + \left (1-\eta \right )\ln \varDelta \tau _{w}\left (1-\alpha \right ) \\ & & +\ln \frac{\left (1 - n_{t+1}\varLambda \right )} {\beta \left (1 -\tau _{w}\right )\left (1-\alpha \right )}\Big[\frac{1} {\alpha } \left (1 +\delta \left (1 +\eta \left (1-\psi \right )\right )\right )\left (1 - n_{t+1}\right )\left (1-\varDelta \right )\tau _{w}\left (1-\alpha \right ) \\ & & \qquad \qquad + \left (1 +\beta +\delta \left (1 +\eta \left (1-\psi \right )\right )\right )\Big] +\gamma \ln \varLambda -\eta \psi \ln n_{t+1}. {}\end{array}$$
(12.45)

See the Appendix 2 for the derivation of (12.44). At the steady state, because (12.42) is constant, the right hand side of (12.45) is also constant. Therefore, ω is constant and the economic growth rate, (12.44) is constant. However, as this economic growth rate depends on parameters, it is difficult to discuss the policy effects on economic growth rate. As the purpose of this part is to examine the policy effects on fertility, hereinafter, we discuss that on fertility.

2.4 Policy Effect

We examine the effects of wage income tax and allocation between public investment in education and social security benefits on fertility, respectively.

Wage Income Tax

We can show the effect of wage income tax on fertility in the steady state. From (12.42),

$$\displaystyle\begin{array}{rcl} & & \frac{dn} {d\tau _{w}} = \\ & & \frac{\delta \left (1-\eta \psi \right )} {\left (1 +\beta +\delta \left (1 +\eta \left (1-\psi \right )\right )\right )\varLambda } \\ & & \times \left [ \frac{\alpha \left (1-\varDelta \right )\left (1-\alpha \right )\beta \left (1 +\beta +\delta \left (1 +\eta \left (1-\psi \right )\right )\right )} {\left [\alpha \left (1 +\beta +\delta \left (1 +\eta \left (1-\psi \right )\right )\right ) + \left (1 +\delta \left (1 +\eta \left (1-\psi \right )\right )\right )\left (1-\alpha \right )\left (1-\varDelta \right )\tau _{w}\right ]^{2}}\right ] > 0.{}\end{array}$$
(12.46)

A higher wage income tax rate always increases fertility . It increases public investment in education and social security benefits. Increasing public investment in education lowers both child care cost as an opportunity cost and the cost of private education as a material sacrifice . Such increases give the incentive to have children. Increasing social security benefits partly compensates for the savings cut. When parents decide to have an additional child, they have to cut their savings to cover the cost of children. To compensate for the savings cut, they need more income and social security benefits. Such tax increases provide the incentive to have children. In this model, the higher wage tax rate encourages the agents to have more children.Footnote 22

Allocation Between Public Investment in Education and Social Security Benefits

Both education and social security can be viewed as mechanisms of intergenerational transfer . To discuss the effects of intergenerational transfers on fertility , we examine the effects on fertility of reallocating public funds from social security benefits to public investment in education. In the steady state, that effect can be shown by, from (12.42),

$$\displaystyle\begin{array}{rcl} & & \frac{dn} {d\varDelta } = \\ & & \frac{\delta \left (1-\eta \psi \right )} {\left (1 +\beta +\delta \left (1 +\eta \left (1-\psi \right )\right )\right )\varLambda } \\ & & \times \left [ \frac{-\alpha \tau _{w}\left (1-\alpha \right )\beta \left (1 +\beta +\delta \left (1 +\eta \left (1-\psi \right )\right )\right )} {\left [\alpha \left (1 +\beta +\delta \left (1 +\eta \left (1-\psi \right )\right )\right ) + \left (1 +\delta \left (1 +\eta \left (1-\psi \right )\right )\right )\left (1-\alpha \right )\left (1-\varDelta \right )\tau _{w}\right ]^{2}}\right ] < 0.\!\!{}\end{array}$$
(12.47)

With a fixed wage income tax rate, reallocating from social security benefits to public expenditure in education decreases fertility. A reallocation from social security benefits to public investment in education has two effects. One is that increasing public investment in education raises the human capital and generates an income effect in the steady state. As discussed before, this increase serves to decrease both the child care cost and the cost of private education. The other is that such reallocation decreases the income for the retirement generation through the social security benefits . Although having children means costs, from the optimal plans, (12.32)–(12.34), it is clear that decreasing the social security benefits gives the agents the incentives to save more for retirement period consumption and to have fewer children.

Capital Income Tax

As discussed above, both education and social security have mechanisms of intergenerational transfers. We have shown that the reallocation of social security benefits to public investment in education decreases fertility. The capital income tax is considered another way of affecting the fertility decision. In this section, we examine the effect of capital income tax on fertility .

We suppose that government adopts the comprehensive capital tax policy, which is a tax not only on wealth but also on interest income.Footnote 23 The budget constraints of a representative agent of generation t in working and the retirement period should be changed, respectively, by

$$\displaystyle{ \left [1 - n_{t+1}\varLambda \right ]\,w_{t}h_{t} = c_{t}^{t} + e_{ t} + s_{t}, }$$
(12.48)

and

$$\displaystyle{ (1 -\tau _{i})\left (1 + r_{t+1}\right )s_{t} + T_{t+1} = c_{t+1}^{t}, }$$
(12.49)

where τ i is the capital income tax rate.Footnote 24

The government budget constraint in period t is

$$\displaystyle{\tau _{i}\left (1 + r_{t}\right )s_{t-1}N_{t-1} = E_{t}N_{t} + T_{t}N_{t-1}.}$$

This investment in education in period t is given by

$$\displaystyle{ E_{t} =\varDelta \tau _{i}\alpha y_{t}. }$$
(12.50)

The one for social security benefits is given by

$$\displaystyle{ T_{t} = (1-\varDelta )\tau _{i}\left (1 + n_{t}\right )\alpha y_{t}. }$$
(12.51)

Government predetermines the sequences of τ i and Δ for simplicity.

Using a similar procedure in deriving (12.42), the optimal number of children in the steady state can be given by

$$\displaystyle\begin{array}{rcl} & & n_{t+1} = \frac{\delta \left (1-\eta \psi \right )} {\left (1 +\beta +\delta \left (1 +\eta \left (1-\psi \right )\right )\right )\varLambda } \\ & & \qquad \quad + \frac{\delta \left (1-\eta \psi \right )} {\left (1 +\beta +\delta \left (1 +\eta \left (1-\psi \right )\right )\right )} \\ & & \times \frac{\tau _{i}\left (1-\varDelta \right )\beta } {\left [(1 -\tau _{i})\left (1 +\beta +\delta \left (1 +\eta \left (1-\psi \right )\right )\right ) + \left (1 +\delta \left (1 +\eta \left (1-\psi \right )\right )\right )\left (1-\varDelta \right )\tau _{i}\right ]}.{}\end{array}$$
(12.52)

The similar explanation in (12.42) applies for the optimal number of children, (12.52).

In the steady state, the effect of capital income tax on fertility is revealed by the differentiation of (12.52) with respect to τ i . That is,

$$\displaystyle\begin{array}{rcl} & & \frac{dn} {d\tau _{i}} = \\ & & \frac{\delta \left (1-\eta \psi \right )\left [(1-\varDelta )\beta \left [\left (1 +\beta +\delta \left (1 +\eta \left (1-\psi \right )\right )\right ) -\varDelta \left (1 +\delta \left (1 +\eta \left (1-\psi \right )\right )\right )\tau _{i}\right ]\right ]} {\left (1 +\beta +\delta \left (1 +\eta \left (1-\psi \right )\right )\right )\varLambda \left [\left (1 +\beta +\delta \left (1 +\eta \left (1-\psi \right )\right )\right ) + \left (1 +\delta \left (1 +\eta \left (1-\psi \right )\right )\right )\tau _{i}\right ]^{2}} \\ & & \quad > 0. {}\end{array}$$
(12.53)

A higher capital income tax increases fertility . Increasing capital income tax generates the substitutable effect on the cost of having children because of increasing public investment in education and social security benefits. The higher capital tax rate encourages the agents to have more children.

Contrary to above discussions, (12.53) shows a positive effect on fertility. A capital income tax is only available as a source of revenue to finance public education and social security and this makes the retirement not only beneficiaries but also contributors to the fiscal system. The working are not taxed and they only enjoy the benefits of public investment in education financed by the capital income tax. We show that the intergenerational income redistribution from the retirement to the working through such income tax can positively influence fertility.

Education Tax and Social Security Tax

When the government budget constraint is decoupled and there are dedicated taxes both for public investment in education and for social security, we can consider the effect of education tax on fertility while maintaining a constant social security tax and that of social security tax with a constant education tax. This discussion makes it much clearer to show how parents’ decision on children depends on public investment in education and/or social security benefits.Footnote 25

The budget constraints of a representative agent of generation t in working and the retirement period should be changed, respectively, by

$$\displaystyle{ \left (1 -\tau _{E} -\tau _{T}\right )\,\left [1 - n_{t+1}\varLambda \right ]\,w_{t}h_{t} = c_{t}^{t} + e_{ t} + s_{t}, }$$
(12.54)

and

$$\displaystyle{ \left (1 + r_{t+1}\right )s_{t} + T_{t+1} = c_{t+1}^{t}, }$$
(12.55)

where τ E is the wage income tax rate for public expenditure on education (education tax) and τ T is the wage income tax rate for social security benefits (social security tax).Footnote 26

The government budget constraint for public education per capita in period t is given by

$$\displaystyle{ E_{t} =\tau _{E}\left (1-\alpha \right )y_{t}. }$$
(12.56)

That for social security benefits is given by

$$\displaystyle{ T_{t} = \left (1 + n_{t}\right )\tau _{T}\left (1-\alpha \right )y_{t}. }$$
(12.57)

For simplicity, τ E and τ t are predetermined.

Using a similar procedure to (12.42), the optimal number of children in the steady state can be given by

$$\displaystyle\begin{array}{rcl} n_{t+1}& =& \frac{\delta \left (1-\eta \psi \right )} {\left (1 +\beta +\delta \left (1 +\eta \left (1-\psi \right )\right )\right )\varLambda } \\ & & \quad + \frac{\delta \left (1-\eta \psi \right )} {\left (1 +\beta +\delta \left (1 +\eta \left (1-\psi \right )\right )\right )\varLambda } \\ & & \times \frac{\tau _{T}\left (1-\alpha \right )\beta } {\left [\alpha \left (1 +\beta +\delta \left (1 +\eta \left (1-\psi \right )\right )\right ) + \left (1 +\delta \left (1 +\eta \left (1-\psi \right )\right )\right )\left (1-\alpha \right )\tau _{T}\right ]}.{}\end{array}$$
(12.58)

A similar explanation in (12.42) applies for the optimal number of children (12.58).

The effect of education tax on fertility while holding the social security tax constant is shown by, from (12.58),

$$\displaystyle{ \frac{dn} {d\tau _{E}} = 0. }$$
(12.59)

With a fixed wage income tax rate for social security benefits, the effect of increasing the wage income tax rate for public investment in education on fertility is neutral. As shown in (12.58), the parameter on wage income tax rate for public investment in education does not affect the number of children in the steady state. For the working, although increasing the education tax decreases their disposable labour income, such an increase does not affect parents’ decision on children. This result depends on the assumption of the logarithm utility function. There are no effects of the education tax on the marginal rate of substitution in this form.Footnote 27 The change in education tax rate affects the amount of saving, but does not influence the savings rate.

On the other hand, in the steady state, the effect of social security tax with constant education tax on fertility is shown by, from (12.58),

$$\displaystyle\begin{array}{rcl} & & \frac{dn} {d\tau _{T}} = \frac{\delta \left (1-\eta \psi \right )} {\left (1 +\beta +\delta \left (1 +\eta \left (1-\psi \right )\right )\right )\varLambda } \\ & & \times \left [ \frac{\left (1-\alpha \right )\beta \alpha \left (1 +\beta +\delta \left (1 +\eta \left (1-\psi \right )\right )\right )} {\left [\alpha \left (1 +\beta +\delta \left (1 +\eta \left (1-\psi \right )\right )\right ) + \left (1 +\delta \left (1 +\eta \left (1-\psi \right )\right )\right )\left (1-\alpha \right )\tau _{T}\right ]^{2}}\right ] > 0.\!\!{}\end{array}$$
(12.60)

With a fixed wage income tax rate for public investment in education, the effect of increasing the wage income tax rate for social security benefits on fertility is positive. As shown in the optimal number of children, (12.58), in the steady state, the optimal number of children does depend not on the wage income tax rate for public investment in education but on the wage income tax rate for social security benefits. In this case, taking into consideration individual behaviours in the retirement period, parents’ decision on having children depends on social security. When parents decide to have an additional child, they have to cut their savings to cover the cost of children. To compensate for the savings cut, they need more social security benefits. In this model, we suppose a pay-as-you-go social security system. Having more children increases the social security benefits for the retirement. Increasing the wage income tax rate for social security benefits encourages the agents to have more children.Footnote 28

The neutrality of education tax with constant social security tax depends on the assumption on utility function. However, even in this model, there exists the positive effect of social security tax with a constant education tax. Social security might have the replacement effect on private saving but education does not have an intertemporal replacement effect. This intertemporal replacement effect changes the individual savings rate and causes the difference between education tax and social security tax.

Finally, in this part, because the model is too complex to examine the welfare effects in this model, this examination is left for the future research.

3 Concluding Remarks

The fertility affect the economic activities in the country. How many parents have children depends on the expenditures to their children including the opportunity cost, the raising cost and the education cost.

In the first half of this chapter, we examined the effects of public subsidy for children and public support for children on economic growth and social welfare. Decreasing public subsidy for children but increasing public support for children enhances fertility. Public support for children increases labour supply and more wage income. Such income gives parents the incentive to have children more. However, at the constant wage income tax rate, such support deceases the economic growth rate. In the steady state, increasing public support for children decreases the economic growth rate but can enhances the social welfare. Whether these policies are favourable or not depends the objective of government policy.

In the second half of this chapter, we have examined the effects of public education and social security on fertility by introducing the fertility decision and the child care cost into the overlapping generations model. Both education and social security can be viewed as mechanisms of intergenerational transfers. The intergenerational transfer from the retirement to the young affects the individual’s fertility decision. We have shown that an increase in income tax which finances public education and social security benefits raises fertility. Especially, the increases of wage income tax, capital income tax, and social security tax with constant education tax raise fertility. On the other hand, a change in allocation from the social security benefits to public investment in education decreases fertility and, with a constant social security tax, the effect of education tax on fertility is neutral.

To have an additional child, parents must cut their savings to cover the cost of children. Public policy, which lowers child care cost and increases social security benefits, partly compensates for the savings cut and gives parents the incentive to have children. In a fertility declining society, when government creates a policy to enhance fertility, government should take into consideration the parental disincentives to have children and the cost of children. Such public policy can help stimulate fertility.