Keywords

These keywords were added by machine and not by the authors. This process is experimental and the keywords may be updated as the learning algorithm improves.

1 Introduction to GMGHS Charged Black Holes in String Theory

In this paper we studied null geodesics of the GMGHS charged black hole in the string theory. Let us first give an introduction to the theory and the resulting black hole solution.

The action corresponding to the GMGHS black hole is given by,

$$\displaystyle{ S = \frac{1} {16\pi }\int {d}^{4}x\sqrt{-g}\left [R - 2{(\bigtriangledown \varPhi )}^{2} - {e}^{-2\varPhi }F_{\mu \nu }{F}^{\mu \nu }\right ] }$$
(1)

Here Φ is the dilaton field, R is the scalar curvature and F μ ν is the Maxwell’s field strength. The static charged black hole solutions to the above action were found first by Gibbons and Maeda[2]. It was also independently found by Garfinkle, Horowitz and Strominger [3] few years later.

The GMGHS black hole solution to the action in Eq. (1) is given by,

$$\displaystyle{ \mathit{ds}_{E}^{2} = -\left (1 -\frac{2M} {r} \right ){\mathit{dt}}^{2} + \frac{1} {\left (1 -\frac{2M} {r} \right )}{\mathit{dr}}^{2} + r\left (r - a\right )({d\theta }^{2} +{ \mathit{sin}}^{2}(\theta ){d\phi }^{2})\quad }$$
(2)

Here, the electric field strength and the dilaton field are given by,

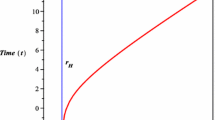
$$\displaystyle{ F_{\mathit{rt}} = \frac{Q} {{r}^{2}};\qquad {e}^{2\varPhi } = 1 - \frac{{Q}^{2}} {\mathit{Mr}};\ \ a = \frac{{Q}^{2}} {M} }$$
(3)

There is an event horizon at r = 2M. How ever, the area of the sphere of the string black hole is smaller and the area approaches zero when \(r = {Q}^{2}/M\). Therefore, \(r = {Q}^{2}/M\) surface is singular. For \({Q}^{2} \leq 2{M}^{2}\), the singular surface is inside the event horizon and the Penrose diagram is identical to the one of the Schwarzschild black hole. When Q 2 = 2M 2, the singular surface coincides with the horizon. This is the extremal limit where a transition between the black hole and the naked singularity occurs.

2 Null Geodesics

The null geodesics for the above black hole are given by the following three equations.

$$\displaystyle{ R{(r)}^{2}\dot{\phi } = L }$$
(4)
$$\displaystyle{ f(r)\dot{t} = E }$$
(5)
$$\displaystyle{ \dot{{r}}^{2} + f(r) \frac{{L}^{2}} {R{(r)}^{2}} = {E}^{2} }$$
(6)

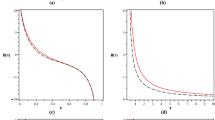
Here \(f(r) = 1 -\frac{2M} {r}\). One can do a change of variable as \(u = \frac{1} {r}\) and combine the above Eqs. (4), (5) and (6) to obtain an equation,

$$\displaystyle{{ \left (\frac{\mathit{du}} {d\phi } \right )}^{2} = g(u) }$$
(7)

where,

$$\displaystyle{ g(u) = -2{\mathit{aMu}}^{4} + (a + 2M){u}^{3} + {u}^{2}\left ({a}^{2}\frac{{E}^{2}} {{L}^{2}} - 1\right ) - 2a\frac{{E}^{2}} {{L}^{2}} u + \frac{{E}^{2}} {{L}^{2}} }$$
(8)

When \(a \rightarrow 0\), \(g(u) \rightarrow 2{\mathit{Mu}}^{3} - {u}^{2} + \frac{{E}^{2}} {{L}^{2}}\) as expected for the Schwarzschild black hole [4]. When a → 0, g(u) has maximum three real roots as described in the book by Chandrasekhar[4].

3 Bending of Light

Once the null geodesics are analyzed in detail for various parameters in the theory such as E, L, M and Q, it is possible to apply that knowledge to study important properties of the black hole geometry. One of them is the gravitational lensing or bending of light by the black hole. We obtained explicit expressions for the closest approach r o of the light ray in terms of the impact parameter D given in Eqs. (9), (10) and (11). There after, we compared the deflection angle of light as a function of D for the GMGHS black hole and the Schwarzschild black hole.

$$\displaystyle{ r_{o}^{\mathit{string}} = 2\sqrt{-\frac{p} {3}}\mathit{cos}\left (\frac{1} {3}{\mathit{cos}}^{-1}\left (\frac{3q} {2p}\sqrt{-\frac{3} {p}}\right )\right ) + \frac{a} {3} }$$
(9)

Here, p and q are given by,

$$\displaystyle{ p = \frac{{a}^{2} - 3{D}^{2}} {3} }$$
(10)
$$\displaystyle{ q = \frac{54{\mathit{MD}}^{2} - 9{\mathit{aD}}^{2} - 2{a}^{3}} {27} }$$
(11)

4 Unstable Null Geodesics and Quasinormal Modes of the Massless Scalar Field in the Eikonal Limit

When a black hole is perturbed, it undergoes damped oscillations; the frequencies of oscillations are called quasinormal modes. Perturbations of a black hole with a scalar field are given by the equation,

$$\displaystyle{ \frac{{d}^{2}\eta } {\mathit{dr}_{{\ast}}^{2}} + \left ({\omega }^{2} -\left (\frac{l(l + 1)} {{R}^{2}} + \frac{\mathit{ff }^{\prime}R^{\prime}} {R} + \frac{{f}^{2}{R}^{{\prime\prime}}} {R} \right )\right )\eta = 0 }$$
(12)

Here, l is the spherical harmonic index and r ∗ is the tortoise coordinate. The computation of quasinormal modes at the eikonal limit and the unstable null geodesics are related [5]. In the eikonal limit, the quasinormal modes are given as,

$$\displaystyle{ \omega _{\mathit{QNM}} =\varOmega _{c}l - i(n + \frac{1} {2})\vert \lambda \vert }$$
(13)

Here, the value n is a nonnegative integer. Ω c is the coordinate angular velocity given by \(\frac{\dot{\phi }} {\dot{t}}\) computed at unstable circular radius of the null geodesics which is given in Eq. (14). λ is the Lyapunov exponent which gives the instability timescale of the unstable circular null geodesics, given in Eq. (15)

$$\displaystyle{ \varOmega _{c} = \frac{\dot{\phi (r_{c})}} {\dot{t}(r_{c})} = \sqrt{ \frac{f(r_{c } )} {R{(r_{c})}^{2}}} = \sqrt{ \frac{r_{c } - 2M} {r_{c}^{2}(r_{c} - a)}} }$$
(14)
$$\displaystyle{ \lambda = \sqrt{\frac{(2M - r_{c } )(3r_{c }^{3 } - 12\mathit{Mr } _{c }^{2 } - 3\mathit{ar } _{c }^{2 } + 16\mathit{aMr } _{c } + {a}^{2 } r_{c } - 6{a}^{2 } M)} {r_{c}^{4}{(r_{c} - a)}^{2}}} }$$
(15)

5 Conclusions

We have studied the null geodesics of the GMHHS black hole. The equations for the geodesics were solved exactly for various values of energy and angular momentum of the photons.

As physical applications of the properties of the null geodesics obtained here, we have studied light bending and quasinormal modes of massless scalar field. The closest approach of the photons bending around the black hole is computed as a function of the impact parameter. The deflection angle α is computed as a function of the impact parameter. A comparison is done with the deflection angle of the Schwarzschild black hole. It was observed that the photons with the same impact parameter bend less around the string black hole compared to the Schwarzschild black hole. These results would be beneficial in computations of gravitational lensing of string black holes.

The unstable circular null geodesics of the black hole are used to compute the quasinormal modes of the black hole in the eikonal limit. We have followed an important result by Cardoso et al. [5] in deriving these results. The Lyapunov exponent λ, which gives the instability time scale is also computed. It was noted that there is a maximum value for λ at \(a = 6M(2 -\sqrt{3})\).