1 Introduction

Equations of Magnetohydrodynamics (the MHD equations) describe the motion of the magnetic field in a conducting fluid. This nonlinear system of PDE’s consists of the Navier-Stokes equations for the velocity field of the fluid and the Maxwell equations for the magnetic field. The equations are coupled by the term, describing the Lorenz force. The MHD equations describe, in particular, evolution of the magnetic fields of planets, stars and galaxies. Usually the viscosity and the resistance of the fluid are small enough and one can study the asymptotic solutions of the system with respect to the corresponding small parameter. This problem was studied in a lot of papers; note that in linear approximation the structure of the asymptotics is the subject of the famous dynamo theory (see, e.g. [1–12]). The main mathematical problem (which is still open) is to prove the existence of exponentially growing solutions.

The alternative effect was studied in [13, 14] (in linear approximation also). Namely, we described the instantaneous growth of the magnetic field, induced by the jump of the velocity field of the fluid. In another words, we studied the asymptotics of the solution for the Cauchy problem for linear induction equation with rapidly varying velocity field. We assumed that this field had a rapid jump in a small vicinity of the fixed 2D surface. We proved that the solution grows rapidly with respect to the corresponding small parameter, and has a delta-type singularity near the surface of the jump. This effect is a result of the non-Hermitian structure of the linearized induction operator—in certain sense the operator of the problem is close to the Jordan block.

Here we study the analogous problem for the complete nonlinear system. We describe the asymptotic structure of the solution with a rapid jump near 2D-surface. Now the surface is not fixed—it moves in time together with the solution. We obtain the special free boundary problem which governs the movement of the surface. We also study the possibility of the instantaneous growth of the magnetic field. It appears that the growth is possible only in the case of so called degenerate Alfwen modes; the latter appear if the main term of the magnetic field is tangent to the surface of the jump.

2 Statement of the Problem

2.1 The Cauchy Problem with the Jump of Initial Fields

We denote by B(x, t) and V(x, t) the magnetic and velocity fields in a conducting fluid (B, V are time-dependent vector fields in \(R^3\)). This pair of vector functions satisfy the following nonlinear MHD system

$$\begin{aligned} \frac{\partial B}{\partial t}+(V, \nabla )B-(B, \nabla )V=\varepsilon ^2\mu \triangle B\end{aligned}$$
(1)
$$\begin{aligned} \frac{\partial V}{\partial t}+(V, \nabla )V-(B, \nabla )B+\nabla P=\varepsilon ^2\nu \triangle V\end{aligned}$$
(2)
$$\begin{aligned} (\nabla , V)=0,\;\; (\nabla , B)=0. \end{aligned}$$
(3)

Here P(x, t) is a scalar function, which can be expressed in terms of B and the pressure of the fluid, \(\nu \) \(\mu \) are positive numbers, characterizing hydrodynamic and magnet viscosities, \(\varepsilon \rightarrow 0\) is a small parameter.

Let us consider for the system (1) initial data of the form

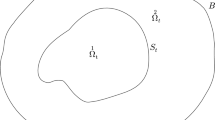
$$\begin{aligned} B|_{t=0}=B^0\left( \frac{\varPhi _0(x)}{\varepsilon },x,\varepsilon \right) ,\quad V|_{t=0}=V^0\left( {\frac{\varPhi _0(x)}{\varepsilon },x,\varepsilon }\right) , \end{aligned}$$
(4)

where \(\varPhi _0(x)\) is a smooth scalar function, divergence free vector fields \(B^0(y,x,\varepsilon )\), \(V^0(y,x,\varepsilon )\) depend smoothly on all arguments and tend to limits \(B^{0,\pm }(x,\varepsilon )\), \(V^{0,\pm }(x,\varepsilon )\) as \(y\rightarrow \pm \infty \) faster then any power of y. We assume that the equation \(\varPhi _0(x)=0\) defines a smooth compact surface \(M_0\subset R^3\) and \(\varPhi (x,t)<0\) inside the domain, bounded by the surface. Without the loss of generality one can assume also that in the vicinity of the surface \(|\varPhi _0|\) equals the distance from \(M_0\) in the normal direction; in particular, in this vicinity \(|\nabla \varPhi _0|=1\).

Remark 1

Vector fields of this type define “smoothened” discontinuities—as \(\varepsilon \rightarrow 0\) they tend to the discontinuous functions with the jump on the surface \(M_0\). The corresponding weak limits have the form

$$ \mathscr {B}^0=B^{0,+}(x,0)+\theta _{M_0}(B^{0,-}(x,0)-B^{0,+}(x,0)), $$
$$ \mathscr {V}^0=V^{0,+}(x,0)+\theta _{M_0}(V^{0,-}(x,0)-V^{0,+}(x,0)), $$

where \(\theta _{M_0}\) is the Heaviside function on \(M_0\).

In the next sections we describe the asymptotic solutions to the Cauchy problem (1)–(4) under some additional assumptions concerning the initial fields. These assumptions define separate nonlinear modes.

2.2 Degenerate and Nondegenerate Alfwen Modes

The structure of the asymptotic solution to the Cauchy problem (1)–(4) depends essentially of the presence of the points of the initial surface, in which \(B^0(y,x,0)\) is tangent to \(M_0\). We will study two limit cases: in the first case there are no such points (nondegenerate modes) while in the second one \(B^0\) is tangent to \(M_0\) everywhere (degenerate mode). We do not study the problem of nonlinear interaction of modes; note that even in linear approximation this problem is highly nontrivial (for WKB-type solutions this problem was studied recently in [15]). Note that it is easy to prove that, according to the equations governing the motion of the surface, the points of tangency can not appear or disappear—the absence or presence of these points is a property of the initial data.

If there is no tangency, on can extract the single nondegenerate mode with the help of additional conditions on the initial fields; these conditions (which are well known in the MHD theory) state that the fields \(\frac{\partial V^0(y,x,0)}{\partial y}\) and \(\frac{\partial B^0(y,x,0)}{\partial y}\) must coincide up to a sign. To be definite, we chose the sign “+”; so for nondegenerate mode we assume additionally that

$$\begin{aligned} \frac{\partial V^0(y,x,0)}{\partial y}=\frac{\partial B^0(y,x,0)}{\partial y}. \end{aligned}$$
(5)

For the degenerate mode we assume that B is tangent to M:

$$\begin{aligned} (B^0(y,x,0),\nabla \varPhi _0))|_{M_0}=0. \end{aligned}$$
(6)

Our goal is the description of the formal asymptotic solution as \(\varepsilon \rightarrow 0\) of the Cauchy problem (1–4) under additional conditions (5) or (6).

3 Formulation of the Results. Nondegenerate Modes

Here we describe the structure of asymptotic solution corresponding to nondegenerate mode. The main term of asymptotics is defined by the free boundary problem for the limit fields as \(y\rightarrow \pm \infty \) and by the equation on the moving surface, describing the profile of the rapidly varying field. Surprisingly, the latter equation appears to be linear.

3.1 Free Boundary Problem for Limit Fields

Let the conditions (5) are fulfilled; we denote by \(u_0(y,x),w_0(x)\) the main terms of the sum and the difference of the velocity field and the magnetic field in the initial instant of time:

$$ u_0=V^0(y,x,0)+B^0(y,x,0),\quad w_0=V^0(y,x,0)-B^0(y,x,0). $$

Let \(u_0^\pm , w_0^\pm \) be the limits of \(u_0, w_0\) as \(y\rightarrow \pm \infty \). Let us consider the following free boundary problem: for a finite time interval \(t\in [0,T]\) we seek for a smooth compact surface \(M_t\in R^3\) and for smooth vector fields \(u^{\pm }(x,t), w^\pm (x,t)\) and scalar functions \(P_0^\pm (x,t)\), defined in the internal (\(D_t^-\)) and external (\(D_t^+\)) domains and satisfying for \(x\in D_t^\pm \) the following equations

$$\begin{aligned}&\frac{\partial u^\pm }{\partial t}+(w^\pm ,\nabla )u^\pm +\nabla P_0^\pm =0,\end{aligned}$$
(7)
$$\begin{aligned}&\frac{\partial w^\pm }{\partial t}+(u^\pm ,\nabla )w^\pm +\nabla P_0^\pm =0,\end{aligned}$$
(8)
$$\begin{aligned}&(\nabla , u^\pm )=(\nabla , w^\pm )=0. \end{aligned}$$
(9)

We put also the conditions on the surface

$$\begin{aligned}{}[w]=0,\quad [P_0]=0,\quad [u_n]=0,\quad -\frac{\partial \varPhi }{\partial t}=w_n,\quad x\in M_t \end{aligned}$$
(10)

and the initial conditions

$$\begin{aligned} \varPhi |_{t=0}=\varPhi _0(x),\quad u^\pm |_{t=0}=u_0^\pm ,\quad w^\pm |_{t=0}=w_0^\pm ,\quad x\in D^\pm _0. \end{aligned}$$
(11)

Here \(\varPhi (x,t)\) denote the distance (with the appropriate sign) from the surface \(M_t\) in the normal direction, \(u_n=(u,\nabla \varPhi )|_{M_t}\), \(w_n=(w,\nabla \varPhi )|_{M_t}\), symbol [f] denotes the jump of the function f:

$$ [f]=f^+|_{M_t}-f^{-}|_{M_t}. $$

Remark 2

The surface \(M_t\) is defined by the equation \(\varPhi (x,t)=0\); the boundary condition \(-\frac{\partial \varPhi }{\partial t}=w_n\) means that the surface moves along the trajectories of the vector field w.

3.2 Linear Equations on the Moving Surface, Describing the Rapidly Varying Part of the Solution

Let \(\varPhi , u^\pm ,w^\pm ,P_0^\pm \) be the smooth solution of the free boundary problem, formulated above. Let us consider 3D surface \(\varOmega \subset R^4\), defined by the equation \(\varPhi (x,t)=0\) (trace of the moving surface \(M_t\)). Note that the field \(\partial /\partial t\), generally speaking, is not tangent to this surface; we denote by \(\partial _t\) the projection of \(\partial /\partial t\) to the tangent plane to \(\varOmega \). We denote by \(\hat{w}\) the projection of the field \(w|_{M_t}\) to the tangent plane to \(M_t\) and let \(\mathcal B\) be the second fundamental form operator (that is the operator in the tangent plane with the eigenvalues equal to the principle curvatures and eigenvectors equal to the principle directions). The rapidly varying part of the main term of asymptotic solution—vector field h on the surface \(M_t\)—satisfies the Cauchy problem

$$\begin{aligned} \mathscr {L}_{\partial _t}h +\alpha y\frac{\partial h}{\partial y}+\hat{\nabla }_{\hat{w}}h-w_n\mathscr {B}h=\frac{1}{2}(\mu +\nu )\frac{\partial ^2h}{\partial y^2}, \end{aligned}$$
(12)
$$\begin{aligned} h|_{t=0}=\varPi (u_0-u_0^-)|_{M_0}. \end{aligned}$$
(13)

Here \(\hat{\nabla }\) denotes the covariant derivative on the surface \(M_t\), \(\mathscr {L}\) denotes Lie derivative on \(\varOmega \), \(\varPi \) is the projection to the tangent plane to \(M_t\),

$$ \alpha =\frac{\partial }{\partial \varPhi }|_{M_t}\left( \frac{\partial \varPhi }{\partial t}+(w,\nabla \varPhi )\right) . $$

Remark 3

Vector field h satisfies the Cauchy problem for the linear parabolic system—evidently, this system has a unique solution for any finite time interval.

Remark 4

Equation (12) is analogous to the advection-diffusion equations on the moving surface \(M_t\). Advection is governed by the field \(\hat{w}\), while diffusion is presented by the terms in the right hand site, containing viscosity. The system additionally contains the second fundamental form operator \(\mathcal B\); the corresponding term describes the influence of the curvature of \(M_t\) on the growth or the decay of the field h. In particular, in the area of hyperbolic points the corresponding term induces the growth of the one component of the field and the decay of the another component, while in the area of elliptic points both components have the tendency to grow or to decrease simultaneously.

3.3 Asymptotic Solution of the Cauchy Problem

General structure of nondegenerate mode is described by the following theorem.

Theorem 1

Let for \(t\in [0,T]\) there exists a smooth solution \(\varPhi ,w^\pm ,u^\pm ,P_0^\pm \) to the free boundary problem (7)–(11) as well as the smooth solution of the corresponding linearized problem (see (36)–(40)) and the analogous problem with a smooth right hand side. Then there exist formal series

$$\begin{aligned} B=\sum _{k=0}^\infty \varepsilon ^k B_k\left( \frac{\varPhi (x,t)}{\varepsilon },x,t\right) ,\quad V=\sum _{k=0}^\infty \varepsilon ^k V_k\left( \frac{\varPhi (x,t)}{\varepsilon },x,t\right) ,\nonumber \\ P=\sum _{k=0}^\infty \varepsilon ^k P_k\left( \frac{\varPhi (x,t)}{\varepsilon },x,t\right) , \end{aligned}$$
(14)

satisfying the Cauchy problem (1)–(4) with the initial fields, satisfying (5). Moreover,

$$ \lim _{y\rightarrow \pm \infty }V_0=\frac{1}{2}(u^\pm +w^\pm ),\quad \lim _{y\rightarrow \pm \infty }B_0=\frac{1}{2}(u^\pm -w^\pm ), $$

the function \(P_0\) does not depend on the rapid variable y and coincides with \(P_0^\pm \) in \(D_t^\pm \). On the surface \(M_t\) the tangent \(\hat{V},\hat{B}\) and normal \(V_n,B_n\) components of the fields \(V_0, B_0\) have the form

$$\begin{aligned} V_n(x,t)=\frac{1}{2}(u_n+w_n),\quad B_n(x,t)=\frac{1}{2}(u_n-w_n), \end{aligned}$$
(15)
$$\begin{aligned} \hat{V}(y,x,t)=\frac{1}{2}(h(y+c(x,t))+u^-(x,t)+\hat{w}(x,t)), \end{aligned}$$
(16)
$$\begin{aligned} \hat{B}(y,x,t)=\frac{1}{2}(h(y+c(x,t))+u^-(x,t)-\hat{w}(x,t)). \end{aligned}$$
(17)

Here c(x, t) is a smooth function, satisfying the equation, obtained in Sect. 5 (see. (34)).

Remark 5

Initial conditions for the function c depend on the vectors \(\frac{\partial }{\partial \varepsilon }|_{\varepsilon =0}B^0\), \(\frac{\partial }{\partial \varepsilon }|_{\varepsilon =0}V^0\). So the asymptotic solution is “asymptotically unstable”—small (\(O(\varepsilon )\)) variation of initial conditions leads to the big (O(1)) variation of the asymptotics. However, the limit fields \(B_0^\pm , V_0^\pm \) and the profile h(y) do not change as a result of such a variation—\(O(\varepsilon )\)—variation of the initial conditions lead to the \(O(\varepsilon )\)—shift of the surface of the jump.

4 Formulation of the Results. Degenerate Mode

Here we describe the structure of asymptotic solution, corresponding to the degenerate mode. Just as in the nondegenerate case, the main term of asymptotic solution is defined from the free boundary problem and from the system of equations on the moving surface. However, the latter equations now are essentially more complicated—they form a nonlinear system for two vector fields and one scalar function. The main property of this system—the possibility of the instantaneous growth of the magnetic field.

4.1 Free Boundary Problem for the Limit Fields

Let the conditions (6) be fulfilled; let us consider the following free boundary problem. On the finite time interval \(t\in [0,T]\) we seek for smooth compact surface \(M_t\in R^3\), vector fields \(B_0^\pm (x,t),V_0^\pm (x,t)\) and scalar functions \(P_0^\pm (x,t)\), defined in the internal (\(D_t^-\)) and external (\(D_t^+\)) domains with respect to \(M_t\) and satisfying for \(x\in D_t^\pm \) the following equations

$$\begin{aligned}&\frac{\partial V_0^\pm }{\partial t}+(V_0^\pm ,\nabla )V_0^\pm -(B_0^\pm ,\nabla )B_0^\pm +\nabla P_0^\pm =0,\end{aligned}$$
(18)
$$\begin{aligned}&\frac{\partial B_0^\pm }{\partial t}+(V_0^\pm ,\nabla )B_0^\pm -(B_0^\pm ,\nabla )V_0^\pm =0, \quad&(\nabla , V_0^\pm )=(\nabla , B_0^\pm )=0,\qquad \qquad \end{aligned}$$
(19)

boundary conditions

$$\begin{aligned} B_n=0,\quad [P_0]=0,\quad [V_n]=0,\quad -\frac{\partial \varPhi }{\partial t}=V_n,\quad x\in M_t \end{aligned}$$
(20)

and initial conditions

$$\begin{aligned} \varPhi |_{t=0}=\varPhi _0(x),\quad V_0^\pm |_{t=0}=V^{0,\pm },\quad B_0^\pm |_{t=0}=B^{0,\pm },\quad x\in D^\pm _0. \end{aligned}$$
(21)

Here \(\varPhi (x,t)\) equals the distance (with the appropriate sign) from the surface \(M_t\) in the normal direction, \(V_n=(V_0,\nabla \varPhi )|_{M_t}\), \(B_n=(B_0,\nabla \varPhi )|_{M_t}\), the symbol [f] denotes the jump of f:

$$ [f]=f^+|_{M_t}-f^{-}|_{M_t}. $$

Remark 6

Surface \(M_t\) is defined by the equation \(\varPhi (x,t)=0\); the boundary condition \(-\frac{\partial \varPhi }{\partial t}=V_n\) means that the surface moves along the trajectories if the field \(V_0\).

4.2 Equations on the Moving Surface, Describing the Rapidly Varying Fields

Let \(\varPhi , V_0^\pm ,B_0^\pm ,P_0^\pm \) be the smooth solution of the free boundary problem, formulated in the previous section. The rapidly varying part of the solution—two vector fields v, b on the surface \(M_t\)—satisfy the Cauchy problem

$$\begin{aligned}&\mathscr {L}_{\partial _t}v+\hat{\nabla }_{v}v+\kappa y\frac{\partial v}{\partial y}-2V_n\mathscr {B}v-\hat{\nabla }_{b}b+\hat{\nabla }\mathscr {P}=a\frac{\partial b}{\partial y}+\nu \frac{\partial ^2 v}{\partial y^2},\end{aligned}$$
(22)
$$\begin{aligned}&\mathscr {L}_{\partial _t}b +\{v,b\}+\kappa y\frac{\partial b}{\partial y}=a\frac{\partial v}{\partial y}+\mu \frac{\partial ^2 b}{\partial y^2},\end{aligned}$$
(23)
$$\begin{aligned}&(\hat{\nabla }, v)=0,\quad (\hat{\nabla },b)+\frac{\partial a}{\partial y}=0, \end{aligned}$$
(24)
$$\begin{aligned} v|_{t=0}=\varPi (V^0|_{M_0}),\quad b|_{t=0}=\varPi (B^0|_{M_0}). \end{aligned}$$
(25)

Here \(\mathscr {P}=(P_0+\frac{1}{2}V_n^2)|_{M_t}\), a is a smooth scalar function, \(\kappa =\frac{\partial }{\partial \varPhi }|_{M}\left( \frac{\partial \varPhi }{\partial t}+(V_0,\nabla \varPhi )\right) \).

Remark 7

Equations (22) are close to the Prandtl equations for the boundary layer and their generalizations, describing vortex structures in the fluid (see [16–18]).

Remark 8

Function a can be excluded from the system—it can be expressed from the last equation:

$$ a(y,x,t)=a^-(x,t)+\int \limits _{-\infty }^y(\hat{\nabla },b)dy. $$

The limit function \(a^-(x,t)\) can be computed from the linearized free boundary problem; at the initial instant of time this function has the form \(\left( \frac{\partial }{\partial \varepsilon }|_{\varepsilon =0}(B^{0,-},\nabla \varPhi _0\right) \). So the \(O(\varepsilon )\)-variation of the initial field implies the variation of the function a and the vector fields v, b—the main term of asymptotic solution. Moreover, using the form of (22) it is easy to see, that even if at the initial instant of time the magnetic field is small (\(B^0=O(\varepsilon )\)), during arbitrary small time \(t>0\) the field grows to the value O(1). The same effect (instantaneous growth of the magnetic field, caused be the jump of the velocity field )—was described in the paper [13] in linear approximation. Note that, analogous to the linear situation, the magnetic field in this case is localized in the small vicinity of \(M_t\) (evidently, \(B_0^\pm =0\) if \(B^0=O(\varepsilon )\)).

4.3 Asymptotic Solution of the Cauchy Problem

The structure of the degenerate mode is described by the following theorem.

Theorem 2

Let for \(t\in [0,T]\) there exists smooth solution \(\varPhi ,V_0^\pm ,B_0^\pm ,P_0^\pm \) for the free boundary problem (18)–(21), as well as the smooth solution for the linearized problem with the smooth right hand side. Let the system (22)–(25) admits smooth solution h, v, a. Then there exist power series

$$\begin{aligned} B=\sum _{k=0}^\infty \varepsilon ^k B_k\left( \frac{\varPhi (x,t)}{\varepsilon },x,t\right) ,\quad V=\sum _{k=0}^\infty \varepsilon ^k V_k\left( \frac{\varPhi (x,t)}{\varepsilon },x,t\right) ,\nonumber \\ P=\sum _{k=0}^\infty \varepsilon ^k P_k\left( \frac{\varPhi (x,t)}{\varepsilon },x,t\right) , \end{aligned}$$
(26)

satisfying the Cauchy problem (1)–(4) with the initial fields, satisfying (6). Moreover,

$$ \lim _{y\rightarrow \pm \infty }V_0=V_0^\pm ,\quad \lim _{y\rightarrow \pm \infty }B_0=B_0^\pm , $$

the function \(P_0\) does not depend on y and coincides in the domains \(D_t^\pm \) with \(P_0^\pm \). On the surface \(M_t\) the tangent \(\hat{V},\hat{B}\) and the normal \(V_n,B_n\) components of the fields \(V_0, B_0\) have the form

$$\begin{aligned} V_n(x,t)=(V_0^+,\nabla \varPhi )|_{M_t},\quad B_n(x,t)=0, \end{aligned}$$
(27)
$$\begin{aligned} \hat{V}(y,x,t)=v(y+d(x,t)),\quad \hat{B}(y,x,t)=b(y+d(x,t)). \end{aligned}$$
(28)

Here d(x, t) is the smooth function, which can be expressed in terms of the limit fields \(V_1^\pm , B_1^\pm \).

5 Construction of the Asymptotic Solution

Here we give the proof of the Theorem 1; the proof of the Theorem 2 is analogous.

5.1 Division in the Asymptotic Modes

We seek for the formal asymptotic solution of the Cauchy problem (1)–(4) (i.e. for the the formal series, satisfying the corresponding equations and initial conditions) in the form (14); we assume that \(\varPhi (x,t)\), \(B_k(y,x,t)\), \(V_k(y,x,t)\), \(P_k(y,x,t)\) are smooth functions of all arguments, and \(B_k\rightarrow B_k^\pm (x,t)\), \(V_k\rightarrow V_k^\pm (x,t)\), \(P_k\rightarrow P_k^\pm (x,t)\) as \(y\rightarrow \pm \infty \) faster than any power of y. We denote by \(M_t\) the surface, defined by the equation \(\varPhi (x,t)=0\); we assume that this surface is smooth and compact, \(\varPhi <0\) inside \(M_t\) and in certain vicinity of this surface \(|\varPhi (x,t)|\) coincides with the distance from the point x to \(M_t\) in the normal direction (we can always provide this property with the help of re-expansions in (14)). Moreover, we assume that \(|\nabla \varPhi |\ge C>0\) in \(R^3\). Further we will usually omit the index t in the notation of the surface.

Let us substitute the series (14) to the equations (1) and consider the summands in the both sides of equality, containing equal powers of \(\varepsilon \). The summands, containing \(\varepsilon ^{-1}\), lead to the equation

$$\begin{aligned} \Big (\frac{\partial \varPhi }{\partial t}+(V_0,\nabla \varPhi )\Big )\frac{\partial B_0}{\partial y}-(B_0,\nabla \varPhi )\frac{\partial V_0}{\partial y}=0, \end{aligned}$$
(29)
$$ \Big (\frac{\partial \varPhi }{\partial t}+(V_0,\nabla \varPhi )\Big )\frac{\partial V_0}{\partial y}-(B_0,\nabla \varPhi )\frac{\partial B_0}{\partial y}+\nabla \varPhi \frac{\partial P_0}{\partial y}=0. $$
$$ \frac{\partial }{\partial y}(B_0,\nabla \varPhi )=0,\;\;\; \frac{\partial }{\partial y}(V_0,\nabla \varPhi )=0. $$

Note that the left hand sides of these equalities decay rapidly as \(|y|\rightarrow \infty \), hence, due to the well-known estimate [19]

$$ F(x,y)=F(x,y)|_{x\in M,y=\varPhi /\varepsilon }+\varPhi \left( \frac{\partial }{\partial \varPhi }F(x,y)\right) |_{x\in M,y=\varPhi /\varepsilon }+\dots $$
$$ =F(x,y)|_{x\in M,y=\varPhi /\varepsilon }+\varepsilon y\left( \frac{\partial }{\partial \varPhi }F(x,y)\right) |_{x\in M,y=\varPhi /\varepsilon }+\dots =F(x,y)|_{x\in M,y=\varPhi /\varepsilon }+O(\varepsilon ) $$

these summands can be \(\mathrm {mod}O(\varepsilon )\) restricted to the surface M. Note that this equality is obtained with the help of Taylor expansion with respect to the distance from M; in further approximations with respect to \(\varepsilon \) we will take into account all the summands of this expansion.

Multiplying (29) by the vector \(\nabla \varPhi \), we obtain

$$ \frac{\partial P_0}{\partial y}|_M=0. $$

We will have to prolong functions, rapidly decaying in y, from M to the vicinity of this surface. We will use the following rule: the functions and the fields will be prolonged in such a way, that they will not depend on \(\varPhi \) (i.e. will satisfy equations \(\nabla _{\nabla \varPhi }F=0\)). In particular, as \(\frac{\partial P_0}{\partial y}|_M=0\), we will assume that this derivative vanishes everywhere.

Note that, if (29) has nontrivial solutions, the determinant of \(2\times 2\) matrix

$$\begin{aligned} \left( \frac{\partial \varPhi }{\partial t}+(V_0,\nabla \varPhi )\right) |_{M} \quad \quad (B_0,\nabla \varPhi )|_{M}\\ (B_0,\nabla \varPhi )|_{M}\quad \quad \left( \frac{\partial \varPhi }{\partial t}+(V_0,\nabla \varPhi )\right) |_{M} \end{aligned}$$

vanishes; this implies one of the two following conditions.

  1. 1.

    The rang of this matrix is equal to unity; in this case we have on M

    $$ \frac{\partial B_0}{\partial y}=\pm \frac{\partial V_0}{\partial y},\quad \frac{\partial \varPhi }{\partial t}+(V_0,\nabla \varPhi )=\pm (B_0,\nabla \varPhi ). $$
  2. 2.

    The rang is equal to zero; in this case

    $$ \frac{\partial \varPhi }{\partial t}+(V_0,\nabla \varPhi )|_M=0,\quad (B_0,\nabla \varPhi )|_M=0, $$

    and we have no conditions on the vectors \(\frac{\partial B_0}{\partial y}, \frac{\partial V_0}{\partial y}\). These two cases correspond to nondegenerate and degenerate modes; consider the first case. We chose the mode, corresponding to the “+” sign; in another words, we assume that the initial fields satisfy (5). So (1) are fulfilled up to \(\mathrm {mod}O(1)\), if

    $$ \frac{\partial P_0}{\partial y}=0,\quad \frac{\partial }{\partial y}(V_0-B_0)=0, $$
    $$ \frac{\partial }{\partial y}(V_0,\nabla \varPhi )=0,\quad \frac{\partial }{\partial y}(B_0,\nabla \varPhi )=0, $$
    $$ \left( \frac{\partial \varPhi }{\partial t}+(V_0-B_0,\nabla \varPhi )\right) |_{M}=0. $$

5.2 Free Boundary Problem for the Limit Fields

Now let us equate summands, containing \(\varepsilon ^0\), in both sides of (1). Consider first these equations in the domains \(D_{\pm }\), i.e. in the points which do not belong to M. At these points \(y\rightarrow \infty \), so in the corresponding equalities one can \(\mathrm {mod}O(\varepsilon ^{\infty })\) pass to the limit \(y\rightarrow \pm \infty \); thus we have

$$ \frac{\partial V_0^\pm }{\partial t}+(V_0^\pm ,\nabla V_0^\pm )-(B_0^\pm ,\nabla )B_0^\pm +\nabla P_0=0, $$
$$ \frac{\partial B_0^\pm }{\partial t}+(V_0^\pm ,\nabla )B_0^\pm -(B_0^\pm ,\nabla V_0^\pm )=0, $$
$$ (\nabla ,V_0^\pm )=(\nabla , B_0^\pm )=0. $$

Let us denote \(u=V_0+B_0\), \(w=V_0-B_0\); in the previous section we showed, that w does not depend on y. Consider the sum and the difference of the equations for \(V_0^\pm \) an \(B_0^\pm \); evidently we obtain (7). As \(P_0, w\) do not depend on y, at the points of M \(P_0^+=P_0^-\), \(w^+=w^-\); moreover, the function \((V_0+B_0,\nabla \varPhi )\) also does not depend on y, hence \(u_n^+=u_n^-\), where \(u_n^\pm \) denote the limits of the normal components of the vector u in the points of M. Thus the fields \(u^\pm ,w\) and the functions \(P_0\), \(\varPhi \) satisfy (10) (we remind, that the equation for the function \(\varPhi \) was obtained earlier). Evidently, initial conditions (11) are also fulfilled. Further we will assume that \(\varPhi ,w,u^\pm , P_0\) is a smooth solution of (7)–(11).

5.3 Equations on the Moving Surface

Let us return to the equations, appearing from the summands, multiplied by \(\varepsilon ^0\). Due to the equations of the previous section, the left hand sides of these equations vanish as \(y\rightarrow \pm \infty \), hence they can be restricted \(\mathrm {mod}O(\varepsilon )\) to the surface M. We have

$$\begin{aligned}&\frac{\partial V_0}{\partial t}+(V_0,\nabla )V_0+(B_0, \nabla \varPhi )\left( \frac{\partial V_1}{\partial y}-\frac{\partial B_1}{\partial y}\right) \\&\quad -(B_1,\nabla \varPhi )\frac{\partial B_0}{\partial y}+(V_1, \nabla \varPhi ) \frac{\partial V_0}{\partial y}-(B_0,\nabla )B_0+\nabla P_0 +\nabla \varPhi \frac{\partial P_1}{\partial y}\\&\quad +y\frac{\partial }{\partial \varPhi }\left( (\varPhi _t+(V_0,\nabla \varPhi ))\frac{\partial V_0}{\partial y}\right) -y\frac{\partial }{\partial \varPhi }\left( (B_0,\nabla \varPhi )\frac{\partial B_0}{\partial y}\right) -\nu \frac{\partial ^2 V_0}{\partial y^2}=0, \\&\frac{\partial B_0}{\partial t}+(B_0, \nabla \varPhi )\left( \frac{\partial B_1}{\partial y}-\frac{\partial V_1}{\partial y}\right) +\{V_0,B_0\}+(V_1,\nabla \varPhi )\frac{\partial B_0}{\partial y}-(B_1, \nabla \varPhi ) \frac{\partial V_0}{\partial y} \\&\quad +y\frac{\partial }{\partial \varPhi }\left( (\varPhi _t+(V_0,\nabla \varPhi ))\frac{\partial B_0}{\partial y}\right) -y\frac{\partial }{\partial \varPhi }\left( (B_0,\nabla \varPhi )\frac{\partial V_0}{\partial y}\right) -\mu \frac{\partial ^2 B_0}{\partial y^2}=0, \end{aligned}$$
$$ \frac{\partial }{\partial y}(V_1,\nabla \varPhi )+(\nabla ,V_0)=0, $$
$$ \frac{\partial }{\partial y}(B_1,\nabla \varPhi )+(\nabla ,B_0)=0. $$

Here we took into account the summands of order O(1), neglected in the previous approximation (second summands of the Taylor expansion with respect to the distance form M).

Let us rewrite the equations in the following form:

$$ (B_0,\nabla \varPhi )\left( \frac{\partial B_1}{\partial y}-\frac{\partial V_1}{\partial y}\right) +(V_1,\nabla \varPhi )\frac{\partial B_0}{\partial y}-(B_1,\nabla \varPhi )\frac{\partial V_0}{\partial y}=F, $$
$$ (B_0,\nabla \varPhi )\left( \frac{\partial V_1}{\partial y}-\frac{\partial B_1}{\partial y}\right) +(V_1,\nabla \varPhi )\frac{\partial V_0}{\partial y}-(B_1,\nabla \varPhi )\frac{\partial B_0}{\partial y}+\nabla \varPhi \frac{\partial P_1}{\partial y}=G, $$
$$\begin{aligned} \frac{\partial }{\partial y}(V_1,\nabla \varPhi )=g\quad \frac{\partial }{\partial y}(B_1,\nabla \varPhi )=f,\quad f-g=0 \end{aligned}$$
(30)

(the last equality follows from the equation \((\nabla ,w)=0\) in \(R^3\)).

Let us project the second vector equation to the tangent plane to M and then let us consider the sum and the difference of the obtained vector equations. Taking into account that \(\frac{\partial V_0}{\partial y}=\frac{\partial B_0}{\partial y}\) as well as (29), we obtain

$$\begin{aligned} 2(B_0,\nabla \varPhi )\frac{\partial w_1}{\partial y}=\varPi (G)-F, \end{aligned}$$
(31)
$$\begin{aligned} 2(w_1,\nabla \varPhi )\frac{\partial V_0}{\partial y}=\varPi (G)+F, \end{aligned}$$
(32)

where \(w_1=V_1-B_1\) and \(\varPi \) is the projector to the tangent plane. Projecting the same equation to the normal direction to M, we obtain

$$\begin{aligned} \frac{\partial P_1}{\partial y}=(G,\nabla \varPhi ). \end{aligned}$$
(33)

Proposition 1

Equation (32) can be reduced to the form

$$\begin{aligned} \mathscr {L}_{\partial _t}H +(\alpha y+\beta )\frac{\partial H}{\partial y}+\hat{\nabla }_{\hat{w}}H-w_n\mathscr {B}H=\frac{1}{2}(\mu +\nu )\frac{\partial ^2 H}{\partial y^2}, \end{aligned}$$
(34)

where \(H=\varPi (u-u^-)|_{M}\), \(\alpha =\frac{\partial }{\partial \varPhi }|_{M}\left( \frac{\partial \varPhi }{\partial t}+(w,\nabla \varPhi )\right) \), \(\beta =(w_1,\nabla \varPhi )\).

Proof

First we prove that the vector F is tangent to M; let us compute its normal component. Taking into account the equality \((\nabla \varPhi ,\partial {V_0}/{\partial y})=0\), after direct computations we obtain

$$ -(F,\nabla \varPhi )=\left( \frac{\partial }{\partial t}+(w,\nabla )\right) (B_0,\nabla \varPhi )-(B_0,\nabla )\left( \frac{\partial \varPhi }{\partial t}+(w,\nabla \varPhi )\right) . $$

Note that the first summand is independent of y; taking into account the equality \((\varPhi _t+(w,\nabla \varPhi ))|_M=0\), one can rewrite the second summand in the form

$$ -(B_0,\nabla \varPhi )\frac{\partial }{\partial \varPhi }|_{M}\left( \frac{\partial \varPhi }{\partial t}+(w,\nabla \varPhi )\right) . $$

This function is also independent of y. Note that the vector F vanishes as \(|y|\rightarrow \infty \), hence \((F,\nabla \varPhi )=0\).

Thus (32) can be rewritten in the form

$$ 2(w_1,\nabla \varPhi )\frac{\partial V_0}{\partial y}=\varPi (G+F) $$

Using the explicit expressions for F and G, we have

$$ \varPi \left( \frac{\partial u}{\partial t}+(w,\nabla )u+\nabla P_0+y\alpha \frac{\partial u}{\partial y}-\frac{\mu +\nu }{2}\frac{\partial ^2 u}{\partial y^2}\right) +\beta \frac{\partial u}{\partial y}=0. $$

Let us subtract from this equation the equality

$$ \varPi \left( \frac{\partial u^-}{\partial t}+(w,\nabla )u^-+\nabla P_0^-\right) |_{M}=0. $$

Note that \(P_0\) does not depend on y and the vector \(\partial u/\partial y\) is tangent to M. Using these facts, we obtain

$$ \varPi \left( \frac{\partial \hat{H}}{\partial t}+(w,\nabla )\hat{H}\right) +(\alpha y+\beta )\frac{\partial H}{\partial y}=\frac{\mu +\nu }{2}\frac{\partial ^2 H}{\partial y^2}, $$

where \(\hat{H}=u-u^-\), \(H=\hat{H}|_M\) (note, that, according to (10), this vector is tangent to M). Further computation of the projection is quite analogous to the calculation, presented in [20]. Namely: we expand the vector \(\partial /\partial t\) to the tangent and normal components to the 3D surface \(\cup _t M_t\subset R^4\). Cumbersome computations lead to the formulae

$$ \varPi \left( \frac{\partial \hat{H}}{\partial t}+(w,\nabla )\hat{H}\right) =\varPi \left( \{\frac{\partial }{\partial t},\hat{H}\}+(\hat{w},\nabla )\hat{H}+w_n\frac{\partial \hat{H}}{\partial \varPhi }\right) $$
$$ =\{\partial _t,H\}+\hat{\nabla }_{\hat{w}}H+\varPi \left( \left( \frac{\partial \varPhi }{\partial t}+w_n\right) \frac{\partial \hat{H}}{\partial \varPhi }-\frac{\partial \varPhi }{\partial t}(\hat{H},\nabla )\frac{\partial }{\partial \varPhi }\right) $$
$$ =\mathscr {L}_{\partial _t}H+\hat{\nabla }_{\hat{w}}H-w_n\mathscr {B}H. $$

Equation (34) contains the coefficient \(\beta \), depending on the first correction \(w_1\); so the equations for the main part of the asymptotics appear to be linked with the equations, appearing in the next approximations. However, the form of the function h appears to be independent on the correction \(w_1\)—the latter function influences only the shift of the argument y, i.e. the small (of order \(\varepsilon \)) shift of the surface \(M_t\). Formally: the following assertion can be verified directly.

Proposition 2

Equation (34) is invariant with respect to the transformation

$$ H(y,x,t)\rightarrow H(y+c(x,t),x,t),\quad \beta \rightarrow \beta +\partial _t(c)+(\hat{w},\nabla )c. $$

Corollary 1

Let h(y, x, t) be the solution of the Cauchy problem (12), while the scalar function c(x, t) on the surface \(M_t\) satisfies the equation

$$\begin{aligned} \partial _t(c)+(\hat{w},\nabla )c+\beta (x,t)=0,\quad c(0)=0. \end{aligned}$$
(35)

Then the vector field \(H(y,x,t)=h(y+c,x,t)\) satisfies (30) and \(H|_{t=0}=(u_0-u_0^-)|_{M_0}\).

5.4 Construction of the Main Part of the Asymptotic Solution and Description of the Further Terms

The free boundary problem determines the functions \(B_0^\pm \), \(V_0^\pm , \varPhi , w,P_0\) and \((u,\nabla \varPhi )\). In order to construct the main term of the asymptotics one has to prolong the vector field h to the entire space and to compute the phase shift c(x, t). Note that \(h\rightarrow 0\) as \(y\rightarrow -\infty \) and \(h\rightarrow (u^+-u^-)|_{M}\) as \(y\rightarrow -\infty \); so the vector field h can be represented in the form

$$ h=\eta (y)(u^+-u^-)|_{M}+h_0(y,x,t),\quad \eta (y)=\frac{1}{2}(1+\tanh y),\quad h_0\rightarrow 0 \quad as \quad y\rightarrow \pm \infty . $$

Let us define the field U(y, x, t) in the entire space as follows

$$ U(y,x,t)=\eta (y)(u^+(x,t)-u^-(x,t))+u_0(y,x,t), $$

where the decaying function \(u_0\) is the standard prolongation of \(h_0\) ((\(\nabla _{\nabla \varPhi }u_0=0\), \(u_0|_M=h_0\))). Now the vector field u is defined in the entire space up to the shift of the argument y (\(u(y,x,t)=U(y+c,x,t)\)). In order to determine this shift, we consider the \(O(\varepsilon ^1)\)-approximation. Considering the corresponding equations in the domains \(D_{\pm }\) and passing to the limits \(y\rightarrow \pm \infty \), we obtain

$$ \frac{\partial V_1^\pm }{\partial t}+(V_1^\pm ,\nabla ) V_0^\pm +(V_0^\pm ,\nabla ) V_1^\pm -(B_0^\pm ,\nabla )B_1^\pm -(B_1^\pm ,\nabla )B_0^\pm +\nabla P_1^\pm =0, $$
$$ \frac{\partial B_1^\pm }{\partial t}+(V_1^\pm ,\nabla )B_0^\pm +(V_0^\pm ,\nabla )B_1^\pm -(B_0^\pm ,\nabla )V_1^\pm -(B_1^\pm ,\nabla )V_0^\pm =0, $$
$$ (\nabla ,V_1^\pm )=(\nabla , B_1^\pm )=0. $$

The sum and the difference of these equations have the form

$$\begin{aligned}&\frac{\partial w_1^\pm }{\partial t}+(u^\pm ,\nabla )w_1^\pm +(u_1^\pm ,\nabla )w+\nabla P_1^\pm =0,\end{aligned}$$
(36)
$$\begin{aligned}&\frac{\partial u_1^\pm }{\partial t}+(w^\pm ,\nabla )u_1^\pm +(w,\nabla )u_1^\pm +\nabla P_1^\pm =0,\end{aligned}$$
(37)
$$\begin{aligned}&(\nabla ,u_1^\pm )=(\nabla ,w_1^\pm )=0, \end{aligned}$$
(38)

where \(u_1=V_1+B_1\). Boundary conditions on the surface \(M_t\) come from (30, 33): integrating them with respect to y, we obtain

$$\begin{aligned}{}[w_1^n]=&0,\quad [w_1]=-\frac{1}{(B_0,\nabla \varPhi )}\int \limits _{-\infty }^\infty Fdy,\quad [u_1^n]=\int \limits _{-\infty }^\infty (f+g)dy, \nonumber \\&\quad \quad \; [P_1]=\int \limits _{-\infty }^\infty (G,\nabla \varPhi )dy. \end{aligned}$$
(39)

Evidently, the initial conditions have the form

$$\begin{aligned} u_1^\pm |_{t=0}=(V_1^0+B_1^0)^\pm ,\quad w_1^\pm |_{t=0}=(V_1^0-B_1^0)^\pm ,\quad V_1^0=\frac{\partial V^0}{\partial \varepsilon }|_{\varepsilon =0},\quad B_1^0\frac{\partial B^0}{\partial \varepsilon }|_{\varepsilon =0}. \end{aligned}$$
(40)

Remark 9

Boundary conditions (39) do not depend on the phase shift c(x, t).

Let \(w_1^\pm , u_1^\pm , P_1^\pm \) be the smooth solution of the problem (36)–(40); substituting the function \(\beta = (w_1,\nabla \varPhi )|_{M}\) (which is independent of y) to (35) and solving this equation, we obtain the phase shift c(x, t). Now the main term of asymptotic solution is described completely.

The corrections can computed analogously; in order to describe the k-th summand of the asymptotic series, one has to take into account three approximations—\(O(\varepsilon ^{k-1})\), \(O(\varepsilon ^{k})\) and \(O(\varepsilon ^{k+1})\).