Abstract
According to the present pluralism in mathematical logic, I translate from classical logic to non-classical logic the predicates of the classical square of opposition. A similar unique structure is obtained. In order to support this new logical structure, I investigate on the rich legacy of the non-classical arguments presented by ingenuity by several authors of scientific theories. A comparative analysis of their ways of arguing shows that each of these theories is severed in two parts; the former one proves a universal predicate by an ad absurdum proof. This conclusion of every theory results to be formalised by the A thesis of the new logical structure. Afterwards, this conclusion is changed in the corresponding affirmative predicate, which in the latter part plays the role of a new hypothesis for a deductive development. This kind of change is the same suggested by Leibniz’ principle of sufficient reason. Instead, Markov’s principle results to be a weaker logical change, from the intuitionist thesis I in the affirmative thesis I. The relevance of all the four theses of the new logical structure is obtained by studying all the conversion implications of intuitionist predicates. In the Appendix, I analyse as an example of the above theories, Markov’s presentation of his theory of real numbers.
Access provided by Autonomous University of Puebla. Download chapter PDF
Similar content being viewed by others
Keywords
Mathematics Subject Classification
1 The Present Pluralism in Logic
Even few decades ago the kinds of logic which differ from the classical one were called ‘deviant logic’.Footnote 1 Instead, at present time a plethora of kinds of logic appear to be relevant at least in some particular situations.Footnote 2
In philosophy we can trace back this logical pluralism to the ancient times. A recent study on Aristotle’s logic concludes: “Thus the first logic was at least in the spirit of an intuitionist logic” [43], which is the most relevant non-classical logic. In last century the mathematical logicians introduced a formal pluralism in mathematical logic. The works by Glyvenko, Kolmogorov and Goedel started to put on the same par of classical logic the intuitionist one [21]. Some more studies established a borderline between classical logic and almost all kinds of non-classical logic; this borderline is constituted by the failure of, better than the law of the excluded middle, the law of double negation [18, 22, 37, 38, 42]; hence, a doubly negated sentence which is not equivalent to the corresponding affirmative sentence (= DNS) belongs to non-classical logic,Footnote 3 whose a first instance is intuitionist logic.
Let us remark that a doubly negated sentence may be a DNS for several reasons; within scientific theories the most important reason is the lack of operative-experimental evidence supporting the corresponding affirmative sentence. This test is easily applied to sentences belonging to scientific theories (which usually include, beyond affirmative sentences about experimental data, also DNSs for theoretical reasons). Hence, this test when applied to a scientific text reveals the author’s choice on the kind of mathematical logic governing his theory. Notice that even a sole, essential DNS plays a discriminating role in an argument or in a text, its presence entails that this argument or this text is governed by non-classical logic. This crucial test, by relying on the evidence of a sentence, links the variety of the kinds of logic to reality in an unprecedented way. No surprise if in the following some important consequences will result.
In the following Sect. 2 the three translations from classical logic to non-classical logic are exploited for defining a SO-like. In order to support such a logical structure, in Sect. 3 I will investigate on the rich legacy of the arguments manifested by the original texts of past scientific theories, through their use of DNSs belonging to non-classical logic. In fact, each of these theories is severed in two parts; in the former part several DNSs play essential roles. In this part a final ad absurdum proof states a doubly negated predicate of universal validity on all the cases at issue. In Sect. 4 the conclusions of the more relevant theories are quoted and then formalised in logical terms; all them result to be equivalent to a non-classical predicate which is the same of the predicate formalising Leibniz’ principle of sufficient reason. In Sect. 5 I illustrate how the author changes this conclusion in the corresponding affirmative predicate, that he then considers as a new hypothesis-axiom from which in the latter part of the theory he deductively develops all consequences according to classical logic; this change is formally the same as the change from the principle of sufficient reason to the corresponding affirmative statement. In Sect. 6 I will take an advantage from Markov’s paper on the theory of constructive mathematics, for investigating on what justifies an author of the above theories to change the kind of logic. Markov performed a similar change of a doubly negated existential predicate, which is both a decidable one and proved by an ad absurdum proof, in the corresponding affirmative predicate (Markov’s principle). In Sect. 7 this change is shown to be a change of the I thesis of the SO-like in the corresponding I of SO, whereas the principle of sufficient reason, together with all previous changes, does the same for the stronger A thesis. In Sect. 8 the list of all valid conversion implications between the couple of intuitionist predicates shows the relevance of the four theses of the SO-like among all the intuitionist predicates. In the Appendix Markov’s paper will be analysed as an example of the above theories through its DNSs.
2 Translations of the Square of Opposition in Non-classical Logic
Let us recall that by introducing in various ways double negations Glyvenko-Goedel, Kolmogorov and Kuroda suggested easy rules for translating predicates of classical logic in the corresponding ones in both intuitionist logic and minimal logic. The first translation adds two negations to each prime and substitutes (in shortened notation) ¬∀¬ for the existential quantifier ∃ (plus the negation of the de Morgan version of the LEM). The second translation is obtained “by simultaneously inserting ¬¬ in front to all subformulas of X (including X itself)”; the third one is obtained by inserting “¬¬ after each occurrence of ∀ and in front to the entire formula”.Footnote 4
Let us apply the above three translations to the four predicates of the classical SO (recall that a triple negation is equivalent to a single negation; the classical thesis O is considered in both versions: “Some S is not P” and “Not every S is P”). I present all them by means of Table 1, where A(x) summarises “S is P”.Footnote 5
I call respectively SOg, SOk, SOq the three SO-like so obtained.
Which relationships among the corresponding predicates of the three translations of SO in non-classical logic? The answer is not easy because whereas the classical predicate logic is a calculus, the intuitionist predicate logic not.
However, there exist some equivalences theorems between the different translations of classical logic to intuitionist predicate logic.Footnote 6 In this logic Pg↔Pk (where P is any predicate). Moreover, the Corollary 3.6 of the previous reference states that negative predicates (i.e. the predicates without ∃ and v and whose prime is negative) enjoys the property  [41, p. 59]. In the following these result will be recalled by merely writing respectively 3.8 and 3.6. In addition, we refer to the list of implications offered by two classical presentations of intuitionism.Footnote 7 As a result, all the three translations of a thesis of SO are mutually equivalent.
[41, p. 59]. In the following these result will be recalled by merely writing respectively 3.8 and 3.6. In addition, we refer to the list of implications offered by two classical presentations of intuitionism.Footnote 7 As a result, all the three translations of a thesis of SO are mutually equivalent.
Table 2 summarises the mutual relationships among the three translations of each thesis of the classical square of opposition in intuitionist logic.
Result 1
The three doubly negated translations of the four theses of SO in intuitionist logic give a unique SO-like which is baptised SOgkq.
3 The Use of Non-classical Logic in the Original Texts of Some Past Scientific Theories
In order to discover the possible use of this SO-like logical structure I will follow Troelstra’s suggestion for a similar question: “to gain further insight into the acquisition of mathematical [and scientific] experience by historical studies” [40, p. 223]. I will investigate on the scientific theories because they represent thinking structures at the highest level as possible of the logical rationality. I will explore the rich legacy of scientific theories in the aim to find out the commonly accepted patterns of arguing suggested by their founders. In other terms, I will deal with an experimental logic whose experimental data are the arguing patterns of some past scientific theories.
Along forty years I investigated on the original texts illustrating scientific theories. I discovered several theories instantiating a specific use of non-classical logic in their respective presentations since they rely on some essential DNSs. These theories are the following ones: L. Carnot’s theories of calculus and geometry, Lagrange’s mechanics, Lavoisier’s chemistry, Avogadro’s atomic theory, S. Carnot’s thermodynamics, Lobachevsky’s non-Euclidean geometry, Galois’ theory of groups, Klein’s Erlangen program, Poincaré’s theory of integer numbers, Einstein’s theory of special relativity, Planck’s theory of quanta, Kolmogorov’s foundation of minimal logic, Church’s thesis, Markov’s theory of constructive functions.
In each text presenting one of these theories the author argues in a little noticed way. By ingenuity he makes use of DNSs so that their mere sequence gives the logical thread of the author’s entire illustration.Footnote 8 Moreover, a comparative analysis on the kind of development shared by the above scientific theories using DNSs, shows that each theory does not begin by stating some axioms from which to draw deductions; that is, it is not organised according to that ideal model of an apodictic (= deductive) theory, which was first suggested by Aristotle, then applied by Euclid in geometry, subsequently confirmed by Newton in mechanics and eventually improved by Hilbert to the model of an axiomatic theory.Footnote 9 Instead, such a theory presents as first a universal problem which at that time was unsolvable by current scientific techniques; to look for a solution requires to find out a new scientific method; which the development of the theory goes to discover.
In the texts written by the above-mentioned scientists a former part concludes, by means of an ad absurdum proof, a doubly negated predicate of an universal validity on all the cases considered by the theory.Footnote 10 Of course, this way of organising a theory is manifestly a non-deductive one. I call such a specific organisation of a theory a problem-based organisation (PO).
By summarising, in his construction of a PO theory a scientist follows a specific logical strategy; he argues by means of DNSs belonging to non-classical logic and he wants to prove by an ad absurdum proof an universal predicate.
Let us recall Leibniz’ logico-philosophical principle of sufficient reason:
Two are the principles of the human mind: the principle of non-contradiction and the principle of the sufficient reason … , [that is] nothing is without reason, or everything has its reason, although we are not always capable of discovering this reason …Footnote 11
The above sentence (“Nothing is without reason”) is a DNS, as the same Leibniz explains why by means of the next sentence: Leibniz wants to conclude “everything has a reason”, but he underlines that not always we have sufficient evidence for affirming with certainty this reason; hence, only the doubly negated sentence holds true. Hence, the above logical strategy may be considered as summarised by Leibniz’ principle of sufficient reason.
4 Qualifying in Formal Terms the Predicate Concluding a Problem-Based Theory
Let us list the universal conclusions of some PO theories (in order to facilitate the reader, I will add to two conclusions their translations in more plain words).
Lobachevsky: “… without leading to any contradiction in the results”; i.e. No contradiction results.Footnote 12
S. Carnot: “… no change of temperature inside the bodies employed for obtaining the motrice power of heat occurs without a change in the volume”.Footnote 13
Poincaré: “If the absence of contradiction of a syllogism whose number is entire implies the absence of contradiction of the following one, one has to not fear any contradiction for each syllogism whose number is entire”.Footnote 14
Kolmogorov: “None of the conclusions of ordinary mathematics that are based on the use outside the domain of the finitary … . can be regarded as firmly established” (and also: “No contradiction from the use of the principle of excluded middle”).Footnote 15
We remark that each above conclusion is formalised by a same logical formula:
Some authors achieved conclusions of a different kind. Avogadro: “[All] The proportions among the quantities in the combinations of the substances do not seem depend other than both the relative number of molecules which combine themselves and the number of the composed molecules which result from them”,Footnote 16 i.e., All proportions among the quantities in the combinations of the substances do not depend other than …”.
Kleene’s statement on Church’s thesis: “Every general recursive function cannot conflict with the intuitive notion which is supposed to complete …” [26, pp. 318–319].
Each of the above statements is formalised by the following formula:
It is a remarkable fact that the latter formula is equivalent to the former formula (6 and 7); both represent the Agkq thesis.
More in general, this fact gives a very important conclusion: a PO theory, searching a new method capable to solve a basic problem, argues according to a sequence of DNSs aimed to eventually obtain an instance of the predicate Agkq.Footnote 17
Result 2
A PO theory is a goal-oriented logical theory to state an universal predicate concluding the last ad absurdum proof of its former part. This predicate is formalised by the thesis Agkq of the SOgk.Footnote 18
Let us add that a Leibniz’ principle of sufficient reason (“Nothing is without reason”) may be formalised by calling S an “event” and P “connected to some events”; the following formula is obtained:
Result 3
Even the principle of sufficient reason is represented by the same predicate of all previous conclusions of the PO theories, i.e. by a thesis Agkq.
Hence, Leibniz’ logico-philosophical principle appears to be the specific principle addressing an author of a PO theory in his non-classical arguing for achieving the final conclusion of.
5 The Change of Kind of Logic and Leibniz’ Principle
The universal nature of the last DNS suggests the author to have ended his inductive arguing and to accept the corresponding affirmative predicate as a new hypothesis for the subsequent part of the theory, to be deductively developed in classical logic. In fact, the author changes this predicate in the corresponding affirmative predicate; which in the latter part is considered as an hypothesis, from which a lot of theorems are deductively drawn in classical logic.
It is remarkable that both the first instance of a PO theory in the history of mathematics—Lobachevsky’s non-Euclidean geometry—, and the first instance of a PO theory in the history of modern theoretical physics—Einstein’s special relativity—originated two scientific revolutions. Each of these celebrated authors declared a change of the organisation of his theory. Lobachevsky wrote in his most relevant work: “[My supposition of two parallel lines] can likewise be admitted [as a principle-axiom for the following, deductive part of my theory] without leading to any contradiction in the results and [deductively] founds a new geometry … .” (emphasis added) [30, Proposition 19]. Einstein wrote in his celebrated paper: “We will raise this conjecture (the substance of which will be hereafter called the “[axiom-]principle of relativity”)…”.Footnote 19
In the following, I will investigate on the question, how qualify in formal terms this change of kind of logic, occurring in the texts of the PO theories.
Each of the above theories makes use of two kinds of logic; in the former part, the non-classical logic and in the latter part, the classical logic. In fact, the author changes the non-classical predicate Agkq, which is representative of the new method discovered by the former part of the PO theory, in a classical predicate A, which is representative of just the beginnings of a deductive theory.
Result 4
The authors of PO theories changed their universal conclusions in the corresponding affirmative predicates of classical logic: Agkq⇒A.
Let us now recall that in order to obtain classical logic from intuitionist logic there exist four ways, each constituted by the addition of one of the following logical features to the intuitionist logic: (i) the law of the excluded middle, (ii) the law of the double negation, (iii) the dilemma and (iv) the change of a conclusion of an ad absurdum argument ¬¬T in an affirmative T [24, 39].
We recognise that all scientists of the quoted PO theories, practiced the last way, by adding a qualification; the predicate ¬¬T is universal in nature with respect to all the problems involved by the basic problem.
Let us now recall Leibniz’s statement for the principle of sufficient reason. He too stated as a first sentence a universal DNS which subsequently changed in the corresponding affirmative sentence “… everything has its reason” (although afterwards he remarked that this sentence may be unsupported by sufficient evidence). Let us call this change PSR°. Remarkably, this change also is represented by the same formula Agkq→A formalising the final move of a PO theory. Notice in addition that even Leibniz’ principle of sufficient reason PSR° is implicitly justified by an ad absurdum argument: “It is absurd to reject it”; which, in its turn, implicitly relies on the principle “It is impossible that the reality is not rational”.
Result 5
The above logical strategy is summarised by Leibniz’ first two sentences of his version of the principle of sufficient reason. Hence, Leibniz’ PSR° may be considered as the logico-philosophical scheme inspiring the change occurring in the final part of a PO theory.Footnote 20
6 Markov’s Principle in His PO Theory on Constructive Mathematics
Fortunately, a further qualification of this change in logic was suggested by a recent Markov’s paper (1962) founding a theory of constructive mathematics [31]. Let us analyse this paper.
A former part (pp. 1–5) includes several DNSs (40; see the Appendix). By scrutinising them, one sees that the first three DNSs present the main problem of the paper, i.e. how the rational numbers may be extended to the real numbers according to the constructive method; that is, without the “use of the abstractions of actual infinity” and “the so-called pure existence theorems”.
In the middle of the paper (p. 6) Markov illustrates the constructive way to build mathematics. Actually, he refers to Church’s thesis on all algorithms; we saw that this thesis—as Kleene writes it—is a DNS. In the following part of Markov’s paper few DNSs (8 out the 48 DNSs in the entire paper) occur and moreover in an occasional way; that means that the logical sequence of the DNSs connected one to another is terminated by the previous universal statement and he is substantially following classical logic. In conclusion, also Markov followed the same theoretical organisation, PO, as the previously mentioned authors did.
This paper deserves further attention because he adds a novelty to the constructive theory of real numbers. At the end of p. 4 he introduces in loose terms a specific problem, when the application of an algorithm A to a word P of an alphabet has an end in a finite number of steps. He introduces his solution by the following words (DNSs nos. 27, 28, 35):
I consider it [is]
[= is not true that it is false] to apply here an argument “by contradiction”, i.e. to assert that the [read: every] algorithm A is applicable to the word P if the assumption that the process of applying A to P continues indefinitely leads to a contradiction. … [In other words,] If we assert on the basis of the proved impossibility of the indefinite continuation of a given procedure that this procedure ends, then this yields a perfectly well-defined method of construction …
i.e. the algorithm is applicable. In other terms, he claims that this predicate is equivalent to its affirmative version (Markov principle, in short MP). In the following of the paper he considers the latter one as a new axiom-principle for the development of the theory in a deductive way (in particular in the middle of p. 7).
Let us analyse the above quotation. In fact, Markov changes a doubly negated predicate of intuitionist logic—“the assumption that the process applying A to P continues indefinitely leads to a contradiction…”, i.e. it is contradictory that the process has no end—in the corresponding affirmative predicate of classical logic—“the algorithm is applicable to the word P”. Markov claims that this change is a valid logical step, although apparently it is supported neither by the constructive method he declared in the beginnings of his paper, nor in logical terms by intuitionist logic, where the double negation law fails.Footnote 21
7 Qualifying in Formal Terms Markov’s Principle Changing Predicates from Intuitionist Logic to Classical Logic
Let us scrutinise Markov’s justifications for the change (DNS no. 30). Apart his “intuition [which] finds it sufficiently clear” and apart the advantage that “arguments of this type make it possible to construct a constructive mathematics that is well able to serve contemporary natural science”, let us consider the third justification: “I see no reasonable basis for rejecting it [= the resulting affirmative predicate]”. It is easy to recognise that, as suggested by Dummett, [18, p. 19] Markov’s claim constitutes an intuitive application of the principle of sufficient reason, concluded by its affirmative version—I accept it. In fact, the logical structure of the above justification is the same of that of PSR: ¬∃S is ¬P.
The usual interpretation of Markov’s paper concludes that his claim concerns a decidable, doubly negated existential predicate which ends an ad absurdum proof—or, in Markov’s terms, it is proved by contradiction.
Without the former requirement on the predicate—to be a decidable one—, Anselm’s proof of God’s existence would be a decisively valid one. Without the latter requirement—to be the conclusion of an ad absurdum proof—, the application of this principle to two co-planar straight lines, conceived as ever more prolonged segments, would erroneously state that they always have a meeting point.Footnote 22
However, for supporting his change Markov does not exhibit a general ad absurdum proof on his predicate; he appeals to its possibility only; nor textbooks suggest the logical origin of Markov’s both requirements. Instead, the two requirements receive support by the past experience of arguing in scientific PO theories, as illustrated in the above. In particular, within a PO theory this requirement is obvious; indeed, a PO theory, in order to achieve a final result which is not assured in experimental terms, has to rely its arguments on concrete, decidable objects.
Result 6
Markov principle, being a tentative application of Leibniz’ principle of sufficient reason, is a similar change of that occurring inside a PO theory; but it is not the result of a specific theory about the algorithms.
MP is usually formalised as follows:
We recognise that in intuitionist logic the former member ¬¬∃x A(x) is the non-classical Igkq, whereas the latter member is the thesis I of SO.
Markov’s principle states no more than the existence of one value of a predicate, whereas PRS° states that all values of a predicate hold true. Owing to the relationship between theses A and I, the change from Igkq to I is weaker than the change of PRS°.
In particular, MP is not enough to change the logic of a PO theory, because the addition of an existential affirmative predicate does not change the entire set of the predicates in the classical ones.Footnote 23
Result 7
Markov’s change of an existential predicate from non-classical logic to the corresponding affirmative predicate of classical logic concerns the thesis Igkq. This change is weaker than PRS°; it alone is not enough for changing the kind of logic.
8 The Relevance of the Four Theses of the Square of Opposition in Intuitionist Predicate Logic
Both minimal predicate logic and predicate intuitionist logic have no calculus and a completeness theorem (stating that if P is valid, P is derivable) either. Being the logical framework of a PO theory so dubious, its sequence of DNS constructed by its author forms a highly creative arguing, possibly facilitated by the specific subject of his theory. However I will prove that to refer to SOgkq enlightens author’s arguing in a PO theory.
First of all, it is a remarkable fact that two above two kinds of change in logical predicates concern the left part (“AffIrmo”) of the SOgkq.
Moreover, the four theses of SOgkq enjoy a specific logical property. Let us consider in intuitionist logic all conversion relationships between a total predicate and an existential predicate. The only equivalence relationships are the following ones:

the last one relationship being obtained by external negation of the second one relationship. Remarkably, these four equivalence relationships concern just the four theses of SOgkq: Agkq, Igkq, Egkq, Ogkq.
Result 8
Only the four theses of the non-classical square of opposition SOgkq enjoy the property of convertibility. Hence, the square of opposition SOgkq enjoys a formal relevance in non-classical predicate logic too.
9 Conclusions
According to Brouwer, mathematics precedes logic. In this vein the present paper discovers some logical features by pondering on the logical experiences of some mathematised theories of the past. It proves that there exists a historical tradition of a way of arguing in non-classical predicate logic; this tradition is formalised by a logical framework which is similar to the classical SO, includes Leibniz’ principle, addressing the arguing of the non-deductive theory to a conclusive non-classical thesis Agkq, which then is changed in the affirmative thesis A. The logical framework includes also Markov’s principle, which does the same but weaker change in the thesis Igkq. However, all four non-classical theses corresponding to those of the classical SO and moreover are shown to be the most relevant predicates of this logical framework.
These results support the first quotation [43] about Aristotle’s logic with respect to the non-classical predicate logic. As a consequence, logical pluralism is traced back to ancient times and the Aristotle’s suggestion of a square of opposition has to be intended as a seminal hint for capturing the basic predicates of all kinds of logic.
Notes
- 1.
The last instance of this qualification is the otherwise excellent text by [23].
- 2.
- 3.
Let us recall that the relationships of an affirmative proposition of propositional classical logic with the corresponding DNS of intuitionist logic is assured by a well-known theorem on their relationship—i.e. once a formula A is true in classical logic, then evenly ¬¬A is true in the latter logic. Instead, to translate a DNSs in an affirmative sentences, owing to the failure of the law of the double negation, is a problematic move [41, 56ff]. Notice also that in intuitionist logic an ad absurdum proof ends by a DNS; to change it in an affirmative sentence is allowed by classical logic only [18, p. 27].
- 4.
[41, pp. 57–59]. In a previous paper [12] I introduced a square of opposition including at the same time two classical predicates (the theses A and E) and two non-classical predicates (the theses I and O) which differ from the traditional ones because the copula ‘is’ is changed in ‘it is not true that it is not’, or ‘is
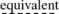 ’; this addition of double negations is not enough to represent one of the above-mentioned translations; however the results of the Sects. 7 and 8 of this paper agree with the above-mentioned translations.
’; this addition of double negations is not enough to represent one of the above-mentioned translations; however the results of the Sects. 7 and 8 of this paper agree with the above-mentioned translations. - 5.
According to modern logicians Aristotle’ square of opposition did not take in account the existential import of the theses. A more accurate investigation by authoritative scholars showed that instead Aristotle did it [33].
- 6.
The same translations hold true for minimal predicate logic, i.e. when the logical law ex falso quodlibet (P→(¬P→Q), where P and Q are whatsoever predicates) is weakened in the law P→(¬P→¬Q), hence the latter logic is strictly weaker than intuitionist logic [41, p. 57]. One may guess that an experimental scientist is not allowed to deduce ex falso everything, included the true; but the false only; hence, his logic is the minimal one. In such a case the above translations in minimal predicate logic give distinct results from those in intuitionist logic when one claims that the copula “is” of a thesis of SO is better translated in logical terms by an equivalence, i.e. a double implication. In such a case the three translations of the theses of SO in minimal predicate logic are not equivalent in both E and I theses. However, according to the next footnote 18, the following results seem hold true for the minimal SO-like too even in the latter case.
- 7.
- 8.
In the past, this way of arguing joining together the DNSs was ignored. Rather some philosophers argued by considering at the same time the three values of a sole proposition A, i.e. A, ¬A and
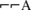 . Cusanus claimed to argue through an opposite’s coincidence of A and
. Cusanus claimed to argue through an opposite’s coincidence of A and 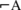 ; Hegel through an almost mechanical addiction of a negation to a
; Hegel through an almost mechanical addiction of a negation to a 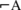 sentence. See my paper [15].
sentence. See my paper [15]. - 9.
It is by appealing to the past experiences of scientific theories that I improved the unsuccessful Beth’s research for a non-deductive way of organising a mathematical theory [2]. D’Alembert first stressed that there exists two kinds of theory organisation; he suggested that, beyond the “rational” kind, an “empirical” one exists [6]. Subsequently, for illustrating these two different models L. Carnot devoted two pages of each his two books on mechanics [3, 4]; he claimed to develop his theory in an “empirical” way. In past century both H. Poincaré [34, 35] and independently A. Einstein again suggested two similar kinds of theory organisation [27, 32].
- 10.
Some of the above-mentioned authors wrote texts which are less structured in logical terms; e.g. L. Carnot’s calculus, Lavoisier’s chemistry, Galois’ theory of groups, Klein’s Erlangen program, Einstein’s theory of special relativity all lack of ad absurdum theorems.
- 11.
[29]. Here and in the following, emphasis is added for manifesting to the reader the two negations within a doubly negated sentence.
- 12.
[30, Proposition 19]. This book was analysed through its DNSs by my paper [14]. Lobachevsky’s main text puts the problem of how much parallel lines to a straight line exist. In order to obtain evidence for his guess—i.e. two parallel lines—he proves through DNSs five theorems, most of which are ad absurdum theorems. At the end of the prop. 22, shown by an ad absurdum theorem, he concludes that his supposition with respect to Euclid’s hypothesis, receives an equivalent evidence in both all points and in all figures in the space.
- 13.
[5, p. 23]. S. Carnot’s thermodynamics puts the problem of the maximum efficiency in the heat/work conversions; in order to solve it, he looks for a new method by arguing through DNSs about his celebrated cycle of four transformations. The list of DNSs ends by means of his well-known ad absurdum theorem about the maximum efficiency in all heat/work conversions. Carnot’s book was analysed through its DNSs by the paper [17].
- 14.
[36, p. 187]. Poincaré criticism to Formalists’ attempt to prove by finitist means the principle of mathematical induction concludes by essentially the following DNS: “… does not exist contradiction” for all entire numbers. The current version of this principle changes it in the corresponding affirmative predicate. See my paper [7].
- 15.
[28]. Kolmogorov’s foundation of the minimal logic argues by means DNSs and an ad absurdum theorem stating the above conclusion. Afterwards, Kolmogorov thinks that nothing opposes to always deductively argue by means of pseudotruths from the axioms of the type A of Hilbert’s formalisation of logic. See also [10].
- 16.
[1, p. 58]. The subsequent sentence (“Hence, it is necessary thus to admit that [there it is not true that do not exist] there exist simple relationships also among the volumes of [all] the gaseous substances and the number of the simple or composed molecules which compose them) constitutes the celebrated “Avogadro’s law” on the molecular constitution of whatsoever kind of matter. An analysis of the paper through its DNSs is given by [16].
- 17.
Reference [20] studies a similar problem in various kinds of logic, but at the propositional level only.
- 18.
Remarkably, Agkq is the same in both minimal logic and intuitionist logic. Notice that one may guess that when an author of a PO theory obtains, by arguing in minimal logic, the universal predicate Agkq, he governs by this final DNS the entire universe of his logical arguing with respect to the basic problem. At this stage, he, when meeting a false sentence, can consider it as belonging to a purely theoretical universe; hence, he can apply the intuitionist law on the false, according to which everything of this theoretical universe follows from it. In other words, when he achieves this predicate, at the same time he implicitly changes the kind of logic from the minimal one to the intuitionist one.
- 19.
- 20.
The two requirements on the predicate on which MP is applied suggests that PRS° would have to be applied according to the same requirements, i.e. on a predicate which is decidable one and it is obtained as the result of an ad absurdum theorem. Such requirements surely would avoid all criticisms to the application of PSS°, first of all the criticism to be a metaphysical principle. In the past, a great debate aimed to clarify the use of the PSR°. From the above we conclude that PSR is not a heuristic principle for validating a mere guess on an isolated event, but an architectural principle, to be applied to an entire PO theory (see my paper [9]). Its theory-dependence explains why in the ancient times, when an analysis on PO theories was premature, it was ignored; and why in modern times, when scholars devoted little attention to both non-deductive theories and non-classical logic, it was misinterpreted.
- 21.
- 22.
It is just after a chain of ad absurdum proofs (propositions nos. 17–22) that Lobachevsky stated the existence of two parallel lines, conceived as ever more prolonged segments, as an alternative hypothesis to the Euclidean one. Markov’s two requirements enlightens the implicit requirements of the common move performed by all the above authors of a PO theory, which of course concerns a method which has to rely on decidable predicates; also the common move occurring at the end of the former parts of OP theories is implicitly justified by not having reason for excluding the affirmative conclusion. Moreover, notice that the same implicit ad absurdum argument that justifies PRS° holds true for Markov’s move: “… otherwise the reality is absurd”.
- 23.
Also Markov states that by means of the DNSs nos. 32, 33, 34.
References
Avogadro, A.: Essay d’une manière de determiner les masses relatives des molecules élémentaires des corps…J. Phys. Chim. Hist. Nat. 73, 58–76 (1811)
Beth, E.W.: Foundations of Mathematics, ch. I, 2. Harper, New York (1959)
Carnot, L.: Essai sur le machines en général, pp. 101–103. Defay, Dijon (1783)
Carnot, L.: Principes de l’équilibre et du mouvement, pp. xii–xv. Deterville, Paris (1803)
Carnot, S.: Réflexions sur la puissance motrice du feu, p. 23. Blanchard, Paris (1824)
Le Ronde d’Alembert, J.: Elémens. In: Le Ronde d’Alembert, J., Diderot, D. (eds.) Éncyclopédie Française, p. 17 (1754)
Drago, A.: Poincaré vs. Peano and Hilbert about the mathematical principle of induction. In: Greffe, J.-L., Heinzmann, G., Lorenz, K. (eds.) Henri Poincaré. Science et Philosophie, pp. 513–527. Blanchard/Springer, Paris/Berlin (1996)
Drago, A.: Mathematics and alternative theoretical physics: the method for linking them together. Epistemologia 19, 33–50 (1996)
Drago, A.: The birth of an alternative mechanics: Leibniz’ principle of sufficient reason. In: Poser, H., et al. (eds.) Leibniz-Kongress. Nihil Sine Ratione, vol. I, pp. 322–330. Berlin (2001)
Drago, A.: A.N. Kolmogoroff and the relevance of the double negation law in science. In: Sica, G. (ed.) Essays on the Foundations of Mathematics and Logic, pp. 57–81. Polimetrica, Milano (2005)
Drago, A.: Four ways of logical reasoning in theoretical physics and their relationships with both the kinds of mathematics and computer science. In: Cooper, S.B., et al. (eds.) Computation and Logic in Real World, pp. 132–142. Univ. Siena Dept. of Math. Sci., Siena (2007)
Drago, A.: The square of opposition and the four fundamental choices. Logica Univers. 2, 127–141 (2008)
Drago, A.: Lo scritto di Einstein sulla relatività. Esame secondo il modello di organizzazione basata su un problema. Atti Fond. G. Ronchi 64, 113–131 (2009)
Drago, A.: A logical analysis of Lobachevsky’s geometrical theory. Atti Fond. G. Ronchi 64(4), 453–481 (2010). Italian version in: Period. Mat., Ser. VIII 4, 41–52 (2004)
Drago, A.: The coincidentia oppositorum in Cusanus (1401–1464), Lanza del Vasto (1901–1981) and beyond. Epistemologia 33, 305–328 (2010)
Drago, A., Oliva, R.: Atomism and the reasoning by non-classical logic. Hyle 5, 43–55 (1999)
Drago, A., Pisano, R.: Interpretation and reconstruction of Sadi Carnot’s Réflexions through non-classical logic. Atti Fond. G. Ronchi 59, 615–644 (2004). Italian version in: G. Fis. 41, 195–215 (2000)
Dummett, M.: Principles of Intuitionism. Clarendon, Oxford (1977)
Einstein, A.: Zur Elektrodynamik bewegter Koerper. Ann. Phys. 17, 891–921 (1905)
Gabbey, D., Olivetti, N.: Goal-oriented deductions. In: Gabbey, D., Guenthner, F. (eds.) Handbook of Philosophical Logic, vol. 9, pp. 134–199. Reidel, Boston (2002)
Goedel, K.: Collected Works, papers 1932 and 1933f. Oxford University Press, Oxford (1986)
Grize, J.B.: Logique. In: Piaget, J. (ed.) Logique et connaissance scientifique. Éncyclopédie de la Pléiade, pp. 135–288 (206–210). Gallimard, Paris (1970)
Haak, S.: Deviant Logic. Oxford University Press, Oxford (1978)
Hand, M.: Antirealism and Falsity. In: Gabbey, D.M., Wansing, H. (eds.) What is Negation?, pp. 185–198 (188). Kluwer, Dordrecht (1999)
Heyting, A.: Intuitionism. An Introduction, Sect. 2. North-Holland, Amsterdam (1971)
Kleene, C.: Introduction to Metamathematics, pp. 318–319. Van Nostrand, Princeton (1952)
Klein, M.J.: Thermodynamics in Einstein’s thought. Science 57, 505–516 (1967)
Kolmogorov, A.N.: On the principle ‘tertium non datur’. Mat. Sb. 32, 646–667 (1924/1925). Engl. translation in: van Heijenoorth, J. (ed.) From Frege to Goedel, pp. 416–437. Harvard University Press, Cambridge (1967)
Leibniz, G.W.: Letter to Arnauld, 14-7-1686, Gerh. II, Q., Opusc. 402, 513
Lobachevsky, N.I.: Geometrische Untersuchungen zur der Theorien der Parallellineen. Finkl, Berlin (1840). Engl. translation as an Appendix to: Bonola, R.: Non-Euclidean Geometry. Dover, New York (1955)
Markov, A.A.: On constructive mathematics. Tr. Mat. Inst. Steklova 67, 8–14 (1962). Also in: Am. Math. Soc. Transl. 98(2), 1–9 (1971)
Miller, A.I.: Albert Einstein’s Special Theory of Relativity, pp. 123–142. Addison-Wesley, Reading (1981)
Parsons, T.: Things that are right wit the traditional square of opposition. Logica Univers. 2, 3–11 (2008)
Poincaré, H.: La Science et l’Hypothèse, Ch. “Optique et Electricité”. Hermann, Paris (1903)
Poincaré, H.: La Valeur de la Science, Ch. VII. Flammarion, Paris (1905)
Poincaré, H.: Science et Méthode, p. 187. Flammarion, Paris (1912)
Prawitz, D.: Meaning and proof. The conflict between classical and intuitionist logic. Theoria 43, 6–39 (1976)
Prawitz, D., Melmnaas, P.-E.: A survey of some connections between classical intuitionistic and minimal logic. In: Schmidt, A., Schuette, H. (eds.) Contributions to Mathematical Logic, pp. 215–229. North-Holland, Amsterdam (1968)
Tennant, N.: Natural Logic, p. 57. Edinburgh University Press, Edinburgh
Troesltra, A.: Remarks on intuitionism and the philosophy of mathematics. In: Costantini, D., Galavotti, M.C. (eds.) Nuovi Problemi della Logica e della Filosofia della Scienza, pp. 211–228 (223). CLUEB, Bologna (1991)
Troelstra, A., van Dalen, D.: Constructivism in Mathematics, vol. 1. North-Holland, Amsterdam (1988)
van Dalen, D.: Intuitionist logic. In: Gabbey, D.M., Guenthner, F. (eds.) Handbook of Mathematical Logic, vol. II, pp. 225–449 (226 and 230). Reidel, Dordrecht (2002)
Woods, J., Irvine, A.: Aristotle’s early logic. In: Gabbey, D.M., Woods, J. (eds) Handbook of History of Logic, vol. 1, pp. 27–99 (66). Elsevier, New York (2004)
Acknowledgements
I acknowledge two anonymous referees for an improvement of my previous logical framework, and David Braithwhaite for the correction on my English text.
Author information
Authors and Affiliations
Corresponding author
Editor information
Editors and Affiliations
Appendix: The analysis through the double negations of [31]
Appendix: The analysis through the double negations of [31]
Notice that modal words are equivalent, via S4 translation, to DNSs; these words are wave underlined.
-
(1)
Recently the constructive trend in mathematics has been significantly developed. Its goal is to base all investigations on constructive objects and to carry them out within the bounds of the abstraction of potential realizability and without use of the abstraction of actual (= not potential) infinity; …
-
(2)
… it rejects the so-called pure existence [= not constructive] theorems, …
-
(3)
… since the existence of an object with given properties is considered proved only when a
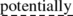 realizable method for the construction of an object with those properties bas been indicated … .
realizable method for the construction of an object with those properties bas been indicated … . -
(4)
… We do not define the concept of a constructive object, but rather
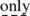 clarify it.
clarify it. -
(5)
In constructive mathematical theories we limit ourselves to the consideration of constructive objects of some standard type, which frees us from the necessity of formulating a general definition of a constructive object.
-
(6)
The abstraction of identity is used here in a natural way; we identify the words (1) and (2); we abstract away from their having any differences; we say that they are the same word … .
-
(7)
… When considering words in a given alphabet we are forced into an abstraction of another kind—into the abstraction of potential existence … .
-
(8)
… It consists in abstracting away from the practical limits of our possibilities in space, time and material when it comes to the existence of words … .
-
(9)
… We cannot write on a given blackboard of a given dimension words of arbitrary [= unlimited] length … .
-
(10)
… We abstract away from this practical impossibility and begin to argue as if this were possible.
-
(11)
This does not at all mean that we begin to consider the “sequence of naturals” as an infinite “object.” …
-
(12)
… Such a consideration would involve an abstraction of an actual infinity, …
-
(13)
… taking us beyond the limits of constructive mathematics and into something characteristic of the so-called “classical” mathematics.
-
(14)
In “classical” mathematics there have been many “pure existence theorems,” which consist in assertions about the “existence” of objects with certain properties even despites a complete ignorance of means to construct such an object.
-
(15)
Constructive mathematics rejects such propositions [on pure existence] …
-
(16)
… In constructive mathematics the existence of an object with certain properties is
 considered proved when a potentially realizable method has been given for the construction of an object with the given properties.
considered proved when a potentially realizable method has been given for the construction of an object with the given properties. -
(17)
This understanding of disjunction does not permit one to take as true the law of the excluded middle: “P or not P.”…
-
(18)
…2. The formulation and development of the constructive trend took place on the basis of work that appeared in the 1930s which made precise the concept of an algorithm, freeing this concept from vagueness and subjectivity… .
-
(19)
As we know, this vague concept of a was made precise in the 30s in the work of several men, who took different approaches: Church, Kleene, Turing, Post. The theories constructed by these men—Kleene’s theory of recursive functions, Church’s calculus of λ-conversion, the theory of Turing machines and Post’s theory of finite combinatory processes—turned out to be
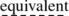 to one another and to lead to essentially the same formulation of the concept of an algorithm …
to one another and to lead to essentially the same formulation of the concept of an algorithm … -
(20)
… New formulations of this concept, also
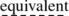 to the previous ones, were constructed one after another by other authors, …
to the previous ones, were constructed one after another by other authors, … -
(21)
… and even in the present time new theories of algorithms are continually being published that are
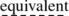 to the previous theories … .
to the previous theories … . -
(22)
… It is not
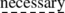 for us at this time to look into these theories to try to find the best one.
for us at this time to look into these theories to try to find the best one. -
(23)
The algorithm also determines the end of the procedure which
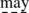
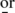
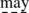
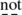 occur.
occur. -
(24)
The theory of normal algorithms is constructed which in the framework of abstract
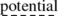 existence … .
existence … . -
(25)
… The words in the alphabet A under consideration and the schemes of the normal algorithms in A are
 realizable constructive objects … .
realizable constructive objects … . -
(26)
… The procedure itself of applying a normal algorithm to a given word is considered by us to be a
 realizable procedure.
realizable procedure. -
(27)
I consider it [is]
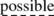 to apply here an argument “by contradiction”,
to apply here an argument “by contradiction”, -
(28)
… i.e. to assert that the algorithm A is applicable to the word P if the assumption that the process of applying A to P continues indefinitely leads to a contradiction.
-
(29)
If I defend this means of argument here, it is not because I find it without error according to my intuition, …
-
(30)
… but rather, firstly, because I see no reasonable reason for rejecting it, …
-
(31)
… and secondly, because arguments of this type make it
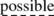 to construct a constructive mathematics that is well able to serve contemporary natural Science … .
to construct a constructive mathematics that is well able to serve contemporary natural Science … . -
(32)
… I insist that this does not go beyond the bounds of the constructive direction: …
-
(33)
… the abstraction of actual infinity is not made, …
-
(34)
… existence continues to coincide with a
 realizable construction …
realizable construction … -
(35)
… If we assert on the basis of the proved impossibility of the indefinite continuation of a given procedure that this procedure ends, then this yields a perfectly well-defined method of construction: continue the process until its completion … .
-
(36)
… The circumstance that the number of steps cannot be bounded “in advance”, here changes nothing of importance … .
-
(37)
… It is even
 that the requirement that this number be bounded in advance will ever be formulated precisely and objectively … .
that the requirement that this number be bounded in advance will ever be formulated precisely and objectively … . -
(38)
… It is not difficult to see that this method for proving the applicability of an algorithm …
-
(39)
…
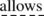 one to justify the following method of argument … .
one to justify the following method of argument … . -
(40)
… Let P a property, ad let there be an algorithm that decide for every natural number n whether or not n has the property P. If the proposition that no number has the property P leads to a contradiction there is a natural number with the property P.
The analysis of this list of DNS is easy. The list presents one only ad absurdum proof; it is exposed wordily by the DNSs 29 and 30. Hence, there is one unit of arguing only. The following DNSs are aimed to illustrate the result obtained. After the DNS 40 the development of the theory assumes the last DNS as a new hypothesis.
Rights and permissions
Copyright information
© 2012 Springer Basel
About this chapter
Cite this chapter
Drago, A. (2012). Pluralism in Logic: The Square of Opposition, Leibniz’ Principle of Sufficient Reason and Markov’s Principle. In: Béziau, JY., Jacquette, D. (eds) Around and Beyond the Square of Opposition. Studies in Universal Logic. Springer, Basel. https://doi.org/10.1007/978-3-0348-0379-3_12
Download citation
DOI: https://doi.org/10.1007/978-3-0348-0379-3_12
Publisher Name: Springer, Basel
Print ISBN: 978-3-0348-0378-6
Online ISBN: 978-3-0348-0379-3
eBook Packages: Mathematics and StatisticsMathematics and Statistics (R0)




 [= is not true that it is false] to apply here an argument “by contradiction”, i.e. to assert that the [read: every] algorithm A is applicable to the word P if the assumption that the process of applying A to P continues indefinitely leads to a contradiction. … [In other words,] If we assert on the basis of the proved impossibility of the indefinite continuation of a given procedure that this procedure ends, then this yields a perfectly well-defined method of construction …
[= is not true that it is false] to apply here an argument “by contradiction”, i.e. to assert that the [read: every] algorithm A is applicable to the word P if the assumption that the process of applying A to P continues indefinitely leads to a contradiction. … [In other words,] If we assert on the basis of the proved impossibility of the indefinite continuation of a given procedure that this procedure ends, then this yields a perfectly well-defined method of construction …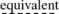 ’; this addition of double negations is not enough to represent one of the above-mentioned translations; however the results of the Sects.
’; this addition of double negations is not enough to represent one of the above-mentioned translations; however the results of the Sects. 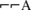 . Cusanus claimed to argue through an opposite’s coincidence of A and
. Cusanus claimed to argue through an opposite’s coincidence of A and 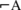 ; Hegel through an almost mechanical addiction of a negation to a
; Hegel through an almost mechanical addiction of a negation to a 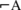 sentence. See my paper [
sentence. See my paper [ realizable method for the construction of an object with those properties bas been indicated … .
realizable method for the construction of an object with those properties bas been indicated … .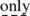 clarify it.
clarify it. considered proved when a potentially realizable method has been given for the construction of an object with the given properties.
considered proved when a potentially realizable method has been given for the construction of an object with the given properties.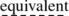 to one another and to lead to essentially the same formulation of the concept of an algorithm …
to one another and to lead to essentially the same formulation of the concept of an algorithm …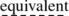 to the previous ones, were constructed one after another by other authors, …
to the previous ones, were constructed one after another by other authors, …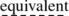 to the previous theories … .
to the previous theories … .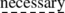 for us at this time to look into these theories to try to find the best one.
for us at this time to look into these theories to try to find the best one.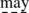
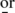
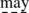
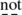 occur.
occur.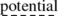 existence … .
existence … . realizable constructive objects … .
realizable constructive objects … . realizable procedure.
realizable procedure.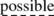 to apply here an argument “by contradiction”,
to apply here an argument “by contradiction”,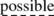 to construct a constructive mathematics that is well able to serve contemporary natural Science … .
to construct a constructive mathematics that is well able to serve contemporary natural Science … . realizable construction …
realizable construction … that the requirement that this number be bounded in advance will ever be formulated precisely and objectively … .
that the requirement that this number be bounded in advance will ever be formulated precisely and objectively … .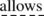 one to justify the following method of argument … .
one to justify the following method of argument … .