Keywords

1 Introduction

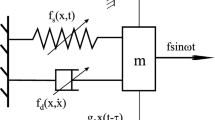
Consider a cylindrical piezoceramic transducer placed in an acoustic medium. Let us assume that the oscillations of a piezoceramic transducer are excited by an analog generator. Let’s also assume that the power of the generator is comparable to the power consumed by the transducer. Under these assumptions, the “generator—piezoceramic transducer” system is a typical nonideal dynamic system according to Sommerfeld-Kononenko (Sommerfeld [1, 2], Kononenko [3]). The mathematical model of such a system was described using a normal system of ordinary differential equations in Krasnopolskaya and Shvets [4].

The mathematical model of the “generator-transducer” system was derived for a real physical system based on the strict principles of the general theory of electroelastic systems in acoustic media. Subsequently, it was revealed that the “generator-transducer” system has a very wide variety of dynamic behavior. So in such a system, all the main types of regular attractors were discovered, such as equilibrium positions, limit cycles and invariant tori (Krasnopolskaya and Shvets [4], Balthazar et al. [5], Shvets and Donetskyi [6]). Chaotic attractors, including hyperchaotic ones, were also found in the “generator-transducer” system (Shvets and Krasnopolskaya [7]). Transitions to chaos (hyperchaos) through a cascade of period doubling bifurcations (Feigenbaum [8, 9] and through intermittency (Manneville and Pomeau [10]) were identified. And finally, in paper Shvets and Donetskyi [6], self-excited, hidden and rare attractors were discovered in the “generator-transducer” system.

The above allows us to assert that the “generator-converter” system has greater variety of dynamic behavior than the classical Lorentz ([11]) and Rössler ([12, 13]) systems. Such system is the “library” of regular and chaotic dynamics and can be used as a basic one in the study of the general theory of dynamical systems.

2 Mathematical Model

Using papers Krasnopolskaya and Shvets [4], Shvets and Donetskyi [6], we write the mathematical model of the “generator-converter” system in the form of a normal system of differential equations:

$$\begin{aligned} \begin{array}{rcl} \frac{d\xi }{d\tau }&{}=&{}\zeta ,\\ \frac{d\zeta }{d\tau }&{}=&{}-\xi +\alpha _{1}\zeta +\alpha _{2}\zeta ^{2}-\alpha _{3}\zeta ^{3}-\alpha _{4}\beta ,\\ \frac{d\beta }{d\tau }&{}=&{}\gamma ,\\ \frac{d\gamma }{d\tau }&{}=&{}-\alpha _{0}\beta +\alpha _{5}\xi +\alpha _{6}\zeta -\alpha _{7}\gamma . \end{array} \end{aligned}$$
(1)

Here phase variables \(\xi ,\zeta \) describe the dynamics of piezoceramic transducer. Accordingly, phase variables \(\beta ,\gamma \) describe the dynamics of analog generator. The physical meaning of these variables and parameters \(\alpha _0,\alpha _1,...,\alpha _7 \) of the system (1) are described in detail in paper Krasnopolskaya and Shvets [4].

Since the system of Eq. (1) is a nonlinear system of differential equations, the study of its dynamic behavior, in the general case, can be carried out only by numerical methods. The methodology for conducting such research is described in the papers Shvets [14], Shvets and Krasnopolskaya [7].

3 Symmetry and Double Symmetry

Typical behavior for dynamical system is when, with increase(decrease) in the value of a bifurcation parameter, the following chain of transitions to chaos is observed: a cascade of bifurcations of period doubling of limit cycles, then chaos, then so called periodicity window, after which this chain repeats: cascade of period doubling \(\rightarrow \) chaos \(\rightarrow \) periodicity window \(\rightarrow \) cascade of period doubling \(\rightarrow \dots \) This behavior is also known as Feigenbaum scenario. Accordingly, with a decrease(increase) in the value of a bifurcation parameter, different chain of transactions to chaos is observed. Namely limit cycle, then intermittency in chaos, then periodicity window, after which this chain repeats: limits cycle \(\rightarrow \) intermittency in chaos \(\rightarrow \) periodicity window \(\rightarrow \) limit cycle \(\rightarrow \dots \) This behaviour is known as Pomeau-Manneville scenario. In this system, however, there are regions of parameters for which violation of strict chain of transactions for either scenarios is observed.

Fig. 1
figure 1

Phase-parametric characteristic

Full size image
Fig. 2
figure 2

Phase-parametric characteristic

Full size image

Let values of parameters be \(\alpha _0 = 0.995,\ \alpha _1 = 0.0535,\ \alpha _3 = 9.95,\ \alpha _4 = - 0.103,\ \alpha _5 = - 0.0604,\ \alpha _6 = - 0.12,\ \alpha _7 = 0.01\). And leave parameter \(\alpha _2\) as bifurcation one. In Fig. 1, for these values of parameters, the phase-parametric characteristic of the system (1), the so-called bifurcation tree, is constructed. Steady-state periodic regimes correspond to individual branches of this tree, and chaotic ones correspond to densely black areas. A careful study of the phase-parametric characteristics allows us to understand the bifurcations occurring in the system. As one may notice, there is some symmetry value of bifurcation parameter (\(\alpha _2 \approx 9.6455\)), relative to which any chain of transitions to chaos is reflected. This means that with increase in the value of the bifurcation parameter both Feigenbaum scenario and Pomeau-Manneville one occur, which is violation of strict chain of transitions to chaos. Same behavior is also true for the case of decrease in the value of the bifurcation parameter. We notice that such situation appears to be natural for this specific system, since it is not the first time when such symmetry in transition to chaos was established (Shvets and Donetskyi[6]). And as we will see further, not the last.

Consider couple more intervals of bifurcation parameter for which symmetric transition to chaos is observed along with some other interesting features.

Let us start with interval \(9.075< \alpha _2 < 9.3\). As we can see from Fig. 2, there is a double symmetry in the alternation of scenarios of transitions to chaos. One of the symmetries is clearly seen over the entire range of variation of the bifurcation parameter. Inside this symmetry, in a much smaller interval, one more symmetry is visible. Such double symmetries (symmetries within symmetries) are quite atypical for dynamical systems. Just like before, we can see violation of strict chain of transitions to chaos both with increase and with decrease in the value of the bifurcation parameter.

In the Fig. 3 you can see couple of bifurcations of Feigenbaum scenario for the Phase-parametric characteristic presented in the Fig. 2. Namely, there are three first period doubling plotted in the Fig. 3a –c. And the chaos presented in the Fig. 3d.

Fig. 3
figure 3

Phase portrait projections: at \(\alpha _2 = 9.1\) (a); at \(\alpha _2 = 9.13\) (b); at \(\alpha _2 = 9.14\) (c); at \(\alpha _2 = 9.15\) (d).

Full size image
Fig. 4
figure 4

Phase-parametric characteristic

Full size image

Another type of symmetry is realized on the interval of variation of the bifurcation parameter \(9.646< \alpha _2 < 9.64625\). The phase-parametric characteristic of the system for this interval is shown in Fig. 4. Here, the transition to chaos occurs, in one bifurcation, through the intermittency both on the left and on the right of the considered interval. Moreover, there are no periodicity windows inside the chaos. Accordingly, no other transitions to chaos are observed according to the Feigenbaum scenario.

4 Generalized Intermittency and Symmetry

Finally, consider the bifurcations that occur in the system on the interval \(9.64624< \alpha _2 <9.64665\). As the parameter \( \alpha _2 \) increases, a cascade of bifurcations of doubling the period of limit cycles begins in the system, which leads to the appearance of a chaotic attractor. Further, as \( \alpha _2 \) increases, the chaotic attractor is replaced with periodicity window. Then this chain of transitions is observed again: a cascade of period doubling bifurcations \(\rightarrow \) chaos \(\rightarrow \) a periodicity window, and so on. However, the sequence of such transitions is interrupted at \( \alpha _2\approx 9.64631 \). Further, an extremely interesting transition occurs from a chaotic attractor of one type to a chaotic attractor of another type according to the scenario of generalized intermittency. This scenario is described in detail in the papers Krasnopolskaya and Shvets [15], Shvets and Sirenko [16]. One of distinctive features of such a transition is the appearance of coarse-grained (rough) laminar phase instead of laminar phase of usual intermittency Fig. 5.

Fig. 5
figure 5

Phase-parametric characteristic

Full size image
Fig. 6
figure 6

Phase portrait projections: at \(\alpha _2 = 9.6463\) (a); at \(\alpha _2 = 9.64631\) (b). Distribution of invariant measure: at \(\alpha _2 = 9.6463\) (c); at \(\alpha _2 = 9.64631\) (d).

Full size image

We notice that all described above behavior is symmetric, i.e. exists some “median” value of bifurcation parameter \(\alpha _2\), such that any transition to chaos is reflected. But there is more than that, since the very first chain of transitions to chaos that happend prior the generalized intermittency is reflected too. It is worth emphasizing due to fact that regularly generalized intermittency is not a part of any other chain of transitions to chaos.

Let us illustrate the scenario of generalized intermittency using phase portraits and distributions of the invariant measure of the corresponding attractors presented in Fig. 6. In the Fig. 6a and c, the phase portrait projection and distribution of invariant measure are presented respectively, prior the generalized intermittency. After the bifurcation, chaotic attractor of one type disappears, and chaotic attractor of other type borns. Phase portrait projection, as well as distribution of invarian measure for this new attractor are presented in the Fig. 6b and d respectively. Behavior of newborn chaotic attractor consists of two main phases: the rough-laminar phase and turbulent one. In the rough-laminar phase, trajectory of the caotic attractor makes chaotic movements near localization of disappeared attractor. During these movements, trajectory of newborn attractor almost coincide with trajectory of disappeared one. These correspond to the much darkened areas in Fig. 6d. Then, at the unpredictable moment of time, turbulent phase begins. During this phase, trajectory leaves localization region and moves to distant regions of the phase space. After some time, trajectory returns to rough-laminar phase. This process of switching phases is repeated infinitely many times.

5 Conclusions

Thus, the paper explored a number of symmetries in the alternation of scenarios of transitions to chaos in a nonideal dynamic system “piezoelectric converter-analog generator”. The existence of double symmetry is established for such alternations of scenarios.

The possibility of transitions “chaotic attractor of one type—chaotic attractor of another type” according to the scenario of generalized intermittency was revealed for the first time.