Introduction

Carbon nanotubes (CNTs) and graphene, the classic low-dimensional nanomaterial, have attracted intensive research interest owing to their outstanding features,1,2,3,4 such as excellent mechanical strength and stiffness,5,6 ultrahigh thermal conductivity,7 chirality-dependent electrical conductivity,8,9 unique optoelectronic characteristics,10 and larger specific surface areas.11 However, the brittle nature and low-dimensional features of such carbon-based nanomaterials (e.g., graphene, CNT, and fullerene) hamper their applications, for example, in wearable and flexible electronics.12,13 Many strategies have been proposed to modify the properties and overcome the shortcomings of graphene/CNT, such as the lattice-like nanotruss CNT networks by linking CNTs covalently,12 bioinspired fibrous/helicoidal CNTs,14 CNT bundles,15 CNT-pillared graphene network,16 three-dimensional (3D) graphene foams,17,18,19 annealing-processed 3D disordered graphene networks,20 and paper-cutting-inspired graphene/CNT kirigami.13,21 However, there are issues that are required to be addressed in the previously mentioned advanced nanomaterials. For example, the lattice-like CNT metamaterials and bioinspired fibrous/helicoidal CNTs are still brittle with limited elasticity and the topological defects in the linkage of CNTs decrease their strength.12 The stretchability of a CNT is improved significantly by introducing kirigami patterns, however, at the cost of reducing its stiffness and strength.13

Origami, the ancient Chinese and Japanese art of paper folding,22 not only results in interesting architectures,23,24,25,26 but also enables a design platform for developing advanced metamaterials with novel mechanical properties, such as increased stretchability,27 negative Poisson’s ratio,22,28 negative coefficient of thermal expansion,29 multistability,30 and zero/negative incremental stiffness.31,32 Owning to the presence of creases in the origami-inspired metamaterials, the entire architecture can be folded and unfolded to obtain deployable structures;28 the overall structural performance of origami is dominated by the underlying architecture-induced kinematic deformation modes.22 Here, we construct the CNT origami (CNT-O) with a Miura-ori tessellation,22 and combine molecular dynamics (MD) simulation and experimentation to demonstrate that the origami design can impart a strategy for developing CNT-based metamaterials with enhanced stretchability, high strength, and negative Poisson’s ratio (i.e., auxeticity). To achieve this aim, we form the CNT-O via surface functionalization with the assistance of hydrogen atoms in MD simulation, which is a feasible way to realize nanomaterials with tunable properties. Significant progress in the hydrogenation of CNT and graphene has been achieved through experimentation33,34,35,36,37,38 (see Supplementary information S1 for more information). For example, the hydrogenation of a CNT has been realized via a modified Birch reduction method36 or using a glow-discharge approach.35 Meanwhile, the hydrogenation mapping such as origami patterning on graphene can be precisely controlled using a photoresist mask.39 The subsequent imaging using fluorescence quenching microscopy (FQM) has revealed that this technology can realize complicated hydrogenation patterns on nanomaterials.39 However, due to the curved surface of the CNT, achieving a precise functionalization mapping remains a challenge with this approach, and new or modified technologies are yet to be developed to make desired hydrogenation patterns on freeform surfaces.

In this article, the hydrogenated CNT-O is virtually formed in a MD simulation, followed by a theoretical analysis and mechanical testing on prototypes on mesoscale counterparts made out of conventional cellulose-based papers. Our results indicate that the stretchability and the strength of CNT-O can be increased simultaneously in some cases (i.e., samples with large folding widths and enhanced number of cells along the circumference of CNT-O), and a variable strain-dependent negative Poisson’s ratio can be attained. All of the mechanical properties of the nanoarchitected origami can be controlled by tuning geometrical parameters. Moreover, we demonstrate that the folding/unfolding of CNT-O enables tunability of the negative Poisson’s ratio, opening avenues for the realization of a new class of nonporous auxetic nanomaterials.

Modeling and methods

MD simulation

MD simulation is conducted using the large-scale atomic molecular massively parallel simulator (LAMMPS)40 to study the surface functionalization and mechanical responses of CNT-O. The Open Visualization Tool (OVITO)41 is employed to visualize the evolution of atomic structures. AIREBO42 potential function is used to describe C–C and C–H interatomic interactions. The cutoff distance between C–C is set at 2.0 Å to avoid the spurious strengthening effect.12,13,27 Periodic boundary condition is applied along the origami carbon nanotube axial direction. To transform a cylindrical CNT to a folded origami configuration, the surface functionalization is performed by placing hydrogen atoms approximately in 1.1 Å distance from the adjacent carbon atom27,43 (Figure 1a). Initially, energy minimization is performed by a conjugate gradient algorithm. Then, the engineered hydrogenated CNT is relaxed in the canonical ensemble (NVT) for 100 picoseconds (ps) and the isothermalisobaric ensemble (NPT) for 3500 ps with a time step of 1 femtosecond (fs). A low temperature of 1 K is set to limit the influence of thermal fluctuation.15 A stable CNT-O with minimal potential energy can be obtained after full relaxation, as shown in Figure 2a. After obtaining the stable CNT-O nanostructures, the samples are subjected to a homogeneous tensile/compressive deformation by rescaling the vertical coordinates of all atoms in the simulation box to study their mechanical responses. The strain rate is set at \(1\times {10}^{-9}\) s−1. The axial stress is calculated by averaging the virial stress of all atoms in the system as14

$$\upsigma_{ij} = \frac{1}{{V_{0} }}\sum\limits_{\upgamma = 1}^{n} {S_{ij}^{\upgamma } },$$
(1)

where V0 is the initial volume of the material; \({S}_{ij}^{\upgamma }\) is the stress tensor for atom γ; and i and j denote the indices associated with axes of the Cartesian coordinate system. The atomic stress of individual carbon atom α in the CNT-O nanostructure is calculated by14

$$S_{ij}^{\upalpha } = \frac{1}{2}m^{\upalpha } v_{i}^{\upalpha } v_{j}^{\upalpha } + \sum\limits_{\upbeta = 1}^{n} {r_{\upalpha \upbeta }^{j} } \,f_{\upalpha \upbeta }^{i},$$
(2)

where \({m}^{\upalpha }\) and \({v}^{\upalpha }\) represent the mass and velocity of atom \(\upalpha\); \({r}_{\upalpha \upbeta }\) and \({f}_{\upalpha \upbeta }\) are the distance and force between atoms \(\upalpha\) and \(\upbeta\).

Figure 1
figure 1

Surface functionalization by hydrogen atoms. (a) The hydrogenation is applied on both surfaces of graphene. The rectangle with dotted line and sold line represents the size of surface-functionalized graphene before and after relaxation. It is found that the surface-functionalized graphene expands in the x- and y-directions, resulting in mechanical strains (ɛxx and ɛyy). (b) Functionalized graphene sheet characterized by different folding widths (t) and their folding angles (θ) as a function of relaxation time in molecular dynamics (MD) simulation. The folding angle is found at an equilibrium state after about 400 ps. The green and red atoms represent carbon and hydrogen atoms, respectively. (c) Schematic illustration of folding induced by bending moment (M), resulting from the hydrogenation. (d) The folding angle of simple graphene origami as a function of folding width based on MD simulation and theoretical analysis. It is found that when the folding width (t) is higher than 0.9 nm, the folding angle is close to zero degree, indicating completely folded.

Full size image
Figure 2
figure 2

Illustration of surface functionalization to transfer a carbon nanotube (CNT) to a carbon nanotube origami (CNT-O). (a) Schematic of the CNT-O with Miura-ori patterns before and after relaxation in molecular dynamics (MD) simulation. The CNT-O is characterized by creases (a = 79.577 Å and b = 115.62 Å), folding width (t), the angle between creases (β = π/6), and diameter (D). (b) Geometry of the Miura-ori pattern folded from 2D material with geometrical parameters (a, b, \(\upbeta \in (0, \uppi /2)\), \(\upvarphi \in [0, \upbeta /2]\), \({\upalpha }_{1}\in [0, \uppi ]\), and \({\upalpha }_{2}\in [0, \uppi ]\)) and dimensions (L, w, and h). The Miura-ori with light green color represents the geometry of the typical unit cell of Miura-ori, where the width of the crease can be neglected (t = 0). The Miura-ori with dark green color represents the graphene/CNT Miura-ori with folding width t (t ≠ 0). The CNT-O is constructed by connecting the bent graphene Miura-ori unit cells with folding width t. The details of the geometrical parameters of the Miura-ori unit cell can be found in Supplementary information Figure S4. (c) The effect of folding width (t) on the diameter (D) of CNT-O (ρ = 0.5) obtained by MD simulation and theoretical analysis. (d) The effect of hydrogenation density (ρ) on the diameter (D) of the CNT-O (n = 5), after relaxation, as a function of the folding width (t). The variation of the diameter of CNT-O with the increased hydrogenation density is small when the folding width t = 1 × 1.23 Å and 3 × 1.23 Å. We plot these two folding widths for different hydrogenation densities with a fine range to better represent the diameter variation with the increased hydrogenation density.

Full size image

Surface functionalization mechanism

We use a simple 2D carbon-based nanomaterial (i.e., graphene) functionalized by hydrogen atoms to illustrate the mechanism of surface functionalization (Figure 1a−b). The hydrogenation generates an sp3-H bond between hydrogen and carbon atoms, which induces accumulated distortion, transforming a 2D graphene sheet into a 3D graphene origami.27,43 The folding angle (θ) of hydrogenated graphene reaches an equilibrium state after about 400 ps (Figure 1b); folding angle at equilibrium enhances with the increased number of hydrogenated rows (i.e., folding width, t).27,43 The definition of the folding width (t) can be found in Supplementary information Figure S1. It is found that when the hydrogen atoms are applied on both sides of the graphene (Figure 1a), the functionalized graphene expands in the in-plane directions resulting in mechanical strains (ɛxx and ɛyy),44 which exhibit an approximately linear relationship with hydrogenation density (ρ) (Supplementary information Figure S2). In the case of hydrogenation density ρ = 100% (the surface functionalization is applied on both sides of the graphene surface; all the carbon atoms are hydrogenated [see Figure 1a and Supplementary information Figure S2]), the hydrogenation-induced in-plane mechanical strains are around \({\upepsilon }_{\uprho =100\%}=0.0278\). Then, the hydrogenation-induced surface force, resulting in the in-plane expansion of the graphene sheet, can be given by

$$F = E\upepsilon \left( \uprho \right)c,$$
(3)

where \(E\approx 1095\) GPa is the effective stiffness of pristine graphene within a small range of mechanical strain (~0.03) and c = 0.335 nm is the effective thickness of graphene; \(\upepsilon \left(\uprho \right)=\uprho {\upepsilon }_{\uprho =100\%}\) is the mechanical strain induced by surface functionalization with a hydrogenation density ρ. If the hydrogen atoms are applied only on one surface (Figure 1b), a bending moment (M) can be generated by the surface stress,44,45 inducing the graphene folding, which is given by (Figure 1c)

$$M = \frac{Fc}{2} = \frac{{E\upepsilon \left( \uprho \right)c^{2} }}{2}.$$
(4)

Given the bending/flexural modulus of the pristine graphene (K ≈ 0.24 nN⋅nm),46,47,48 based on the classic plate theory, the curvature of the cylindrical folding and the associated folding angle (θ) can be given by44

$$\frac{1}{R} = \frac{M}{K} = \frac{{E\upepsilon \left( \uprho \right)c^{2} }}{2K}$$
(5a)
$$\uptheta = \uppi - \frac{t}{R} = \uppi - \frac{{E\upepsilon \left( \uprho \right)c^{2} t}}{2K}.$$
(5b)

As shown in Figure 1d, the hydrogenation-induced folding angles calculated by theory based on Equation 5b fit well with the MD simulation results; the folding angle decreases when the folding width increases. It is worth mentioning that in this study the highest hydrogenation density on one side of the surface is ρ = 0.5, and the largest folding width is t = 9 × 1.23 Å. When the hydrogenation density is much higher than 0.5, the functionalized graphene cannot reach a stable state during the relaxation process in MD simulation, and forms defects on the surface of graphene, resulting in failure, instead of reconfiguration after relaxation.27,43 To avoid forming defects during the surface functionalization, the hydrogenation density is controlled to not exceed 0.5.

Results and discussion

Functionalized CNT origami

Figure 2a shows the schematic of the surface functionalized CNT-O by hydrogen atoms. The hydrogenation is applied to the carbon atoms within the area marked by yellowish solid lines on the outside of the CNT to generate mountain folds. On the inside of the CNT, the hydrogen atoms are placed on the top of carbon atoms within the area enclosed by the yellowish dashed lines to generate valley folds. After the full relaxation in MD simulation, the functionalized CNT reaches a minimum-energy equilibrium state, transforming a CNT into a CNT-O with well-known Miura-ori origami patterns characterized by crease lengths (a = 79.577 Å and b = 115.62 Å), acute angle between creases (β = 30°), and folding width (t), as shown in Figure 2a. The detailed relaxation process of hydrogenated CNT in MD simulation can be found in Supplementary information Figure S3. The hydrogenation formed sp3 carbon–hydrogen bonds induces local bending, which is affected by the folding width (t) and the hydrogenation density (ρ is defined as the ratio between the number of hydrogen atoms and carbon atoms within the crease area), resulting in the shape morphing of CNT. Because the one-dimensional (1D) CNT has a cylinder-like architecture constructed from the rolling up of single-layer graphene with a nanometer-range diameter, the CNT-O can be considered to be formed by bending/rolling the graphene Miura-ori (Figure 2b). To understand the relationship between the diameter of CNT-O and folding width, we assume the centerline length (L + 2t) and the height (h) of the graphene Miura-ori cell does not change during the bending (Figure 2b). Hence the diameter of CNT-O is given by

$$D = \frac{{n\left( {L + 2t} \right)}}{\uppi } + h,$$
(6)

where n is the number of graphene unit cells in CNT-O (e.g., n = 6 in Figure 2b) and L + 2t is the length of the graphene Miura-ori unit cell. Figure 2b shows the schematic of the Miura-ori unit cell before bending/rolling. The outer dimensions of Miura-ori without considering the folding width t are then given by22,28

$$L = 2b\sin \left( {{\upvarphi \mathord{\left/ {\vphantom {\varphi 2}} \right. \kern-0pt} 2}} \right)$$
(7a)
$$w = 2a\frac{\cos \upbeta }{{\cos \left( {{\upvarphi \mathord{\left/ {\vphantom {\varphi 2}} \right. \kern-0pt} 2}} \right)}}$$
(7b)
$$h = \frac{{a\sqrt {\sin^{2} \upbeta - \sin^{2} \left( {{\upvarphi \mathord{\left/ {\vphantom {\varphi 2}} \right. \kern-0pt} 2}} \right)} }}{{\cos \left( {{\upvarphi \mathord{\left/ {\vphantom {\varphi 2}} \right. \kern-0pt} 2}} \right)}},$$
(7c)

where \(\varphi \in [0^\circ ,2\upbeta ]\) is the projection angle between two adjacent “valley” creases, characterizing the folding of the Miura-ori unit cell; φ = 0° and 2β represent the completely collapsed and planar states, respectively. Considering the folding width t, the length and width of the graphene Miura-ori is L + 2t and w + 2t while h does not show a significant change (Figure 2b). Another two dihedral angles \({\upalpha }_{1}, {\upalpha }_{2}\in [0^\circ ,180^\circ ]\) can also characterize the geometry of Miura-ori, which are given by

$$\upalpha_{1} = \cos^{ - 1} \left[ {1 - 2\frac{{\sin^{2} \left( {{\upvarphi \mathord{\left/ {\vphantom {\varphi 2}} \right. \kern-0pt} 2}} \right)}}{{\sin^{2} \upbeta }}} \right]$$
(8a)
$$\upalpha_{2} = \cos^{ - 1} \left[ {1 - 2\cot^{2} \upbeta \tan^{2} \left( {{\upvarphi \mathord{\left/ {\vphantom {\varphi 2}} \right. \kern-0pt} 2}} \right)} \right].$$
(8b)

The dihedral angle (i.e., folding angle) in graphene origami is induced by hydrogenation, which is dependent on the folding width and hydrogenation density.27 Based on Equations 6–8, we can theoretically obtain the diameter of CNT-O with a given cell number (n) and the dimension of graphene Miura-ori unit cell. For simplicity, the CNT-O characterized by (n, m), where n is the number of the cells along the circumference of CNT-O and m means the folding thickness t = m × 1.23 Å, is written as CNT-O (n, m) in the following text. Figure 2c shows the effect of folding width (t = m × 1.23 Å, m = 1, 3, 5, 7, and 9) on CNT-O diameter for different cell numbers (n = 2, 3, 4, 5, and 6). The theoretical analysis and the simulation results show a good agreement except for the folding width t = 9 × 1.23 Å. The deviation could emanate from (1) CNT-O cannot be completely collapsed/folded because of the van der Waals force between adjacent CNT origami panels; (2) rolling up the completely collapsed graphene Miura-ori to CNT-O could cause the unfolding of the creases, especially for limited cells (e.g., n = 2). Hence, the simulation results are higher than the theory when t = 9 × 1.23 Å, as shown in Figure 2c. In addition, defects are found near the creases during the relaxation process when t = 9 × 1.23 Å (see Supplementary information Figure S5). Hence, the CNT-O with folding thickness t = 9 × 1.23 Å is excluded in the following study. Considering the effect of hydrogen density on the CNT-O diameter, the hydrogen atoms within the crease region are deleted randomly to generate creases with different hydrogen densities (ρ = 0.1, 0.2, 0.3, 0.4, and 0.5). Results in Figure 2d show that the diameter of CNT-O (n = 5) decreases with the increased hydrogen density for all folding widths. To better present the variation of the diameter with the increased hydrogenation density when folding width t = 1 × 1.23 Å and 3 × 1.23 Å, we plot these two folding widths for different hydrogenation densities with a fine range in Figure 2d.

Mechanical properties of CNT origami

After obtaining the stable CNT-O by surface functionalization, we further study the effect of folding widths (t) and numbers of the cells (n) on the mechanical response of CNT-O. Figure 3a shows a typical stress–strain response and mechanical deformation of CNT-O (6, 5) upon uniaxial compression/tension in the z-direction. We also show the atomic configurations of CNT-O at different mechanical strains (ɛzz = −0.100, 0.000, 0.266, and 0.268) in Figure 3a. Here, we need to clarify the CNT/CNT-O stress calculation. As shown in Equation 1, the axial stress is calculated by averaging the virial stress of all atoms in the system. Hence, the definition of V0 is critical for stress calculation. To compare the mechanical properties between CNT-O and CNT, \({V}_{0}={l}_{x}{l}_{y}{l}_{z}\) is defined as the volume of the outside cuboid of the CNT/CNT-O unit cell at the equilibrium state (see Figure 3b and Supplementary information Figure S6). In addition, the corresponding CNT shares the same diameter with the CNT-O before relaxation (Supplementary information Figure S6). Three distinct regimes can characterize the CNT-O stress–strain response: the densification of CNT-O in compression (Regime I); a plateau stress regime caused by the folding/unfolding of creases and the bucking (e.g., (i) in Figure 3a) of CNT-O panels in compression (Regime II); and the stretching of carbon atomic bonds (e.g., (iii) in Figure 3a) in tension (Regime III). It is found that the stress increases slightly with increased strain in Regime II; also, the potential energy of CNT-O seems unchanged in this regime, indicating the low stiffness of CNT-O. Further observation of the von Mises stress distribution in CNT-O (see atomic configurations in Figure 3a) finds that the stress is concentrated in origami creases, revealing that the low stiffness results from the hydrogenated creases (e.g., (iii) in Figure 3a). Finally, the crack forms at the junction of creases and propagates rapidly, leading to a brittle failure of CNT-O ((iv) in Figure 3a). We also apply cycle tensile loading/unloading on CNT-O in Supplementary information Figure S7; the overlapped stress–strain responses imply that the deformation of CNT-O is reversible, and CNT-O has excellent mechanical resilience.

Figure 3
figure 3

Mechanical response of carbon nanotube origami (CNT-O). (a) The stress–strain curve of CNT-O (n = 6, t = 5 × 1.23 Å) and the atomic potential energy with respect to the applied tensile/compressive strains. The atomic configurations of CNT-O (n = 6, t = 5 × 1.23 Å) at different mechanical strains (ɛzz = –0.100, 0.000, 0.266, and 0.268; the corresponding positions in the stress–strain curve are marked by asterisk signs) are shown. Contour presents the von Mises stress distribution. (b) The definition of the initial effective volume (\({V}_{0}={l}_{x}\times {l}_{y}\times {l}_{z}\)) of the CNT and CNT-O at the equilibrium state (after relaxation in molecular dynamics (MD) simulation) for calculating the stress. (c) The effect of folding width (t) on failure strain (ɛf), stiffness (E), and ultimate tensile stress (σu) as a function of the number of cells along the circumference of CNT-O (n = 2, 3, 4, 5, and 6). Here, the mechanical properties (e.g., stiffness and ultimate strength) of pristine CNT are calculated based on V0 as shown in Figure 3b. For comparison, the diameter of pristine CNT is the same as the diameter of CNT-O before relaxation in MD simulation. The detailed mechanical properties of pristine CNT with different diameters can be found in Supplementary information Table SI.

Full size image

Next, we turn our attention toward three specific mechanical values (i.e., failure strain εf, stiffness E, and ultimate strength σu) to evaluate the mechanical properties of CNT-O in Figure 3c. The detailed mechanical properties of pristine CNT can be found in Supplementary information Table SI. The surface functionalization-induced origami feature increases the stretchability through the unfolding of creases. In contrast, hydrogenation also causes stress concentration (see Figure 3a), decreasing the failure strain. Hence, the tradeoff between the unfolding of creases and the stress concentration determines the failure strain of CNT-O. Compared with the pristine CNT, the stretchability of CNT-O is increased in the case of samples with more cells (n) and large folding width (t). In general, the failure strain of CNT-O increases with increased folding width and number of cells along the circumference of CNT-O. Because the stiffness of CNT-O is related to the unfolding of the creases subjected to tension, the stiffness of CNT-O is much smaller than that of pristine CNT (Figure 3c). For example, the stiffness of CNT-O (2,7) is 3.373 GPa, while the stiffness of the corresponding CNT is 107.046 GPa. It is worth mentioning that this stiffness is calculated based on the volume of the outside cuboid, which varies with the diameter of the CNT. If the stiffness of CNT is calculated based on the volume of the cylinder, the calculated stiffness is 899.108 GPa and independent of the CNT diameter (see Supplementary information Figure S6 and Table SI). Interestingly, the stiffness of CNT-O shows an opposite trend with increased folding width and number of cells along the circumference of CNT-O compared to the trend of the failure strain. We also report the ultimate strength of CNT-O in Figure 3c. In the case of samples with more cells (n) and large folding width (t), the ultimate strength of CNT-O is found to be even higher than that of CNT due to the decreased initial volume (V0) induced by hydrogenation. In return, the hydrogenation of carbon atoms causes the stress concentration around creases (Figure 3a), resulting in the decreased strength in most cases of CNT-O. It is worth mentioning that the ultimate strength of CNT-O does not show a clear trend to the variable parameters (t and n) compared to the contour plots of failure strain and stiffness. It is due to that the stress calculation is dependent on the effective cross-sectional area of CNT-O, and both parameters (t and n) show a nonlinear relationship between the diameter of CNT-O (Figure 2c). Generally, CNT-O can realize the increase of stretchability and strength simultaneously through geometrical parameter engineering.

Tunable auxeticity of CNT-O

The most interesting property of the Miura-ori-related structures is the strain-dependent negative Poisson’s ratio.22,49 Figure 4a presents the axial stress and transverse strain of CNT-O (6, 5) as a function of loading strain. The error area shown in the yellow curve in Figure 4a results from the difference between the transverse strain of CNT-O (6, 5) in the x- and y-directions. To better exhibit the auxeticity of CNT-O, the atomic configurations of CNT-O (6, 5) in different tensile/compressive strains are shown in Figure 4b. The diameter of CNT-O (6, 5) is found increased with the increased tensile strains and decreased with the increased compressive strains, indicating a negative Poisson’s ratio of CNT-O. Figure 4c shows the outer edge of CNT-O characterized by different folding widths under certain strains. In addition to the negative Poisson’s ratio of CNT-O observed in Figure 4c, the change of the CNT-O diameter is more evident in samples with larger folding width (i.e., CNT-O (6, 7)), indicating that the Poisson’s ratio of CNT-O decreases with the increased folding width. Moreover, the distance between the adjacent outer edge is variable, which seems to decrease with the increased tensile strains, revealing the strain-dependent Poisson’s ratio of CNT-O.

Figure 4
figure 4

Tunable auxeticity of CNT-O. (a) Axial stress (σzz) and transverse strain (ɛxx, ɛyy) of carbon nanotube origami (CNT-O) (6, 5) as a function of tensile/compressive strain (ɛzz). (b) The atomic configurations of CNT-O (n = 6, t = 5 × 1.23 Å) in different tensile/compressive strains. The diameter of CNT-O is observed to increase with the increased tensile strain and decrease with the increased compressive strains, indicating a negative Poisson’s ratio. (c) The evolution of the CNT-O outer edge subjected to tensile/compressive strains. The CNT-O is characterized by (n, m), where n is the number of the cells along the circumference of CNT-O and m means the folding thickness t = m × 1.23 Å. (d) The Poisson’s ratio (v) of CNT-O (n = 6) characterized by different folding widths obtained by molecular dynamics (MD) simulation and theoretical analysis. The effect of (e) the number of circular cells (n = 2, 6, 20, 100, and 1000) and (f) folding thickness (t = 1.23 Å, 3.69 Å, 6.15 Å, 8.61 Å, and 11.07 Å), on v as a function of folding angle (α1). (g) The effect of the angle between creases (\(\upbeta \in (0, \uppi /2)\)) on \(ln(-v)\) of CNT-O (n = 100 and t = 5 × 1.23 Å) as a function of folding angle (α1).

Full size image

To better understand the auxeticity of CNT-O, we calculate the Poisson’s ratio of CNT-O theoretically. Assuming that the panels that constitute CNT-O are rigid, the thickness of CNT-O is neglected, and the creases are ideal (the stiffness of creases is much smaller than that of panels), the Poisson’s ratio (v), defined as the ratio between the strains in the transverse direction and the loading direction, is given by

$$\nu = - \frac{{{{dD} \mathord{\left/ {\vphantom {{dD} D}} \right. \kern-0pt} D}}}{{{{d\left( {w + 2t} \right)} \mathord{\left/ {\vphantom {{d\left( {w + 2t} \right)} {\left( {w + 2t} \right)}}} \right. \kern-0pt} {\left( {w + 2t} \right),}}}}$$
(9)

where \(dD\) and \(d\left(w+2t\right)\) refer to the derivations of the diameter and height of CNT-O, respectively. Based on Equations 6 and 7b, \(dD/D\) and \(d(w+2t)/(w+2t)\) can be obtained as

$$\frac{dD}{D} = \left\{ {\frac{{bn\cos \left( {{\upvarphi \mathord{\left/ {\vphantom {\varphi 2}} \right. \kern-0pt} 2}} \right)}}{\uppi D} - \frac{{a\,\sin \left( {{\upvarphi \mathord{\left/ {\vphantom {\varphi 2}} \right. \kern-0pt} 2}} \right)\cos^{2} \left( \upbeta \right)}}{{2D\cos^{2} \left( {{\upvarphi \mathord{\left/ {\vphantom {\varphi 2}} \right. \kern-0pt} 2}} \right)\sqrt {\sin^{2} \upbeta - \sin^{2} \left( {{\upvarphi \mathord{\left/ {\vphantom {\varphi 2}} \right. \kern-0pt} 2}} \right)} }}} \right\}d\upvarphi$$
(10a)
$$\frac{{d\left( {w + 2t} \right)}}{{\left( {w + 2t} \right)}} = \frac{{a\cos \left( \upbeta \right)\sin \left( {{\upvarphi \mathord{\left/ {\vphantom {\varphi 2}} \right. \kern-0pt} 2}} \right)}}{{2\cos \left( {{\upvarphi \mathord{\left/ {\vphantom {\varphi 2}} \right. \kern-0pt} 2}} \right)\left[ {a\cos \upbeta + t\cos \left( {{\upvarphi \mathord{\left/ {\vphantom {\varphi 2}} \right. \kern-0pt} 2}} \right)} \right]}}d\upvarphi.$$
(10b)

Substituting Equation 8a into Equations 10a and 10b, the Poisson’s ratio of CNT-O characterized by different geometrical parameters (a, b, β, t, and n) can be expressed as a function of dihedral angle α1 (\({\upalpha }_{1}\in (0^\circ , 180^\circ )\)). α1 = 0° and 180° represent the completely collapsed state and the completely unfolded state, respectively. Figure 4d displays the Poisson’s ratio of CNT-O (n = 6) characterized by different folding widths obtained by theoretical analysis and MD simulation. The MD results are obtained by calculating the slope of the transverse-loading strain of CNT-O in the strain interval from −1 percent to 1 percent. The theoretical results are calculated based on Equations 8a, 9, and 10 with certain dihedral angle α1 of the corresponding CNT-O at the equilibrium state. Both the theory and the simulation show that Poisson’s ratio decreases with the increased folding thickness. The deviation between the theory and simulation results from three aspects: (1) The theory of the Poisson’s ratio is driven based on the assumption of the rigid panel in CNT-O while panels of CNT-O are flexible/deformable in MD simulation; (2) the effective thickness of CNT-O cannot be neglected when α1 is too small (i.e., large folding thickness in Figure 4d); and (3) with a limited number of cells (n) along the circumference of CNT-O, the determination of the dihedral angle α1 in 3D space loses accuracy with decreased folding width. This inaccuracy of α1 can also be observed in Figure 4e when discussing the effect of n on the Poisson’s ratio of CNT-O. The Poisson’s ratio equals zero, meaning that the CNT-O is in a completely unfolded state. In the theoretical analysis, this state is reached when α1 = 180 degrees. However, we find that the α1 is smaller than 180 degrees when the Poisson’s ratio of CNT-O is zero for the sample with small n (e.g., n = 2, 6, and 20 in Figure 4e). This is because the Miura-ori unit cells of CNT-O with limited n are in a curved shape when the origami creases are unfolded. It is also found in Figure 4e that the Poisson’s ratio of CNT-O increases with the decreased n at the same α1. Figure 4f shows the effect of folding thickness on the CNT-O’s Poisson’s ratio (n = 100 and β = 30°), which increases with the increased folding thickness at the same α1. In Figure 4g, we show the effect of β on the Poisson’s ratio of CNT-O (n = 100 and t = 5 × 1.23 Å) at different α1 and find that the Poisson’s ratio of CNT-O increases with the increased α1 and β.

Scale-independent auxeticity

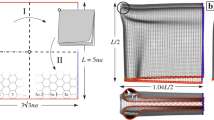
To further understand the scale-independent Poisson’s ratio of the Miura-ori tube, we fabricate the Miura-ori tube structures out of the paper. In the manufacturing process shown in Figure 5a, the creases of Miura-ori patterns are first introduced by laser cutting (Trotec, Speedy 100) with dotted lines (characterized by geometrical parameters a, b, and β) followed by the hand-folded Miura-ori sheet. The Miura-ori tubes are then fabricated by rolling the Miura-ori sheet and attaching their two opposite edges to each other with glue. Three samples characterized by a = b = 25 mm, and β = 45°, 60°, and 75°, comprising 15 × 5 Miura-ori unit cells (15 unit cells and 5 layers along the circumference and the axial directions of the tube, respectively) are fabricated, as shown in Figure 5b–d. To study the auxeticity property of Miura-ori tubes, the Miura-ori tubes are first placed in a completely collapsed state and fixed at the two ends by the grippers of a uniaxial tensile test machine (ADMET, eXpert 8612) ((I) in Figure 5b–d). Then the top gripper goes up slowly, and the deformations of Miura-ori tubes are recorded using a digital camera (Sony RX100IV). The snapshots of Miura-ori tubes subjected to certain deformation strains are shown in Figure 5b–d. It is found clearly that the Miura-ori tubes expand significantly with the increased strains (i.e., the increased axial length). Here, the axial length of Miura-ori tubes (W) is directly read from the testing machine whereas the diameter (D) is obtained by averaging the three-time tests of the central layer (e.g., (I) in Figure 5b–d). Such scale-independent auxeticity response of Miura-ori tubes can be characterized and predicted by the kinematics of the folding/unfolding, as concluded in Equations 9 and 10. However, considering the limited unit cells in the axial direction of Miura-ori tubes and the effect of the tail,50 the axial length (W) is given by

$$W = 2qa\frac{\cos \upbeta }{{\cos \left( {{\upvarphi \mathord{\left/ {\vphantom {\varphi 2}} \right. \kern-0pt} 2}} \right)}} + b\cos \left( {{\upvarphi \mathord{\left/ {\vphantom {\varphi 2}} \right. \kern-0pt} 2}} \right),$$
(11)

where q represents the layer of the Miura-ori cells along the axial direction of the Miura-ori tube and q = 5 in all three samples. The diameter of Miura-ori tubes with different axial lengths (i.e., subjected to different strains) can be predicted by Equations 6 and 11 with t = 0. As shown in Figures 5b–d, we find a good agreement between the theoretical predictions and experimental results, which reveals the scale-independent auxeticity of Miura-ori tubes. The deviation between the theory and experiments results from three main factors: (1) the defects/imperfection introduced during the fabrication (i.e., hand folding and rolling); (2) the boundary condition effect resulting from the limited layers of cells along axial direction; and (3) error in measuring the diameter of samples.

Figure 5
figure 5

The experiment of Miura-ori tubes. (a) The manufacturing process of a Miura-ori tube made out of paper that includes laser cutting, hand folding, and rolling. The auxeticity of Miura-ori tubes characterized by a = b = 25 mm, and (b) β = 45°, (c) β = 60°, and (d) β = 75°, obtained by experimental test and theoretical analysis. The column values inside the right graph represent the diameter (D) of Miura-ori tubes. For each sample at certain strains, the diameter of the Miura-ori tube is tested for three times. The experimental and theoretical results indicate the scale-independent and strain-dependent negative Poisson’s ratio of the Miura-ori tube.

Full size image

Concluding remarks

In summary, we have combined MD simulation and theoretical analysis to demonstrate that the surface functionalization-assisted origami strategy imparts a new platform for designing CNT metamaterials with tunable mechanical properties. Specifically, we have shown that altering the folding width, topological parameters of Miura-ori patterns, and the number of cells along the circumference of Miura-ori CNTs can tune stiffness, ultimate strength, failure strain, and the range of attainable negative Poisson’s ratios. Our results demonstrate that applying mechanical strains can further tune Poisson’s ratio of hydrogenated CNT-O. Additionally, comparing the experimental results of a Miura-ori tube made by folding/rolling a paper at the macroscale and the atomic scale numerical simulation results for nanoscale origamis confirms that the strain-dependent negative Poisson’s ratio governed by the kinematics of the crease folding/unfolding is scale-independent, and can be well predicted by the proposed continuum theory. By systematic design of CNT-O, we are not only able to enhance the mechanical properties (i.e., failure strain and ultimate strength in some cases) and achieve exotic properties (e.g., negative Poisson’s ratio), but we can also tailor the underlying architectural parameters (e.g., a, b, β, t, and n) to engineer and program the properties of CNTs. The present study provides new opportunities for the realization of the next generation of low-dimensional nanomaterials capable of achieving tunable mechanical properties and auxeticity for applications in wearable nanoelectronics and soft nanorobots.