Avoid common mistakes on your manuscript.
Karaṇapaddhati by Putumana Somayājī is a treatise on the vākya system of astronomy, that forms the basis of the ephemerides most commonly used today among Tamils, in India or abroad: the vākkiya pañcāṅgam or “ephemerides [based on] phrases”. In this system, very accurate constants are encoded in the form of sequences of letters with a numerical value, chosen to make meaningful phrases,Footnote 1 for ease of memorization. These constants have been constantly improved in the light of new observations and theories. The authors stress two aspects of Karaṇapaddhati: it fully explains the construction of the elements of the vākya system, and contains mathematical results not found earlier elsewhere, particularly on rational approximation. They suggest the time frame 1532–1566 as most likely, for reasons spelled out in their introduction. Karaṇapaddhati is “not a manual prescribing computations; rather it enunciates the rationale behind such manuals” (xxx).Footnote 2 It also has a wider scope: “[a]ll the topics necessary to make the [ephemeris] are not treated [in it], whereas several other items not pertaining to manuals are dealt with” (ibid.).
This volume provides the text with an English translation and a modern mathematical commentary based on earlier work, including the edition by Sāmbaśiva Śāstrī in 1937, and the one by P. K. Koru, published in 1953 with detailed notes in Malayalam, as well as other texts in manuscript form, in addition to the sizeable secondary literature. It is a welcome complement to the recent publication of two important works of the same school and period, namely Tantrasaṅgraha by Nīlakaṇṭha Somayājī (1444–1545),Footnote 3 and Yuktibhāṣā (c. 1530) by Jyeṣṭhadeva.Footnote 4 As is now well-known, both works document important innovations, namely power series for the arctangent, sine and cosine functions, as well as decisive steps towards heliocentrism. While there is significant overlap between Karaṇapaddhati and these two works, its relation to them seems complex: “the question as to whether Putumana Somayājī was indeed aware of and followed the modified planetary model of Nīlakaṇṭha is still an open question” (216).
1 Structure of Karaṇapaddhati of Putumana Somayājī
Roddam Narasimha’s foreword sets the stage by recalling that the vākya system was noticed by historians as early as the late eighteenth century (xxv–xxvi). This work is among the first primary sources of the history of ancient Indian science, and one of the last to be translated. After some information about the authorsFootnote 5 (xxvii), the Introduction (xxix–xlviii) describes source materials, discusses the date of the text and summarizes its contents. The ten chapters of Karaṇapaddhati contain 214 Sanskrit verses in various meters, given here both in Nagari script and in transliteration, with translation and commentary (1–317); this material is supplemented by eight appendices (A to H) (319–407), a glossary (409–424), a bibliography (425–432), an index (433–444), and an index of half-verses (445–450). The (standard) transliteration scheme and a conversion table for kaṭapayādi numerals are found on pages v and 3 respectively. The many constants that occur in the text are collected in convenient tables of which a list is found pp. xix–xxiii, after the contents (vii–xiii) and the list of figures (xv–xvii). In particular, the main constants, for each celestial body, are given in Appendices C and D, with worked-out examples of calculations. Appendices G and H show that it is very likely that the constants listed without derivation in two other texts were generated by the methods of Karaṇapaddhati or closely related ones. The concepts and technical terms of Indian astronomy are used throughout, with approximate modern equivalents often provided as well. Background information on the models underlying the determination of true longitudes in Chapter 7, and on the vākya system, is provided in Appendices B and D respectively, with references. The reader unfamiliar with Indian astronomy may also want to refer to the references in n. 5 on page xxx, and to the recent translation and analysis of Tantrasaṅgraha (Ramasubramanian and Sriram, op. cit., esp. its App. F). The vākyas for the Moon, both in Vararuci’s and Mādhava’s versions, are presented in App. E; the latter “give the true longitudes correct to a second” (365), as opposed to a minute for the former. Note the corrections to vākyas 25, 174, 181, 234 and 242 (and of a misprint in vākya 98 found in earlier editions); the process of correction is worked out in each case.
Karaṇapaddhati opens with a brief but pregnant maṅgalācaraṇa, the first half of which is a “signature verse” often used to identify works by this author (xxxiii). “This is perhaps a unique case of a famous Indian astronomer whose actual [personal] name is not found mentioned anywhere either in his works or in the commentaries” (xxxiii). Putumana is the name of his illam “house” and Somayājī suggests that he performed a Soma ritual. The work’s concluding verses (10.11–12) mention Viṣṇu as being also kālarūpa “of the form of time”, and refer to the author as “someone” (ko’pi) hailing from ŚivapuraFootnote 6 and who is a yajvā, deliberately withholding his name. By contrast, the opening only pays respects to the navagrahas and to the guru who is cidānandamaya, arising in the author’s hṛdākāśa “space within the heart”, without direct reference to Viṣṇu or Gaṇapati as might be expected. Perhaps both could be reconciled if we take the signature verse as a reference to the daharavidyā.Footnote 7 The author would then mean that Brahman, that is classically saccidānanda, identified with the inner guru, resides in a minute space (dahara) within his heart. Referring to the author of his work as ko’pi would then carry the suggestion that, for him, all results should be attributed to the inner guru who is not different from the Brahman.Footnote 8 This would mean that Putumana Somayājī had strong philosophical leanings.
The opening verse is followed by a discussion of mean motions and the calculation of the ahargaṇa group of days” or number of civil days from the beginning of a convenient epoch. Since the ahargaṇa from the beginning of Kaliyuga has typically seven digits, and the multipliers and divisors required to compute mean or true motions are even larger, the vākya system introduces reduced epochs, called khaṇḍa-s “portions”, at the beginning of which the planetary positions are simple to obtain; thus, for the Moon, a khanḍa is “a day close to the ahargaṇa when the anomaly is close to zero at the mean sunrise” (78). Mean longitudes at the end of a khaṇḍa are called dhruvasFootnote 9 “fixed”. Chapters 3 to 5 proceed to determine these khaṇḍas and dhruvas for various bodies and to generate a sequence of finer and finer rational approximations to the various periods involved in the correction processes prescribed by the relevant astronomical model. The mathematics of the process is an outgrowth of the technique of vallyupasaṃhāra “reduction of the creeper” familiar in the solution of simultaneous congruence problems. Recall that the kuṭṭākāra method for solving congruences is based on the list of quotients in mutual division of the two moduli involved, arranged vertically, forming a vallī “creeper”, hence the name of the method.
Chapter 6 of Karaṇapaddhati starts a second line of thought centered around the sine and arctangent functions and series. Many passages are reminiscent of earlier work: the generation of sines by halving arcs (154–162) is similar to Brahmagupta’s procedure in chapter 21 of his Brāhmaspuṭasiddhānta, and the iterative method of Sect. 6.9 (166–166) seems to be a rationale for Āryabhaṭa’s list of sines.Footnote 10 The exact series expansions for the sine and arctangent are also familiar since the publication of Yuktibhāṣā. However, the authors stress that the combination of consecutive terms of series (6.10) (Prop. 6.4) for the circumference, to obtain a series with non-negative terms of order \(1/{n}^{4}\), see Eq. (6.12), does not seem to be found in other sources. The way the author indicates its derivation is noteworthy and typical of Indian discursive strategies: Prop. 6.1 gives Mādhava’s (slowly convergent) series for the circumference of a circle in terms of its diameter. Prop. 6.2 gives the result of the transformation of the series by acceleration of convergence, and Prop. 6.4 describes the series (6.12) in which terms have been grouped in pairs. In-between, we find the seemingly anticlimactic Prop. 6.3 that simply explains how to reduce expressions of the form \(\frac{d}{{h}_{1}}\pm \frac{d}{{h}_{2}}\) to the same denominator – a result known for over a millennium in India. We suggest that this proposition expresses that Prop. 6.4 is obtained by applying Prop. 6.3 to the result of Prop. 6.2 – which is consistent with the derivation proposed by the editors in Eq. (6.14). This is an example of what may be called an apodictic discourseFootnote 11 (a motivated and conclusive discourse that is a proof in itself).
The following chapters give numerous applications of trigonometric relations to astronomical models. Chapter 7 shows how to obtain planetary longitudes and the associated vākyas. Chapter 8 contains a detailed study of gnomonic shadow and its application to the determination of quantities such as latitude (§ 8.5–7), the declination of the Sun (§ 8.9) and Moon (§ 8.13), the apparent dimensions of celestial bodies (§ 8.19–20), to name a few. Chapter 9 is devoted to several methods for finding the madhyāhnakālalagna “which is the time interval between the rise of the [vernal point] and the instant when a star with a non-zero latitude is on the meridian. Algorithms [for its determination] have no equivalents in Tantrasaṅgraha. These algorithms [pp. 291–303] involve very careful analysis of the properties of spherical triangles” (285). While modern methods are freely used by the authors,Footnote 12 derivations closer to the conceptual framework of the text have been proposed more recently by two of them.Footnote 13 Chapter 10 determines the right ascension (natakāla or vāyukāla) and the longitude from it, or from the madhyāhnakālalagna.
The concluding lines of the work (10.12–13) have already been discussed earlier in this review.
Regarding methods, the basic relations on rational approximations in the text, that the authors rightfully stress as significant, are proved by them using continued fractions (Appendix A). They state that Putumana Somayājī’s method “is essentially the same as the technique of computing the convergents of a continued fraction” (66), implying (correctly) that continued fractions appear nowhere in the text and indeed, in no work of the Āryabhaṭa school. We show that the text suggests a different derivation, so that continued fractions may be eschewed altogether.
2 Did Putumana Somyājī work with continued fractions?
This is a moot point since continued fractions do not seem to be attested in India or elsewhere before 1613.Footnote 14 Brahmagupta gives, in his Brāhmasphuṭasiddhānta from 628 a classification of compound fractional expressions, and their reductions to the multiplier-over-divisor form, that does not include continued fractions (Prop. 12.8–9); similar lists occur in later works. If continued fractions were a necessary tool for his solution of the congruence problem, he would have included them in his classification. Actually, C.-O. Selenius observed that the Indian solution of congruences and of the varga-prakṛti problem cannot be reproduced exactly using any known variant of the continued fraction process; he proposed a new variant that would mimic it,Footnote 15 but did not suggest that Indian authors used his method, only that their results are optimal.
The tool that is used systematically by Indian authors since Āryabhaṭa (499) is division with remainder and more precisely, (iterated) mutual division. Starting from two quantities a and b, one divides a by b, keeping aside the quotient q, and replaces a by the remainder r = a – bq. One then applies the same process to the divisor b and the remainder r. And this procedure is iterated. This method of mutual division – which treats division as a symmetric operation! – does not seem to be attested outside IndiaFootnote 16; it is sometimes identified with the Euclidean algorithm from the beginning of Book VII of the Elements, but this is a misnomer: Euclid’s algorithm is a mutual subtraction algorithm, for finding the “common measure” of two lines, in which the quotients of division are never introduced. There is no evidence that it was the source of the mutual division method. Continued fractions seem to have been developed first in Renaissance Italy in connection with methods of square root extraction similar to those in the Bakhshālī manuscript. It appears that Indian mathematicians did not introduce continued fractions because they had at hand a mathematical tool that made them unnecessary.
It has recently been shown that Brahmagupta provided at the end of the twelfth chapter of Brāhmasphuṭasiddhānta a derivation of the kuṭṭākāra method based solely on mutual division.Footnote 17 This suggests that Putumana Somayājī’s main result on the reduction of the creeper also is a natural modification of the mutual division technique, and that he intimated this point through the discursive structure of his exposition.Footnote 18 Let us show that this is the case.
First, recall that the “creeper”, or list of quotients, is constructed from the mutual division of a by b by arranging the quotients one under the otherFootnote 19 in a vertical column, followed (typicallyFootnote 20) by 1. The standard operation called vallyupasaṃhāra enables one to modify and shorten the list of quotients to produce the numerators and denominators of fractions closely related to a/b, including convergents in the sense of the theory of continued fractions, by replacing iteratively the last three terms, say u; v; w, by the two terms uv + w; v. This reduction step may be viewed as a reverse of the division process: if \(0\le w<v\), then \(u\) is the quotient of the division of \(uv+w\) by \(v\), and \(w\) is the remainder. The creeper is shortened by one term at each step of reduction, and the process terminates when only two quantities are left in the creeper. One can show that this operation, carried to the end, enables one to recover the denominator and numerator b and a if the fraction is in lowest terms; if the division process is stopped at some intermediate stage, and the remainder in the last division that has been performed is neglected, one obtains in this way an approximation of the fraction.
Now, Putumana Somayājī, after describing this standard procedure in Prop. 2.5, observes in Prop. 2.6 (see Sects. 2.5.1–2) that it is possible to obtain the same denominator by putting 1 before the list of quotients rather than after, and performing the reduction of the creeper from the top rather than from the bottom. He adds that the numerator is obtained from a similar list in which the first quotient is omitted. Thus, in this case, two creepers are needed. It is the truncation of this inverted form of the creeper that yields the sequence of simplified fractions that plays a central role in his work. His main theorem expresses that the reduction of a creeper generates the same final number as the reduction of the inverted creeper with the same quotients, this number being the denominator or numerator of the desired fraction according to the creeper in question. Thus, Putumana Somayājī showed by the mere sequence of his propositions that the main new point in his discourse was the inversion of the order of quotients, implying that everything else follows from it.
Let us show that Putumana Somayājī’s theorem is correct and implies the recursion relations (A.11–12) that, as is shown in Appendix A, immediately imply all of his other results. Consider the reduction of a vallī \({q}_{1};\,{q}_{2};\,{ q}_{3};\,{\ldots;\,\,q}_{n} ; 1\) (reduced from the right). The procedure terminates when only two terms, say \({Q}_{n};\,\,{Q}_{n}^{{\prime}}\), remain. Similarly, the reduction of \(1;\,\,{q}_{1};\,{q}_{2};\,{ q}_{3};\,{\ldots;\,q}_{n}\) (starting from the left), leads to two terms that we call \({P{^{\prime}}}_{n} ;{P}_{n}\). Equivalently, the reduction of \({q}_{n}{ ; \,q}_{n-1};\,{ q}_{n-2};\,{\ldots;\,q}_{1}; 1\) (reduced from the right) leads to \({{P}_{n }; P}_{n}^{{\prime}}\). Putumana Somayājī’s result is that \({P}_{n}={Q}_{n}\). In other words, \({Q}_{n}\) remains unaltered when the order of the quotients is reversed.
This result may be proved by observing that the \({Q}_{n}\) are formed by a rule which is not altered when the order of the quotients \({q}_{k}\) is reversed. Indeed, examination of creepers with 2, 3, 4 and 5 termsFootnote 21 suggests that \({Q}_{n}\) may be obtained by the following rule:
First form the product \({q}_{1}{q}_{2}\ldots {q}_{n}.\) Then divide through by the products of pairs of adjacent terms \({q}_{k}{q}_{k+1}\), one pair at a time. Iterate the process as long as there are two or more factors left. Finally, add all the terms thus obtained (which include 1 if \(n\) is even), counting each term once.
All that remains is to prove that \({Q}_{n}\) is indeed given by this rule. We work out the argument by induction. First, one checks by inspectionFootnote 22 that the rule holds for \(n=2,\ldots , 5.\) Let us call \({Q}_{n}=({q}_{1} ;\ldots ;\,\,{q}_{n})\) the result of the reduction from the left, of a creeper with n quotients, putting 1 after the quotients. Assume \(\left({q}_{1 };\ldots ;\,{q}_{k}\right)\) is given by the indicated rule for any set of \(k\) quotients with \(k<n\). From the procedure for the reduction of the creeper, it follows that \(({q}_{1} ;\ldots ;\,{q}_{n})\) is obtained by reducing the three-term creeper \({q}_{1} ; \left({q}_{2 };\ldots ;\,{q}_{n}\right); ({q}_{3 };\ldots ;\,{q}_{n})\). Therefore,
Now, start from the product \({q}_{1}{q}_{2}\ldots {q}_{n}\), and delete terms of the form \({q}_{k}{q}_{k+1}\), in all possible ways, as many times as possible. In this process, the only way \({q}_{1}\) could be deleted is when the product \({q}_{1}{q}_{2}\) is. Now, either we delete \({q}_{1}{q}_{2}\) at some point of the process, or we never do. In the first case, none of the other deletions may involve \({q}_{1}\) or \({q}_{2}\) (if \({q}_{2}{q}_{3}\) is deleted, the product \({q}_{1}{q}_{2}\) is not present anymore, and therefore, cannot be removed at a later stage). All the other deletions are thus precisely those that would be performed by applying the rule to \({q}_{3}{q}_{4}\ldots {q}_{n}\). In this first case, we therefore recover all the terms in \(({q}_{3 };\,\,\ldots ;\,\,{q}_{n})\) since, by the induction hypothesis, the result is assumed to be valid for \(n-2\) quotients. In the second case, we never delete \({q}_{1}{q}_{2}\), so that the deleted pairs never involve \({q}_{1}\). They are therefore are precisely those that are deleted in the calculation of \({q}_{1}\left({q}_{2} ;\ldots ;\,{q}_{n}\right)\), using now the induction hypothesis for \(n-1\) quotients. Putting these two together, the result follows.
Finally, the recurrence relations (A.11–12) are obtained by applying the recurrence relation (*) to the list of quotients in the reverse order, namely \({q}_{n}{ ; \quad q}_{n-1} ;\,\, { q}_{n-2}; \quad {\ldots ;\,\, q}_{1}\), which yields
On can derive all the other results from this (Appendix A). Therefore, Putmana Somayājī’s discourse does not require the introduction of continued fractions, and indeed, his argument is more natural if we do not.
The existence of such a procedure to generate a hierarchy of approximations from more refined ones has implications for the articulation of calculation and measurement: the approximation of the “true rate of motion of the anomaly [of the Moon] by ratios of smaller numbers such as 9/248, 110/3031, 449/12372, 6845/188611, etc.” (p. xli) does not imply that observations were carried out over 248, 3031, let alone 188,611 days. A much smaller set of observations would suffice. This is consistent with Nilakantha Sastri’s words about Parameśvara who, “from his direct personal observation of the movements of the sun and the moon invented the system of driggaṇitaFootnote 23 in 1431, a correction of [Haridatta’s] Parahita system”.Footnote 24 It follows that the data for the revised system were obtained in a single generation, confirming the hypothesis suggested by Karaṇapaddhati.
To sum up, the publication of Karaṇapaddhati is a significant event for our understanding of the History and Mathematics and Astronomy. It not only closes the sequence opened by the accounts of the Karaṇapaddhati and related texts by Warren (1825),Footnote 25 Whish (1834)Footnote 26 or Hoisington (1848),Footnote 27 it opens a new phase of analysis that gives us the hope of a better understanding of the evolution of astronomy in India as driven by a keen sense of reality, an awareness of the interdependence of measurement,Footnote 28 theory, and the subject who deals with both. It seems that the conviction that “reality alone triumphs” was taken quite literally by our authors.
Change history
01 January 2022
Due to a font problem some mistakes occurred that have been corrected.
Notes
In Sanskrit or Tamil (Sanskrit: vākya, Tamil: vākkiyam). This volume considers only the Sanskrit ones. The other important class of ephemerides current in Tamil Nadu is the tirukkaṇita pañcāṅkam.
Throughout, page numbers in parentheses refer to the work under review. Here, the authors are quoting K.V. Sarma.
K.V. Sarma, K. Ramasubramanian, M.D. Srinivas and M.S. Sriram, Hindustan Book Agency & Springer, 2008.
K. Ramasubramanian and M. S. Sriram, Hindustan Book Agency & Springer, 2011. The dates for these works are taken from this volume (pp. xxxiii–xxxiv), and the work under review (pp. xxxiv–xxxvii). For a discussion, see the latter, as well as S. Madhavan (Sadratnamālā of Śaṅkara Varman, Kuppuswami Sastri Research Institute, Chennai, 2011), pp. xvii–xxi.
In particular, they have a strong background in Physics.
Presumably Covvaram and not Trichur (xxxii–xxxiii).
Chāndogya Upaniṣad, VIII, 1, VIII, 3.3; Taittīriya Upaniṣad, I, 6.1.
Recall that ko’pi = kaḥ + api, literally “someone”, but that kaḥ is also a name of Brahman. For the early history of the identification of Kaḥ, Prajāpati and Brahman, starting from the interpretations of Ṛgveda X.121, see J. Gonda, Prajāpati’s relations with Brahman, Bṛhaspati and Brahmā, North-Holland, Amsterdam, 1989, especially pp. 61–62 and note 14 on p. 52.
Short for khaṇḍāntyadhruva.
Aryabhaṭīya I.10. See also Brāhmasphuṭasiddḥānta II.2–9, and Mādhava’s improved list of 24 sines given by R.C. Gupta (Gaṇitānanda, edited by K. Ramasubramanian, Springer, 2018, p. 383).
S. Kichenassamy, “Brahmagupta’s apodictic discourse”, Gaṇita Bhāratī, 41:1 (2019) 93–113.
This is not anachronistic so long as one does not assume that Putumana Somayājī used these methods – which the authors do not claim.
Venketeswara Pai R. and M.S. Sriram, “Madhyāhnakālalagna in Karaṇapaddhati of Putumana Somayājī”, Gaṇita Bhāratī, 33:1, (2017) 55–74.
C. Brezinski, History of continued fractions and Padé approximants, Springer, Berlin, 1991. Apparently, a symbolism for continued fractions first appears in a work by Cataldi published in 1613 (see p. 65).
“Rationale of the Chakravala Process of Jayadeva and Bhaskara II”, Historia Mathematica 2 (1975) 167–184. It is conceivable that the theory of continued fractions was eventually an outgrowth of the kuṭṭākāra, but that is a different issue. It is futile to speculate about transmission before the conceptual background of major texts has been ascertained by internal analysis. And so far, this task has only be accomplished for a few propositions.
It could be an outgrowth of the calculus on cords with variable unit, or heterometry, that we have shown to be necessary in order to account for Baudhāyana’s results (S. Kichenassamy, “Baudhāyana’s rule for the quadrature of the circle”, Historia Mathematica, 33:2 (2006), 149–183, http://doi.org/10.1016/j.hm.2005.05.001). Indeed, relations between two ideal cords (without width) already come up in a symmetric fashion: typically, one cord is divided into b parts, of which a make up the other; therefore, it is immediate that the second may be divided into a parts, of which b make up the first.
S. Kichenassamy (2019), op. cit. n. 12. Brahmagupta’s remarkable argument does not seem to have any modern equivalent.
This is a general feature of Indian mathematical exposition, but is also common elsewhere and in fact, seems quite common among innovative works (S. Kichenassamy, “Translating Sanskrit Mathematics”, Aestimatio, N.S. 1, (2020), 183–204 https://ircps.org/aestimatio/aestimatio-ns-volumes/ns-1/183-204/).
The standard phrase is adho’dho sthāpyam. The name vallī does not seem to occur in the earliest treatments, but is is not needed, since the construction of the creeper and its reduction are fully described by this and other phrases.
It would take too long to explain the various ways in which the list of quotients has been used in the Indian literature.
One finds, for \(n\,=\,2, 3, 4, \mathrm{and }5\), \({{Q}_{2}=q}_{1}{ q}_{2}+1\), \({{Q}_{3}=q}_{1}{q}_{2}{q}_{3}+{q}_{1}+{q}_{3}\), \({{Q}_{4}=q}_{1}{q}_{2}{q}_{3}{q}_{4}+{q}_{1}{q}_{2}+{{q}_{1}q}_{4}+{ q}_{3}{q}_{4}+1\) and \({{Q}_{5}= q}_{1}{q}_{2}{q}_{3}{q}_{4}{q}_{5}+{q}_{3}{q}_{4}{q}_{5}+{ q}_{1}{q}_{4}{q}_{5}+{q}_{1}{q}_{2}{q}_{5}+{ q}_{1}{q}_{2}{q}_{3}+{q}_{1} {+ q}_{3}+{q}_{5}\). Although we do not develop this point here for expediency, it is very likely that the result was suggested by the inspection of a number of special cases such as these.
We omit this straightforward, but lengthy verification.
The term dṛggaṇitaikya is already found in Brāhmasphuṭasiddhānta 11.61.
K.A. Nilakantha Sastri, A History of South India, fourth ed., Oxford Univ. Press, 1976, p. 363. He also stresses the existence of other poles of excellence in Kerala: “[t]here were families in Kerala which specialized for generations in particular subjects, like the Thaikkāṭṭu illam in architecture. […] In Āyurveda (medicine) the eight great families […] are well-known” (op. cit., p. 362), and mentions grammarians on pages 363–4. There are stray references to architecture in Yuktibhāṣā.
John Warren (1825), Kala Sankalita: A Collection of Memoirs on the Various Modes according to which the Nations of the Southern Parts of India divide Time: to which are added Three General Tables, wherein may be found by mere inspection the beginning, character, and roots of the Tamul, Tellinga, and Mohammedan Civil Years, concurring, viz. the two former with the European Years of the XVIIth, XVIIlth and XIXth Centuries, and the latter with those from A. D. 622 (A. H. 1) to 1900, Madras, dated Feb. 18, 1825. We omit the details of earlier accounts, from the late eighteenth century.
Charles M. Whish (1834), “On the Hindú Quadrature of the Circle, and the infinite Series of the proportion of the circumference to the diameter exhibited in the four S’ástras, The Tantra Sangraham, Yucti Bhásá, Carana Padhati and Sadratnamála”, Transactions of the Royal Asiatic Society of Great Britain and Ireland, 3(3), 509–523 (read 15th of December, 1832). The passages from Karaṇapaddhati that he quotes (6.5, 6.7) or paraphrases (6.4) are given and discussed in the volume under review on pages 150–154.
H.R. Hoisington,
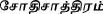 : The Oriental Astronomer, being a Complete System of Hindu Astronomy, accompanied with a translation and numerous notes, with an Appendix. American Mission Press, Jaffna, 1848, in two volumes. It seems to be closely related to the earliest known fully developed form of the system, the Vākyakaraṇa, probably composed in Tamil Nadu between 1282 and 1316. I gather the first and third author plan to edit this work. We mention a different set of vākyas, also in Tamil, in
: The Oriental Astronomer, being a Complete System of Hindu Astronomy, accompanied with a translation and numerous notes, with an Appendix. American Mission Press, Jaffna, 1848, in two volumes. It seems to be closely related to the earliest known fully developed form of the system, the Vākyakaraṇa, probably composed in Tamil Nadu between 1282 and 1316. I gather the first and third author plan to edit this work. We mention a different set of vākyas, also in Tamil, in 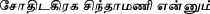
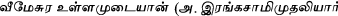 and sons, Chennai, 1939), pages 261–264.
and sons, Chennai, 1939), pages 261–264.For recent progress on measurement, see for instance R. Venketeswara Pai and B.S. Shylaja, “Measurement of coordinates of Nakṣatras in Indian astronomy”, Current Science, 111(9) (10 Nov. 2016), 1551–1558.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Kichenassamy, S. Karaṇapaddhati of Putumana Somayājī by Venketeswara Pai, K. Ramasubramanian, M.S. Sriram and M.D. Srinivas, [Hindustan Book Agency, New Delhi and Springer Nature, Singapore, 2018, xlviii + 450 pp., ISBN 978–981-10-6813-3; DOI: 10.1007/978-981-10-6814-0]. Indian J Hist. Sci. 56, 149–153 (2021). https://doi.org/10.1007/s43539-021-00015-z
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s43539-021-00015-z


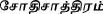 : The Oriental Astronomer, being a Complete System of Hindu Astronomy, accompanied with a translation and numerous notes, with an Appendix. American Mission Press, Jaffna, 1848, in two volumes. It seems to be closely related to the earliest known fully developed form of the system, the Vākyakaraṇa, probably composed in Tamil Nadu between 1282 and 1316. I gather the first and third author plan to edit this work. We mention a different set of vākyas, also in Tamil, in
: The Oriental Astronomer, being a Complete System of Hindu Astronomy, accompanied with a translation and numerous notes, with an Appendix. American Mission Press, Jaffna, 1848, in two volumes. It seems to be closely related to the earliest known fully developed form of the system, the Vākyakaraṇa, probably composed in Tamil Nadu between 1282 and 1316. I gather the first and third author plan to edit this work. We mention a different set of vākyas, also in Tamil, in 
 and sons, Chennai, 1939), pages 261–264.
and sons, Chennai, 1939), pages 261–264.