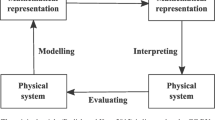
It is well known that the science of physics is a highly math-intensive discipline; that is to say, it makes extensive use of mathematics in the formulation of scientific laws and the investigation of their consequences. The laws offer mathematical models of physical systems—typically, in the form of differential equations, describing in a general way how specific physical quantities vary in space and time. By imposing constraints, in the form of initial or boundary conditions, one may then solve the equations to obtain descriptions of specific physical systems.

Such mathematical models provide a very powerful tool for solving problems in physics: in particular, they can be used to predict the evolution in time of a physical system, or retrodict its behaviour in the past. However, they can also give rise to conceptual problems, in the physical interpretation of mathematical objects and relations. As we shall see, many of these problems bear on the fundamental epistemic dichotomy of realism versus relativism. We will examine a few such issues below.

The central issues to be addressed here are: What is the relation between the mathematical objects and techniques and the physical objects they describe? What role is played by the mathematical concepts, in our understanding of concrete physical systems and their behaviour? And how well does the mathematical account “match” or “cover” the physical entities and processes it purports to represent?

The following questions will also be touched on: Are there true theories of physics—i.e. ultimately correct ways to describe the physical world; and if so, can we discover these theories? More specifically, does the world of physical phenomena possess an objective (i.e. observer-independent) ordering—an intrinsic structure? And, if so, can this structure be revealed to us through scientific investigation?

I will argue here that the answer to the questions in these last two points is no! But first, we need to clarify our basic terms.

1 Physics and Mathematics

The term physics is defined in the Oxford English Dictionary as “the science dealing with the properties and interactions of matter and energy”. Clearly, this definition covers a wide field: indeed, some have argued that it may be taken, in essence, to contain all the natural sciences! This is one vision of scientific reductionism, a controversial issue which will not be addressed here.

In the present context, the scope of physics is taken to be more narrow. It is identified as a set of more or less well-defined sub-disciplines: physical theories, all formulated in mathematical terms. A non-exhaustive list will include:

  • classical mechanics and electromagnetism,

  • special and general relativity theory,

  • thermodynamics and statistical mechanics,

  • hydrodynamics and solid-state physics,

  • quantum mechanics and electrodynamics,

  • high-energy particle physics,

  • …

In each such theory is then implicit a description of a certain domain of physical objects, characterising the kind of system that the theory is designed to model: macroscopic matter, molecules and atoms, elementary particles, electric charges, and fields of various kinds. The theory will then feature a number of relationships, generally referred to as physical laws, which govern the behaviour of the objects within the domain. We note that these theories all deal, in one way or another, with motions of material bodies, and forces acting on such bodies—this is, in fact, the “definition” of physics that is commonly offered in text books at the secondary school educational level. It serves to distinguish physics from the other “school sciences”, such as chemistry and biology.

How, then, does the discipline of mathematics impact on physics, as defined here? To answer this, it will be instructive to take a brief overview of the historical development of this topic.

The origin of mathematical thinking would seem, as far as can be known today, to have its roots in concrete practical needs. As human civilisation developed in early times, it became necessary to handle measurement and quantitative treatment of various items. For instance, we may consider the acts of counting and subdivision of various items—for instance, in the weighing of goods for trade—leading on to the conception of integers and fractions, which form the basis of arithmetic, the art of using numbers and computation. Other such deliberations addressed the topic of geometry—dealing with such notions as position, angle, length, area and volume—which is clearly also of considerable commercial interest, e.g. for the buying and selling of plots of land.

Gradually a set of concepts and computational procedures emerged and developed. It should be noted that this “proto-mathematics” was in its start a very practical enterprise, serving the needs of trade, production of agricultural and manufactured items, and administration of taxes, military organisation, etc.

As experience with the concepts of mathematics accumulated, many rules and regularities emerged. It was, for instance, noticed early that the ratio of the circumference of a circle to its diameter seems always to have the same value: a number slightly larger than 3, conventionally denoted today by the symbol π. Also the interior angle sum of any triangle seems always to be the same: a value conventionally set to 180°. And the contemplation of such rules and regularities then led to a daring and remarkable leap of the imagination: the idea that it should be possible to summarise them in a logical system, where all valid mathematical results are logically deducible from a small set of fundamental propositions, so-called axioms.

The origin of this new “axiomatic” way of thinking about mathematics is usually ascribed to classical Greece, though similar ideas did appear also in other societies in early history. However, it is the Greek tradition that has been the main inspiration for the development of science and mathematics from ancient times up to the present day. So, let us take a look at how they thought about these matters.

2 The Axiomatic Approach

First, the basic concepts were sharpened and idealised. Thus, for instance, geometry was regarded as a set of relations between different types of abstract geometrical objects: a point had no extension, serving only as a marker of position; a straight line had no thickness, serving only to mark the shortest way between any two points on it; etc. Similarly, numbers were thought of as quantities that had exact values. These objects and quantities were assumed to actually exist, i.e. to inhabit an abstract world of pure ideas which constituted the basic reality of existence; and the task of mathematics, according to this new way of thinking, was to investigate the properties of such idealised entities. This notion of an ideal world, existing somewhere “above” or “beyond” our physical world, is usually associated with the Greek philosopher Plato, and often called the platonic reality.

The physical world that we can observe around us was assumed to be only an imperfect representation of the platonic ideal reality. Thus any point that we might try to draw or mark in a concrete situation must necessarily possess some extension; any straight line that we can draw necessarily has some thickness and can never be “perfectly straight”; and an actual concrete measurement (say, of the distance between two points) cannot be performed with perfect accuracy. Nevertheless, these more or less “fuzzy” and inexact physical objects and quantities were assumed to reflect in some way the corresponding entities of the abstract ideal world; and moreover, relations between entities in the abstract world were assumed to govern relations between the corresponding physical entities. In other words, logical investigation of the abstract ideal world—i.e. mathematics—will enable us to draw valid conclusions about the physical world we inhabit!

One may well pause and focus on this last statement: it describes a truly remarkable conceptual leap in the way we, as human beings, can deal with the world of our experience. In fact, this approach has been instrumental in the development of modern science and technology. However, as we shall see, it has also served to constrain our thinking about the nature of knowledge in science—i.e. about scientific epistemology.

The task of the mathematicians was then thought of as being: (1) to establish a certain set of fundamental relations (axioms) connecting the entities of the ideal world; and (2) to deduce other relations (theorems) that follow logically from these axioms. The number of axioms in the set is generally small; and the beauty of mathematical theory is then that so many interesting theorems can be deduced from such a slender axiomatic base.

The following example may serve to indicate how this approach works.

3 Arithmetic

The conception of numbers arose out of very practical considerations: counting and measurement of concrete physical quantities, as already noted. Gradually, however, a theory of these entities (an arithmetic) developed, where properties of numbers and relations between them were to be derived from certain fundamental propositions (axioms). The definition of the basic entities then went through a well-known process of extension: Starting with integers (whole numbers), one extended the set of axioms to define rational numbers (fractions)—which include integers as a special case (fractions with unit denominator). From there, the axiomatic basis was further extended to define real numbers (comprising rational and irrational numbers), complex numbers (comprising real and imaginary numbers), and so forth.

Note, however, that this progressive extension of the concept of a number rapidly loses direct contact with its concrete physical origin. Let us consider the operation of a concrete measurement, of some quantity associated with a given physical object. This is, in principle, done by comparing the object with some conventionally chosen unit; and the result of the comparison is then given by a rational number: a fraction a/b, where a and b are non-zero integers. Thus, for instance, the statement that a given length l is 5/8 m long is equivalent to saying: if a meter stick is divided into eight equal segments, and the length l is laid out along the stick, it will cover five of these segments. Similar considerations are easily seen to apply to other measuring procedures, such as pointer positions on a dial, digital displays, etc. So, the upshot of all this is: physical measurements yield rational values. Moreover, measurements can in principle be performed with any desired accuracy, by suitably increasing the numerator and denominator of the fraction: thus, for instance, the statement l = 3557/10000 m means that the length l is given as 35.57 ± 0.005 cm. This relates to the fact that the set of rational numbers is infinite and dense, in the sense that for any two different rational numbers x and y, it is always possible to find a rational number z lying between x and y.

Early on, it was realised that there are numbers which cannot be expressed as fractions: one example is √2 = 1.4142… Such non-fractional quantities, whose values are generally defined by converging infinite sequences, were given the somewhat unfortunate appellation irrational numbers. The set of irrationals is, like the set of rationals, infinite and dense; and moreover, the two sets are densely interspersed, in the sense that it is possible to find rational numbers arbitrarily close to any given irrational number, and vice versa. Together, they form the set of real numbers.

As is well known, it is the real numbers that form the basis of mathematical analysis, as applied to science. But note that it is only the rational numbers that are conceptually connected with physical measurements: one never actually measures—in the concrete sense of comparing object and unit, as indicated above—an irrational value for a given physical quantity! Thus, we may pose the question: why does the formal mathematical manipulation of scientific quantities require that one uses the complete set of real numbers? Would it not be more logical, and economical, to stay with the rational numbers—which, after all, are the ones directly associated with the act of physical measurement—and base the mathematical analysis of scientific laws on them?

The answer is that a theory of mathematical analysis that admits only rational numbers, though possible in principle, would be quite intractable in practice! The reason is, loosely speaking, that the set of rational numbers alone is “not dense enough”. In the analysis one deals with algebraic equations of a very general kind; and it would be extremely cumbersome to “filter out” all equations that have irrational solutions, leaving only equations with rational solutions for the legitimate description of scientific relationships. As a very simple example, one would then have to allow the equation x 2 – 4 = 0, which has the rational solutions x = ±2, but disallow the equation x 2 – 3 = 0, with the irrational solutions x = ±√3. In fact, since rational and irrational numbers are densely interspersed as already noted, this would play havoc with the whole notion of continuity, which is fundamental in the treatment of mathematical functions.

To avoid such formal complications, we allow both rational and irrational values for physical quantities, as solutions emerging from the mathematical treatment of the scientific laws that govern these quantities. In other words, we accept the physical validity of propositions such as ‘the length l of this object is √2 m’, and take this to have the following operational meaning: when the length l is actually measured, the result (in meters) will be a rational number which, with an increasing accuracy of measurement, can be assumed to approach the “exact value” 1.4142… of the irrational number √2, as determined by mathematical theory. But note that this is purely conventional: there are rational numbers arbitrarily close to √2; and we can never actually get to the exact value of this number, as defined by a limiting process, to single it out as the true value of the length l.

Thus, as we can see, the definition of a number has been extended from its physical origin (as a result of counting and measurement), to allow for a more convenient mathematical treatment of scientific relationships. And note that this process of extension does not stop here! Many algebraic equations have solutions that are not real numbers—one simple example is x 2 + 1 = 0. The desire to include such equations then leads to a further extension of the axiomatic basis, to define the so-called complex numbers, which admit values involving square roots of negative numbers; such values have, as is well known, been given the rather unfortunate name imaginary numbers. And again, this extension has also proved to be very useful in the mathematical treatment of physical systems.

Summing up so far: The topic of arithmetic, originally devised and thought of as a more or less direct and concrete description of measured quantities, has evolved and migrated into the idealised realm of mathematics. And this realm is populated by abstract objects—irrational numbers, complex numbers, etc.—which do not match well with anything observable or measurable in the physical world. One might say that there is an ontological mismatch between the mathematical and physical entities. And yet, these abstract arithmetical concepts and techniques have turned out to be extremely useful. Thus, for instance, we accept without questioning the proposition that the circumference of any circle is π times the diameter, without ever having observed or measured the value of the number π. In fact, most scientists would regard these concepts and techniques as indispensable, in our description and manipulation of the observable physical world!

We note that this has been a lively issue in the philosophical discourse. To cite a few examples: The Nobel laureate physicist Eugene Wigner published, some 50 years ago, a paper named ‘On the unreasonable effectiveness of mathematics in the natural sciences’ (Wigner 1960), discussing what he found to be a most remarkable fact: that the purely theoretical constructs (i.e. concepts and techniques) of mathematics can give such a deep and successful description and understanding of physical phenomena! And more recently, the British mathematical physicist Roger Penrose has declared himself to be a “mathematical platonist”: a believer in the objective reality of mathematics, as the necessary basis for understanding the true nature of the Universe. In his book The Road to Reality (2004), Penrose addresses several of the issues raised here—such as the effectiveness of mathematics in physics, and the role of mathematical idealisations in physics—from a perspective of epistemic realism. Pincock (2010) discusses the applicability of mathematics in science on a more general level; while da Costa and French (2003) argue that successful mathematical models of physical systems should be regarded as partially (rather than wholly) true descriptions of Nature.

To conclude: there is a poor match between many of the mathematical entities which appear in theoretical physics and the physical objects that these entities are assumed to represent. In a sense, the mathematics is too rich: it contains objects that do not have a physical counterpart, but nevertheless are absolutely essential in the mathematical treatment of physical systems. Usually, this causes no great problem in practice: We just translate back and forth, between the sharp, exact, idealised mathematical concepts and the corresponding fuzzy, inexact physical objects that are being studied. In this way we can use the mathematical model to analyse the evolution of concrete physical systems.

It is manifest that this approach (i.e. representing physics in mathematical terms) has been very successful in actual scientific practice, and has contributed substantially to the development of physics. It has, in fact, led to great advances in our knowledge and understanding of how the natural world operates. But as we shall see, some other awkward features also appear

4 Unphysical Solutions

One consequence of this great success is that it has led many physicists to adopt as a guideline the following tacit assumption, or hypothesis: In a good physical theory, the mathematics will contain the physics! In other words: the mathematical description of a physical system is “rich enough”, so to speak, to deliver all possible concrete manifestations of the system, even those that we might not at first hand expect, or even think of! Indeed, a lot of research in theoretical physics will employ this as a general strategy: take the mathematical laws (typically, differential equations) that describe a certain class of phenomena, impose some constraints (typically, initial and/or boundary conditions), in order to focus on a concrete physical system, and then explore all the possible solutions that the analysis may yield.

And the usual (tacit or explicit) assumption is then that, however strange and unexpected such a solution may seem, it will always identify something that exists—i.e. it will describe a physically realisable situation. That is to say, valid mathematical reasoning will always lead to valid physics. Concrete examples of this are abundant in the history of physics. Thus, for instance, we may consider the positron. It originally emerged as a positively charged, and unexpected, solution of the Dirac wave equation for the electron, and was then subsequently observed to exist as an elementary particle (the anti-electron, or positron) in Nature.

Indeed, this predictive ability of the formal deductions is often highlighted in the teaching of physics, particularly at the secondary school level, as demonstrating the “power of math” in science. The fundamental premise is then that “Nature is mathematical”—that is to say, it runs according to mathematical laws. Thus, once we have found the “right” mathematical description of the world, this will give us a complete insight into its physical manifestations.

But this viewpoint is not unproblematic. It is a fact that sometimes the results of the formal analysis—i.e. particular solutions of the equations that define the theory—can be rather weird; and one may then well wonder whether such solutions does in fact describe real physical situations. It is perhaps somewhat reassuring to note that such “weird solutions” generally do not usually appear in the physics that is usually taught at primary and secondary school level.

As an example, we consider the theory of classical electromagnetism, as described by the Maxwell-Lorentz (M-L) equations. As is well known, this theory has been remarkably successful in describing a wide variety of electric, magnetic and optical phenomena; and moreover, it provides a solid basis for further developments in physical theory, such as the theories of special and general relativity, and the theories of quantum mechanics and quantum electrodynamics. Indeed, the M-L equations may be said to constitute the fundament of much of modern physics.

However, as it turns out, certain mathematical results of this theory are rather odd and difficult to understand on physical grounds. Here let us just consider one example: The M-L equations are symmetric with respect to time inversion. As a consequence of this, the theory allows for two kinds of electromagnetic wave solutions, known as retarded and advanced waves. In simple terms, this means that when an electromagnetic emitter generates waves at a point P in space, at a given time t P , some of these (called retarded waves) will arrive at another point Q at a time later than t P , while others (advanced waves) will arrive at Q at a time earlier than t P . Loosely speaking, one might say that retarded waves propagate into the future, arriving at other points in space after the time of emission, while advanced waves propagate into the past, arriving at other points before the time of emission!

I will not demonstrate the math here—for those who are interested, comprehensive and quite readable accounts of this topic are given in several well-known textbooks on electrodynamics, for instance Rohrlich (2007).

Now, this scenario appears to be quite contrary to our physical intuition about what is possible in Nature—certainly, no concrete instances of such queer behaviour have ever been observed in the laboratory! In fact, it seems that the advanced waves are regarded by most physicists as being unphysical, in the sense of not describing any physically realisable situation.

So, what happens then to the fundamental assumption that was referred to above, asserting that valid mathematics leads to valid physics? As it turns out, many physicists have felt this whole situation to be unsatisfactory, and have searched for ways to get rid of offending solutions such as the advanced waves. And indeed, this goal can be achieved in a variety of ways, by introducing additional mathematical features into the theory. This has in fact been an active field of research for many years, resulting in a large amount of publications to be found in the literature, as one may readily verify by googling search strings such as ‘advanced waves’. For instance, one may lay down restrictions on the allowed initial or boundary conditions, or even impose auxiliary constraints on the field equations themselves. Strategies such as these will serve to reduce the space of permissible solutions of the equations; and this reduction can in fact be implemented in such a way as to exclude the unwanted solutions.

However, it may be argued that this is not really a physically satisfactory resolution of the problem, unless one can justify these additional formal additions/restrictions by relating them to other parts of the theory—say, by demonstrating that they have observable physical implications. After all, if the only effect of these additional items is to mathematically disallow specific unwanted solutions, one may regard them as physically inconsequential and epistemically ad hoc—just a more complicated way of saying that “we do not want to consider such solutions”. Here opinions differ, and it seems fair to say that no accepted consensus has been reached among physicists, as to whether such “unphysical” solutions can be eliminated from the theory of electromagnetism in an epistemically satisfactory way.

Another possible strategy is to accept the reality of the strange solutions, but then try to devise some physically plausible account for why they are not observed. One classical example of this is the well-known absorber theory proposed by Wheeler and Feynman (1949). Here it is assumed that both advanced and retarded waves indeed do exist in Nature, and that they together form a complex network of electromagnetic signals travelling both forwards and backwards in time. However, if certain cosmological conditions are satisfied—so-called perfect absorption, where every emitted wave is eventually absorbed—it can be shown that the advanced waves to and from any point P will cancel out by destructive interference, leaving only the retarded waves to be observed at P! And these retarded waves, of course, behave as one would expect from standard electromagnetic theory. In other words: the absorber theory offers the same predictions, for locally observed electromagnetic phenomena, as does the standard M-L theory!

Are the advanced waves then to be considered as unphysical? As noted, in the absorber theory they make no difference to the course of local physical phenomena, and are hence not observable. In particular, since the advanced waves cancel out in the observable present, it is not possible to receive any “signals from the future”! For this reason, most physicists would ignore them as being harmless—a physically inconsequential oddity inherent in the mathematical theory. Of course, the very notion of propagation backwards in time, into the past, will seem a little disquieting to some. But note that it is electromagnetic waves that are doing this, not material bodies—so, there is no question of time travel involved here! However, as we shall see, this question does become a serious issue in the next example to be considered.

We now take a brief look at Einstein’s theory of general relativity—another theory that enjoys great prestige and authority as a fundament of modern physics. Recall here that the Einstein field equations describe gravitation as a manifestation of the geometry of space and time—in other words, the geometrical properties of any universe will determine its gravitational field. Thus a solution of these equations will describe a particular kind of universe, with a particular geometrical structure. And, as it turns out, some of these “solution universes” can be rather bizarre.

One example is the celebrated Gödel universe, which was proposed in 1949 by the Austrian logician Kurt Gödel as a solution of Einstein’s field equations of gravitation. For those who are interested in the mathematical details, the source reference is Gödel (1949).

The Gödel universe features a strange phenomenon, generally referred to as closed timelike worldlines. Loosely speaking, this means that the passage of time can, for some observers, be cyclic, implying that it is in principle possible for such an observer to revisit his own past in time and space!

This is illustrated in the figure. Imagine an observer, who moves along a timelike worldline in the future direction indicated by the arrows, and who measures all moments of time on his own local clock. He starts at a point P in space and time, and here observes the time on his clock to be t1. Moving on through space and time, he arrives after a while at another point on his worldline, and here observes a later time t2 > t1. After this, he arrives at yet another point on his worldline, and observes a later time t3 > t2… and so it goes on. But eventually he will arrive back at P, at a still later time t4 > t3, as observed on his local clock. But remember that P is the observer’s original point of departure, in both space and time! So, he will meet here at this point his younger self, about to start the same journey at the earlier time t1, as measured on his (i.e. the younger one’s) local clock!

Of course it may be, and indeed repeatedly has been, argued on philosophical grounds that this solution is grossly unphysical, since it opens up for all sorts of logical contradictions and absurdities. In particular, it suggests the possibility of time travel—a favourite topic of science fiction—with all the paradoxes that this notion implies. For example: Imagine that the revisiting observer decides to kill his younger self, when he meets him in this encounter—how could he (the older self) then continue to exist after that, and thus be able to revisit in the first place? In fact, Gödel’s solution is considered by many (though not all) physicists to be an anomaly, which does not describe any realisable physical situation. And then, of course, the same problem arises that was referred to above, for the case of electromagnetism: How can we get rid of this unwanted solution, in a physically meaningful way?

As it turns out, Gödel’s solution is not an isolated mathematical oddity—there are many other solutions of Einstein’s field equations which also allow closed timelike worldlines. Indeed, the study of such solutions has over the years been the subject of a large amount of theoretical research, which has produced a considerable number of publications—as may be readily verified by googling the search string ‘Gödel’ + ‘timelike’. These publications vary in their approach to the problem. Broadly speaking, some try to propose plausible physical arguments supporting the view that such worldlines cannot be physically realised, or at least that they would involve very extreme and intolerable physical conditions for the prospective time traveller; while others explore the possible consequences for physical theory of allowing this strange kind of worldlines to exist. A comprehensive (though rather technical) survey of much of this work is given by Thorne (1993), who discusses in this context the conjecture of chronology protection, as proposed by Hawking (1992). This conjecture states that the laws of physics must somehow provide for mechanisms that prevent time travel into the past—and hence also closed timelike worldlines—from ever coming into being in the real universe.

Thorne concludes that there appears to be no compelling physical reasons to forbid such entities, though they do lead to some pretty odd situations. And this conclusion seems to hold up even today—at least, I am not aware of any later work that would seriously challenge it. In other words: it seems fair to say that the elusive goal of chronology protection has not been reached—though Thorne speculates that this may in fact be possible in the future, in the context of a working theory of quantum gravitation. (It may be noted that such a theory has still not been satisfactorily established in physics.) His final remark is worth quoting:

In summary, these studies are giving us glimpses of how […closed timelike worldlines…] influence physics; but whether these glimpses are teaching us something deep and important, or we are just playing fun mental games, is far from clear. (Thorne 1993, p. 21)

5 Conclusion

5.1 The Epistemic Dichotomy

Summing up the situation: There is a fundamental assumption—widely adopted by practising physicists, as well as by physics teachers and students—that valid mathematical reasoning will generally lead to valid physics. To be sure, a few anomalies will sometimes appear; but they tend to be “swept under the carpet”, or even ignored altogether.

It should be noted that this assumption is ontological: It is based on the premise that the physical world is made up such as to be discoverable by mathematical deduction, and that such deduction will then yield physically valid results. In fact, we may recognise it as a modern version of the classical position known as platonism: There exists a unique ideal world, governed by abstract logical and mathematical laws; and the observable physical world, with all the laws that govern it, is nothing but an imperfect reflection of this ideal world.

Clearly this platonic ontology fits in well with the position of epistemic realism: the view that the true nature of the world is “lying out there” in some sense, waiting for us to find it. In other words: there exist objectively true propositions about the physical world and how it operates, and it is (in principle, at least) possible for us to discover these truths. Or, to put it another way: the world possesses an intrinsic structure, with an objective existence which is independent of how we choose to describe it; and the task of physics and other natural sciences is then to identify and explicate the properties of this structure. It is in this perspective one must assess the present efforts to establish a Final Theory of physics—aka the Theory Of Everything (TOE), see e.g. Weinberg (1993).

This may be compared with the position of epistemic relativism, which does not imply this strong ontological connection between mathematics and physics. This is the perspective adopted by the theory of radical constructivism, as has been extensively discussed elsewhere (Quale 2008). In the present context, the radical-constructivist epistemic position can be briefly outlined as follows.

A physical theory is regarded as a mathematical model of some domain of physical phenomena, formulated in mathematical terms. And it is important to note here that the phenomena in themselves are not assumed to exhibit any pre-existing structure, which is “lying out there” waiting to be discovered. Instead, the physical world is taken to be intrinsically amorphous, without any objectively manifest structure of its own! The model is then constructed by the physicist, and imposed on the phenomena, for the purpose of gaining an understanding of them. Specifically, it is devised in such a way as to clarify, and hopefully answer, whatever questions the physicist may want to ask about these phenomena. In the terminology of radical constructivism, such a model is said to be viable if: (1) it yields predictions that agree with observation, and (2) it fits in with the ontological preferences of its practitioners (the physicists).

Here a possible objection may be noted: How can an amorphous world (of experienced phenomena) be described in a meaningful way by an ordered scheme (a physical theory)—must there not, logically speaking, be some pre-existing order in this world in the first place, for the theory to “capture” in its description? The answer is no: there is nothing that logically forbids one from imposing an ordered structure, chosen by personal preference, upon material that is initially unstructured. One simple example: Consider the set of all possible musical notes and musical instruments. There is no initial structure to be found in this set. However, a composer may impose a particular organisation on it, and thus produce a piece of music—say, a symphony. Here there is indeed a structure, an ordering, as given by the musical score and orchestral arrangement. But note that this order is constructed by the composer; it is not something that was lying there—inherent in the notes and instruments, so to speak—waiting for the composer to discover it!

It is in the nature of such mathematical models that they do not (and indeed cannot) deliver a faithful representation of the physical phenomena they are designed to represent. There will always be some aspects of the phenomena that are not matched in the model. In other words, our experiential world will always be more complex than any model we can construct to describe it! In other words: The phenomena will invariably turn out, under certain circumstances, to behave in ways that are not well matched by the model. A well-known example is the failure of Newtonian mechanics to account satisfactorily for bodies that are moving in very strong gravitational fields—in this case, as is well known, one gets better results with Einstein’s theory of general relativity.

And conversely, as shown in the present discussion, there will be some features of the model that do not match the phenomena it is designed to model. In other words: the mathematical reasoning will sometimes yield features that do not fit in well with the physical phenomena that are being studied—as witness the advanced waves, and the Gödel universe, that have been discussed here.

In a simplified formulation, one might describe the situation as follows: on the one hand, the mathematical analysis will sometimes lead to physical systems that are unrealistic; and on the other hand, the analysis will sometimes “miss out” on physical systems that are realistic!

Is this a problem for theoretical physics? If one decides to adopt the epistemic perspective of realism, the answer would seem to be yes: Within the framework of this epistemology, one is searching for the objectively true mathematical description of the physical world; and it cannot then be satisfactory to observe the mismatch that emerges, between the mathematical model and the physical entities that are modelled. Of course, one can always argue that this is because we have not yet found the right mathematical framework, the one that does describe the physical world exhaustively and correctly. On the other hand, when viewed from the epistemic position of relativism, as adopted in radical constructivism, this problem does not arise! Here it is recognised that we establish our knowledge and understanding of the physical world by constructing mathematical models of it. However, these models are not then assumed to be perfect, in the sense of giving a complete and exhaustive representation of the world. Thus, a mathematical model of some physical system may indeed be very successful in its account of how the system behaves. But there will always be some physical aspects of this system that the model does not, or cannot easily, capture: either because the analysis throws up unphysical solutions (as discussed here), or because it fails to describe some physically realistic situations. However, this causes no problem in the philosophy of physics—and it certainly does not stop physicists from utilising these models! On the contrary, the physicists will employ the mathematical model as a tool, to be put to work whenever it is useful—that is to say, whenever it yields results that are viable: i.e. agree with observation, and give satisfactory answers to the questions that are asked. And conversely, if and when the mathematical analysis throws up some feature that does not match well with the physical problem that is being studied, this will cause no worry—it will just be a case of the tool (i.e. the model) not being well designed to handle this particular aspect of the problem! To remedy this situation, the physicist may then either try to redesign the tool (refine the mathematical model) or else switch to another tool (use some other theory).

This has implications for the teaching of physics. Students (at any level of education) will often ask questions such as: How can we know that this theory really does describe correctly the physical phenomena that are being studied? Can we trust the mathematics to keep us on the right track? Evidently, this kind of question presupposes a realist ontology, in which there by assumption exists a “right track”—i.e. a correct, complete and exhaustive mathematical description of the physical world, which is there for the physicists to discover. At lower school levels, up to and including secondary school, such an ontology is easily imparted to the students, since (as indicated above) they will in general only be exposed to concrete instances where valid mathematics actually will lead to valid physics. But at a higher (university or college) level, discrepancies may well emerge: some theoretical predictions will not match with the observed phenomena (i.e. the math does not adequately describe the physical situation); and/or unphysical solutions will emerge (such as e.g. advanced waves in electrodynamics, and closed timelike worldlines in general relativity).

As we have seen, this ontological conflict has stimulated a number of attempts to “save realism”, as it were, by devising ways to formally exclude the unwanted predictions. It seems fair to say that so far nothing conclusive or convincing has resulted from these efforts. So, I would suggest that one adopt an alternative strategy: Let the students be exposed, from the start of their education in physics, to the relativist ontology that is implicit in by radical constructivism. Here, the mathematical formulation of physical theory is just a model, devised to analyse and describe certain physical phenomena. And, as we all know, this strategy can work remarkably well, for many features of the phenomena that are being studied. However, it offers no promise that such a model will, or can, deliver “the true description” of the physical world.

This situation may be depicted graphically. In the following illustrations, P denotes the set of all physical systems {p}, and M denotes the set of mathematical models {m} that can be devised to describe these systems.

Now, consider the following combinations of M and P: This is the ideal situation envisaged by a realist epistemology a faithful and correct match between M and P. That is to say: For any physical system p ⊂ P, there exists a uniquely given mathematical model m which gives a complete and correct description mp of this system; and there are no models m ⊂ M that describe unphysical systems—i.e. systems that cannot be realised in Nature. This depicts the relativist epistemology of radical constructivism: Here M is a set of mathematical models {m}, constructed in such a way as to give a viable description of as many as possible of the physical systems P = {p}; these viable models are depicted by mp in the graph. However, there will then be some physical systems p ⊂ P that are not covered by the model—our experiential world will always be more complex than any theoretical model we can construct to portray it. Also, there will in general be some models m ⊂ M, resulting from the mathematical analysis of particular physical systems, that do not describe realisable physics—i.e. unphysical solutions, such as those discussed in the present paper.