1 Introduction

Optical transform systems for light beams such as Fourier transform, Hankel transform, Hilbert transform and Airy transform have been used as a valuable tool in many areas in physics due to their usefulness in beam shaping, beam analysis, image processing, signal processing and beam conversion (Goodman 2005; Ozaktas et al. 2001; Davis et al. 1998, 2000). The Airy transform was firstly introduced in mathematics by Widder (1979), then and its optical concept was introduced by Jiang et al. (2012a, b) in 2012. The authors have used the optical Airy transform in direct generating and controlling curved non-diffracting laser beams named Airy beams (Berry and Balazs 1979). These latters have attracted much attention because of their unique properties among them transverse self-acceleration, parabolic trajectories and self healing. So, they can be used in many areas such as particle clearing (Baumgartl et al. 2008), plasma physics (Polynkin et al. 2009), optical micromanipulation (Ellenbogen et al. 2009), optical switching (Chremmos and Efremidis 2012), optical trapping (Jia et al. 2010) and optical routing (Rose et al. 2013). In the other hand, the so-called finite Airy beams were observed as a new beams family of paraxial light beams (Siviloglou et al. 2007). These beams are asymmetric, with one main spot at the center and a series of secondary lobes. In the literature, there are different methods to generate the finite Airy beams like the cubic phase, 3/2 phase-only pattern and three-wave mixing processes in asymmetric nonlinear photonic crystals (Dai et al. 2009; Hu et al. 2010; Polynkin et al. 2010; Cottrell et al. 2009; Dolev et al. 2009).

In the last years, dark hollow beams with zero central intensity have attracted great attention because of their wide applications in modern optics and atomic optics (Wang et al. 2004; Cai et al. 2003; Ito et al. 1996; Kuga et al. 1997; Cai and Ge 2006). With the advancement of laser research, various hollow laser beams with different intensity distributions have been proposed; their propagation possesses an advantage to reduce the effect of linear and non-linear phenomena (Peng et al. 2008). In Liu et al. (2014) introduced a new model of hollow beams called DHIGH beams. This family of hollow laser beams possesses a central dark hollow between the symmetrical double-peaks whose size can be controlled by the beam waist radius. The propagation properties of DHIGH beams through a fractional Fourier transform optical system have been studied in detail by Saad et al. (2018). Recently, Ez-zariy et al. (2016, 2018) have obtained theoretically a novel finite Airy related beam from hyperbolic-cosine Gaussian beam illuminating an optical Airy transform system. The results show that the distance between the modules of the created Finite Airy-related beams can be adjusted and controlled. The idea of the present work is to study the effect of the well-known optical transforms such as the optical Airy transform system. The theoretical work may be beneficial to the field of high energy laser beams and have complementary results to those related to Gaussian or flat-topped Gaussian beams propagating through such optical system which have been studied by Jiang et al. (2012a, b).

In the present paper, we investigate the conversion of DHIGH beam into a superposition of finite Airy beams by using an optical Airy transform system. We consider the electrical field expressions in the Cartesian coordinates and the two dimensional Airy transform formulas. The characteristics of the transverse intensity of the new generated Airy Gauss beam are analyzed and discussed numerically.

2 Airy transform for the DHIGH beam

The electrical field of DHIGH beam is expressed at the input plane (z = 0) in the cylindrical coordinate as (Liu et al. 2014)

$$E\left( {r,z = 0} \right) = \left[ {1 - \exp \left( {\frac{{ - r^{2} }}{{w_{0}^{2} }}} \right)} \right]\,H\left( r \right),$$
(1)

where \(r\) denotes the radial radius, \(w_{0}\) is the beam waist radius and the function \(H\left( r \right)\) is defined by the following hard-edged function

$$H\left( r \right) = circ\left( {\frac{r}{{\omega_{0} }}} \right) = \left\{ {\begin{array}{*{20}l} 1 \hfill & {if\;r \le \omega_{0} } \hfill \\ 0 \hfill & {esle.} \hfill \\ \end{array} } \right.$$
(2)

As it is well known the expansion expression of the hard aperture function into a finite sum of complex Gaussian functions is given as follows (Wen and Breazeale 1988)

$$H\left( r \right) = \sum\limits_{k = 1}^{N} {A_{k} \exp \left( { - \frac{{B_{k} }}{{w_{0}^{2} }}r^{2} } \right)} ,$$
(3)

where N = 10 and \(A_{k}\) and \(B_{k}\) denote the expansion and Gaussian coefficients, respectively. The numerical values of \(A_{k}\) and \(B_{k}\) are given in Wen and Breazeale (1988).Substituting expression of Eq. (3) into Eq. (1) and by taking \(r = \sqrt {x^{2} + y^{2} }\) where \(\vec{r}\) represents the position vector, the amplitude of DHIGH beam at the initial plane can be rewritten as

$$E\left( {x,y} \right) = \sum\limits_{k = 1}^{N} {A_{k} } \exp \left[ { - \frac{{B_{k} }}{{w_{0}^{2} }}\left( {x^{2} + y^{2} } \right)} \right]\left\{ {1 - \exp \left[ { - \left( {\frac{{x^{2} + y^{2} }}{{w_{0}^{2} }}} \right)\,} \right]} \right\}.$$
(4)

In Fig. 1, we illustrate the transverse intensity of the incident DHIGH beam, for three values of the beam waist. It is seen from Fig. 1 that the intensity reaches its maximum at the edge of central spot and the diameter of the dark central region increases as the beam waist becomes larger. So, the double half inverse Gaussian beam and the flat-topped Gaussian beam (Jiang et al. 2012) have clearly complementary profiles. Indeed, there exists a correspondence between the bright spot of the flat-topped Gaussian beam and the dark central spot of the hollow Gaussian beam.

Fig. 1
figure 1

Transverse intensity profile of a DHIGH beams at the source plane z = 0 for different values of \(w_{0}\): a \(w_{0}\) = 0.8 mm, b \(w_{0}\) = 1.5 mm and c \(w_{0}\) = 3 mm

Full size image

Let us consider the optical Airy transform system (Jiang et al. 2012) as schematized in Fig. 2. The optical set up contains two thin lenses with focal length f and a spatial light modulator (SLM) used to impose to the incident beam a phase modulation \(\varphi \left( {x,y} \right) = \frac{{\alpha^{3} k_{x}^{3} + \beta^{3} k_{y}^{3} }}{3} - \left( {4kf + \pi } \right)\), where \(\alpha\) and \(\beta\) are real constants and \(k_{x} {\kern 1pt} {\kern 1pt} = \frac{{k{\kern 1pt} \cdot x}}{f}\) and \(k_{y} {\kern 1pt} {\kern 1pt} = \frac{{k{\kern 1pt} \cdot y}}{f}\) are the components of the wave number k.

Fig. 2
figure 2

A schematic description of the Airy transform setup

Full size image

By adopting the paraxial approximation and by using a formal translation of the principle of the Huygens diffraction, the two dimensional Airy transform of any incident electric field \({\rm E}_{i} \left( {x_{i} ,y_{i} } \right)\) in the Cartesian coordinate is defined as (Jiang et al. 2012)

$${\rm E}_{o} \left( {x_{o} ,y_{o} } \right) = \frac{1}{\left| \alpha \right|\left| \beta \right|}\int\limits_{ - \infty }^{ + \infty } {\int\limits_{ - \infty }^{ + \infty } {{\rm E}_{i} \left( {x_{i} ,y_{i} } \right)Ai\left( {\frac{{x_{o} - x_{i} }}{\alpha }} \right)Ai\left( {\frac{{y_{o} - y_{i} }}{\beta }} \right)} } dx_{i} dy_{i} ,$$
(5)

where \(\left( {x_{i} ,y_{i} } \right)\) and \(\left( {x_{0} ,y_{0} } \right)\) are the Cartesian coordinates of the electric field of the beam, at the input and the output planes, respectively, \(\alpha\) and \(\beta\) are real constants and Ai(·) denotes the Airy function.Substituting Eq. (4) in Eq. (5), and after some rearrangements of the double integral, we obtain

$${\rm E}_{o} \left( {x_{o} ,y_{o} } \right) = \frac{1}{\left| \alpha \right|\left| \beta \right|}\sum\limits_{k = 1}^{N} {A_{k} \left[ {I_{k} \left( {x_{o} ,y_{o} } \right) - J_{k} \left( {x_{o} ,y_{o} } \right)} \right]} \,\,,$$
(6)

where \(I_{k} \left( {x_{0} ,y_{0} } \right)\) and \(J_{k} \left( {x_{0} ,y_{0} } \right)\) are two dimensional Airy transform of Gaussian modes and are given by

$$I_{k} \left( {x_{0} ,y_{0} } \right) = \int\limits_{ - \infty }^{ + \infty } {\int\limits_{ - \infty }^{ + \infty } {\exp \left[ { - \frac{{B_{k} }}{{w_{0}^{2} }}\left( {x_{i}^{2} + y_{i}^{2} } \right)} \right]Ai\left( {\frac{{x_{o} - x_{i} }}{\alpha }} \right)Ai\left( {\frac{{y_{o} - y_{i} }}{\beta }} \right)} } dx_{i} dy_{i} ,$$
(7)

and

$$J_{k} \left( {x_{0} ,y_{0} } \right) = \int\limits_{ - \infty }^{ + \infty } {\int\limits_{ - \infty }^{ + \infty } {\exp \left[ { - \left( {\frac{1}{{w_{0}^{2} }} + \frac{{B_{k} }}{{w_{0}^{2} }}} \right)\,\left( {x_{i}^{2} + y_{i}^{2} } \right)} \right]Ai\left( {\frac{{x_{o} - x_{i} }}{\alpha }} \right)Ai\left( {\frac{{y_{o} - y_{i} }}{\beta }} \right)} } dx_{i} dy_{i} .$$
(8)

After performing a variable separation, the two-dimensional double integrals in Eqs. (7) and (8) are expressed as product of one-dimensional integrals

$$I_{k} \left( {x_{0} ,y_{0} } \right) = \int\limits_{ - \infty }^{ + \infty } {\exp \left[ { - \frac{{B_{k} }}{{w_{0}^{2} }}\left( {x_{i}^{2} } \right)} \right]Ai\left( {\frac{{x_{o} - x_{i} }}{\alpha }} \right)dx_{i} } \,\int\limits_{ - \infty }^{ + \infty } {\exp \left[ { - \frac{{B_{k} }}{{w_{0}^{2} }}\left( {y_{i}^{2} } \right)} \right]Ai\left( {\frac{{y_{o} - y_{i} }}{\beta }} \right)} \,dy_{i} ,$$
(9)

and

$$J_{k} \left( {x_{0} ,y_{0} } \right) = \int\limits_{ - \infty }^{ + \infty } {\exp \left[ { - \left( {\frac{{1 + B_{k} }}{{w_{0}^{2} }}} \right)\,\left( {x_{i}^{2} } \right)} \right]Ai\left( {\frac{{x_{o} - x_{i} }}{\alpha }} \right)dx_{i} } \,\int\limits_{ - \infty }^{ + \infty } {\exp \left[ { - \left( {\frac{{1 + B_{k} }}{{w_{0}^{2} }}} \right)\,\left( {y_{i}^{2} } \right)} \right]Ai\left( {\frac{{y_{o} - y_{i} }}{\beta }} \right)} \,dy_{i} \,.$$
(10)

To perform these last integrals we use the integral representation of the Airy function (Vallée and Soares 2004)

$$Ai\left( X \right) = \frac{1}{2\pi }\int\limits_{ - \infty }^{ + \infty } {\exp \left( {i\frac{{t^{3} }}{3} + iXt} \right)} dt.$$
(11)

Then by putting \(U\left( {x_{0} ,y_{0} } \right) = I_{k} \left( {x_{0} ,y_{0} } \right)\) or \(J_{k} \left( {x_{0} ,y_{0} } \right)\), one can substitutes Eq. (11) in Eqs. (9) and (10), and one obtains the following representation integral in one dimension

$$U\left( j \right) = \int\limits_{ - \infty }^{ + \infty } {\exp \left( {i\frac{{t^{3} }}{3}} \right)} \left\{ {\int\limits_{ - \infty }^{ + \infty } {\exp \left[ { - \delta j^{2} + ijt} \right]dj} } \right\}dt,$$
(12)

where \(j = X\) or \(Y\) and \(\delta = \frac{{B_{k} }}{{w_{0}^{2} }}\) or \(\frac{{1 + B_{k} }}{{w_{0}^{2} }}\).Using the well known integral formulas (Vallée and Soares 2004; Gradshteyn and Ryzhik 1994)

$$\int\limits_{ - \infty }^{ + \infty } {\exp \left( { - p^{2} y^{2} \pm qy} \right)} dy = \frac{\sqrt \pi }{p}\exp \left( {\frac{{q^{2} }}{{4p^{2} }}} \right),\quad \text{Re} (p^{2} )\,\,\rangle \,0,$$
(13)

and

$$\int\limits_{ - \infty }^{ + \infty } {\exp \left( {i\left[ {\frac{{t^{3} }}{3} + \alpha t^{2} + \beta t} \right]} \right)} dt = 2\pi \exp \left( {i\alpha \left[ {\frac{{2\alpha^{2} }}{3} - \beta } \right]} \right)Ai\left( {\beta - \alpha^{2} } \right),$$
(14)

and after some algebraic manipulation the electric field at the output plane reads

$$E_{0} (x_{o} ,y_{o} ) = \frac{\pi }{{\left| {\alpha \beta } \right|}}\sum\limits_{k = 1}^{N} {A_{k} } \left\{ {\frac{1}{{a_{0k} }}f_{\alpha \,k} \left( {x_{o} } \right) \times f_{\beta \,k} \left( {y_{o} } \right) - \frac{1}{{\delta_{0k} }}g_{\alpha \,k} \left( {x_{o} } \right) \times g_{\beta \,k} \left( {y_{o} } \right)} \right\}\,,$$
(15)

where

$$f_{\alpha \,k} \left( {x_{o} } \right) = \exp \left( {\frac{2}{3}\chi_{\alpha \,k}^{3} } \right)Ai\left( {\frac{{x_{0} }}{\alpha } + \chi_{\alpha \,k}^{2} } \right)\exp \left( {\frac{{x_{0} }}{\alpha }\chi_{\alpha \,k} } \right)\,,$$
(16)
$$f_{\beta \,k} \left( {y_{o} } \right) = \exp \left( {\frac{2}{3}\chi_{\beta \,k}^{3} } \right)Ai\left( {\frac{{y_{0} }}{\beta } + \chi_{\beta \,k}^{2} } \right)\exp \left( {\frac{{y_{0} }}{\beta }\chi_{\beta \,k} } \right)\,,$$
(17)
$$g_{\alpha \,k} \left( {x_{o} } \right) = \exp \left( {\frac{2}{3}\eta_{\alpha \,k}^{3} } \right)Ai\,\left( {\frac{{x_{0} }}{\alpha } + \eta_{\alpha \,k}^{2} } \right)\exp \left( {\frac{{x_{0} }}{\alpha }\eta_{\alpha \,k} } \right)\,,$$
(18)

and

$$g_{\beta \,k} \left( {y_{o} } \right) = \exp \left( {\frac{2}{3}\eta_{\beta \,k}^{3} } \right)Ai\left( {\frac{{y_{0} }}{\beta } + \eta_{\beta \,k}^{2} } \right)\exp \left( {\frac{{y_{0} }}{\beta }\eta_{\beta \,k} } \right),$$
(19)

with

$$a_{0k} = \frac{{B_{k} }}{{\omega_{0}^{2} }}\,,$$
(20)
$$\delta_{0k} = \frac{{1 + B_{k} }}{{\omega_{0}^{2} }},$$
(21)
$$\chi_{\alpha \,k} = \frac{1}{{4\alpha^{2} a_{0k} }},$$
(22)
$$\chi_{\beta \,k} = \frac{1}{{4\beta^{2} a_{0k} }},$$
(23)
$$\eta_{\alpha \,k} = \frac{1}{{4\alpha^{2} \delta_{0k} }},$$
(24)

and

$$\eta_{\beta \,k} = \frac{1}{{4\beta^{2} \delta_{0k} }}.$$
(25)

The field distribution expressed by Eq. (15) is the main result of the present work. It can be regarded as superposition of finite Airy beams. As a kind of important remark, the terms of the coefficients \(\chi_{\alpha \,k}\) and \(\chi_{\beta \,k}\) are symmetrical if we switch \(\alpha\) by \(\beta\); the same to the coefficients \(\eta_{\alpha \,k}\) and \(\eta_{\alpha \,k}\). A simple comparison between Eq. (15) and that one obtained in Jiang et al. (2012), shows that the coefficient of the two series are different; in the hollow beam case, the coefficients \(A_{k}\) and \(B_{k}\) are evaluated after the result of Wen and Breazeale (1988), and their number are fixed at N = 10, whereas in the flat-topped beam case, the number of summation N is arbitrary. Furthermore, there are additional terms \(\frac{{\omega_{0}^{2} }}{{B_{k} }}\) and \(\frac{{\omega_{0}^{2} }}{{1 + B_{k} }}\) which are assigned to the Airy beam components. In the next section, we will analyze numerically the influence of the constants \(\alpha\) and \(\beta\) and the parameters of the incident beam.

3 Numerical analysis and discussion

In this paragraph, we examine numerically the intensity profile the finite sum of finite Airy beam generated at the output plane of the optical Airy transform illuminated by DHIGH beam based on the main expression of Eq. (15). In the plots of Fig. 3, we present the intensity profile obtained for the same values of \(\alpha\) and \(\beta\) (in modulus). As it can be seen, the localization of the output field is determined by the relative signs of \(\alpha\) and \(\beta\). For instance, in Fig. 3b, the field appears in the second quadrant when \(\alpha\) and \(\beta\) are negative, meanwhile, the field appears in the fourth quadrant (Fig. 3d) when \(\alpha\) is negative and \(\beta\) is positive. So, the Airy transform converts the dark central spot of the incident beams into peak intensity. Furthermore, we can adapt the localization of the outgoing electrical field in which part of the plan the secondary lobes are visualized according to the sign of \(\alpha\) and \(\beta\). These results are in good agreement with theoretical and experimental studies (Jiang et al. 2012a, b; Ez-zariy et al. 2016, 2018).

Fig. 3
figure 3

The transverse intensity of the output electric field when \(w_{0}\) = 0.8 mm for different signs of the Airy transform parameters \(\alpha\) and \(\beta\): a \(\alpha = \left( { - \beta } \right) = 2\,{\text{mm}}\), b \(\left( { - \alpha } \right) = \left( { - \beta } \right) = 2\,{\text{mm}}\), c \(\alpha = \beta = 2\,{\text{mm}}\) and d \(\left( { - \alpha } \right) = \beta = 2\,{\text{mm}}\)

Full size image

To examine the influence of the Airy transform parameters \(\alpha\) and \(\beta\) on the generated beam, we present in Fig. 4, the transverse intensity in the output plane. As it is shown that as these parameters increases, the intensity of the output field becomes more intense for the central peak and the secondary lobes. We note also that the radius size of the beam becomes bigger. Therefore, the nature of the electrical field at the receiver plane can be controlled by varying the parameters \(\alpha\) and \(\beta\) of the SLM. In Fig. 5, we present the intensity distribution of generated beam for different values of \(\alpha\) and \(\beta\). In Fig. 5a–c, as \(\beta\) increases, the output field takes a flattened (or elliptic) shape, and the lobes radius increases while their numbers decrease. In Fig. 5d–f, we interchange the value of \(\beta\) and \(\alpha\), we have the same phenomenon, the only difference is the beamlets swap their positions according to the axes of the plan.

Fig. 4
figure 4

The transverse intensity of the output electric field when \(w_{0}\) = 3 mm for different values of the Airy transform parameters \(\alpha\) and \(\beta\). a \(\alpha = \left( { - \beta } \right) = 2\,{\text{mm}}\), b \(\alpha = \left( { - \beta } \right) = 4\,{\text{mm}}\) and c \(\alpha = \left( { - \beta } \right) = 5\,{\text{mm}}\)

Full size image
Fig. 5
figure 5

The intensity distribution of the output electric field when \(w_{0}\) = 0.8 mm for different values of the Airy transform parameters \(\alpha\) and \(\beta\): a \(\alpha = 2\,{\text{mm}}\) and \(\beta = 3\,{\text{mm}}\), b \(\alpha = 2\,{\text{mm}}\) and \(\beta = 5\,{\text{mm}}\), c \(\alpha = 2\,{\text{mm}}\) and \(\beta = 6\,{\text{mm}}\), d \(\alpha = 3\,{\text{mm}}\) and \(\beta = 2\,{\text{mm}}\), e \(\alpha = 5\,{\text{mm}}\) and \(\beta = 2\,{\text{mm}}\) and f \(\alpha = 6\,{\text{mm}}\) and \(\beta = 2\,{\text{mm}}\)

Full size image

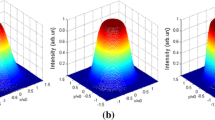
Plots in Fig. 6 are depicted to illustrate the effect of the radius size of the input field on the transverse intensity. We can see from these figures (for \(w_{0} = 0.8\,\), 1.5 and 3 mm), that the diameter size of the central spot remains unchanged but the secondary lobes disappear progressively with the increasing of the radius size \(w_{0}\). Then, in Fig. 6d, the central peak intensity is divided to three lobes when the radius is equal to 6 mm and the secondary lobes disappear completely. But when the radius is greater than 6 mm (Fig. 6e, f), the outgoing field loses its appearance of the finite Airy beam and takes its dark-hollow profile. Moreover, it can be clearly seen from Fig. 6e, f that a further increase of the spot radius affect the additional terms \(\frac{{\omega_{0}^{2} }}{{B_{k} }}\) and \(\frac{{\omega_{0}^{2} }}{{1 + B_{k} }}\) which are assigned to the Airy components under summation, of the beam; these terms become dominant and the interference between the Airy components may affect drastically the Airy-like profile and then gives a complex pattern as in Fig. 6d–f. It is worthy to note that this result is consistent with the result of previous works (Jiang et al. 2012; Ez-zariy et al. 2018), on the output field generated for Gaussian and the hyperbolic-cosine Gaussian beams through the Airy transform system.

Fig. 6
figure 6

The intensity distribution of the output electric field when \(\alpha = \left( { - \beta } \right) = 2\,{\text{mm}}\) for different values of the beam waist \(w_{0}\): a \(w_{0}\) = 0.8 mm, b \(w_{0}\) = 1.5 mm, c \(w_{0}\) = 3 mm, d \(w_{0}\) = 6 mm, e \(w_{0}\) = 10 mm and f \(w_{0}\) = 20 mm

Full size image

4 Conclusion

In summary, we have investigated the conversion of a hollow DHIGH beam into a finite sum of finite Airy beam by using the optical Airy transform. Numerical simulations were performed to study the effect of the phase pattern’s constants of the Airy transform system and the radius size of the incident DHIGH beam. It’s shown that the sign of constants \(\alpha\) and \(\beta\) play a key role on the localization of the output field in the plane. Moreover if these constants aren’t equal, we observe that the output electrical field takes an elliptic shape and the peak intensity. It is shown that when the radius size \(w_{0}\) of the incident beam increases the secondary lobes vanish gradually and the central peak intensity is divided when the radius becomes important. These results may be useful in many practical applications concerning hollow beams.