The optimal financing of a conglomerate firm with hidden information and costly state verification

Abstract

This manuscript addresses the issue, particularly interesting for a conglomerate firm, of the choice of the optimal financing method (namely, the most efficient one) between the joint one and the separate one. In particular, the authors identify the properties of the optimal financing contract for three investment projects under the assumptions of the literature on Costly State Verification (CSV), namely, uncorrelated returns, hidden information (the return of a single project is a borrower’s private information), lender performing sequential audit and residual claimant borrower. The authors’ research method consists of solving the optimization problem of the borrower’s expected utility subject to appropriate incentive constraints and the lender’s participation constraint. The novelty of this contribution is the demonstration that joint financing with return pooling between the high and low states is more efficient than separate financing, as it implies a lower expected audit cost for the lender and, if the investment cost is not too high, also less credit rationing for the borrower. Joint financing with return pooling between the intermediate and low states, instead, is found to be less efficient than separate financing in terms of expected audit cost and, in the presence of sufficiently high investment cost, also credit rationing.

1 Introduction

One of the main problems currently faced by economic research is the incorporation of uncertainty into equilibrium models.

As Arrow (1964) and Debreu (1959) argue, this problem may be addressed in a relatively simple way in the case of complete information, even taking into account the uncertainty about the environment (namely, the state of nature known from the beginning until the end of an economy).

Under asymmetric information, however, the question of the existence and identification of the Pareto-efficient equilibrium becomes much more complex. Arrow (1974), in fact, notes that, in all those situations in which the outcome of a certain random variable is observable only by one of the two parties (agent), the set of possible contracts is limited to those whose outcome is verifiable by both of them (principal and agent). Radner (1968), instead, observes that the information structure of an economy is endogenous and costly. In particular, he argues that in an economy in which there is asymmetric information, namely, the realisation of a certain state of nature is private information of one of the two parties (agent), the other (principal) is able to acquire this information only through a specific verification procedure (audit), implying a cost. This intuition serves as the foundation for Costly State Verification (CSV), a branch of theory aimed at writing down the optimal financing contract, that is, the one minimising the lender's expected audit cost and the borrower's credit rationing.

This minimization problem is important because, as highlighted by Carlier and Renou (2005), the higher the expected audit cost, the smaller the set of optimal contracts (and then the more credit rationing).

The first contributions to the CSV theory are those of Spence and Zeckhauser (1971), Shavell (1979), Harris and Raviv (1979), who analyse the case in which the hidden information concerns the level of commitment made by the agent.

Instead, in his well-known manuscript on the insurance market, Townsend (1979) focuses on the more general case in which the hidden information is given by the realisation of a random variable.

The four main issues relating to the Costly State Verification are: the choice between stochastic and deterministic audit; the choice between direct and delegated monitoring; the choice between joint and separate financing of several investment projects; the choice between debt and equity contract.

Regarding the first question, Townsend (1979) demonstrates that, under the assumption of deterministic audit (namely, that associating a probability equal to either 0 or 1 with the execution of the verification procedure), it is optimal to carry out the audit only when the reported return is below a certain threshold. However, he also finds out that, in specific cases, stochastic audit (assigning a probability ranging between 0 and 1 to the execution of the verification procedure) dominates deterministic monitoring.

Mookherjee and Png (1989), who focus on the credit market, prove that deterministic audit is optimal only when there are two possible states of nature (low income and high income), while, when more than two realizations are possible, stochastic audit is optimal.

Border and Sobel (1987) discover that the optimal audit probability is a decreasing function of the return of the investment project. Menichini and Simmons (2006), on the other hand, demonstrate that, by adding a layer of ex-ante information acquisition correlated with future project returns, optimal audit becomes deterministic and targeted on some signal-state combinations.

Regarding the second issue relating to CSV, Diamond (1984) highlights that delegated monitoring, consisting of the execution of the audit by an appropriate intermediary (delegated monitor), is optimal when the same investment project is financed by several creditors, because it avoids unnecessary cost multiplication. However, other scholars have pointed out that delegated monitoring is optimal only under additional conditions (Leland and Pyle 1977; Chan 1983) and, in any case, always implies a problem of double asymmetric information, because the creditor is exposed to the risk that the delegated monitor gets her compensation without doing her job (Krasa and Villamil 1992).

With regard to the third issue relating to CSV, joint financing of several investment projects dominates separate financing only if the recovery of efficiency obtained thanks to cross-pledging, namely, the fact that the returns of successful projects act as a guarantee for the unsuccessful ones, is greater than the loss of efficiency due to risk contamination, that is to the fact that the failure of one or more projects can also have a negative impact on the outcome of the others. Otherwise, separate financing dominates joint funding. The criteria for choosing between joint and separate financing, however, have been identified by Banal-Estañol et al. (2013).

With respect to the fourth issue relating to CSV, the optimal repayment covenant between standard debt and equity contract depends on the assumptions of each model. For example, according to both Townsend (1979) and Gale and Hellwig (1985), it is optimal to pledge to the lender both the entire lowest state return and a constant repayment in all the other states (standard debt contract). On the other hand, in Webb's model (1992) it is optimal to pledge to the lender a repayment depending on the state of nature (like-equity contract).

The literature on Costly State Verification is constantly growing because of the increasing importance of the economic sectors concerned with the phenomenon of asymmetric information (such as insurance and credit markets). As pointed out in the next paragraph, the goal of this manuscript is to make a contribution to this literature by finding the properties of the optimal financing contract for three investment projects.

The paper is structured as follows: in Sect. 1, a short introduction to the theory of Costly State Verification (CSV) is offered; in Sect. 2, the research aims of this manuscript are explained; in Sect. 3, the hypotheses of the theoretical model are described; in Sect. 4, the authors’ theoretical model is presented; in Sect. 5, the properties of the optimal financing contract for the three investment projects under the assumption of separate financing are shown; in Sects. 6, 7, 8 and 9, the properties of the optimal financing contract for three investment projects under different assumptions about return pooling are reported; in Sect. 10, separate and joint financing are compared in order to identify the most efficient financing method between them. Finally, in Sect. 11, the conclusions of this research are drawn. A detailed bibliography and an Appendix conclude the paper.

2 Research aims

In this paper, the authors aim at identifying the properties of the optimal financing contract for three investment projects under the assumptions of the literature on Costly State Verification (CSV): the returns of the projects are uncorrelated; the net present value (NPV) of each project is positive; the lender performs a sequential audit; there are only two possible states of nature (high state and low state); the audit cost is constant and the borrower is a residual claimant (namely, her compensation is given by that fraction of the return exceeding the repayment pledged to the lender).

More precisely, from an analytical point of view, the model proposed in this manuscript consists of an optimization problem of the borrower’s expected utility, under a constraint that guarantees that the expected return promised to the lender is at least such as to compensate the investment cost and the audit costs (participation constraint) and under a set of constraints aimed at discouraging the borrower from reporting a lower return than that actually obtained (incentive constraints).

This model has been solved in three distinct cases in order to discover the properties of the optimal contract in each of them: joint financing of the three investment projects without return pooling; joint financing of the three investment projects with return pooling from three successes and from two successes and one fail; joint financing of the three investment projects with return pooling from three successes, from two successes and one fail and from two fails and one success.

The authors also compare joint financing with separate financing to find the most efficient financing method (namely, the one that implies the lowest expected audit cost and the least credit rationing).

In other words, this paper focuses on the issue of choosing the optimal financing method between joint financing and separate financing.

The authors arrive at two main results: the first one is that the optimal contract for the joint financing of three projects has similar properties to the optimal contract for the joint financing of two projects found by Menichini and Simmons (2017), while the second one is that joint financing of three investment projects is more efficient than separate financing only under appropriate assumptions regarding return pooling and investment cost.

The importance of this manuscript consists in the fact that it deals with a topic (namely, the choice of the most efficient financing method between joint and separate financing) that is particularly relevant in the case of conglomerate firms, namely, the groups made up of companies linked together by a system of crossholdings or informal relations (as in the case of Japanese keiretsu) and often operating in different sectors. In this regard, Faure-Grimaud and Inderst (1999) underline that conglomeration (and therefore the joint financing of investment projects) is efficient when the profitability of the companies in the group is such that the pooling of expected cash flows facilitates the access to the credit market. When, instead, some of the companies in the group are less profitable, the credit rationing constraint becomes more binding, because of the risk of contamination and, therefore, only separate financing makes the projects viable.

Inderst and Müller (2003) add that joint financing may slacken the credit rationing constraint of the conglomerate firm only in the presence of capital market discipline.

Banal-Estañol et al. (2013), instead, identify the criteria under which joint financing dominates separate financing (and vice versa). In particular, they suggest taking into account the average returns of investment projects, the variability of returns, the correlation between the returns and the distribution of the returns (especially fat tails and kurtosis).

Menichini and Simmons (2017) deepen the results of Banal-Estañol et al. (2013) focusing on a further relevant element for the choice between joint and separate financing: the number of projects. More specifically, they show that joint financing of two investment projects always dominates separate financing (namely, is more efficient as it always implies a lower expected audit cost and less credit rationing) thanks to the fact that the creditor is able to reduce the audit frequency in the intermediate state (one success and one fail) and increase it in the low state (two fails).

This manuscript falls into the path traced by the scholars cited above and, more precisely, extends the work of Menichini and Simmons (2017) by considering the case in which there are three investment projects to be financed.

The main innovation of this paper compared to that of Menichini and Simmons (2017) is demonstrating that joint financing of three investment projects dominates separate financing only under specific conditions about return pooling and the size of the investment costs. In other words, this paper proves that the result of Menichini and Simmons (2017) is not robust with respect to the number of investment projects: it is (at least partially) refuted when the number of projects grows from two to three.

3 The assumptions of the model

Consider a risk-neutral investor bargaining with a lender to finance three investment projects with uncorrelated returns.

The investment cost of a single project is equal to $I$.

The return of each project is a random variable that may assume only two possible outcomes: success ($H$) and failure ($L$).

Let $S\subset \left\{H,L\right\}$ with $H>I>L>0$ be the set of random returns of a single project. The realisation probability of the high state $H$ is equal to $p$, while the probability of the low state $L$ is equal to $(1-p)$.

Let $N\in \left\{{0,1},\text{2,3}\right\}$ be the number of the successful projects (or the number of projects with a high state return). For $N=0$, all projects fail ($LLL$); for $N=1$, one project succeeds, and two projects fail ($HLL$); for $N=2$, two projects succeed, and one fails ($HHL$); for $N=3$, all projects succeed ($HHH$).

Since the borrower is a residual claimant and is the only one who may observe the outcome of the projects, she has an incentive to report a return lower than the effective.

Let $\sigma \in \left\{{0,1},\text{2,3}\right\}$ be the number of possible successes reported by the borrower.

For $N=3$, $\sigma$ may take a value of 0, 1, 2 or 3, namely, the borrower may either be fair by reporting three successes ($HHH$) or lie by reporting two successes and one fail ($HHL$), one success and two fails ($HLL$) and three fails ($LLL$).

For $N=2$, $\sigma$ may take a value of 0, 1 or 2, namely, the borrower may be fair by reporting two successes and one fail ($HHL$), or she may lie by reporting one success and two fails ($HLL$) and three fails ($LLL$). For $N=1$, $\sigma$ may take the value 0 or 1, namely, the borrower may be fair by reporting one success and two fails ($HLL$), or she may lie by reporting three fails ($LLL$).

For $N=0$, the borrower always reports three fails ($LLL$) as she is unable to lie $(\sigma =0)$.

The lender may know the effective return obtained by the borrower only through a sequential audit, whose cost $c>0$ is constant.

Let $\vartheta \in \left\{H,L\right\}$, ${R}_{N|{\vartheta \vartheta \vartheta }}$ and ${m}_{N,\vartheta \vartheta \vartheta }\in [{0,1}]$ be, respectively, the outcome of the single investment project reported by the borrower, the return of each of the three investment projects reported by the borrower and the probability of carrying out a sequential audit on each of the three projects.

The return ${R}_{N|{\vartheta \vartheta \vartheta }}$ may be audited only once (audit on the first project), twice (audit on the first and second project), three times (audit on the first, second and third projects), or it may not be audited at all. The only case in which ${R}_{N|{\vartheta \vartheta \vartheta }}$ is not audited for sure is ${R}_{N|{\vartheta \vartheta \vartheta }}={R}_{3}$, as the borrower reports three successes ($HHH$).

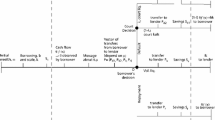
The sequence of events, represented in the game tree in Fig. 1, is the following:

• (1) The borrower and the lender sign the financing contract;

• (2) The borrower observes the outcome of the three investment projects ($N$);

• (3) The borrower, except in the case $N=0$ in which is unable to lie, ($\sigma$); decides how many successes to report ($\sigma$);

• (4) If $\sigma <3$, the lender audits each project with a probability ranging between 0 and 1 (${m}_{N,\vartheta \vartheta \vartheta }$) and, doing so, she discovers the true returns of the three projects (${R}_{N|{\vartheta \vartheta \vartheta }}$);

• (5) The borrower repays the lender.

Fig. 1

Game tree

From the game tree, the borrower's expected utility $(E{\Pi }_{B})$ is:

$$\begin{aligned} E{\Pi }_{B}=\,&{p}^{3}\left(3H-{R}_{3}\right)+3{p}^{2}\left(1-p\right)\left[2H+L-{m}_{2}{R}_{2|\text{L }}-\left(1-{m}_{2}\right){R}_{2|. }\right]\\ &+3p{\left(1-p\right)}^{2}\left\{2L+H-{m}_{1}\left[{m}_{1,L}{R}_{1|\text{ LL}}+\left(1-{m}_{1,L}\right){R}_{1|\text{L}. }\right]\right.\\ &\left.-\left(1-{m}_{1}\right){R}_{1|. }\right\}+{\left(1-p\right)}^{3}\\ &\left\{3L-{m}_{0}\left[{m}_{0,L}\left({m}_{0,LL}{R}_{0|\text{LLL}} +\left(1-{m}_{0,LL}\right){R}_{0|\text{LL}.}\right)\right.\right.\\ &\left.\left.+\left(1-{m}_{0,L}\right){R}_{0|\text{L}.} \right]-\left(1-{m}_{0}\right){R}_{0|.}\right\}\end{aligned}$$

and the lender’s participation constrain ($PC$) is:

$$ \begin{aligned} E{\Pi }_{L}=\,&{p}^{3}{R}_{3}+3{p}^{2}\left(1-p\right)\left\{{m}_{2}\left({R}_{2|\text{L }}-c\right)+\left(1-{m}_{2}\right){R}_{2|. }\right\}\\ &+3p{\left(1-p\right)}^{2}\left\{{m}_{1}\left[{m}_{1,L} \left({R}_{1|\text{LL}.}-c\right)+\left(1-{m}_{1,L}\right){R}_{1|\text{L}.}-c\right] +\left(1-{m}_{1}\right){R}_{1|.}\right\}\\ &+{\left(1-p\right)}^{3}\left\{{m}_{0} \left[{m}_{0,L}\left({m}_{0,LL}\left({R}_{0|\text{LLL}}-c\right)\right.\right.\right.\\ & \left.\left.\left. + \,\left(1-{m}_{0,LL}\right){R}_{0|\text{LL}.}-c\right) +\left(1-{m}_{0,L}\right){R}_{0|\text{L}.} -c\right]+\left(1-{m}_{0}\right){R}_{0|.}\right\}\ge 3I \end{aligned}$$

namely, the project may be financed only if the expected return promised to the lender, net of the expected audit cost, is at least equal to the investment cost. Put differently, the $PC$ indicates that the lender may know the true return obtained by the borrower from each project only thanks to a costly sequential audit whose cost $c>0$ is constant and reduces her expected utility.

Also from the game tree, it is possible to derive the incentive constraints, namely, the constraints that must be met to discourage the borrower from lying, for every possible outcome $N$.

For $N=3$, there are three incentive constraints:

$${R}_{3}\le \left(1-{m}_{2}\right){R}_{2|. }+{m}_{2}{R}_{2|\text{H}}$$

$${R}_{3}\le \left(1-{m}_{1}\right){R}_{1|. }+{m}_{1}\left[{m}_{1,H}{R}_{1|\text{HH }}+\left(1-{m}_{1,H}\right){R}_{1|\text{H}.}\right]$$

$$\begin{aligned}{R}_{3}\le &\,\left(1-{m}_{0}\right){R}_{0|. }+{m}_{0}\left\{{m}_{0,H}\left[{m}_{0,HH}{R}_{0|\text{ HHH}}+\left(1-{m}_{0,HH}\right){R}_{0|\text{ HH}.}\right]\right.\\ &\left.+\left(1-{m}_{0,H}\right){R}_{0|\text{H}.}\right\} \end{aligned}$$

For $N=2$, the incentive constraints are two:

$$\begin{aligned} &\left(1-{m}_{2}\right){R}_{2|.}+{m}_{2}{R}_{2|\text{L}}\le \left(1-{m}_{1}\right){R}_{1|.}+{m}_{1}\left\{{0,5} \left[{m}_{1,H}{R}_{1|\text{HL }}\right.\right.\\ &\qquad\left.\left.+\left(1-{m}_{1,H}\right){R}_{1|\text{H}.}\right]+{0,5} \left[{m}_{1,L}{R}_{1|LH}+\left(1-{m}_{1,L}\right){R}_{1|\text{L}.}\right]\right\} \end{aligned}$$

$$\begin{aligned} \left(1-{m}_{2}\right){R}_{2|.}+{m}_{2}{R}_{2|\text{L}}& \le \left(1-{m}_{0}\right){R}_{0|.}\\ &\quad +{m}_{0}\left\{{0,5}\left[{m}_{0,H}\left[{0,5} \left(\left(1-{m}_{0,HL}\right){R}_{0|\text{HL}.}\right.\right.\right.\right.\\ &\quad \left.\left.+{m}_{0,HL}{R}_{0|\text{HLH}}\right)+\,{0,5}\left(\left(1-{m}_{0,HH}\right){R}_{0|\text{HH}.} +{m}_{0,HH}{R}_{0|\text{HHL}}\right)\right] \\ &\quad \left.+\left(1-{m}_{0,H}\right){R}_{0|\text{H}.}\right] +\,{0,5}\left.\left[{m}_{0,L}\left(\left(1-{m}_{0,LH}\right){R}_{0|\text{LH}.}+{m}_{0,LH}{R}_{0|\text{LHH}}\right)\right.\right.\\ &\quad \left.\left.+\left(1-{m}_{0,L}\right){R}_{0|\text{L}.}\right]\right\}\end{aligned}$$

For $N=1$, there is only one incentive constrint:

$$\begin{aligned} \left(1-{m}_{2}\right){R}_{2|.}+{m}_{2}{R}_{2|\text{L}}&\le \left(1-{m}_{0}\right){R}_{0|.}\\ & +{m}_{0}\left\{{0,5}\left[{m}_{0,H}\left[{0,5}\left(\left(1-{m}_{0,HL}\right){R}_{0|\text{HL}.}+{m}_{0,HL}{R}_{0|\text{HLH}}\right)\right.\right.\right.\\ & \qquad\left.+\, {0,5}\left(\left(1-{m}_{0,HH}\right){R}_{0|\text{HH}.}+{m}_{0,HH}{R}_{0|\text{HHL}}\right)\right]\\ &\qquad \left. +\left(1-{m}_{0,H}\right){R}_{0|\text{H}.}\right]+{0,5}\left[{m}_{0,L}\left(\left(1-{m}_{0,LH}\right){R}_{0|\text{LH}.}\right.\right.\\ &\qquad \left.\left.\left.+{m}_{0,LH}{R}_{0|\text{LHH}}\right)+\left(1-{m}_{0,L}\right){R}_{0|\text{L}.}\right]\right\}\end{aligned}$$

For $N=0$, as specified above, the borrower is unable to lie and, therefore, it is unnecessary to impose any incentive constraint.

The other constraints are represented by the limited liability conditions:

$${R}_{3},{R}_{2|\text{H}.}, {R}_{1|\text{HH}.},{R}_{0|\text{HHH}}\le 3H$$

$${R}_{2|.},{R}_{2|\text{L}},{R}_{1|\text{HL}},{R}_{1|\text{LH}},{R}_{0|\text{HH}}{,R}_{0|\text{HHL}},{R}_{0|\text{HLH}},{R}_{0|\text{LHH}}\le 2H+L$$

$${R}_{1|.},{R}_{1|\text{L}},{R}_{1|\text{HL}},{R}_{1|\text{LH}},{R}_{0|\text{H}.},{R}_{0|\text{HL}.},{R}_{0|\text{LH}.},{R}_{0|\text{HLL}}\le H+2L$$

$${R}_{0|.},{R}_{0|\text{L}.},{R}_{0|\text{LL}.},{R}_{0|\text{LLL}}\le 3L$$

Moreover, in order to make the financing contract feasible, the following condition must hold:

Condition 1

(Individual Feasibility ($IF$)). The Net Present Value (NPV) of each project must be higher than the investment cost and the expected audit cost:

$$IF=pH+ \left(1-p\right)L-I > \left(1-p\right)c$$

Condition 1 guarantees that the NPV of the joint financing of the three projects is strictly positive.

Finally, it is possible to define two different pooling feasibility conditions

Condition 2

(First Pooling Feasibility (${PF}_{1}$)). The returns coming from two successes and one fail ($2H+L$), from one success and two fails ($H+2L$) and from three fails ($3L$) are at least such as to offset the investment cost and the expected audit cost:

$$\begin{aligned}{p}^{3}\left(2H+L\right)&+3{p}^{2}\left(1-p\right)\left(2H+L\right)+3p{\left(1-p\right)}^{2}\left(H+2L-2c\right)\\&+{\left(1-p\right)}^{3}3L\ge 3\left[I+{\left(1-p\right)}^{3}c\right]\end{aligned}$$

To get ${PF}_{1}$, it is necessary to set ${m}_{2}=0$; ${m}_{1}={m}_{1,L}={m}_{0}={m}_{0,L}={m}_{0,LL}=1$; ${R}_{3}={R}_{2|. }={R}_{2|\text{L }}=2H+L$; ${R}_{1|.}={R}_{1|\text{L}.}={R}_{1|\text{LL}.}=H+2L$ and ${R}_{0|.}={R}_{0|\text{L}.}={R}_{0|\text{LL}.}={R}_{0|\text{LLL}}=3L$ in $PC$.

Condition 3

(Second Pooling Feasibility (${PF}_{2}$)). The returns coming from two fails and one success ($H+2L$) and from three fails ($3L$) are at least such as to offset the investment cost and the expected audit cost:

$$\begin{aligned}{p}^{3}\left(H+2L\right)&+3{p}^{2}\left(1-p\right)\left(H+2L\right)+3p{\left(1-p\right)}^{2}\left(H+2L\right)\\&+{\left(1-p\right)}^{3}3L\ge 3\left[I+{\left(1-p\right)}^{3}c\right]\end{aligned}$$

To get ${PF}_{2}$, it is necessary to set ${m}_{2}={m}_{1}={m}_{1,L}=0$; ${m}_{0}={m}_{0,L}={m}_{0,LL}=1$; ${R}_{3}={R}_{2|. }={R}_{2|\text{L }}={R}_{1|.}={R}_{1|\text{L}.}={R}_{1|\text{LL}.}=H+2L$ and ${R}_{0|.}={R}_{0|\text{L}.}={R}_{0|\text{LL}.}={R}_{0|\text{LLL}}=3L$ in $PC$.

4 The model

In order to find the properties of the optimal financing contract, it is necessary to solve the following optimization problem of the borrower's expected utility under the participation constraint, the incentive constraints and the limited liability conditions:

$$\begin{aligned}\text{max}E{\Pi }_{B}&= {p}^{3}\left(3H-{R}_{3}\right)+3{p}^{2}\left(1-p\right)\left[2H+L-{m}_{2}{R}_{2|\text{L}. }-\left(1-{m}_{2}\right){R}_{2|. }\right]\\ &\quad +3p{\left(1-p\right)}^{2}\left\{H+2L-{m}_{1}\left[{m}_{1,L}{R}_{1|\text{ LL}.}+\left(1-{m}_{1,L}\right){R}_{1|\text{L}. }\right]-\left(1-{m}_{1}\right){R}_{1|. }\right\}\\ &\quad+\left(1-p\right)^{3}\left\{3L-{m}_{0} \left[{m}_{0,L} \left({m}_{0,LL}{R}_{0|\text{LLL}}+ \left(1-{m}_{0,LL}\right){R}_{0|\text{LL}.}\right)\right.\right.\\ &\quad \left.{ \left. +\,\left(1-{m}_{0,L}\right){R}_{0|\text{L}.}\right]- \left(1-{m}_{0}\right){R}_{0|.}}\right\}\end{aligned} $$

subject to:

$$\begin{aligned} {p}^{3}{R}_{3}&+3{p}^{2}\left(1-p\right)\left\{{m}_{2}\left({R}_{2|\text{L }}-c\right)+\left(1-{m}_{2}\right){R}_{2|. }\right\}\\ &+3p{\left(1-p\right)}^{2}\left\{{m}_{1}\left[{m}_{1,L}\left({R}_{1|\text{LL}}-c\right)+\left(1-{m}_{1,L}\right){R}_{1|\text{L}.}-c\right]+\left(1-{m}_{1}\right){R}_{1|.}\right\}\\ &+{\left(1-p\right)}^{3}\left\{ {m}_{0}\left[ {m}_{0,L}\left({m}_{0,LL} \left({R}_{0|\text{LLL}}-c\right)+\left(1-{m}_{0,LL}\right){R}_{0|\text{LL}.}-c\right) \right.\right.\\ & \left.\left.+\, \left(1-{m}_{0,L}\right){R}_{0|\text{L}.}-c\right]+\left(1-{m}_{0}\right){R}_{0|.}\right\}\ge 3I \end{aligned}$$

(1)

$${R}_{3}\le \left(1-{m}_{2}\right){R}_{2|. }+{m}_{2}{R}_{2|\text{H}}$$

(2)

$${R}_{3}\le \left(1-{m}_{1}\right){R}_{1|. }+{m}_{1}\left[{m}_{1,H}{R}_{1|\text{HH}. }+\left(1-{m}_{1,H}\right){R}_{1|\text{H}.}\right]$$

(3)

$$\begin{aligned} {R}_{3}&\le \left(1-{m}_{0}\right){R}_{0|. }+{m}_{0}\left\{{m}_{0,H}\left[{m}_{0,HH}{R}_{0|\text{ HHH}}+\left(1-{m}_{0,HH}\right){R}_{0|\text{ HH}.}\right]\right. \\ &\quad\left.+\left(1-{m}_{0,H}\right){R}_{0|\text{H}.}\right\} \end{aligned} $$

(4)

$$\begin{aligned} \left(1-{m}_{2}\right){R}_{2|.}&+{m}_{2}{R}_{2|\text{L}}\le \left(1-{m}_{1}\right){R}_{1|.}+{m}_{1}\left\{{0,5} \left[{m}_{1,H}{R}_{1|\text{HL}}+\left(1-{m}_{1,H}\right){R}_{1|\text{H}.}\right]\right. \\ &+{0,5}\left. \left[{m}_{1,L}{R}_{1,LH}+\left(1-{m}_{1,L}\right){R}_{1|\text{L}.}\right]\right\} \end{aligned} $$

(5)

$$\begin{aligned} {}&\left(1-{m}_{2}\right){R}_{2|.}+{m}_{2}{R}_{2|\text{L}}\le \left(1-{m}_{1}\right){R}_{1|.}+{0,5} {m}_{1}3\left\{H+L\right\} \left(1-{m}_{2}\right){R}_{2|.}\\ &\quad+{m}_{2}{R}_{2|\text{L}}\le \left(1-{m}_{0}\right){R}_{0|.}+{m}_{0}\left\{{0,5}\left[{m}_{0,H}\left[{0,5}\left(\left(1-{m}_{0,HL}\right){R}_{0|\text{HL}.}+{m}_{0,HL}{R}_{0|\text{HLH}}\right)\right.\right.\right.\\ & \quad\left.+\left.{0,5}\left(\left(1-{m}_{0,HH}\right){R}_{0|\text{HH}.}+{m}_{0,HH}{R}_{0|\text{HHL}}\right)\right]+\left(1-{m}_{0,H}\right){R}_{0|\text{H}.}\right] \\ &\quad\left.+\,{0,5}\left[{m}_{0,L}\left(\left(1-{m}_{0,LH}\right){R}_{0|\text{LH}.}+{m}_{0,LH}{R}_{0|\text{LHH}}\right)+\left(1-{m}_{0,L}\right){R}_{0|\text{L}.}\right]\right\} \end{aligned} $$

(6)

$$\begin{aligned} \left(1-{m}_{1}\right){R}_{1|.}&+{m}_{1}\left[\left(1-{m}_{1,L}\right){R}_{1|\text{L}.}+{m}_{1,L}{R}_{1|\text{LL}.}\right]\\ &\le \left(1-{m}_{0}\right){R}_{0|.}+{m}_{0}\left\{{0,5}\left[{m}_{0,H}\left(\left(1-{m}_{0,HL}\right){R}_{0|\text{HL}.}+{m}_{0,HL}{R}_{0|\text{HLL}}\right)\right.\right.\\ & \left.+\,\left(1-{m}_{0,H}\right){R}_{0|\text{H}.}\right] +{0,5}\left[{ m}_{0,L}\left[{0,5}\left(\left(1-{m}_{0,LL}\right){R}_{0|LL.}+{m}_{0,LL}{R}_{0|\text{LLH}}\right)\right.\right.\\ &\left.\left.\left.+\,{0,5}\left(\left(1-{m}_{0,LH}\right){R}_{0|LH.}+{m}_{0,LH}{R}_{0|\text{LHL}}\right)\right] +\left(1-{ m}_{0,L}\right){R}_{0|\text{L}.}\right]\right\} \end{aligned} $$

(7)

$${R}_{3},{R}_{2|\text{H}.}, {R}_{1|\text{HH}.},{R}_{0|\text{HHH}}\le 3H$$

$${R}_{2|.},{R}_{2|\text{L}},{R}_{1|\text{H}.},{R}_{1|\text{HL}},{R}_{1|\text{LH}},{R}_{0|\text{HH}}{,R}_{0|\text{HHL}},{R}_{0|\text{HLH}},{R}_{0|\text{LHH}}\le 2H+L$$

$${R}_{1|.},{R}_{1|\text{L}},{R}_{0|\text{H}.},{R}_{0|\text{HL}.},{R}_{0|\text{LH}.},{R}_{0|\text{HLL}},{R}_{0|\text{LLH}}{R}_{0|\text{LHL}}, \le H+2L$$

$${R}_{0|.},{R}_{0|\text{L}.},{R}_{0|\text{LL}.},{R}_{0|\text{LLL}}\le 3L$$

where the return ${R}_{N|\vartheta \vartheta \vartheta }$ and the audit probability ${m}_{N,\vartheta \vartheta \vartheta }$ are the choice variables and $H,L,I$ and $c$ are parameters.

The authors identify the solution of this model, that is the solution of the optimization problem, in three distinct cases: the general case in which there is no return pooling, the case in which Condition 2 holds and the case in which Condition 3 holds.

Condition 1, instead, is, by hypothesis, verified in each of these three cases.

Furthermore, the optimal values of the returns of the lower true state ${R}_{0|.},{R}_{0|L.},{R}_{0|LL.},{R}_{0|LLL}$ and of the punishment repayment (${R}_{1|HH.},{R}_{0|HHH},{R}_{1|HL.},{R}_{1|LH.},{R}_{0|HH.}{,R}_{0|HHL},{R}_{0|HLH},{R}_{0|HL.},{R}_{0|LLH},{R}_{0|LHL}$) are common to all three cases (in fact, they are calculated assuming that only Condition 1 holds).

5 Properties of the optimal contract with separate financing

Before presenting the properties of the optimal financing contract in each of the three cases already mentioned, the authors identify the properties of the optimal contract with separate financing of the three investment projects.

Menichini and Simmons (2017) derived properties of the optimal contract with separate financing. They consider the problem of choosing between joint and separate financing of two investment projects and their results are summarized in Proposition 1:

Proposition 1

Given three investment projects, if these are separately financed and only Condition 1 holds, the optimal contract involves:

• (I) Maximum punishment for false low state report: ${R}_{0|\text{H}.}^{sin}=H$;

• (II) Borrower’s zero low state return (three fails): ${R}_{0|\text{L}.}^{sin}={R}_{0|.}^{sin}=L$;

• (III) Random audit probability of low state report (${m}_{0}^{sin}\equiv {m}_{0}$):

$${m}_{0}^{sin}=\frac{I-L}{p\left(H-L\right)-\left(1-p\right)c}<1$$

(8)

• (IV) Repayment obtained by the lender following a high state report equal to:

  $${R}_{1|.}^{sin}= \frac{\left(H-L\right)I-\left(1-p\right)L\left(H-L+c\right)}{p\left(H-L\right)-\left(1-p\right)c}<H$$

• (V) Expected return to the borrower equal to the expected return net of the expected audit cost:

$${E\Pi }_{B}^{sin}=pH+\left(1-p\right)L-I-\frac{I-L}{p\left(H-L\right)-\left(1-p\right)c}\left(1-p\right)c>0$$

(9)

If Condition 1 does not hold, ${m}_{0}^{sin}\ge 1$ and the expected return to the borrower is negative. Thus, financing does not occur.

From (9), may be deduced:

$$3 {m}_{0}^{sin}\left(1-p\right)c=\frac{3(I-L)\left(1-p\right)c}{p\left(H-L\right)-\left(1-p\right)c}<3$$

(10)

and:

$$3 {E\Pi }_{B}^{sin}=3pH+3\left(1-p\right)L-3I-\frac{3(I-L)\left(1-p\right)c}{p\left(H-L\right)-\left(1-p\right)c}>0$$

(11)

that are, respectively, the expected audit cost and the borrower’s expected utility with separate financing of three projects.

6 Optimal returns from the low true state and punishment repayment with joint financing

In this section, the authors expose the optimal values ​​of the returns of the lower true state and of the punishment repayment in the three cases of joint financing.

These optimal values ​​are common to all three cases of joint financing, namely: joint financing without pooling; joint financing with pooling between the returns coming from three successes and from two successes and one fail; joint financing with return pooling from three successes, from two successes and one fail and from one success and two fails.

The first result obtained by the authors is the following:

Proposition 2

Given three investment projects, if they are jointly financed and Condition 1 holds, the optimal contract involves:

• (VI) Punishment repayments set at their maximum values:

  $${R}_{2|\text{H }},{R}_{0|\text{HHH}}=3H$$
  $${R}_{1|\text{HH}.},{R}_{1|\text{HL}.},{R}_{1|\text{LH}.},{R}_{0|\text{HH}.}{,R}_{0|\text{HHL}},{R}_{0|\text{HLH}}=2H+L$$
  $${R}_{0|\text{HL}.},{R}_{0|\text{LLH},}{R}_{0|\text{LHL}}=H+2L$$

• (VII) Lower true state returns set at their maximum values: ${R}_{0|.},{R}_{0|\text{L}.},{R}_{0|\text{LL}.},{R}_{0|\text{LLL}}=3L$

• (VIII) Strictly positive audit probability of low state report: ${m}_{0}>0$

The proof of Proposition 2 is in the Appendix.

7 Properties of the optimal contract with joint financing and no pooling

In this paragraph, the authors show the properties of the optimal financing contract under the assumption that only Condition 1 holds, namely, that there is no return pooling. They obtain the following result, whose proof is in the Appendix:

Proposition 3

Given three investment projects, if these are jointly financed and only Condition 1 holds, the optimal financing contract involves:

• (IX) Random audit of low state report $\widehat{{m}_{0}}{\equiv m}_{0}$:

  $$\widehat{{m}_{0}}=\frac{{D}_{1}}{{D}_{2}}$$

  where:

  $${D}_{1}=3I-{p}^{3}\left(2H+L\right)-\left[3{p}^{2}\left(1-p\right)+3p{\left(1-p\right)}^{2}+{\left(1-p\right)}^{3}\right]3L$$

  and:

  $$\begin{aligned}{D}_{2}&=3{p}^{2}\left(1-p\right)(H-L)(1+\widehat{{m}_{0,L}})+3p{\left(1-p\right)}^{2}\\&\left\{{0,5}\left(H-L\right)[1 +{0,5}\widehat{{m}_{0,L}}(1+\widehat{{m}_{0,LL}})]-c\right\}-{\left(1-p\right)}^{3}\left[1+\widehat{{m}_{0,L}}(1+\widehat{{m}_{0,LL}}\right]c\end{aligned}$$

• (X) Deterministic audit of intermediate state report:$\widehat{{m}_{1}}{\equiv m}_{1}=1; \widehat{{m}_{2}} \equiv {m}_{2}=0$

• (XI) Zero intermediate state returns (one success and two fails and two successes and one fail) for the borrower: $\widehat{{R}_{1|.}}=H+2L; \widehat{{R}_{2|.}}=2H+L$

• (XII) Top state return (three successes) lower than its maximum value: $\widehat{{R}_{3}}=2H+L<3H$

• (XIII) Borrower’s expected utility:

  $$\begin{aligned}\widehat{{E\Pi }_{B}}&={p}^{3}\left(H-L\right)+6{p}^{2}\left(1-p\right)\left(H-L\right)+3p{\left(1-p\right)}^{2}\left(H-L\right)\\ &\quad -\frac{{D}_{1}\left\{(1+\widehat{{m}_{0,L}})+{0,5}[1 +{0,5}\widehat{{m}_{0,L}}(1+\widehat{{m}_{0,LL}}]\right\}}{{D}_{2}}\end{aligned}$$

  where the minimum value of $\widehat{{E\Pi }_{B}}$ is ${p}^{3}\left(H-L\right)$ and it is obtained setting $\widehat{{m}_{0,L}}=\widehat{{m}_{0,L}}=1$, while the maximum value of $\widehat{{E\Pi }_{B}}$ is ${p}^{3}\left(H-L\right)+3{p}^{2}\left(1-p\right)2\left(H-L\right)+3p{\left(1-p\right)}^{2}\left(H-L\right)$ and it is obtained setting $\widehat{{m}_{0,L}}=\widehat{{m}_{0,L}}=0$.

8 Properties of the optimal contract with joint financing and ${{\varvec{P}}{\varvec{F}}}_{1}$

In this paragraph, the authors expose the properties of the optimal contract under the hypothesis that, in addition to Condition 1, also Condition 2 holds, namely that there is pooling between the returns deriving from three successes and from two successes and one fail. They obtain the following result, whose proof is in the Appendix:

Proposition 4

Given three investment projects, if these are jointly financed and both Condition 1 and Condition 2 hold, the optimal financing contract involves:

• (XIV) Random audit probability of low state report ${m}_{0}^{*}\equiv {m}_{0}$:

  $${m}_{0}^{*}=\frac{{D}_{3}}{{D}_{4}}$$

  with:

  $${D}_{3}=-3\left[I-{\left(1-p\right)}^{3}L\right]-6p{\left(1-p\right)}^{2}c+\left[{p}^{3}+3{p}^{2}\left(1-p\right)+3p{\left(1-p\right)}^{2}\right]3L$$

  and:

  $$\begin{aligned} {D}_{4}&=\left[{m}_{0,L}^{*}\left(1+{m}_{0,LL}^{*}\right)+1\right]{\left(1-p\right)}^{3}c-\left[{p}^{3}+3{p}^{2}\left(1-p\right)\right]\left(H-L\right)\left(1+{m}_{0,L}^{*}\right) \\ &\quad -3p{\left(1-p\right)}^{2}\left[1 +{0,5}{m}_{0,L}^{*}\left(1+{m}_{0,LL}^{*}\right) \right]{0,5}\left(H-L\right) \end{aligned} $$

  becoming deterministic when ${PF}_{1}$ is binding.

• (XV) Repayments with one success and two fails and repayment with two successes and one fail, respectively, equals to:

  $${R}_{1|\text{L}.}^{*}=\frac{{D}_{3}\left\{\left[1 +{0,5} {m}_{0,L}^{*}\left(1+{m}_{0,LL}^{*}\right)\right]{0,5}\left(H-L\right)+3L\right\}}{{D}_{4}}$$

  and:

  $${R}_{2|.}^{*}=3L+\frac{\left(H-L\right)\left(1+{m}_{0,L}\right){D}_{3}}{{D}_{4}}$$

  where ${R}_{1|\text{L}.}^{*}=H+2L$ and ${R}_{2|.}^{*}=2H+L$ when the audit is deterministic ${(m}_{0,LL}^{*}={m}_{0,L}^{*}=1)$ and ${PF}_{1}$ is binding, while ${R}_{1|\text{L}.}^{*}<H+2L$ and ${R}_{2|.}^{*}<2H+L$ in all the other cases.

• (XVI) Borrower’s expected utility:

  $$ \begin{aligned} E\Pi _{B}^{*} & = p^{3} 3H + 3p^{2} \left( {1 - p} \right)\left( {2H + L} \right) + 3p\left( {1- p} \right)^{2} \left( {H + 2L} \right) \\ &-D_{3}- \frac{[p^{3} + 3p^{2} ( {1 - p})] ( {H-L})(1 + m_{{0,L}}^{*})+3p(1-p)^{2}\{[ {1 + 0,5m_{{0,L}}^{*} ({1 +m_{{0,LL}}^{*} } )} ] 0,5( {H - L}) + 3L \}}{D_{4} }\\ &\quad -[ {p^{3} + 3p^{2} ( {1 - p} )} ] 3L \end{aligned} $$

  More precisely, it is equal to its minimum ${p}^{3}(H-L)$ when the audit is deterministic $({m}_{0,LL}^{*}={m}_{0,L}^{*}=1)$ and ${PF}_{1}$ is binding, while it is higher than this value in all the other cases.

9 Properties of the optimal contract with joint financing and ${{\varvec{P}}{\varvec{F}}}_{2}$

Finally, in this paragraph, the authors expose the properties of the optimal financing contract under the hypothesis that, in addition to Condition 1, also Condition 3 holds, namely that there is return pooling from three successes, from two successes and one fail and from one success and two fails. They obtain the following result, whose proof is in the Appendix:

Proposition 5

Given three investment projects, if these are jointly financed and both Condition 1 and Condition 3 hold, the optimal financing contract involves:

• (XVII) Random audit probability of low state report $\widetilde{{m}_{0}}\equiv {m}_{0}$:

  $$\widetilde{{m}_{0}}=\frac{3\left(I-L\right)}{{D}_{5}}$$

  with:

  $$\begin{aligned}{D}_{5}&=\left[{p}^{3}+3p(1-p)\right]{0,5}\left(H-L\right)+\widetilde{{m}_{0,L}}\left(1+\widetilde{{m}_{0,LL}}\right)\\ & \quad\times \left\{\left[{p}^{3}+3p(1-p)\right]{0,25}\left(H-L\right)-{\left(1-p\right)}^{3}c\right\}-{\left(1-p\right)}^{3}c\end{aligned}$$

  becoming deterministic (equal to 1) when ${PF}_{2}$ is binding.

• (XVIII) Repayment in the top state (three successes) and in the intermediate state (both two successes and one fail and one success and two fails) equal to:

  $$\widetilde{{R}_{1|\text{LL}}}=\frac{3\left[I-L{\left(1-p\right)}^{3}\right]}{{p}^{3}+3p(1-p)}+\frac{3\left(I-L\right){\left(1-p\right)}^{3}\left[{p}^{3}+3p(1-p)\right]\left[\widetilde{{m}_{0,L}}\left(1+\widetilde{{m}_{0,LL}}\right)+1\right]c}{{D}_{5}}$$

  where $\widetilde{{R}_{1|\text{LL}}}$ is equal to $H+2L$ when the audit is deterministic ($\widetilde{{m}_{0,L}}=\widetilde{{m}_{0,LL}}=1$) and ${PF}_{2}$ is binding, while it is lower than this value in all the other cases.

• (XIX) Borrower’s expected utility equal to:

  $$\begin{aligned} {\widetilde{E\Pi }}_{B}&={p}^{3}3H+3{p}^{2}\left(1-p\right)\left(2H+L\right)+3p{\left(1-p\right)}^{2}\left(H+2L\right)-3\left[I-L{\left(1-p\right)}^{3}\right]\\ &\quad -\frac{3\left(I-L\right){\left(1-p\right)}^{3}\left[\widetilde{{m}_{0,L}}\left(1+\widetilde{{m}_{0,LL}}\right)+1\right]c}{{D}_{5}} \end{aligned} $$

  with:

  $$\begin{aligned}{\widetilde{E\Pi }}_{B}&={p}^{3}3H+3{p}^{2}\left(1-p\right)\left(2H+L\right)+3p{\left(1-p\right)}^{2}\left(H+2L\right)\\& -3\left[I-L{\left(1-p\right)}^{3}+{\left(1-p\right)}^{3}c\right] \end{aligned}$$

  when the audit is deterministic $(\widetilde{{m}_{0,L}}=\widetilde{{m}_{0,LL}}=1)$ and ${PF}_{2}$ is binding.

10 Comparison between separate financing and joint financing

In this section, the authors compare separate financing both with joint financing with $P{F}_{1}$ and joint financing with $P{F}_{2}$ with the aim of identifying the most efficient financing method between them.

In order to prove and graphically represent the results provided in this Section in the quickest and most intelligible fashion, the authors define three new expressions.

The first one consists in the Individual Feasibility condition for the three investment projects ($3IF$) net of investment cost ($3I$):

$$3 I{F}^{\mho }=3pH+ 3\left(1-p\right)L-3\left(1-p\right)c$$

that is a linear function of $c$ whose vertical intercept and slope are, respectively, $3 I{F}^{0}=3pH+ 3\left(1-p\right)L>0$ and $-3\left(1-p\right)c<0$.

The second one is the first pooling feasibility condition $({PF}_{1})$ net of the investment cost:

$$\begin{aligned}{PF}_{1}^{\mho }&=\left[{p}^{3}+3{p}^{2}\left(1-p\right)\right]\left(2H+L\right)+3p{\left(1-p\right)}^{2}\left(H+2L\right)\\ & \quad +{\left(1-p\right)}^{3}3L-3{\left(1-p\right)}^{3}c-6p{\left(1-p\right)}^{2}c\end{aligned}$$

that is a linear function of $c$ whose vertical intercept and slope are, respectively, $P{F}_{1}^{0}=\left[{p}^{3}+3{p}^{2}\left(1-p\right)\right]\left(2H+L\right)+3p{\left(1-p\right)}^{2}\left(H+2L\right)+{\left(1-p\right)}^{3}3L>0$ and $-3{\left(1-p\right)}^{3}c-6p{\left(1-p\right)}^{2}c<0$.

The third one, instead, is the second pooling feasibility condition $({PF}_{2})$ net of the investment cost:

$${PF}_{2}^{\mho }=\left[{p}^{3}+3{p}^{2}\left(1-p\right)+3p{\left(1-p\right)}^{2}\right]\left(H+2L\right)+{\left(1-p\right)}^{3}3L-3{\left(1-p\right)}^{3}c$$

that is a linear function of $c$ whose vertical intercept and slope are, respectively, $P{F}_{2}^{0}=\left[{p}^{3}+3{p}^{2}\left(1-p\right)+3p{\left(1-p\right)}^{2}\right]\left(H+2L\right)+{\left(1-p\right)}^{3}3L$ and $-3{\left(1-p\right)}^{3}c<0$.

10.1 Comparison between separate financing and joint financing with ${\varvec{P}}{{\varvec{F}}}_{1}$

In this subsection, the authors carry out the first comparison, that is, the one between separate financing and joint financing with return pooling from three successes and from two successes and one fail ($P{F}_{1}$). They obtain the following result, whose proof is in the Appendix:

Proposition 6

The joint financing of three investment projects with return pooling from three successes and from two successes and one fail implies:

• (XX) A higher expected audit cost than separate financing:

  $$3 {m}_{0}^{sin}\left(1-p\right)c<{m}_{0}^{*}\left[3{\left(1-p\right)}^{3}+6p{\left(1-p\right)}^{2}\right]c$$

• (XXI) A smaller credit rationing region than separate financing, if the investment cost is low enough as in Fig. 2 below:

Fig. 2

Comparison between joint financing with PF₁ and separate financing with low investment cost

Figure 2 indicates that up to point $A$, the expected profit net of the expected audit plus investment cost is such that the three projects may be financed separately, or, alternatively, they may be financed jointly without pooling between the high and intermediate states. Between points $A$ and $B$, the three projects may be financed separately, or, alternatively, jointly by pooling the returns of the high and intermediate states. Between points $B$ and $C$, the three projects may only be financed through joint financing with pooling between the high and intermediate states. Beyond point $C$ the three projects are not viable. Figure 2 shows also that the credit rationing area associated with separate financing is larger than that associated with joint financing.

• (XXII) A larger credit rationing region than separate financing, if the investment cost is high enough as in Fig. 3 below:

Fig. 3

Comparison between joint financing with PF₁ and separate financing with high investment cost

Figure 3 shows that up to point $D$, the three projects may be financed separately, or, alternatively, jointly and without pooling between the high and intermediate states. Between points $D$ and $E$, the three projects may only be financed separately. Beyond point $E$, the three projects are not viable. When $3I$ is between $3 I{F}^{0}$ and $P{F}_{1}^{0}$, the three projects are viable only through separate financing of all projects (look at the $3 I{F}^{^{\prime}}$ line). Finally, Fig. 3 shows that the credit rationing area associated with joint financing is larger than that associated with separate financing.

10.2 Comparison between separate financing and joint financing with ${\varvec{P}}{{\varvec{F}}}_{2}$

In this subsection, the authors make the second comparison, namely that one between separate financing and joint financing with return pooling from three successes, from two successes and one fail and from one successes and two fails. They obtain the following result, whose proof is in the Appendix

Proposition 7

The joint financing of the three investment projects with return pooling from three successes, from two successes and one fail and from two fails and one success, implies:

Fig. 4

Comparison between joint financing with PF₂ and separate financing with low investment cost

• (XXIII) A lower expected audit cost than separate financing:

  $$3 {m}_{0}^{sin}\left(1-p\right)c>\widetilde{{m}_{0}}{\left(1-p\right)}^{3}c$$

• (XXIV) A smaller credit rationing region than separate financing, if the investment cost is low enough as in Fig. 4 below:

Figure 4 indicates that up to point $F$, the expected profit net of the expected audit plus investment cost is such that the three projects may be financed separately, or, alternatively, they may be financed jointly without pooling between the intermediate and low states. Between points $F$ and $G$, the three projects may be financed separately, or, alternatively, jointly by pooling the returns of the intermediate and low states. Between points $G$ and $H$, the three projects may only be financed through joint financing with pooling between the intermediate and low states. Beyond point $H$, the three projects are not viable. Figure 4 shows also that the credit rationing area associated with separate financing is larger than that associated with joint financing.

• (XXV) A larger credit rationing region than separate financing, if the investment cost is high enough as in Fig. 5 below:

Fig. 5

Comparison between joint financing with PF₂ and separate financing with high investment cost

Figure 5 shows that up to point $K$, the three projects may be financed separately, or, alternatively, jointly and without pooling between the intermediate and low states. Between points $K$ and $L$, the three projects may only be financed separately. Beyond point $L$, the three projects are not viable. When $3I$ is between $3 I{F}^{0}$ and $P{F}_{2}^{0}$, the three projects are viable only through separate financing of all projects (look at the $3 I{F}^{^{\prime}}$ line). Finally, Fig. 5 shows that the credit rationing area associated with joint financing is larger than that associated with separate financing.

11 Conclusions

This manuscript identifies the properties of the optimal financing contract for three investment projects with uncorrelated returns in four distinct cases: separate financing; joint financing without return pooling; joint financing with pooling between high and intermediate states; joint financing with return pooling between intermediate and low states.

The obtained findings indicate that in the three cases of joint financing:

• The like-debt contract is optimal;

• The maximum punishment of the borrower is optimal;

• Auditing at least the first investment project when the borrower reports three fails is optimal;

• The optimal combination between stochastic and deterministic audit depends on the assumptions about the return pooling.

The results presented here are similar to those of Menichini and Simmons for the joint financing of two investment projects (2017). However, this manuscript provides criteria for when joint financing is more efficient than separate financing (and vice versa). More specifically, it highlights that joint financing with pooling between high and intermediate states (namely return pooling from three successes, two successes and one fail and from one success and two fails), is more efficient than separate financing. This is because it involves lower expected audit cost and, if investment cost is not too high, less credit rationing. Furthermore, it emphasises that joint financing with pooling between intermediate and low states (namely, joint financing with return pooling from three successes and two successes and one fail) is never more efficient than separate financing, because it implies a higher expected audit cost and, if investment cost is very high, more credit rationing. This result differs from joint financing of two projects in Menichini and Simmons (2017), where they argue that joint financing is always more efficient than separate financing, due to the fact that the lender reduces audit frequency in the intermediate state (one success and one fail) and increases it in the low state (two fails). In other words, the main innovation of this paper is proving precisely that, when there are three projects to finance, joint financing is not always more efficient than separate financing.

Appendices

Appendix

Proof of Proposition 2

2.1 Maximum punishment for false report

Note that punishment repayments are found only on the second member of the incentive constraints (namely in the constraints (2), (3), (4), (5), (6) and (7)) and are completely absent both in the objective function and in the participation constraint (Eq. (1)).

Therefore, it is possible to increase the generic punishment repayment by simultaneously reducing the relative audit probabilities in each of the incentive constraints. In this way, the generic constraint becomes slacker, and it is possible to reduce ${R}_{3}$ leaving $PC$ and $E{\Pi }_{B}$ unchanged.

More precisely, the authors proceed as follows:

(i) Increase ${R}_{0|\text{HL}.}$ and reduce ${m}_{0}$ while keeping ${m}_{0}{m}_{0,H}\left(1-{m}_{0,HL}\right){R}_{0|\text{HL}.}$ constant in (6) and (7). Doing so, these constraints become slacker because the term $\left(1-{m}_{0}\right){R}_{0|.}$ increases. So, the expected audit cost ${m}_{0}c$ and ${R}_{3}$ may be reduced. Then, set ${R}_{0|\text{HL}.}=H+2L.$ The second members of the (6) and (7) are increasing in ${m}_{0,H}{m}_{0,HL}$, but since this term is absent in the objective function and in the participation constraint, it may be set equal to 1 to slacken the (6) and (7) as much as possible.

A similar argument may be applied to ${R}_{0|LLH}$ and to the changes in ${m}_{0}$ making ${m}_{0}{m}_{0,L}{m}_{0,LL}{R}_{0|LLH}$ constant in the (7). Even in this case, it si possible to set ${R}_{0|LLH}=H+2L$ to slacken the (7). However, here it is impossible to set ${m}_{0,L}{m}_{0,LL}=1$ because this term appears both in the objective function and in the participation constraint. Again, in the (7) it is possible to increase ${R}_{0|LHL}$ and reduce ${m}_{0}$ while keeping ${m}_{0}{m}_{0,L}{m}_{0,LH}{R}_{0|LHL}$ constant and, at the same time, raise $\left(1-{m}_{0}\right){R}_{0|.}$. Doing so allows the reduction of the expected audit cost ${m}_{0}c$ in the (1) and, consequently, the decrease in ${\text{R}}_{3}$. So, set ${R}_{0|LHL.}=H+2L$. The second member of the (7) is increasing in ${m}_{0,LH}$ and, since this term appears neither in the objective function nor in the participation constaint, it may be set to 1 to slacken the (7).

(ii) Increase ${R}_{1|HL}$ and reduce at the same time ${m}_{1}$ while keeping ${m}_{1}{m}_{1,H}{R}_{1|HL}$ constant in the (5). Doing so slackens the same constraint because of the growth of the term $\left(1-{m}_{1}\right){R}_{1|.}$ allowing also to reduce the expected audit cost ${m}_{1}c$ and ${R}_{3}$ into the (1). So, set ${R}_{1|HL.}=2H+L.$ The second member of the (5) is increasing in ${m}_{1,H}$, but since this term does appear neither in the objective function nor in the participation constraint, it may be set equal to 1 to slacken the (5) as much as possible.

A similar argument may be applied to ${R}_{1|LH}$ and to the changes of ${m}_{1}$ while keeping ${m}_{1}{m}_{1,L}{R}_{1,LH}$ constant in the (5). Again, set ${R}_{1|LH}=2H+L$ to slacken the (5). However, in this case, it is impossible to set ${m}_{1,L}=1$ because this term appears both in the objective function and in the participation constaint. In any case, in the (1) the reduction of ${m}_{1}$ allows to decrease ${R}_{3}$.

It is also possible to raise ${R}_{1|H.}$ and reduce at the same time ${m}_{1}$ keeping ${m}_{1}\left(1-{m}_{1,H}\right){R}_{1|H.}$ constant into the (3) and allow to reduce ${R}_{3}$ in the (1) thanks to a decrease of ${m}_{1}c$. Set ${R}_{1|H.}=2H+L$ and ${m}_{1,H}=1$ to slacken the (3) as much as possible. Doing so is possible because of the absence of ${m}_{1,H}$ both in the objective function and in the participation constraint.

(iii) Increase ${R}_{0|\text{HH}.}$ reducing at the same time ${m}_{0}$ and keeping ${m}_{0}{m}_{0,H}\left(1-{m}_{0,HH}\right){R}_{0|\text{ HH}.}$ constant in the (4) and (6). Doing so raises $\left(1-{m}_{0}\right){R}_{0|.}$ and then slackens these constraints. In the (1), then, it is possible to decrease ${m}_{0}c$ and, consequently, also ${R}_{3}$. So, set ${R}_{0|\text{HH}.}=2H+L.$ The second members of the (4) and (6) are increasing in ${m}_{0,H}{m}_{0,HH}$ but, since these terms appears neither in the objective function nor in the participation constraint, they may be set equal to 1 to slacken the (4) and (6) as much as possible. Moreover, the reduction of ${m}_{0}$ in the (1) allows to reduce ${R}_{3}$ in the same constaint.

(iv) Increase ${R}_{0|\text{HHL}}$ and reduce ${m}_{0}$ while keeping ${m}_{0}{m}_{0,H}{m}_{0,HH}{R}_{0|\text{HHL}}$ constant in the (6). Doing so slackens the same constaint because of the growth of $\left(1-{m}_{0}\right){R}_{0|.}$ and lower ${m}_{0}c$ in the (1), allowing to reduce ${R}_{3}$ in the same expression. So, set ${R}_{0|\text{HHL}}=2H+L$. The second member of the (6) is increasing in ${m}_{0,H}{m}_{0,HH}$, but, since this term appears neither in the objective function and in the participation constraint, it may be set equal to 1 to slacken the (6) as much as possible.

Decreaing ${m}_{0}$ into the (1) allows to reduce ${R}_{3}$.

Again, raise ${R}_{0|\text{LHL}}$ and reduce ${m}_{0}$ while keeping ${m}_{0}{m}_{0,L}{ m}_{0,LH}{R}_{0|\text{LHH}}$ constant in the (6). Doing so slackens the second member of this constraint because of the reduction of $\left(1-{m}_{0}\right){R}_{0|.}$ and decreases ${m}_{0}c$ in the (1), allowing a reduction of ${R}_{3}$. So, set ${R}_{0|\text{LHH}}=2H+L$. Note also that ${m}_{0,LH}$ appears neither in the objective function and in the participation constraint and then this term may be set equal to 1 to slacken the (6) as much as possible.

(v) Increase ${R}_{1|\text{HH}.}$ and reduce ${m}_{0}$ while keeping ${m}_{1}{m}_{1,H}{R}_{1|\text{HH}}$ constant in the (3). Doing so slackens the same constraint because of the growth of $\left(1-{m}_{1}\right){R}_{1|.}$ and decreases ${m}_{1}c$ allowing a reduction of ${R}_{3}$ in the (1). So, set ${R}_{1|\text{HH}.}=2H+L.$ The second member of the (3) is increasing in ${m}_{1,H}$ and, since this term appears neither in the objective function nor in the participation constraint, it may be set equal to 1 to slacken the (3) as much as possible.

(vi) Increase ${R}_{0|\text{H}.}$ and reduce ${m}_{0}$ while keeping ${m}_{0}\left(1-{m}_{0,H}\right){R}_{0|\text{H}.}$ constant in the (4) and (6). Doing so slackens these constraints because of the growth of the term $\left(1-{m}_{0}\right){R}_{0|.}$ and allows a reduction of ${m}_{0}c$ in the (1) and, consequently, also of ${R}_{3}$. Then, set ${R}_{0|\text{H}.}=H+2L.$ The second members of the (4) and (6) are increasing in ${m}_{0,H}$ and, since this term is absent both in the participation constraint and in the incentive constraint, it may be set equal to 1 to slacken the (4) and (6) as much as possible.

(vii) Increase ${R}_{0|HHH}$ and reduce ${m}_{0}$ while keeping ${m}_{0}{m}_{0,H}{m}_{0,HH}{R}_{0|\text{ HHH}}$ constant in the (6). Doing so slackens the same constraint because of the growth of the term $\left(1-{m}_{0}\right){R}_{0|.}$ and lower ${m}_{0}c$ in the (1) allowing, therefore, a reduction of ${R}_{3}$. So, set ${R}_{0|\text{HHH}}=3H.$ The second member of the (6) is increasing in ${m}_{0,H}{m}_{0,HH}$ and, since it appears neither in the objective function nor in the participation constraint, this term may be set equal to 1 to slacken the (6) as much as possible.

A similar argument holds for ${R}_{2|\text{H}.}$. Increase this term and reduce at the same time ${m}_{2}$ while keeping ${m}_{2}{R}_{2|\text{H}}$ constant in the (2). Doing so slackens the same constraint because of the growth of $\left(1-{m}_{2}\right){R}_{2|.}$, lowers ${m}_{2}c$ and, consequently, allows also to reduce ${R}_{3}$ in the same constraint. Then, set ${R}_{2|\text{H }}=3H$. However, it is impossible to set ${m}_{2}=1$, because this term appears both in the objective function and in the participation constraint.

Following all of these modifications, the participation constraint becomes binding, as the punishment repayments are set at their maximum values, and the corresponding expected audit cost and repayment ${R}_{3}$ have been reduced as much as possible.

So, the initial optimization problem of the borrower’s expected utility becomes:

$$\begin{aligned} {}&\text{max} {p}^{3}\left(3H-{R}_{3}\right)+3{p}^{2}\left(1-p\right)\left[2H+L-{m}_{2}{R}_{2|\text{L}. }-\left(1-{m}_{2}\right){R}_{2|. }\right]\\ &\quad +3p{\left(1-p\right)}^{2}\left\{2L+H-{m}_{1}\left[{m}_{1,L}{R}_{1|\text{ LL}.}+\left(1-{m}_{1,L}\right){R}_{1|\text{L}. }\right]-\left(1-{m}_{1}\right){R}_{1|. }\right\}\\ &\quad +{\left(1-p\right)}^{3}\left\{3L-{m}_{0}\left[{m}_{0,L}\left({m}_{0,LL}{R}_{0|\text{LLL}}+\left(1-{m}_{0,LL}\right){R}_{0|\text{LL}.}\right)\right.\right.\\ &\quad+\left.\left.\left(1-{m}_{0,L}\right){R}_{0|\text{L}.}\right]-\left(1-{m}_{0}\right){R}_{0|.}\right\} \end{aligned} $$

subject to:

$$\begin{aligned}{p}^{3}{R}_{3}&+3{p}^{2}\left(1-p\right)\left\{{m}_{2}\left({R}_{2|\text{L }}-c\right)+\left(1-{m}_{2}\right){R}_{2|. }\right\}\\ &+3p{\left(1-p\right)}^{2}\left\{{m}_{1}\left[{m}_{1,L}\left({R}_{1|\text{LL}}-c\right)+\left(1-{m}_{1,L}\right){R}_{1|\text{L}.}-c\right]+\left(1-{m}_{1}\right){R}_{1|.}\right\}\\ &+{\left(1-p\right)}^{3}\left\{{m}_{0}\left[{m}_{0,L}\left({m}_{0,LL}\left({R}_{0|\text{LLL}}-c\right)+\left(1-{m}_{0,LL}\right){R}_{0|\text{LL}.}-c\right)\right.\right. \\ &+\left.\left.\left(1-{m}_{0,L}\right){R}_{0|\text{L}.}-c\right]+\left(1-{m}_{0}\right){R}_{0|.}\right\}=3I \end{aligned}$$

(12)

$${R}_{3}\le \left(1-{m}_{2}\right){R}_{2|. }+{m}_{2}3H$$

(13)

$${R}_{3}\le \left(1-{m}_{1}\right){R}_{1|. }+{m}_{1}\left(2H+L\right)$$

(14)

$${R}_{3}\le \left(1-{m}_{0}\right){R}_{0|. }+{m}_{0}3H$$

(15)

$$\begin{aligned}\left(1-{m}_{2}\right){R}_{2|.}&+{m}_{2}{R}_{2|\text{L}}\le \left(1-{m}_{1}\right){R}_{1|.}\\&+{m}_{1}\left\{{0,5} \left(2H+L\right)+{0,5} \left[{m}_{1,L}\left(2H+L\right)+\left(1-{m}_{1,L}\right){R}_{1|\text{L}.}\right]\right\}\end{aligned}$$

(16)

$$\begin{aligned}\left(1-{m}_{2}\right){R}_{2|.}&+{m}_{2}{R}_{2|\text{L}}\le \left(1-{m}_{0}\right){R}_{0|.}\\&+{m}_{0}\left\{{0,5}(2H+L)+{0,5}\left[{m}_{0,L}(2H+L)+\left(1-{m}_{0,L}\right){R}_{0|\text{L}.}\right]\right\}\end{aligned}$$

(17)

$$\begin{aligned} \left(1-{m}_{1}\right){R}_{1|.}&+{m}_{1}\left[\left(1-{m}_{1,L}\right){R}_{1|\text{L}.}+{m}_{1,L}{R}_{1|\text{LL}.}\right]\le \left(1-{m}_{0}\right){R}_{0|.}\\ &+{m}_{0}\left\{{0,5}\left(H+2L\right) +{0,5}\left[{ m}_{0,L}\left[{0,5}\left(\left(1-{m}_{0,LL}\right){R}_{0|LL.}\right.\right.\right.\right.\\ &\left.\left.\left.\left.+{m}_{0,LL}\left(H+2L\right)\right)+{0,5}\left(H+2L\right)\right]+\left(1-{ m}_{0,L}\right){R}_{0|\text{L}.}\right]\right\} \end{aligned}$$

(18)

2.2 Lower true state returns

Since ${R}_{0|\text{L}.}<3L$, then it is possible to reduce ${R}_{3}$ and increase ${R}_{0|\text{L}.}$ keeping ${p}^{3}{R}_{3}+{\left(1-p\right)}^{3}{m}_{0}\left(1-{m}_{0,L}\right){R}_{0|\text{L}.}$ into the (12) constant until ${R}_{0|\text{L}.}=3L$. Doing so, the (13), (14), (15) and (18) become slacker, allowing to decrease ${m}_{0}$, while the objective function and the participation constraint remain unchanged.

Replicating the same argument for ${R}_{0|.}$ and ${R}_{0|\text{LL}.}$ it results ${R}_{0|.}={R}_{0|\text{L}.}={R}_{0|\text{LL}.}=3L$. The return ${R}_{0|\text{LLL}}$ only appears in the objective function and in the participation constraint. In the (12), by replacing ${R}_{0|.}={R}_{0|\text{L}.}={R}_{0|\text{LL}.}=3L$, by lowering ${R}_{3}$ and by increasing ${R}_{0|\text{LLL}}$ keeping, at the same time, ${p}^{3}{R}_{3}+{\left(1-p\right)}^{3}{m}_{0}{m}_{0,L}{m}_{0,LL}{R}_{0|\text{LLL}}$ constant, the (13), (14) and (15) become more slack and the objective function and the participation constraint remain unchanged. Then, also ${R}_{0|\text{LLL}}=3L$.

Moreover, it ever holds ${R}_{3}>3L>0$ because, if ${R}_{3}\le 3L$, the return of the three investment projects would be ever insufficient to repay the investment cost.

The new reduced form of the problem becomes:

$$\begin{aligned}& \text{max} {p}^{3}\left(3H-{R}_{3}\right)+3{p}^{2}\left(1-p\right)\left[2H+L-{m}_{2}{R}_{2|\text{L}. }-\left(1-{m}_{2}\right){R}_{2|. }\right]+3p{\left(1-p\right)}^{2}\\&\quad \times\left\{2L+H-{m}_{1}\left[{m}_{1,L}{R}_{1|\text{ LL}.}+\left(1-{m}_{1,L}\right){R}_{1|\text{L}. }\right]-\left(1-{m}_{1}\right){R}_{1|.}\right\}\end{aligned}$$

subject to:

$$\begin{aligned}{p}^{3}{R}_{3}&+3{p}^{2}\left(1-p\right)\left\{{m}_{2}\left({R}_{2|\text{L }}-c\right)+\left(1-{m}_{2}\right){R}_{2|. }\right\}\\&+3p{\left(1-p\right)}^{2}\left\{{m}_{1}\left[{m}_{1,L}\left({R}_{1|\text{LL}}-c\right)+\left(1-{m}_{1,L}\right){R}_{1|\text{L}.}-c\right]+\left(1-{m}_{1}\right){R}_{1|.}\right\}\\&+{\left(1-p\right)}^{3}\left\{3L-{m}_{0}{m}_{0,L}\left(1+{m}_{0,LL}\right)c-{m}_{0}c\right\}=3I\end{aligned}$$

(19)

$${R}_{3}\le \left(1-{m}_{2}\right){R}_{2|. }+{m}_{2}3H$$

(20)

$${R}_{3}\le \left(1-{m}_{1}\right){R}_{1|. }+{m}_{1}\left(2H+L\right)$$

(21)

$${R}_{3}\le \left(1-{m}_{0}\right)3L+{m}_{0}3H$$

(22)

$$\begin{aligned}\left(1-{m}_{2}\right){R}_{2|.}&+{m}_{2}{R}_{2|\text{L}}\le \left(1-{m}_{1}\right){R}_{1|.}\\&+{m}_{1}\left\{{0,5} \left(2H+L\right)+{0,5} \left[{m}_{1,L}\left(2H+L\right)+\left(1-{m}_{1,L}\right){R}_{1|\text{L}.}\right]\right\}\end{aligned}$$

(23)

$$\begin{aligned} \left(1-{m}_{2}\right){R}_{2|.}&+{m}_{2}{R}_{2|\text{L}}\le \left(1-{m}_{0}\right)3L\\ &+{m}_{0}\left\{{0,5}(2H+L)+{0,5}\left[{m}_{0,L}(2H+L)+\left(1-{m}_{0,L}\right)3L\right]\right\} \end{aligned} $$

(24)

$$\begin{aligned}\left(1-{m}_{1}\right){R}_{1|.}&+{m}_{1}\left[\left(1-{m}_{1,L}\right){R}_{1|\text{L}.}+{m}_{1,L}{R}_{1|\text{LL}.}\right]\\ &\le \left(1-{m}_{0}\right)3L+{m}_{0}\left\{{0,5}\left(H+2L\right) +{0,5}\left[{ m}_{0,L}\right.\right.\\ &\left[{0,5}\left(\left(1-{m}_{0,LL}\right)3L+{m}_{0,LL}\left(H+2L\right)\right)\right.\\ &\left.\left.\left.+\,{0,5}\left(H+2L\right)\right]+\left(1-{ m}_{0,L}\right)3L\right]\right\} \end{aligned}$$

(25)

${{\varvec{m}}}_{0}>0$

If it were ${m}_{0}=0$, from (22), from (24) and from (25) one would deduce ${R}_{3}$, ${R}_{2|.}$, ${R}_{2|\text{L}}$, ${R}_{1|.}$, ${R}_{1|\text{L}.}$, ${R}_{1|\text{LL}.}\le 3L$ and the participation constraint (19) would no longer hold, because it would result:

$$3L-3{p}^{2}\left(1-p\right){m}_{2}c-3p{\left(1-p\right)}^{2}{m}_{1}c\left({m}_{1,L}c-1\right)<3I$$

So, it must necessarily hold ${m}_{0}>0$.

Also note that (24) and (25) must necessarily be binding. Otherwise, it would be possible to further reduce ${m}_{0}$ by slackening the participation constraint and allowing an additional reduction of ${R}_{3}$.

Proof of Proposition 3

3.1 Optimal values of $\widehat{{R_{3} }} \equiv R_{3}$,$\widehat{{R_{2|.} }} \equiv R_{2|.} ,\widehat{{R_{1|.} }} \equiv R_{1|.}$, $\widehat{{m_{2} }} \equiv m_{2} ,$ $\widehat{{m_{1} }} \equiv m_{1}$ $e$ $\widehat{{m_{1,L} }} \equiv m_{1,L}$

From the Eqs. (24) and (25) are obtained, respectively:

$$\left(1-{m}_{2}\right){R}_{2|.}+{m}_{2}{R}_{2|\text{L}}= 3L+(H-L){m}_{0}(1+{m}_{0,L})$$

and:

$$\begin{aligned}\left(1-{m}_{1}\right){R}_{1|.}&+{m}_{1}\left[\left(1-{m}_{1,L}\right){R}_{1|\text{L}.}+{m}_{1,L}{R}_{1|\text{LL}.}\right]\\ &=3L+{0,5} \left(H-L\right) {m}_{0}[1 +{0,5}{ m}_{0,L}(1+{m}_{0,LL})]\end{aligned}$$

Since the authors have just deduced the value of $\left(1-{m}_{2}\right){R}_{2|.}+{m}_{2}{R}_{2|L}$ from the constraint (24), the constraint (23) becomes negligible.

By replacing the two expressions above into the objective function and the participation constraint (19), the optimization problem becomes:

$$\begin{aligned}\text{max} {p}^{3}\left(3H-{R}_{3}\right)&+3{p}^{2}\left(1-p\right)(H-L)\left[2-{m}_{0}\left(1+{m}_{0,L}\right)\right]\\&+3p{\left(1-p\right)}^{2}(H-L)\left\{1-{m}_{0}[1 +{0,5}{ m}_{0,L}(1+{m}_{0,LL})]\right\}\end{aligned}$$

subject to:

$$\begin{aligned} {p}^{3}{R}_{3}&+3{p}^{2}\left(1-p\right)\left\{3L+(H-L){m}_{0}(1+{m}_{0,L})-{m}_{2}c\right\}\\ &+3p{\left(1-p\right)}^{2}\left\{3L+{0,5}\left(H-L\right){m}_{0}[1 +{0,5}{ m}_{0,L}(1+{m}_{0,LL})]-{m}_{1}\left(1+{m}_{1,L}\right)c\right\}\\ &+{\left(1-p\right)}^{3}\left\{3L-{m}_{0}\left[1+{m}_{0,L}\left(1+{m}_{0,LL}\right)\right]c\right\}=3I \end{aligned}$$

(26)

$${R}_{3}\le \left(1-{m}_{2}\right){R}_{2|. }+{m}_{2}3H$$

(27)

$${R}_{3}\le \left(1-{m}_{1}\right){R}_{1|. }+{m}_{1}\left(2H+L\right)$$

(28)

$${R}_{3}\le \left(1-{m}_{0}\right)3L+{m}_{0}3H$$

(29)

Note that it is possible to slacken the (27) and (28) leaving the constraints (26) and (29) and the borrower's expected utility unchanged by setting ${R}_{2|.}$ and ${R}_{1}$ to their corresponding maximum values:

$$\widehat{{R}_{2|. }}=2H+L$$

and:

$$\widehat{{R}_{1}}=H+2L$$

Note also that the borrower’s expected utility may be increased complying with the constraints (26), (27), (28) and (29) only by simultaneously reducing ${R}_{3}$, ${m}_{2}$, ${m}_{1}$ e ${m}_{1,L}$.

More precisely, these four values may be reduced until (27) and (28) become binding and ${m}_{1,L}$ goes to zero ($\widehat{{m}_{1,L}}=0$).

From the binding (27):

$${R}_{3}=\left(1-{m}_{2}\right){R}_{2|. }+{m}_{2}3H$$

and from the binding (28):

$${R}_{3}=\left(1-{m}_{1}\right){R}_{1|. }+{m}_{1}\left(2H+L\right)$$

it is deduced:

$${R}_{3}=\left(1-{m}_{2}\right)(2H+L)+{m}_{2}3H=\left(1-{m}_{1}\right)(H+2L)+{m}_{1}\left(2H+L\right)$$

The values of ${m}_{1}$ and ${m}_{2}$ solving this equation are $\widehat{{m}_{1}}=1$ and $\widehat{{m}_{2}}=0$ and they imply $\widehat{{R}_{3}}=2H+L$.

3.2 Optimal value of ${\widehat{{{\varvec{m}}}_{0}}\equiv {\varvec{m}}}_{0}$ and borrower’s expected utility optimal value

By replacing $\widehat{{R}_{3}}=2H+L$ in the (26) it is obtained:

$$\widehat{{m}_{0}}=\frac{{D}_{1}}{{D}_{2}}$$

with:

$${D}_{1}=3I-{p}^{3}\left(2H+L\right)-\left[3{p}^{2}\left(1-p\right)+3p{\left(1-p\right)}^{2}+{\left(1-p\right)}^{3}\right]3L$$

and:

$$\begin{aligned}{D}_{2}&=3{p}^{2}\left(1-p\right)(H-L)(1+\widehat{{m}_{0,L}})+3p{\left(1-p\right)}^{2}\\&\quad\times\left\{{0,5}\left(H-L\right)[1 +{0,5}\widehat{{m}_{0,L}}(1+\widehat{{m}_{0,LL}})]-c\right\}-{\left(1-p\right)}^{3}\left[1+\widehat{{m}_{0,L}}(1+\widehat{{m}_{0,LL}}\right]c\end{aligned}$$

and by replacing $\widehat{{R}_{3}}$ and $\widehat{{m}_{0}}$ in the objective function it is deduced:

$$\begin{aligned}\widehat{{E\Pi }_{B}}&={p}^{3}\left(H-L\right)+6{p}^{2}\left(1-p\right)\left(H-L\right)+3p{\left(1-p\right)}^{2}\left(H-L\right)\\&-\frac{{D}_{1}\left\{(1+\widehat{{m}_{0,L}})+{0,5}[1 +{0,5}\widehat{{m}_{0,L}}(1+\widehat{{m}_{0,LL}}]\right\}}{{D}_{2}}\end{aligned}$$

Proof of Proposition 4

4.1 Optimal value of ${{\varvec{m}}}_{0}^{\boldsymbol{*}}\equiv {{\varvec{m}}}_{0}$

The hypothesis that ${PF}_{1}$ holds, namely that the contract provides for the return pooling from three successes and from two successes and one fail, implies ${m}_{2}=0$; ${m}_{1}={m}_{1,L}=1$; ${R}_{3}=$ ${R}_{2|.}=$ ${R}_{2|\text{L}}\le 2H+L$ and ${R}_{1|.}=$ ${R}_{1|\text{L}.}={R}_{1|\text{LL}.}\le H+2L$.

By replacing these values ​​in the objective function and into the constraints (19), (20), (21), (22), (23), (24) and (25) the reduced form of the optimization problem under the hypothesis that Condition 2 holds is obtained:

$$\text{max} {p}^{3}\left(3H-{R}_{2|. }\right)+3{p}^{2}\left(1-p\right)(2H+L-{R}_{2|. })+3p{\left(1-p\right)}^{2}(2L+H-{R}_{1|\text{ L}.})$$

subject to:

$$\begin{aligned}{p}^{3}{R}_{2|. }&+3{p}^{2}\left(1-p\right){R}_{2|. }+3p{\left(1-p\right)}^{2}({R}_{1|\text{L}}-2c)+{\left(1-p\right)}^{3}\\&\times\left\{3L-{m}_{0}{m}_{0,L}\left(1+{m}_{0,LL}\right)c-{m}_{0}c\right\}=3I\end{aligned}$$

(30)

$${R}_{2|. }\le \left(1-{m}_{0}\right)3L+{m}_{0}3H$$

(31)

$${R}_{2|.}= \left(1-{m}_{0}\right)3L+{m}_{0}\left\{{0,5}(2H+L)+{0,5}\left[{m}_{0,L}(2H+L)+\left(1-{m}_{0,L}\right)3L\right]\right\}$$

(32)

$$ \begin{aligned} R_{{1{\text{L}}.|}} & = \left( {1 - m_{0} } \right)3L + m_{0} \left\{ 0,5\left( {H + 2L} \right) + 0,5\left[ m_{{0,L}}\right. \right. \\ & \quad \left. \left. \left[ 0,5\left( {\left( {1 - m_{{0,LL}} } \right)3L + m_{{0,LL}} \left( {H + 2L} \right)} \right) + 0,5\left( {H + 2L} \right) \right]+ \left( {1 - m_{{0,L}} } \right)3L \right] \right\} \end{aligned} $$

(33)

From the (30) it is deduced:

$$\begin{aligned} {}&{m}_{0}[{m}_{0,L}\left(1+{m}_{0,LL}\right)+1]\\&=\frac{\left[{p}^{3}+3{p}^{2}\left(1-p\right)\right]{R}_{2|. }+3p{\left(1-p\right)}^{2}{R}_{1|\text{L}}-3\left[I-{\left(1-p\right)}^{3}L\right]-6p{\left(1-p\right)}^{2}c}{{\left(1-p\right)}^{3}c}\end{aligned}$$

(34)

while from the (32) and from the (33) are obtained, respectively:

$${R}_{2|.}={m}_{0}\left(H-L\right)\left(1+{m}_{0,L}\right)+3L$$

(35)

and:

$${R}_{1|\text{L}.}={m}_{0}\left[1 +{0,5}{m}_{0,L}\left(1+{m}_{0,LL}\right) \right]{0,5}\left(H-L\right)+3L$$

(36)

By replacing the (35) and (36) in the (34), it is deduced:

$${m}_{0}^{*}=\frac{{D}_{3}}{{D}_{4}}$$

(37)

where ${m}_{0}^{*}$ is ever higher than zero for the constraint (31),

$${D}_{3}=-3\left[I-{\left(1-p\right)}^{3}L\right]-6p{\left(1-p\right)}^{2}c+\left[{p}^{3}+3{p}^{2}\left(1-p\right)+3p{\left(1-p\right)}^{2}\right]3L$$

and:

$$\begin{aligned} {D}_{4}&=\left[{m}_{0,L}^{*}\left(1+{m}_{0,LL}^{*}\right)+1\right]{\left(1-p\right)}^{3}c-\left[{p}^{3}+3{p}^{2}\left(1-p\right)\right]\left(H-L\right)\left(1+{m}_{0,L}^{*}\right)\\ &\quad -3p{\left(1-p\right)}^{2}\left[1 +{0,5}{m}_{0,L}^{*}\left(1+{m}_{0,LL}^{*}\right) \right]{0,5}\left(H-L\right) \end{aligned} $$

By setting ${m}_{0,L}^{*}={m}_{0,LL}^{*}=1$, it is deduced:

$${m}_{0}^{*}=\frac{-3\left[I-{\left(1-p\right)}^{3}L\right]-6p{\left(1-p\right)}^{2}c+\left[{p}^{3}+3{p}^{2}\left(1-p\right)+3p{\left(1-p\right)}^{2}\right]3L}{3{\left(1-p\right)}^{3}c-2\left[{p}^{3}+3{p}^{2}\left(1-p\right)\right]\left(H-L\right)-3p{\left(1-p\right)}^{2}\left(H-L\right)}$$

and using the binding ${PF}_{1}$:

$$\begin{aligned}& \left[{p}^{3}+3{p}^{2}\left(1-p\right)\right]\left(2H+L\right)+3p{\left(1-p\right)}^{2}\left(H+2L\right)-3{\left(1-p\right)}^{3}c\\ &\quad =3\left[I-{\left(1-p\right)}^{3}L\right]+6p{\left(1-p\right)}^{2}c\end{aligned}$$

(38)

this expression becomes:

$${m}_{0}^{*}=\frac{\begin{aligned}\left[ -{p}^{3}-3{p}^{2}\left(1-p\right)\right]\left(2H+L\right)-3p{\left(1-p\right)}^{2}\left(H+2L\right)\\ +3{\left(1-p\right)}^{3}c+\left[{p}^{3}+3{p}^{2}\left(1-p\right)+3p{\left(1-p\right)}^{2}\right]\end{aligned} 3L}{3{\left(1-p\right)}^{3}c-2\left[{p}^{3}+3{p}^{2}\left(1-p\right)\right]\left(H-L\right)-3p{\left(1-p\right)}^{2}\left(H-L\right)}$$

from which, by adding the terms in the numerator, it is obtained:

$${m}_{0}^{*}=\frac{2\left[-{p}^{3}-3{p}^{2}\left(1-p\right)\right]\left(H-L\right)-3p{\left(1-p\right)}^{2}\left(H-L\right)+3{\left(1-p\right)}^{3}c}{3{\left(1-p\right)}^{3}c-2\left[{p}^{3}+3{p}^{2}\left(1-p\right)\right]\left(H-L\right)-3p{\left(1-p\right)}^{2}\left(H-L\right)}=1$$

Setting ${m}_{0,L}^{*}={m}_{0,LL}^{*}=0$ in the (37), instead, it is deduced:

$${m}_{0}^{*}=\frac{-3\left[I-{\left(1-p\right)}^{3}L\right]-6p{\left(1-p\right)}^{2}c+\left[{p}^{3}+3{p}^{2}\left(1-p\right)+3p{\left(1-p\right)}^{2}\right]3L}{{\left(1-p\right)}^{3}c-\left[{p}^{3}+3{p}^{2}\left(1-p\right)\right]\left(H-L\right)-3p{\left(1-p\right)}^{2}{0,5}\left(H-L\right)}$$

(39)

The maximum value of the (39) is computed by the (38) and it is equal to:

$$-\left[{p}^{3}+3{p}^{2}\left(1-p\right)\right]2\left(H-L\right)-3p{\left(1-p\right)}^{2}\left(H-L\right)+3{\left(1-p\right)}^{3}c$$

and by replacing this value into the (39) it is obtained:

$${m}_{0}^{*}=\frac{-\left[{p}^{3}+3{p}^{2}\left(1-p\right)\right]2\left(H-L\right)-3p{\left(1-p\right)}^{2}\left(H-L\right)+3{\left(1-p\right)}^{3}c}{{\left(1-p\right)}^{3}c-\left[{p}^{3}+3{p}^{2}\left(1-p\right)\right]\left(H-L\right)-3p{\left(1-p\right)}^{2}{0,5}\left(H-L\right)}$$

The difference between the numerator and the denominator of the previous expression is:

$$2{\left(1-p\right)}^{3}c-\left[{p}^{3}+3{p}^{2}\left(1-p\right)\right]\left(H-L\right)-\text{1,5}p{\left(1-p\right)}^{2}\left(H-L\right)$$

and one may easily prove that this term is negative. From the (38), in fact, it is deduced:

$$\left[{p}^{3}+3{p}^{2}\left(1-p\right)\right]2\left(H-L\right)+3p{\left(1-p\right)}^{2}\left(H-L\right)-3{\left(1-p\right)}^{3}c=3(I-L)+6p{\left(1-p\right)}^{2}c$$

from which:

$$\begin{aligned} & 2{\left(1-p\right)}^{3}c-\left[{p}^{3}+3{p}^{2}\left(1-p\right)\right]\left(H-L\right)-\text{1,5}p{\left(1-p\right)}^{2}\left(H-L\right)\\ &\quad =-{\left(1-p\right)}^{3}c-3(I-L)-6p{\left(1-p\right)}^{2}c\\&\qquad+\left[{p}^{3}+3{p}^{2}\left(1-p\right)+3p{\left(1-p\right)}^{2}\right]\left(H+2L\right)-3p{\left(1-p\right)}^{2}{0,5}\left(H-L\right)\end{aligned}$$

For ${PF}_{1}$, this expression should necessarily be less than zero. So, ${m}_{0}^{*}<1$.

4.2 Optimal value of ${{\varvec{R}}}_{2|.}^{\boldsymbol{*}}\equiv {{\varvec{R}}}_{2|.}$

By replacing the (35) into the (30) it is obtained:

$${R}_{2|.}^{*}=3L+\frac{\left(H-L\right)\left(1+{m}_{0,L}\right){D}_{3}}{{D}_{4}}$$

where, for ${m}_{0,L}^{*}={m}_{0,LL}^{*}=1$ and ${PF}_{1}$ binding, as already proved in the Sect. 4.1 of the Appendix, it is deduced:

$${m}_{0}^{*}=\frac{{D}_{3}}{{D}_{4}}=1$$

and consequently, ${R}_{2|.}^{*}=2\left(H-L\right)+3L=2H+L$.

For ${m}_{0,L}^{*}={m}_{0,LL}^{*}=0$, instead, as already proved in the Sect. 4.1 of the Appendix, holds:

$${m}_{0}^{*}=\frac{{D}_{3}}{{D}_{4}}<1$$

and then ${R}_{2|.}^{*}<2H+L$.

4.3 Optimal value of ${{\varvec{R}}}_{1|{\varvec{L}}.}^{\boldsymbol{*}}\equiv {{\varvec{R}}}_{1|{\varvec{L}}.}$

By replacing the (36) in the (30), it is obtained:

$${R}_{1|\text{L}.}^{*}=\frac{{D}_{3} \left\{\left[1 +{0,5 }{m}_{0,L}^{*}\left(1+{m}_{0,LL}^{*}\right)\right]{0,5}\left(H-L\right)+3L\right\}}{{D}_{4}}$$

where, for ${m}_{0,L}^{*}={m}_{0,LL}^{*}=1$ and ${PF}_{1}$ binding, as proved in the Sect. 4.1 of the Appendix, it is deduced:

$${m}_{0}^{*}=\frac{{D}_{3} }{{D}_{4}}=1$$

and, consequently, ${R}_{1|\text{L}.}^{*}=\left(H-L\right)+3L=H+2L$.

For ${m}_{0,L}^{*}={m}_{0,LL}^{*}=0$, instead, as already proved in the Sect. 4.1 of the Appendix, holds:

$${m}_{0}^{*}=\frac{{D}_{3} }{{D}_{4}}<1$$

and then ${R}_{1|\text{L}.}^{*}<H+2L$.

4.4 The optimal borrower’s expected utility

By replacing ${R}_{1|\text{L}.}^{*}$ and ${R}_{2|.}^{*}$ in the objective function, it is obtained:

$$\begin{aligned}{E\Pi }_{B}^{*}&={p}^{3}3H+3{p}^{2}\left(1-p\right)\left(2H+L\right)+3p{\left(1-p\right)}^{2}\left(H+2L\right)-\left[{p}^{3}+3{p}^{2}\left(1-p\right)\right]3L\\&-\frac{{D}_{3} \left\{\left[1 +{0,5}{m}_{0,L}^{*}\left(1+{m}_{0,LL}^{*}\right) \right]{0,5}\left(H-L\right)+3L+\left[{p}^{3}+3{p}^{2}\left(1-p\right)\right]\left(H-L\right)\left(1+{m}_{0,L}^{*}\right)\right\}}{{D}_{4}}\end{aligned}$$

that may rewritten as:

$$\begin{aligned}{E\Pi }_{B}^{*}&={p}^{3}3H+3{p}^{2}\left(1-p\right)\left(2H+L\right)+3p{\left(1-p\right)}^{2}\left(H+2L\right)-\left[{p}^{3}+3{p}^{2}\left(1-p\right)\right]3L\\&-\frac{{D}_{3}\left\{\left[{p}^{3}+3{p}^{2}\left(1-p\right)\right]\left(H-L\right)\left(1+{m}_{0,L}^{*}\right)-3p{\left(1-p\right)}^{2}\left\{\left[1 +{0,5}{m}_{0,L}^{*}\left(1+{m}_{0,LL}^{*}\right) \right]{0,5}\left(H-L\right)+3L\right\}\right\}}{{D}_{4}}\end{aligned}$$

Since, as proved in the Sect. 4.1 of the Appendix, with ${m}_{0,L}^{*}={m}_{0,LL}^{*}=1$ and ${PF}_{1}$ binding holds:

$${m}_{0}^{*}=\frac{{D}_{3}}{{D}_{4}}=1$$

it derives:

$$\begin{aligned}{E\Pi }_{B}^{*}&={p}^{3}3H+3{p}^{2}\left(1-p\right)\left(2H+L\right)+3p{\left(1-p\right)}^{2}\left(H+2L\right)-\left[{p}^{3}+3{p}^{2}\left(1-p\right)\right]\\ & \times 2\left(H-L\right)-3p{\left(1-p\right)}^{2}[\left(H-L\right)+3L]-\left[{p}^{3}+3{p}^{2}\left(1-p\right)\right]3L={p}^{3}(H-L)\end{aligned}$$

Moreover, since, as proved in the Sect. 4.1 of the Appendix, ${m}_{0,L}^{*}={m}_{0,LL}^{*}=0$ implies:

$${m}_{0}^{*}=\frac{{D}_{3}}{{D}_{4}}<1$$

then ${E\Pi }_{B}^{*}>{p}^{3}(H-L)$.

Proof of Proposition 5

5.1 Optimal value of $\widetilde{{{\varvec{R}}}_{1|{\varvec{L}}{\varvec{L}}.}}\equiv {{\varvec{R}}}_{1|{\varvec{L}}{\varvec{L}}.}$

The hypothesis that ${PF}_{2}$ holds, namely that the contract provides for the return pooling from three successes, from two successes and one fail and from one success and two fails, implies ${m}_{2}={m}_{1}={m}_{1,L}=0$; $\widetilde{{m}_{0}}=\widetilde{{m}_{0,L}}=\widetilde{{m}_{0,LL}}\le 1$ and ${R}_{3}=$ ${R}_{2|.}=$ ${R}_{2|\text{L}}=$ ${R}_{1|.}=$ ${R}_{1|\text{L}.}={R}_{1|\text{LL}.}\le H+2L$.

By replacing these values into the objective function and into the constraints (19), (20), (21), (22), (23), (24) and (25), the reduced form of the optimization problem under the hypothesis that Condition 3 holds is derived:

$$\text{max} {p}^{3}\left(3H-{R}_{1|\text{LL}.}\right)+3{p}^{2}\left(1-p\right)\left[(2H+L)-{R}_{1|\text{LL}.}\right]+3p{\left(1-p\right)}^{2}[(H+2L)-{R}_{1|\text{LL}.}]$$

subject to:

$$\begin{aligned}{p}^{3}{R}_{1|\text{LL}.}&+3{p}^{2}\left(1-p\right){R}_{1|\text{LL}.}+3p{\left(1-p\right)}^{2}{R}_{1|\text{LL}.}+{\left(1-p\right)}^{3}\\&\times\left\{3L-{m}_{0}{m}_{0,L}\left(1+{m}_{0,LL}\right)c-{m}_{0}c\right\}=3I\end{aligned}$$

(40)

$${R}_{1|\text{LL}.}\le \left(1-{m}_{0}\right)3L+{m}_{0}3H$$

(41)

$${R}_{1|\text{LL}.}= \left(1-{m}_{0}\right)3L+{m}_{0}\left\{{0,5}(2H+L)+{0,5}\left[{m}_{0,L}(2H+L)+\left(1-{m}_{0,L}\right)3L\right]\right\}$$

(42)

$$\begin{aligned} {R}_{1|\text{LL}.}&=\left(1-{m}_{0}\right)3L+{m}_{0}\left\{{0,5}(H+2L)+{0,5}\left[{m}_{0,L}\left[{0,5}\left((1-{m}_{0,LL})3L\right.\right.\right.\right.\\ &\quad \left.\left.\left.\left. +{m}_{0,LL}\left(H+2L\right)\right)+{0,5}\left(H+2L\right)\right]+\left(1-{m}_{0,L}\right)3L\right]\right\}\end{aligned}$$

(43)

From the (40) and from the (43) are deduced, respectively:

$$\widetilde{{R}_{1|\text{LL}}}=\frac{3\left[I-L{\left(1-p\right)}^{3}\right]+{\left(1-p\right)}^{3}\left\{\widetilde{{m}_{0}}\left[\widetilde{{m}_{0,L}}\left(1+\widetilde{{m}_{0,LL}}\right)+1\right]c\right\}}{{p}^{3}+3p(1-p)}$$

(44)

and:

$$\widetilde{{m}_{0}}=\frac{2(\widetilde{{R}_{1|\text{LL}}}-3L)}{\left[1 +{0,5} \widetilde{{m}_{0,L}}\left(1+\widetilde{{m}_{0,LL}}\right)\right](H-L)}\le 1$$

(45)

By replacing the (45) into the (44) it is obtained:

$$\widetilde{{R}_{1|\text{LL}}}=\frac{3\left[I-L{\left(1-p\right)}^{3}\right]}{{p}^{3}+3p(1-p)}+\frac{3\left(I-L\right){\left(1-p\right)}^{3}\left[\widetilde{{m}_{0,L}}\left(1+\widetilde{{m}_{0,LL}}\right)+1\right]c}{{D}_{5}}$$

(46)

with:

$$\begin{aligned}{D}_{5}&=\left[{p}^{3}+3p(1-p)\right]{0,5}\left(H-L\right)+\widetilde{{m}_{0,L}}\left(1+\widetilde{{m}_{0,LL}}\right)\\ &\quad\times\left\{\left[{p}^{3}+3p(1-p)\right]{0,25}\left(H-L\right)-{\left(1-p\right)}^{3}c\right\}-{\left(1-p\right)}^{3}c\end{aligned}$$

By setting $\widetilde{{m}_{0,L}}=\widetilde{{m}_{0,LL}}=1$ and assuming that ${PF}_{2}$ is binding, the previous expression becomes:

$$\widetilde{{R}_{1|\text{LL}}}=\frac{3\left[I-L{\left(1-p\right)}^{3}\right]+{\left(1-p\right)}^{3}c}{{p}^{3}+3p(1-p)}$$

(47)

and may be easily proved that this expression is equal to $H+2L$. In fact, the binding ${PF}_{2}$:

$${p}^{3}\left(H+2L\right)+3{p}^{2}\left(1-p\right)\left(H+2L\right)+3p{\left(1-p\right)}^{2}\left(H+2L\right)+{\left(1-p\right)}^{3}3L=3\left[I+{\left(1-p\right)}^{3}c\right]$$

may be rewritten as:

$$H+2L=\frac{3\left[I+{\left(1-p\right)}^{3}c\right]-{\left(1-p\right)}^{3}3L}{\left[{p}^{3}+3p(1-p)\right]}$$

from which:

$$\left[{p}^{3}+3p(1-p)\right](H-L)+3L=3\left[I+{\left(1-p\right)}^{3}c\right]$$

(48)

Finally, by replacing the (48) in the (47), it is deduced $\widetilde{{R}_{1|\text{LL}}}=H+2L$.

5.2 Optimal value of $\widetilde{{{\varvec{m}}}_{0}}\equiv$ ${{\varvec{m}}}_{0}$

By replacing the (45) in the (46) it is obtained:

$$\widetilde{{m}_{0}}=\frac{3\left(I-L\right)}{{D}_{5}}$$

(49)

The lowest value of $\widetilde{{m}_{0}}$ is obtained by setting $\widetilde{{m}_{0,L}}=\widetilde{{m}_{0,LL}}=1$ (namely setting $\widetilde{{m}_{0,L}}\left(1+\widetilde{{m}_{0,LL}}\right)=2$) in the (49):

$$\frac{3\left(I-L\right)}{\left[{p}^{3}+3p(1-p)\right]\left(H-L\right)-3{\left(1-p\right)}^{3}c}$$

(50)

where $\left[{p}^{3}+3p(1-p)\right]\left(H-L\right)$ is the top state and intermediate state expected return net of the high state and intermediate state expected loss:

$$\left[{p}^{3}+3p(1-p)\right]\left(H-L\right)=\left[{p}^{3}+3p\left(1-p\right)\right]\left(H+2L\right)-\left[{p}^{3}+3p\left(1-p\right)\right]3L$$

The denominator of the (50) is higher than 0. In fact, the ${PF}_{2}$:

$$\left[{p}^{3}+3p(1-p)\right](H+2L)\ge 3\left[I+{\left(1-p\right)}^{3}c\right]-{\left(1-p\right)}^{3}3L$$

may be rewritten as:

$$\left[{p}^{3}+3p\left(1-p\right)\right]\left(H-L\right)+3L\ge 3I+3{\left(1-p\right)}^{3}c$$

(51)

This expression represents a necessary condition to finance three investment projects with the pooling between the top and the intermediate states: the expected return net of the expected loss in the top and intermediate states and the return in the low state have to be at least equal to the investment cost and the expected audit cost.

Since in the (51) $3\left(I-L\right)>0$, then $\left[{p}^{3}+3p(1-p)\right]\left(H-L\right)-3{\left(1-p\right)}^{3}c+3L>0$.

When $\widetilde{{m}_{0,L}}=\widetilde{{m}_{0,LL}}=1$ and ${PF}_{2}$ is binding, the (50) is equal to 1.

The highest value of $\widetilde{{m}_{0}}$, instead, is computed setting $\widetilde{{m}_{0,L}}=\widetilde{{m}_{0,LL}}=0$ in the (50) (namely by setting $\widetilde{{m}_{0,L}}\left(1+\widetilde{{m}_{0,LL}}\right)=0$):

$$\frac{3\left(I-L\right)}{\left[{p}^{3}+3p(1-p)\right]{0,5}\left(H-L\right)-{\left(1-p\right)}^{3}c}$$

(52)

It is possible to prove that the denominator of this expression is positive. From the (51), in fact, it may be deduced:

$$\left[{p}^{3}+3p\left(1-p\right)\right]{0,5}\left(H-L\right)-{\left(1-p\right)}^{3}c\ge {0,5}[3\left(I-L\right)+{\left(1-p\right)}^{3}c]$$

where ${0,5}[3\left(I-L\right)+{\left(1-p\right)}^{3}c]$ is higher than zero because $I>L$.

It may also be proved that the (52) must necessarily be less than 1 because, otherwise, the condition (51) would be violated.

To this end, consider the difference between the numerator and the denominator of the (52):

$$3\left(I-L\right)-\left[{p}^{3}+3p(1-p)\right]{0,5}\left(H-L\right)-{\left(1-p\right)}^{3}c$$

(53)

The maximum value of $3\left(I-L\right)$ may be calculated assuming that the (51) is binding:

$$3\left(I-L\right)=\left[{p}^{3}+3p\left(1-p\right)\right]\left(H-L\right)-3{\left(1-p\right)}^{3}c$$

The (53), then, becomes:

$$\left[{p}^{3}+3p\left(1-p\right)\right]\left(H-L\right)-4{\left(1-p\right)}^{3}c$$

and, since according to the (51):

$$\left[{p}^{3}+3p\left(1-p\right)\right]\left(H-L\right)-3{\left(1-p\right)}^{3}c=3\left(I-L\right)$$

results:

$$\left[{p}^{3}+3p\left(1-p\right)\right]\left(H-L\right)-4{\left(1-p\right)}^{3}c<3(I-L)$$

then:

$$\left[{p}^{3}+3p\left(1-p\right)\right]\left(H-L\right)+3L<3I+4{\left(1-p\right)}^{3}c$$

The investment project, therefore, is unviable because the expected return net of the expected loss in the top, intermediate low states are less than the investment cost and the expected audit cost.

This result has been obtained by assuming binding ${PF}_{2}$. When ${PF}_{2}$ is slack, the minuend of (52), that is $3\left(I-L\right)$, is smaller than that of the case in which ${PF}_{2}$ is binding and so this result remains valid.

5.3 Optimal borrower’s expected audit cost ${{\widetilde{{\varvec{E}}{\varvec{\Pi}}}}_{{\varvec{B}}}\equiv {\varvec{E}}{\varvec{\Pi}}}_{{\varvec{B}}}$

By replacing the (46) in the objective function, it is obtained the borrower’s utility with ${PF}_{2}$:

$${\widetilde{E\Pi }}_{B}=\frac{3\left[I-L{\left(1-p\right)}^{3}\right]}{{p}^{3}+3p(1-p)}+\frac{3\left(I-L\right){\left(1-p\right)}^{3}\left[{p}^{3}+3p(1-p)\right]\left[\widetilde{{m}_{0,L}}\left(1+\widetilde{{m}_{0,LL}}\right)+1\right]c}{{D}_{5}}$$

(54)

while by etting $\widetilde{{m}_{0,L}}=\widetilde{{m}_{0,LL}}=1$ in the previous it is deduced:

$$\begin{aligned}{\widetilde{E\Pi }}_{B}&={p}^{3}3H+3{p}^{2}\left(1-p\right)\left(2H+L\right)+3p{\left(1-p\right)}^{2}\left(H+2L\right)-3\left[I-L{\left(1-p\right)}^{3}\right]\\&\quad-\frac{9\left(I-L\right){\left(1-p\right)}^{3}c}{\left[{p}^{3}+3p(1-p)\right]\left(H-L\right)-3{\left(1-p\right)}^{3}c}\end{aligned}$$

and assuming that ${PF}_{2}$ is binding, it is obtained:

$$\begin{aligned}{\widetilde{E\Pi }}_{B}&={p}^{3}3H+3{p}^{2}\left(1-p\right)\left(2H+L\right)+3p{\left(1-p\right)}^{2}\left(H+2L\right) \\&\quad-3\left[I-L{\left(1-p\right)}^{3}+{\left(1-p\right)}^{3}c\right]\end{aligned}$$

Proof of Proposition 6

6.1 $3 {{\varvec{m}}}_{0}^{{\varvec{s}}{\varvec{i}}{\varvec{n}}}\left(1-{\varvec{p}}\right){\varvec{c}}<{{\varvec{m}}}_{0}^{*}\left[3{\left(1-{\varvec{p}}\right)}^{3}+6{\varvec{p}}{\left(1-{\varvec{p}}\right)}^{2}\right]{\varvec{c}}$

Consider the difference between the expected audit cost with separate financing and the expected audit cost of the joint financing with ${PF}_{1}$:

$$3 {m}_{0}^{sin}\left(1-p\right)c-{m}_{0}^{*}\left[3{\left(1-p\right)}^{3}c+6p{\left(1-p\right)}^{2}\right]c$$

The maximum value of ${m}_{0}^{*}\left[3{\left(1-p\right)}^{3}+6p{\left(1-p\right)}^{2}\right]c$ is $3{\left(1-p\right)}^{3}c+6p{\left(1-p\right)}^{2}c$ and it is obtained by setting ${m}_{0,L}^{*}={m}_{0,LL}^{*}=1$ and assuming that ${PF}_{1}$ is binding.

The previous expression, under these two assumptions, becomes:

$$3 {m}_{0}^{sin}\left(1-p\right)c-\left[3{\left(1-p\right)}^{3}c+6p{\left(1-p\right)}^{2}\right]c$$

that is equal to:

$$\frac{3(I-L)\left(1-p\right)c}{p\left(H-L\right)-\left(1-p\right)c}-\left[3{\left(1-p\right)}^{3}c+6p{\left(1-p\right)}^{2}\right]c$$

from which:

$$\frac{3\left(I-L\right)\left(1-p\right)c-\left[p\left(H-L\right)-\left(1-p\right)c\right]\left[3{\left(1-p\right)}^{3}c+6p{\left(1-p\right)}^{2}\right]c}{p\left(H-L\right)-\left(1-p\right)c}$$

Since:

$$p\left(H-L\right)-\left(1-p\right)c>3(I-L)\left(1-p\right)c$$

then holds:

$$3\left(I-L\right)\left(1-p\right)c<\left[p\left(H-L\right)-\left(1-p\right)c\right]\left[3{\left(1-p\right)}^{3}c+6p{\left(1-p\right)}^{2}\right]c$$

from which:

$$\frac{3\left(I-L\right)\left(1-p\right)c-\left[p\left(H-L\right)-\left(1-p\right)c\right]\left[3{\left(1-p\right)}^{3}c+6p{\left(1-p\right)}^{2}\right]c}{p\left(H-L\right)-\left(1-p\right)c}<0$$

Since $3 {m}_{0}^{sin}\left(1-p\right)c<\left[3{\left(1-p\right)}^{3}c+6p{\left(1-p\right)}^{2}\right]c$ and ${m}_{0}^{*}\in [{0,1}]$, then $3 {m}_{0}^{sin}\left(1-p\right)c<{m}_{0}^{*}\left[3{\left(1-p\right)}^{3}c+6p{\left(1-p\right)}^{2}\right]c$.

6.2 Graphical representation of the credit rationing regions with ${{\varvec{P}}{\varvec{F}}}_{1}$

Consider $3 I{F}^{\mho }$:

$$3 I{F}^{\mho }=3pH+ 3\left(1-p\right)L-3\left(1-p\right)c$$

and ${PF}_{1}^{{\mho }}$:

$$\begin{aligned}{PF}_{1}^{\mho }&=\left[{p}^{3}+3{p}^{2}\left(1-p\right)\right]\left(2H+L\right)+3p{\left(1-p\right)}^{2}\left(H+2L\right)\\ &-6p{\left(1-p\right)}^{2}c+{\left(1-p\right)}^{3}3L-3{\left(1-p\right)}^{3}c\end{aligned}$$

Note that:

$$3pH+ 3\left(1-p\right)L>\left[{p}^{3}+3{p}^{2}\left(1-p\right)\right]\left(2H+L\right)+3p{\left(1-p\right)}^{2}\left(H+2L\right)+{\left(1-p\right)}^{3}3L$$

namely, the vertical intercept of $3 I{F}^{\mho }$ is higher than that one of ${PF}_{1}^{\mho }$. Also note that, for every $p\in [{0,1}]$ and $c>0$, the inequality:

$$-6p{\left(1-p\right)}^{2}c-3{\left(1-p\right)}^{3}c>-3\left(1-p\right)c$$

ever holds, i.e., the slope of $3 I{F}^{\mho }$ is lower than that of ${PF}_{1}^{\mho }$. In fact, by dividing the right-hand side and the left-hand side for $3\left(1-p\right)c$, the following equivalent inequality is obtained:

$$-2p\left(1-p\right)-{\left(1-p\right)}^{2}>-1$$

and the difference between the right-hand side and the left-hand side of the previous expression gives:

$${p}^{2}>0$$

Finally, from the resolution of the system including ${PF}_{1}^{\mho }$ and $3 I{F}^{\mho }$ may be deduced that the two corresponding lines have an intersection point given by:

$${c}^{*}=\frac{\left[{p}^{3}+3{p}^{2}\left(1-p\right)\right]\left(2H+L\right)+3p{\left(1-p\right)}^{2}\left(H+2L\right)+{\left(1-p\right)}^{3}3L-3pH- 3\left(1-p\right)L}{-3\left[1-2p\left(1-p\right)-{\left(1-p\right)}^{2}\right](1-p)}$$

where ${c}^{*}>0$, because $\left[{p}^{3}+3{p}^{2}\left(1-p\right)\right]\left(2H+L\right)+3p{\left(1-p\right)}^{2}\left(H+2L\right)+{\left(1-p\right)}^{3}3L-3pH- 3\left(1-p\right)L<0$ and $-3\left[1-2p\left(1-p\right)-{\left(1-p\right)}^{2}\right](1-p)<0$ for every $p\in [{0,1}]$.

Proof of Proposition 7

7.1 $3 {{\varvec{m}}}_{0}^{{\varvec{s}}{\varvec{i}}{\varvec{n}}}\left(1-{\varvec{p}}\right){\varvec{c}}>\widetilde{{{\varvec{m}}}_{0}}{\left(1-{\varvec{p}}\right)}^{3}{\varvec{c}}$

To prove $3 {m}_{0}^{sin}\left(1-p\right)c>\widetilde{{m}_{0}}{\left(1-p\right)}^{3}c$, is sufficient to demonstrate that the difference between the minimum value of $\widetilde{{m}_{0}}{\left(1-p\right)}^{3}c$ (namely that obtained with $\widetilde{{m}_{0,L}}\left(1+\widetilde{{m}_{0,LL}}\right)=2$) and $3 {m}_{0}^{sin}\left(1-p\right)c$ is less than zero. This difference is equal to:

$$\begin{aligned}{}&\widetilde{{m}_{0}}{\left(1-p\right)}^{3}c-3 {m}_{0}^{sin}\left(1-p\right)c=\frac{9\left(I-L\right){\left(1-p\right)}^{3}c}{\left[{p}^{3}+3p(1-p)\right]\left(H-L\right)-{\left(1-p\right)}^{3}c}\\&\quad-\frac{3\left(I-L\right)\left(1-p\right)c}{p\left(H-L\right)-\left(1-p\right)c}\end{aligned}$$

and may be rewritten as:

$$\widetilde{{m}_{0}}{\left(1-p\right)}^{3}c-3 {m}_{0}^{sin}\left(1-p\right)c=\frac{3\left(I-L\right)(1-p)c}{\frac{\left\{\left[{p}^{3}+3p(1-p)\right]\left(H-L\right)-{\left(1-p\right)}^{3}c\right\}}{3{\left(1-p\right)}^{2}}}-\frac{3\left(I-L\right)\left(1-p\right)c}{p\left(H-L\right)-\left(1-p\right)c}$$

Given the same numerator, for every $p\in \left[{0,1}\right]$, it is obtained:

$$\frac{\left\{\left[{p}^{3}+3p(1-p)\right]\left(H-L\right)-{\left(1-p\right)}^{3}c\right\}}{3{\left(1-p\right)}^{2}}-\left(1-p\right)c>0$$

Since the denominator of $\widetilde{{m}_{0}}{\left(1-p\right)}^{3}c$ is higher than the denominator of $3 {m}_{0}^{sin}\left(1-p\right)c$ and the numerator is the same, then $3 {m}_{0}^{sin}\left(1-p\right)c>\widetilde{{m}_{0}}{\left(1-p\right)}^{3}c$.

7.2 Graphical representation of the credit rationing regions with ${{\varvec{P}}{\varvec{F}}}_{2}$

Consider again $3 I{F}^{\mho }$ and ${PF}_{2}^{\mho }$ and note that:

$$3pH+ 3\left(1-p\right)L>\left[{p}^{3}+3{p}^{2}\left(1-p\right)+3p{\left(1-p\right)}^{2}\right]\left(H+2L\right)+{\left(1-p\right)}^{3}3L$$

namely the vertical intercept of $3 I{F}^{\mho }$ is higher than that one of ${PF}_{2}^{\mho }$.

Note also that, for every $p\in [{0,1}]$ and $c>0$, the inequality:

$$ - 3\left( {1 - \rho } \right)c < - 3\left( {1 - \rho } \right)^{3} c $$

ever holds, i.e., the slope of $3 I{F}^{\mho }$ is lower than that one of ${PF}_{2}^{\mho }$.

By solving the system formed by ${PF}_{2}^{\mho }$ and $3 I{F}^{\mho }$ is deduced that the two corresponding lines have an intersection point given by:

$${c}^{**}=\frac{\left[{p}^{3}+3{p}^{2}\left(1-p\right)+3p{\left(1-p\right)}^{2}\right]\left(H+2L\right)+{\left(1-p\right)}^{3}3L-3pH- 3\left(1-p\right)L}{-3\left[1-{\left(1-p\right)}^{2}\right]\left(1-p\right)}$$

where ${c}^{**}>0$, because $\left[{p}^{3}+3{p}^{2}\left(1-p\right)+3p{\left(1-p\right)}^{2}\right]\left(H+2L\right)+{\left(1-p\right)}^{3}3L-3pH- 3\left(1-p\right)L<0$ and $-3\left[1-{\left(1-p\right)}^{2}\right]\left(1-p\right)<0$.

Finally, note that the intercept of ${PF}_{1}^{\mho }$ is higher than that of ${PF}_{2}^{\mho }$:

$${PF}_{1}^{0}-{PF}_{2}^{0}=\left[{p}^{3}+3{p}^{2}\left(1-p\right)\right]\left(2H+L\right)-\left[{p}^{3}+3{p}^{2}\left(1-p\right)\right]\left(H+2L\right)>0$$

and that the slope of ${PF}_{2}^{\mho }$ is higher than that of ${PF}_{1}^{\mho }$:

$$ - 3\left( {1 - p} \right)^{3} c - 6p\left( {1 - p} \right)^{2} c < - 3\left( {1 - p} \right)^{3} c $$