1 Introduction

Surface wettability, determined by the surface free energy, has been an important topic recently due to its highly potential application in various fields (Bocquet and Barrat 2007; Rauscher and Dietrich 2008; Roach et al. 2008; Shirtcliffe et al. 2010; Drelich et al. 2011; Yan et al. 2011). For example, hydrophobicity could be used for anti-bacteria, anti-corrosion, self-cleaning, drag reduction (Qu et al. 2004; Truesdell et al. 2006; Roach et al. 2008), while hydrophilicity is applicable to anti-fogging, biofouling, contact lenses, bone-like structures and enhancement of boiling heat transfer (Drelich et al. 2011).

In micro/nano-channel studies, it has been found that surface wettability plays an essential role in drag reduction and heat transfer performance (Xue et al. 2003; Qu et al. 2004; Truesdell et al. 2006; Kim et al. 2008; Cao et al. 2009; Li et al. 2010). The surface wettability microscopically originates from the solid–liquid interaction, therefore, determines the resistance of the momentum and energy transportation across the solid–liquid interface. The velocity slip (u s) and temperature jump (T j), defined as the velocity and temperature differences between the wall and liquid adjacent to it, respectively, are the characteristic quantities. For example, a superhydrophobic surface could give a giant velocity slip (Lee et al. 2008), while a superhydrophilic surface (Thompson and Robbins 1990) could lead to a negative velocity slip. In practice, the slip length (L s) is widely used to quantify the degree of u s, according to the linear Navier boundary condition, as (Gad-el-Hak 1999):

$$L_{\text{s}} = u_{\text{s}}/\left. {\frac{\partial u}{\partial n}} \right|_{\text{w}}$$
(1)

Similarly, the Kapitza length (L K) is widely used to quantify the degree of T j as (Kim et al. 2008):

$$L_{\text{K}} = T_{\text{j}} /\left. {\frac{\partial T}{\partial n}} \right|_{\text{w}}$$
(2)

where \(\left. {\left( {\partial u/\partial n} \right)} \right|_{\text{w}}\)and \(\left. {\left( {\partial T/\partial n} \right)} \right|_{\text{w}}\) are the velocity and temperature gradients of a liquid at wall surface, respectively. Accurate predictions of L s and L K are crucial for design of micro/nano-fluidic devices because experiments have proved that the velocity and temperature profiles greatly deviates from the analytical solutions (Swartz and Pohl 1989; Choi et al. 2003; Joseph and Tabeling 2005; Truesdell et al. 2006; Byun et al. 2008). The problem is complicated because it is influenced by many parameters such as the solid–liquid interaction (Thompson and Robbins 1990; Thompson and Troian 1997; Xue et al. 2003; Priezjev 2007; Soong et al. 2007; Kim et al. 2008; Liu and Li 2009; Kim et al. 2010; Liu et al. 2010), commensurability of solid and liquid densities (Thompson and Robbins 1990; Thompson and Troian 1997), solid structure (Soong et al. 2007), surface geometry (Cao et al. 2006), surface temperature (Kim et al. 2008), driving force (Liu and Li 2009; Liu et al. 2010), shear rate (Priezjev 2007; Kim et al. 2010), flow rate (Liu and Li 2009; Liu et al. 2010), viscous dissipation (Kim et al. 2010), temperature gradient (Kim et al. 2008). Among all these factors, the solid–liquid interaction has been widely accepted as the predominant one. On the other hand, although L s and L K are qualitatively counterparts, it is not easy to measure both simultaneously in nano-confined liquid flows though L s is measurable by microparticle image velocimetry (micro-PIV) measurement (Joseph and Tabeling 2005; Truesdell et al. 2006; Byun et al. 2008; Lee et al. 2008) and L K is accessible via time resolved reflectivity or absorption measurement (Gavrila et al. 2009), separately. Therefore, a correlation between L s and L K is important. Recently, we have found such explicit correlations between L s and L K in Couette-type (shear-driven) flows (Sun et al. 2013a):

$$L_{\text{K}} /\sigma = 2.3,\beta > 2$$
(3a)
$$L_{\text{K}} /\sigma = 2.3 + 0.04\left( {L_{s} /\sigma } \right)^{2.8} ,\beta \le 2$$
(3b)

where σ is the length characteristic parameter, \(\beta = \varepsilon_{\text{sl}} /\varepsilon\) is the proportional factor of solid–liquid bonding strength (ɛ sl and ɛ being the energy characteristic parameters for solid–liquid and liquid–liquid interactions, respectively).

It has been found that the decoupling emerges and the hybrid simulation becomes invalid when U > 3.0 στ −1 in shear flows (Sun et al. 2013a), where U is the driving velocity, and \(\tau = \sqrt {m\sigma^{2} /\varepsilon }\) is the characteristic time (m being the mass of a liquid molecule). As was pointed out, this is solely related to the viscous dissipation. To clarify, in the present work, the nano-confined shear flows are studied using full molecular dynamics simulation (MDS) in order to (1) show the effects of viscous dissipation on the velocity, temperature and density profiles and (2) examine the applicability of the hybrid simulation and validity of the correlation between L s and L K.

2 Simulation method

The modified Lennard-Jones (L-J) potential function (1973) is used for liquid–liquid interaction:

$$\varphi (r) = 4\varepsilon \left\{ {\left[ {\left( {\frac{\sigma }{r}} \right)^{12} - \left( {\frac{\sigma }{r}} \right)^{6} } \right]} \right. + \left[ {6\left( {\frac{\sigma }{{r_{\text{c}} }}} \right)^{12} - 3\left( {\frac{\sigma }{{r_{\text{c}} }}} \right)^{6} } \right]\left( {\frac{r}{{r_{\text{c}} }}} \right)^{2} \left. { - \left[ {7\left( {\frac{\sigma }{{r_{\text{c}} }}} \right)^{12} - 4\left( {\frac{\sigma }{{r_{\text{c}} }}} \right)^{6} } \right]} \right\}$$
(4)

where r is the intermolecular separation and \(r_{\text{c}} = 2.5\ \sigma\) is the cutoff radius. The solid–liquid interaction is also described by Eq. (4) but with different length and energy characteristic parameters of \(\sigma_{\text{sl}} = 0.91\ \sigma\) and \(\varepsilon_{\text{sl}} = \beta \varepsilon\). The value of β varies from 0.1 to 10, corresponding to the surface free energy from low to high. This approach has been widely used in MDS studies due to its simplicity and clear physical meaning (Thompson and Robbins 1990; Thompson and Troian 1997; Xue et al. 2003; Priezjev 2007; Kim et al. 2008; 2010).

Shear-driven flow conducted in a planar nano-channel with two confining surfaces separated by a distance H = 54.5σ is considered in the present work (see Fig. 1). The overall system size is l x  × l y  × l z  = 28.5σ × 7.0σ × 60.1σ, where \(l_{i} , \, i = x,y,z\) are the dimensions in x, y and z directions. The channel height is H = 54.4σ. The two parallel walls are kept at a constant temperature T w with the upper wall moving at a constant velocity U. The periodic boundary conditions are used in horizontal directions. Two atomically smooth walls are arranged at the upper and lower sides in parallel. Each wall is represented by 3 layers of solid atoms in face-centered cubic (111) pattern normal to z-axis with the lattice constant of \(\sigma_{\text{s}} = 0.814\ \sigma\) (therefore \(\rho_{\text{s}} = 2.62\ \sigma^{ - 3}\)), where σ s and \(\rho_{\text{s}}\) are the characteristic length and number density of solid, respectively. Neighboring atoms are connected by Hookean springs with the constant k = 3,249.1ɛσ −2 (Yi et al. 2002). Two extra layers of atoms are set below/above the lower/upper walls (Maruyama 2000; Sun et al. 2009). The outmost layers are stationary as a frame while the second outmost layers are governed by the Langevin thermostat.

Fig. 1
figure 1

Schematic of computational model

Full size image
$$\frac{{{\text{d}}{\mathbf{p}}_{i} (t)}}{{{\text{d}}t}} = - \alpha {\mathbf{p}}_{i} (t) + {\mathbf{f}}_{i} (t) + {\mathbf{F}}_{i} (t)$$
(5)

where t is time; \({\mathbf{p}}_{i} \left( t \right)\) is the momentum vector of ith atom; \({\mathbf{f}}_{i} \left( t \right)\) is the interaction force vector between ith atom and its neighbors; \({\mathbf{F}}_{i} \left( t \right)\) is the exciting force vector, of which the components are randomly sampled from the Gaussian distribution with mean \(\mu_{\text{G}} = 0\) and standard deviation \(\sigma_{\text{G}} = \sqrt {2\alpha k_{\text{B}} T_{\text{w}} /\delta t}\) (α = 168.3τ −1 being the damping constant, \(k_{\text{B}} = 1.38 \times 10^{ - 23}\ {\text{J}\ \text{K}^{ - 1}}\) being the Boltzmann constant, \(T_{\text{w}} = 1.1\ \varepsilon k_{\text{B}}^{ - 1}\) being the preset wall temperature and \(\delta t = 0.005\ \tau\) being the time step). The overall solid atom number is 3,500.

The system is initialized by 8,928 liquid molecules uniformly arranged between the walls with the number density ρ = 0.81 σ −3. A period of 200 τ is used for thermal equilibrium at \(T = 1.1\ \varepsilon k_{\text{B}}^{ - 1}\). The following 6,000 τ is the production period, during which the upper wall velocity is kept constant at U = 1.0–5.0 στ −1. The sampling is carried out every time step, and the averaging is over the final 2,000 τ. The leapfrog scheme is used for integrating the equations of motion, and the cell subdivision technique is employed to improve the computational efficiency (Allen and Tildesley 1987; Rapaport 2004).

Note that the system size is the same, but the boundary conditions at the upper wall are different between the hybrid simulations (Sun et al. 2013b) and the present MDSs. In the hybrid simulation, the liquid near the upper wall is solved by the finite volume method with no slippage at the interface while in the present MDSs, slippage exists at both upper and lower interfaces.

3 Results and discussion

The u-velocity, temperature and density profiles with β = 0.1–10 and U = 1.0–5.0 στ −1 are shown in Fig. 2. For a given U, all the profiles are significantly influenced by β. u s varies from positive to negative with increasing β because liquid molecules could move freely under weak solid–liquid interaction, i.e., small β, while strong interaction, i.e., large β, tends to trap the liquid molecules around. The shear rate \(\dot{\gamma }\) is found to increase correspondingly. A non-monotonic variation of temperature profile is seen. When β ≤ 4, the temperature profile is found to drop as a whole and T j correspondingly decreases. Afterward, T j exists within the liquid rather than at the solid–liquid interface and remains almost constant but the maximum value of the temperature taking at the middle increases when β > 4. The density profiles clearly show fading oscillation in liquid away from the wall surface due to the, experimentally proved, layering structure near the solid–liquid interface (Magnussen et al. 1995; Regan et al. 1995; Huisman et al. 1997). At least five peaks, the range of which is about 4.9σ, can be clearly seen, indicating that the five layers of liquid molecules are more influenced by the solid–liquid interaction than by the liquid–liquid interaction. Based on the competition between the boundary and bulk factors (Sun et al. 2013b), the liquid adjacent to the solid is referred to as the boundary region, where the liquid molecules would experience the combined effect of β and \(\dot{\gamma }\), while the rest of the liquid is referred to as the bulk region, where the boundary-related effect (β) is absent. The amplitude of the oscillation increases with increasing β because stronger solid–liquid interaction tends to keep the liquid molecules stacked more densely. Due to the strong solid–liquid interaction (β > 2), the first one or two layers of liquid molecules within the boundary region are completely dominated by β and behave like extensional solid with the u-velocity, temperature and density all very close to those of the solid.

Fig. 2
figure 2

(Color online) u-velocity, temperature and density profiles at a U = 1.0 στ −1 b U = 3.0 στ −1 and c U = 5.0 στ −1. The dash lines show constant density at ρ = 0.81 σ −3

Full size image

On the other hand, the effect of viscous dissipation becomes more intensive with increasing \(\dot{\gamma }\); the maximum value of the temperature generally rises from \(1.14 - 1.18\ \varepsilon k_{\text{B}}^{ - 1}\) (U = 1.0 στ −1) to \(1.99 - 2.40\;\varepsilon k_{\text{B}}^{ - 1}\) (U = 5.0 στ −1). The large rise in temperature profile due to large \(\dot{\gamma }\), in turn, changes \(\dot{\gamma }\) from uniform to non-uniform, especially for large β cases (see Fig. 2c). The density profile varies diversely due to viscous dissipation; the density in the boundary region does not show appreciable change, while that in the bulk region bends lower at the middle and rises at the ends, showing weak thermal expansibility and compressibility of the liquid.

The aforementioned non-monotonic variation of the temperature profile is a result of typically competitive phenomenon, as was discussed in the Poiseuille flows (Sun et al. 2013b). To clearly illustrate this, we take the average temperature T ave instead of the whole temperature profile for analysis. Figure 3a shows the energy balance between the viscous dissipation rate per unit volume Φ (=\(\int_{0}^{H} {2\mu \dot{\gamma }} {\text{d}}z\), μ being the dynamic viscosity) in the liquid and the heat flux q (=T j/R K, R K being the interfacial thermal resistance) at the solid–liquid interface. For a given U, on the one hand, increasing β means decreasing R K (Xue et al. 2003), which reduces T j, therefore T ave tends to decrease; on the other hand, increasing β results in decrease in \(u_{\text{s}}\) (see Fig. 2) and consequently increase in \(\dot{\gamma }\), which increases Φ, therefore \(T_{\text{ave}}\) tends to increase. For clarity, the analysis is simply expressed as for a given U, β↑ → R K↓ → T j↓ → T ave↓, meanwhile, β↑ → \(u_{\text{s}}\)↓ → \(\dot{\gamma }\)↑ → Φ↑ → T ave↑. The opposite influences of β on T ave form a competitive condition and an extremum are predictable. Figure 3b shows the variations of T ave with β for different U. The minimum values of T ave are clearly seen at β ≈ 4. It is also found that for a given β, T ave monotonically increases with U. The reason can be expressed as for a given β, U↑ → \(\dot{\gamma }\)↑ → Φ↑ → T ave↑.

Fig. 3
figure 3

a Schematic of heat transfer and b variation of average temperature (T ave) against solid–fluid bonding factor (β)

Full size image

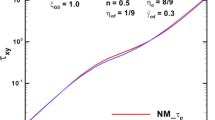
In Fig. 4a, L s and L K are seen to be nonlinearly decreasing functions of β with a threshold of β = 2. They quickly drop when β ≤ 2 and become scattered when β > 2, especially for L s, because the solid–liquid interaction is strong that the one or two layers of liquid molecules adjacent to the wall behave as extensional solid and consequently the velocity slip and temperature jump are found within the liquid rather than at the solid–liquid interface (see Fig. 2). This diverse variation agrees well with our hybrid simulation results (Sun et al. 2013a) and Thompson and Robbins’ work (Thompson and Robbins 1990). In Fig. 4b, L s and L K are seen as decreasing functions of \(\dot{\gamma }\). If viscous dissipation is negligible, u(z) varies as a linear function of \(\dot{\gamma }\), i.e., \(u\left( z \right) = \dot{\gamma }z + u_{\text{s}}\). Considering u(H) = U and \(L_{\text{s}} = u_{\text{s}} /\dot{\gamma }\), we have \(L_{\text{s}} = U/\dot{\gamma } - H\), which is also plotted in Fig. 4b. The hybrid simulation results are found to agree well with the analytical solutions because the shear rate is low and the dissipation effect is weak. In fact, it had been found that the decoupling emerges and the hybrid simulation becomes invalid when U > 3.0 στ −1. When the viscous dissipation is weak (i.e., low shear rate cases), the MDS results agree with the analytical solutions, whereas when the viscous dissipation is strong (i.e., high shear rate cases), the MDS results apparently deviate from the analytical solutions. Similar trend is also seen in the variation of L K against \(\dot{\gamma }\). Besides, it is found that for a given β, L K is essentially a decreasing function of \(\dot{\gamma }\) when β ≤ 2 and L K becomes much less sensitive to β when β > 2.

Fig. 4
figure 4

Variations of slip length (L s) and Kapitza length (L K) against a solid–fluid bonding factor (β) and b shear rate (\(\dot{\gamma }\)). The hybrid simulation results are taken from (Sun et al. 2013a)

Full size image

The variation of L K against L s is summarized covering all the cases in Fig. 5. Good agreement is seen between the present MDS and hybrid simulation results (Sun et al. 2013a) even though the velocity range in the present MDS is larger. The general correlation between L K and L s is presented based on the data fitting of all the simulation results. Note that the effects of \(\dot{\gamma }\) and β are implicitly included. Since the scenarios and mechanisms are different for small β (β ≤ 2) and large β (β > 2) cases, L K is found basically constant when β > 2, while it grows as a power function of L s when β ≤ 2. Therefore, the correlation has different forms, as shown in Eq. (3a, 3b). This different form again emphasizes that the solid–liquid interaction plays a dominant role in the boundary region. It is noteworthy that Eq. (3a, 3b) is refitted using only the present MDS results, and it does not show appreciable change (see Fig. 5). Therefore, it is confirmed that Eq. (3a, 3b) is valid within U = 1.0–5.0 στ −1 (\(\dot{\gamma } = 0.012 - 0.094\ \tau^{ - 1}\)) and β = 0.1–10. In fact, the dissipation effect has already been taken into account. This strongly suggests that L K and L s may have inherent correlation and no matter how L s is generated, e.g., identical L s by different combinations of \(\dot{\gamma }\) and β, L K varies correspondingly following Eq. (3a, 3b) regardless of \(\dot{\gamma }\). Moreover, it has been confirmed the applicability of the hybrid simulation (Sun et al. 2013b).

Fig. 5
figure 5

Variation of Kapitza length (L K) against slip length (L s). The hybrid simulation results are taken from (Sun et al. 2013a)

Full size image

Note that the computational cost for the hybrid simulation is remarkably cheaper as it is generally proportional to the particle numbers involved and could be largely saved further considering the application of symmetric boundary condition and flexible region decomposition (Sun et al. 2012). Besides, it is shown recently that the computational efficiency could be further increased by improvement of time-step coupling (Lockerby et al. 2013).

4 Concluding remarks

In this work, a full MDS of nano-confined shear flows has been performed. The molecular dynamics results are compared with the hybrid simulation ones. It is demonstrated that the applicability of the hybrid simulation is highly dependent on the effect of viscous dissipation. The previously revealed non-monotonic variation of the average temperature in pressure-driven flows by the hybrid simulation is also found in shear-driven flows by MDS. It is confirmed that Eq. (3a, 3b) is valid within U = 1.0–5.0 στ −1 (\(\dot{\gamma } = 0.012 - 0.094\ \tau^{ - 1}\)) and β = 0.1–10. The fact that although the dissipation effect causes deviation in hybrid simulation results from MDS results, the correlation between L s and L K still holds, indicating an inherent correlative dependence.