1 Introduction

During the past two decades, nanomechanics has been one of the main fields of research in mechanics and engineering. In particular, the bifurcation analysis of nanostructures, such as rods, has attracted significant attention from researchers. The initial step in such an analysis is the formulation of appropriate constitutive equations which take into account relevant physical phenomena. Namely, due to the enormous computational cost of molecular dynamics and objective problems in performing experiments at nanoscale, nonlocal continuum constitutive theories remain the most common tools for analyzing the nanostructures. The foundational works in nonlocal continuum mechanics theories are presented in [1,2,3]. Subsequently, several nonlocal continuum constitutive theories have been developed, including Eringen’s nonlocal integral model (strain-driven integral model) [4], Eringen’s differential model [5], Eringen’s two-phase local/nonlocal model (local/nonlocal strain-driven model) [4, 6], the nonlocal integral stress-driven model [7,8,9], the two-phase local/nonlocal stress-driven model [10], the modified couple stress and the nonlocal strain gradient theory. Among these theories, the most commonly used is Eringen’s differential model. Its application to the buckling and dynamic behavior of nanorods began with [11] and since then it has been used many times. Although Eringen’s differential model has allowed researchers to obtain many significant analytical results, it has become apparent that this method lacks consistency. Among other papers, these inconsistencies are addressed in [11] and [12]. One of these inconsistencies is the well-known paradox of a cantilever beam subjected to a concentrated load at its end. To overcome these inconsistencies, some researchers [13,14,15] have attempted to utilize Eringen’s nonlocal integral model. However, as shown by [9] the use of Eringen’s nonlocal integral model, in general, leads to ill-posed problems. A solution to the aforementioned problems was found by employing Eringen’s two-phase local/nonlocal model [9] which is widely accepted in the literature [8, 12, 15,16,17,18,19,20,21,22,23,24,25,26,27,28,29].

Therefore, our main focus in the following will be on Eringen’s two-phase local/nonlocal model. The rationale for its use is justified by the fact that Eringen’s two-phase local/nonlocal model yields a well-posed problem, eliminating the paradox of a cantilever with a concentrated load at its end [9, 12]. Additionally, the aforementioned model, produces a softening effect [12, 18] which is physically acceptable in the buckling problem addressed here. Alongside Eringen’s two-phase local/nonlocal model, we will also utilize Eringen’s differential model to compare the two models.

As mentioned earlier, the main aim of this paper is to focus on the linear and nonlinear analysis of nonlocal elastic rods. In order to demonstrate the practical applications of nonlocal theory in column buckling and post-buckling, we refer to several studies. Some notable buckling problems include: the buckling of nanotubes with classical boundary conditions [18, 30], buckling of embedded nanotubes under thermal effects [31], buckling of shearable nanorods [32], buckling of rotating nanorods [33], buckling of multiwalled carbon nanotubes [34], buckling of heavy nanorods [35], lateral-torsional buckling [36], among others. It is worth noting that some results presented in this paper are consistent with those of [32] and [18]. Regarding the post-buckling of nanorods, some of relevant papers are [37,38,39,40,41,42,43,44,45,46]. Among these papers, it is important to mention [45], as we will apply the same analytical method for nonlinear analysis.

In this paper, we will examine a nanotube through which a nanostring passes with a constant velocity. The nanotube will be clamped at its ends, contrary to the case treated in [47]. In order to simplify the analysis, we assume that the nanostring is inextensible, henceforth referred to as a string in the remainder of the paper. Owing to the development of nanotechnology this system can be considered as part of nanodevices and nanomachines [48]. The goal of this paper is to perform a nonlinear local bifurcation analysis of the divergence type of the nanotube. This means that, as in [47], only the number of equilibrium configurations near bifurcation points will be examined. This goal will be achieved by employing the analytical methods of nonlinear bifurcation analysis. In particular, the stability boundary and post-buckling behavior will be examined by using the Lyapunov–Schmidt method. It is noteworthy that the use of analytical methods in nonlinear analysis is one of the advantages of this paper, as numerical methods are typically applied in the analysis of nanorods described by two-phase local/nonlocal models [46]. The governing equations will be nonlinear due to geometric nonlinearity since the constitutive equations will be linear. We assume that the nanotube is inextensible and unshearable. The main focus of the paper will be to determine the influence of the parameters in the constitutive equations (the small length scale parameter and the phase parameter) on the buckling and post-buckling behavior of the nanotube.

In particular, for both models under consideration, we will first derive the governing equations for the nanotube in a suitable form for bifurcation analysis. Subsequently, we will analytically determine characteristic equations and solutions to the linear governing equations. It is worth noting that the form of the characteristic equations allows us to distinguish between symmetrical and antisymmetrical solutions. Next, we will apply nonlinear analysis to obtain bifurcation equations. Finally, the influence of nonlocal effect on the critical buckling load, the type of bifurcation and the post-critical shape of the nanotube is presented for both models.

2 Mathematical formulation

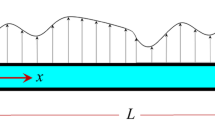
Let us consider an inextensible and unshearable nanotube of length L. Through the nanotube an inextensible string is passing. On the left hand side of the nanotube, the string is pulled to the left with a constant velocity v, while on the right hand side, the string moves freely over a smooth surface. The nanotube is clamped at both ends with the movable right support (Fig. 1).

Fig. 1
figure 1

A nanotube through which passes a string

Full size image

In the bifurcation analysis that follows, we assume that there is a friction force between the nanotube and the string. The frictional force per unit length F is assumed to be an arbitrary function of the velocity v. In this case, as shown in [47], the normal force N between the nanotube and the string per unit length is of the form

$$\begin{aligned} N=\left[ \rho v^{2}+F(S-L)\right] \varphi ^{\prime }, \end{aligned}$$
(1)

where S is the arc length of the nanotube, \(\frac{d(\cdot )}{\textrm{d}S}=(\cdot )^{\prime }\),\(\ \rho \) is the uniform mass density of the string per unit length and \(\varphi \) is the slope of the tangent to the nanotube axis (Fig. 2).

Fig. 2
figure 2

An elementary part of the nanotube

Full size image

In order to derive the equilibrium equations of the nanotube we introduce the coordinate system xAy and the unit normal and tangential vectors \(\textbf{n}\) and \(\textbf{t}\), respectively (Figs. 1 and 2). Since an elementary part of the nanotube is in equilibrium the resultant force of the system acting on that part is equal to zero, i.e.,

$$\begin{aligned} \textbf{R}^{\prime }=\left\{ \left[ \rho v^{2}+F(S-L)\right] \textbf{t}\right\} ^{\prime }, \end{aligned}$$
(2)

where \(\textbf{R}\) is the contact force (Fig. 2). From (2) it follows

$$\begin{aligned} \textbf{R}=\left[ \rho v^{2}+F(S-L)\right] \textbf{t}+D_{1}\mathbf{i+}D_{2} \textbf{j,} \end{aligned}$$
(3)

or, in scalar form

$$\begin{aligned} R_{x}=\left[ \rho v^{2}+F(S-L)\right] \cos \varphi +D_{1},\ \ \ \ \ \ \ R_{y}=\left[ \rho v^{2}+F(S-L)\right] \sin \varphi +D_{2}, \end{aligned}$$
(4)

where \(D_{1}\) and \(D_{2}\) are arbitrary constants and \(R_{x}\) and \(R_{y}\) are the components of the contact force along the x and y axes with the unit vectors \(\textbf{i}\) and \(\textbf{j}\). Because the resultant moment also has to be zero in equilibrium, it follows that

$$\begin{aligned} M^{\prime }=D_{1}\sin \varphi -D_{2}\cos \varphi , \end{aligned}$$
(5)

where (4) is used and M is the bending moment. For an inextensible nanotube the following geometrical relations hold

$$\begin{aligned} x^{\prime }=\cos \varphi ,\hspace{1cm}y^{\prime }=\sin \varphi . \end{aligned}$$
(6)

With the help of the geometrical relations (6), the equilibrium Eq. (5) can be integrated to obtain

$$\begin{aligned} M=D_{1}y-D_{2}x+D_{3}, \end{aligned}$$
(7)

Since the right support of the nanotube moves freely in the horizontal direction the following boundary conditions hold (Fig. 1)

$$\begin{aligned} x(0)=0,~~y(0)=0,\ \varphi (0)=0,~\ R_{x}(L)=0,\ \ y(L)=0,\ \ \varphi (L)=0. \end{aligned}$$
(8)

From (4)\(_{1}\) and (8)\(_{4,6}\), we get

$$\begin{aligned} D_{1}=-\rho v^{2}, \end{aligned}$$
(9)

while using (6), (7) and (8)\(_{1,2,3,5,6}\) it follows that

$$\begin{aligned} D_{2}=\frac{M(0)-M(L)}{\int _{0}^{L}\cos \varphi dS},\ \ D_{3}=M(0). \end{aligned}$$
(10)

Substituting (9) and (10)\(_{1}\) into the equilibrium Eq. (5) we obtain

$$\begin{aligned} M^{\prime }=-\rho v^{2}\sin \varphi -\frac{M(0)-M(L)}{\int _{0}^{L}\cos \varphi dS}\cos \varphi . \end{aligned}$$
(11)

Next, we introduce constitutive equations for the nanotube. Our intent is to address both Eringen’s two-phase local/nonlocal model and Eringen’s differential model.

2.1 Eringen’s two-phase local/nonlocal model

First, we adopt a model that takes into account both local and nonlocal elasticity i.e., Eringen’s two-phase local/nonlocal model [4, 6, 49,50,51]. This model consists of the convex mixture of local and nonlocal phases and is widely used as mentioned before. In particular, this means that the normal stress in a cross section of the nanotube is given by

$$\begin{aligned} \sigma (S)=\zeta E\varepsilon (S)+\frac{(1-\zeta )}{2L_{c}} \int \limits _{0}^{L}e^{-\frac{\left| S-\xi \right| }{L_{c}} }E\varepsilon (\xi )d\xi , \end{aligned}$$
(12)

where \(0<\zeta \le 1\) is the phase parameter, \(L_{c}>0\) is the small length scale parameter, E is the modulus of elasticity, and \(\varepsilon \) is the normal strain. We note that in (12) the Helmholtz kernel \(e^{- \frac{\left| S-\xi \right| }{L_{c}}}\) is used. Following the standard procedure, as presented in [11], Eq. (12) can be adapted to the theory of inextensible and unshearable nanorods with constant cross-sections. In particular, we obtain

$$\begin{aligned} M(S)=\zeta EI\kappa (S)+\frac{(1-\zeta )}{2L_{c}}\int \limits _{0}^{L}e^{- \frac{\left| S-\xi \right| }{L_{c}}}EI\kappa (\xi )\textrm{d}\xi , \end{aligned}$$
(13)

where I is the second moment of inertia and \(\kappa \) is the curvature of the rod axis. We note that (13) is in agreement with [23, 25]. As shown in [9, 52], the constitutive Eq. (13) is equivalent to

$$\begin{aligned} M-L_{c}^{2}M^{\prime \prime }=EI\kappa -\zeta L_{c}^{2}EI\kappa ^{\prime \prime }. \end{aligned}$$
(14)

subject to

$$\begin{aligned} M(0)-L_{c}M^{\prime }(0)= & \zeta EI\kappa (0)-\zeta L_{c}EI\kappa ^{\prime }(0), \nonumber \\ M(L)+L_{c}M^{\prime }(L)= & \zeta EI\kappa (L)+\zeta L_{c}EI\kappa ^{\prime }(L). \end{aligned}$$
(15)

Accounting for the definition of the curvature of the inextensible nanotube axis

$$\begin{aligned} \kappa =\varphi ^{\prime }, \end{aligned}$$
(16)

and then substituting (16) into (14) and (15) we obtain

$$\begin{aligned} M-L_{c}^{2}M^{\prime \prime }=EI\varphi ^{\prime }-\zeta L_{c}^{2}EI\varphi ^{\prime \prime \prime }, \end{aligned}$$
(17)

subject to

$$\begin{aligned} M(0)-L_{c}M^{\prime }(0)= & \zeta EI\varphi ^{\prime }(0)-\zeta L_{c}EI\varphi ^{\prime \prime }(0), \nonumber \\ M(L)+L_{c}M^{\prime }(L)= & \zeta EI\varphi ^{\prime }(L)+\zeta L_{c}EI\varphi ^{\prime \prime }(L). \end{aligned}$$
(18)

Next, we transform Eqs. (8)\(_{3,6},\) (11), (17) and (18) into suitable forms for bifurcation analysis. To that end, we first define new quantities

$$\begin{aligned} K=M-\zeta EI\varphi ^{\prime },\ \ \ \ \ r=M(0)-M(L),\ \ \ \ \ \ Q=K^{\prime }. \end{aligned}$$
(19)

With the help of (19), Eqs. (8)\(_{3,6},\) (11), ( 17) and (18) become the fifth-order system of ordinary differential equations

$$\begin{aligned} M^{\prime }= & -\rho v^{2}\sin \varphi -\frac{r\cos \varphi }{ \int _{0}^{L}\cos \varphi \textrm{d}S}, \nonumber \\ K^{\prime }= & Q, \nonumber \\ \varphi ^{\prime }= & \frac{M-K}{\zeta EI}, \nonumber \\ Q^{\prime }= & \frac{\left( \zeta -1\right) M+K}{\zeta L_{c}^{2}}, \nonumber \\ r^{\prime }= & 0, \end{aligned}$$
(20)

subject to

$$\begin{aligned} \varphi (0)=0,~\ \varphi (L)=0,\ \ K(0)=L_{c}Q(0),\ \ K(L)=-L_{c}Q(L),\ \ r(0)=M(0)-M(L). \end{aligned}$$
(21)

If we introduce the following dimensionless quantities

$$\begin{aligned} t= & \frac{S}{L},\ \overline{M}=\frac{ML}{EI},\ \overline{K}=\frac{KL}{EI},\ \overline{Q}=\frac{QL^{2}}{EI}, \nonumber \\ \mu= & \frac{\rho v^{2}L^{2}}{EI}, \overline{r}=\frac{rL}{EI},\ l_{c}=\frac{L_{c}}{L},\ \overline{x}= \frac{x}{L},\ \overline{y}=\frac{y}{L}, \end{aligned}$$
(22)

Equations (20) and (21) become

$$\begin{aligned} \overset{{\cdot }}{{\overline{M}}}= & -\mu \sin \varphi -\frac{\overline{r}\cos \varphi }{\int _{0}^{1}\cos \varphi \textrm{d}t}, \nonumber \\ \overset{{\cdot }}{{\overline{K}}}= & \overline{Q}, \nonumber \\ \overset{{\cdot }}{\varphi }= & \frac{\overline{M}-\overline{K}}{\zeta }, \nonumber \\ \overset{{\cdot }}{{\overline{Q}}}= & \frac{\left( \zeta -1\right) \overline{M}+ \overline{K}}{\zeta l_{c}^{2}}, \nonumber \\ \overset{{\cdot }}{{\overline{r}}}= & 0, \end{aligned}$$
(23)

subject to

$$\begin{aligned} \varphi (0)=0,~\ \ \varphi (1)=0,\ \ \ \overline{K}(0)=l_{c}\overline{Q} (0),\ \ \ \overline{K}(1)=-l_{c}\overline{Q}(1),\ \ \ \overline{r}(0)= \overline{M}(0)-\overline{M}(1), \end{aligned}$$
(24)

where the notation \(\frac{d(\cdot )}{\textrm{d}t}=\overset{{\cdot }}{(\cdot )}\) is used. We note that for \(\zeta =1\) we cover the classical Bernoulli–Euler theory since in this case (23)\(_{2,4}\) and (24)\(_{3,4}\) imply \( \overline{Q}=\overline{K}=0\), which transforms (23) and (24) into

$$\begin{aligned} \overset{{\cdot }}{{\overline{M}}}= & -\mu \sin \varphi -\frac{\overline{r}\cos \varphi }{\int _{0}^{1}\cos \varphi \textrm{d}t}, \nonumber \\ \overset{{\cdot }}{\varphi }= & \overline{M}, \nonumber \\ \overset{{\cdot }}{{\overline{r}}}= & 0, \end{aligned}$$
(25)

subject to

$$\begin{aligned} \varphi (0)=0,~\ \ \varphi (1)=0,\ \ \overline{r}(0)=\overline{M}(0)- \overline{M}(1). \end{aligned}$$
(26)

In order to simplify the derivations, from now on we will assume that \(0<\zeta <1\). The results corresponding to the classical Bernoulli–Euler theory, follow as a special case when \(\zeta \rightarrow 1\). This can be checked by analyzing (25) and (26) instead of (23) and (24). Now, we put (23) and (24) into a compact form by defining a vector \(\textbf{x}=( \overline{M},\overline{K},\varphi ,\overline{Q},\overline{r})^{T}\) and two function spaces

$$\begin{aligned} \textbf{X}= & \Bigg \{ \textbf{x}:\textbf{x}\in C^{1}\left( \left[ 0,1\right] , \mathbb {R} ^{5}\right) ,\varphi (0)=0,~\varphi (1)=0,\ \Bigg . \nonumber \\ & \Bigg . \overline{K}(0)=l_{c}\overline{Q}(0),\ \overline{K}(1)=- l_{c}\overline{Q}(1),\ \overline{r}(0)=\overline{M}(0)-\overline{M}(1)\Bigg \}, \nonumber \\ \textbf{Y}= & \left\{ \textbf{y}:\textbf{y}\in C\left( \left[ 0,1\right] , \mathbb {R} ^{5}\right) \right\} . \end{aligned}$$
(27)

These spaces are endowed with the usual sup norms [53,54,55]. Now, we can construct the operator \({\varvec{\Phi }}:\textbf{U}\times \mathbb {R} _{+} \rightarrow \textbf{Y}\) as

$$\begin{aligned} {\varvec{\Phi }} \mathbf{(x,}\mu \mathbf{)=}\left\{ \begin{array}{c} \overset{{\cdot }}{{\overline{M}}}+\mu \sin \varphi +\frac{\overline{r}\cos \varphi }{\int _{0}^{1}\cos \varphi dt} \\ \overset{{\cdot }}{{\overline{K}}}-\overline{Q} \\ \overset{{\cdot }}{\varphi }-\frac{\overline{M}-\overline{K}}{\zeta } \\ \overset{{\cdot }}{{\overline{Q}}}-\frac{\left( \zeta -1\right) \overline{M}+ \overline{K}}{\zeta l_{c}^{2}} \\ \overset{{\cdot }}{{\overline{r}}} \end{array} \right. , \end{aligned}$$
(28)

where \(\textbf{U}\) is a small neighborhood of \(\textbf{x}=(0,0,0,0,0)^{T}\) in \(\mathbf{X.}\) With the help of (28), Eqs. (23) and (24) become

$$\begin{aligned} {\varvec{\Phi }} {\textbf {(x,}}{{\mu }} {\textbf {) = 0.}} \end{aligned}$$
(29)

which is the governing equation for Eringen’s two-phase local/nonlocal model.

2.2 Eringen’s differential model

In the case of Eringen’s differential model, the constitutive equation in dimensionless form reads [45, 56, 57]

$$\begin{aligned} \overline{M}-l_{c}^{2}\overset{{\cdot \cdot }}{{\overline{M}}} = \overset{{\cdot }}{ \varphi }, \end{aligned}$$
(30)

which along with (22), (23)\(_{1,5}\) and (24)\(_{1,2,5}\) leads to the dimensionless governing equations

$$\begin{aligned} \overset{{\cdot }}{{\overline{M}}}= & -\mu \sin \varphi -\frac{\overline{r}\cos \varphi }{\int _{0}^{1}\cos \varphi \textrm{d}t}, \nonumber \\ \overset{{\cdot }}{\varphi }= & \frac{\overline{M}}{1-l_{c}^{2}\left( \mu \cos \varphi -\frac{\overline{r}\sin \varphi }{\int _{0}^{1}\cos \varphi \textrm{d}t} \right) }, \nonumber \\ \overset{{\cdot }}{{\overline{r}}}= & 0, \end{aligned}$$
(31)

subject to

$$\begin{aligned} \varphi (0)=0,~\ \varphi (1)=0,\ \ \overline{r}(0)=\overline{M}(0)-\overline{ M}(1). \end{aligned}$$
(32)

After defining a vector \(\textbf{z}=(\overline{M},\varphi ,\overline{r})^{T}\) and two function spaces

$$\begin{aligned} \textbf{Z}= & \left\{ \mathbf{z:z}\in C^{1}([0,1], \mathbb {R} ^{3}),\ \varphi (0)=0,~\varphi (1)=0,\ \overline{r}(0)=\overline{M}(0)- \overline{M}(1)\right\} , \nonumber \\ \textbf{W}= & \left\{ \mathbf{w:w}\in C([0,1], \mathbb {R} ^{3})\right\} , \end{aligned}$$
(33)

endowed with the usual sup norms we introduce the operator \( {\varvec{\Psi }}:\textbf{V}\times \mathbb {R} _{+}\rightarrow \textbf{W}\) as

$$\begin{aligned} {\varvec{\Psi }} \mathbf{(z,}\mu \mathbf{)=}\left\{ \begin{array}{c} \overset{{\cdot }}{{\overline{M}}}+\mu \sin \varphi +\frac{\overline{r}\cos \varphi }{\int _{0}^{1}\cos \varphi \textrm{d}t} \\ \overset{{\cdot }}{\varphi }-\frac{\overline{M}}{1-l_{c}^{2}\left( \mu \cos \varphi -\frac{\overline{r}\sin \varphi }{\int _{0}^{1}\cos \varphi dt} \right) } \\ \overset{{\cdot }}{{\overline{r}}} \end{array} \right. , \end{aligned}$$
(34)

where \(\textbf{V}\) presents a small neighborhood of \(\textbf{z}=(0,0,0)^{T}\) in\( \mathbf{Z.}\) Now, the system given by (31) and (32) is of the form

$$\begin{aligned} {\varvec{\Psi }} {{\textbf {(z}}},{{\mu }} {{\textbf {)}}} = \textbf{0}. \end{aligned}$$
(35)

which presents the governing equation for Eringen’s differential model.

3 Bifurcation analysis

In this section, we perform a local bifurcation analysis for the two models of the nanotube. For a bifurcation parameter, we choose \(\mu \).

3.1 Eringen’s two-phase local/nonlocal model

Before proceeding further, it is worth mentioning that the operator \({ {\varvec{\Phi }} }\) possesses two interesting properties

$$\begin{aligned} {\varvec{\Phi }} \mathbf{(0,}\mu \mathbf{)=0}\ \ \forall \mu ,\ \ \ \ \ \ \ \ \ \ \ \ {\varvec{\Phi }} \mathbf{(-x,} \mu \mathbf{)}=-{\varvec{\Phi }} \mathbf{(x,}\mu \mathbf{)}. \end{aligned}$$
(36)

Equation (36)\(_{1}\) means that \(\mathbf{x=0}\) is a trivial solution. In particular, our goal in this section is to find two things: the values of \( \mu \) at which nontrivial solutions branch off from the trivial solution (bifurcation points) and the type of bifurcation. As a consequence, we have to solve the equation

$$\begin{aligned} {\varvec{\Phi }} \mathbf{(x,}\mu \mathbf{)}=\textbf{0,} \end{aligned}$$
(37)

in the neighborhood of \(\mathbf{x=0}\). As the first step in that direction, the continuous linear operator \(\textbf{L}(\mu ):\textbf{X}\rightarrow \textbf{Y}\) is defined as

$$\begin{aligned} \textbf{L}(\mu )\textbf{x}=D_{\textbf{x}}{\varvec{\Phi }} \mathbf{(0,}\mu \mathbf{)x=}\left\{ \begin{array}{c} \overset{{\cdot }}{{\overline{M}}}+\mu \varphi +\overline{r} \\ \overset{{\cdot }}{\overline{K}}-\overline{Q} \\ \overset{{\cdot }}{\varphi }-\frac{\overline{M}-\overline{K}}{\zeta } \\ \overset{{\cdot }}{\overline{Q}}-\frac{\left( \zeta -1\right) \overline{M}+ \overline{K}}{\zeta l_{c}^{2}} \\ \overset{{\cdot }}{\overline{r}} \end{array} \right. , \end{aligned}$$
(38)

where \(D_{\textbf{x}} {\varvec{\Phi }} \mathbf{(0,}\mu \mathbf{)}\) is the Fréchet derivative of \({ {\varvec{\Phi }} }\) with respect to \(\textbf{x}\)at \(\mathbf{x=0.}\) Next, we solve the equation

$$\begin{aligned} \textbf{L}(\mu )\mathbf{x=0}. \end{aligned}$$
(39)

From this equation we get

$$\begin{aligned} \zeta l_{c}^{2}\overset{{\cdot \cdot \cdot \cdot }}{{\overline{M}}}+\left( \mu l_{c}^{2}-1\right) \overset{{\cdot \cdot }}{{\overline{M}}}-\mu \overline{M}=0, \end{aligned}$$
(40)

subject to

$$\begin{aligned} \overset{{\cdot }}{{\overline{M}}}(0)-\overset{{\cdot }}{{\overline{M}}}(1)= & 0, \nonumber \\ \overset{{\cdot }}{{\overline{M}}}(0)+\overline{M}(0)-\overline{M}(1)= & 0, \nonumber \\ \mu \overline{M}(0)+\zeta \overset{{\cdot \cdot }}{{\overline{M}}}(0)-\mu l_{c} \overset{{\cdot }}{{\overline{M}}}(0)-\zeta l_{c}\overset{{\cdot \cdot \cdot }}{{ \overline{M}}}(0)= & 0, \nonumber \\ \mu \overline{M}(1)+\zeta \overset{{\cdot \cdot }}{{\overline{M}}}(1)+\mu l_{c} \overset{{\cdot }}{{\overline{M}}}(1)+\zeta l_{c}\overset{{\cdot \cdot \cdot }}{{ \overline{M}}}(1)= & 0. \end{aligned}$$
(41)

We note that both operators determined by (39) and by (40), (41) lead to the same necessary condition for the existence of a nontrivial solution to (39). Also, both operators are non-self-adjoint.

Before continuing we comment on the linear problem (40), (41) to provide it with additional physical meaning. Namely, this problem can be used for linear analysis of a compressed clamped nanotube with Eringen’s two-phase local/nonlocal model if the dimensionless velocity \(\mu \) is identified with a dimensionless compressive force. To see that we linearize (6)\(_{2}\) and then use the dimensionless quantities (22) to get

$$\begin{aligned} \overset{{\cdot }}{{\overline{y}}}=\varphi . \end{aligned}$$

Now, from (39) we obtain

$$\begin{aligned} \overline{M}=-\mu \overline{y}-\overline{r}t-C_{5}, \end{aligned}$$

where \(C_{5}\) is an arbitrary constant. Using the above equation and the boundary conditions (8)\(_{2,5},\) the system (40), (41) can be transformed into

$$\begin{aligned} \overset{{\cdot \cdot \cdot \cdot }}{{\overline{y}}}+\left( \frac{\mu }{\zeta }- \frac{1}{\zeta l_{c}^{2}}\right) \overset{{\cdot \cdot }}{{\overline{y}}}-\frac{ \mu }{\zeta l_{c}^{2}}\overline{y}=\frac{r}{\zeta l_{c}^{2}}t+\frac{C_{5}}{ \zeta l_{c}^{2}}, \end{aligned}$$
(42)

subject to

$$\begin{aligned} \overline{y}(0)= & \overline{y}(1)=\overset{{\cdot }}{\overline{y}}(0)=\overset{\cdot }{\overline{y}}(1)=0, \nonumber \\ \overset{{\cdot \cdot \cdot }}{{\overline{y}}}(0)-\frac{1}{l_{c}}\overset{{\cdot \cdot }}{{\overline{y}}}(0)= & -\frac{1}{\zeta }\left\{ \mu \overset{{\cdot }}{ \overline{y}}(0)+r-\frac{\mu \overline{y}(0)+C_{5}}{l_{c}}\right\} , \nonumber \\ \overset{{\cdot \cdot \cdot }}{{\overline{y}}}(1)+\frac{1}{l_{c}}\overset{{\cdot \cdot }}{{\overline{y}}}(1)= & -\frac{1}{\zeta }\left\{ \mu \overset{{\cdot }}{ \overline{y}}(1)+r+\frac{\mu \overline{y}(1)+r+C_{5}}{l_{c}}\right\} . \end{aligned}$$
(43)

The system (42), (43) has the same form as the system (25), (27) and (30) obtained in [18], where the linear analysis of a compressed nanotube clamped at both ends is performed for Eringen’s two-phase local/nonlocal model.

In order to solve (40) and (41), we first introduce a new independent variable

$$\begin{aligned} \overline{t}=t-1/2, \end{aligned}$$
(44)

to obtain the following

$$\begin{aligned} \zeta l_{c}^{2}\frac{d^{4}\overline{M}}{\textrm{d}\overline{t}^{4}}+\left( \mu l_{c}^{2}-1\right) \frac{d^{2}\overline{M}}{\textrm{d}\overline{t}^{2}}-\mu \overline{ M}=0, \end{aligned}$$
(45)

subject to

$$\begin{aligned} \frac{\textrm{d}\overline{M}}{\textrm{d}\overline{t}}(-1/2)-\frac{\textrm{d}\overline{M}}{\textrm{d}\overline{t}} (1/2)= & 0, \nonumber \\ \frac{d\overline{M}}{\textrm{d}\overline{t}}(-1/2)+\overline{M}(-1/2)-\overline{M} (1/2)= & 0, \nonumber \\ \mu \overline{M}(-1/2)+\zeta \frac{d^{2}\overline{M}}{\textrm{d}\overline{t}^{2}} (-1/2)-\mu l_{c}\frac{\textrm{d}\overline{M}}{\textrm{d}\overline{t}}(-1/2)-\zeta l_{c}\frac{ d^{3}\overline{M}}{\textrm{d}\overline{t}^{3}}(-1/2)= & 0, \nonumber \\ \mu \overline{M}(1/2)+\zeta \frac{d^{2}\overline{M}}{\textrm{d}\overline{t}^{2}} (1/2)+\mu l_{c}\frac{\textrm{d}\overline{M}}{\textrm{d}\overline{t}}(1/2)+\zeta l_{c}\frac{ d^{3}\overline{M}}{\textrm{d}\overline{t}^{3}}(1/2)= & 0. \end{aligned}$$
(46)

The general solution to (45) reads

$$\begin{aligned} \overline{M}=C_{1}\cosh \gamma _{1}\overline{t}+C_{2}\sinh \gamma _{1} \overline{t}+C_{3}\cos \gamma _{2}\overline{t}+C_{4}\sin \gamma _{2} \overline{t}, \end{aligned}$$
(47)

where \(C_{i}\ i=1,\ldots ,4\) are arbitrary constants and

$$\begin{aligned} \gamma _{1,2}=\sqrt{\frac{\mp \left( \mu l_{c}^{2}-1\right) +\sqrt{\left( \mu l_{c}^{2}-1\right) ^{2}+4\zeta \mu l_{c}^{2}}}{2\zeta l_{c}^{2}}}. \end{aligned}$$
(48)

After substituting (47) into (45) and using (46) we obtain the condition that ensures the existence of a nontrivial solution of ( 45) and (46), i.e., the characteristic equation

$$\begin{aligned} A_{1}A_{2}=0, \end{aligned}$$
(49)

where

$$\begin{aligned} A_{1}= & \beta \left( \alpha ^{2}\zeta +\mu \right) \sin \frac{\beta }{2} +\alpha \left[ \left( \mu -\beta ^{2}\zeta \right) \cos \frac{\beta }{2} +\beta l_{c}\zeta \left( \alpha ^{2}+\beta ^{2}\right) \sin \frac{\beta }{2} \right] \tanh \frac{\alpha }{2}, \nonumber \\ A_{2}= & \alpha \left\{ l_{c}\zeta \left( \alpha ^{2}+\beta ^{2}\right) \cos \frac{\beta }{2}-\left[ \zeta \left( 2\alpha ^{2}l_{c}-\beta ^{2}\right) +\mu \left( 1+2l_{c}\right) \right] \sin \frac{\beta }{2}\right\} \nonumber \\ & +\left\{ \beta \left[ \mu \left( 1+2l_{c}\right) +\zeta \left( \alpha ^{2}-2\beta ^{2}l_{c}\right) \right] \cos \frac{\beta }{2}-2\zeta \left( \alpha ^{2}+\beta ^{2}\right) \sin \frac{\beta }{2}\right\} \tanh \frac{ \alpha }{2}. \end{aligned}$$
(50)

It is worth noting that the characteristic Eq. (49) is in agreement with the characteristic Eq. (40) derived by [18], however (49) has more tractable form. Given \(\zeta \) and \(l_{c},\) the values of \(\mu \) satisfying (49) will be called eigenvalues and will be denoted by \(\mu _{n}^{t},\) \(n\in \mathbb {N} \). Numerical analysis suggests that the set of all eigenvalues is countably infinite and that the odd values of n correspond to \( A_{1}=0\) while the even values of n correspond to \(A_{2}=0.\) Hereafter, we refer to \(\mu _{1}^{t}\) and \(\mu _{2}^{t}\) as the lowest and second lowest eigenvalues, respectively. The solutions to (39), corresponding to \(\mu =\mu _{n}^{t}\), follow from (40), (41) and (44)–(48) as

$$\begin{aligned} \textbf{x}_{n}=B_{1n}\left\{ \begin{array}{c} \overline{M}_{n} \\ \overline{K}_{n} \\ \varphi _{n} \\ \overline{Q}_{n} \\ \overline{r}_{n} \end{array} \right\} =B_{1n}\left\{ \begin{array}{c} g(t) \\ g+\frac{\zeta }{\mu _{n}}\overset{{\cdot \cdot }}{g} \\ -\frac{\overset{{\cdot }}{g}+g(0)-g(1)}{\mu _{n}} \\ \overset{{\cdot }}{g}+\frac{\zeta }{\mu _{n}}\overset{{\cdot \cdot \cdot }}{g} \\ g(0)-g(1) \end{array} \right\} , \end{aligned}$$
(51)

where \(B_{1n}\) are arbitrary constants and the function g(t) is of the form

$$\begin{aligned} g(t)=\left\{ \begin{array}{c} \frac{\beta \text {csch}\left( \frac{\alpha }{2}\right) \sin \left( \frac{ \beta }{2}\right) }{\alpha }\cosh \left[ \alpha \left( t-\frac{1}{2}\right) \right] +\cos \left[ \beta \left( t-\frac{1}{2}\right) \right] \ \ \ \ \ \ if\ \ \ A_{1}=0 \\ \frac{\left[ 2\sin \left( \frac{\beta }{2}\right) -\beta \cos \left( \frac{ \beta }{2}\right) \right] }{\alpha \cosh \left( \frac{\alpha }{2}\right) -2\sinh \left( \frac{\alpha }{2}\right) }\sinh \left[ \alpha \left( t-\frac{1 }{2}\right) \right] +\sin \left[ \beta \left( t-\frac{1}{2}\right) \right] \ \ if\ \ \ A_{2}=0 \end{array} \right. . \end{aligned}$$
(52)

We note that numerical analysis shows that if (49) holds then the case \(A_{1}=A_{2}=0\) is not observed for the values of the parameters under consideration. Equations (51) and (52) reveal the following

$$\begin{aligned} g(t)=\left\{ \begin{array}{c} g(1-t)\ \ \ \ \textrm{if}\ \ \ \ \ \ \ A_{1}=0 \\ -g(1-t)\ \ \ \ \textrm{if}\ \ \ \ \ \ \ A_{2}=0 \end{array} \right. , \end{aligned}$$

which in turn leads to the symmetry and antisymmetry of solutions of the linear problem (51). In particular, we get

$$\begin{aligned} \begin{array}{c} \overline{M}_{n}(t)=\overline{M}_{n}(1-t) \\ \overline{K}_{n}(t)=\overline{K}_{n}(1-t)\ \\ \varphi _{n}(t)=-\varphi _{n}(1-t) \\ \overline{Q}_{n}(t)=-\overline{Q}_{n}(1-t) \\ \overline{r}_{n}(t)=0 \end{array} \ \ \ \ \ \ \ \textrm{if}\ \ \ \ \ \ \ A_{1}=0, \end{aligned}$$

and

$$\begin{aligned} \begin{array}{c} \overline{M}_{n}(t)=-\overline{M}_{n}(1-t)\ \\ \overline{K}_{n}(t)=-\overline{K}_{n}(1-t) \\ \varphi _{n}(t)=\varphi _{n}(1-t) \\ \overline{Q}_{n}(t)=\overline{Q}_{n}(1-t) \\ \overline{r}_{n}(t)=2g(0)=2\overline{M}_{n}(0) \end{array} \ \ \ \ \ \ \ \textrm{if}\ \ \ \ \ \ \ A_{2}=0. \end{aligned}$$

From (51) and the preceding observations, we can conclude that if \( A_{1}=A_{2}=0\) is not satisfied, then the dimension of the null space of \( \textbf{L}(\mu _{n}^{t})\) is one, i.e., dim \(N(\textbf{L}(\mu _{n}^{t}))=1.\) Next, we define an inner product on \(\textbf{Y}\) in the following way

$$\begin{aligned} \left\langle \textbf{y}_{1},\textbf{y}_{2}\right\rangle =\int \limits _{0}^{1} \textbf{y} _{1}^{T} \textbf{y}_{2}\textrm{d}t\ \ \ \ \ \ \ \ \ \ \forall \ \textbf{y}_{1},\textbf{y}_{2}\in \mathbf{Y.} \end{aligned}$$
(53)

This definition allows us to introduce the adjoint \(\textbf{L}^{*}(\mu )\) of \(\textbf{L}(\mu )\) as

$$\begin{aligned} \left\langle \textbf{L}(\mu )\textbf{x},\textbf{q}\right\rangle =\left\langle \textbf{x}, \textbf{L}^{*}(\mu )\textbf{q}\right\rangle , \end{aligned}$$
(54)

where \(\textbf{q}=(q_{m},q_{k},q_{\varphi },q_{q},q_{r})^{T}\in \textbf{Y}\). Our next aim is to solve an adjoint problem, i.e., the operator equation

$$\begin{aligned} \textbf{L}^{*}(\mu )\mathbf{q=0.} \end{aligned}$$
(55)

Keeping (53) and (54) in mind the adjoint problem (55) can be written in the form

$$\begin{aligned} \overset{{\cdot }}{q}_{m}= & -\frac{q_{\varphi }}{\zeta }-\frac{(\zeta -1)q_{q} }{\zeta l_{c}^{2}}, \nonumber \\ \overset{{\cdot }}{q}_{k}= & \frac{q_{\varphi }}{\zeta }-\frac{q_{q}}{\zeta l_{c}^{2}}, \nonumber \\ \overset{{\cdot }}{q}_{\varphi }= & \mu q_{m}, \nonumber \\ \overset{{\cdot }}{q}_{q}= & -q_{k}, \nonumber \\ \overset{{\cdot }}{q}_{r}= & q_{m}, \end{aligned}$$
(56)

subject to

$$\begin{aligned} q_{r}(0)+q_{m}(0)= & 0, \nonumber \\ q_{r}(0)+q_{m}(1)= & 0, \nonumber \\ l_{c}q_{k}(0)+q_{q}(0)= & 0, \nonumber \\ l_{c}q_{k}(1)-q_{q}(1)= & 0, \nonumber \\ q_{r}(1)= & 0. \end{aligned}$$
(57)

By comparing (39) to Eqs. (56) and (57), we can draw a conclusion that the solution to the adjoint problem reads

$$\begin{aligned} \textbf{q}_{n}=B_{2n}\left\{ \begin{array}{c} q_{mn} \\ q_{kn} \\ q_{\varphi n} \\ q_{qn} \\ q_{rn} \end{array} \right\} =B_{2n}\left\{ \begin{array}{c} \varphi _{n} +\frac{\overline{r}_{n}}{\mu _{n}^{t}} \\ -\frac{l_{c}^{2}\overline{Q}_{n}}{\zeta -1} \\ -\overline{M}_{n} \\ \frac{l_{c}^{2}\overline{K}_{n}}{\zeta -1} \\ \frac{1}{\mu _{n}^{t}}\left[ \overline{M}_{n}(1)-\overline{M}_{n}\right] \end{array} \right\} , \end{aligned}$$
(58)

where \(B_{2n}\) are arbitrary constants. From (51) and (58), it follows that the dimension of the null space of the adjoint operator \(\textbf{L} ^{*}(\mu _{n}^{t})\) is also one, i.e., dim \(N(\textbf{L}^{*}(\mu _{n}^{t}))=1.\) Let \(\textbf{q}^{*}\) be a nonzero element of the null space of the adjoint operator \(\textbf{L}^{*}(\mu _{n}^{t}).\) Then, by using the Fredholm alternative [58, 59], the range of \( \textbf{L}(\mu _{n}^{t})\) can be characterized by

$$\begin{aligned} R(\textbf{L}(\mu _{n}^{t}))=\left\{ \textbf{y}:\textbf{y}\in \textbf{Y,} \int \limits _{0}^{1} \textbf{y}^{T} \textbf{q}^{*}dt=0\right\} . \end{aligned}$$
(59)

This allows the splitting of the space \(\textbf{Y}\) as \(\textbf{Y}=\) \(N(\textbf{L} ^{*}(\mu _{n}^{t}))\oplus R(\textbf{L}(\mu _{n}^{t}))\). As a consequence, codim \(R(\textbf{L}(\mu _{n}^{t}))=1<\infty \) implying that \(\textbf{L}(\mu _{n}^{t})\) is the Fredholm operator with index zero which, in turn, makes it possible to use the method of Lyapunov–Schmidt [60,61,62,63,64,65]. In particular, we first set \(\mu =\mu _{n}^{t}+\Delta \mu \) in (37) and assume the solution to (37) in the form

$$\begin{aligned} \textbf{x}=a\textbf{x}_{n} \mathbf{+u}(a\textbf{x}_{n},\Delta \mu ), \end{aligned}$$
(60)

where \(\textbf{u}(a\textbf{x}_{n},\Delta \mu )\) belongs to the closed complement of \(N(\textbf{L}(\mu _{n}^{t}))\) in \(\textbf{X}\) and a is a real parameter. Substituting (60) into (37) and then projecting (37) onto \(N(\textbf{L}^{*}(\mu _{n}^{t}))\) along \(R(\textbf{L}(\mu _{n}^{t}))\) we get the following bifurcation equation

$$\begin{aligned} \Gamma (a,\Delta \mu )=\int _{0}^{1}{ {\varvec{\Phi }} (}a \mathbf{x_{n}+u(}a\textbf{x}_{n} \textbf{,}\Delta \mu \mathbf{),}\mu _{n}^{t}+\Delta \mu \mathbf{)}^{T} \textbf{q}_{n}\textrm{d}t=0. \end{aligned}$$
(61)

Using (59) and \(\int _{0}^{1}\varphi _{n}\textrm{d}t=0\), the Taylor expansion of the bifurcation Eq. (61) in the neighborhood of \((a,\Delta \mu )=(0,0)\) yields

$$\begin{aligned} \Gamma (a,\Delta \mu )=b_{1n}a\Delta \mu +b_{3n}a^{3}+O(a^{5},a^{3}\Delta \mu ,a(\Delta \mu )^{2})=0, \end{aligned}$$
(62)

where the coefficients read

$$\begin{aligned} b_{1n}= & \int _{0}^{1}\varphi _{n}^{2}\textrm{d}t>0, \nonumber \\ b_{3n}= & -\int _{0}^{1}\left( \mu _{n}^{t}\frac{\varphi _{n}^{4}}{6}+\frac{2 \overline{r}_{n}\varphi _{n}^{3}}{3}\right) \textrm{d}t. \end{aligned}$$
(63)

If \(A_{1}=0\), then \(\overline{r}_{n}=0\) which leads to

$$\begin{aligned} b_{3n}=-\int _{0}^{1}\mu _{n}^{t}\frac{\varphi _{n}^{4}}{6}\textrm{d}t\ <0. \end{aligned}$$
(64)

Taking into account (36)\(_{2}\), the bifurcation Eq. (62) becomes

$$\begin{aligned} \Gamma (a,\Delta \lambda )=a\left[ b_{1n}\Delta \mu +b_{3n}a^{2}+O(a^{4},a^{2}\Delta \mu , (\Delta \mu )^{2})\right] =0, \end{aligned}$$

which after the use of the implicit function theorem yields the solution that bifurcates from the trivial one

$$\begin{aligned} \Delta \mu =-\frac{b_{3n}}{b_{1n}}a^{2}+O(a^{4}). \end{aligned}$$
(65)

Thus, if \(b_{1n}\ne 0\), \(b_{3n}\ne 0,\) then (65) leads to the main conclusion of this section that at \((\textbf{x},\mu )=(\textbf{0},\mu _{n}^{t}) \) pitchfork bifurcations occur. It is noteworthy that the application of the Lyapunov–Schmidt method to the classical Bernoulli–Euler case, described by (25) and (26), yields the same form of the bifurcation equation as the one given by (62) and (63) since the parameters \( l_{c}\) and \(\zeta \) do not appear explicitly in (63).

3.2 Eringen’s differential model

In the case of the nanotube described by Eringen’s differential model, the governing equation is given by (35). We remark that the operator \({ {\varvec{\Psi }} }\) satisfies equations which are analogous to (36). Since the bifurcation analysis of (35) requires the same procedure as in the case of Eringen’s two-phase local/nonlocal model, we will present only the main results. First, taking the Fréchet derivative of (35), the following is obtained

$$\begin{aligned} \overset{{\cdot }}{{\overline{M}}}= & -\mu \varphi -\overline{r}, \nonumber \\ \overset{{\cdot }}{\varphi }= & \frac{\overline{M}}{1-\mu l_{c}^{2}}, \nonumber \\ \overset{{\cdot }}{\overline{r}}= & 0, \end{aligned}$$
(66)

subject to

$$\begin{aligned} \varphi (0)=0,~\varphi (1)=0,\ \ \overline{r}(0)=\overline{M}(0)-\overline{M} (1). \end{aligned}$$
(67)

From (66) to (67), we obtain

$$\begin{aligned} \overset{{\cdot \cdot }}{{\overline{M}}}+\frac{\mu }{1-\mu l_{c}^{2}}\overline{M} =0, \end{aligned}$$
(68)

subject to

$$\begin{aligned} \overset{{\cdot }}{{\overline{M}}}(0)-\overline{M}(1)+\overline{M}(0)= & 0, \nonumber \\ \overset{{\cdot }}{{\overline{M}}}(1)-\overline{M}(1)+\overline{M}(0)= & 0. \end{aligned}$$
(69)

Applying a procedure similar to that used for Eringen’s two-phase local/nonlocal model to the system (68) and (69), we can transform it into

$$\begin{aligned} \overset{{\cdot \cdot }}{{\overline{y}}}+\frac{\mu }{1-\mu l_{c}^{2}}\overline{y} =-\frac{1}{1-\mu l_{c}^{2}}\left( rt+C_{6}\right) , \end{aligned}$$
(70)

subject to

$$\begin{aligned} \overline{y}(0)=\overline{y}(1)=\overset{{\cdot }}{\overline{y}}(0)=\overset{{ \cdot }}{{\overline{y}}}(1)=0 \end{aligned}$$
(71)

where \(C_{6}\) is an arbitrary constant. Now, (71) and the twice differentiated form of Eq. (70) are equivalent to Eqs. (22) and (24) obtained by [32] in the case Bernoulli–Euler theory. This confirms that linear analysis of a compressed nanotube clamped at both ends is equivalent to the one performed in this paper in the case of Eringen’s differential model.

As in the case of Eringen’s two-phase local/nonlocal model, linearization of the nonlinear operator (34) leads to the linear non-self-adjoint operator defined by (66) and (67). In contrast, the linear operator determined by (68) and (69) is self-adjoint, unlike (40) and (41), and provides a necessary condition for the existence of a nontrivial solution to (66) and (67) in the form

$$\begin{aligned} G_{1}G_{2}=0, \end{aligned}$$
(72)

where

$$\begin{aligned} G_{1}=\sin \left( \frac{\sqrt{\chi }}{2}\right) ,\ \ G_{2}=\chi \cos \left( \frac{\sqrt{\chi }}{2}\right) -2\sqrt{\chi }\sin \left( \frac{\sqrt{\chi }}{2 }\right) ,\ \ \chi =\frac{\mu }{1-\mu l_{c}^{2}}. \end{aligned}$$
(73)

The values of \(\mu \) satisfying (72) are again eigenvalues and are denoted by \(\mu _{n}^{d},\) \(n\in \mathbb {N} \). The odd values of n correspond to \(G_{1}=0\), while even values of n correspond to \(G_{2}=0\). It is worth noting that \(G_{1}=0\) is in agreement with Eq. (35) derived by [32] in the case Bernoulli–Euler theory. Again, \(\mu _{1}^{d}\) and \(\mu _{2}^{d}\) stand for the lowest eigenvalue and the second lowest eigenvalue, respectively. If \(\mu =\mu _{n}^{d}\), then the solutions to Eqs. (66) and (67) are of the form

$$\begin{aligned} \textbf{z}_{n}=H_{1n}\left\{ \begin{array}{c} \overline{M}_{n} \\ \varphi _{n} \\ \overline{r}_{n} \end{array} \right\} =H_{1n}\left\{ \begin{array}{c} f(t) \\ -\frac{\overset{{\cdot }}{f}+f(0)-f(1)}{\mu _{n}^{d}} \\ f(0)-f(1) \end{array} \right\} , \end{aligned}$$
(74)

where \(H_{1n},\) \(n\in \mathbb {N} \) are arbitrary constants and

$$\begin{aligned} f(t)=\left\{ \begin{array}{c} \cos \left[ \left( t-1/2\right) \sqrt{\chi }\right] \ \ \ \ \ \ \ \ if\ \ \ \ \ \ G_{1}=0 \\ \sin \left[ \left( t-1/2\right) \sqrt{\chi }\right] \ \ \ \ \ \ \ \ \ if\ \ \ \ \ \ G_{2}=0 \end{array} \right. . \end{aligned}$$
(75)

In this case, the solutions to (66) and (67) satisfy

$$\begin{aligned} \begin{array}{c} \overline{M}_{n}(t)=\overline{M}_{n}(1-t)\ \\ \varphi _{n}(t)=-\varphi _{n}(1-t) \\ \overline{r}_{n}(t)=0 \end{array} \ \ \ \ \ \ \ \textrm{if}\ \ \ \ \ \ \ G_{1}=0, \end{aligned}$$
(76)

and

$$\begin{aligned} \begin{array}{c} \overline{M}_{n}(t)=-\overline{M}_{n}(1-t)\ \\ \varphi _{n}(t)=\varphi _{n}(1-t) \\ \overline{r}_{n}(t)=2f(0)=2\overline{M}_{n}(0) \end{array} \ \ \ \ \ \ \ \textrm{if}\ \ \ \ \ \ \ G_{2}=0. \end{aligned}$$
(77)

The Lyapunov–Schmidt method now yields the bifurcation equation

$$\begin{aligned} \Pi (a,\Delta \mu )=a\left[ c_{1n}\Delta \mu +c_{3n}a^{2}+O(a^{4},a^{2}\Delta \mu ,(\Delta \mu )^{2})\right] =0, \end{aligned}$$
(78)

where

$$\begin{aligned} c_{1n}= & \int _{0}^{1}\left[ \varphi _{n}^{2}+l_{c}^{2}(1+l_{c}^{2}\chi _{n})^{2}\overline{M}_{n}^{2}\right] \textrm{d}t>0, \nonumber \\ c_{3n}= & -\int _{0}^{1}\frac{3l_{c}^{2}\left( 1+l_{c}^{2}\chi _{n}\right) \overline{M}_{n}^{2}\varphi _{n}\left[ 2\left( 1+l_{c}^{2}\chi _{n}\right) \overline{r}_{n}+\chi _{n}\varphi _{n}\right] }{6}\textrm{d}t \nonumber \\ & -\int _{0}^{1}\frac{4\left( 1+l_{c}^{2}\chi _{n}\right) \overline{r} _{n}\varphi _{n}^{3}+\chi _{n}\varphi _{n}^{4}}{6\left( 1+l_{c}^{2}\chi _{n}\right) }\textrm{d}t. \end{aligned}$$
(79)

In the case of \(G_{1}=0\), the coefficient \(c_{3n}\) reduces to

$$\begin{aligned} c_{3n}=-\frac{\chi _{n}}{6\left( 1+l_{c}^{2}\chi _{n}\right) }\int _{0}^{1} \left[ 3l_{c}^{2}\left( 1+l_{c}^{2}\chi _{n}\right) ^{2}\overline{M} _{n}^{2}+\varphi _{n}^{2}\right] \varphi _{n}^{2}\textrm{d}t<0, \end{aligned}$$
(80)

since \(\overline{r}_{n}=0.\) Again, the implicit function theorem leads to the nontrivial branch

$$\begin{aligned} \Delta \mu =-\frac{c_{3n}}{c_{1n}}a^{2}+O(a^{4}), \end{aligned}$$
(81)

meaning that if \(c_{1n}\ne 0,c_{3n}\ne 0\) then pitchfork bifurcations occur at \((\textbf{z},\mu )=(\textbf{0},\mu _{n}^{d})\).

4 Discussion and results

In this section, we present the results concerning the critical buckling load, the type of bifurcation and the post-buckling shape of the nanotube described by the two types of constitutive equations introduced in Sect. 2.

4.1 Critical buckling load

In what follows, we refer to \(\mu _{1}^{t}\) as the critical buckling load of Eringen’s two-phase local/nonlocal model. In order to describe the influence of the parameters \( l_{c}\) and \(\zeta \) on the critical buckling load \(\mu _{1}^{t}\), we present Figs. 3, 4 and 5. These figures reveal that for a fixed value of \(l_{c}\) a decrease in \(\zeta \) (\(\zeta \) only tends to 0 and 1) causes a decrease in \(\mu _{1}^{t}\). On the other hand, \(\mu _{1}^{t}\) decreases as \(l_{c}\) increases from 0.01 to 0.2, with \( \zeta \) fixed. Figures 3, 4 and 5 suggest that when \(l_{c}\) approaches 0 or \( \zeta \) tends to 1, the critical buckling load \(\mu _{1}^{t}\) tends to \( 4\pi ^{2}\) which corresponds to the classical Bernoulli–Euler theory. From a physical point of view the above remarks mean that an increase in the nonlocal effect causes the nanotube to become less rigid. Therefore, the presence of softening effect is confirmed, i.e., the critical buckling load is reducing if the influence of nonlocality increases.

Fig. 3
figure 3

Dependence of the critical buckling load on the phase parameter \(\zeta \) for Eringen’s two-phase local/nonlocal model and \(l_{c}=0.01\)

Full size image
Fig. 4
figure 4

Dependence of the critical buckling load on the phase parameter \(\zeta \) for Eringen’s two-phase local/nonlocal model and \(l_{c}=0.1\)

Full size image
Fig. 5
figure 5

Dependence of the critical buckling load on the phase parameter \(\zeta \) for Eringen’s two-phase local/nonlocal model and \(l_{c}=0.2\)

Full size image

According to the above introduced terminology \(\mu _{1}^{d}\) is the critical buckling load of Eringen’s differential model. From \(G_{1}=0\), it follows that

$$\begin{aligned} \mu _{1}^{d}=\frac{\left( 2\pi \right) ^{2}}{1+\left( 2l_{c}\pi \right) ^{2}}. \end{aligned}$$
(82)

which is in agreement with the results presented by [30] and [32].

From the characteristic Eq. (49) and (82), we can numerically determine the value of the phase parameter \(\zeta =\zeta _{b}\) at which the critical buckling load for Eringen’s two-phase local/nonlocal model coincides with that for Eringen’s differential model. The values of \(\zeta _{b}\) for three values of the small length scale parameter \(l_{c}\in \{0.01,0.1,0.2\}\) are presented in Figs. 3, 4 and 5, respectively. These figures allow us to compare the critical buckling loads for the two models under consideration. Figure 3 shows that for \(\zeta \in (\zeta _{b},1)\) the critical buckling load for Eringen’s differential model is lower than that for Eringen’s two-phase local/nonlocal model whereas for \(\zeta \in (0,\zeta _{b})\) the opposite is true. Additionally, for small values of \(l_{c}\), Fig. 3 suggests that there are almost no differences between these two models.

For greater values of \(l_{c}\) (Figs. 4 and 5), the differences between the critical buckling loads of Eringen’s differential model and the ones corresponding to Eringen’s two-phase local/nonlocal model become significant and the interval \((\zeta _{b},1) \) enlarges. However, for all values of \(l_{c}\in \left\{ 0.01,0.1,0.2\right\} \) there exists an interval \((0,\zeta _{b})\) such that for \(\zeta \in (0,\zeta _{b})\) the critical buckling loads for Eringen’s two-phase local/nonlocal model is lower than the ones for Eringen’s differential model (Figs. 3, 4 and 5). In the authors’ opinion, this is a novelty which is important for engineers. It is worth mentioning that numerical investigation reveals that for all \( l_{c}\in (0,1)\) the aforementioned observations still hold.

As mentioned, numerical analysis of (49) and (50) leads to the conclusion that the second lowest eigenvalue \(\mu _{2}^{t}\) of Eringen’s two-phase local/nonlocal model is the lowest solution to \(A_{2}=0\) for \((l_{c},\zeta )\in (0,0.2]\times (0,1).\) Similarly, the second lowest eigenvalue \(\mu _{2}^{d}\) of Eringen’s differential model follows from \(G_{2}=0\) if \( l_{c}\in [0,0.2]\).

4.2 The type of bifurcation

In this subsection, we present the results concerning the type of bifurcation. As shown, for both models we are in the presence of pitchfork bifurcations at \((\textbf{x},\mu )=(\textbf{0},\mu _{n})\) and \((\textbf{z},\mu )=( \textbf{0},\mu _{n}).\) The question that still needs to be answered is whether they are super or subcritical bifurcations. Thus, we will determine the curvatures of the nontrivial branches at the bifurcation points for the relevant values of \(\zeta \) and \(l_{c}\). In particular, the values of \(- \frac{b_{3n}}{b_{1n}}\) and \(-\frac{c_{3n}}{c_{1n}}\) will be calculated. The results are divided into four parts.

  1. (a)

    In the first part we analyze Eringen’s two-phase local/nonlocal model when \(\mu _{n}^{t}\) is a solution to \(A_{1}=0.\) From (63) and (65), it follows that \(-\frac{b_{3n}}{b_{1n}}>0\) meaning that we are in the presence of a supercritical bifurcation. From the mechanical point of view, this conclusion discovers the following two facts. The phase parameter \(\zeta \) and the small length scale parameter \(L_{c}\) do not influence the type of bifurcation. Second, the bifurcation at \((\textbf{x},\mu )=(\textbf{0},\mu _{n}^{t})\) is always supercritical if \(\mu _{n}^{t}\) is a solution to \( A_{1}=0.\) This is very important since it means that at \((\textbf{x},\mu )=( \textbf{0},\mu _{1}^{t})\), only supercritical bifurcation occurs. From Fig. 6, we can conclude that for \((l_{c},\zeta )\in (0,0.2]\times (0,1),\) an increase in \(l_{c}\) or a decrease in \(\zeta \) rises the curvature \(- \frac{b_{31}}{b_{11}}\) of the nontrivial branch that bifurcates from the trivial branch at \((\textbf{x},\mu )=(\textbf{0},\mu _{1}^{t})\). Also, we remark that when \(\zeta \) tends to 1, the curvature \(-\frac{b_{31}}{b_{11}}\) tends to 1/8 showing that the classical Bernoulli–Euler theory also leads to supercritical bifurcation.

  2. (b)

    The second part deals with the study of Eringen’s two-phase local/nonlocal model in the case \((\textbf{x},\mu )=(\textbf{0},\mu _{2}^{t})\). As mentioned in the previous subsection \(\mu _{2}^{t}\) is the second lowest eigenvalue and a solution to \(A_{2}=0.\) The values of the curvature \(-\frac{ b_{32}}{b_{12}}\) of the nontrivial branch that bifurcates from the trivial one at \((\textbf{x},\mu )=(\textbf{0},\mu _{2}^{t})\) are given in Fig. 7. This figure reveals that for \((l_{c},\zeta )\in (0,0.2]\times (0,1)\) the curvature \(-\frac{b_{32}}{b_{12}}\) is positive, so that supercritical bifurcation occurs. We remark that when \(\zeta \) is approaching 1 the value of \(-\frac{b_{32}}{ b_{12}}\) is approaching 0.089607, indicating that for the classical Bernoulli–Euler theory supercritical bifurcation occurs. Again, for \((l_{c},\zeta )\in (0,0.2]\times (0,1),\) an increase in \(l_{c}\) or a decrease in \(\zeta \) rises the curvature \(-\frac{b_{32}}{b_{12}}\). Figures 6 and 7 also show that both cases \(-\frac{b_{31}}{b_{11}}\geqq \) \(-\frac{b_{32}}{b_{12}}\) and \( -\frac{b_{31}}{b_{11}}<\) \(-\frac{b_{32}}{b_{12}}\) can occur depending on the values of \(\zeta \) and \(l_{c}\). It is worth mentioning that for other values of \( \mu _{n}^{t}\) which are solutions to \(A_{2}=0,\) a similar procedure can be used to determine the values of \(-\frac{b_{3n}}{b_{1n}}\).

  3. (c)

    The third part treats Eringen’s differential model when \(\mu _{n}^{d}\) is a solution to \(G_{1}=0.\) From (73), (74), (75), (80) and (82) it follows that

    $$\begin{aligned} -\frac{c_{3n}}{c_{1n}}=\frac{1}{8}\left( 1+l_{c}^{2}\chi _{n}\right) =\frac{1 }{8}\left[ 1+\left( 2nl_{c}\pi \right) ^{2}\right] . \end{aligned}$$
    (83)

    This shows that only supercritical bifurcations occur when \(G_{1}=0.\) Again, as in the case of Eringen’s two-phase local/nonlocal model the small dimensionless length scale parameter \(l_{c}\) increases the curvature \(-\frac{ c_{3n}}{c_{1n}}\) and does not influence the type of bifurcation. Also, at \(( \textbf{z},\mu )=(\textbf{0},\mu _{1}^{d})\) supercritical bifurcation occurs.

  4. (d)

    In the fourth part we study Eringen’s differential model in the case of the second lowest eigenvalue \(\mu _{2}^{d}\), which is a solution to \(G_{2}=0\). By integrating (79) and then using \(\mu _{2}^{d}\) from (73)\(_{2,3}\) we get a lengthy expression describing the dependence of \(-\frac{c_{32}}{c_{12}}\) on \(l_{c}\). This relation is presented in Fig. 8 which reveals that we are in the presence of a supercritical bifurcation.

Fig. 6
figure 6

Dependence of the curvature \(-\frac{b_{31}}{b_{11}}\) on \(\zeta \) and \(l_{c}\) for Eringen’s two-phase local/nonlocal model

Full size image
Fig. 7
figure 7

Dependence of the curvature \(-\frac{b_{32}}{b_{12}}\) on \(\zeta \) and \(l_{c}\) for Eringen’s two-phase local/nonlocal model

Full size image
Fig. 8
figure 8

Dependence of the curvature \(-\frac{c_{32}}{c_{12}}\) on \(l_{c}\) for Eringen’s differential model

Full size image

In concluding this subsection, it is worth noting that the types of bifurcation in both models are inherited from the classical Bernoulli–Euler rod theory. This is similar to what was observed in [35, 37, 38, 41, 42].

4.3 The post-buckling shape of nanotube

In order to analyze the post-buckling shape of the nanotube described by Eringen’s two-phase local/nonlocal model, we begin by transforming Eqs. (23) and (24). This means that a new quantity p is introduced as

$$\begin{aligned} p=\frac{\overline{r}}{\int _{0}^{1}\cos \varphi \textrm{d}t}. \end{aligned}$$
(84)

In this way, by using (6), (8) and (22), Eqs. (23) and (24) become the following two-point nonlinear boundary value problem

$$\begin{aligned} \overset{{\cdot }}{{\overline{M}}}= & -\mu \sin \varphi -p\cos \varphi , \nonumber \\ \overset{{\cdot }}{\overline{K}}= & \overline{Q}, \nonumber \\ \overset{{\cdot }}{\varphi }= & \frac{\overline{M}-\overline{K}}{\zeta }, \nonumber \\ \overset{{\cdot }}{\overline{Q}}= & \frac{\left( \zeta -1\right) \overline{M}+ \overline{K}}{\zeta l_{c}^{2}}, \nonumber \\ \overset{{\cdot }}{\overline{x}}= & \cos \varphi , \nonumber \\ \overset{{\cdot }}{\overline{y}}= & \sin \varphi , \nonumber \\ \overset{{\cdot }}{p}= & 0, \end{aligned}$$
(85)

subject to

$$\begin{aligned} \varphi (0)=0,\ \varphi (1)=0,\ \overline{K}(0)=l_{c}\overline{Q}(0),\ \overline{K}(1)=-l_{c}\overline{Q}(1),\ \overline{x}(0)=0,\ \overline{y} (0)=0,\ \overline{y}(1)=0. \end{aligned}$$
(86)

By combining (6), (8), (22), (31), (32) and (84) we get the equations that describe the post-buckling shape in the case of Eringen’s differential model

$$\begin{aligned} \overset{{\cdot }}{{\overline{M}}}= & -\mu \sin \varphi -p\cos \varphi , \nonumber \\ \overset{{\cdot }}{\varphi }= & \frac{\overline{M}}{1-l_{c}^{2}\left( \mu \cos \varphi -p\sin \varphi \right) }, \nonumber \\ \overset{{\cdot }}{\overline{x}}= & \cos \varphi , \nonumber \\ \overset{{\cdot }}{\overline{y}}= & \sin \varphi , \nonumber \\ \overset{{\cdot }}{\overline{p}}= & 0, \end{aligned}$$
(87)

subject to

$$\begin{aligned} \varphi (0)=0,\ \varphi (1)=0,\ \overline{x}(0)=0,\ \overline{y}(0)=0,\ \overline{y}(1)=0. \end{aligned}$$
(88)

Since the bifurcation is supercritical in both cases, we solve the above systems (85), (86) and (87), (88) with \( \mu =\mu _{1}+\Delta \mu \) and \(\Delta \mu >0\). In particular, for the fixed increment \(\Delta \mu =1\), (\(\mu _{1}\) changes depending on the values of parameters), we first numerically solve Eqs. (85) and (86) with \(K= \overline{Q}=0,\zeta =1\) (Bernoulli–Euler model) and then the same equations with \(\zeta =0.2\) and \(l_{c}\in \{0.1,0.2\}\) (Eringen’s two-phase local/nonlocal model). The results for the deflection \(\ \overline{y}(t)\) and bending moment \(\overline{M}(t)\) are given in Figs. 9 and 10, respectively.

Fig. 9
figure 9

Deflections of the nanotube for Eringen’s two-phase local/nonlocal model with \(\zeta =0.2\) and (1) \(l_{c}=0\), (2) \(l_{c}=0.1\), (3) \(l_{c}=0.2\)

Full size image
Fig. 10
figure 10

Bending moments of the nanotube for Eringen’s two-phase local/nonlocal model with \(\zeta =0.2\) and (1) \(l_{c}=0\), (2) \(l_{c}=0.1\), (3) \(l_{c}=0.2\)

Full size image

Next, we apply the same numerical procedure to solve (87) and (88) with \(l_{c}\in \{0,0.1,0.2\}\). The graphical representations for this case are given in Figs. 11 and 12.

Fig. 11
figure 11

Deflections of the nanotube for Eringen’s differential model for (1) \(l_{c}=0\), (2) \(l_{c}=0.1\), (3) \(l_{c}=0.2\)

Full size image
Fig. 12
figure 12

Bending moments of the nanotube for Eringen’s differential model for (1) \(l_{c}=0\), (2) \(l_{c}=0.1\), (3) \(l_{c}=0.2\)

Full size image

From these figures, it can be concluded that for the fixed increment \(\Delta \mu =1,\) an increase in \(l_{c}\) results in several interesting qualitative observations. First, for both models, the deflection \(\overline{y}(t)\) increases while the bending moment in the middle \(\overline{M} (1/2) \) decreases (Figs. 9, 10, 11 and 12). Second, in the case of Eringen’s two-phase local/nonlocal model the points at which the bending moments vanish are shifted to the ends of the nanotube while for Eringen’s differential model these points do not change their positions. As a consequence the values of the bending moment at the ends and in the middle of the nanotube, in the case of Eringen’s two-phase local/nonlocal model, are not the same (the bending moment in the middle is greater than the ones at the ends of the nanotube). In contrast, in the case of Eringen’s differential model the values of the bending moment in the middle and at the ends are equal.

However, from a quantitative point of view numerical analysis reveals that there are no significant differences in the deflections between the models. Namely, for \(\Delta \mu =1\), \(\zeta =0.2\) and \(l_{c}\in \{0.1,0.2\}\) the differences between maximum deflections of the two models are less than \( 10^{-2}.\) We note that if we fixed \(\mu \) instead of \(\Delta \mu ,\) the differences in the deformed configurations of the two models can be significant.

5 Conclusions

This paper deals with a clamped nanotube through which a string is moving with a constant velocity. In order to account for a nonlocal effect and compare different nonlocal theories two models for the constitutive equation are used: Eringen’s two-phase local/nonlocal model and Eringen’s differential model. The most important results of this paper are as follows:

  1. 1.

    The governing equations of the nanotube for both Eringen’s two-phase local/nonlocal model and Eringen’s differential model are derived in suitable forms given by (29) and (35), respectively. These governing equations consist of nonlinear ordinary differential equations with corresponding boundary conditions. The governing equations do not depend on the frictional force.

  2. 2.

    The characteristic equations for Eringen’s two-phase local/nonlocal model (49) and Eringen’s differential model (72) are derived. From these equations, the critical buckling loads are determined for both models. The differences between the buckling loads in these two cases can be significant, although the effect of nonlocality reduces the critical buckling load in both cases. The critical buckling load corresponding to Eringen’s two-phase local/nonlocal model is lower than that corresponding to Eringen’s differential model for \(\zeta \) in the vicinity of zero and small values of \(l_{c}.\) On the other hand, the critical buckling load of Eringen’s differential model is lower than that of Eringen’s two-phase local/nonlocal model for \(\zeta \) in the vicinity of 1 and small values of \(l_{c}\).

  3. 3.

    The Lyapunov–Schmidt method confirms that the bifurcation points of both models are determined by the eigenvalues of the linearized systems.

  4. 4.

    The bifurcation equations for both models reveal that only pitchfork bifurcations can occur. In the case of Eringen’s two-phase local/nonlocal model, the bifurcation types at the two lowest eigenvalues are supercritical for \((l_{c},\zeta )\in [0,0.2]\times (0,1)\). Additionally, for \(l_{c}\in [0,0.2]\) the types of bifurcation at the two lowest eigenvalues of Eringen’s differential model are supercritical. This means that for these values of \(l_{c}\) and \(\zeta \) the bifurcation types are the same as in the case of the classical Bernoulli–Euler model. However, the parameters \(l_{c}\) and \(\zeta \) influence the curvatures of the nontrivial branches at the bifurcation points in the sense that an increase in \(l_{c}\) or a decrease in \(\zeta \) increases the curvature.

  5. 5.

    By numerical analysis, the post-buckling behaviors of the two models of nanotube are obtained by solving two-point nonlinear boundary value problems. There is a qualitative difference in post-buckling shapes. Also, the post-buckling shapes of the nanotube confirm the existence of a softening effect for both models.