Impact dynamics of droplets on convex structures: an experimental study with a maximum spreading diameter model for convex surface impacts

Abstract

Droplet impact is a common phenomenon in daily life and various industrial applications. Previous research shows that surface geometry significantly influences impact outcomes. However, there is a gap in systematic research on how convex structures, similar in size to the droplet, influence impact behaviors. To address this, our study focused on producing various targets with different convexity to investigate the morphological evolution of droplet impact. Using high-speed imaging techniques, we examined these impacts with Weber numbers ranging from 5 to 346. The experimental results show that dry convex surfaces increase the maximum spreading diameter of droplets by altering liquid mass redistribution. Reduced air entrapment diminishes the circumferential instability of deformed droplets on these surfaces, as evidenced by fewer fingers formed. This study also proposes a hybrid model to predict the maximum spreading diameter on convex surfaces using the energy conservation method. Benefiting from models for flat surfaces, this new approach accounts for convex surface impacts, which alter the impact characteristics according to the convexity of the impact geometry. The model assumes that the droplet at its maximum spreading diameter resembles either a disc or a rim. Notably, the rim assumption was quite evident in several convex surface impacts, presenting a donut-shaped expansion. These results are combined through weighted summation The hybrid model’s predictions show a superior agreement with the experimental data compared to existing models. Additionally, the model’s weighting factors provide insights into the distribution of liquid mass between the central film and the surrounding rim.

Graphical abstract

1 Introduction

Droplet impact is a ubiquitous natural phenomenon and has many practical applications such as spray cooling, inkjet printing, and microfluidic applications (Derby 2010; Breitenbach et al. 2018; Jomantas et al. 2023). Research on droplet impacting onto dry, flat surfaces elucidates the fundamental mechanism behind droplet deformation. Although the underlying mechanism of droplet impact on a dry flat surface has been well-elucidated with the help of high-speed imaging techniques and computational fluid dynamics (CFD), ideal flat surfaces are commonly not found in both nature and industry (Yarin 2006). In various industrial applications, droplets often impact on curved surfaces, such as raindrops on turbine blades (Verma et al. 2020) and oil droplets on hot solid catalyst particles in reactors (Nayak et al. 2005). Additionally, impacts on convex surfaces can enhance agricultural practices, including pesticide application and crop protection (Wei et al. 2020). Furthermore, findings from studies on convex surface impacts improve the development of fabrics with improved water repellency and breathability (Dong et al. 2023). Consequently, it is crucial to investigate droplet impacts on diverse surfaces, with this article focusing on convex geometric structures.

Bird et al. (2013) reported that superhydrophobic surfaces with macrostructures can alter the droplet hydrodynamics and reduce contact time. Since then, numerous experimental and numerical studies on droplet impact on superhydrophobic surfaces with varying convex surfaces have been carried out, for example, on cylindrical ridges (Hu et al. 2021; Guo et al. 2018; Andrew et al. 2017; Gauthier et al. 2015), cuboid ridges (Yun 2021; Hu et al. 2021; Lin et al. 2019), and wedge-shaped ridges (Liu et al. 2020; Regulagadda et al. 2017). In recent years, the focus of these studies shifted toward target surfaces with convex morphology of comparable size to the impacting droplet instead of a ridged flat plate with infinite length scale in one dimension. For example, Ding et al. (2021) conducted an experimental study of droplet impact on a superhydrophobic surface with a small pillar and indicated that the surface prevents the droplet from splashing. With respect to the curvature of the surface, the droplet diameter also affects the spreading diameter and the threshold Weber number for splashing (Dalgamoni and Yong 2021).

As a similar geometry to the current work’s convex geometry, recent works have been conducted extensively to investigate the dynamics of droplet impact on conical geometries. Early studies started with predicting the equilibrium positions of axisymmetric drops on conical surfaces based on drop volume, needle geometry and shape, surface wettability, liquid surface tension, and the relative magnitude and orientation of gravity (Hanumanthu and Stebe 2006). Shen et al. (2017) were pioneers in examining the bouncing dynamics of droplets on conical convex surfaces. They discovered that the reduction in contact time is primarily due to the rapid retraction process. Specifically, the droplet deforms into an annular shape upon impact with the hydrophobic cone, creating a new contact line that spreads and retracts swiftly. These insights culminated in the discovery that superhydrophobic cone surfaces with cone angles between 60 and 120 degrees significantly enhance droplet rebound (Tang et al. 2023). This enhancement originates from the suppression of boundary layer buildup during early impact and the weakening of viscous flow at the moving edge for the remainder of the droplet’s interaction. Additionally, a numerical study of droplet impact on a superhydrophobic cone by Luo et al. (2021) identified three distinct outcomes: rebound without impalement, rebound with impalement, and formation of a liquid ring. Subsequently other studies explored the droplet impaling phenomenon on superhydrophobic cones with a broader range of cone angles (Tang et al. 2023). At an intermediate cone angle, the vertical momentum of the droplet finds a balance, resulting in purely radial motion leading to the stretching of the liquid ring until corrugations appear, and eventually breaking up into satellite droplets due to Rayleigh–Plateau instability. Although there is an increasing interest in this subject, most studies focus on droplet impacts on cones larger than the droplets themselves, overlooking the expansion behavior on the substrate where the cone is placed.

One particular interest in droplet impact studies is the spreading diameter due to its crucial role in related practical applications, such as spray cooling (Breitenbach et al. 2018) and inkjet printing (Derby 2010). The maximum spreading diameter of an impacting drop has been studied extensively, including the effect of fluid properties (Xu et al. 2022; Derby 2010), the shape of the drop (Derby 2010), the surface properties (Breitenbach et al. 2018; Jomantas et al. 2023), the surrounding air pressure, the substrate temperature, the inclination of the surface, or the influence of an external field. The maximum spreading diameter of a droplet denoted as the maximum spreading ratio $\beta _\textrm{max} = D_\textrm{max}/D$, where $D_\textrm{max}$ is the maximal spreading diameter and D is the initial diameter of the drop, is primarily influenced by the Reynolds number (Re) and the Weber number (We). In the capillary regime, kinetic energy transforms into surface energy at maximum spreading, resulting in $\beta _\textrm{max} \sim \text {We}^{1/2}$ (Roisman et al. 2002). In contrast, in the viscous regime, energy dissipation occurs via viscosity, leading to $\beta _\textrm{max} \sim \text {Re}^{1/5}$ (Clanet et al. 2004; Fedorchenko et al. 2005). Typically, both capillary and viscous influences are necessary for a comprehensive description of spreading dynamics. Eggers et al. (2010) integrated these effects into a unified scaling law: $\beta _\textrm{max} \propto \text {Re}^{1/5} f_c(\text {WeRe}^{2/5})$. Laan et al. (2014) enhanced this with an analytical formula, $\beta _\textrm{max}\text {Re}^{1/5} = P^{1/2}/(A + P^{1/2})$, where $P = \text {WeRe}^{2/5}$ and A is a fitting constant. Lee et al. (2016) included a correction for the zero impact velocity limit, taking into account surface wetting properties: $(\beta _\textrm{max}^2 - \beta _0^2)^{1/2} \text {Re}^{1/5} = \text {We}^{1/2}/(A + \text {We}^{1/2})$.

While the maximum spreading diameter has been predicted well for flat surface impacts, non-ideal surfaces require different prediction models due to significant changes in the underlying mechanism. For non-ideal surfaces, Wang et al. (2022) derived scaling laws for the maximum spreading factor of nanodroplets on solid surfaces, finding $\beta _\textrm{max} \sim \text {We}^{1/5}$ for low-Weber-number and $\beta _\textrm{max} \sim \text {We}^{2/3} \text {Re}^{-1/3}$ for high-Weber-number impacts. Similarly, Ma and Huang (2023) proposed a new scaling law for $\beta _{max}$ for droplets on flexible substrates across a wide range of Weber numbers. A recent study by Zhang et al. (2024) proposed a model for the maximum spreading diameter on conical tips, using an energy approach and treating the impact phenomenon as either a film or a ring. However, there is a gap when it comes to systematically produced well defined convex surfaces with mathematically linked to the droplet diameter. Existing research has been conducted to systematically study only on drop impacts on conical geometries. Moreover, models for maximum spreading diameter, developed for flat surfaces, are not directly applicable to convex surfaces. The models for conical surfaces do not consider the continued expansion of the droplet on the substrate where the conical tip is placed, which remarkably changes the expansion behavior and leads to a different perturbation formation on the rim.

2 Theoretical development of prediction models

The predictive models for the maximum spreading diameter of droplets based on energy conservation have gained wider attention, which essentially considers the droplet before impact as an ideal sphere and its shape at maximum expansion as a disc. The energy-based model calculates $\beta _\textrm{max}$ by solving the energy balance equation for states pre- and post-impact. In contrast, the analytical methods, which were relatively ignored, have higher accuracy but are difficult to derive for complex geometries. Therefore, this paper will utilize crucial findings from Roisman (2009)’s theory to develop an improved energy-based prediction model.

2.1 Prediction models for maximum spreading diameter using energy approach in previous research

Chandra and Avedisian (1991) introduced a novel energy-based analysis method, presuming the droplet takes on a disc (or cylinder) shape with a maximum spreading diameter $D_\textrm{max}$ and a height of h at its maximum spreading diameter, as depicted in Fig. 1. The normalized maximum spreading diameter $\beta _\textrm{max}$ is defined for a droplet with an initial diameter $D_{0}$:

$$\begin{aligned} {\beta }_\textrm{max}={D}_\textrm{max}/D_0 \end{aligned}$$

(1)

Fig. 1

Sketch of droplet before and after impact

In subsequent discussions, subscripts “1” and “2” pertain to conditions prior to impact and at maximum spread, respectively. The conservation of energy between these phases is expressed as:

$$\begin{aligned} E_{\textrm{K}_{1}}+E_{\textrm{S}_{1}}=E_{\textrm{K}_{2}}+E_{\textrm{S}_{2}}+W \end{aligned}$$

(2)

where $E_\textrm{K}$, $E_\textrm{S}$, and W refer to the kinetic energy, surface energy, and dissipation energy, respectively. Subsequently resulting energy equation is derived by Chandra and Avedisian (1991) as:

$$ \frac{3}{2}\frac{{{\text{We}}}}{{\text{Re} }}\beta _{{\max }} ^{4} + (1 - \cos \theta )\beta _{{\max }} ^{2} - \left( {\frac{1}{3}{\text{We}} + 4} \right) = 0,{\text{ }} $$

(3)

where We $= \rho U_0^2D_0/\sigma$ and Re $= \rho U_0D_0/\mu$ are the dimensionless parameters Weber number and Reynolds number, respectively. $U_0$ is the impact velocity, $\sigma$ is the surface tension, $\mu$ is the dynamic viscosity of the droplet, and $\rho$ denotes the liquid density. $\theta$ is the static contact angle. Afterward Pasandideh-Fard et al. (1996) proposed a modification to the dissipation characteristic time, $t_c={8D_0}/{3U_0}$, which has been widely adapted thereafter. Additionally, they recommended using the boundary layer thickness, $\delta =2{D_0}/{\sqrt{\text{Re}}}$, instead of h when calculating the dissipation energy, W, resulting in:

$$\begin{aligned} W=\frac{\pi }{3}\rho {U_0}^2{D_0}{D_\text{max}}^2\frac{1}{\sqrt{\text{Re}}} \end{aligned}$$

(4)

Upon substituting the terms into the energy balance Eq. 2, the normalized maximum spreading diameter $\beta _\textrm{max}$ is obtained by Pasandideh-Fard et al. (1996) as:

$$\begin{aligned} \beta _\textrm{max}=\sqrt{\frac{\textrm{We}+12}{3(1-\cos \theta )+4(\textrm{We}/{\sqrt{\textrm{Re}}})}}. \end{aligned}$$

(5)

Mao et al. (1997) further modified the model by introducing adjustments to the surface energy at Stage 2 by considering the disc’s side walls, yielding:

$$\begin{aligned} E_{S_{2}}=\left( \frac{\pi }{4}{D_\textrm{max}}^2(1-\cos \theta )+\frac{2}{3}\pi \frac{{D_0}^3}{D_\textrm{max}}\right) \sigma _\textrm{LV} \end{aligned}$$

(6)

Here $\sigma _\textrm{LV}$ denotes the surface tension between the liquid and air. Furthermore, they proposed a modified equation for dissipation energy using empirical correlations:

$$\begin{aligned} W=0.2\frac{\textrm{We}^{0.83}}{\textrm{Re}^{0.33}}{\beta _\textrm{max}}^2\pi {D_0}^2\sigma _\textrm{LV} \end{aligned}$$

(7)

Their model after substitution is:

$$ \left[ {\frac{1}{4}\left( {1 - \cos \theta } \right) + 0.2\frac{{{\text{We}}^{{0.83}} }}{{{\text{Re}}^{{0.33}} }}} \right]\beta _{{{\text{max}}}} ^{3} - \left( {\frac{{{\text{We}}}}{{12}} + 1} \right)\beta _{{{\text{max}}}} + \frac{2}{3} = 0.{\text{ }} $$

(8)

2.2 Improvement in film thickness calculation

In contrast to the energy-based analysis, which assumes the shape of the droplet at maximum expansion as a disc with uniform thickness and overlooks the internal flow dynamics, Roisman et al. (2002)’s theoretical investigation takes into account the presence of viscous flow within the liquid film. They introduced the concept of residual film thickness $h_\textrm{res}$, defined as the thickness at the point when velocity in the lamella nearly reaches zero. Consequently, the calculation of dissipation energy is enhanced by substituting the characteristic length with the residual film thickness proposed by Roisman (2009).

$$\begin{aligned} h_\textrm{res}=0.79D_0\textrm{Re}^{-2/5} \end{aligned}$$

(9)

2.3 Hybrid model on flat surfaces

In previous studies utilizing the energy approach, at Stage 2 (maximum spreading) the droplet is assumed as a disc (cylinder). However, in the actual physical process, the profile of the deformed droplet at its maximum spreading diameter comprises a thin liquid film in the center surrounded by a larger rim on the periphery, as depicted in Fig. 2a (Roisman et al. 2002). Therefore, this paper proposes that the droplet’s shape at its largest size can be assumed as a hybrid of a complete disc and a complete rim, as shown in Fig. 2. The maximum spreading factor for the hybrid model can be determined by linearly adding up the values calculated by the two assumptions with specific weighting factors. In this way, this study not only enables a more accurate simulation of the droplet’s real profile, but the weighting factors can also serve as an indicator of the ratio between the disc and rim.

Fig. 2

Illustration of droplet shape at maximum deformation and hybrid model assumptions. a The simulation image depicted herein is a product of our own research and analysis conducted as part of this academic study, flat surface impact, D = 3.2 mm, $U_0=0.5$ m/s, We = 5

In the following discussion, the subscripts “d” and “r” indicate the disc and rim assumptions, respectively. Considering that the gravitational potential energy $E_G$ represents only a small fraction of the total energy ( 1%), the term related to $E_G$ is neglected in this study. The conservation of energy between two stages is given in Eq. 2. Prior to impact, the kinetic energy ($E_{K_1}$) and surface energy ($E_{S_1}$) of the droplet are defined as follows:

$$\begin{aligned}{} & {} E_{\textrm{K}_{1}}=\left( \frac{1}{2}\rho {U_{0}^2}\right) \left( \frac{1}{6}\pi {D_{0}^3}\right) =\frac{\pi }{12}\rho {U_0}^2{D_0}^3 \end{aligned}$$

(10)

$$\begin{aligned}{} & {} \quad E_{\textrm{S}_1}=\pi {D_0}^2\sigma _\textrm{LV} \end{aligned}$$

(11)

At Stage 2, the system is assumed to be static; therefore, referring to Mao et al. (1997), the surface energy of the liquid disc is obtained as:

$$\begin{aligned} E_{S_{2}}=\left( \frac{\pi }{4}{D_\textrm{max}}^2(1-\cos \theta )+\frac{2}{3}\pi \frac{{D_0}^3}{D_\textrm{max}}\right) \sigma _\textrm{LV} \end{aligned}$$

(12)

The energy dissipation caused by viscosity, denoted as W, is approximately formulated as:

$$\begin{aligned} W=\int _{0}^{t_c}\int _{\Omega }^{}\Phi \,d\Omega {dt}\ \approx \Phi \Omega {t_c} \end{aligned}$$

(13)

where $t_c={8D_0}/{3U_0}$ is modified dissipation characteristic time by Pasandideh-Fard et al. (1996), $\Omega =\frac{\pi }{4}{D_\textrm{max}}^2{h}$ stands for the volume of viscous layer, and $\Phi$ represents the dissipation function which, for Newtonian fluid, is given by:

$$\begin{aligned} \Phi =\mu \left( \frac{\partial v_i}{\partial x_j} + \frac{\partial v_j}{\partial x_i}\right) \frac{\partial v_i}{\partial x_j}\approx \mu {\left( \frac{U_0}{h}\right) }^2 \end{aligned}$$

(14)

Substituting the characteristic length with the residual film thickness proposed by Roisman (2009):

$$\begin{aligned} h_\textrm{res}=0.79D_0\textrm{Re}^{-2/5} \end{aligned}$$

(15)

The corresponding dissipation function is therefore adapted to:

$$\begin{aligned} \phi = \mu \left( \frac{U_0}{h_\textrm{res}}\right) ^2 \end{aligned}$$

(16)

Furthermore, the viscous volume is modified as follows:

$$\begin{aligned} \Omega =\frac{\pi }{4}{D_\textrm{max}}^2{h_\textrm{res}} \end{aligned}$$

(17)

the modified equation of dissipation energy is expressed as:

$$\begin{aligned} W=\Phi \mathrm {\Omega }t_c=\frac{200\pi }{237}\mu U_0D_\textrm{max}^2\textrm{Re}^{0.4} \end{aligned}$$

(18)

Due to energy conservation between Stage 1 and Stage 2, the equilibrium equation for disc model can be written as:

$$\begin{aligned} E_{\textrm{K}_1}+E_{\textrm{S}_1}=E_{\textrm{K}_2}+E_{\textrm{S}_2,d}+W_d \end{aligned}$$

(19)

Since the system at Stage 2 is static, it can be assumed that $E_{K_2}$=0. Referring to Mao et al. (1997), the surface energy of the liquid disc is obtained as:

$$\begin{aligned} E_{S_2,d}=\left( \frac{\pi }{4}{D_{\textrm{max},d}}^2(1-\cos {\theta }) +\frac{2}{3}\pi \frac{{D_0}^3}{D_{\textrm{max},d}}\right) \sigma _\textrm{LV} \end{aligned}$$

(20)

Substituting all terms and the results from disc assumption is calculated as:

$$\begin{aligned}{} & {} \left[ 3\left( 1-\cos \theta \right) +\frac{800}{79}\textrm{We}\textrm{Re}^{-0.6}\right] \nonumber \\{} & {} \quad {\beta _{\textrm{max},d}}^3-\left( \textrm{We}+12\right) \beta _{\textrm{max},d}+8=0. \end{aligned}$$

(21)

Concerning the rim assumption, when the droplet deforms to its maximum spreading diameter, it takes on a rim-like shape with an annular diameter of $D_{\textrm{max},r}$ and a cross-sectional rim diameter of $D_\textrm{rim}$, as shown in Fig. 2c. Based on the experimental observations, the cross-sectional rim diameter $D_\textrm{rim}$ is significantly smaller than the annular diameter $D_{\textrm{max},r}$ indicating $D_\textrm{rim}\ll D_{\textrm{max},r}$. Thus, due to conservation of mass, the rim diameter can be determined as

$$\begin{aligned} \frac{\pi }{6}{D_0}^3=\frac{\pi }{4}{D_\textrm{rim}}^2\pi {D_{\textrm{max},r}} \end{aligned}$$

(22)

which gives:

$$\begin{aligned} D_\textrm{rim}=\frac{\sqrt{2{D_0}^3}}{3\pi D_{\textrm{max},r}} \end{aligned}$$

(23)

The corresponding surface energy of the rim is calculated as:

$$\begin{aligned} E_{S_2,r}=\pi ^2{D_{\textrm{max},r}}{D_\textrm{rim}}\sigma _\textrm{LV}=\sqrt{\frac{2\pi ^3}{3}}\sigma _{LV}\sqrt{{D_0}^3D_{\textrm{max},r}} \end{aligned}$$

(24)

The dissipation function calculation remains the same as in $W_d$:

$$\begin{aligned} W_r=W_d \end{aligned}$$

(25)

The terms $E_{K_1}$, $E_{S_1}$, and $E_{K_2}$ remain the same as those in the disc model, leading to the equilibrium equation for the rim assumption:

$$\begin{aligned} E_{\textrm{K}_1}+E_{\textrm{S}_1}=E_{\textrm{K}_2}+E_{\textrm{S}_2,r}+W_\textrm{r} \end{aligned}$$

(26)

which yields:

$$\begin{aligned} \frac{800}{79}\textrm{We}\textrm{Re}^{-0.6}{\beta _{\textrm{max},r}}^2 +4\sqrt{6\pi }{\beta _{\textrm{max},r}}^\frac{1}{2}-\left( \textrm{We}+12\right) =0. \end{aligned}$$

(27)

Subsequently, the hybrid model is proposed as the weighted sum of the results calculated using the disc and rim assumptions, given by:

$$\begin{aligned} \beta _{\textrm{max}, \textrm{hybrid}}={C_d\cdot \beta }_{\textrm{max},d}+{C_r\cdot \beta }_{\textrm{max}, \textrm{r}}, \end{aligned}$$

(28)

where $C_d$ and $C_r$ are weighting factors which can be determined empirically using the experimental data and satisfy:

$$\begin{aligned} C_d+C_r=1. \end{aligned}$$

(29)

Specifically, the values of $C_d$ and $C_r$ are acquired by minimizing the squared sum of errors between the experimental data and corresponding value calculated by the hybrid model at the same impact velocity. The physical reasoning behind the hybrid maximum spreading diameter model presented in Eq. 29 involves the coefficients $C_d$ and $C_r$, which serve as weighting factors indicating the distribution of the impact energy. Specifically, $C_d$ represents the fraction of the impact energy that spreads out as the central disc, while $C_r$ quantifies the energy contributing to the formation of the surrounding rim. These coefficients are crucial for accurately capturing the mass distribution and energy dissipation during droplet impact. On more convex surfaces, a greater proportion of the droplet’s mass is directed toward the rim, altering the relative sizes of the disc and rim. Consequently, the model reflects the observed behaviors of droplet impact more accurately by considering how the surface’s geometry influences energy partitioning between the disc and rim. This approach provides a more precise prediction of the maximum spread diameter, as it accounts for the varying dynamics of mass distribution and energy dissipation depending on the convexity of the surface.

2.4 Target definition

Before generalizing the hybrid model to non-flat surfaces, it is necessary to define the quantitative parameters of convexity. In this work, the contour of the convex surface is defined as a curve that follows Gaussian distribution which has a consistent height with different spans. The contour function is given as:

$$\begin{aligned} h_T(x)=H\cdot \exp \left(-\frac{x^2}{{{2\sigma }_T}^2}\right), \end{aligned}$$

(30)

where $h_T$ is the height of the target at the corresponding radial distance x. H is the maximum height in the center and $\sigma _T$ is the standard deviation of the function, as illustrated in Fig. 3a. Since the mass of the target is concentrated within the range of $-2\sigma _T\le x\le 2\sigma _T$, the nominal diameter of the convex geometry is thus determined as $D_T=4\sigma _T$. Throughout this study, the central height H of the target remains constant and is equal to the initial drop size, $D_0$. In this way, the aspect ratio, $\lambda$, is defined as the ratio of the radial span to the vertical height, which gives:

$$\begin{aligned} \lambda =\frac{D_T}{H}=\frac{4\sigma _T}{D_0}. \end{aligned}$$

(31)

Fig. 3

a Gaussian surface profile definition. b Targets used in experiments with corresponding aspect ratios

In particular, the aspect ratio of a flat surface is defined as $\lambda =\infty$. The targets for experiment are produced by rotating the Gaussian curves around their axes, which are shown in Fig. 3b.

2.5 Hybrid model generalization to convex surfaces

In order to simplify the calculation process on convex surfaces, the Gaussian contours are first fitted linearly, as illustrated in Fig. 4. Specifically, within the range of $\left[ -2\sigma _T,2\sigma _T\right]$, the curved profile is linearly fitted to two oblique lines that are symmetrical about the axis. The included angle, $\phi$, of the fitted lines is defined as the cone angle.

Fig. 4

Linear fitting of the Gaussian contours of convex targets

Figure 5 shows the model sketch of droplet at maximum spreading diameter based on disc and rim assumptions on a convex surface. The nomenclature of parameters is defined as follows: $A_d^\textrm{conv}$ refers to the parameter A with conv representing convex and d representing disc while r representing rim. After calculation (details can be found in Appendix A), the equation for disc assumption model with $D_{\textrm{max},d}^\textrm{conv}<2D_0\tan {\left( \frac{\phi }{2}\right) }$ is given as:

$$\begin{aligned}{} & {} \frac{3\left( 1-{\text{cos}}\theta \right) +\frac{800}{79}\textrm{We}\textrm{Re}^{-0.6}}{\sin \left( \frac{\phi }{2}\right) } {\beta _{\textrm{max},d}^\textrm{conv}}^3\nonumber \\{} & {} \quad -\left( {\text{We}}+12\right) \beta _{\textrm{max},d}^\textrm{conv}+8=0. \end{aligned}$$

(32)

For $D_{max,d}^\textrm{conv}\ge 2D_0\tan {\left( \frac{\phi }{2}\right) }$, the disc model gives:

$$ \left[ {3\left( {1 - \cos \theta } \right) + \frac{{800}}{{79}}{\text{We}}\text{Re} ^{{ - 0.6}} } \right]\beta _{{\max ,d}}^{{conv\;3}} + {\text{ }}\left\{ {12\left( {1 - \cos \theta } \right)\left[ {\tan \left( {\frac{\phi }{2}} \right)^{2} - \frac{{\tan \left( {\frac{\phi }{2}} \right)}}{{\cos \left( {\frac{\phi }{2}} \right)}}} \right]\frac{{3200}}{{79}}{\text{We}}\text{Re} ^{{ - 0.6}} - We - 12} \right\}{\text{ }}\beta _{{\max ,d}}^{{{\text{conv}}}} + 8 = 0. $$

(33)

Similarly, the equation for rim assumption model with $D_{max,r}^{conv}<2D_0tan{\left( \frac{\phi }{2}\right) }$ is given as:

$$\begin{aligned}{} & {} \frac{\frac{800}{79}\textrm{We}\textrm{Re}^{-0.6}}{\sin \left( \frac{\phi }{2}\right) }{\beta _{\textrm{max},r}^\textrm{conv}}^2\nonumber \\{} & {} \quad +4\sqrt{6\pi }{\beta _{\textrm{max},r}^\textrm{conv}}^\frac{1}{2}-\left({\text{We}}+12\right) =0. \end{aligned}$$

(34)

For $D_{max,r}^{conv}\ge 2D_0tan{\left( \frac{\phi }{2}\right) }$, the rim model is:

$$ \begin{gathered} \frac{{\frac{{800}}{{79}}{\text{We}}\text{Re} ^{{ - 0.6}} }}{{\sin \left( {\frac{\phi }{2}} \right)}}\beta _{{\max ,r}}^{{{\text{conv}}\;{\text{2}}}} + 4\sqrt {6\pi } \beta _{{\max ,r}}^{{{\text{conv}}\;\frac{1}{2}}} \hfill \\ + \frac{{3200}}{{79}}\left[ {\frac{{\tan \left( {\frac{\phi }{2}} \right)}}{{\cos \left( {\frac{\phi }{2}} \right)}} - \tan \left( {\frac{\phi }{2}} \right)^{2} } \right]{\text{We}}\text{Re} ^{{ - 0.6}} - {\text{We}} - 12 = 0. \hfill \\ \end{gathered} $$

(35)

Fig. 5

Model sketch based on disc and rim assumptions on a convex surface

The hybrid model, therefore, can be obtained based on the calculated results as:

$$\begin{aligned} \beta _{\textrm{max},\textrm{hybrid}}^\textrm{conv}={C_d}^\textrm{conv}\cdot \beta _{\textrm{max},r}^\textrm{conv}+{{C_r}^\textrm{conv}\cdot \beta }_{\textrm{max},r}^\textrm{conv}, \end{aligned}$$

(36)

where ${C_d}^\textrm{conv}$ and ${C_r}^\textrm{conv}$ can be determined by minimizing the squared sum of error between corresponding data from experiments.

3 Experimental section

3.1 Experimental setup

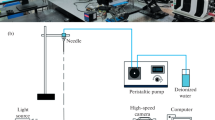
To validate the proposed hybrid model, droplet impact experiments were conducted using the test rig shown in Fig. 6. The droplets were generated from a hypodermic needle connected to a syringe powered by a computer-controlled syringe pump (Leien Linz-9A). When the falling droplet passes through the laser diodes, it initiates the recording with an adjustable delay. The impingement process was recorded using a color high-speed camera (Photron FASTCAM SA3) with a fixed Macro Lens (LAOWA FF100mm F2.8 CA-Dreamer Macro 2X macro lens) with a fixed image pixel resolution of $512 \times 512$ at 5,000 fps. In the experiments, the high-speed camera was positioned horizontally and vertically to provide both front-view and top-view images. The top-view camera was placed at an 85-degree angle relative to the targets to create an unobstructed view. We found this setup provided the optimal balance between capturing a “near” true top-view perspective at the same time avoiding any interference from the experimental setup. With positioning the camera just a little bit off the vertical axis, we were able to maintain a more vertical line of sight to the impact phenomena while ensuring the scene is not blocked by the syringe needle. The front-view imaging was conducted with the assistance of an orthogonal camera which was placed at 0-degree angle relative to the targets, to minimize the deviation of impact location and ensure the consistency of experiments.

Fig. 6

Schematic of overall experimental setup

3.2 Materials and properties

The solid targets used in the experiments that are shown in Fig. 3b are produced using a special high-resolution ($\sim$0.05 mm) photosensitive resin named “red wax.” All of the targets have the same thickness (5.2 mm) and the same diameter (30 mm). The color of the droplet is enhanced by adding blue dye. The colored liquid is produced with the concentration of 0.24 g of methylene blue (Macklin) in 1000 mL of deionized water (abbreviated as MB solution). The contact angle of the MB solution on the target surface as well as the surface tension of the MB solution were measured using a contact angle tester (Drop Shape Analyzer - DSA100, KRÜSS). Pendant droplet method was used to measure surface tension of the liquid surface. The static contact angle is measured as $112.4^\circ \mp 2.8^\circ$ which indicates that our surface is hydrophobic and the liquid surface tension is measured to be $133.15\mp 0.15$ mN/m. While we measured the static surface tension of water with methylene blue, the dynamic surface tension might be different because it changes over time as the dye moves to the surface. Although we did not measure dynamic surface tension in this study, it is important to investigate in the future studies. The viscosity of the liquid was measured as 0.00089 $\text {Pa}\cdot \text {s}$ and found to have a negligible effect on the viscosity of the deionized water. The results are obtained by taking the average value of three tests. The density of the MB solution liquid is measured by a density meter (Density Meter DDM 2910, Rudolph Research Analytical), as 992.9 kg/$\mathrm m^3$.

3.3 Illustrating location-dependent impacts on convex targets using a dual-camera system

When a droplet collides with a target of similar size, the impact location becomes a critical factor in determining the outcome. While simulations offer precise control over the impact position, achieving accuracy in experimental approaches is challenging due to the droplet’s unpredictable free fall. This challenge is particularly significant when investigating the convexity or surface geometry of the target because even slight variations in impact location can result in significantly different outcomes.

While existing experimental studies have explored the influence of geometric features on collision outcomes, few have underscored the significance of impact position deviation. Especially those studies relying on back-lit images cannot guarantee impact location accuracy. Given the primary focus of this work on the effects of symmetric convex surfaces on droplet impingement behaviors, a synchronized dual-camera system has been developed as the primary experimental tool to ensure accurate impact location.

Fig. 7

Centered and offset impact behaviors on convex targets from the front and top views, $U_0=1.7$ m/s, We = 72

In this study, droplet impact experiments were simultaneously recorded using front- and top-view cameras to ensure accuracy in impact location. The influence of impact location on convex surface impacts is highlighted in Fig. 7 to underscore its significance. Notable distinctions in the deformation process and results were observed between droplets impacting the center and those with offset impacts on convex surfaces. In the offset impact scenario, the droplet hits approximately 4.8 mm away from the intended location, which is the tip of the convex structure. This displacement places the impact point at the back side of the structure, as viewed from the front perspective. Droplets hitting the center spread evenly across the surface, while those with offset impacts quickly rerouted to form a crescent-shaped liquid film.

Furthermore, comparing front-view photos for both centered and offset impacts shows minimal or no difference. This indicates that back-lit images alone are unreliable for capturing precise impact location, thus complicating the description of the droplet deformation process. Conversely, the comparable top-view photos demonstrate the benefits of the dual-camera system by clearly distinguishing whether the droplet hits the precise center of the convex target.

3.4 Image processing methods

Considering the contour of the droplet edge has an irregular shape instead of a perfect circle, the equivalent diameter is calculated to measure the size of the expanding lamella. To determine the equivalent diameter using top-view images, image processing techniques such as background subtraction, binarization, noise filtering, and edge detection are applied, as shown in Fig. 8. The area and equivalent diameter of the shape surrounded by the identified edge are calculated using MATLAB. The equation of the equivalent diameter, denoted by $D_{eq}$, is defined as:

$$\begin{aligned} D_\textrm{eq}=\sqrt{\frac{4A}{\pi }}, \end{aligned}$$

(37)

where A represents the calculated area which is obtained by calculating the actual number of pixels in the region.

Fig. 8

Illustration of image processing procedures

We carefully monitored and controlled every experimental run to reduce variability in order to address the uncertainty in these measurements. The average of three successful experiments for each case is used to compute the deviations in our model. In addition to the controlled experimental conditions, several other sources of uncertainty could influence the accuracy of our measurements. One significant source is the resolution of the images used for analysis. Our 512 $\times$ 512 pixel images represent each millimeter with approximately 11 pixels. This resolution may impose an error as the exact boundaries of the droplet’s spread might not align perfectly with the pixel grid. As a result, there could be slight discrepancies in the measurement of the droplet’s dimensions, which may result in an uncertainty within one or two pixels. To quantify this uncertainty:

Each pixel corresponds to approximately

$$\begin{aligned} \frac{1 \text { mm}}{11 \text { pixels}} \approx 0.0909 \text { mm/pixel} \end{aligned}$$

An error of two pixels translates to an uncertainty of

$$\begin{aligned} 2 \times 0.0909 \text { mm} = 0.1818 \text { mm} \end{aligned}$$

Given that the spreading diameter we are measuring is around 10–15 mm, the percentage difference introduced by a 2-pixel measurement error can be calculated as follows:

For a diameter of 10 mm:

$$\begin{aligned} \frac{0.1818 \text { mm}}{10 \text { mm}} \times 100 \approx 1.82\% \end{aligned}$$

For a diameter of 15 mm:

$$\begin{aligned} \frac{0.1818 \text { mm}}{15 \text { mm}} \times 100 \approx 1.21\% \end{aligned}$$

These calculations indicate that a 2-pixel error results in a percentage difference of approximately 1.21–1.82% of the actual spreading diameter, which is relatively small.

3.5 Test matrix

In the experiments, the droplet diameter was maintained constant at $D_0 = 3.2$ mm which was calculated using front-view images. The impact velocity $U_0$ was calculated by analyzing 30 image frames captured before the droplet hit the surface. In the experiments, $U_0$ was varied within the range between 0.5 and 3.8 m/s by changing the release height. The corresponding Weber numbers were in the range between $We= 5\sim 346$. As for surface convexity, three convex targets with aspect ratios $\lambda =$ 1, 2, 4 are selected in comparison with a flat benchmark. The evolution of droplet impact on flat and convex surfaces is recorded from both front view and top view. For each test condition, the experiments were conducted five times to ensure the data are reliable.

4 Results and discussion

4.1 Hybrid model in viscid and inviscid limits

Our model is aimed to predict droplet behavior on convex surfaces, which fundamentally changes the impact dynamics compared to flat surfaces. The behavior on convex surfaces involves unique factors such as expansion on a curved surface comparable in size to the droplet, different air entrapment mechanisms, and potential impalement from the center in some cases. The model utilizes a hybrid approach that integrates both disc and rim models, which are prominently visible in droplet impacts on convex surfaces.

For the disc approach, our model adheres to the We$^{1/2}$ scaling in the inviscid limit and Re$^{0.3}$ scaling in the viscous limit. We believe these scalings are appropriate for our cases, where the droplet impacts a convex surface with a size comparable to the droplet itself. The inclined nature of the surface promotes greater expansion in the viscous limit than a flat surface, making the Re$^{0.3}$ scaling more applicable than the traditional Re$^{0.2}$ scaling.

The rim approach accounts for the droplet taking a donut shape during impact, a phenomenon frequently observed in convex surface impacts. This approach is fundamentally different from flat surface impacts, where expansion of a droplet as a complete rim is not present, and thus we do not expect it to follow the same scaling in the inviscid limit. It follows the Re$^{0.3}$ scaling in the viscous limit. Additionally, the model includes weighting factors that provide insights into the distribution of liquid mass between the central film and the surrounding rim.

4.2 Morphological results on flat surfaces

The morphological evolution of the impacting droplet on different targets from front and top views at $U_0=0.5$ m/s, $U_0=1.7$ m/s, and $U_0=3.5$ m/s is displayed in Fig. 10, 11, 12, 13, 14, 15. For impact on the flat surface, Fig. 12(a1)–(a6) and Fig. 13 (a1)–(a5) demonstrate that after impact, the upper part of the droplet remains spherical while the lower part is rapidly flattened, resulting in a profile resembling a fried egg. Besides, with the droplet hitting the substrate, azimuthal perturbations appear around the propagating liquid rim and grow larger. The perturbations become pronounced at maximum spread, which are often termed as “fingerings” in existing literature. When the droplet spreads to its maximum diameter, the viscous force drags the advancing rim while the liquid in the central film continues to feed the lobbed rim, giving rise to an excessive surface energy. Capillary waves echo inward before the liquid in thickened rim flows back into the central film, and the whole droplet retracts to form a rebound pillar.

4.3 Model validation on flat surfaces

Although the hybrid model is primarily designed for convex surface impacts, it demonstrates a good fit for flat surface impacts when using selected $C_d$ and $C_r$ coefficients within the experimental range. The values of $C_d$ and $C_r$ of the hybrid model are calculated as:

$$\begin{aligned} \left\{ \begin{array}{lc} C_d=0.48 \\ C_r=0.52\\ \end{array}, \right. \end{aligned}$$

(38)

which produces the equation of hybrid model as:

$$\begin{aligned} \beta _\textrm{max,hybrid}={0.48\cdot \beta }_\textrm{max,d}+{0.52\cdot \beta }_\textrm{max,r}. \end{aligned}$$

(39)

The maximum spreading diameters of droplets observed in the experiments are captured using high-speed imaging and presented in Fig. 9. The predicted results from the hybrid model, along with disc and rim assumption models, are also depicted in the same figure for comparison with Roisman et al. (2010)’s model, which shows pretty good agreement with our data. It is evident that while the model based on the disc assumption slightly underestimates the experimental results, it follows the overall trend closely and shows a reasonable agreement. Conversely, the model based on the rim assumption tends to slightly overestimate the results but performs better than the disc-based model.

Fig. 9

Maximum spreading factor obtained from experiment and hybrid model on dry, flat surfaces

The deviation values for these models are presented in Table 1. The hybrid model, initially developed for convex surface impacts, performs adequately on flat surfaces, with a deviation around 3.8 percent. This observation indicates the presence of disc and rim formations in flat surface impacts, demonstrating good alignment with the experimental data. While the Roisman model exhibits commendable performance on flat surfaces, it has limitations when applied to convex surfaces. Furthermore, newer models developed for flat surface impacts also prove inadequate for convex surface applications. These limitations underscore the necessity of developing a new model specifically tailored for convex surface impacts, as discussed in the subsequent sections.

Table 1 Deviation values of rim, disc, and hybrid models compared to experimental values

Fig. 10

Image sequences of droplet impact on flat and convex targets from front view. $U_0=0.5$ m/s, We = 5

Fig. 11

Image sequences of droplet impact on flat and convex targets from top view. $U_0=0.5$ m/s, We = 5

Fig. 12

Image sequences of droplet impact on flat and convex targets from front view. $U_0=1.7$ m/s, We = 72

Fig. 13

Image sequences of droplet impact on flat and convex targets from top view. $U_0=1.7$ m/s, We = 72

Fig. 14

Image sequences of droplet impact on flat and convex targets from front view. $U_0=3.5$ m/s, We = 285

Fig. 15

Image sequences of droplet impact on flat and convex targets from top view. $U_0=3.5$ m/s, We = 285

4.4 Morphological results on convex surfaces

The evolution of droplet on convex targets is more complicated. Firstly, droplet impacting on both targets with aspect ratio $\lambda =2$ and $\lambda =1$ are impaled by the apexes as shown in Figs. 10, 12, and 14. The fast-spreading inner edge created by the impalement converges with the receding outer edge at the bottom, generating an unstable liquid toroid which subsequently breaks up into a ring of satellite droplets, specifically referring to Fig. 13(c5), (c6) and (d5), (d6). On the contrary, for droplet impact on the target $\lambda =4$, without being disrupted, the spreading droplet recedes and forms a liquid column which is even taller than that on the flat surface, as shown in Fig. 10(b5) and more evidently in Fig. 12(b5). This can be explained that due to the mass redistribution caused by the additional descending motion, the surface energy of the front rim at maximum spreading phase on the convex surface is greater than that on a flat surface. The redundant surface energy therefore initiates stronger retraction and finally leads to a greater retraction height. We do not see this mechanism in higher impact speed case in Fig. 14(b5), when the droplet is disrupted during the receding phase. Secondly, comparing snapshots at 3.4 ms and 6.4 ms in Figs. 11, 13, and 15 (i.e., vertical selection of (a3)–(d3), (a4)–(d4)), it is evident that the time of reaching the maximum diameter is increased on convex targets as convexity efficiently reduces the radial instability in the circumferential rim. Specifically, the rim on target $\lambda =1$ is almost not perturbed after the impact (corresponding to Fig. 13(d3)) and the average size of fingerings on target $\lambda =4$ is smaller than that on a flat substrate (referring to Fig. 13 (b3) and (a3)). Lastly, in low impact case We = 5, we observed a complete rim expansion in Fig. 11(d5) and (d6), indicating that as $\lambda$ decreases, the expanding liquid in the droplet inclined to accumulate in the rim by disrupting disc formation.

Fig. 16

Transformation of impact images into contours for roundness calculations. a is impact on the flat surface, c is impact on the convex target with $\lambda =1$. b and d are the detected contours by image analysis

To quantify the differences between the high-speed images, specifically the rim formations on different target impacts, the circularity values are measured based on droplets maximum expansions. After the background subtraction, the images are converted into grayscale images and black and white versions are created based on these grayscale images. The contour is detected with the help of the image processing methods as it shown in Fig. 16. The circularity of these contours is measured using equation:

$$\begin{aligned} \textrm{Circularity}\,=\,{\frac{4{\pi }A}{l^2}}, \end{aligned}$$

(40)

Fig. 17

Measured circularity values of expanding droplets at maximum diameter for flat and convex surfaces

where A is the encircled area and l is the length of the contour. Figure 17 depicts the measured values for flat surface ($\lambda =\infty$) and convex surfaces. Clearly all of the convex cases have better circularity values than the flat surface one. This means that they have less amount of perturbations and less number of finger formations at the time of maximum expansion. One particular reason behind this alteration is the reduction in air entrapment within the expanding droplet. Kelvin–Helmholtz instability is known to be one of the main contributors of this circumferential instability (Liu et al. 2015). In flat surface impacts, since the ultrathin air under the expanding droplet is thinner than the mean free path of the air molecules, air inside the liquid film starts to move with an exceptionally high speeds and causes to create much larger stress. This stress is large enough to trigger Kelvin–Helmholtz instability, causing more fingers on the rim. In their work, Liu et al. (2015) showed how the ultrathin air under the expanding droplet alters the rim formation and splashing. Having a mechanism with tiny holes to discharge air resulted in less number of perturbations in their work. Although there is no hole to discharge the air in our setup, having a structure that traps less air than the flat surface decreases the amount of air responsible from instabilities and resulted in more circular rim formation for the same impact conditions. It means that the impact becomes more stable in terms of Kelvin–Helmholtz instability.

When the convex surfaces compared between each other within the experimental set, the more convex the surface, the more stable the droplet expansion is. Since our convex geometries have sizes comparable to droplet, the droplet experiences a better fit during the impact and traps less air as it shown in Fig. 18a. Moreover, the sharper convex surfaces (smaller $\lambda$) resulted in even higher rim circularity which also points another source of air escape mechanism. For We = 72, both $\lambda =2$ and $\lambda =1$ cases’ sharper tip causes expanding droplet to be disrupted and accounts for another source for air ejection. This type of mechanism occurs before reaching the maximum expansion and adds another source for stability on the rim during expansion period. However, the same mechanism is also responsible from the instabilities thereafter and results in more complex receding process causing droplet to divide into smaller droplets. After the impalement, the disrupted inner film moves radially outward and clashes with the receding rim as it is presented in Fig. 18b. This interaction causes inward moving rim to slow down and fall into couple of smaller pieces.

Fig. 18

a Droplet’s initial impact position on a convex target. b Receding process on a convex target with a disrupted film condition

4.5 Model validation on convex surfaces

As we had seen in the experiment results, the convexity of the surface introduces significant variations in impact properties including maximum spreading diameter. The nuanced interplay between surface morphology and impact dynamics challenges the capabilities of existing models, which often struggle to accurately capture the subtle effects of convex geometries. Figure 19 shows the maximum spreading factors $\beta _{max}$ on convex surfaces calculated by the hybrid model, along with the data obtained from experiments. Specifically, the models with convex cases $\lambda =2$ and $\lambda =4$ demonstrate the strongest agreement with the data across all test conditions, particularly for high We. The under-prediction observed in the results for convex target with $\lambda =1$ could be due to the additional expansion resulting from tip impalement, which is not considered in the current calculation.

Fig. 19

Hybrid model on dry convex surfaces. The markers represent the experimental data, and the solid lines indicate the results from hybrid model

4.6 Weighting factors

The weighting factors used in the hybrid models for each test case are shown in Fig. 20. It can be observed that the values of $C_d$ for convex targets are generally lower than the values of $C_r$, indicating that the mass of liquid in the peripheral rim is greater than that in the central lamella. When we test out the same analysis for the concave surfaces, the $C_d$ values were all considerably higher than the $C_r$ values, signifying that it is harder for the deformed droplet on concave surfaces to form a peripheral rim. These findings strongly support the conclusions reached from the morphological analysis. The ratio between $C_d$ and $C_r$ reveals the distribution of mass in the deformed droplet when it reaches its maximum spreading diameter.

Fig. 20

Weighting factors of disc and rim models for each convexity

We acknowledge the importance of explaining and justifying our model, while stating its limitations. The accuracy of our model requires on thorough experimental data in order to tune the weighting factors properly. The weighting factors $C_d$ and $C_r$, based on experimental data, indirectly reflect the effects we did not consider to put in our model, though determining these factors requires further refinement. These tuning constants depend on several factors beyond impacting velocity, such as substrate wettability, fluid surface tension, viscosity, and target geometry, which makes our model reliant on experimental data for accuracy.

In the hybrid model development section, we represented surface tension energy in terms of the surface area of the rim. However, we recognize the need to consider the scenario where the liquid in the disc transforms into the rim, which introduces an additional dissipation term. The precise formulation of this term depends on various factors, including geometry, spreading dynamics, and the physical properties of the liquid and substrate, which increases the model’s complexity. Accurate modeling of this phenomenon typically relies on numerical simulations and experimental data to complement analytical approaches.

5 Conclusions

In this work, the effect of surface convexity on droplet impact has been experimentally investigated. The morphological and parametric analyses provide a comprehensive description of the droplet deformation process and clarify the variations introduced by systematically designed convex surfaces. The key findings are summarized as follows:

Convex surfaces can increase both the maximum spreading diameter and the time taken for droplets to reach this maximum diameter. In certain cases, the droplet was impaled by the tip of the target, while in others, the rebound height was higher than that on flat surfaces. This effect is mainly due to the mass redistribution resulting from the additional downward motion. The extra surface energy stored in the enlarged rim can sometimes cause the droplet to recede, leading to a higher retraction height. Additionally, convex surfaces reduced circumferential instability, decreasing the number of finger-like protrusions.

A hybrid model has been proposed to predict the maximum spreading diameter of droplets impacting convex surfaces. This model integrates two assumptions for the deformed droplet shape: disc and rim. It has shown good agreement with experimental data on convex surfaces. The weighting factors on surfaces with different levels of convexity illustrate the mass distribution between the central film and the surrounding rim.

While the hybrid model has limitations, as it does not account for internal fluid flow on a convex surface, resulting in some deviations, it opens new avenues for researchers studying various geometries. Future work could enhance the model by incorporating additional weighting factors for different geometries, focusing on the liquid mass distribution between the peripheral rim and the central lamella.

Appendix: Derivation of hybrid model on convex surfaces

Fig. 21

Model sketch based on disc and rim assumptions on a convex surface

Firstly, the disc model on a convex surface is focused on, as shown in Fig. 21a, disc assumption model. The terms “disc” on a convex surface here represents a layer of liquid with a vertical thickness $h_d^\textrm{conv}$ within the range of $D_{\textrm{max},d}^\textrm{conv}$. When the droplet has no contact with the bottom surface, $D_{\textrm{max},d}^\textrm{conv}<2D_0\tan {\left( \frac{\phi }{2}\right) }$, according to the conservation of mass, the thickness of the disc can be calculated as:

$$\begin{aligned} h_d^\textrm{conv}=\frac{2{D_0}^3}{3{D_{\textrm{max},d}^\textrm{conv}}^2}{\sin \left( \frac{\phi }{2}\right) } \end{aligned}$$

(41)

The contact area between the deformed droplet and the convex surface is:

$$\begin{aligned} S_d^\textrm{conv}=\frac{\pi {D_{\textrm{max},d}^\textrm{conv}}^2}{4\sin \left( \frac{\phi }{2}\right) }. \end{aligned}$$

(42)

Therefore, the surface energy and dissipation energy of the disc can be calculated by:

$$\begin{aligned}{} & {} E_{S2,d}^\textrm{conv}=\left[ \frac{\pi }{4}{D_{\textrm{max},d}^\textrm{conv}}^2\left( 1-\cos \theta \right) +\frac{2}{3}\pi \frac{{D_0}^3}{D_{\textrm{max},d}^\textrm{conv}}\right] \sigma , \end{aligned}$$

(43)

$$\begin{aligned}{} & {} \quad W_d^\textrm{conv}=\Phi \Omega t_c=\frac{200\pi }{237}\mu U_0\textrm{Re}^{0.4}\frac{{D_{\textrm{max},d}^\textrm{conv}}^2}{\sin \left( \frac{\phi }{2}\right) }. \end{aligned}$$

(44)

With regard to the case $D_{\textrm{max},d}^\textrm{conv}\ge 2D_0\tan {\left( \frac{\phi }{2}\right) }$, the above term can be modified as:

$$\begin{aligned}{} & {} S_d^\textrm{conv}=\pi \left \{\frac{{D_{\textrm{max},d}^\textrm{conv}}^2}{4}-{D_0}^2\left[ {\tan \left( \frac{\phi }{2}\right) }^2 -\frac{\tan \left( \frac{\phi }{2}\right) }{\cos \left( \frac{\phi }{2}\right) }\right] \right \}, \end{aligned}$$

(45)

$$\begin{aligned}{} & {} \quad E_{S2,d}^\textrm{conv}=\left[ S_d^\textrm{conv}\left( 1-\cos \theta \right) +\frac{2}{3}\pi \frac{{D_0}^3}{D_{\textrm{max},d}^\textrm{conv}}\right] \sigma , \end{aligned}$$

(46)

$$\begin{aligned}{} & {} \quad W_d^\textrm{conv}=\Phi \Omega t_c=\frac{800\pi }{237}\mu U_0\textrm{Re}^{0.4}S_d^\textrm{conv}. \end{aligned}$$

(47)

Secondly, the calculation of rim model on a convex surface follows similar process, which is displayed in Fig. 21b. The rim diameter can be primarily obtained based on mass conservation as:

$$\begin{aligned} D_{\textrm{rim},r}^\textrm{conv}=\sqrt{\frac{2{D_0}^3}{3\pi D_{\textrm{max},r}^\textrm{conv}}}. \end{aligned}$$

(48)

The contact surface area, surface energy, and energy dissipation for $D_{\textrm{max},r}^\textrm{conv}<2D_0\tan {\left( \frac{\phi }{2}\right) }$ are given as:

$$\begin{aligned}{} & {} S_r^\textrm{conv}=\frac{\pi {D_{\textrm{max},r}^\textrm{conv}}^2}{4\sin \left( \frac{\phi }{2}\right) },\end{aligned}$$

(49)

$$\begin{aligned}{} & {} \quad E_{S2,r}^\textrm{conv}=\sqrt{\frac{2\pi ^3}{3}}\sigma \sqrt{{D_0}^3D_{\textrm{max},r}^\textrm{conv}},\end{aligned}$$

(50)

$$\begin{aligned}{} & {} \quad W_r^\textrm{conv}=\Phi \phi \Omega t_c=\frac{200\pi }{237}\mu U_0\textrm{Re}^{0.4}\frac{{D_{\textrm{max},r}^\textrm{conv}}^2}{\sin \left( \frac{\phi }{2}\right) }. \end{aligned}$$

(51)

While for $D_{\textrm{max},r}^\textrm{conv}<2D_0\tan {\left( \frac{\phi }{2}\right) }$, the terms are given as:

$$\begin{aligned}{} & {} S_r^\textrm{conv}=\pi \left\{ \frac{{D_{\textrm{max},r}^\textrm{conv}}^2}{4}-{D_0}^2\left[ {\tan \left( \frac{\phi }{2}\right) }^2 +\frac{\tan \left( \frac{\phi }{2}\right) }{\cos \left( \frac{\phi }{2}\right) }\right] \right\} , \end{aligned}$$

(52)

$$\begin{aligned}{} & {} \quad E_{S2,r}^\textrm{conv}=\sqrt{\frac{2\pi ^3}{3}}\sigma \sqrt{{D_0}^3D_{\textrm{max},r}^\textrm{conv}}, \end{aligned}$$

(53)

$$\begin{aligned}{} & {} \quad W_r^\textrm{conv}=\Phi \phi \Omega t_c=\frac{800\pi }{237}\mu U_0\textrm{Re}^{0.4}S_r^\textrm{conv}. \end{aligned}$$

(54)

After substitution of terms into the energy conservation equation, the maximum spreading factors based on the disc and rim assumptions can be calculated.