1 Introduction

Both the dark hollow beam and the flat-topped beam are widely investigated in these years due to their important applications in optical micromanipulation, atom cooling, microscopy, optical communication, material processing, medical treatment and so on [1,2,3,4,5,6]. Various types of dark hollow beams are realized in these years [7,8,9,10,11,12,13,14,15,16,17,18,19,20]. High-order Bessel beams [7], Laguerre–Gaussian beams, ring Gaussian beams [8, 21,22,23] may be the most prevalent ones. However, most dark hollow beams have single or multiple gaussian-like light rings; there are few investigations on hollow light beams with flat intensity profile. At the same time, various models of flattop beams are proposed, such as super-Gaussian beam, multi-Gaussian beam, flat-topped Gaussian beam, partially coherent beam with flat source and so on [24,25,26,27,28]; different techniques are applied to generate flat-topped beams [29,30,31,32,33]. Recently, the flat-top beam generated by intra-cavity shaping method is utilized to enhance the brightness and efficiency of laser beam [34]. However, the beam combining hollow shape and flat profile together has never been studied yet; the analytical formula for the propagation of this kind of beam has not been given. Undoubtfully, the flat-topped beam with hollow profile could greatly improve the application efficiency of hollow beams in many fields. For example, in STED microscopy, the use of hollow flat-topped beam as depletion beam could reduce the injury to the sample.

Usually, a ring aperture could be used to generate light ring with any width, but this light ring is not flat because of the incident Gaussian beam, and most importantly, ring aperture causes much energy waste; and there is no analytical solution for the propagation of light beams through hard aperture, which prevents detailed investigations about it. The flat-topped beams with optical vortex seem satisfy our needs, but in fact, the introduction of optical vortex would change intensity profile, and results in non-flatness [35]. Similar things also happen to other flat-topped Gaussian-related beams [36]. Obviously, a new model for flat light ring and an analytical formula for propagation of this beam would be of great importance in the fields mentioned before.

On the other hand, the optical cage, also called optical bottle, is a 3D dark spot surrounded by bright regions in all directions [37]. The optical cage could be generated by interference methods, special laser beams, caustic method and so on [38,39,40,41,42,43,44]. In optical micromanipulation, optical cage is quite useful in trapping low-index particles, and the common bright focal spot is used to trap high-index particles. In this paper, we find that the hollow flat beam presented in this paper could conveniently create both spots while propagating in free space; this would be helpful in optical micromanipulation.

In this paper, we propose a new theoretical model for such flat light ring, which is called hollow flat-topped Gaussian beam (HFTGB). We obtain an analytical approximate formula for propagation of HFTGB through any ABCD optical system by decomposing this beam into a series of hollow Gaussian beams. We find that both bright 3D focal spot and dark 3D focal spot exist in the autofocusing process of HFTGB (here, “autofocusing” means increasing of intensity while propagating in free space, without lens or other nonlinearities [11, 12]). Thus, a perfect optical cage could be conveniently formed in free space. We could also control the property of this optical cage by varying parameters of HFTGB. Finally, the HFTGBs are generated in our experiment, and all the theoretical results are demonstrated.

2 Analytical formula for HFTGB propagating through ABCD optical system

According to the expression of flat-topped Gaussian beam [24], the hollow flat-topped Gaussian beam in the initial plane can be easily expressed as,

$$E\left( r \right) = G\sum\limits_{n = 1}^{N} {\frac{{( - 1)^{n - 1} }}{N}\left( {\begin{array}{*{20}c} N \\ n \\ \end{array} } \right)\exp \left[ { - \frac{{n(r - r_{0} )^{2} }}{{w_{0}^{2} }}} \right]} ,$$
(1)

where r is the radial coordinate in the initial plane, r0 defines the radius of the hollow region, w0 can be considered a scaling factor; N is an integer and is proportional to the width of the flat top, as shown in Fig. 1. When N = 1, the HFTGB is actually the well-known ring Gaussian beam, which could be generated by the Fourier transform of Bessel–Gaussian beam [13]. When N is larger, the flat top would be broadened, and the edge of the flat top would be steeper; G is a constant which is related with the input power P:

$$P{ = 2}\pi \int\limits_{0}^{\infty } {\left| {E\left( r \right)} \right|^{2} } r{\text{d}}r = G^{2} \sum\limits_{n = 1}^{N} {\frac{{\left( { - 1} \right)^{n} }}{N}} \left( {\begin{array}{*{20}c} N \\ n \\ \end{array} } \right)\left[ {\frac{{\pi w_{0}^{2} }}{2n}\exp \left( {\frac{{ - 2nr_{0}^{2} }}{{w_{0}^{2} }}} \right) + \frac{{\pi^{{{3 \mathord{\left/ {\vphantom {3 2}} \right. \kern-\nulldelimiterspace} 2}}} r_{0} w_{0} }}{\sqrt 2 n}{\text{erfc}}\left( {\frac{{ - \sqrt 2 nr_{0} }}{{w_{0} }}} \right)} \right].$$
(2)
Fig. 1
figure 1

Normalized Intensity profile of HFTGB in the initial plane

Full size image

Note that we should discriminate between the HFTGB expressed by Eq. (1) and previous multi-Gaussian beam [27], though their expressions look similar: The multi-Gaussian beam is defined in x–y coordinate system, and owns a rectangular profile [27]; HFTGB in this paper is defined in polar coordinate system, and its profile is a hollow ring, thus exhibits different properties. On the other side, it is easy to get an analytical formula for propagation of multi-Gaussian beam, but for HFTGB, as we can show below, only an approximate formula could be obtained.

As shown in Eq. (1), the HFTGB could be considered superposition of ring Gaussian beams with different ring widths \(w_{n} = {{w_{0} } \mathord{\left/ {\vphantom {{w_{0} } {\sqrt n }}} \right. \kern-\nulldelimiterspace} {\sqrt n }}.\) And when \(a = {{r_{0} } \mathord{\left/ {\vphantom {{r_{0} } {w_{n} }}} \right. \kern-\nulldelimiterspace} {w_{n} }}\) is large enough, the ring Gaussian beam has nearly the same intensity profile with the hollow Gaussian beam [17], i.e.,

$$E{ = }G\sum\limits_{n = 1}^{N} {\frac{{( - 1)^{n - 1} }}{N}\left( {\begin{array}{*{20}c} N \\ n \\ \end{array} } \right)\exp \left[ { - \frac{{n(r - r_{0} )^{2} }}{{w_{0}^{2} }}} \right]} \approx G\sum\limits_{n = 1}^{N} {\frac{{( - 1)^{n - 1} }}{N}\left( {\begin{array}{*{20}c} N \\ n \\ \end{array} } \right)A_{{m_{H} }} \left( {\frac{{r^{2} }}{{w_{H}^{2} }}} \right)^{{m_{H} }} \exp \left[ { - \frac{{r^{2} }}{{w_{H}^{2} }}} \right]} ,$$
(3)

where mH, wH and AmH are corresponding coefficients determined by Eq. (4). Based upon the fact that the hollow Gaussian beam should have the same ring width wn and radius r0 with the ring Gaussian beam, we can get the equations to solve mH, wH and AmH as shown below,

$$r_{0} = w_{H} \sqrt {m_{H} } ;$$
$$s^{{m_{H} }} e^{ - s} = m_{H}^{{m_{H} }} e^{{ - m_{H} - 1}} ,s = \left( {\frac{{r_{0} \pm w_{n} }}{{w_{H} }}} \right);$$
(4)
$$A_{{m_{H} }} = e^{{m_{H} }} m_{H}^{{ - m_{H} }} .$$

Equation (4) could be solved by numerical methods. Figure 2 shows that when a is large enough (a ≥ 4 in this paper), the approximation expression 3 agrees quite well with HFTGB. Equation (3) not only indicates an analytical solution for propagation of HFTGB, but also offers an effective method to generate HFTGB by superposition of hollow Gaussian beams [45, 46], which we would investigate further in the future.

Fig. 2
figure 2

Comparison between HFTGB and its approximation expression with different a = r0/w0: aa = 2; ba = 4; ca = 6

Full size image

There is no analytical solution for the propagation of HFTGB expressed by Eq. (1), which is also the main difficulty for the detailed study about it. However, if we use the approximate expression of Eq. (3), we could get an analytical expression for simulating the propagation of HFTGB through any optical system, which would be more efficient and convenient.

The light beam that propagates through any ABCD system can be calculated by Collins formula [47],

$$E(r,z) = \frac{ik}{B}\exp ( - ikz)\exp \left( { - \frac{{ikDr^{2} }}{2B}} \right)\int\limits_{0}^{\infty } {E(r^{\prime})\exp \left( { - \frac{{ikAr^{{\prime}{2}} }}{2B}} \right)J_{0} \left( {\frac{{krr^{\prime}}}{B}} \right)r^{\prime}{\text{d}}r^{\prime}} ,$$
(5)

where r and r' are radial coordinates in the initial plane and output plane, respectively; z is the position of the output plane; ABCD are the elements of the ray transfer matrix; k is the wave vector, and J0 is the zero-order Bessel function. By substituting the approximation expression into Eq. (4), we can get

$$\begin{gathered} E(r,z) = G\frac{ik}{B}\exp ( - ikz)\exp \left( { - \frac{{ikDr^{2} }}{2B}} \right)\sum\limits_{n = 1}^{N} {\frac{{( - 1)^{n - 1} }}{N}\left( {\begin{array}{*{20}c} N \\ n \\ \end{array} } \right)A_{{m_{H} }} } \hfill \\ \, \times \int\limits_{0}^{\infty } {\left( {\frac{{r^{{\prime}{2}} }}{{w_{H}^{2} }}} \right)^{{m_{H} }} \exp \left[ { - \frac{{r^{{\prime}{2}} }}{{w_{H}^{2} }}} \right]\exp \left( { - \frac{{ikAr^{{\prime}{2}} }}{2B}} \right)J_{0} \left( {\frac{{krr^{\prime}}}{B}} \right)r^{\prime}{\text{d}}r^{\prime}} . \hfill \\ \end{gathered}$$
(6)

Using the integral formula [48],

$$\int\limits_{0}^{\infty } {r^{{\prime}2n + 1} e^{{ - pr^{{\prime}{2}} }} J_{0} \left( {qr^{\prime}} \right)} {\text{d}}r^{\prime} = \frac{{\Gamma \left( {n + 1} \right)}}{{2p^{n + 1} }}\exp \left( { - \frac{{q^{2} }}{4p}} \right){}_{1}F_{1} \left( { - n,1,\frac{{p^{2} }}{4q}} \right),$$
(7)

We can get an analytical solution for the propagation of HFTGB through ABCD optical system,

$$\begin{gathered} E(r,z) = G\frac{ik}{{2B}}\exp ( - ikz)\exp \left( { - \frac{{ikDr^{2} }}{2B}} \right)\sum\limits_{n = 1}^{N} {\frac{{( - 1)^{n - 1} }}{N}\left( {\begin{array}{*{20}c} N \\ n \\ \end{array} } \right)} A_{{m_{H} }} \frac{1}{{w_{H}^{{2m_{H} }} }} \hfill \\ \, \times \frac{{\Gamma (m_{H} + 1)}}{{\left( {\frac{ikA}{{2B}} + \frac{1}{{w_{H}^{2} }}} \right)^{{m_{H} + 1}} }}{}_{1}F_{1} \left[ { - m_{H} ,1, - \frac{{\left( \frac{kr}{B} \right)^{2} }}{{4\left( {\frac{ikA}{{2B}} + \frac{1}{{w_{H}^{2} }}} \right)}}} \right]\exp \left[ { - \frac{{\left( \frac{kr}{B} \right)^{2} }}{{4\left( {\frac{ikA}{{2B}} + \frac{1}{{w_{H}^{2} }}} \right)}}} \right], \hfill \\ \end{gathered}$$
(8)

where Γ is the Gamma function, and 1F1 is the Kummer confluent hypergeometric function.

3 Simulation results for HFTGB propagating in free space

By setting A = 1, B = z, C = 0, D = 1 in Eq. (8), we can simulate propagation of HFTGB in free space. In our simulation, we assume w0 = 0.16 mm, r0 = 4w0 = 0.64 mm unless other state; the wavelength λ = 632.8 nm; the input power is P = 10 mW. The propagation characteristics of HFTGB are simulated in Fig. 3, where the properties of beams with different N are compared. It is found that HFTGB owns an interesting property, which is that both bright spot and hollow spot would appear in the propagating process. We can define the position where the central intensity becomes maximum as bright focal spot, and minimum as dark focal spot [32].

Fig. 3
figure 3

Intensity profiles of HFTGB at different output planes: az = 0; bz = 0.2 m; cz = 0.4 m; dz = 0.5 m; ez = 0.6 m; fz = 0.8 m

Full size image

At first, all the beams are hollow (Fig. 3a); then, a central peak would appear (Fig. 3b). This central peak appears earlier with a larger N. After having reached the first focal bright spot, the intensity would decrease to a minimum value and the beam becomes hollow again. As we can see in Fig. 3d, e, the beam with N = 5 or N = 20 becomes hollow or nearly hollow at z = 0.5 m or z = 0.6 m, respectively. These positions could be defined as dark focal planes. After the dark focal plane, the beam experiences diffraction and becomes Bessel-like profile; the intense profile would not become hollow any more (Fig. 3f). But for the ring Gaussian beam (N = 1), which does not have the dark focal point, the central intensity just continues decreasing after the first bright focal plane.

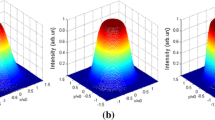
Similar conclusions could also be found in Fig. 4a, where the on-axis intensity distribution is plotted. As shown in Fig. 4a, there are two main intensity peaks before and behind the hollow region along the propagating direction. It is obvious that this hollow region is longitudinally and transversely confined by high intensity, which means that an “optical cage” is effectively formed by HFTGB. The property of this optical cage is much better with a larger N. For N = 20, the intensity at the first bright focal position is larger, and the intensity at the dark focal position is closer to zero; for N = 1, the optical cage would not even be created. We can also note that there are also some intensity oscillations before the first focal point when N is large. However, these oscillations are quite small and could be just neglected.

Fig. 4
figure 4

On-axis intensity distributions of HFTGB with different parameters: a different N, while w0 = 0.16 mm, r0 = 0.64 mm; b different w0, while N = 20, r0 = 0.64 mm; c different r0, while N = 20, w0 = 0.16 mm

Full size image

The property of the optical cage could also be controlled by varying the values of w0 and r0, as shown in Fig. 4b, c. When the scaling parameter w0 and radius r0 increase, the bright focal intensity would decrease and the dark focal position would move forward. The intensity at the dark focal position almost does not change with w0, but it becomes lower with a larger r0.

4 Experimental results

The holographic method is used to generate HFTGB in the experiment [49]. The specially devised hologram for beam with N = 20 is shown in the inset of Fig. 5. A linear phase grating is added in the hologram to separate different diffraction orders [49], and a random pixel mask technique is employed to improve the quality of output beams [50]. In this technique, the final phase mask ΦSLM could be expressed as

$$\Phi_{{{\text{SLM}}}} = \Theta \left( {{\text{rnd}} - {\Phi \mathord{\left/ {\vphantom {\Phi {2\pi }}} \right. \kern-\nulldelimiterspace} {2\pi }}} \right),$$
(9)

where Θ is the heaviside function, rnd is the random number between (0,1), Φ is the original.

Fig. 5
figure 5

Experiment setup to generate HFTGB; the inset shows the hologram for N = 20

Full size image

Phase mask of common holographic method [49]. This method is especially efficient for amplitude-only modulation with Gaussian-like profiles.

The experimental setup is shown in Fig. 5. The wavelength of He–Ne laser is 632.8 nm, the focal length of the Fourier lens is 0.4 m. When the hologram is loaded on the SLM (Spatial Light Modulator, Holoeye, LETO), the HFTGB would be directly generated in the first diffraction order, and detected by the laser beam profiler (Spiricon, SP620). In our experiment, the inner radius and outer radius of the generated HFTGB with N = 20 are 0.44 mm and 0.84 mm, respectively.

The intensity profiles in the initial plane and different output planes are measured and compared in Fig. 6. The experiment results agree quite well with the simulation results. In Fig. 6, Itheory is the theoretical intensity distribution, Ix,exp and Iy,exp are the experimental intensity distributions along x and y axes. Although there are some intensity fluctuations in the initial flat profile (Fig. 6a, c), the creation of the bright focal spot (Fig. 6d, f), the dark focal spot (Fig. 6g, i) and the optical cage (Fig. 7) are demonstrated in experiment. The first main focal point appears at z = 0.42 m; after this bright focal spot, the hollow region would appear again at z = 0.62 m, which could be determined as dark focal position; the beam keeps its hollow profile until a central peak appears again at z = 0.75 m. Similar conclusion could also be obtained in Fig. 7, where the propagation dynamics in x–z plane are plotted. In Fig. 7, we can clearly see that an optical cage is formed between z = 0.5 m and z = 0.75 m.

Fig. 6
figure 6

The experimental (first column) and theoretical (second column) intensity profiles for HFTGB with N = 20; the third column shows the comparison between the experimental and theoretical intensity distributions for N = 20 along x or y axis. a–c Initial plane, z = 0; d–f bright focal plane, z = 0.42 m; g–i dark focal plane, z = 0.62 m; j–lz = 0.75 m

Full size image
Fig. 7
figure 7

Propagation dynamics of HFTGB with N = 20: a experimental results; b simulation results. The white dotted lines show the bright focal plane and the dark focal plane

Full size image

We also measure the propagation characteristics of HFTGB with different N in free space. As we can see in Fig. 8, the beam with N = 1, i.e., the ring Gaussian beam, has only one autofocal point and could not generate optical cage. The modulation of the position and the property of the optical cage with N are also demonstrated in experiment. With a larger N, the dark focal position would be moved forward, and its intensity becomes slightly lower; the intensity of the bright focal point also increases with N.

Fig. 8
figure 8

Experimental on-axis intensity distributions of HFTGB

Full size image

5 Conclusions

In summary, the propagating characteristics of HFTGB are theoretically and experimentally investigated in this paper. The analytical formula for simulating the propagation of this beam through any ABCD optical system is obtained. Based upon this formula, the properties of HFTGB are investigated in detail. Comparing with the common ring Gaussian beams or other similar hollow beams, the HFTGB exhibits two main advantages. First, it has a flat intensity profile, and the width of the flat top could be modulated by the order N. This flat ring profile is helpful to improve the application of hollow beam in various fields. Second, both 3D bright spot and dark spot could be created during the propagating process; thus, a perfect optical cage would be formed. This would be of great help in optical micromanipulation to trapping different particles or atoms.

We also investigate the modulation of this optical cage by changing corresponding parameters of HFTGB. With a larger N, the position of the optical cage would move forward, and the intensity contrast between the bright spot and dark spot would increase; so, a better optical cage could be obtained. As the scaling parameter w0 or radius r0 increases, the position of the optical cage would also move forward; the central minimum intensity at the dark focal position would decrease with r0.

Finally, we generate HFTGB in experiment, the optical cage and its modulation methods are proved. We believe that this hollow flat-topped beam and the cage generated by it would have great applications in optical micromanipulation, microscopy and other fields; the analytical formula presented in this paper would also be helpful for the application of this beam.