1 Introduction

Compound semiconductors make up a basic class of photorefractive (PR) materials. One of their most important advantages over conventional dielectric PR materials (such as ferroelectric and cubic oxides) is their much larger charge mobility, which results in a faster response time and greater sensitivity to light power [1]. However, their main drawback is their low linear electro-optic (EO) coefficients, which impede the application of these materials to optical signal processing. An effective method of overcoming this limitation and enhancing the PR effect is using a high external electric field and wavelengths near the band edge where the Franz–Keldysh effect contributes to changes in the absorption coefficient and refractive index through a quadratic EO effect. In this way, the gain coefficient in beam coupling experiments in GaAs and InP materials increases to on the order of 10 cm−1 [2]. A much higher PR gain (exceeding 1,000 cm−1 [3]) can be obtained in semi-insulating photorefractive multiple quantum wells (PMQWs). In this case, a stronger band-edge resonance effect occurs as a result of field ionization of excitons in quantum wells, which is associated with large light absorption and a quadratic dependence on the electric field. As a result, PMQW structures exhibit markedly shorter response times (~μs) and higher sensitivity to light illumination (~μW/cm2) than bulk PR semiconductors [4, 5]. Because of the strong absorption, PMQW samples are typically used as thin layers (~1 μm) in a transmission geometry with an applied external field [4, 5]. However, it was shown that a waveguide with a GaAs–AlGaAs MQW guiding layer can potentially be used to support screening of PR spatial solitons [6]. Such solitons based on the quadratic EO effect would be faster and require lower nonlinearity than solitons obtained due to the linear EO effect in bulk PR semiconductors.

This combination of properties makes PMQWs specifically suited to dynamic holography and optical signal processing. Application proposals for PMQWs are related to various areas of optics such as image correlators [7], femtosecond pulse shaping [8], adaptive ultrasound detection [9, 10], and, in recent years, holographic 3D and optical coherence tomography imaging [11, 12].

With the growing number of demonstrated applications, an important issue arises: the correctness of the theoretical model describing PR transport in PMQWs.

For PMQWs operating in the so-called Franz–Keldysh geometry with an applied field in the planes of the quantum wells, a band transport model was developed by Wang et al. [3] (essentially the same model was developed by Magaňa et al. [13]) to account for the PR response of PMQWs in a stationary state. The proposed model was based on the similar rate equations that constitute the two-band model with one deep-level species and bipolar transport to describe the properties of conventional bulk PR materials [5].

The most important difference from the conventional model applied to nonresonant PR materials is that in PMQWs, free electrons and holes are created mainly by interband transitions, whereas the photoexcitation of carriers from deep defects can be neglected. This difference has a pronounced effect on PR behaviour.

In work [14], a modification of the model was proposed in which nonlinear hot electron transport was included to explain the appearance of a large phase shift in PMQWs for fields exceeding a certain critical value. This hypothesis was confirmed experimentally in [15].

Although the model explains the basic properties of PMQWs, note that, to date, only a motionless grating under the small-contrast approximation has been analysed.

Balasubramanian et al. [16] presented in the results of a comprehensive study of wave mixing experiments on GaAs/AlGaAs PMQWs in the Franz–Keldysh geometry using the moving fringe technique. The paper contains a number of relations that illustrate the response of PMQWs as a function of the frequency offset between writing beams.

The main purpose of this paper is to investigate the nonlinear band transport model in the presence of a moving grating and compare the results with the experimental data given in [16]. It offers an opportunity to verify the correctness of the PR transport model and indicate possible inconsistencies. In addition, the predictions of the model are examined in regard to the grating recording kinetics and the possibility of obtaining a strong resonance in PMQWs using a running grating.

Note that the studied model of the PR effect is a microscopic model that includes a number of material parameters, some of which are not precisely known. In PMQWs in which proton implantation technology is employed to make the MQWs semi-insulating, it is difficult to estimate the concentration and species of deep active defects. Therefore, one can expect to obtain qualitative rather than quantitative agreement between the theory and experimental results.

2 Photorefractive grating and wave mixing in PMQWs

Wang et al. [3, 17] and Nolte et al. [18] described the recording of a grating and wave mixing in PMQWs. A concise summary is given here for the sake of completeness and because some of the formulas given here will be used later in the paper.

2.1 Electrorefraction and electroabsorption in PMQWs

A diffractive grating with a spatial period Λ in a PMQW film with thickness L ~ 1 μm is classified as a thin grating for the wavelength λ if a dimensionless parameter Q = 2πλL/(n 0Λ2) < 1. For a GaAa/AlGaAs MQW structure, assuming L = 1 μm, λ = 840 nm, and the refractive index n f  = 3.5, Q ≈ 1.5 μm2/Λ2; hence, for Λ > 2 μm, the grating should be treated as a thin grating working in the Raman–Nath regime, where higher orders of diffraction are observed.

In wave mixing experiments, two coherent plane waves with intensities I 1 and I 2 and wave vectors k 1 and k 2 illuminate a sample, creating the interference pattern

$$I\left( {z,t} \right) = I_{0} (t) \times \left[ {1 \, + m \times \cos \left( {Kz} \right)} \right],$$
(1)

where I 0(t) = I 1(t) + I 2(t) is the sum of the two writing beam intensities, \(m = 2\sqrt {I_{1} I_{2} } /I_{0}\) denotes the index of the modulation depth (fringe contrast), K = |k 1 − k 2| × cos[(θ 1 − θ 2)/2] = 2π/Λ is the grating wave vector along the sample, and Λ is the grating period.

During the PR phenomenon, the light pattern (1) leads to the appearance of a spatially periodic distribution of the space-charge field E sc(z), which together with a dc external field E a produces the total electric field inside a material. Under the small-contrast approximation (m << 1), we can assume that the distribution of E sc(z) mimics the intensity pattern, but owing to spatial nonlocality, the field E sc(z) may be phase shifted by ϕ with respect to the interference distribution I(z):

$$E\left( z \right) \, = E_{a} + E_{\text{sc}} \left( z \right) \, = \left[ {E_{a} + mE_{1m} \times \cos (Kz + \phi )} \right],$$
(2)

where E 1m denotes the amplitude of E sc calculated for m = 1.

In PMQWs, the electric field E(z) ionizes excitons in the quantum wells, reducing their lifetime. According to the uncertainty principle (Δω ~ 1/τ), this broadens and lowers the exciton absorption peaks. As a result, spatially periodic changes in the absorption (electroabsorption) and refraction (electrorefraction) are generated, producing an amplitude–phase grating. The absorption and index gratings contribute approximately equally to the diffracted signal, although with a different wavelength relationship [4, 5]. A PR grating can be described by a complex modulation index, ñ(z) = n(z) + iκ(z), which is the sum of the refractive index n and the extinction coefficient κ = αλ/(4π).

For moderate fields, E a  < 7 ÷ 8 kV/cm, the changes in Δn and Δκ are proportional to the squared electric field [5, 18] and can be written in a similar form to those for PR materials with a linear EO effect [3]:

$$\Updelta n(z,\lambda ) = - \left( {1/2} \right)n_{f}^{3} s_{1} (\lambda )E^{2} (z),\quad \Updelta \kappa (z,\lambda ) = - \left( {1/2} \right)n_{f}^{3} s_{2} (\lambda )E^{2} (z),$$
(3)

where s 1 and s 2 denote the quadratic EO coefficients determined from the electrorefraction and electroabsorption spectra [3], respectively, and n f is the average refractive index of the material.

At higher fields, the changes in Δn are smaller than the quadratic dependence predicts, and in Eq. (3), an amendment should be considered that is proportional to the fourth power of the electric field [19]. Substituting E(z) from Eq. (2) into Eq. (3), we obtain for the complex refractive index the change in the mean value of the index and amplitudes of a grating with wave vectors K and 2 K:

$$\Updelta \tilde{n}(z) = \tilde{n}_{0} + \tilde{n}_{1} \cos (Kz + \phi ) + \tilde{n}_{2} \cos (2Kz + 2\phi ),$$
(4)

where \(\tilde{n}_{0} \propto E_{a}^{2} + (m^{2} /2)E_{1m}^{2}\), \(\tilde{n}_{1} \propto 2mE_{a} E_{1m} ,\) and \(\tilde{n}_{2} \propto (m^{2} /2)E_{1m}^{2} .\)

In the small modulation depth approximation (m << 1), only the first-order harmonic grating is important for the investigation into two-wave mixing (TWM) and four-wave mixing (FWM).

2.2 Raman–Nath diffraction and wave mixing in PMQWs

The electric field amplitude E = E 0exp[i(k z z + k y y)] of the plane wave passing through the PR grating is modulated, and the transmitted field amplitude is

$$E^{\prime } = E \times {\text{e}}^{{ikL^{\prime } \tilde{n}(z)}} = E \times {\text{e}}^{{i\left[ {\delta_{0} + \delta_{1} \cos (Kz + \phi )} \right]}} ,$$
(5)

with the complex phase factors \(\delta_{0} = \delta_{0}^{\prime } + i\delta_{0}^{\prime \prime } = kL^{\prime } (n_{0} + i\kappa_{0} )\) and \(\delta_{1} = \delta_{1}^{\prime } + i\delta_{1}^{\prime \prime } = kL^{\prime } (n_{1} + i\kappa_{1} )\), where L′ = L/cosθ′ denotes the distance travelled by the wave in the sample, taking into account the angle of refraction θ′. Expanding function (5) in the Fourier series, we can write the output field as a superposition of plane waves whose amplitudes in the far field (y >> L) are given by [20]

$$E_{\nu } (z,y) = E_{0} {\text{e}}^{{i(\delta_{0} + \nu \phi + \nu \pi /2)}} \times J_{\nu } (\delta_{1} ){\text{e}}^{{i\left[ {k_{y} y + (k_{z} + \nu K)z} \right]}} ,$$
(6)

where \(k_{y}^{2} + \left( {k_{z} + \nu K} \right)^{2} = k^{2} = \left( {{{2\pi } \mathord{\left/ {\vphantom {{2\pi } \lambda }} \right. \kern-0pt} \lambda }} \right)^{2}\), ν = 0, ±1, ±2… is the diffraction order, J ν (δ 1) denotes the Bessel function of the first kind and νth order, and J −ν (δ 1) = (−1)ν J v(δ 1).

The modulation depths of the index and absorption PR gratings are small, i.e. n 1, κ 1 = α 1/(2 k) << 1; thus, with good accuracy, we can use the approximation of the Bessel function in the form \(J_{\nu } (\delta_{1} ) \approx \delta_{1}^{\nu } /(2^{\nu } \nu !)\). The geometries for wave mixing in PMQW sample are shown in Fig. 1 for a grating written by plane waves.

Fig. 1
figure 1

a TWM in a thin PMQW grating: coherent wave interference between the zeroth and first diffraction orders causes nonreciprocal energy transfer from one beam to the other. Simultaneously with TWM, degenerate FWM occurs. Diffracted beams of first and second order from harmonic grating with wave vector K are shown. b Non-degenerate FWM: two beams with frequency ω write record the PR grating, and a signal beam with frequency ω experiences Raman–Nath diffraction. In the small-contrast approximation, only the fundamental harmonic grating is considered

Full size image

2.3 Two-wave mixing equations and photorefractive gain

An analysis of coupling waves passing through a thin PR grating is simpler than in the case of Bragg holograms in PR bulk materials and does not require the use of the wave equation. In accordance with Eq. (6), transmitted waves of the zeroth and first orders are formed by wave front modulation. The first-order diffraction ν = ±1 from one beam overlaps the direction of propagation from the second beam, ν = 0, and coherent interference occurs; this is called a TWM process. If we neglect surface reflections, the amplitudes of the transmitted waves \(E_{1}^{\prime } = E_{1(0)}^{\prime } + E_{2( - 1)}^{\prime }\) and \(E_{2}^{\prime } = E_{2(0)}^{\prime } + E_{1( + 1)}^{\prime }\) are

$${\text{e}}^{{ - i\delta_{0} }} E_{1}^{\prime } = E_{1} J_{0} (\delta_{1} ) + E_{2} {\text{e}}^{i( - \phi - \pi /2)} J_{ - 1} (\delta_{1} ) \approx E_{1} + E_{2} {\text{e}}^{ - i(\phi + \pi /2)} \delta_{1} /2,$$
(7a)
$${\text{e}}^{{ - i\delta_{0} }} E_{2}^{\prime } = E_{2} J_{0} (\delta_{1} ) + E_{1} {\text{e}}^{i(\phi + \pi /2)} J_{ + 1} (\delta_{1} ) \approx E_{2} + E_{1} {\text{e}}^{i(\phi + \pi /2)} \delta_{1} /2,$$
(7b)

where the approximations J 0(δ 1) ≈ 1 and J ±1(δ 1) ≈ ±δ 1/2 were used.

The diffracted beam is phase shifted relative to the zeroth-order beam by ±(ϕ + π/2); additionally, because δ 1 = |δ 1|exp(iϕ exc) is a complex quantity, the diffracted beam experiences a phase shift ϕ exc, which is called the excitonic spectral phase and is dependent on the wavelength [19].

The intensity of the beams corresponding to amplitudes \(E_{1}^{\prime }\) and \(E_{2}^{\prime }\) is calculated as \(I_{1}^{\prime } = \left| {I_{1}^{\prime } } \right|^{2}\) and \(I_{2}^{\prime } = \left| {E_{2}^{\prime } } \right|^{2}\), which yields

$$I_{1}^{\prime } = \left[ {I_{1} + I_{2} \frac{{|\delta_{1} |^{2} }}{4} + \sqrt {I_{1} I_{2} } \left( {\delta_{1}^{\prime } \sin \phi - \delta_{1}^{\prime \prime } \cos \phi } \right)} \right]\exp \left( { - 2\delta_{0}^{\prime \prime } } \right),$$
(8a)
$$I_{2}^{\prime \prime } = \left[ {I_{1} \frac{{|\delta_{1} |^{2} }}{4} + I_{2} + \sqrt {I_{1} I_{2} } \left( { - \delta_{1}^{\prime } \sin \phi - \delta_{1}^{\prime \prime } \cos \phi } \right)} \right]\exp \left( { - 2\delta_{0}^{\prime \prime } } \right).$$
(8b)

Further, \(2\delta_{0}^{\prime \prime } = \alpha_{0} L^{\prime }\) is substituted in the exponential factor.

Because |δ 1|2/4 << 1, the terms I 1,2|δ 1|2/4 may be neglected relative to I 1,2. In refs. [3–5], the plus sign is not correct before the terms with cosϕ [Eqs. (8a, 8b)], although in [16] it is correctly used. If the phase shift ϕ ≠ 0, the signal beam \(I_{1}^{\prime }\) = I s is amplified at the expense of the pumping beam \(I_{2}^{\prime }\) = I p , and the resulting nonreciprocal energy transfer between beams is most effective for ϕ = π/2. In semiconductor PMQWs with a direct gap, such as GaAs/AlGaAs, the electrons’ mobility changes in a strong electric field, causing a phase shift ϕ ~ π/2 [14, 15]. Owing to the symmetry of the phenomenon, the sign of ϕ changes depending on the direction of the applied field E a , which determines the direction of energy transfer in TWM. The TWM gain is defined as the ratio of intensities for a signal beam I s = I 1 at a sample output after and without mixing [5]:

$$\gamma = \frac{{I_{1}^{\prime } }}{{I_{1} \exp \left( { - \alpha_{0} L^{\prime } } \right)}} = 1 + \sqrt \beta kL^{\prime } \left( {n_{1} \sin \phi - \kappa_{1} \cos \phi } \right),$$
(9)

where the beam intensity ratio \(\beta = I_{1} /I_{2} \le 1,\) and we substituted \(\delta_{1}^{\prime } = kL^{\prime } n_{1}\) and \(\delta_{1}^{\prime \prime } = kL^{\prime } \kappa_{1} = L^{\prime } \alpha_{1} /2.\)

In experiments, the differential gain coefficient is usually measured by changing the polarity of the external field E a [16], which changes the sign of ϕ as

$$\gamma_{\text{diff}} = \frac{{\gamma ( + E_{a} ) - \gamma ( - E_{a} )}}{2} = \frac{{I_{1}^{\prime } ( + E_{a} ) - I_{1}^{\prime } ( - E_{a} )}}{{2I_{1} }} = \sqrt \beta L^{\prime } kn_{1} \sin \phi .$$
(10)

If β << 1, the fringe contrast \(m = 2\sqrt \beta /(1 + \beta ) \ll 1\), and we can assume n 1 ≈ m × n 1m , where n 1m  = n 1(m = 1). This allows us to write formula (10) using the PR gain Γ (cm−1) [5]:

$$\gamma_{\text{diff}} = \frac{\beta }{\beta + 1}\Upgamma L,\quad {\text{where}}\quad \Upgamma (E_{a} ,\lambda ) = \frac{{2kn_{1m} }}{{\cos \theta^{\prime } }}\sin \phi .$$
(11)

2.4 Degenerate and nondegenerate four-wave mixing in PMQWs

In a thin PMQW sample, degenerate FWM occurs simultaneously with TWM. For FWM, we take into account diffracted beams of the order ν > 1 (with very small intensity) and beams of the order ν = 1 from higher harmonic PR gratings. Under the small-contrast approximation, only the fundamental grating with the wave vector K [see Eq. (4)] is considered.The diffraction efficiency is defined as the ratio of the diffracted beam intensity relative to the incident beam intensity. For the fundamental grating, the diffraction efficiency in FWM to the strongest first order (ν = ±1) is given by [see Eq. (6)]:

$$\eta_{ \pm 1} (K) = \frac{{I_{1}^{\prime } }}{{I_{1} }} = \exp ( - \alpha_{0} L^{\prime } )\left| {J_{1} (\delta_{1} )} \right|^{2} .$$
(12)

Using approximation \(J_{1} (\delta_{1} ) \approx \delta_{1} /2 = (kL^{\prime } /2)\left( {n_{1} + i\kappa_{1} } \right),\) we obtain

$$\eta_{ \pm 1} (K) \approx \frac{1}{4}\left( {kL^{\prime } } \right)^{2} \exp \left( { - \alpha_{0} L^{\prime } } \right)\left( {n_{1}^{2} + \kappa_{1}^{2} } \right) \propto m^{2} .$$
(13)

The PR grating in PMQWs can also be written using a visible light beam. Photons having sufficiently high energy are absorbed in both quantum wells and barrier areas, so a trapped space-charge grating is formed within the entire MQW structure. However, electroabsorption and electrorefraction are connected with exciton resonances lying in the infrared; therefore, the changes in the absorption and refraction indices are limited to this range of wavelengths. Hence, writing beams do not ‘see’ the recording grating, whereas the signal beam having a frequency tuned to the exciton resonances is diffracted. This is the nondegenerate FWM process. The infrared signal beam erases the grating in the GaAs well layers, but because of the small photon energy, interband absorption does not occur in the AlGaAs barrier layers, where the grating remains robust. This allows low-intensity visible writing beams to control a strong infrared signal beam [21].

3 Photorefractive transport model in PMQWs

Carrier transitions and transport in MQWs with an electric field parallel to the well layers can be described similarly to those in bulk materials using the standard material equations. The layered structure of the MQWs is neglected, and the sample is treated as a strongly anisotropic continuous material, which allows us to employ 1D transport equations. Electron transport is characterized by the electric-field-dependent mobility, referred to as nonlinear transport. For holes, transport remains linear even in strong electric fields [3].

3.1 Nonlinear transport of hot electrons

The temperature T e (E) of electrons heated by an electric field can be determined from the energy balance equation by introducing the energy relaxation time τ r [22]. The time τ r determines the rate at which the accelerated electrons reach thermal equilibrium as a result of kinetic energy dissipation to the crystal lattice [5, 22],

$$qEv(E) = (3/2)k_{B} \left( {T_{e} (E) - T_{L} } \right)/\tau_{r} ,$$
(14)

where v(E) = μ e (E)E is the field-dependent velocity, k B denotes the Boltzmann constant, and q is the elementary charge. The electron temperature under a high electric field can exceed the lattice temperature T L by several hundred degrees. Therefore, in direct gap semiconductors such as GaAs, under electric fields higher than a critical value (about 3 ÷ 4 kV/cm for GaAs), a significant fraction of the electrons is excited from the lower central valley (Γ) to the lower-mobility upper valleys (L) of the conduction band. In the simple two-valley model, the electron mobility is a weighted average:

$$\mu_{e} (E) = {{(\mu_{e\Upgamma } n_{e\Upgamma } + \mu_{eL} n_{eL} )} \mathord{\left/ {\vphantom {{(\mu_{e\Upgamma } n_{e\Upgamma } + \mu_{eL} n_{eL} )} {(n_{e\Upgamma } + n_{eL} )}}} \right. \kern-0pt} {(n_{e\Upgamma } + n_{eL} )}},$$
(15)

where μ eΓ, μ eL and n eΓ, n eL are the electron mobilities and electron concentrations in the central and satellite valleys, respectively. The electron population ratio is given by the Boltzmann distribution:

$${{n_{eL} } \mathord{\left/ {\vphantom {{n_{eL} } {n_{e\Upgamma } }}} \right. \kern-0pt} {n_{e\Upgamma } }} = R\exp \left( { - \Updelta W/k_{B} T_{e} (E)} \right),$$
(16)

where R is the density of states ratio, and ΔW is the energy separation between valleys Γ and L. For GaAs, R ≈ 94, ΔW ≈ 0.3 eV, and the energy relaxation time τ r  ~ 1 ps [23]. Using relations (14)–(16), we can determine numerically the functions T e (E) and μ e (E).

3.2 Material equations of band transport model

Owing to the strong absorption of photons tuned to exciton peaks, PR transitions are dominated by the photogeneration of electron–hole pairs across the band gap. Hence, the creation of carriers by photoionization from deep defects and thermal excitation can be neglected. Heterostructured MQWs such as GaAs/AlGaAs belong to fully compensated materials, where two-level defects of opposite species are assumed in the band gap. In the considered model, the deep defect level of donors fully compensates for the shallow completely ionized acceptors. It is assumed that the shallow level does not participate in transitions. The 1D PR material equations including carrier photogeneration, relaxation, transport, and recombination of carriers are as follows [5, 14]:

$$\frac{{\partial n_{e} }}{\partial t} = \frac{\alpha }{h\nu }I - \gamma_{e} n_{e} N_{D}^{ + } + \frac{1}{q}\frac{{\partial j_{e} }}{\partial z},$$
(17.1)
$$\frac{{\partial n_{h} }}{\partial t} = \frac{\alpha }{h\nu }I - \gamma_{h} n_{h} N_{D}^{0} - \frac{1}{q}\frac{{\partial j_{h} }}{\partial z},$$
(17.2)
$$\frac{\partial }{\partial t}\left( {N_{D}^{ + } - N_{A}^{ - } + n_{h} - n_{e} } \right) = - \frac{\partial }{\partial z}\left( {j_{e} + j_{h} } \right),$$
(17.3)
$$j_{e} = q\mu_{e} (E)En_{e} + k_{B} \frac{\partial }{\partial z}\left( {\mu_{e} (E)T_{e} (E)n_{e} } \right),$$
(17.4)
$$j_{h} = q\mu_{h} En_{h} - k_{B} \mu_{h} T_{L} \frac{{\partial n_{h} }}{\partial z},$$
(17.5)
$$\frac{\partial E}{\partial z} = \frac{q}{{\varepsilon_{0} \varepsilon }}\left( {N_{D}^{ + } - N_{A}^{ - } + n_{h} - n_{e} } \right),$$
(17.6)

where N D  = N 0 D  + N + D , N − A  ≈ N + D are the donor, ionized donor, neutral donor, and ionized acceptor concentrations, respectively. The indices e and h refer to electrons and holes, respectively; n denotes the concentration of free carriers, j is the current density, γ is the recombination coefficient, and μ denotes the mobility of carriers along the quantum wells. The term αI/hν represents the interband carrier generation rate assuming that the quantum efficiency of carrier creation equals unity. The direct electron–hole recombination rate was neglected as being much slower than the recombination rate to traps. The set of equations is supplemented by the boundary condition associated with applying the voltage U to a crystal with length L:

$$U = \int\limits_{L} {E(z){\text{d}}z} .$$
(18)

The values of the parameters used in the calculations are listed in Table 1; the material parameters for GaAs were taken from [2]. Note that as an alternative to the adopted donor model (deep level: donors N D , shallow level: acceptors N A  = rN D  < N D ), the acceptor model can be used (deep level: acceptors N A , shallow level: donors N D  = r′N A  < N A ), where r, r′ < 1 denote the compensation coefficients of deep defects. Both models lead to the same results when we formally substitute \(N_{A}^{ - }\) = rN D  → N 0 A  = (1 − r′)N A , N 0 D  = (1 – r)N D  → N + D  = r′N A .

Table 1 Material parameters used in calculations
Full size table

4 Solutions under small signal approximation

If one of the two interfering beams is shifted in frequency, ω 2 = ω 1 ± Ω, where the frequency detuning Ω << ω 1, ω 2, then the interference pattern

$$I(z,t) = I_{0} (t)\left[ { 1 { } + m \times { \exp }(iKz \pm i\Upomega t)} \right]$$
(19)

moves at a speed v = ω/K, and the carrier gratings and space-charge field travel together with the light distribution.

This is a well-known technique for enhancing the space-charge field amplitude by producing an additional phase shift between the light pattern and the space-charge grating [26]. To find the analytical solutions under the small-contrast approximation, the material equations are linearized by expanding all the variables as a Fourier series to the first order:

$$V(z,t) \approx V_{0} \left( t \right) \, + V_{ 1} \left( t \right){ \exp }\left[ {i(Kz \pm \Upomega t)} \right],$$
(20)

where V 1(t) are complex quantities in which the imaginary parts represent their phase shifts with respect to the light intensity pattern. Furthermore, taking into account the nonlinear electron transport, the quantities μ e (E), T e (E), and v e (E) = μ e (E)E are expanded to the first order of the Taylor series around the average value of the electric field E 0 = E a . For low-intensity beams, the change in the average ionized donor concentration can be neglected (linear recombination approximation), i.e. \(\left\langle {N_{D}^{ + } } \right\rangle \approx N_{A}\) and \(\left\langle {N_{D}^{0} } \right\rangle \approx N_{D} - N_{A}\). As a result, one obtains the zeroth-order solutions for the electron and hole densities:

$${\text{d}}n_{e0} /{\text{d}}t = \alpha I_{0} (t)/h\nu - n_{e0} (t)/\tau_{e} ,\quad {\text{d}}n_{h0} /{\text{d}}t = \alpha I_{0} (t)/h\nu - n_{h0} (t)/\tau_{h} .$$
(21)

In the above relation, \(\tau_{e} = 1/\left( {\gamma_{e} N_{D}^{ + } } \right) = 1/\left( {\gamma_{e} N_{A} } \right)\) and \(\tau_{h} = {1 \mathord{\left/ {\vphantom {1 {\left( {\gamma_{h} N_{D}^{0} } \right)}}} \right. \kern-0pt} {\left( {\gamma_{h} N_{D}^{0} } \right)}} = {1 \mathord{\left/ {\vphantom {1 {\left[ {\gamma_{h} (N_{D} - N_{A} )} \right]}}} \right. \kern-0pt} {\left[ {\gamma_{h} (N_{D} - N_{A} )} \right]}}\) denote the average recombination times for electrons and holes, respectively, and typically τ e,h  < 1 ns.

Note that the described hot electron model is valid if the carrier recombination time is markedly greater than the thermalization time τ r in Eq. (14). Otherwise, carriers cannot reach the thermal equilibrium state. The first-order equations written in matrix notation are as follows:

$$\frac{\text{d}}{{{\text{d}}t}}\left( {\begin{array}{*{20}c} {n_{e1} } \\ {n_{h1} } \\ {N_{D1}^{ + } } \\ \end{array} } \right) = \left( {\begin{array}{*{20}c} { - \Upgamma_{\text{tote}} \pm i\Upomega } & {\Upgamma_{\text{dien}} } & {\Upgamma_{\text{dien}} - \Upgamma_{\text{e}} } \\ {\Upgamma_{\text{dih}} } & { - \Upgamma_{\text{toth}} \pm i\Upomega } & { - \Upgamma_{\text{dih}} + \Upgamma_{\text{h}} } \\ { - \Upgamma_{\text{Re}} } & {\Upgamma_{\text{Rh}} } & { - \Upgamma_{\text{e}} - \Upgamma_{\text{h}} \pm i\Upomega } \\ \end{array} } \right)\left( {\begin{array}{*{20}c} {n_{e1} } \\ {n_{h1} } \\ {N_{D1}^{ + } } \\ \end{array} } \right) + m\frac{\alpha }{h\nu }I_{0} (t)\left( {\begin{array}{*{20}c} 1 \\ 1 \\ 0 \\ \end{array} } \right).$$
(22)

The form of the transition rate matrix is the same for both the nonlinear and linear electron transport models. In the latter, the electron mobility does not change in an electric field, and the equalities μ diff = μ n = μ eΓ and T e  = T L are satisfied (see Table 2) footnote.

Table 2 The rates of transport processes and transitions
Full size table

If the light intensity varies on a time scale much longer than the carrier recombination times, the carrier densities follow the light intensity changes with a negligible delay. This quasi-equilibrium, or so-called adiabatic approximation, allows us to set dn e,h0/dt = 0 in Eq. (21) and dn e,h1/dt = 0 in Eq. (22). Neglecting the small terms in matrix (22), one arrives at a differential equation in N + D1 :

$$\frac{{{\text{d}}N_{D1}^{ + } (t)}}{{{\text{d}}t}} = P\left( m \right) - Q\left( \Upomega \right)N_{D1}^{ + } (t),$$
(23)

where

$$P\left( m \right) = m\frac{\alpha }{h\nu }I_{0} (t)\left( {\frac{{\Upgamma_{\text{Rh}} }}{{\Upgamma_{\text{toth}} }} - \frac{{\Upgamma_{\text{Re}} }}{{\Upgamma_{\text{tote}} }}} \right),\quad Q\left( \Upomega \right) = \frac{{\Upgamma_{\text{Re}} \Upgamma_{\text{dien}} }}{{\Upgamma_{\text{tote}} }} + \frac{{\Upgamma_{\text{Rh}} \Upgamma_{\text{dih}} }}{{\Upgamma_{\text{toth}} }} \pm i\Upomega .$$
(24)

From Eq. (23), we see that the complex space-charge amplitude in a stationary state is given by \(N_{D1}^{ + } (\infty ) = P(m)/Q\left( \Upomega \right)\), and \(\tau_{N} = 1/Q(\Upomega )\) is the complex time constant describing the evolution of the ionized donor density. The term S = − Q(Ω) is one of three eigenvalues of the matrix in Eq. (22). The other two eigenvalues determine the time constants τ ne , τ nh  << τ N governing the evolution of the electron and hole distributions, and referring to the adiabatic approximation, they are not taken into account. Knowing \(N_{1D}^{ + }\), we can determine the amplitude of the space-charge field E sc from Gauss’s law (17.6):

$$E_{{{\text{sc}}1}} = \frac{q}{{i\varepsilon_{0} \varepsilon K}}\left( {N_{D1}^{ + } + n_{h1} - n_{e1} } \right) = \frac{q}{{i\varepsilon_{0} \varepsilon K}}N_{D1}^{ + } ,$$
(25)

where under typical small beam intensities, the carrier densities can be neglected when compared to N +1D . A solution for the space-charge field amplitude on the basis of Eqs. (24) and (25) is given by

$$E_{{{\text{sc}}1}} (t) = E_{1} (\infty )\left[ {1 - \exp ( - t/\tau_{N} )} \right].$$
(26)

The time constant τ N is complex, and the solution in (26) is a combination of exponential and oscillatory functions. The real component of τ N defines the time constant τ sc of the space-charge formation, whereas the imaginary component defines the angular frequency ω sc(Ω) of damped oscillations associated with the recording process according to the expression

$$1/\tau_{N} = 1/\tau_{\text{sc}} + i\omega_{\text{sc}} \left( \Upomega \right).$$
(27)

The angular frequency \(\omega_{\text{sc}} (\Upomega ) = \left| {\omega_{\text{sc}} \left( 0 \right) - \Upomega } \right|\) is the difference between the oscillation frequency ω sc(0) under a stationary light pattern and the frequency offset Ω. Temporal changes in the spatial distribution of the space-charge field grating are described by the real part of E sc1(t), i.e. E sc(z, t) = Re[E sc1(t)exp[i(Kz − Ωt)]. Setting (Kz + ϕ) = 0, where ϕ is the phase shift of the distribution E sc(z, t) with respect to I(z, t), we trace the grating amplitude (26), which can be written as follows:

$$E_{{{\text{sc}}1}} (t) = \left| {E_{1} (\infty )} \right|\left\{ {1 - \exp ( - t/\tau_{\text{sc}} )\cos \left[ {\left( {\omega_{\text{sc}} (0) - \Upomega } \right)t} \right]} \right\},$$
(28)

The physical interpretation of the expressions for E 1(∞), τ sc, and ω sc in terms of the rates Γ obtained from Eqs. (23), (24), and (27) is not legible. Because PMQW samples always work under a high external electric field, we can make an approximation neglecting the diffusion currents, which leads to the equation

$$\frac{{{\text{d}}N_{D1}^{ + } (t)}}{{{\text{d}}t}} \approx m\frac{\alpha }{h\nu }I_{0} \left( {\frac{1}{{1 + iKL_{\text{Eh}} }} - \frac{1}{{1 - iKL_{\text{Ee}} }}} \right) - \left( {\frac{{{1 \mathord{\left/ {\vphantom {1 {\tau_{\text{dih}} }}} \right. \kern-0pt} {\tau_{\text{dih}} }}}}{{1 + iKL_{\text{Eh}} }} + \frac{{{1 \mathord{\left/ {\vphantom {1 {\tau_{\text{dien}} }}} \right. \kern-0pt} {\tau_{\text{dien}} }}}}{{1 - iKL_{\text{Ee}} }} \pm i\Upomega } \right)N_{D1}^{ + } (t),$$
(29)

expressed in terms of the electron and hole drift lengths and dielectric relaxation times given by L Ee = μ e(E)τ e E a , L Eh = μ h τ h E a , and \(\tau_{\text{dien}} = 1/\Upgamma_{\text{dien}}\), \(\tau_{\text{dih}} = 1/\Upgamma_{\text{dih}}\), respectively.

The electron nonlinear dielectric relaxation time τ dien is a function of the applied field, and at high electric fields, τ dien increases greatly owing to the reduction in the nonlinear electron mobility μ n → μ diff. To check the impact of light pattern motion on the space-charge formation, we make one more simplification, assuming KL Eh, KL Ee >> 1, i.e. a large value of the applied field E a . Now, Eq. (29) takes the form

$$i\frac{{{\text{d}}N_{D1}^{ + } (t)}}{{{\text{d}}t}} \approx m\frac{\alpha }{h\nu }\frac{{I_{0} }}{K}\left( {\frac{1}{{L_{\text{Eh}} }} + \frac{1}{{L_{\text{Ee}} }}} \right) - \left( {\frac{1}{{\tau_{\text{dih}} KL_{\text{Eh}} }} - \frac{1}{{\tau_{\text{dien}} KL_{\text{Ee}} }} \pm \Upomega } \right)N_{D1}^{ + } (t).$$
(30)

The first term on the right side of Eq. (30) represents the rate of electron and hole interband photogeneration. For the space-charge field grating, the effective photogeneration rate is modified by the carrier drift lengths. The second term describes the hole and electron dielectric relaxation rates with opposite signs, which are also modified by the drift lengths and wave vector. In the stationary state, this equation may be viewed as a balance equation between the generation and relaxation of electrons and holes. From the approximate Eq. (30), we can see that if Ω = 0, the photogeneration rate and relaxation rate increase in proportion to the light intensity. As a result, the space-charge field amplitude does not depend on the average illumination. This conclusion also remains valid if the full set of equations (22) is applied. To achieve a large amplitude of the E sc field, a low rate of dielectric relaxation is preferable. Under a large applied field, free carriers move over many fringe spacings before they are trapped, which slows the relaxation process. One can see that under the right direction of fringe pattern movement, the relaxation of electrons or holes is diminished.

As a result, the space-charge field grating amplitude may be increased with a properly chosen value of Ω. If Ω is too large, there is not enough time for effective trapping of carriers, and the amplitude of the field E sc decreases. On the other hand, slow dielectric relaxation increases the grating build-up time, reducing the element speed. Neglecting diffusion, on the basis of Eqs. (25) and (29), we obtain the amplitude of the space-charge field as

$$E_{{1{\text{sc}}}} = m\frac{{\alpha I_{0} }}{h\nu }\frac{q}{{\varepsilon_{0} \varepsilon }}\frac{{\left( {L_{\text{Ee}} + L_{\text{Eh}} } \right)}}{{ \pm \Upomega K\left( {L_{\text{Ee}} - L_{\text{Eh}} } \right) - \left( {\frac{1}{{\tau_{\text{dien}} }} + \frac{1}{{\tau_{\text{dih}} }}} \right) + i\left[ {K\left( {\frac{{L_{\text{Ee}} }}{{\tau_{\text{dien}} }} - \frac{{L_{\text{Eh}} }}{{\tau_{\text{dih}} }}} \right) \pm \Upomega \left( {1 + K^{2} L_{\text{Ee}} L_{\text{Eh}} } \right)} \right]}}.$$
(31)

Note that, in contrast to the case of a motionless light pattern, under a moving grating, the magnitude of the space-charge field depends on the average illumination. Because the relaxation times τ dien and τ dih are also functions of the light intensity (τ dien, τ dih ~ 1/I 0), the dependence E sc(I 0) is not proportional to I 0, as the first term in Eq. (31) may suggest.

The phase shift between the space-charge field distribution and the interference pattern can be determined from Eq. (31); it is given by

$$\tan (\Upphi ) = \frac{{K\left( {L_{\text{Ee}} /\tau_{\text{dih}} - L_{\text{Eh}} /\tau_{\text{dien}} } \right) \pm \Upomega \left( {1 + K^{2} L_{\text{Ee}} L_{\text{Eh}} } \right)}}{{\left( {1/\tau_{\text{dien}} + 1/\tau_{\text{dih}} } \right) \pm \Upomega K\left( {L_{\text{Ee}} - L_{\text{Eh}} } \right)}}.$$
(32a)

This expression may be recast in the form

$$\tan (\Upphi ) = \frac{{q\frac{\alpha }{h\nu }I_{0} KE_{a} \mu_{h} \tau_{h} \tau_{e} \left( {\mu_{e} - \mu_{\text{diff}} } \right) \pm \Upomega \varepsilon \left( {1 + \mu_{h} \tau_{h} \mu_{e} \tau_{e} K^{2} E_{a}^{2} } \right)}}{{q\frac{\alpha }{h\nu }I_{0} \left( {\mu_{h} \tau_{h} + \mu_{\text{diff}} \tau_{e} } \right) \pm \Upomega \varepsilon KE_{a} \left( {\mu_{h} \tau_{h} - \mu_{e} \tau_{e} } \right)}},$$
(32b)

which shows explicitly the dependence of the phase shift on the electric field, grating period, and light intensity. In particular, in the presence of the running grating, the phase shift is a function of the light intensity. The resonance for the moving grating increases the E sc amplitude when |Q(Ω)| = min, [see Eq. (24)], which occurs when Im[Q(Ω)] = 0, i.e. ω sc(Ω) = ω sc(0) − Ω = 0. From Eq. (31), the optimum frequency detuning for the moving grating is as follows:

$$\Upomega_{\text{opt}} = \frac{{q\alpha I_{0} }}{{\varepsilon h\nu KE_{a} }}\left( {\frac{{K^{2} L_{\text{Eh}}^{2} }}{{1 + K^{2} L_{\text{Eh}}^{2} }} - \frac{{K^{2} L_{\text{Ee}} L_{\text{Een}} }}{{1 + K^{2} L_{\text{Ee}}^{2} }}} \right).$$
(33)

It can be seen from (33) that Ωopt is proportional to the average light intensity I 0. This relationship stems from the fact that increasing the light intensity leads to faster generation of carriers and a shorter time to record the grating. To maintain the resonance at the accelerated time scale, the fringes must move faster. At the optimum frequency shift, the space-charge field magnitude is as follows:

$$E_{\text{sc1}} \left( {\Upomega_{\text{opt}} } \right) = mE_{a} \frac{{\left( {L_{\text{Ee}} + L_{\text{Eh}} } \right)}}{{L_{\text{Een}} \sqrt {\frac{{1 + K^{2} L_{\text{Eh}}^{ 2} }}{{1 + K^{2} L_{\text{Ee}}^{ 2} }}} + L_{\text{Eh}} \sqrt {\frac{{1 + K^{2} L_{\text{Ee}}^{ 2} }}{{1 + K^{2} L_{\text{Eh}}^{ 2} }}} }}.$$
(34)

The characteristic time τ sc for build-up of the space-charge according to (24) and (27) is as follows:

$$\tau_{\text{sc}} = \frac{{\varepsilon_{0} \varepsilon E_{a} }}{{q\left( {{\alpha \mathord{\left/ {\vphantom {\alpha {h\nu }}} \right. \kern-0pt} {h\nu }}} \right)I_{0} }} \times \frac{{1 + K^{2} L_{\text{Ee}}^{2} }}{{L_{\text{Een}} + L_{\text{Eh}} \frac{{1 + K^{2} L_{\text{Ee}}^{2} }}{{1 + K^{2} L_{\text{Eh}}^{2} }}}}.$$
(35a)

From the above formula, we can see that the motion of the fringes does not affect the grating formation time, whereas this time constant depends on the light intensity and applied electric field. The application of a strong field, on the one hand, shortens the drift length for electrons L Ee because of the smaller mobility μ e(E), which consequently reduces the time τ sc [16, 27]. However, this effect is compensated by increasing the dielectric relaxation time τ diel ~ I −10 (μ diff τ e + μ h τ h)−1, where the differential mobility μ diff may be negative [28]. The application of an external electric field leads to damped oscillations with the frequency

$$\omega_{\text{sc}} (\Upomega ) = \left| {\Upomega_{\text{opt}} - \Upomega } \right|$$
(35b)

in the grating recording process.

5 Predictions of nonlinear transport model for moving grating

In this section, the results of numerical analysis using the nonlinear transport model discussed above are presented in terms of the variation in the grating amplitude, PR gain, and grating kinetics as a function of the frequency shift between two writing beams.

The obtained plots are compared with the corresponding characteristics reported in [16]. The experiment described in that paper was a detailed study of TWM and FWM for a sample of GaAs/AlGaAs PMQWs operating in the Franz–Keldysh geometry using a moving grating technique. For the calculations here, the same values of the parameters are assumed as in [16] for the fringe spacing Λ = 20 μm and the applied field E a  = 7.5 kV/cm. The light intensity is taken as I 0 = 500 μW/cm2. The other parameter values are listed in Table 1.

In particular, the compensation coefficient is assumed to be r = N A /N D  = 0.5, so the average concentration of holes is twice that of electrons. For this value of r, the models with deep donor defects and with deep acceptor defects both yield the same results. To emphasize the role played by nonlinear electron transport in the PMQW response, the solutions according to the linear transport model, i.e. without taking into account hot electron transport, are also shown for comparison.

For the moving grating experiment with wave mixing, the PR response of the PMQW sample varies with the applied field polarity or, equivalently, with the direction of the light pattern motion. In an experiment, it is easier to change the sign of the electric field. In the above formulas, the signs simply have to be interchanged: +Ω to −Ω.

The modulus of the space-charge field amplitude is plotted in Fig. 2a as a function of the frequency offset for positive and negative electric field polarity. Note that, considering the linear transport model under the motionless interference pattern and neglecting the diffusion current, we obtain the space-charge field amplitude E sc = −mE a (here, 0.75 kV/cm). Formally, we get this outcome by noting that the fourth term in the denominator in expression (31) equals zero. As one can see, including hot electron transport may significantly increase the space-charge field amplitude. In addition, in the presence of moving fringes, resonance occurs for different polarities of the applied electric field, as we can see by comparing the solutions from the linear and nonlinear transport models.

Fig. 2
figure 2

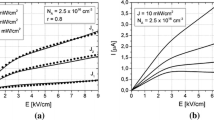
a Space-charge field amplitude and b PR phase shift as a function of frequency detuning calculated from nonlinear and linear transport models for positive and negative external electric fields. Experimental data points from [16] are also shown. Applied field and fringe spacing are assumed to be E a  = 7.5 kV/cm and Λ = 20 μm, respectively. Values of other parameters used in simulations are listed in Table 1

Full size image

Figure 2b illustrates the phase shift (32) of the space-charge field grating with respect to the light pattern. At low frequency detuning due to hot electron transport, the phase shift is close to π/2 and near zero in the linear transport theory. During the motion of the fringes, an additional dynamic phase shift is induced because the grating lags relative to the light pattern. Depending on the direction of the field polarization, static and dynamic shifts are added or subtracted. In the first case (+E a in Fig. 2b) for frequencies Ω > Ωopt, the phase shift reaches nearly π/2, whereas in the second case for Ω > Ωopt, the phase shift goes through zero, changes sign, and tends towards −π/2. Similar behaviour is predicted by the linear electron transport model. The PR phase shift was investigated experimentally in [16], and data points taken from this paper are plotted in Fig. 2b for comparison. The experiment gave a slightly different resonant frequency detuning, and hence a shift in the experimental and theoretical graphs relative to each other. For negative field polarity, the plot exhibits good qualitative agreement with the experimental results (black circles). However, for the opposite field polarity, the model does not provide for the drop in the PR shift to zero for large values of Ω, which was observed in the experiment (white circles). Within the considered model, the decrease in the phase shift at high values of Ω is expected when the electron concentration is much larger than the hole concentration. However, this assumption seems to be in contradiction with the results of electrical measurements in PMQW samples [3]. It should be noted that the given solutions were obtained under a small-contrast approximation, whereas in the wave mixing experiment, the ratio of the interfering beams intensities was close to unity. Therefore, to verify the indicated discrepancy, it is necessary to analyse the model at a large modulation depth, but this requires resorting to numerical methods. Numerical simulations for the high fringe contrast of the motionless interference pattern in PMQWs have been conducted [29]. Among other things, it was found that the static PR phase shift does not depend on the modulation depth. However, an additional dynamic shift appears for a moving grating; hence, the total shift may change. The PR gain Γ in TWM defined by Eq. (11) versus the frequency detuning is shown in Fig. 3a, and the diffracted first-order signal in FWM given by Eq. (13) is displayed in Fig. 3b. In this case, the characteristics for both directions of the field polarity exhibit good qualitative conformity with the experimental graphs in [16].

Fig. 3
figure 3

a PR gain according to Eq. (11) in TWM and b diffraction efficiency according to Eq. (16) in FWM versus detuning frequency predicted by nonlinear and linear transport models for positive and negative voltage polarity. All conditions are identical to those given in Fig. 2

Full size image

Note by the way that, the optimum frequency detuning (Ωopt = 15 kHz) for the assumed values of the parameters is close to the value found in the experiment (about 10 kHz).

The optimum detuning frequency is a function of the light intensity and the electric field, as shown in Fig. 4a, b. On the basis of Eq. (33), a linear relationship of Ωopt versus the average light intensity is expected (Fig. 4a). The same relationship was obtained in the experiment. Figure 4b shows the dependence of Ωopt on the applied field. The linear and nonlinear models predict significantly different behaviour. In the latter case, for an applied field >4 kV/cm, the optimum frequency reaches a constant value of about 15 kHz, in contrast to the prediction of the linear transport model.

Fig. 4
figure 4

a Optimum detuning between writing beams as a function of average light intensity and b dependence of optimum detuning on applied electric field according to linear and nonlinear transport models

Full size image

The predictions of the models regarding the dynamics of the PR response in PMQWs during grating recording are presented in Fig. 5a, b. The transient behaviour under a stationary interference pattern was analysed in [28]. Here, the effect of the frequency detuning on the grating kinetics is shown. The real part of the complex time constant (27), which defines the time to formation of the grating (35a), does not depend on the frequency offset, whereas the oscillation period changes with the detuning frequency according to Eq. (35b).

Fig. 5
figure 5

a Oscillation period during recording of PR grating versus frequency detuning. For resonance detuning, complete attenuation of oscillations is observed. b Time evolution of space-charge field amplitude in response to step-like illumination

Full size image

For the resonance condition Ω = Ωopt, the oscillations disappear completely (T → ∞), as we can see in Fig. 5a. Figure 5b shows the temporal evolution of the amplitude of E sc in response to step-like illumination (abruptly switched on writing beams). The time is normalized by the dielectric relaxation time (about 42 μs). At high frequency differences (Ω >> Ωopt), the amplitude of E sc decreases, and the oscillations become more noticeable.

To sum up, the studied model of PR transport in PMQWs quite successfully explains the wave mixing experimental results. However, for large values of Ω, a discrepancy was observed in the behaviour of the dynamic PR phase shift.

The PR response of PMQWs strongly depends on the number of dominant photocarriers. For the assumed value of the defect concentrations N D and N A , the average density of holes is about twice that of electrons. Changing this ratio may profoundly affect the PR properties in the presence of travelling fringes. This is illustrated in Fig. 6a, b.

Fig. 6
figure 6

Compensation donor–acceptor ratio of r = 0.9 is assumed, corresponding to a ratio of free carrier densities n h0/n e0 ≈ 20. a A greatly enhanced PR gain in TWM is predicted by the nonlinear model. b Diffracted signal in FWM from the first spatial harmonic grating is also sharply increased

Full size image

For example, assuming the compensation coefficient r = N A /N D  = 0.9 in the considered donor model, which corresponds to r = N D /N A  = 0.1 in the acceptor model, the ratio of the hole density to the electron density increases to n h0/n e0 ≈ 20. For a small detuning frequency, it reduces the TWM gain (Fig. 6a) and the diffraction efficiency in FWM (Fig. 6b), but simultaneously causes a dramatic enhancement of the resonance magnitude. From simulations in which the other parameters values were unchanged, we obtain Γ approaching the value of 3,000 cm−1 and a several-fold increase in the diffraction efficiency in FWM.

Note that the occurrence of a large value of the space-charge field under resonance renders the use of a small signal approximation inadequate. In this situation, numerical techniques have to be applied, especially in an analysis of high-contrast cases. The occurrence of such a strong resonance is a direct consequence of nonlinear electron transport. The linear transport model does not predict a similar enhancement of the PR response.

6 Conclusions

This work presents a theoretical analysis of TWM and FWM in semi-insulating films of PMQWs working in the Franz–Keldysh geometry and the presence of a moving grating. Calculations for the steady and temporally evolving states are based on a band transport model, considering the hot electron effect under a small signal approximation. The adopted material parameters are those of the GaAs/AlGaAs system.

The simulation results for the stationary state were compared with available experimental data. Qualitative conformity was found between the variations in the gain Γ, diffraction signal, and PR shift as a function of the detuning frequency for the writing beams. The only exception is a discrepancy in the phase shift resulting from the addition of static and dynamic shifts. To confirm this result, simulations should be made for a beam intensity ratio close to one. Interpreting the results in the context of theoretical model verification, we can state that the simple model including nonlinear electron transport satisfactorily explains most of the observed properties of semi-insulating PMQWs. The single observed discrepancy remains to be explained.

In summary, hot electron transport in PMQWs has a significant and beneficial influence on the formation of a PR grating in a wave mixing geometry under moving fringes. In particular, the nonlinear model predicts an intriguing possibility of observing a very strong resonance, provided that the hole current strongly dominates the electron current.