1 Introduction

In the past decades, dark hollow beam (DHB) whose intensity in the beam center is zero has been studied extensively due to its important applications in free-space optical communications, laser optics, particles trapping, medical sciences, atomic and binary optics [1–36]. Up to now, several theoretical models have been proposed to describe various DHBs [10–20]. As a special kind of DHB, hollow Gaussian beam (HGB) was introduced by Cai et al. [13] 10 years ago, and it displays unique propagation properties which are much different from those of the conventional DHBs, such as Bessel Gaussian beam [10], TEM *01 beam (also known as doughnut beam) [11], and Mathieu beam [12]. The properties of the M2-factor of a HGB with and without aperture were reported in [21, 22]. Focusing properties of a HGB were studied in [23, 24]. Propagation properties of a HGB through apertured aligned and misaligned optical systems were investigated in [25, 26]. Non-paraxial propagation properties and vectorial structure of a HGB were explored in [27–31]. Propagation properties of a HGB in a magnetoplasma were examined in [32]. Phase singularities of a focused HGB beam were studied in [33]. Several methods have been proposed to generate a HGB [34–36]. Recently, Weitenberg et al. [37] demonstrated quasi-imaging in a high-finesse femtosecond enhancement cavity, which can be used to tailor the transverse mode and generate DHB by inserting an obstacle in the beam path. In this paper, we propose a new method which is based on outer-cavity modulation to generate a HGB through transforming a Laguerre–Gaussian beam into a HGB, and we successfully generate a HGB in experiment based on the proposed method. The focusing properties of the generated HGB are also studied and compared with the theoretical results.

2 Theory

According to [13], the electric field of a HGB at the source plane is defined as

$$E(r,\theta ) = \left( {\frac{{r^{2} }}{{\omega_{0}^{2} }}} \right)^{n} \exp \left( { - \frac{{r^{2} }}{{\omega_{0}^{2} }}} \right),$$
(1)

where \(r,\theta\) are the radial and azimuthal (angle) coordinates, n is the order of the HGB, when n = 0, Eq. (1) reduces to the electric field of a fundamental Gaussian beam, \(\omega_{0}\) represents the source beam waist size of a fundamental Gaussian beam. As shown in Fig. 1, the area of the dark region increases as the value of the beam order n increases.

Fig. 1
figure 1

Normalized intensity distribution of a HGB for different values of n with ω 0 = 1 mm

Full size image

Applying the following expansion formula [38]

$$x^{2n} = \frac{n!}{{2^{n} }}\sum\limits_{m = 0}^{n} {\left( { - 1} \right)^{m} \left( {\begin{array}{*{20}c} n \\ m \\ \end{array} } \right)L_{m}^{0} \left( {2x^{2} } \right)} , \quad n = 0,1, \ldots$$
(2)

where \(\left( {\begin{array}{*{20}c} n \\ m \\ \end{array} } \right)\) denotes a binomial coefficient and \(L_{m}^{0}\) is the mth Laguerre polynomial with radial index m and azimuthal index 0, we also can express the electric field of a HGB as a superposition of a series of Laguerre–Gaussian modes with radial index m and azimuthal index 0

$$E(r,\theta ) = \frac{n!}{{2^{n} }}\sum\limits_{m = 0}^{n} {\left( { - 1} \right)^{m} \left( {\begin{array}{*{20}c} n \\ m \\ \end{array} } \right)L_{m}^{0} \left( {2\frac{{r^{2} }}{{\omega_{0}^{2} }}} \right)\exp \left( { - \frac{{r^{2} }}{{\omega_{0}^{2} }}} \right)} ,$$
(3)

The electric field of a Laguerre–Gaussian beam with radial index 0 and azimuthal index l at the source plane is expressed as [39]

$$E(r,\theta ) = \left( {\frac{r}{{\omega_{0} }}} \right)^{l} \exp \left( { - \frac{{r^{2} }}{{\omega_{0}^{2} }}} \right)\exp \left( {il\theta } \right).$$
(4)

Comparing Eqs. (1) and (4), one finds that the only difference between them is the spiral phase term \(\exp \left( {il\theta } \right)\) if the condition \(l = 2n\) is satisfied. Thus, one can expect to generate a HGB with n = l/2 from a Laguerre–Gaussian beam with radial index 0 and azimuthal index l by counteracting the spiral phase term \(\exp \left( {il\theta } \right)\). A Laguerre–Gaussian beam can be generated experimentally by cooperative frequency locking in a helium–neon laser, in fiber-coupled laser diode end-pumped lasers [40–43], through the conversion of Hermite–Gaussian beam by an astigmatic mode converter [44, 45] or through a computer hologram [46, 47]. If the experimentally generated Laguerre–Gaussian beam with radial index 0 and azimuthal index l passes through a spiral phase plate with transmission function \(T\left( {r,\theta } \right) = \exp \left( { - il\theta } \right)\), the intrinsic spiral phase term \(\exp \left( {il\theta } \right)\) will be counteracted, and the transmitted beam will become a HGB with n = l/2.

Within the framework of the paraxial approximation, the propagation of a laser beam through a paraxial optical system can be treated by the following Collins formula [48]

$$E(r_{1} ,\theta_{1} ) = \frac{ik}{2\pi b}\int\limits_{0}^{\infty } {\int\limits_{0}^{2\pi } {E(r,\theta )\exp \left[ { - \frac{ik}{2b}\left( {ar^{2} - 2rr_{1} \cos (\theta_{1} - \theta ) + dr_{1}^{2} } \right)} \right]} } r{\text{d}}r{\text{d}}\theta ,$$
(5)

where a, b, c, d are the elements of the transfer matrix of the paraxial optical system.

Substituting Eq. (1) into (5), we can obtain the following analytical propagation formula for a HGB passing through a paraxial ABCD optical system [13]

$$E(r_{1} ,\theta_{1} ) = \frac{ikan!}{{2b\omega_{0}^{2n} }}\left( {\frac{1}{{\omega_{0}^{2} }} + \frac{ika}{2b}} \right)^{ - n - 1} \exp \left( { - ikz - \frac{{ikdr^{2} }}{2b}} \right)\exp \left[ { - \frac{{\left( \frac{kr}{2b} \right)^{2} }}{{\left( {\frac{1}{{\omega_{0}^{2} }} + \frac{ika}{2b}} \right)}}} \right]L_{n}^{0} \left[ {\frac{{\left( \frac{kr}{2b} \right)^{2} }}{{\left( {\frac{1}{{\omega_{0}^{2} }} + \frac{ika}{2b}} \right)}}} \right]$$
(6)

In the above derivations, the following integral formulae have been used [49]

$$J_{0} \left( x \right) = \frac{1}{2\pi }\int\limits_{0}^{2\pi } {\exp \left( {ix\cos \theta^{'} } \right){\text{d}}\theta^{'} } ,$$
(7)
$$\int\limits_{0}^{\infty } {\exp \left( { - pt} \right)t^{v/2 + n} J_{v} \left( {2\alpha^{1/2} t^{1/2} } \right)dt = n!a^{v/2} p^{ - (n + v + 1)} \exp \left( { - a/p} \right)L_{n}^{v} \left( {a/p} \right)}$$
(8)

With the help of Eq. (6), one can study the propagation properties of a HGB conveniently. As shown in [13], the propagation properties of a HGB in free space are much different from those of the conventional DHBs, such as Bessel Gaussian beam and TEM *01 beam; its beam profile varies on propagation in free space, and its DHB profile disappears totally in the far field. Due to the existence of the spiral phase term in the conventional DHBs, the beam profiles of the conventional DHBs remain invariant on propagation although their beam spots spread on propagation.

As a numerical example, we study the focusing properties of a HGB. The focusing geometry is shown in Fig. 2. The transfer matrix between the source plane and the output plane is given as

$$\left( {\begin{array}{*{20}c} a & b \\ c & d \\ \end{array} } \right) = \left( {\begin{array}{*{20}c} 1 & {l_{2} } \\ 0 & 1 \\ \end{array} } \right)\left( {\begin{array}{*{20}c} 1 & 0 \\ { - 1/f} & 1 \\ \end{array} } \right)\left( {\begin{array}{*{20}c} 1 & {l_{1} } \\ 0 & 1 \\ \end{array} } \right) = \left( {\begin{array}{*{20}c} {1 - l_{2} /f} & {l_{1} + l_{2} - l_{1} l_{2} /f} \\ { - 1/f} & {1 - l_{1} /f} \\ \end{array} } \right)$$
(9)
Fig. 2
figure 2

Focusing geometry

Full size image

We calculate in Fig. 3 the normalized intensity distribution (contour graph) and the corresponding cross line ( \(y_{1} = 0\)) of a focused HGB at several propagation distances with \(n = 1\), \(\omega_{0} = 1\,{\text{mm}}\), \(l_{1} = f = 40\,{\text{cm}},\) and \(\lambda = 632.8\,{\text{nm}}\). One finds from Fig. 3 that the focusing properties of a HGB are also different from those of the conventional DHBs with a spiral phase term. The intensity distributions of the focused conventional DHBs with a spiral phase term always have DHB profiles on propagation, while the intensity distribution of a focused HGB varies on propagation, and its DHB disappears at the focal plane. One can explain this phenomenon by the fact that the HGB is not a pure mode, but a superposition of a series of Laguerre–Gaussian modes (see Eq. 3). Different modes overlap and interfere on propagation, thus leading to the unique focusing properties of a HGB.

Fig. 3
figure 3

Normalized intensity distribution (contour graph) and the corresponding cross line ( \(y_{1} = 0\)) of a focused HGB with \(n = 1\)at several propagation distances

Full size image

3 Experimental results

In this section, based on the method proposed in Sect. 2, we carry out experimental generation of a HGB and study the focusing properties of the generated HGB.

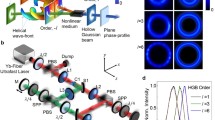
Figure 4 shows our experimental setup for generating a HGB and measuring its focused intensity. A beam emitted from the He–Ne laser ( \(\lambda = 632.8\,{\text{nm}}\)) is reflected by a reflecting mirror, and then, it passes through a beam expander (BE). The beam from the BE goes toward a spatial light modulator (SLM, Holoeye LC2002), which acts as a grating with fork pattern designed by the method of computer-generated holograms. The first-order diffraction pattern of the beam from the SLM is regarded as a Laguerre–Gaussian beam with radial index 0 and azimuthal index l and is selected out by a circular aperture. The order l of the generated Laguerre–Gaussian beam equals to the number of the dislocations in the grating [50], and thus, we can generate a Laguerre–Gaussian beam with different l by introducing different fork patterns onto the SLM. The generated Laguerre–Gaussian beam passes through a spiral phase plate with transmission function \(T\left( {r,\theta } \right) = \exp \left( { - il\theta } \right)\) and then becomes a HGB with \(n = l/2\). The intensity distribution of the generated beam is measured by a beam profile analyzer (BPA).

Fig. 4
figure 4

Experimental setup for generating a HGB and measuring its focused intensity. RM reflecting mirror, BE beam expander, SLM spatial light modulator, CA circular aperture, SPP spiral phase plate, BPA beam profile analyzer, PC personal computer

Full size image

In our experiment, the order l is designed to be 2, and then, the order n of the generated HGB equals to 1. Figure 5 shows our experimental results of the intensity distribution and the corresponding normalized intensity distribution at cross line y = 0 (dotted curve) of the generated HGB beam with n = 1 just after the SPP. The solid curve is a result of the theoretical fit for the experimental data. From Fig. 5, one sees that we indeed generate a HGB in experiment based on the proposed method. There is a small but undesired side lobe around the HGB, which may be caused by the diffraction effect of the grating with fork pattern, while this undesired side lobe almost does not affect the propagation properties of the generated HGB as shown later. From the theoretical fit of the experimental data, the beam waist size is found to be \(\omega_{0} \approx 0.75{\text{mm}}\).

Fig. 5
figure 5

Experimental results of the intensity distribution and the corresponding normalized intensity distribution at cross line y = 0 (dotted curve) of the generated HGB beam with n = 1 just after the SPP. The solid curve is a result of the theoretical fit for the experimental data

Full size image

Now we study the focusing properties of the generated HGB experimentally. In Fig. 4, the generated HGB from the SPP first passes through a thin lens with focal length \(f = 40\,{\text{cm}}\) and then arrives at the BPA, which measures the focused intensity. The distance \(l_{1}\) between the SPP and the thin lens equals to f, and the distance between the thin lens and the SPA is \(l_{2}\). The transfer matrix between the SPP and the BPA is given by Eq. (9). Figure 6 shows our experimental results of the intensity distribution and the corresponding normalized intensity distribution at cross line \(y_{1} = 0\) (dotted curve) of the generated HGB beam with n = 1 after passing through a thin lens at several propagation distances. For the convenience of comparison, the theoretical results (solid curves) calculated by Eqs. (6) and (9) with the beam parameters n = 1 and \(\omega_{0} \approx 0.75\,{\text{mm}}\) are also shown in Fig. 6. One finds from Fig. 6 that the beam profile of a focused HGB indeed varies on propagation, and the DHB profile disappears at the focal plane as expected in Fig. 3. Furthermore, our experimental results agree quite well with the theoretical results. Thus, our new method for generating a HGB is reliable.

Fig. 6
figure 6

Experimental results of the intensity distribution and the corresponding normalized intensity distribution at cross line \(y_{1} = 0\) (dotted curve) of the generated HGB beam with n = 1 after passing through a thin lens at several propagation distances. The solid curves denote the theoretical results calculated by Eqs. (6) and (9)

Full size image

4 Conclusion

In conclusion, we have proposed a new method to generate a HGB by transforming a Laguerre–Gaussian beam with radial index 0 and azimuthal index l into a HGB with mode n = l/2. In order to verify the feasibility of our method, we have successfully carried out experimental generation of a HGB with n = 1 by imposing a spiral phase on a Laguerre–Gaussian beam with radial index 0 and azimuthal index 2. We also have studied the focusing properties of the generated HGB both theoretically and experimentally, and our results are found to be consistent with the theoretical predictions. Our method provides a simple and convenient way for generating a HGB, which will be useful for its applications in particle trapping and free-space optical communications.