1 Introduction

Absorption imaging is a sensitive technique for measuring atomic densities with spatial resolution. It is widely used in experiments with ultracold atomic gases [1], as well as cold neutral plasmas [2] and molecules [3]. Recent experiments have even demonstrated absorption imaging of single atoms [4]. Here, we report on absorption imaging of mesoscopic atomic clouds with a resolution of \(\varDelta_{\rm det}=3.7\) atoms. In the following sections, we will give a short introduction to our experimental setup and the imaging calibration procedure. We will then discuss the sources of detection noise, the methods used for analysis and finally point out limits for the atom number resolution.

2 Experimental setup

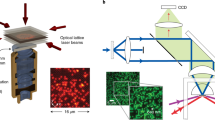
In our experimental setup, we realize up to 35 spatially separated Bose–Einstein condensates (BEC) of 87Rb, each containing 50–600 atoms (see details in [5]). The laser beam for absorption imaging is tuned to resonance with respect to the D2 line of 87Rb near 780 nm and has a diameter of 2 mm. The shadow of the atomic cloud is imaged onto a CCD camera (Princeton Instruments PIXIS BR1024) using an objective with a high numerical aperture (NA = 0.45, f 1 = 31.23 mm) and a secondary lens with a focal length of f 2 = 1,000 mm. This leads to an effective magnification of 30.96. The depth of field was measured to be 6.8 μm, which is larger than the spatial extent of the cloud, but, as will be shown in Sect. 5, limits the atom number resolution. Two bandpass filters centered at 780 nm (Semrock BrightLine HC 780/12) with a width of 24 nm block stray light from the dipole traps. We deduce a total quantum efficiency of 0.79 for the combined optical imaging setup including the CCD camera. The imaging resolution according to the Rayleigh criterion is 1.1 μm, which is smaller than the distance of 5.5 μm between the individual BEC clouds.

The column density of the atoms is deduced from an absorption image and a reference image taken in the absence of atomic absorption. Both images are corrected for a constant CCD camera offset.

The density of the atomic sample can be extracted using the Beer–Lambert law. Assuming resonant light and including saturation effects, the Beer–Lambert law for absorption in the direction z of the imaging beam propagation reads [6]

$$ \frac{\hbox{d}I}{\hbox{d}z}=-n\sigma_0 \cdot \left(\frac{1}{\alpha+\frac{I}{I_{\rm sat}}}\right)\cdot I, $$
(1)

where I is the intensity of the imaging light, n is the density of the atomic cloud, σ 0 is the resonant scattering cross section, α is a dimensionless parameter correcting for polarization and optical pumping effects, and I sat is the polarization-dependent saturation intensity of the atomic transition. In units of counts on the CCD camera, this quantity also includes the microscopic properties of our experimental system and thus needs to be calibrated (see Sect. 3). Equation 1 can be integrated along the beam direction and solved for the atomic column density, yielding [6]

$$ n_c=\int n \hbox{d}z=-\frac{1}{\sigma_0} \left[\alpha \cdot \ln\left(\frac{I_{\rm f}}{I_{\rm i}}\right)+\frac{I_{\rm f}-I_{\rm i}}{I_{\rm sat}}\right], $$
(2)

with the initial intensity I i and the final intensity I f after passing through the cloud. After evaluating the column density for each pixel, the atom number is extracted by summing over a small region of interest around each cloud (rectangular or oval mask, see Fig. 1).

Fig. 1
figure 1

A typical experimental image. The hyperfine components are separated by a Stern–Gerlach pulse and subsequently imaged. Using the absorption and the reference image, the atomic column density is calculated for each pixel using Eq. 2. The number of atoms in each component is calculated by summing over all pixels in a region of interest around the individual clouds. The areas without atoms (outlined in green) are used to evaluate the detection noise

Full size image

The atoms are released from the trap 1.3 ms before the imaging pulse in order to reduce the variation of the column density over the pixel size (420 nm in object space), as the atomic cloud expands by a factor of three during time of flight. This ensures the correspondence of the observed absorption for each pixel to the column density as given in Eq. 2. It is important to note that this connection is nonlinear.

The linearly polarized imaging light is tuned on resonance with respect to the transition between the \(F=2 \rightarrow F^{\prime}=3\) hyperfine levels and lasts for 15 μs. For imaging atoms in the F = 1 manifold of the ground state, repumping light first transfers all atoms into the F = 2 manifold.

The reference image is taken with a delay of 1.25 ms after the absorption image. Since the atoms acquire velocity during the imaging process due to photon recoil (see Sect. 5), after this time all atoms have left the field of view. Thus, no spurious atomic absorption signal is present on the reference image.

3 Atom number calibration using coherent spin states

The atom number can be deduced from the measured intensity profiles on the CCD camera if α and I sat are known. These parameters are determined experimentally as they are connected to the microscopic details of the system of interest, e.g., polarization, quantum efficiency of the whole system and residual detuning. This is accomplished by observing the characteristic particle number fluctuations, i.e., quantum projection noise [7], of a coherent spin state and ensuring that they are independent of imaging intensity. The coherent spin state is realized by preparing N tot independent particles in a superposition of two Zeeman sublevels of the 52 S 1/2 ground state, \(|1\rangle \equiv |F=1,m_F=-1\rangle\) and \(|2\rangle \equiv |F=1, m_F=+1\rangle.\) This is achieved using a two-photon transition corresponding to a π/2 pulse as shown in the inset of Fig. 2a. The different Zeeman substates are spatially separated by a Stern–Gerlach pulse and the atom number difference N − is detected with a single absorption image. For this coherent spin state, the probability of measuring N 2 particles in state \(|2\rangle\) is

$$ P(N_{2}; N_{\rm tot},p)=\frac{N_{\rm tot}!}{N_{2}!(N_{\rm tot}-N_{2})!}p^{N_{2}} (1-p)^{N_{\rm tot}-N_{2}}, $$
(3)

where p is the single atom probability of being in state \(|2\rangle.\) The variance of this binomial distribution is given by

$$ \varDelta^2 (N_2;N_{\rm tot},p)=\varDelta^2 (N_1;N_{\rm tot},1-p)=p(1-p)N_{\rm tot}, $$
(4)

and the covariance

$$ \hbox{Cov} (N_{1},N_{2})=-p(1-p)N_{\rm tot} $$
(5)

has the same absolute value but opposite sign. Measuring the variance of N −, one expects

$$ \varDelta^{2} N_{-} =\varDelta^2 N_{1}+\varDelta^2 N_{2}-2\cdot \hbox{ Cov}(N_{1},N_{2})+\varDelta^2_{\rm det} $$
(6)
$$ \varDelta^{2} N_{-} =4p(1-p)\langle N_{\rm tot}\rangle+\varDelta^2_{\rm det} $$
(7)

assuming only an additional detection noise term \(\varDelta^2_{\rm det}\) that does not depend on atom number. Thus, an accurate determination of α and I sat results in a linear dependence of \(\varDelta^2 N_-/(4p(1-p))\) on the atom number with unity slope for all imaging intensities (see inset Fig. 2a). The offset, however, is intensity dependent as the detection noise varies with intensity. An example for a calibration graph is shown in Fig. 2a.

Fig. 2
figure 2

Characteristic fluctuations of a coherent spin state. We prepare the atoms in a superposition state of the hyperfine levels \(|1\rangle\) and \(|2\rangle,\) corresponding to a π/2 pulse. The pure state of the N tot particle system can be mapped onto a generalized Bloch sphere with radius J = N tot/2 and z projection J z = N −/2 [12]. a For the right choice of the parameters α and I sat, the variance of the atom number difference \(\varDelta^2 N_{-}/(4p(1-p))\) scales linearly with the measured total atom number N tot with unity slope for all intensities (inset). The main graph shows the data (red) and fit (green line) for 11 I sat, the dashed blue line indicates unity slope. b Technical noise in the preparation pulse corresponds to an error δ tech in the preparation angle (inset), which leads to a quadratic noise term \(\varDelta_{\rm tech}^2=(\delta_{\rm tech} \cdot N_{\rm tot})^2.\) This is illustrated by a calibration measurement in the presence of increased technical noise. Here, fluctuations in the Rabi rate of about 5 % lead to a deviation from the characteristic linear dependence for the fluctuations of a coherent spin state. For all other measurements, this noise source was removed

Full size image

Equation 7 assumes the ideal case, in which the coherent spin state is prepared in a perfectly reproducible manner. Technical noise in the preparation pulse, such as fluctuations of the irradiated radio frequency power, introduces an uncertainty δ tech in the rotation angle, as indicated in the inset of Fig. 2b. This results in a quadratic variance contribution \(\varDelta^2_{\rm tech}=\left(N_{\rm tot}\cdot \delta_{\rm tech}\right)^2.\) An example of a calibration measurement in the presence of increased technical noise due to power fluctuations of a microwave source is given in Fig. 2b. This technical noise source was removed for all other measurements and the respective data was not used for calibration purposes.

4 Limits on atom number resolution

In our experiment, the most important contribution to the detection noise is photon shot noise (PSN) from the imaging light, but other sources like noise from interference fringes and the readout noise from the CCD camera also contribute.

One measure for the detection noise is the offset \(\varDelta^2_{\rm det}\) in the atom number calibration. A more precise value for \(\varDelta^2_{\rm det}\) can be determined by calculating the variance of N − in regions without atomic absorption signal. We checked that the detection noise is homogeneous over the whole picture by analyzing pictures without atomic signal. The values for the total detection noise derived from both methods agree and are shown in Fig. 3. The Gaussian behavior of the detection noise is confirmed by examining the distribution function of N − in empty regions of the image (see inset of Fig. 3). In the following, we will discuss in detail the different noise sources contributing to the observations.

Fig. 3
figure 3

Detection noise for different evaluation methods. The detection noise \(\varDelta_{\rm det}\) can be significantly reduced by evaluating the atom numbers in elliptically masked areas instead of rectangular boxes, as shown in Fig. 1. Here, the photon shot noise calculated from Eq. 8 typically contributes around 80 % of the detection noise. By finding the optimal reference images using the algorithm described in [8], both the fringe noise and PSN of the reference image are reduced. For each method, the fit offset of the atom number calibration (green squares) agrees with the result obtained in empty regions. We find a detection noise of \(\varDelta_{\rm det}=3.7\) atoms with a Gaussian distribution (inset)

Full size image

4.1 Photon shot noise

Since the imaging process is performed with a classical light source, i.e., coherent laser radiation, the detected number of photons on each pixel fluctuates by at least \(\sqrt{\langle N_{\rm phot} \rangle}\) for a given mean photon number \(\langle N_{\rm phot} \rangle.\) For each measurement, this noise is present on both the absorption and the reference image. These fluctuations in the photon signal contribute to the uncertainty of the deduced atomic column density. This can be quantified by propagating errors in Eq. 2 concerning variations in absorption image \(\varDelta^2 I_{\rm f}\) and reference image \(\varDelta^2 I_{\rm i},\) yielding

$$ \varDelta^2 n_c =\frac{1}{\sigma_0^2}\left(\left[\frac{\alpha}{I_{\rm f}}+\frac{1}{I_{\rm sat}}\right]^2\varDelta^2 I_{\rm f} +\left[\frac{\alpha}{I_{\rm i}}+\frac{1}{I_{\rm sat}}\right]^2 \varDelta^2 I_{\rm i}\right). $$
(8)

The photon shot noise contribution to the detection noise can be estimated from the pixel intensity using the fit results of a noise calibration measurement of the CCD camera. This is done by homogeneously illuminating the camera with incoherent light and measuring the variance of the counts on the CCD chip for different mean count numbers. For this calibration, a flat-field correcting analysis is employed to cancel small pixel-to-pixel variations of the CCD sensitivity.

In our setup, photon shot noise accounts for almost 80 % of the total detection noise, as can be seen in Fig. 3.

4.2 Other noise sources

Fringes due to the imaging with coherent laser light, e.g., caused by small dust particles on the optical components, can add to the detection noise. Ideally, those fringes do not contribute additional noise since the reference image exhibits the same fringe structure. However, external perturbations, such as mechanical vibrations of the imaging optics or air movement, may shift the fringes between the absorption and the reference image, leading to a remaining fringe structure on the deduced atomic density.

The readout amplifier of the CCD chip also adds noise. The amplitude of the readout noise can be quantified by the variance of a dark image of the CCD. We use the slowest readout mode of our camera, which gives a total contribution for \(\varDelta^2_{\rm det}\) of typically 0.4 atom2 and is therefore only a small contribution in our setup.

4.3 Minimizing the detection noise

The photon shot noise contribution is about equal for all pixels, independent of whether they contain absorption signal or not. For obtaining minimal detection noise for our atom signal, all pixels that do not contain atomic absorption are removed. We do this in post-processing by masking an area around the atomic sample that has the same elliptical shape as the cloud. To make sure no atomic signal is lost by the mask, the size of the ellipse is varied and the final size is chosen such that the deduced atom number is well saturated.

Both photon shot noise from the reference image and fringe noise can be reduced by applying the fringe removal algorithm described in [8]. The algorithm constructs an optimal reference image r opt from a linear combination of different reference images r opt = ∑ i c i r i . The coefficients c i for the different reference images r i are found by least squares fitting of r opt to empty regions of the absorption image.

In our case, we use a combination of about 700 different reference images that were taken after each of the absorption images during the same measurement period. The optimized image is used as a reference for the entire BEC array. The main effect of this procedure is to reduce photon shot noise on the optimal reference image.

4.4 Experimentally obtained atom number resolution

For detecting a few hundred atoms, we use an imaging intensity of 11 I sat and a pulse duration of 15 μm which yield close to optimal signal-to-noise ratio for our setup. For the evaluation with rectangular boxes and a single reference image, we get a detection noise for atom numbers of \(\varDelta_{\rm det}=7.1\) atoms. Reducing the counting area yields a reduction to \(\varDelta_{\rm det}=5.2\) atoms. The detection noise is reduced further using the optimal reference image, giving a value of \(\varDelta_{\rm det}=3.7\) atoms, as can be seen in Fig. 3. The main noise contribution is photon shot noise, accounting for \(\sqrt{\varDelta_{\rm PSN}^{2}} = 3.6\) atoms, whereas the remaining noise, e.g., from moving interference fringes, leads to an additional uncertainty of 1 atom. Compared to our previous experiments [5], the resolution of our imaging setup is improved by a factor of two.

5 Limits due to photon recoil

The light intensity in the imaging beam is far above the saturation intensity. Therefore, all atoms scatter photons close to the effective saturation scattering rate \(\varGamma_{\rm e}.\) The total number of scattered photons and thus the signal-to-noise ratio is mainly constrained by the radiation pressure resulting from the anisotropic absorption of photons from the imaging beam and the recoil heating due to their subsequent isotropic reemission. Both processes lead to an effective blurring of the image on the CCD chip and thus impose a limit on the largest reasonable illumination time (Fig. 4).

Fig. 4
figure 4

Blurring effects due to photon scattering. The radiation pressure of the imaging light pushes the atoms out of focus of the imaging system, blurring the image on the CCD chip (green). The isotropic recoil of photon emission heats the cloud and leads to transversal expansion (blue clouds). The lower graph shows numerical results of the two blurring effects for a point-like cloud versus illumination time. The blue curve shows the effect of diffusion due to recoil heating as given by Eq. 9. The rms width of the defocused point spread function is shown in green. For our imaging setup, the blurring due to defocusing is the dominant contribution. The minimal integrated image size of the cloud for an illumination time of 15 μs is obtained if the atoms are slightly defocused initially (z i = −1.7 μm) and subsequently get pushed through the focus during the imaging pulse

Full size image

Radiation pressure from the light pushes the atoms out of focus during the imaging process (defocusing). After an imaging pulse duration of τ, the atoms have moved by \(\varDelta z = \frac{v_{\rm rec}\varGamma_{\rm e}}{2}\cdot \tau^2\) in the direction of the objective, with v rec being the recoil velocity. Since the cloud is accelerated during the light pulse, the best imaging settings are obtained if the atoms are slightly out of focus at the beginning and travel through the focal plane. In our case, typically 200 photons are scattered in 15 μs. Due to the recoil, the atoms move about 9 μm during the pulse, compared to a depth of field of 6.8 μm. Note that the reduction of the scattering rate caused by the Doppler shift is still negligible because of the strong saturation.

The second effect is transversal diffusion of the atomic cloud due to recoil heating [1]. The rms cloud width w rms due to the heating is given by [9]

$$ w_{\rm rms} = \frac{2\cdot v_{\rm rec}}{3} \sqrt{\varGamma_{\rm e}}\tau^{\frac{3}{2}}. $$
(9)

The relative strength of the two blurring effects depends on the scattering rate and the optical setup. Experimentally, we choose the integration time such that there is no significant overlap of the signal from the individual clouds. Numerical simulations show that the rms width of the defocused point spread function is smaller than the distance between adjacent clouds for up to 15 μs, as depicted in Fig. 4.

6 Outlook

In this letter, we demonstrated absorption imaging with a resolution of \(\varDelta_{\rm det}=3.7 \hbox{ atoms}.\) This resolution is important, for example, in realizing interferometric sensitivity beyond the standard quantum limit. Such quantum enhanced metrology relies on entangled states exhibiting narrow features in their projected distribution functions that must be resolved by the detection. A perfect detector would be able to count the atom number exactly. Our demonstrated resolution with absorption imaging is sufficient for directly observing interferometric precision 13.5 dB below the standard quantum limit. Further improvements in absorption imaging may be possible, for example, by applying Bayesian estimation in the image analysis [8] or by mitigating the effects of radiation pressure during the exposure time. Other imaging techniques such as phase contrast imaging, for which the radiation pressure effects are reduced, or fluorescence imaging, which has demonstrated single atom resolution for atomic samples in deep traps [10, 11], could offer alternative routes.