1 Introduction

Quasi-phase-matching (QPM) provides not only high efficiency, but also a large degree of freedom in designing nonlinear optical frequency conversion devices [1]. In a conventional QPM device, the grating vector for nonlinearity modulation is perpendicular to the entrance surface and parallel to a principal axis of the crystal, aiming at non-critical QPM. However, angular tuning of a QPM device provides more flexibility than the conventional use. For example, Brand et al. [2] studied angular QPM second-harmonic generation (SHG) and difference frequency generation in periodically-poled MgO-doped LiNbO3, taking advantage of the large aperture of 5 × 5 mm2. They polished it into a sphere, and investigated the frequency conversion processes at full angles of wave vectors and the corresponding orthogonal polarizations of the interacting waves, obtaining refined Sellmeier’s formulas for the crystal. We also demonstrated a broad tuning range of SHG by angular tuning of a direct-bonded thick periodically-poled congruent LiNbO3 (PPLN) crystal [3].

A question arises regarding the direction of the generated second-harmonic (SH) wave vector, when the fundamental wave propagates in the QPM device making an angle α with the grating vector. In this case, one can think of two models:

  1. a.

    The generated SH wave propagates collinearly with the fundamental, with the QPM grating period enlarged from Λ to Λ/cos α (the modulus of the grating vector reduced to \(\vert\bf{K}^{\prime}_{\text{g}}\vert = \vert \bf{K}_{\text{g}}\vert cos\;\alpha\)) as described in Fig. 1a.

  2. b.

    The generated SH wave walks off the fundamental, with the two-dimensional QPM condition satisfied by a vector sum as described in Fig. 1b.

Fig. 1
figure 1

Wave vector diagrams for two possible QPM models. Fundamental (k 1) and second-harmonic (k 2) wave vectors in XY-plane, and QPM grating vector K g parallel to X-axis. a Model-1: SH wave propagates collinearly with fundamental, both at angle α with respect to X-axis. b Model-2: SH wave propagates at angle β (≠α), satisfying vector sum k 2 = 2k 1 + K g

Full size image

Brand et al. [4] interpreted their experimental results based on Model (a), while Model (b) was used by the other authors [1, 5]. When the grating vector is much smaller than the fundamental wave vector, or when α << 1, the difference between the results predicted by the two models would be too small to be resolved by experiments. For QPM devices with short periods and large slant angles, however, the difference would be observable, and the two models can predict different results in terms of the propagation direction of the SH wave and the corresponding QPM condition.

In Model (b), the wave vector matching or the photon momentum conservation (k 2 = 2k 1 + K g) is not obvious in the wave picture. Furthermore, one cannot predict how the SH wave vector propagates under near or off-QPM conditions by using the simple momentum conservation diagram. In this work, we solved the electromagnetic boundary value problem for the SH wave at the interface of two dielectric media, one of which is a nonlinear medium with a one-dimensional nonlinearity modulation along general direction. Then, we interpreted the results in terms of diffraction from the nonlinear grating. We performed SHG experiments in a PPLN crystal with a slanted QPM grating using different fundamental beam sizes, in order to test our interpretation for the SH propagation.

2 Theory

Bloembergen and Pershan derived the laws of reflection and refraction for harmonic waves at the boundary of nonlinear dielectric media [6]. We follow the similar steps in order to calculate the SH wave vector in a nonlinear medium with a periodic modulation of quadratic electric susceptibility χ (2). The geometry for our calculation is described in Fig. 2. The periodic modulation of χ (2) can be represented by a QPM grating vector K g, which is parallel to the crystallographic X-axis of the nonlinear medium. The slab medium has a cut angle Φ, which is the angle between the grating vector and the surface normal of the entrance/exit boundaries. The coordinate axes (x,y) for our calculation is rotated by the angle Φ around the Z-axis from the crystallographic axes (X,Y). Then, Φ is the angle between the crystallographic X-axis and the surface normal. We assume that a plane wave with a wave vector k 1,in and an angular frequency ω (wavelength λ) enters the planar boundary of the nonlinear dielectric medium from vacuum, making an angle of incidence θ. We consider only the case where both of the fundamental and the SH wave are assumed to be polarized in the Z-direction, corresponding to the nonlinear interaction using d 33.

Fig. 2
figure 2

Configuration of crystal axes X, Y, and wave vectors of fundamental and SH waves. Vertical lines indicate QPM grating structure. Short dashed line describes continuity of the tangential components of the fundamental wave vectors. Long dashed line indicates continuity of the tangential components of the SH wave vectors including grating vector under off-QPM condition

Full size image

First, for the propagation of the fundamental wave, one can easily obtain the laws of reflection and refraction at the boundary: the tangential components of the wave vectors of the incident, reflected and the transmitted light are continuous at the boundary (x = 0), as indicated by the short dashed line in Fig. 2.

The SH wave in the medium is determined by solving the wave equation for the electric field at angular frequency 2ω, driven by the SH polarization source term proportional to χ (2) E 21 (r, t). After some manipulation, one obtains the inhomogeneous Helmholtz equation for the SH electric field amplitude,

$$ \nabla^{2} E_{2} + k_{2}^{2} E_{2} = - 2k_{0}^{2} \chi^{(2)} E_{1}^{2} {\text{e}}^{{i\bf{k}_{\text{b}} \cdot \bf{r}}} , $$
(1)

where k 0 = ω/c, E 1 is the fundamental electric field amplitude, and k b = 2k 1. Picking up the m-th Fourier component only, we can write \( \chi^{(2)} = d_{m} {\text{e}}^{{im\bf{K}_{g} \cdot \bf{r}}} \), where d m is the modulus. Then Eq. (1) becomes:

$$ \nabla^{2} E_{2} + k_{2}^{2} E_{2} = - 2k_{0}^{2} d_{m} E_{1}^{2} {\text{e}}^{{i{{\mathbf{k}}}_{{\mathbf{c}}} \cdot {{\mathbf{r}}}}} , $$
(2)
$$ \bf{k}_{\text{c}} = 2\bf{k}_{1} + m\bf{K}_{\text{g}} . $$
(3)

Then, the next procedures are similar to those in Ref. [6], with the bound wave vector replaced by k c. If we further assume negligible depletion of the fundamental, E 1 can be considered as a constant, and the solution to Eq. (2) can be expressed as:

$$ E_{{2{\text{R}}}} = B{\text{e}}^{{i\bf{k}_{{ 2 {\text{R}}}} \cdot \bf{r}}} \,\,\,\,{\text{in}}\quad x < \, 0 \, \left( {\text{in vacuum}} \right), $$
(4-1)
$$ E_{{2{\text{T}}}} = ae^{{i\bf{k}_{2} \cdot \bf{r}}} + be^{{i\bf{k}_{\text{c}} \cdot \bf{r}}} \,\,\,\,{\text{in}}\quad x > \, 0 \, \left( {\text{in medium}} \right), $$
(4-2)
$$ {\text{where}}\,b = \frac{{2k_{0}^{2} d_{m} E_{1}^{2} }}{{k_{\text{c}}^{2} - k_{2}^{2} }}, $$
(4-3)

and k 2R is the wave vector for the reflected SH, whose modulus is k 2R = 2k 0. Note that the transmitted SH electric field E 2T in Eq. (4-2) consists of a free wave (the first term with wave vector k 2) and a bound wave (the second term with wave vector k c). The directions of the wave vectors, and the coefficients B and a are determined by the following electromagnetic boundary conditions: E y and H y must be continuous at x = 0, respectively. The results are:

$$ k_{2y} = k_{{2{\text{R}}y}} = k_{{{\text{c}}y}} = 2k_{0} \sin \theta + mK_{{{\text{g}}y}} , $$
(5-1)
$$ k_{2x} = \sqrt {k_{2}^{2} - k_{2y}^{2} } = 2k_{0} [n_{2}^{2} - (\sin \theta + mr)^{2} ]^{1/2} , $$
(5-2)
$$ k_{{2{\text{R}}x}} = - \sqrt {k_{{2{\text{R}}}}^{2} - k_{{2{\text{R}}y}}^{2} } = - 2k_{0} [1 - (\sin \theta + mr)^{2} ]^{1/2} , $$
(5-3)
$$ k_{{{\text{c}}x}} = 2k_{1x} + mK_{{{\text{g}}x}} , $$
(5-4)
$$ {\text{where}}\,\,\,\,r \equiv K_{{{\text{g}}y}} /2k_{0} ,K_{{{\text{g}}x}} = K_{\text{g}} \cos \phi , \, K_{{{\text{g}}y}} = K_{\text{g}} \sin \phi , $$
(5-5)

and n 2 is the index of refraction for the SH wave. Equation (5-1) states that all the tangential components of the SH wave vectors are continuous, including the grating vector [1]. This situation is indicated by the long dashed line in Fig. 2.

The coefficients in Eq. (4) are also determined from the boundary conditions,

$$ a = - \frac{{k_{{2{\text{R}}x}} - k_{{{\text{c}}x}} }}{{k_{{2{\text{R}}x}} - k_{2x} }}b, $$
(6-1)
$$ B = a + b = \frac{{k_{{{\text{c}}x}} - k_{2x} }}{{k_{{2{\text{R}}x}} - k_{2x} }}b. $$
(6-2)

Thus, the reflected and the transmitted SH electric fields in Eq. (4) have been determined. One can show that the transmitted SH electric field E 2T reaches the maximum under the QPM condition k 2 = k c = 2k 1 + m K g which agrees with Model-2 in Fig. 1b, while the reflected SH electric field E 2R does not exhibit any phase-matching behavior [6].

From the results in Eq. (5), when m ≠ 0, we note that the directions of the reflected and transmitted SH waves differ from those obtained for a uniform χ (2)-medium by the factor r given in Eq. (5-5). Then, the laws of reflection and refraction for the SH wave deviate from those of a uniform medium. The angle of reflection θ 2R is determined by Eq. (5-1) with k 2R = 2k 0,

$$ \sin \theta_{{2{\text{R}}}} = k_{{2{\text{R}}y}} /k_{{2{\text{R}}}} = \sin \theta + mr $$
(7)

Here, we note from Eq. (5-5) that

$$ r = \frac{{\lambda_{2} }}{\Lambda /\sin \Phi } = \frac{{\lambda_{2} }}{\Lambda '}, $$
(8)

where λ 2 (=λ/2) is the wavelength of the SH wave in vacuum, and \( \Lambda ' = \Lambda /\sin \Phi \) is the period of the grating at the entrance plane. Thus, the law of reflection in linear optics does not hold for the SH wave, but is replaced by the grating Eq. (7). Instead, the reflected SH wave is radiated by the phase-reversed array of the SH polarizations with a period Λ′ at the surface.

The angle of refraction of the transmitted free wave (θ 2T = β − Φ) can also be determined by the same procedure using Eq. (5-1),

$$ n_{2} \sin \theta_{{2{\text{T}}}} = n_{2} k_{2y} /k_{2} = \sin \theta + mr. $$
(9)

This result can also be interpreted as a diffraction phenomenon inside the medium of index n 2. Upon exiting the exit-boundary of a slab medium, the angle of transmitted SH wave vector θ 2 is obtained by applying Snell’s law to Eq. (9),

$$ \sin \theta_{2} = \sin \theta + mr. $$
(10)

Contrary to the reflected SH, the transmitted SH is not interpreted solely as diffraction from the surface grating with a period Λ′. A specific order m can be greatly enhanced during propagation inside the medium, for the direction satisfying the QPM condition k 2 = k c = 2k 1 + m K g, as can be verified by using Eqs. (4) and (6). For a conventional QPM grating (Φ = 0), the SH wave propagation is governed simply by Snell’s law, because r = 0 by Eq. (8). Thus, the SH wave walks off from the fundamental only inside the medium, and then propagate parallel to the fundamental after exiting the medium. In this case the beam separation would be hard to detect in practice, because the walk-off caused inside the medium is typically small. On the other hand, for a slanted QPM grating (Φ ≠ 0) the SH wave continues to deviate from the fundamental after exiting the medium according to Eq. (10), even at normal incidence. As a result, an apparent beam separation is expected far from the medium.

Although the above derivation is based on the assumption that the incident fundamental is a plane wave which is uniform all over the transverse dimension, one can extend it to the case of finite beam extent in the medium. For a finite beam, it is expected that the transmitted far-field SH beam would be centered at the location dictated by Eq. (10) and the QPM condition, and distributed with a finite width which is inversely proportional to the SH (and fundamental) beam size in the medium.

3 Experiments and results

The above theoretical result is supported by our recent experiment on beam separation by using a Q-switched laser [3]. The result verifies our prediction by Eq. (10). In this case, the fundamental laser beam diameter at the sample was about 200 μm, which covered several periods at the entrance boundary (Λ′). In the present work, we tried to reduce the beam diameter smaller than Λ′/2, in order to investigate whether the SH beam is angularly separated from the fundamental beam even when a fraction of a domain is illuminated.

We used a continuous-wave (cw) laser beam to obtain much smaller beam waists than those of the Q-switched laser. A DFB laser amplified by an Er-doped fiber amplifier (1,550 nm, 170 mW cw) was focused to a PPLN sample, made by poling a 0.5-mm thick congruent LiNbO3 crystal with a QPM period of 18.5 μm. The PPLN was cut and the end faces were polished as depicted in Fig. 2 with a slant angle of Φ = 19.3° in the XY-plane, making Λ′ = 56.0 μm on the end faces. The PPLN channel was 5-mm long and 3-mm wide. The laser beam was focused to the PPLN sample making a small angle of incidence for a slanted QPM with α ≈ 14° [3]. Pictures were taken of the transmitted fundamental and the SH beams illuminating on a screen placed after the sample by using an infrared vidicon and a Si-CCD camera, respectively. This procedure was repeated for different beam diameters by replacing lenses with different focal lengths. The focused beam diameters were measured by the knife-edge scanning method.

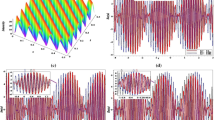
For a beam with a Gaussian radius (w 0) of 17.0 μm or larger, a distinct SH beam separation was observed with respect to the fundamental beam as shown in Fig. 3a. The measured angular separation was 1.0° ± 0.2°, which roughly agrees with the prediction by Eq. (10), 0.793° [7]. Because the beam diameter of 34.0 μm (Fig. 3a) was somewhat larger than the domain width or the half-period at the boundary (Λ′/2 = 28.0 μm), we further reduced the beam radius down to 13.3 μm in order to confine the beam in a single domain on the entrance surface of the PPLN. The result is shown in Fig. 3b. A smaller beam at focus diverges faster, causing a larger uncertainty in the measurement of the angular separation. In spite of the larger uncertainty, we could estimate a similar angular separation of ~1° by fitting the beam profiles on the screen to Gaussian functions.

Fig. 3
figure 3

Images of fundamental (bottom) and the SH (top) beams observed on a screen placed after PPLN. a w 0 = 17.0 μm, b w 0 = 13.3 μm, both on a screen located 185 mm from PPLN

Full size image

The angular separation for a smaller beam than a domain width cannot be explained in terms of diffraction from the nonlinear surface grating. However, during the fundamental beam propagation through the medium with a slanted QPM grating, whenever the beam crosses the domain boundary, the transverse cross-section of the beam spans two neighboring domains with opposite signs of χ(2). In this case, the two different parts of the cross-section generate SH waves oscillating with a phase difference of π with respect to each other. Thus, interference takes place, and a portion of the beam is further enhanced along the direction dictated by the noncollinear QPM condition (Fig. 1b or Eq. (10)).

Another important parameter in the observation of the angular separation is the ratio between the wavelength and the QPM grating period. If it is too small, diffraction effect may not be clear. In our experiment it was ~12, and the separation was obvious as shown in Fig. 3. However, if the grating period is larger, and the focusing condition is different (as in the Refs. [2, 8]), the beam separation may not be clearly observed.

4 Summary

We theoretically and experimentally investigated the propagation of the SH wave in a QPM nonlinear medium with a slanted grating. The SH beam walk-off was interpreted as diffraction from the nonlinearity modulation within the transverse beam extent. The noncollinear QPM wave vector diagram results from the electromagnetic boundary conditions on the harmonic field. By solving the electromagnetic boundary value problems for the SH wave, we derived the laws of reflection and refraction for the SH wave which are different from the linear optical counterparts. Such phenomena were interpreted in terms of diffraction, supported by SHG experiments on a PPLN crystal with a slanted QPM grating.