Single mode operation of elliptical gain guided and index antiguided fibers

Abstract

Elliptical gain guiding fibers in which gain guiding effects are dominant compared to conventional index guiding fiber is analyzed by solving Mathieu equations with complex-valued fiber parameters. The properties of mode propagation and single mode operation are evaluated in this elliptical gain guiding fiber with the assumption of a uniform gain distribution in the active core medium. Threshold for lossless mode propagation increases exponentially with the eccentricity of the elliptical cross section. Further, the difference in threshold between the lowest two order modes is constant for arbitrary eccentricity.

1 Introduction

The development of single mode, high power fiber lasers has been limited by nonlinear effects in the core glass where the optical intensity may become very large. Attempts to successfully increase the lowest order mode output power of fiber lasers are discussed in [1] and include a number of techniques to obtain large mode area (LMA) fibers to minimize the intensity in the core. Hence, nonlinear effects and optical damage limitations to the operation of fiber lasers would be diminished. One type of LMA fibers, gain guided and index antiguided (GG IAG) fibers was first considered by Siegman [2]. Subsequently, experimental demonstration of single mode lasing using GG IAG fiber showed extremely large mode areas corresponding to fibers with core diameter up to 400 μm [3]. Theoretical discussions of GG IAG fibers were published [4–7] and experimental reports, including improved laser diode pumped GG IAG fiber lasers are described in [8–10].

A recent experimental report on GG IAG fiber lasers utilizes a scalable side pumping scheme, to try to obtain high power, lowest order mode output [11]. The slope efficiency in [11] was not high and the results, though suggestive, indicated the need for more efficient utilization of the pump light. Gain guided, index antiguided fibers with elliptical cross section shaped cores are discussed in this paper because pumping along the major axis of the elliptical core could increase the efficiency by providing an increased absorption length for pumping.

The optical properties of weakly guided elliptical fibers have been analyzed using the Mathieu equation and function [12–17]. In this paper, elliptical fibers in which gain guiding plays a significant role are discussed using the same approach. The results provide an extended discussion of the gain guiding effect in dielectric waveguides, where the gain coefficient in the core region is considered as the imaginary part of a complex refractive index.

2 Theory

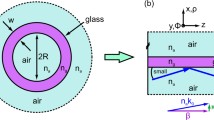
An elliptical fiber consists of an elliptical cross section core with semimajor axis a, semiminor axis b, semifocal length c, and the eccentricity as defined $e = \frac{c}{a}$. The elliptical coordinate system is defined by the relations with Cartesian coordinates in (1) and as shown in Fig. 1 [14]:

$$ \left\{\begin{array}{l}x = c \operatorname{cosh}( \xi)\cos( \eta) \\[2pt]y = c\operatorname{sinh}(\xi)\sin(\eta) \\\end{array} \right.$$

(1)

where ξ is quasiradial direction and varies from 0 to infinity and η is quasiangular direction ranging from 0 to 2π. These are similar to ρ and θ in a cylindrical coordinate system. ξ=ξ ₀ is the boundary between core and cladding so that e=1/cosh (ξ ₀).

Fig. 1

Elliptical coordinate system

In the approximation of a weakly guided fiber and the conditions of harmonic time dependence and positive z-axis propagation, the transverse electric field wave equation

$$ \bigl( \nabla^{2} + k^{2}n^{2} - \beta^{2} \bigr)E = 0$$

(2)

can be rewritten in the elliptical coordinate system as

$$ \biggl(\frac{\partial ^{2} }{\partial \xi ^{2} }+\frac{\partial ^{2} }{\partial \eta ^{2} } \biggr)E+p^{2} c^{2}\bigl(\cosh ^{2} \xi -\cos ^{2} \eta \bigr)E=0$$

(3)

where p ²=k ² n ²−β ², the value of p ² is positive in the core region and negative in the cladding region in conventional index guided fibers. The solutions of this wave equation were characterized as Mathieu functions [14–16].

In the following discussion, an active medium core is evaluated using a complex refractive index as defined in [2], so that p ² is complex. Therefore, the parameters are all complex-valued including those in the Mathieu functions. However, previous reports used different notations when reporting solutions to specific problems. As a result, standard expressions and definitions could not be found for use in Matlab [17] when the parameter p ² is complex. Consequently, we rederived the mathematical form in the case of complex Mathieu equations as given in the Appendix.

Applying the separation of variable method to (3), the transverse electric field is written as E=R(ξ)Θ(η) so that the following ordinary differential equations in the core and cladding regions of the fiber are developed (Fig. 1):

In the core where ξ<ξ ₀ (standard Mathieu equation)

(4)

(5)

In the cladding where ξ>ξ ₀ (modified Mathieu equation)

(6)

(7)

where α is the characteristic parameter (eigenvalue) resulting from the separation of variables and is obtained from (A.2). The gamma fiber parameters

(8)

(9)

are similar to the definitions of transverse parameters U ² and W ² in Bessel function solutions for circular fibers. The sum of $\gamma_{U}^{2}$ and $\gamma_{W}^{2}$ is analogous with the square of the fiber parameter V ²

$$ \gamma_{V}^{2} = \gamma_{U}^{2} + \gamma_{W}^{2} = k^{2}c^{2} \bigl(n_{1}^{2}- n_{2}^{2}\bigr)/4$$

(10)

$\gamma_{U}^{2}$, $\gamma_{W}^{2}$, and $\gamma_{V}^{2}$ will be used in the following mathematical evaluation, but they might not be suitable to present fiber properties. So, the ordinary transverse fiber parameters U ², W ², and V ² are redefined below for the elliptical fiber of interest with major axis a including their relations with $\gamma_{U}^{2}$, $\gamma_{W}^{2}$, and $\gamma_{V}^{2}$.

(11)

(12)

(13)

Theoretical and experimental evaluations of conventional elliptical fibers have been studied previously [12–14]. In this paper, our physical model is a specific elliptical fiber with an active core and negative index step called a GG IAG fiber. To be an active core fiber, the power gain coefficient g in the core must compensate for losses at the core–cladding interface. It is expressed as the imaginary part of the now complex-valued refractive index, i.e., $n = n_{0} +i\frac{g}{2k_{0}}$. Then the normalized square of the fiber parameter V ² (13), can be rewritten in the complex-valued form [1]:

$$ V^{2} = N + jG = k_{0}^{2}a^{2} \bigl(n_{1}^{2} - n_{2}^{2}\bigr)$$

(14)

where the real part N and imaginary part G

(15)

(16)

are proportional to the index step Δn and gain coefficient g, respectively.

Two adjectives “standard” or “modified” are used before the Mathieu equation or function to differentiate the problem in the core region or cladding region. This distinction parallels that used for Bessel function solutions for circular fibers.¹ In addition, the notation “angular” or “radial” is used to differentiate the angular or radial function. Therefore, the solutions of the standard Mathieu equations (4), (5) and the modified Mathieu equations (6), (7) are denoted as Se _KF;m (η) and J _KF;m,n (ξ) and Sd _KF;m (η) and K _KF;m,n (ξ), respectively. Here, the subscripts KF, m, n refer to three orthogonal mode types and the notation for the functions J and K are not Bessel functions, they are Mathieu functions of quasiradial position. Owing to the disruption of circular symmetry the degenerate mode distribution is disrupted. Mathematically, the angular Mathieu functions are represented by an expansion in trigonometric functions. So, the solution of the angular Mathieu equations, the angular Mathieu functions, Se _KF;m (η) and Sd _KF;m (η), are categorized into four groups by the subscript KF, KF could be (ee, eo, oe, oo) to stand for different basis trigonometric functions. Here, the first letter e or o means the basis function is a cosine function (even function) or a sine function (odd function) and the second letter e or o means the factor before argument η is even 2j or odd 2j+1. For example, KF=eo is for the basis function of cos[(2j+1)η]. The details for solving Mathieu equations and the subscripts KF,m,n are given in the Appendix. Note the mode order number m (m=1, 2, 3, …) is an integer and its relation with the ordinary physical angular mode order number (t), showing the number of maxima between 0 and π, is

$$ t = \left\{ \begin{array}{l@{\quad }l}2m - 2, & \mathrm{KF} = \mathit{ee} \\2 m - 1,& \mathrm{KF} = \mathit{eo}\\2 m, & \mathrm{KF} = \mathit{oe} \\2m -1, & \mathrm{KF} = \mathit{oo}\end{array} \right.$$

(17)

The linearly polarized mode solutions of the radial Mathieu equation are

$$ R_{\mathrm{KF},m,n}( \xi ) = \left\{\begin{array}{l@{\quad }l}A\frac{J_{\mathrm{KF},m,n}(\xi ,\gamma _{U}^{2})}{J_{\mathrm{KF},m,n}( \xi_{0},\gamma _{U}^{2} )} & \mbox{core} \\[8pt]A\frac{K_{\mathrm{KF},m,n}(\xi,\gamma_{W}^{2})}{K_{\mathrm{KF},m,n}( \xi _{0},\gamma_{W}^{2} )}& \mbox{clad} \\\end{array} \right.$$

(18)

where A is a constant governed by optical power. According to the relationship between the axial field component E _z and the transverse field E _y in weakly guided fibers:

$$ E_{z} = \frac{j}{\beta }\frac{\partial E_{y}}{\partial y}$$

(19)

The conditions for continuity of E _y and E _z at the core–cladding interface result the dispersion equation

$$ \frac{J'_{\mathrm{KF},m,n}(\xi _{0} ,\gamma _{U}^{2} )}{J_{\mathrm{KF},m,n}(\xi _{0} ,\gamma _{U}^{2} )} =\frac{K'_{\mathrm{KF},m,n}(\xi _{0} ,\gamma _{W}^{2} )}{K_{\mathrm{KF},m,n} (\xi _{0} ,\gamma _{W}^{2} )}$$

(20)

For a specific elliptical fiber with given physical parameters including refractive index n ₁, n ₂ in the core and cladding, working wavelength λ ₀ and core–cladding boundary ξ ₀, the dispersion equation is a transcendental equation of mode dependent (KF, m, n) phase constant β. After obtaining the mode dependent phase constant β _KF,m,n by numerical methods applied to the dispersion equation, the field distribution E _KF,m,n , and related fiber parameters are evaluated easily using (8)–(13) and (18). The patterns in Fig. 2 show the computed lowest order four categories of elliptical fiber modes.

Fig. 2

Contours of mode profiles in an elliptical GG IAG fiber when e=30/50, m=1, and n=1. The dashed line is the core-cladding boundary

3 Results and discussions

To be analogous to a circular GG IAG fiber, an elliptical GG IAG fiber has to satisfy a similar condition for lossless propagation. That is, the real part of γ _w should be positive. Therefore, substitute the mathematical condition Re (γ _w )=0 into the dispersion equation, (20), to obtain the values of $\gamma_{U}^{2}$ and $\gamma_{V}^{2}$ for a given sequence of purely imaginary numbers γ _w while the solutions of U ² and V ² are obtained from (11) and (13). For the case of e=0.2, a fiber that is close to circular, the functions of complex U ² and V ² for lossless propagation are shown in Figs. 3(a) and 3(b), respectively. In Fig. 3, the horizontal axis and vertical axis are the real part and imaginary parts of the complex parameters.

Fig. 3

Complex value plane of U ² (a) and V ² (b) for LP modes in an elliptical fiber for categories of modes KF=ee (dotted), eo (solid), oe (dot–dash), oo (dashed), and m=1, n=1. Eccentricity e=10/50 (half focal length c=10 μm, half major axis a=50 μm)

In Fig. 3(a), curves indicate the corresponding complex U ² of the four lowest order modes under the condition that Re (γ _w )=0, i.e., $\gamma_{W}^{2} \le 0$. As the absolute value of $\gamma_{W}^{2}$ increases, U ² follows the semicircle-like solid line from left to right. Meanwhile, the left crossing point on the horizontal axis is the mode cutoff condition for ordinary index guided fiber without gain $(\gamma_{W}^{2} = 0)$. When $\gamma_{W}^{2} \to -\infty$ the curves tends to the right crossing point on the horizontal axis which is the mode limitation where all energy of the mode is confined in the core region as a result of the restriction that there is no E-field in the cladding. When $\gamma_{W}^{2} \to + \infty$ for ordinary index guiding fiber, the value of real U ² tends to the same crossing point as when $\gamma_{W}^{2} \to - \infty$, indicating the mode limitation point is reached where all energy of the mode is in the core region. The allowed region in the complex plane of U ² for delivering lossless modes in an elliptical gain guiding fiber is a closed region surrounded by semicircle-like curves and a part of the horizontal axis.

In Fig. 3(b), curves in the complex plane of V ² indicate the corresponding complex-valued V ² of the four lowest order modes under the condition that Re (γ _w )=0 i.e. $\gamma_{W}^{2} \le 0$. As the absolute value of $\gamma_{W}^{2}$ increases, V ² follows the solid line from right to left. The right crossing point on the horizontal axis is the mode cutoff for an ordinary index guided fiber ($\gamma_{W}^{2} = 0$). When $\gamma_{W}^{2} \to - \infty$, so that V ² also tends to −∞ indicating a mode limitation case is reached where all the energy of the mode is confined in the core region. The allowed region in the complex plane of V ² for a specific mode is outside the mode dependent solid curve. So, from the complex-valued definition of V ², (14)–(16), the curves in Fig. 3(b) are also functions of threshold gain coefficient $g_{\mathrm{KF},m,n}^{\mathrm{th}}$ versus index step for given modes to experience lossless propagation.

In the case of mode limitation, e.g., when $\gamma_{W}^{2} \to \pm \infty$, the optical field at the core–cladding boundary $J_{\mathrm{KF},m}(\xi_{0},\gamma_{U_{0}}^{2} ) = 0$. The corresponding value of the fiber gamma parameter in the core region is denoted by $\gamma_{U_{0}}^{2}$ with a subscript ‘0’, and also the fiber parameter $U_{0}^{2}$ is obtained from (11). Figure 4 shows the properties of mode limitation for elliptical fibers with different eccentricities. In the case of ordinary mode cutoff, γ _w =0, the corresponding value of fiber gamma parameter in the core region is denoted by $\gamma_{U_{c}}^{2}$ with a subscript “c”, and also the fiber parameter $U_{c}^{2}$ is obtained from (11). $U_{c}^{2}$ is shown in Fig. 5 plotted versus core eccentricity. No matter the condition of mode limitation $J_{\mathrm{KF},m}(\xi_{0},\gamma_{U_{0}}^{2} ) = 0$ or mode cutoff γ _w =0, the specific values of fiber parameter U ² are both real and only depend on the eccentricity of elliptical core. However, they are independent of the size of the elliptical core. This property is in accord with the circular fiber (e.g., e=0) where the value of mode cutoff $U_{c}^{2}$ is independent of fiber size for a given mode.

Fig. 4

$U_{0}^{2}$ when all mode energy is confined in the core region for categories of modes KF=ee (dotted), eo (solid), oe (dot–dash), oo (dashed) and m=1, n=1

Fig. 5

$U_{c}^{2}$ at the cutoff for categories of modes KF=ee (dotted), eo (solid), oe (dot–dash), oo (dashed), and m=1, n=1

According to the plots of $U_{0}^{2}$ or $U_{c}^{2}$ versus eccentricity in Figs. 4 and 5, respectively, as the eccentricity increases the values of $U_{0}^{2}$ and $U_{c}^{2}$ both increase exponentially, so that there is a higher threshold for lossless propagation in an elliptical core index antiguided fiber with high eccentricity than in one with lower eccentricity.

For a specific elliptical GG IAG fiber with physical parameters that are the same as in [2] and [8]: refractive index of the core n ₁=1.5689, refractive index of the cladding n ₂=1.5734, wavelength λ ₀=1.06 μm, half major axis a=50 μm result in a large negative value of V ²=−1242. The thresholds for delivering the two lowest order lossless modes for this fiber are shown in Fig. 6 (KF=ee,eo; m=1, n=1). As the eccentricity increases, the threshold increases dramatically. However, the difference between the thresholds for lossless propagation of the two lowest order modes is roughly independent of the arbitrary eccentricity of the elliptical core IAG fiber.

Fig. 6

Gain coefficient threshold (in units of m⁻¹) for lossless propagation of the two lowest order modes (solid line for the lowest mode KF=ee, dashed line for the second order mode KF=eo) and their difference (dotted line) versus eccentricity of the core’s elliptical cross section

Active fiber devices using elliptical GG IAG fibers such as fiber lasers or amplifiers could be in the state of single mode operation when the gain coefficient in the fiber is sufficient to compensate the lowest order mode losses and keep the second order mode net loss still between the two solid curves shown in Fig. 6. The threshold for lossless propagation is increased by increasing the eccentricity of elliptical fibers because the fiber modes are no longer degenerate.

4 Conclusions

Gain guided index antiguided fibers can serve as single mode fiber amplifiers and fiber lasers. Such fibers can deliver high optical power with minimal nonlinear effects because they have large mode area together with single mode operation. For a circular GG IAG fiber, the threshold for lossless mode propagation is inversely proportional to the cube of the core radius and the square root of the absolute value of the negative index step. When a GG IAG fiber has an elliptical cross section the case of single mode operation was studied as a function of increasing core eccentricity. The calculations show that the threshold for lossless mode propagation increases exponentially with increasing core cross section eccentricity. Concurrently, the difference between the thresholds of the two lowest order modes is constant for GG IAG fibers having cores of arbitrary ellipticity. As a consequence of these two results, elliptical gain guided index antiguided fibers are not an attractive alternative to circular cross section fibers.

Appendix: Mathematics on Mathieu function and Mathieu equation

When the parameters $\gamma_{U}^{2}$ and $\gamma_{W}^{2}$ in the Mathieu equation (4)–(7) is real, i.e., real index guided fiber, solutions of the two coupled Mathieu equations were obtained in [15]. If these two parameter $\gamma_{U}^{2}$ and $\gamma_{W}^{2}$ are complex, those mathematical expressions were not suitable. We suggest the following redefinition for solving the Mathieu equations (4)–(7) with complex parameters.

In the core region, the mode distribution is a product of two orthogonal functions of quasiangular direction η and quasiradial direction ξ, which are governed by the angular Mathieu equations (4) and (5) and the radial Mathieu equations (6) and (7). Owing to the asymmetric cross section, the angular Mathieu function is divided into four categories which are expanded as the series of trigonometric functions.

$$ \mathit{Se}(\eta ) =\left\{ \begin{array}{l@{\quad }l}\displaystyle\sum_{j= 0}^{\infty}A_{\mathit{ee}}^{j + 1}\cos( 2j\eta),& \mathrm{KF} = \mathit{ee} \\[5pt]\displaystyle\sum_{j =0}^{\infty} A_{\mathit{eo}}^{j + 1}\cos \bigl[(2j + 1)\eta\bigr],&\mathrm{KF} = \mathit{eo}\\[5pt]\displaystyle \sum_{j = 0}^{\infty}A_{\mathit{oe}}^{j + 1}\sin\bigl[ (2j +2)\eta \bigr],& \mathrm{KF} = \mathit{oe} \\[5pt]\displaystyle \sum_{j = 0}^{\infty} A_{\mathit{oo}}^{j +1}\sin\bigl[ ( 2j + 1 )\eta \bigr], & \mathrm{KF} = \mathit{oo}\end{array} \right.$$

(A.1)

Substituting (A.1) into the (4) and using the orthogonal property of trigonometric functions, the expansion coefficients A _KF are obtained from the KF dependent matrix equation:

$$ [ M_{\mathrm{KF}} ]\vec{A}_{\mathrm{KF}} = \alpha\vec{A}_{\mathrm{KF}}$$

(A.2)

Here, the matrix M _KF is a function matrix of $\gamma_{U}^{2}$ as shown below and α is the same parameter as in (4)–(7). As a consequence its m-order eigenvalue α _m and eigenvector $\vec{A}_{\mathrm{KF},m}$ is used to obtain the solution of the standard Mathieu equations (4) and (5). The matrix M _KF(x) is:

KF=ee mode

$$M( x ) = \left[ \begin{array}{c@{\quad }c@{\quad }c@{\quad }c}0^{2} & x & 0 & \ldots \\2x & 2^{2} & x & \ldots \\0 & x & 4^{2}& \ldots\\\vdots& \vdots& \vdots & \ddots \end{array} \right]$$

KF=eo mode

$$M( x ) = \left[ \begin{array}{c@{\quad }c@{\quad }c@{\quad }c}1 + x & x & 0 & \ldots\\x & 3^{2} & x & \ldots \\0 & x & 5^{2} & \ldots\\\vdots & \vdots & \vdots & \ddots \end{array} \right]$$

KF=oe mode

$$M( x ) = \left[ \begin{array}{c@{\quad }c@{\quad }c@{\quad }c}2^{2} & x & 0 & \ldots \\x & 4^{2} & x & \ldots \\0 & x & 6^{2} & \ldots\\\vdots & \vdots & \vdots & \ddots\\\end{array} \right]$$

KF=oo mode

$$M( x ) = \left[ \begin{array}{c@{\quad }c@{\quad }c@{\quad }c}1 - x & x & 0 & \ldots \\x & 3^{2} & x & \ldots\\0 & x & 5^{2} & \ldots\\\vdots & \vdots & \vdots & \ddots \end{array} \right]$$

To achieve effective convergence of the proposed solution, the radial Mathieu solutions can be expanded in a series of products of Bessel functions given below [15]:

(A.3a)

(A.3b)

(A.3c)

(A.3d)

where υ ₁=γ _U ⋅exp(−ξ), υ ₂=γ _U exp(ξ). The derivatives of these Bessel functions are

(A.4a)

(A.4b)

(A.4c)

(A.4d)

The solution of the angular Mathieu equation (6) in the cladding region Sd _KF;m (η) is obtained by replacing $\gamma_{U}^{2}$ by $-\gamma_{W}^{2}$ in (A.1).

However, this easy replacement is not suitable on the modified radial Mathieu function (7). Because of the difference between derivatives of the Bessel J functions and Bessel K functions [17], the sign in the rectangular bracket of (A.5b) and (A.5d) are different from the sign in J _eo,m and J _oo,m . This difference is found when comparing the derivative of each Bessel J and K functions.

(A.5a)

(A.5b)

(A.5c)

(A.5d)

where υ ₁=γ _W exp(−ξ), υ ₂=γ _W exp(ξ). The derivatives are

(A.6a)

(A.6b)

(A.6c)

(A.6d)

where the B _KF,m are obtained from the KF dependent matrix equation

$$ [ M'_{\mathrm{KF}} ]\vec{B}_{\mathrm{KF}} =\alpha \vec{B}_{\mathrm{KF}}$$

(A.7)

and where $M'(\gamma_{W}^{2} )= M( - \gamma _{W}^{2})$.