STATE AND ACTUALITY OF THE PROBLEM

The electric conduction current i0(t) of different types, including direct, alternating, and pulse currents, possessing different amplitude and time parameters (ATPs) in metal conductors of various designs is known to have the quantum-wave nature [1–3]. In this regard, the behavior in the space and time t of the collectivized free electrons drifting in their crystal structures under the action of the electrical voltage u0(t) applied to the opposite edges of these conductors obeys to some quantized (discrete) Schroedinger wave ψn-functions characterized by the integral eigenvalues n = 1,2,3…, which are called quantum values in quantum physics (wave mechanics) [4]. The finite maximal value of the quantum number n in the general case appears to be equal to nm. In the case of practical calculations of the drift of free electrons in the conductors under study with the electric conduction current i0(t) with different ATPs, the specified value of the quantum number is to be defined separately. The longitudinal quantized wave Schroedinger ψnz(z, t) functions with regard to the cylindrical conductor with a longitudinal axis OZ and axial current i0(t) possessing arbitrary ATPs have harmonic character and lead to the origin in its micro- and macrostructures of quantized standing longitudinal electron de Broglie waves λezn in length moving in this conductor along the coordinates z of the mentioned electrons [2]. It was previously established that along the metal conductor l0 in length with the current i0(t) there is always placed only an integral number n of quantized standing de Broglie half-waves λezn/2 in length satisfying the relation nλezn/2 = l0 [1]. The minimum value of the quantity λezn/2 is just determined by the maximum value of the quantum number n, i.e., nm. In addition, this quantum number nm also determines the maximal number of longitudinal wave electron packages (WEPs) recurrent along the length l0 of the conductor with the electric current i0(t), and each of them contains one relatively “hot” longitudinal section Δznh in length and one relatively “cold” longitudinal section Δznc in length [2], [5]. For each WEP conductor with the current i0(t) of different types and ATPs, the following equality always holds [2]:

$${{{{\lambda }_{{ezn}}}} \mathord{\left/ {\vphantom {{{{\lambda }_{{ezn}}}} 2}} \right. \kern-0em} 2} = \left( {\Delta {{z}_{{nh}}} + \Delta {{z}_{{nc}}}} \right).$$

The elements of the theory of the longitudinal periodic localization of drifting free electrons in the cylindrical conductor with an electrical axial current of different types and ATPs were set forth in [1–3]. One characteristic feature of this localization of electrons drifting along the electron conductor is the fact that the values of their averaged volume density neh on the hot longitudinal sections in the extreme case at n → nm will significantly exceed (maximum by 3.5 times) the values of the averaged volume density nec of the drifting electrons on the cold longitudinal sections of the same conductor [1–3].

The inequality of the kind neh/nec > 1 will lead to the fact that the specific power of heat (Joule) losses on the hot longitudinal sections of the conductor will significantly exceed the specific power of these losses on its cold longitudinal sections. If so, the temperature of heating with the current i0(t) of its hot longitudinal sections will be much higher than the temperature of the corresponding heating of the cold longitudinal sections of the conductor. This distinction in the Joule heating temperatures for hot and cold longitudinal sections of conductors are particularly vivid in the case of an emergency operation of the cable and wire products (CWPs) of the electrical power circuits of energy facilities (for example, upon a short circuit (SC) or current overloads in them) [6] and in the normal mode of operation of the current-carrying cylindrical busbar arrangement of the powerful high-voltage generators of pulse currents (GICs) [7] when the amplitude of current density δ0m in the transverse cross sections of conductors will take a value of about 0.1 kA/mm2 and more. In these modes of CWP operation, its hot longitudinal sections will overheat and break down [8]. The heating temperature of the thin strands of CWPs for their hot longitudinal sections (at δ0m ≈ 0.4 kA/mm2 about Δznh ≈ 5.3 mm in length (width) [2]) can exceed the melting temperature Tm of copper Cu (Tm ≈ 1083°С [9]) and cause the ignition of the extreme (protective) isolation of CWPs, which is fraught with fire both on the energy object and in the circuit of the energy consumer.

The experimental data presented in Fig. 1 for the galvanized steel wire r0 = 0.8 mm in radius and l0 = 320 mm in length (at the thickness of its zinc coating of Δ0 = 5 μm) included into the discharge circuit of the powerful high-voltage GIC with an aperiodic pulse of the axial current i0(t) of the temporary form 9 ms/160 ms at δ0m ≈ 0.37 kA/mm2 and n = 1 [7] clearly demonstrate this possibility of the local melting of the current-carrying part of CWPs in the middle of this steel wire on its single hot longitudinal section Δznh ≈ 7 mm in length (width). In practice, during the operation of various CWPs, it is necessary to know the local overheating zones of its current carrying parts in the emergency (for example, at the circuit SC) and normal (for example, in the circuits of powerful GICs) modes of its operation and predict both their number and the place of the possible appearance along the CWPs used in the power circuits.

Fig. 1.
figure 1

Empirical demonstration of local melting of galvanized steel wire (r0 = 0.8 mm; l0 = 320 mm; Δ0 = 5 μm) by discharge pulse of axial current i0(t) of temporal form 9 ms/160 ms from powerful GIC (δ0m ≈ 0.37 kA/mm2) in the zone of its only (n = 1) hot longitudinal section Δznh ≈ 7 mm long, which is cooling in air and on fire-resistant asbestos cloth.

Full size image

Please note that the heating temperature on the only (n = 1) hot longitudinal section of the bare (without insulation) bimetallic wire used in this experiment (see Fig. 1) was not less than the melting temperature of its steel base (~1533°С [10]), and the heating temperature on its two cold longitudinal sections Δznc ≈ 156.5 mm long with the bolted edges did not exceed the melting temperature of its galvanized coating (~419°С [10]). In this regard, in the field of both electricity, power electrical equipment, and high-voltage pulse engineering and electrophysical processing of metals by large pulse currents, it is necessary to be able to determine qualitatevely the maximum value of the quantum number n = nm for the longitudinal quantized de Broglie half-waves λezn/2 = l0/n long and, accordingly, the maximal number nm of the quantized zones of the longitudinal localization Δznh long of the drifting electrons in the cylindrical conductors with the electric current i0(t) of different forms and ATPs.

The aim of this article is to calculate the maximum value of the quantum number n = nm of the longitudinal quantized de Broglie half-waves λezn/2 = l0/n long and accordingly the maximal number nm of the quantized zones of the longitudinal periodic localization Δznh long of the drifting electrons in the metal of the cylindrical conductor with the electric axial current i0(t) of different types and ATPs.

PROBLEM STATEMENT

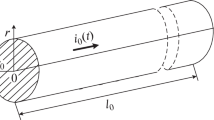
Let us consider a solitary solid straight round cylindrical conductor r0 in radius and l0 ⪢ r0 in length (Fig. 2) in which an axial electric conduction current i0(t) of arbitrary ATPs flows in its longitudinal direction. Let the geometric dimensions of the conductor and the ATPs of the electric current allow its almost uniform distribution over the cross section S0 of the conductor under study. We believe that the longitudinal distribution of the drifting collectivized free electrons in the conductor obey the one-dimensional (longitudinal) quantized wave Schroedinger ψnz(z, t)-functions [4].

Fig. 2.
figure 2

General view of round cylindrical conductor l0 in length and r0 in radius with axial conduction current i0(t), where Δznh and Δznc are lengths (widths) of hot and cold longitudinal sections of conductor, respectively [2].

Full size image

With account for the quantum-mechanical approach to the longitudinal distribution of the drifting electrons in the crystalline structure of the conductor under calculation study, it is necessary to determine in the accepted approximation the maximum value of the quantum number n = nm for the longitudinal electron de Broglie half-waves λezn/2 = l0/n in length and accordingly the maximal number nm of the quantized zones of the longitudinal periodic localization Δznh long of these electrons in the round cylindrical conductor with the axial current of different types and ATPs.

MAIN CALCULATION RELATIONSHIPS

For the metal conductor under study with drifting free electrons, we write down the law of conservation of its elementary carriers of electricity in the following form:

$${{n}_{{eh}}}\Delta {{z}_{{nh}}}{{S}_{0}}{{n}_{m}} + {{n}_{{ec}}}\Delta {{z}_{{nc}}}{{S}_{0}}{{n}_{m}} \approx {{n}_{{em}}}{{l}_{0}}{{S}_{0}},$$
(1)

where nem is the averaged volume density of free electrons in the conductor metal before the axial conduction current i0(t) flows in it.

As is known, the quantity nem is equal to the concentration N0 of the conductor metal atoms multiplied by its valence determined by the number of the unpaired bound electrons on the valence electron subshells of these atoms (for example, for copper Cu, zinc Zn, and iron Fe, the valence is two [4], [10]). The concentration N0 (m–3) of the atoms in the conductor metal with its mass density d0 (kg/m3) before the beginning of the flow of current i0(t) in it is determined by known formula [4]

$${\text{ }}{{N}_{0}} = {{d}_{0}}{{({{M}_{{\text{a}}}} \times 1.6606 \times {{10}^{{ - 27}}})}^{{ - 1}}},$$
(2)

where Ma is the atomic mass of the conductor metal indicated in the periodic system of chemical elements developed by Mendeleev and practically equal to the mass number of the nucleus of the conductor metal atom counted in the atomic mass units (one atomic mass unit is about 1.6606 × 10–27 kg [10]).

The volume densities of the electrons drifting under the action of the voltage u0(t) applied to the conductor with the current i0(t) on the hot neh and cold nec longitudinal sections can be calculated at n → nm according to the following approximate analytic relations [2]:

$${{n}_{{eh}}} \approx 4\pi {{n}_{{em}}}{{[8 + {{(\pi - 2)}^{2}}]}^{{ - 1}}};$$
(3)
$${{n}_{{ec}}} \approx \pi (\pi - 2){{n}_{{em}}}{{[8 + {{(\pi - 2)}^{2}}]}^{{ - 1}}}.$$
(4)

As for the length Δznh of the hot longitudinal section of the conductor under consideration with the current i0(t), it is determined by the approximate relation of form [2]

$$\Delta {{z}_{{nh}}} \approx {{e}_{0}}{{n}_{{em}}}h{{({{m}_{e}}{{\delta }_{{0m}}})}^{{ - 1}}}{{[8 + {{(\pi - 2)}^{2}}]}^{{ - 1}}},$$
(5)

where e0 = 1.602 × 10–19 C is the module of the electric charge of the electrode [4]; h = 6.626 × 10–34 J s is the Planck constant [4]; me = 9.109 × 10–31 kg is the electron rest mass [4]; δ0m ≈ I0m/S0 is the amplitude of the electric current density in the conductor material; I0m is the amplitude of the electric conduction current i0(t).

For the length Δznc of the internal cold longitudinal section of the conductor with the electric conduction current i0(t), we have [2]

$$\Delta {{z}_{{nc}}} \approx {{l}_{0}}n_{m}^{{ - 1}} - {{e}_{0}}{{n}_{{em}}}h{{({{m}_{e}}{{\delta }_{{0m}}})}^{{ - 1}}}{{[8 + {{(\pi - 2)}^{2}}]}^{{ - 1}}}.$$
(6)

After substituting (3)–(6) into (1) and elementary transformations for the maximum value of the quantum number of the longitudinal electron de Broglie half-waves λezn/2 = l0/n long and the maximal number nm of the quantized zones of the longitudinal periodic localization Δznh in length (width) in the conductor under study, we obtain

$${{n}_{m}} \approx {{m}_{e}}{{\delta }_{{0m}}}{{l}_{0}}{{({{e}_{0}}{{n}_{{em}}}h)}^{{ - 1}}}{{K}_{0}},$$
(7)

where K0 = {[8 + (π – 2)2]2 – π(π – 2)[8 + (π – 2)2]} × [π(6 – π)]–1 is the coefficient about 5.922 numerically.

The analysis of calculation relationship (7) and the results of the quantum-mechanical calculations of the longitudinal wave distribution of the drifting free electrons in the cylindrical conductor with an electric current i0(t) of different types and ATPs presented in [1–3] shows that, to ensure a low calculation error, the quantum number nm according to (7) should be chosen for the cases when the values of the length l0 of the conductor on the electrotechnological conditions of its operation in the electric circuit and the length λezn/2 in it of the longitudinal quantized electron de Broglie half-waves are the minimum possible and the value of the amplitude δ0m of the current density in the conductor metal on the conditions of its thermal resistance is the maximum possible and, accordingly, when the value of the length Δznh of its hot longitudinal sections is the smallest. Assuming that in the round solid steel wire (r0 = 0.8 mm; l0 = 290 mm; nem = 16.82 × 1028 m–3 [2]) along its longitudinal axis OZ (see Fig. 2) there flows the aperiodic pulse of the axial electric current i0(t) of the temporary form 9 ms/160 ms (δ0m ≈ 0.37 kA/mm2 [2, 8]), from (7) for the maximum value of the quantum number n = nm of the longitudinal electron de Broglie half-waves λezn/2 = l0/n long and, accordingly, of the maximal number of the quantized zones of the longitudinal periodic localization Δznh ≈ 5.7 mm long (wide) by (5) of its drifting free electrons, it follows that nm ≈ 32.

VERIFICATION OF THE RESULTS FOR CALCULATING THE QUANTUM VALUE n m

We verify the validity (efficiency) of formula (7) for choosing the maximum value of the quantum number n = nm in the first approximation comparing the calculation results for the quantity nm according to (7) and earlier recommended relation of the following form [2]:

$${{n}_{m}} = 2n_{0}^{2},$$
(8)

where n0 is the main quantum number for the atoms of the conductor metal under study equal to the number of electron shells in these atoms and, accordingly, to the period number of this conductor metal in the periodic system of chemical elements developed by Mendeleev (for example, for copper Cu, zinc Zn, and iron Fe n0 = 4 [4]). It is seen that formula (8) does not take into account the impact of the current i0(t) ATPs and the geometric parameters of the conductor on the choice of the quantum number nm. This formula was derived on the basis of the author’s scientific hypothesis concerning the fact that the maximal number of the kinds of free electrons according to their orbital l, magnetic ml, and spin ms quantum numbers in the conductor metal is equal to the maximal number \(2n_{0}^{2}\) of the bound electrons in its atoms with the same main quantum number n0. From (8) for steel wire (n0 = 4) with the pulse current i0(t) of the mentioned temporal form 9 ms/160 ms (δ0m ≈ 0.37 kA/mm2), we find that in this particular case under consideration nm = 32. This quantitative result coincides with the numerical indicators for the number nm obtained by (7).

The calculation results of the averaged number n0m of the longitudinal quantized electron de Broglie half-waves in the metal conductor with the axial electric current of different ATPs presented in [11] also confirm the efficiency of derived calculation relationship (7). According to the sufficiently strict quantum mechanical approach at the estimation of the quantum number n0m in [11], the following formula was obtained:

$${{n}_{{0m}}} \approx {{m}_{e}}{{\delta }_{{0m}}}{{l}_{0}}{{({{e}_{0}}{{n}_{{em}}}h)}^{{ - 1}}}{{K}_{m}},$$
(9)

where Km ≈ 1.414 is the coefficient determined by the mathematical procedure of averaging the density of the axial electric current δ0(t) ≈ i0(t)/S0 in the conductor under study.

Comparing (7) and (9), we can state their surprising analytic similarity and the presence in them (these formulas) of immutable universal constants and identical quantities δ0m, l0, and nem, typical for the the considered electric conduction current i0(t) and the metal conductor. And we should not forget that these original quantum-mechanical formulas associated with the calculation of now little-studied wave longitudinal distributions of drifting free electrons in the conductor metal with the axial conduction current i0(t) of different kinds and ATPs were obtained by totally different ways. Equations (7) and (9) show that there is an inequality of the form: nm/n0m > 1. This is the way it should be for the conductor under study with any electric current i0(t) and any current-carrying (metal) section.

Please note that the validity of formula (9) to determine the averaged quantum number n0m for the longitudinal de Broglie electron half-waves λezn/2 long in the conductor with electric current i0(t) was confirmed by the data of the high-temperature experiments [2], [11] carried out with the direct participation of the author using the powerful high-voltage GIC with the aim of investigating the quantized WEPs and the hot longitudinal sections Δznh long (wide) in the galvanized steel wire (r0 = 0.8 mm, l0 = 320 mm, and Δ0 = 5 μm) with the aperiodic current pulse of the temporary form 9 ms/160 ms of a high density (δ0m ≈ 0/37 kA/mm2). According to (9), at these initial data for l0, nem, and δ0m, the required quantum value is n0m ≈ 9. Figure 3 presents a general view of this solid steel wire for n0m = 9 after the action of the powerful current pulse i0(t) with the abovementioned ATPs in the GIC high-current discharge circuit.

Fig. 3.
figure 3

General view of a galvanized steel wire (r0 = 0.8 mm, l0 = 320 mm, and Δ0 = 5 μm) after the passage of axial electric current i0(t) of a temporary aperiodic form 9 ms/160 ms of large density (I0m ≈ 745 A, δ0m ≈ 0.37 kA/mm2, n0m = 9, and Δznh ≈ 7 mm) [2].

Full size image

Four hot longitudinal sections Δznh ≈ 7 mm long (wide) heated to white heat (a temperature no less than 1200°С corresponds to this thermal condition of steel heating [10]), which become spherical due to the steel wire base melted on them and boil in these quantized (n0m = 9) zones of the longitudinal periodic localization of the drifting free electrons of the zinc coating of this wire, are clearly seen in Fig. 3 [2], [11]. It should be noted that according to (5) the calculated value of the parameter Δznh for the considered case (nem = 16.82 × 1028 m–3; δ0m ≈ 0.37 kA/mm2) is roughly Δznh ≈ 5.7 mm. As you can see, the differences between the experimental and calculated values for the length (width) Δznh of the hot longitudinal sections with regard to the steel wire under study (r0 = 0.8 mm; l0 = 320 mm; Δ0 = 5 μm) with this aperiodic pulse of the axial electric current i0(t) does not exceed 19%.

CONCLUSIONS

(1) According to the results of an analytic study of the longitudinal wave distribution of drifting free electrons in the isotropic metal of the thin cylindrical conductor of finite dimensions (l0 in length and r0 in radius) with an electrical axial conduction current of different types and ATPs, calculation quantum-mechanical relationship (7) has been derived to determine approximatively the maximum value of the quantum number n = nm in this metal conductor with the current i0(t) for the longitudinal quantized electron de Broglie half-waves λezn/2 = l0/n long and, accordingly, the maximum value nm for the quantized zones of the longitudinal periodic localization Δznh long (wide) of free electrons drifting under the action of the electric voltage u0(t) applied to its opposite edges.

(2) The verification results for derived relationship (7) indicate its validity (efficiency) in the region of both the high-voltage impulse equipment and the electrophysical processing of metals by the pressure of the pulse electric conduction current i0(t) of a high density (about 0.1 kA/mm2 and more).

(3) The results obtained for the approximate calculation selection of the quantum value show the efficiency of the author’s scientific hypothesis that the maximal number of varieties of the drifting collective free electrons in the isotropic metal of the conductor under study with the electric conduction current i0(t) of different types and ATPs is determined by the maximal number 2n02 of the bound electrons in the atoms of the metal used in the conductor with the same main quantum number n0.