Intrinsic quartet states and band-like structures in $N=Z$ nuclei

Abstract

The band structure of $N=Z$ nuclei is constructed by projecting states of good angular momentum from intrinsic states defined in terms of quartets. The simplest of these intrinsic states is a condensate of collective quartets with isospin $T=0$. The other intrinsic states are built by promoting one quartet of the condensate to an excited $T=0$ configuration. From these intrinsic states, by angular momentum projection, band structures are generated that approximate well the experimental ones. The projected states also reproduce to a very good extent the spectra resulting from configuration interaction calculations based on the same quartets forming the intrinsic states. These results show that the quartet-based intrinsic states provide an appropriate framework to understand in a simple and intuitive manner the emergence of band-like structures in $N=Z$ nuclei.

1 Introduction

As pointed out already in the sixties and seventies [1,2,3,4,5,6,7,8,9,10,11,12], quartets play an important role in $N=Z$ nuclei. Quartets are meant as quasi-independent 4-body structures of two neutrons and two protons which are correlated in the configuration space. It is worth emphasizing that the quartets, as defined here, are different from the $\alpha$-clusters. The latter, predicted since the thirties [13,14,15,16], are commonly defined as a grouping of two neutrons and two protons in relative s-wave states and are characterized by close spacial correlations.

One of the first indications of quartet structures in the excited states of $N=Z$ nuclei came from the excited 0$^+$ states in $^{16}$O and $^{40}$Ca. These states, described in terms of 4p–4h configurations [5, 17, 18], are interpreted as a quartet excited from the closed shell to the next major shell. Quartet-type excitations have been also identified in various $\alpha$ transfer reactions. For example, quartet excitations of low energies, around 3–5 MeV, have been populated by $\alpha$ transfer on medium weight nuclei [10]. The fact that these quartet excitations appear at low energies, below the particle–hole excitations, is related to the small energy necessary to break the bonds between the quartets, which is smaller than the energy necessary to break a particle from a quartet [9].

On the theoretical side, the quartet structures in the excited states of $N = Z$ nuclei have been studied in the framework of various approximations, e.g., the stretched scheme [5,6,7,8], the roton model [9], the shell-model [19], the extended IBM model [20,21,22], the algebraic models [23,24,25,26], the multi-step shell model [27]. In addition, one finds many studies of excited states in $N=Z$ nuclei related to the more general issue of $\alpha$-clustering, which are based on cluster models, antisymmetric molecular dynamics, THSR model, etc (e.g., see [28] and the references quoted therein).

In recent years, the excited states of $N = Z$ nuclei have been also described by diagonalizing the shell model Hamiltonian in a quartet basis [29,30,31,32]. Relevant for the present paper is Ref. [32], in which we have shown that configuration interaction calculations, done with the quartets extracted from a set of special trial states, are able to describe rather well the band structures of light $N=Z$ nuclei. The goal of this paper is to demonstrate that the trial states employed in Ref. [32] actually play the role of true intrinsic states from which the bands of $N=Z$ nuclei can be generated directly by angular momentum projection. It will be shown that this approach provides a novel quartet-based framework, simpler than the one employed in Ref. [32], which is able to grasp the basic features of band-like structures in $N=Z$ nuclei.

In Sect. 2, we will define the intrinsic states in the formalism of quartets and outline the projection technique employed in this work. The criterion adopted for the choice of the intrinsic states will then be illustrated. In the same section we will compare spectra of $N=Z$ nuclei in the sd and pf shells obtained from the projection of these states with those resulting from configuration interaction calculations, shell model (SM) calculations and experimental data. In Sect. 3, we will summarize the results and draw the conclusions.

2 Formalism and applications

In this study we focus on the low-lying excited states of even-even $N=Z$ nuclei which are generated by the interaction of the valence nucleons outside a double-magic core. The excitations will be described in a quartet formalism analogous to the collective pair approximation (CPA) which has been employed long ago for the description of deformed systems of like-particles [33,34,35]. We remind that in the CPA approach the bands are associated to trial states expressed in terms of intrinsic pairs, composed by a linear superposition of pairs with well-defined angular momenta. Thus, the ground band is generated, through the angular momentum projection, from a condensate of intrinsic pairs. Furthermore, the $\beta$ band is associated to a trial state obtained by breaking an intrinsic pair from the pair condensate and promoting it into an “excited” intrinsic pair [35]. These trial states resemble the IBM intrinsic states employed to describe the deformed nuclei [36, 37]. In this case, the role of pairs with angular momenta $J=0$ and $J=2$ is played by the s and d bosons.

To describe the band structures in $N=Z$ nuclei we shall use a procedure similar to the CPA approach, but based on collective quartets instead of pairs. The quartets are formed by two neutrons and two protons coupled to the total isospin $T=0$. The quartet creation operator, of a well-defined angular momentum J, is defined by

$$\begin{aligned} q^+_{JM}= & {} \sum _{i_1j_1J_1}\sum _{i_2j_2J_2}\sum _{T'} q_{i_1j_1J_1,i_2j_2J_2,{T'}}\nonumber \\{} & {} \left[ \left[ a^+_{i_1}a^+_{j_1}\right] ^{J_1{T'}}\left[ a^+_{i_2}a^+_{j_2}\right] ^{J_2{T'}}\right] ^{JT=0}_{M}, \end{aligned}$$

(1)

where $a^+_{i\tau }$ creates either a proton or a neutron (depending on the isospin projection $\tau$) on the spherically-symmetric state $i \equiv \{n_i,l_i,j_i\}$. No restrictions on the intermediate couplings $J_1T'$ and $J_2T'$ are introduced and the amplitudes $q_{i_1j_1J_1,i_2j_2J_2,{T'}}$ are supposed to guarantee the normalization of the operator.

In the representation spanned by the quartets (1) we construct a set of intrinsic quartet states. Thus, following Ref. [32], we introduce the ground intrinsic state

$$\begin{aligned} \Big |\Theta _{g}\Big \rangle = {\mathcal {N}}_g\left( Q^+_g\right) ^n\Big |0\Big \rangle , \end{aligned}$$

(2)

where by n is denoted the number of quartets which can be formed with the valence nucleons outside the closed core, denoted by $|0\rangle$. As can be noticed, $|\Theta _{g}\rangle$ is a condensate of the intrinsic quartet $Q^+_g$ defined by

$$\begin{aligned} Q^+_g =\sum _J \alpha ^{(g)}_J\left( q^+_g\right) _{J0}, \end{aligned}$$

(3)

where

$$\begin{aligned} \left( q^+_g\right) _{J0}= & {} \sum _{i_1j_1J_1}\sum _{i_2j_2J_2}\sum _{T'} q^{(g)}_{i_1j_1J_1,i_2j_2J_2,{T'}}\nonumber \\{} & {} \left[ \left[ a^+_{i_1}a^+_{j_1}\right] ^{J_1{T'}}\left[ a^+_{i_2}a^+_{j_2}\right] ^{J_2{T'}}\right] ^{JT=0}_{0} \end{aligned}$$

(4)

In order to fix $Q^+_g$, we minimize the energy of the state $|\Theta _{g}\rangle$ with respect to the coefficients $q^{(g)}_{i_1j_1J_1,i_2j_2J_2,{T'}}$ and $\alpha _{g,J}$.

In addition to the ground intrinsic state, we introduce a set of “excited” intrinsic states which are generated by promoting one of the quartets $Q^+_g$ of $|\Theta _{g}\rangle$ to an excited $T=0$ configuration. These states have the general form

$$\begin{aligned} |\Theta _k\rangle = \left. \left. {\mathcal {N}}_k Q^\dag _k \left( Q^\dag _g\right) ^{(n-1)}\right| 0\right\rangle , \end{aligned}$$

(5)

with

$$\begin{aligned} Q^\dag _k= & {} \sum _J \alpha ^{(k)}_J\left( q^\dag _k\right) _{Jk}, \end{aligned}$$

(6)

$$\begin{aligned} \left( q^+_k\right) _{Jk}= & {} \sum _{i_1j_1J_1}\sum _{i_2j_2J_2}\sum _{T'} q^{(k)}_{i_1j_1J_1,i_2j_2J_2,{T'}}\nonumber \\{} & {} \left[ \left[ a^+_{i_1}a^+_{j_1}\right] ^{J_1{T'}}\left[ a^+_{i_2}a^+_{j_2}\right] ^{J_2{T'}}\right] ^{JT=0}_{k} \end{aligned}$$

(7)

Assuming that the quartet $Q^+_g$ has already been fixed, we construct the new quartet $Q^+_k$ by minimizing the energy of $|\Theta _k\rangle$ with respect to the coefficients $q^{(k)}_{i_1j_1J_1,i_2j_2J_2,{T'}}$ and $\alpha ^{(k)}_J$ (under the constraint of orthogonality when various states with the same k are involved). The states (5) will be identified with the value of the quantum number k. It can be seen that these states, as well as the state (2), have an undefined angular momentum.

In this work we will explore the ability of the trial states (2) and (5) to represent proper intrinsic states of $N=Z$ nuclei. To this end we will generate the spectrum of these nuclei by projecting states of good angular momentum from them. The projection technique which has been used employs standard tensor coupling rules which do not deserve special explanations. What is instead worth of being noticed is the fact that when projecting ${\mathcal {N}}$ intrinsic states associated to a given nucleus one can generate up to ${\mathcal {N}}$ states with the same angular momentum J. This projection does not guarantee neither that these states are orthogonal with each other nor that the Hamiltonian matrix is diagonal with respect to them. Thus, in these cases, a proper definition of the spectrum implies that we have first to build an orthonormal basis out of these projected states and then to diagonalize the Hamiltonian in such a basis. The maximum size of these basis in the calculations that we are going to present has been 6.

The approach described above for constructing spectra, based on angular momentum projection of the intrinsic quartet states (2, 5), will be contrasted with the one we have used in Ref. [32]. Namely, in Ref. [32] the spectrum of a $N=Z$ nucleus was constructed by performing a configuration interaction (CI) calculation in the space spanned by all the quartets of well-defined angular momentum which characterise the intrinsic states (2, 5). By working in the m-scheme, this space if formed by the states

$$\begin{aligned} \left| \Psi ^{(n)}_{{{\overline{M}}}},\{N_{JM}\}\right\rangle = \left. \left. \prod _{J,M\in {(-J,J)} }\left( q^+_{JM}\right) ^{N_{JM}}\right| 0\right\rangle \end{aligned}$$

(8)

under the conditions

$$\begin{aligned} \sum _{JM}N_{JM}=n,~~~~~~~\sum _{JM}MN_{JM}={\overline{M}}. \end{aligned}$$

(9)

The calculation requires first the orthonormalization of the states (8) and then the diagonalization of the Hamiltonian in this basis for the various ${{{\overline{M}}}}$ (in order to identify the angular momentum of the states). This approach will be referred to with the acronym QM (Quartet Model). It is worth noticing that the QM approach and the projection method are based on different calculations schemes. Indeed, in the projection method the linear combination of the quartets which define a state of given angular momentum is set already by the projection while in the QM method this combination is determined by the diagonalization of the Hamiltonian. For this reason, as seen below, the QM approach provides results closer to the exact results.

The formalism presented above is applied for the even-even $N=Z$ nuclei with the valence nucleons in the sd and pf major shells. Thus, the vacuum state $|0\rangle$ of the previous expressions stands for the nuclei $^{16}$O and $^{40}$Ca. The calculations for the sd and pf shell nuclei have been performed with the USDB [38] and KB3G [39] interactions, respectively.

Fig. 1

Energies of the intrinsic states (2) and (5) for $^{24}$Mg, $^{28}$Si and $^{48}$Cr. The numbers at the bottom represent the quantum number k characterizing the intrinsic states. Only states with energies below the dashed-dotted lines have been included in the calculations

The first problem that has to be faced in the approach just described is that of defining the most appropriate set of intrinsic states to involve in the calculations. In this work we have adopted the criterion of selecting the intrinsic states on the basis of their energy. In Fig. 1, we show the energies of the lowest intrinsic states (with the associate value of the quantum number k) in the cases of $^{24}$Mg, $^{28}$Si and $^{48}$Cr. The values of the angular momentum J entering the summations of Eqs. (3) and (6) have been restricted to $J=0,2,4$ for $|\Theta _{g}\rangle$ and $|\Theta _0\rangle$ while for $|\Theta _k\rangle$ ($k\ne 0$) we included the values $J=k,k+1,k+2$. Only in the case of $^{48}$Cr an extra $J=6$ quartet has been added to $|\Theta _{g}\rangle$. In all cases the lowest intrinsic state has been found to be the condensate (2), followed by a k=0 or 2 state (5). The calculations for the three nuclei quoted above have been done with the intrinsic states lying below the dashed-dotted lines shown in Fig. 1.

Fig. 2

$^{24}$Mg: experimental (EXP), projected (PROJ), QM and SM spectra. See text for details

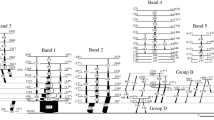
In Figs. 2, 3 and 4, the low-lying states obtained within the projected approach are compared with the experimental spectra and the results of QM and shell model (SM) calculations. For the experimental spectra we have shown only the states with certain angular momenta and parities. The numbers next to each level of the QM and SM spectra give the overlaps with the corresponding projected states while those at the bottom represent the ground state energies. At this point it is worth mentioning that a simple SM calculation provides only a sequence of states. Associating them with specific band-like structures, such as ground, $\beta$ and $\gamma$-like bands, requires additional analysis. In Figs. 2, 3 and 4 we have split the SM states in groups of levels following the correspondence with the band-like structures associated to the QM and the projected intrinsic states.

From Figs. 2, 3 and 4 one can notice that, in general, there is a clear correspondence among projected, QM and SM states. The only exception is in the case of $^{28}$Si, where one generates only two $J=2$ projected states and, in correspondence with the second of them, one finds two QM and three SM $J=2$ states. It can be also observed that the overlap of the projected states with the QM and SM states are significant, especially for the ground band. For the reason explained above, the QM levels are closer to the SM ones and, since the SM interaction is fitted to the data, the QM levels are also closer to the experimental spectrum.

Fig. 3

As in Fig. 1 for $^{28}$Si

Fig. 4

As in Fig. 2 for $^{48}$Cr

As seen from Figs. 2, 3 and 4, the agreement between projected states with the experimental data is rather good for all the nuclei. We find quite surprising that this agreement is obtained within such a simple approach, based only on projected states and a subsequent diagonalization in very reduced spaces (only a few units). These results support the definition of the states (2) and (5) as proper intrinsic states and show that the quartet structure of these intrinsic states is able to encapsulate the most important correlations which determine the spectra of even-even $N=Z$ nuclei.

3 Summary and conclusions

In this paper we have shown that the band structure of deformed $N=Z$ nuclei can be associated with intrinsic states based on quartets. The simplest of these states is just a condensate of a collective quartet with isospin $T=0$ and an undefined angular momentum. The other intrinsic states are built by promoting one quartet of this condensate to an excited $T=0$ configuration. A criterion has been introduced to select the most appropriate set of these states. We have shown that the band structure of $^{24}$Mg, $^{28}$Si and $^{48}$Cr can be generated to a very good extent by projecting states of good angular momentum from the above intrinsic states. These results demonstrates that the emergence of band-like structures in $N=Z$ nuclei can be simply understood in terms of quartet-based intrinsic states. This simple manner of generating bands from intrinsic quartet states is similar to the one employed earlier for describing deformed like-particle systems [33,34,35]. The basic difference is that in the latter case the intrinsic states are built in terms of pairs instead of quartets. This interesting analogy between quartets and pairs had already emerged in a previous analysis of the proton-neutron pairing Hamiltonian [41] where it had been observed that quartets played the same role as Cooper pairs in the case of a like-particle pairing Hamiltonian.

In the present study we have focused on the validity of the quartet approach for the energy spectra of $N=Z$ nuclei. An open issue, which will be addressed in a future study, is how good is this approach for describing the electromagnetic transitions in these nuclei.

We like to emphasize the interesting analogy between the formalism presented in the present work, based on general two-body interactions of SM type, and the one employed in the case of the isovector-plus-isoscalar proton-neutron pairing interaction, discussed in Ref. [40]. In the latter case, it was evidenced that a very accurate approximation of the ground and excited states could be provided, respectively, by a condensate of $T=0$, $J=0$ quartets (each built with isovector and isoscalar pairs) and by states obtained by promoting one quartet of this condensate to an excited $T=0$ configuration. The intrinsic states employed in the present work appear to be a generalization of these states in the case of deformed systems. The basic difference between the states of Ref. [40] and the intrinsic states of this work is that while the former have a well defined angular momentum, the latter do not. Thus, in the present case, in order to generate the spectrum of a $N=Z$ nucleus it has been necessary to go through an additional step, namely to project states of good angular momentum from the intrinsic states.

Finally we would like to comment on the relation between the present quartet approach and the semi-microscopic algebraic quartet model which has been recently employed to describe the band structures of sd and pf shell nuclei [23,24,25,26]. In this model the authors use a phenomenological interaction expressed in terms of the invariant operators of the $U(3) \supset SU(3) \supset SO(3)$ group-chain. This interaction contains essentially a harmonic oscillator term, a quadrupole–quadrupole force and a rotational term. This interaction is very different from the general two-body interaction employed in the present study. In particular, the Hamiltonian of the algebraic quartet model does not include the isovector-isoscalar proton-neutron pairing interaction which plays a major role in the low-lying states of $N=Z$ nuclei [42]. Whether the algebraic quartet model could be extended to include the proton-proton pairing interaction, preserving a simple algebraic structure, is an interesting question worth to be explored.