Computer Modeling of Phase Transformations and Critical Properties of the Frustrated Heisenberg Model for a Cubic Lattice

Abstract

The investigation of the phase transformations and critical properties of the Heisenberg antiferromagnetic model on a cubic lattice was performed by the Monte Carlo method with account for interaction of nearest and next-nearest neighbors. The next-nearest neighbors exchange couplings are considered ranged in 0.0 ≤ r ≤ 1.0. The phase diagram of dependence of the critical temperature on the next-nearest neighbors exchange coupling is plotted. It is shown that a phase transformation of the second kind is observed in the considered range of exchange values r. Using the theory of finite-dimensional scaling, the values of all main static critical indices are computed. It is demonstrated that the class of universality of the critical behavior of this model preserves in the range 0.0 ≥ r ≥ 0.4.

1 INTRODUCTION

Today, the phase transformations (PT) and the critical properties in spin systems with competing exchange interactions are being intensively studied. The competition between the exchange interaction mechanisms may lead to appearance of the frustration effects in the system. The presence of frustrations in the system implies several fundamental changes in the properties [1–4]. The spin systems with frustrations predominately manifest the properties different from the corresponding nonfrustrated systems. This difference is expressed by a rich variety of phases and PTs, by a strong degeneration of the ground state, and by the high susceptibility of frustrated systems to various disturbing interactions [4, 5].

By now, a lot of interesting results have been obtained for the two- and three-dimensional Ising model with competing exchange interactions on various types of lattices [6–11]. The PTs and the critical properties of the classical Heisenberg model with competing exchange interactions are practically not studied for the three-dimensional case.

In this work, using the replica algorithm of the Monte Carlo method (MC), we investigate the PTs and the critical properties of the Heisenberg antiferromagnetic model on a cubic lattice with account for interaction of nearest and next-nearest neighbors in the range 0.0 ≥ r ≥ 1.0, where r = J₂/J₁ is the next-nearest neighbors exchange coupling (J₁ and J₂ are the exchange constants of nearest and next-nearest neighbors, respectively).

The interest to the studied model is caused by the fact that this model becomes frustrated when we take into account the antiferromagnetic interactions of next-nearest neighbors. In spin systems with frustrations many physical properties are strongly dependent on the next-nearest neighbors exchange coupling. In addition, the Heisenberg antiferromagnetic model on a cubic lattice with account for interaction of nearest and next-nearest neighbors is still underinvestigated. Thus, the investigation of this model based on the modern method allows obtaining several answers to the questions related with the PTs and critical properties of frustrated spin systems.

2 MODEL AND METHOD OF INVESTIGATION

The Heisenberg antiferromagnetic model with account for interactions of nearest and next-nearest neighbors is described by the following Hamiltonian

$$H = - {{J}_{1}}\sum\limits_{\left\langle {i,j} \right\rangle } {({{{\mathbf{S}}}_{j}} \cdot {{{\mathbf{S}}}_{j}})} - {{J}_{2}}\sum\limits_{\left\langle {i,l} \right\rangle } {({{{\mathbf{S}}}_{j}} \cdot {{{\mathbf{S}}}_{l}}),} $$

(1)

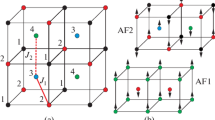
where S_i is the three-component unit vector S_i = (${\mathbf{S}}_{i}^{x}$, ${\mathbf{S}}_{i}^{y}$, ${\mathbf{S}}_{i}^{z}$). The lattice consists of two-dimensional square layers disposed one above the other along the orthogonal axis. The first term in formula (1) characterizes the antiferromagnetic interaction of all nearest neighbors, and this interaction is taken identical both inside the layers and between the layers (J_l < 0). The second term characterizes the antiferromagnetic interaction of next-nearest neighbors situating in the same layer (J₂ < 0).

Today, the PTs and critical properties of frustrated spin systems on the basis of microscopic Hamiltonians are rather successfully studied by the MC methods [12–18]. The MC methods allow investigating the physical properties of spin systems of practically arbitrary complexity. By now, using the MC methods, the entire classes of spin systems have been studied and the critical indices of a wide spectrum of models have been computed. In this study we used the replica-exchange algorithm of the MC method [19], which is the most powerful and efficient tool for studying frustrated spin systems.

3 RESULTS OF MODELING

The computations were carried out for the systems with periodic boundary conditions and with linear dimensions L × L × L = N, L = 24–60. To settle the system to the state of thermodynamic equilibrium, we chop the segment with the length τ₀ = 4 × 10⁵ MC steps/spin, which is several times larger than the length of nonequilibrium segment. We averaged the thermodynamic values along the Markov chain with the length τ = 500τ₀ MC steps/spin.

To observe the temperature dependences of the heat capacity C and susceptibility χ, we used the expressions [20]

$$C = (N{{K}^{2}})\left( {\left\langle {{{U}^{2}}} \right\rangle - {{{\left\langle \, \right\rangle }}^{2}}} \right),$$

(2)

$$\chi = \left\{ \begin{gathered} (NK)\left( {\left\langle {{{m}^{2}}} \right\rangle - {{{\left\langle {\left| m \right|} \right\rangle }}^{2}}} \right),\quad T < {{T}_{{\text{N}}}}, \hfill \\ (NK)\left\langle {{{m}^{2}}} \right\rangle ,\quad T \geqslant {{T}_{{\text{N}}}}, \hfill \\ \end{gathered} \right.$$

(3)

where K = |J₁|/k_BT, N is the number of particles, T_N is the critical temperature, U is the internal energy, and m is the order parameter (U and m are normalized quantities).

We calculated the order parameter m of the system using the formula

$${{m}_{i}} = \frac{4}{N}\sum\limits_{i \in \lambda } {{{{( - 1)}}^{z}}{\mathbf{S}}} ,\quad {\text{where}}\quad \lambda = 1,2,3,4,$$

(4)

$${{m}^{a}} = [{{m}_{1}} + {{m}_{2}} - ({{M}_{3}} + {{m}_{4}})]{\text{/}}4,$$

(5)

$${{m}^{b}} = [{{m}_{1}} + {{m}_{4}} - ({{m}_{2}} + {{m}_{3}})]{\text{/}}4,$$

(6)

$$m = \sqrt {{{{({{m}^{a}})}}^{2}} + {{{({{m}^{b}})}}^{2}}} ,$$

(7)

where m₁, m₂, m₃, and m₄ are the order parameters in the sublattices and z is the number of layer of the lattice.

In Figs. 1 and 2 we present the temperature dependences of the order parameter at L = 30 for different values of r (from now on the statistical error does not exceed the size of the symbols used to plot the dependences). Note that, as the value r increases in the interval 0.0 ≤ r ≤ 0.5, the drop in the order parameter shifts towards lower temperatures. In the range 0.6 ≤ r ≤ 1.0 we observe the opposite pattern. As r increases from 0.6 to 1.0, the drop of the order parameter shifts towards higher temperatures.

Fig. 1.

Order parameter m over temperature k_BT/|J₁| for values r in range 0 ≤ r ≤ 0.5.

Fig. 2.

Order parameter m over temperature k_BT/|J₁| for values r in range 0.6 ≤ r ≤ 1.0.

Figures 3 and 4 show the temperature dependences of the susceptibility obtained at L = 30 for different values of r. Note that, as the value r increases in the range 0.0 ≤ r ≤ 0.5, the maximums shift towards lower temperatures, and, simultaneously, we see a growth in the absolute values of the susceptibility maximums. The increase in the absolute values of maximums occurs due to competition between nearest and next-nearest neighbors. In the case 0.6 ≤ r ≤ 1.0 we observe an opposite pattern. With an increase in r from 0.6 to 1.0, a decrease in the absolute values of susceptibility maximums is observed and the maximums shift towards higher temperatures.

Fig. 3.

Susceptibility of χ over temperature k_BT/|J₁| for values r in range 0 ≤ r ≤ 0.5.

Fig. 4.

Susceptibility of χ over temperature k_BT/|J₁| for values r in range 0.6 ≤ r ≤ 1.0.

The increase in the interaction of next-nearest neighbors in this interval leads to an increase in the interaction energy by its absolute value, which strengthens the stiffness of the system and the temperature of the phase transformation therefore grows.

To determine the critical temperature T_N, we used the method of the fourth-order Binder cumulants U_L [21]:

$${{U}_{L}} = 1 - \frac{{\left\langle {{{m}^{4}}} \right\rangle }}{{3\left\langle {{{m}^{2}}} \right\rangle _{L}^{2}}}.$$

(8)

According to the theory of finite-dimensional scaling, the point of intersection of all curves U_L(T) is a critical point [21]. Expression (8) allows determining the critical temperature with a high degree of accuracy.

In Fig. 5 we plot the typical dependences of U_L on temperature at r = 0.7 for different values of L. The figure demonstrates the degree of accuracy of critical temperature determination. We observe a clearly pronounced point of intersection (T_N = 0.963; from now on, the temperature is given in units |J₁|/k_B) in the critical domain. Similarly, we determined the critical temperatures for the remaining values of r.

Fig. 5.

Binder cumulant U_L over temperature k_BT/|J₁| for r = 0.7 and different L.

In Fig. 6 we draw the phase diagram of the critical temperature dependence on the next-nearest neighbors exchange coupling.

Fig. 6.

Phase diagram of dependence of critical temperature on next-nearest neighbors exchange coupling.

In the diagram we see that three different phases intersect near the value r = 0.5: I antiferromagnetic phase, II paramagnetic phase, and III superantiferromagnetic phase. It was shown in work [22] that the PTs of the second kind are observed for all considered values of r.

To compute the static critical indices of heat capacity α, order parameter β, susceptibility γ, and correlation radius ν, we applied the relations of the theory of finite-dimensional scaling. From the theory of finite-dimensional scaling it follows that, in the system with dimensions L × L × L, the following expressions are satisfied at k_BT/|J₁| = k_BT_N/|J₁| and relatively large L [23–25]

$$m\sim {{L}^{{ - \beta /\nu }}},$$

(9)

$$\chi \sim {{L}^{{\gamma /\nu }}},$$

(10)

$${{V}_{i}}\sim {{L}^{{1/\nu }}}{{g}_{{{{V}_{i}}}}},$$

(11)

where ${{g}_{{{{V}_{i}}}}}$ is the constant and in place of V_i we may use

$${{V}_{i}} = \frac{{\left\langle {{{m}^{i}}E} \right\rangle }}{{\left\langle {{{m}^{i}}} \right\rangle }} - \left\langle E \right\rangle \quad (i = 1,2,3).$$

(12)

We used these expressions to determine the values of critical indices β, γ, and ν.

To approximate the temperature dependence of the heat capacity on L, the following formula is commonly used in practice [6]

$${{C}_{{\max }}}(L) = {{A}_{1}} - {{A}_{2}}{{L}^{{\alpha /\nu }}},$$

(13)

where A₁ and A₂ are some coefficients.

In Fig. 7 in the double logarithmic plot we present the typical dependences of parameters V_i at i = 1, 2, 3 on linear dimensions of lattice L for r = 0.7. We see in the figure that all points lie on the straight line in the graphs within the error. The dependences in the figure plotted by the least-squares method are parallel to each other. The angle of inclination of the straight line determines the value 1/ν. The value ν calculated in this manner was used to determine the critical indices of heat capacity α, order parameter β, and susceptibility γ.

Fig. 7.

Parameter V_i over linear dimensions of system L at T = T_N for r = 0.7.

In Figs. 8 and 9 in the double logarithmic plot we draw the typical dependences of the magnetic order parameter m and of the susceptibility χ on the linear dimensions of lattice L for r = 0.7. All points lie on the straight lines within the error. The inclination angles of these straight lines determine the values β/ν and γ/ν. Using this scheme, we determined the value for the heat capacity α/ν. With the data on ν we computed the static critical indices α, β, and γ.

Fig. 8.

Order parameter m over linear dimensions of system L at T = T_N for r = 0.7.

Fig. 9.

Susceptibility of χ over linear dimensions of system L at T = T_N for r = 0.7.

This procedure was applied to compute the critical indices for all considered values of r. All values of static critical indices obtained in this manner are given in Table 1. The procedure used by us to determine the Fisher index η is described in more detail in work [26]. The data that we obtained for the Fisher index η are also provided in the table.

Table 1. Values of critical parameters for antiferromagnetic Heisenberg model on a cubic lattice

Note that for the value r = 0.5 we did not succeed in computing the critical indices with an admissible error. We suggest that it is related with the fact that in this point three different phases coexist.

It is seen in Table 1 that the critical temperature k_BT_N/|J₁| decreases as the next-nearest neighbors exchange coupling increases until the value r = 0.6. As r continues to increase, the critical temperature begins to increase. All values of critical indices which we computed in the range 0.0 ≤ r ≤ 0.4 coincide within the error with the values of critical indices in the three-dimensional nonfrustrated Heisenberg model [27]. This indicates that this model in the range 0.0 ≤ r ≤ 0.4 belongs to the same class of universality of the critical behavior as the nonfrustrated Heisenberg model. The values of critical indices computed by us in the range 0.6 ≤ r ≤ 1.0 are significantly different from the data obtained in the range 0.0 ≤ r ≤ 0.4. We may suggest that the account for interaction of next-nearest neighbors inside the layers of the lattice leads to the change in the class of universality of the critical behavior for the antiferromagnetic Heisenberg model on a cubic l-attice.

4 CONCLUSIONS

We studied the phase transformations and critical behavior of the antiferromagnetic Heisenberg model on a cubic lattice with account for interaction of nearest and next-nearest neighbors inside the layers of the lattice using the high-efficient replica algorithm of the Monte Carlo method. We plotted the phase diagram of dependence of the critical temperature on the next-nearest neighbors exchange coupling. We determined the values of critical temperatures and computed the values of all main static critical indices in the range 0.0 ≤ r ≤ 1.0. We established the laws of variation in the critical parameters in the considered range of r. We revealed that in the range 0.0 ≤ r ≤ 0.4 the system demonstrates the universal critical behavior. We showed that the considered model exhibits another critical behavior in the range 0.6 ≤ r ≤ 1.0.