Geodesics in the Wormhole Gravitational Field

Abstract

The structure of spacetime near a wormhole (WH) and possible observational consequences are investigated theoretically. In connection with the growing accuracy of observations and the prospects of a new gravitational-wave channel, the problem of distinguishing between astrophysical manifestations of black holes (BHs) and hypothetical WHs is becoming relevant. WHs, along with BHs, naturally arise within general relativity (GR). Observational searches for WHs require knowledge of the characteristic trajectories of bodies in its vicinity, including the trajectories entering its throat. Equations of motion of a test particle in the WH metric are derived, and the most interesting properties of these motions are considered. A general equation of geodesics in the WH metric is derived, and some properties of these geodesics are considered. The exact solution for circular orbits of test particles around a WH, as well as an approximate analytical solution of the geodesic equations, is analyzed. The shift of the pericenter of the orbit of a test particle in the WH field is considered, and possible observational consequences are discussed. Examples of test particle trajectories near a WH are presented that are obtained by numerical simulation.

1 INTRODUCTION

In general relativity (GR) several solutions arise that describe relativistic objects the speed of test particles near which is comparable to the speed of light. First of all, these are black holes (BHs)—solutions of GR equations found by Schwarzschild and Kerr. BHs have been discovered both in the electromagnetic observation channel [1] and in the gravitational-wave channel [2]. The discovery of BHs inspires confidence that other solutions of GR (to date, only theoretical ones) can exist in space. One of such hypothetical solutions is wormholes (WHs). Today there exist several solutions of the WH type [3, 4]; see review [5]. Both GR solutions and observational manifestations of WHs have been considered in the literature [6–8].

To describe the observational manifestations near a WH, it is necessary to know the test particles motion law, in other words, the shape of geodesics near the WH. In the present study, we derive equations of motion of a test particle in the WH metric and consider the most interesting properties of these motions.

In Section 2 we consider the WH metric and some general properties of this metric. In Section 3 we derive the general equation of geodesics in the WH metric and consider some properties of these geodesics. In Section 4 we analyze the exact solution for circular orbits of test particles around a WH. In Section 5 we consider an approximate analytical solution of geodesic equations and some of its properties. Finally, in Section 6 we consider the orbit pericenter shift of a test particle in the WH field and discuss possible observational consequences. The Appendix presents examples of test particle trajectories near a WH, obtained by numerical simulation.

2 THE WORMHOLE METRIC AND ITS PROPERTIES

Let us take the WH metric in the simplest form:

$$\begin{gathered} d{{s}^{2}} = \left( {1 - \frac{{{{r}_{{\text{g}}}}}}{r}} \right){{c}^{2}}d{{t}^{2}} - \frac{{d{{r}^{2}}}}{{1 - {{r}_{0}}{\text{/}}r}} \\ \, - {{r}^{2}}(d{{\theta }^{2}} + {{\sin }^{2}}\theta d{{\varphi }^{2}}). \\ \end{gathered} $$

(1)

Here ct, r, θ, and φ are coordinates x⁰, x¹, x², and x³, respectively. The quantity r_g is the gravitational radius of the WH, and r₀ is its throat radius. Unlike the Schwarzschild metric, metric (1) is a two-parameter one and is determined by the parameters r_g and r₀.

We can transform the radial coordinate as follows:

$$dl = \pm \frac{{dr}}{{\sqrt {1 - {{r}_{0}}{\text{/}}r} }}.$$

Then the WH metric (1) can be rewritten as

$$\begin{gathered} d{{s}^{2}} = \left( {1 - \frac{{{{r}_{{\text{g}}}}}}{{r(l)}}} \right){{c}^{2}}d{{t}^{2}} - d{{l}^{2}} \\ \, - r{{(l)}^{2}}(d{{\theta }^{2}} + {{\sin }^{2}}\theta d{{\varphi }^{2}}). \\ \end{gathered} $$

(2)

In order that a WH have no BH horizons, the function g₀₀(l) should be subject to a condition according to which it must be greater than zero in the whole range (–∞ < l < ∞). Here, under the condition r₀ > r_g, the function g₀₀(l) is greater than zero. In this case, the function r²(l) (the squared radius) should attain its minimum at the WH throat, which is determined by the points l = 0 and r = r₀. The transformation from the radial coordinate r, which is ambiguous (the same value of r can belong to different folds of the whole space), to the radial coordinate l, which uniquely determines the position of each point in the whole space, is given by

$$l = + \int\limits_{{{r}_{0}}}^r {\frac{{dr}}{{\sqrt {1 - \frac{{{{r}_{0}}}}{r}} }} = \sqrt {r(r - {{r}_{0}})} } + \frac{{{{r}_{0}}}}{4}{\text{ln}}\left\{ {\sqrt {\frac{r}{{{{r}_{0}}}}} + \sqrt {\frac{r}{{{{r}_{0}}}} - 1} } \right\}$$

for the upper fold of the WH space and by

$$l = - \int\limits_{{{r}_{0}}}^r {\frac{{dr}}{{\sqrt {1 - \frac{{{{r}_{0}}}}{r}} }} = - \sqrt {r(r - {{r}_{0}})} } - \frac{{{{r}_{0}}}}{4}\ln \left\{ {\sqrt {\frac{r}{{{{r}_{0}}}}} + \sqrt {\frac{r}{{{{r}_{0}}}} - 1} } \right\}$$

for the lower fold of the WH space.

Metric (2), as well as the first and second derivatives of the radial coordinate with respect to l, are regular in the whole range (–∞ < l < +∞):

$$\frac{{dr}}{{dl}} = \pm \sqrt {1 - \frac{{{{r}_{0}}}}{r}} ,\quad \frac{{{{d}^{2}}r}}{{d{{l}^{2}}}} = \frac{{{{r}_{0}}}}{{2{{r}^{2}}}}.$$

(3)

Metric (2) corresponds to the energy–momentum tensor $T_{k}^{i}$, which violates the null energy condition (NEC) for radial photons.

The nonzero components of this tensor have the form

$$T_{r}^{r} = \frac{{{{r}_{0}} - {{r}_{g}}}}{{8\pi {{r}^{2}}(r - {{r}_{g}})}} > 0,$$

$$T_{\theta }^{\theta } = T_{\varphi }^{\varphi } = \frac{{({{r}_{g}} - {{r}_{0}})(2r - {{r}_{g}})}}{{32\pi {{r}^{2}}{{{(r - {{r}_{g}})}}^{2}}}} < 0.$$

Below we discuss the shape of the trajectories of test particles both in the r-coordinate system and in the l-coordinate system.

3 EQUATIONS OF MOTION OF A TEST PARTICLE AROUND A WORMHOLE

3.1 Analysis of Geodesics in the WH Spacetime

Let us analyze the equation of geodesics in the WH spacetime. We take the metric in the form (1) (r is the radial coordinate). The geodesics are also the equations of motion of test particles. We will assume that the equations of motion obtained refer to the motion of a test particle along the upper fold of the space.

The ordinary derivative of the particle trajectory with respect to proper time s is expressed in terms of its components

$${{u}^{i}} \equiv \frac{{d{{x}^{i}}}}{{ds}}.$$

In GR, this quantity is also called a contravariant 4-velocity. The geodesic equation is defined for the tangent vector along the particle trajectory uⁱ,

$$\frac{{d{{u}^{i}}}}{{ds}} + \Gamma _{{jk}}^{i}{{u}^{j}}{{u}^{k}} = 0.$$

For the covariant components of the tangent vector u_i, the corresponding equation has the form

$$\frac{{d{{u}_{i}}}}{{ds}} = \frac{1}{2}\frac{{\partial {{g}_{{jk}}}}}{{\partial {{x}^{i}}}}{{u}^{j}}{{u}^{k}}.$$

Since the metric (1) is static and spherically symmetric, we can immediately write two integrals of motion [9]:

$${{u}_{0}} \equiv \epsilon = {\text{const}},$$

(4)

$${{u}_{3}} = h = {\text{const}}{\text{.}}$$

(5)

The first integral is the conservation of the total energy of the system, and the second is the conservation of angular momentum. Moreover, it can be shown that the test particle moves in a plane, which means that θ(s) = const. We can choose θ(s) = π/2. Then the integrals can be rewritten as

$$\begin{gathered} \left( {1 - \frac{{{{r}_{{\text{g}}}}}}{r}} \right)\frac{{dt}}{{ds}} = \epsilon , \\ {{r}^{2}}\frac{{d\varphi }}{{ds}} = h. \\ \end{gathered} $$

(6)

Thus, we have equations for three coordinates. Let us derive an equation for the evolution of the radial coordinate r. We will derive this equation in the same way as it is done in the analysis of motion in the BH metric. To find the equations of motion along the radius, we use the metric equation:

$$\begin{gathered} 1 = \left( {1 - \frac{{{{r}_{{\text{g}}}}}}{r}} \right)\frac{{d{{t}^{2}}}}{{d{{s}^{2}}}} - \frac{1}{{1 - \frac{{{{r}_{0}}}}{r}}}\frac{{d{{r}^{2}}}}{{d{{s}^{2}}}} \\ \, - {{r}^{2}}\left( {\frac{{d{{\theta }^{2}}}}{{d{{s}^{2}}}} + {{{\sin }}^{2}}\theta \frac{{d{{\varphi }^{2}}}}{{d{{s}^{2}}}}} \right). \\ \end{gathered} $$

We will consider the case of a massive test particle (moving at a speed less than the speed of light).

Substitute θ = π/2 into the metric equation and obtain the equation

$$1 = \left( {1 - \frac{{{{r}_{{\text{g}}}}}}{r}} \right)\frac{{d{{t}^{2}}}}{{d{{s}^{2}}}} - \frac{1}{{1 - {{r}_{0}}{\text{/}}r}}\frac{{d{{r}^{2}}}}{{d{{s}^{2}}}} - {{r}^{2}}\frac{{d{{\varphi }^{2}}}}{{d{{s}^{2}}}}.$$

This equation can be transformed as

$$\left( {1 - \frac{{{{r}_{{\text{g}}}}}}{r}} \right)\frac{{d{{t}^{2}}}}{{d{{s}^{2}}}} - \frac{{d{{\varphi }^{2}}}}{{d{{s}^{2}}}}\left( {\frac{1}{{1 - {{r}_{0}}{\text{/}}r}}\frac{{d{{r}^{2}}}}{{d{{\varphi }^{2}}}} + {{r}^{2}}} \right) = 1.$$

Substitute the integrals of motion and obtain the equations

$$\frac{{{{\epsilon }^{2}}}}{{1 - {{r}_{{\text{g}}}}{\text{/}}r}} - \frac{{{{h}^{2}}}}{{{{r}^{4}}}}\left( {\frac{1}{{1 - {{r}_{0}}{\text{/}}r}}\frac{{d{{r}^{2}}}}{{d{{\varphi }^{2}}}} + {{r}^{2}}} \right) = 1,$$

(7)

$${{({{u}^{r}})}^{2}} = \left( {1 - \frac{{{{r}_{0}}}}{r}} \right)\left( {\frac{{{{\epsilon }^{2}}}}{{1 - {{r}_{{\text{g}}}}{\text{/}}r}} - 1 - \frac{{{{h}^{2}}}}{{{{r}^{2}}}}} \right).$$

(8)

Next, we make the substitution

$$u = \frac{1}{r},$$

which finally leads to the equation

$${{\left( {\frac{{du}}{{d\varphi }}} \right)}^{2}} + (1 - {{r}_{0}}u){{u}^{2}} = \frac{{{{\epsilon }^{2}} - 1 + {{r}_{{\text{g}}}}u}}{{{{h}^{2}}}}\frac{{1 - {{r}_{0}}u}}{{1 - {{r}_{{\text{g}}}}u}}.$$

(9)

When analyzing the equations of motion in the BH metric, one has a similar equation that looks like

$${{\left( {\frac{{du}}{{d\varphi }}} \right)}^{2}} + (1 - {{r}_{{\text{g}}}}u){{u}^{2}} = \frac{{{{\epsilon }^{2}} - 1}}{{{{h}^{2}}}} + \frac{{{{r}_{{\text{g}}}}}}{{{{h}^{2}}}}u.$$

We can also compare (9) with the nonrelativistic equation of motion. It is obtained if we neglect r₀u and r_gu compared to unity:

$${{\left( {\frac{{du}}{{d\varphi }}} \right)}^{2}} + {{u}^{2}} = \frac{{{{\epsilon }^{2}} - 1 + {{r}_{{\text{g}}}}u}}{{{{h}^{2}}}}.$$

Let us write some more useful equations.

For a WH (in contrast to a BH), the radial coordinate r can be considered as a component of the metric (1). We can say that r is the transverse radial coordinate, and the longitudinal radial coordinate is given by l from the metric (2).

Therefore, the vanishing of the derivative $\dot {r}$ does not mean the cessation of motion along the longitudinal radial coordinate l. For this reason, the condition $\dot {r}$ = 0 is always satisfied at the WH throat; however, in the general case, $\dot {l}$ ≠ 0 at the WH throat.

3.2 Reachability Condition of a Wormhole Throat by a Free-Falling Particle

Let us write down the value of $\dot {l}$ at the throat of a WH. Taking into account expressions (3) and (8), we obtain the following relation at an arbitrary point:

$$\frac{{dl}}{{ds}} = {{u}^{l}} = \pm \sqrt {\frac{{{{\epsilon }^{2}}}}{{1 - {{r}_{{\text{g}}}}{\text{/}}r}} - 1 - \frac{{{{h}^{2}}}}{{{{r}^{2}}}}} .$$

At a throat point, r = r₀,

$${{(u_{0}^{l})}^{2}} = \frac{{{{\epsilon }^{2}}}}{{1 - {{r}_{{\text{g}}}}{\text{/}}{{r}_{0}}}} - 1 - \frac{{{{h}^{2}}}}{{r_{0}^{2}}},$$

(10)

for a purely radial fall we have h = 0; therefore

$${{(u_{{00}}^{l})}^{2}} = \frac{{{{\epsilon }^{2}}}}{{1 - {{r}_{{\text{g}}}}{\text{/}}{{r}_{0}}}} - 1.$$

(11)

This expression shows that, in the case of radial fall, the overwhelming majority of trajectories reach the WH throat with nonzero longitudinal velocity, and vice versa, for the particle not to reach the WH throat, it is necessary that the right-hand side of expression (10) or (11) be negative.

In this case, there are no restrictions on the value of the integral of motion for the specific total energy $\epsilon$ except that $\epsilon$ > 0. The value $\epsilon$ = 1 corresponds to the fact that the particle has zero velocity at infinity. The range of values $\epsilon$ > 1 corresponds to the fact that the particle trajectory is infinite, and vice versa, the range of values $\epsilon$ < 1 corresponds to the fact that the particle is gravitationally captured and its trajectory is finite.

Trajectories can be analyzed either in r-coordinates (where the equations of motion look simpler) or in l‑coordinates. In the second case, the equations contain not only the square of the derivatives but also nonlinear functions of l, which are also implicit functions of this variable. Nevertheless, we will also analyze the equations of motion in l-coordinates. The reason is that, in r-coordinates, the equations of motion are subject to constraints of the form r ≥ r₀, which reduces the problem under consideration to the problem of motion with equations with nonholonomic unilateral constraints [10, 11]. Such equations require special treatment, which can be avoided in our case by considering the problem of motion in l-coordinates. It is especially convenient to consider the problem of motion of test particles in l-coordinates near the WH throat. In this case, r ≈ r₀, and the equations of motion can be analyzed in the limit of small l, i.e., for l ≪ r₀.

3.3 Eigenfrequency of Small Oscillations through the Wormhole Throat

Let us find the oscillation frequency of a test particle through the WH throat under the assumption that the oscillation amplitude is small.

We write the equation of motion of the particle in the form

$$\frac{{d{{u}_{k}}}}{{ds}} = \frac{1}{2}\frac{{\partial {{g}_{{ij}}}}}{{\partial {{x}^{k}}}}{{u}^{i}}{{u}^{j}}.$$

Then for k = r we have

$$\frac{{d({{u}^{r}}{{g}_{{rr}}})}}{{ds}} = \frac{1}{2}\left[ {\frac{{\partial {{g}_{{tt}}}}}{{\partial r}}{{{({{u}^{t}})}}^{2}} + \frac{{\partial {{g}_{{rr}}}}}{{\partial r}}{{{({{u}^{r}})}}^{2}}} \right].$$

Denoting the derivative with respect to proper time ds by a dot, we obtain

$$\begin{gathered} - \frac{{\ddot {r}}}{{1 - {{r}_{0}}{\text{/}}r}} + \frac{{{{r}_{0}}{\text{/}}{{r}^{2}}}}{{{{{(1 - {{r}_{0}}{\text{/}}r)}}^{2}}}}{{{\dot {r}}}^{2}} \\ = \frac{1}{2}\left[ {\frac{{{{r}_{{\text{g}}}}}}{{{{r}^{2}}}}\frac{{{{\epsilon }^{2}}}}{{{{{(1 - {{r}_{{\text{g}}}}{\text{/}}r)}}^{2}}}} + \frac{{{{r}_{0}}{\text{/}}{{r}^{2}}}}{{{{{(1 - {{r}_{0}}{\text{/}}r)}}^{2}}}}{{{\dot {r}}}^{2}}} \right]. \\ \end{gathered} $$

(12)

Here $\dot {r}$ ≡ u^r is defined by expression (20) (see below).

Let us transfer all terms in (12) to the right-hand side and multiply them by (1 – r₀/r)²:

$$\left( {1 - \frac{{{{r}_{0}}}}{r}} \right)\ddot {r} - \frac{{{{r}_{0}}}}{{2{{r}^{2}}}}{{\dot {r}}^{2}} + \frac{{{{r}_{{\text{g}}}}{{\epsilon }^{2}}{{{(1 - {{r}_{0}}{\text{/}}r)}}^{2}}}}{{2r_{0}^{2}{{{(1 - {{r}_{{\text{g}}}}{\text{/}}r)}}^{2}}}} = 0$$

or, denoting δr ≡ r – r₀, we obtain

$$\frac{{\delta r\delta \ddot {r}}}{r} - \frac{{{{r}_{0}}(\delta {{{\dot {r}}}^{2}})}}{{2{{r}^{2}}}} + \frac{{{{r}_{{\text{g}}}}{{\epsilon }^{2}}{{{(\delta r)}}^{2}}}}{{2r_{0}^{2}{{{(r - {{r}_{{\text{g}}}})}}^{2}}}} = 0.$$

(13)

Near the throat, r can be expanded in a series in small values of l. To this end, we use expression (3):

$$\begin{gathered} r(l) \approx {{r}_{0}} + \frac{{{{l}^{2}}}}{{4{{r}_{0}}}},\quad \delta r \approx \frac{{{{l}^{2}}}}{{4{{r}_{0}}}},\quad \delta \dot {r} \approx \frac{{l\dot {l}}}{{2{{r}_{0}}}}, \\ \delta \ddot {r} \approx \frac{{l\ddot {l}}}{{2{{r}_{0}}}} + \frac{{{{{(\dot {l})}}^{2}}}}{{2{{r}_{0}}}}. \\ \end{gathered} $$

(14)

Dividing (13) by δr/r and substituting the values of the derivatives from (14) into this expression, we obtain the following equation in the quadratic approximation with respect to l:

$$\frac{{l\ddot {l}}}{{2{{r}_{0}}}} + \frac{{{{{(\dot {l})}}^{2}}}}{{2{{r}_{0}}}} - \frac{{{{r}_{0}}}}{{2r}}\frac{{{{l}^{2}}{{{(\dot {l})}}^{2}}{\text{/}}(4r_{0}^{2})}}{{{{l}^{2}}{\text{/}}(4{{r}_{0}})}} + \frac{{{{r}_{{\text{g}}}}{{\epsilon }^{2}}{{l}^{2}}}}{{8r_{0}^{2}{{{({{r}_{0}} - {{r}_{{\text{g}}}})}}^{2}}}} = 0.$$

(15)

In the quadratic approximation in l, the second and third terms in (15) cancel each other out:

$$\frac{{l\ddot {l}}}{{2{{r}_{0}}}} + \frac{{{{r}_{{\text{g}}}}{{\epsilon }^{2}}{{l}^{2}}}}{{8r_{0}^{2}{{{({{r}_{0}} - {{r}_{{\text{g}}}})}}^{2}}}} = 0.$$

Hence we obtain the equation of harmonic oscillations in l:

$$\ddot {l} + {{\omega }^{2}}l = 0,\quad {{\omega }^{2}} \equiv \frac{{{{\epsilon }^{2}}{{r}_{{\text{g}}}}}}{{4{{r}_{0}}{{{({{r}_{0}} - {{r}_{{\text{g}}}})}}^{2}}}}.$$

The quantity ω determines the eigenfrequency of small oscillations near the WH throat for the longitudinal physical coordinate l as a function of proper time s.

4 ANALYSIS OF THE EXACT SOLUTION IN THE WORMHOLE METRIC IN THE CASE OF A CIRCULAR ORBIT

4.1 Circular Orbits and Their Stability

Consider the important case of circular orbits around a WH. We will analyze the exact equation of motion (9). Circular orbits are defined by the relation

$$\frac{{dr}}{{dt}} = \frac{{{{u}^{r}}}}{{{{u}^{t}}}} = 0.$$

(16)

In addition, there is another relation that defines stable and unstable orbits. In order to obtain the stability criterion, we should derive from (16) an equation for the derivative of the radius with respect to time:

$$\begin{gathered} {{\left( {\frac{{dr}}{{dt}}} \right)}^{2}} = \left( {1 - \frac{{{{r}_{0}}}}{r}} \right)\left( {1 - \frac{{{{r}_{{\text{g}}}}}}{r}} \right){{\epsilon }^{{ - 2}}} \\ \times \left[ {{{\epsilon }^{2}} - \left( {1 - \frac{{{{r}_{{\text{g}}}}}}{r}} \right)\left( {1 + \frac{{{{h}^{2}}}}{{{{r}^{2}}}}} \right)} \right]. \\ \end{gathered} $$

(17)

The extrema of the function

$$U(r) = \sqrt {\left( {1 - \frac{{{{r}_{{\text{g}}}}}}{r}} \right)\left( {1 + \frac{{{{h}^{2}}}}{{{{r}^{2}}}}} \right)} $$

determine the stability of the orbit. The minima of the function correspond to stable orbits, and the maxima, to unstable ones. The form of the function shows that it coincides with the energy function defining circular orbits in the Schwarzschild metric with gravitational radius r_g. Therefore, just as for the Schwarzschild BH, the last stable circular orbit in the WH metric (1) lies at r = 3r_g and has the following parameters: h = $\sqrt 3$r_g and $\epsilon$ = $\sqrt {8{\text{/}}9}$ (see [9, §102]).

It follows from the aforesaid that a stable circular orbit corresponds to the simultaneous solution of the two equations

$$\begin{gathered} {{\epsilon }^{2}} - \left( {1 - \frac{{{{r}_{{\text{g}}}}}}{r}} \right)\left( {1 + \frac{{{{h}^{2}}}}{{{{r}^{2}}}}} \right) = 0, \\ U'(r) = 0. \\ \end{gathered} $$

(18)

Here and below, the prime denotes the derivative with respect to r. Solving simultaneously system (18), we obtain

$$\begin{gathered} {{h}^{2}} = \frac{{{{r}_{{\text{g}}}}r}}{{2 - 3{{r}_{{\text{g}}}}{\text{/}}r}}, \\ {{\epsilon }^{2}} = \frac{{{{{(1 - {{r}_{{\text{g}}}}{\text{/}}r)}}^{2}}}}{{1 - (3{\text{/}}2){{r}_{{\text{g}}}}{\text{/}}r}}. \\ \end{gathered} $$

(19)

These two equations define the relationship between the specific angular momentum h and the specific total energy $\epsilon$ on the one hand and the radius r of a stable circular orbit of the particle on the other.

Let us draw special attention to the fact that expressions (4) and (19) do not depend on r₀. This is due to the fact that the quantities $\epsilon$ and h do not depend on the g_rr component of the metric (1). In the nonrelativistic limit r_g/r → 0, the second expression in (19) becomes the well-known Newton formula for the energy constant of a particle in a circular orbit around a massive center with mass M:

$$\epsilon \to 1 - M{\text{/}}(2r).$$

The minimal radius of the unstable orbit is r = (3/2)r_g; in this case, h → ∞ and $\epsilon$ → ∞.

Since orbits with r < r₀ are impossible in the WH metric, the presence of the last stable orbit, and even more so of the last unstable orbit, is determined by the relation between the gravitational radius of the WH and its throat radius. For r₀ > 3r_g, all circular orbits are stable.

In the case of r₀ = r_g, the last stable orbit appears. In the case of the Schwarzschild metric, a test particle bypassing r = (3/2)r_g makes less than one revolution around the BH. Let us calculate the total change in the angle when the particle leaves the last stable orbit in the case of a WH. Let us write the equation for u^r:

$${{({{u}^{r}})}^{2}} = \left( {1 - \frac{{{{r}_{0}}}}{r}} \right)\left( {\frac{{{{\epsilon }^{2}}}}{{1 - {{r}_{{\text{g}}}}{\text{/}}r}} - 1 - \frac{{{{h}^{2}}}}{{{{r}^{2}}}}} \right).$$

(20)

On the other hand, we have

$$\frac{{d\varphi }}{{dr}} = \frac{{{{u}^{\varphi }}}}{{{{u}^{r}}}} = \frac{{{{u}_{\varphi }}{{g}^{{\varphi \varphi }}}}}{{{{u}^{r}}}} = - \frac{h}{{{{r}^{2}}{{u}^{r}}}}.$$

Substituting here (20), we obtain

$$\frac{{d\varphi }}{{dr}} = \frac{{h\sqrt {1 - {{r}_{g}}{\text{/}}r} }}{{{{r}^{2}}\sqrt {[{{\epsilon }^{2}} - (1 + {{h}^{2}}{\text{/}}{{r}^{2}})(1 - {{r}_{g}}{\text{/}}r)](1 - {{r}_{0}}{\text{/}}r)} }}.$$

(21)

The total change in the angle φ is found from this formula by integrating over the radius.

In the case of r₀ = r_g, we integrate expression (21) and obtain the total change in the angle from the time of exit from the minimal stable orbit to the gravitational radius:

$$\Delta \varphi = \int\limits_{3{{r}_{{\text{g}}}}}^{{{r}_{{\text{g}}}}} {\frac{{9hdr}}{{{{r}^{2}}{{{(3{{r}_{{\text{g}}}}{\text{/}}r - 1)}}^{{3/2}}}}}} .$$

This integral diverges at the point r = 3r_g, which corresponds to an infinite number of revolutions when leaving the minimal stable orbit for a BH.

4.2 Proper Time of Revolution along a Circular Orbit

Let us calculate the proper time of revolution of a test particle around a WH (or BH). Let us take into account that the proper time element is just an invariant element of the interval ds:

$$ds \equiv \frac{{d\varphi }}{{{{u}^{\varphi }}}} = \frac{{d\varphi }}{{h{{g}^{{\varphi \varphi }}}}}.$$

Hence, for the complete revolution δφ ≡ 2π we obtain

$$\delta s = \frac{{2\pi {{r}^{2}}}}{h}.$$

Substituting here the first expression in (19) for h, we obtain

$$\delta s = 2\pi r\sqrt {2\frac{r}{{{{r}_{{\text{g}}}}}} - 3} = 2\pi r\sqrt {2\frac{r}{{{{r}_{{\text{g}}}}}}} \sqrt {1 - \frac{3}{2}\frac{{{{r}_{{\text{g}}}}}}{r}} .$$

Here we can see that the expression for the proper time δs of revolution around a WH at infinity coincides with its Newton limit δτ:

$$\delta \tau = 2\pi r\sqrt {r{\text{/}}M} .$$

In addition, the proper time δs does not depend on the parameter r₀ for the WH; i.e., it is the same for a WH and a BH.

5 APPROXIMATE ANALYSIS OF THE EQUATIONS OF MOTION

5.1 Equation of the Trajectory of a Test Particle

We could not find an analytical form of the solution of Eq. (9); therefore, we will analyze the approximate equation. Note that this is not just a post-Newtonian approximation adopted in GR. Since the condition r_g < r₀ holds, we can also consider the approximation in the small parameter r_g/r₀. Depending on the value of this ratio, the expansion can be quite accurate. In this section, we assume that r_g ≪ r₀.

To do this, we make the change x = r₀u; then Eq. (9) takes the form

$$\begin{gathered} {{\left( {\frac{{dx}}{{d\varphi }}} \right)}^{2}} + (1 - x){{x}^{2}} \\ = {{\left( {\frac{{{{r}_{0}}}}{h}} \right)}^{2}}\left( {{{\epsilon }^{2}} - 1 + \frac{{{{r}_{{\text{g}}}}}}{{{{r}_{0}}}}x} \right)\frac{{1 - x}}{{1 - \frac{{{{r}_{{\text{g}}}}}}{{{{r}_{0}}}}x}}. \\ \end{gathered} $$

(22)

The new variable x lies in the interval 0 ≤ x ≤ 1. In our approximation, (r_g/r₀)x can be neglected compared to unity, but it cannot be neglected compared to ${{\epsilon }^{2}}$ – 1, which is itself small. Therefore, Eq. (22) simplifies to

$$\begin{gathered} {{\left( {\frac{{dx}}{{d\varphi }}} \right)}^{2}} + (1 - x){{x}^{2}} \\ = {{\left( {\frac{{{{r}_{0}}}}{h}} \right)}^{2}}\left( {{{\epsilon }^{2}} - 1 + \frac{{{{r}_{{\text{g}}}}}}{{{{r}_{0}}}}x} \right)(1 - x). \\ \end{gathered} $$

This equation can be transformed into

$${{\left( {\frac{{dx}}{{d\varphi }}} \right)}^{2}} = f(u) = (1 - x)(A + Bx - {{x}^{2}}).$$

(23)

Let us introduce the following definition

$$A \equiv {{\left( {\frac{{{{r}_{0}}}}{h}} \right)}^{2}}({{\epsilon }^{2}} - 1),$$

$$B \equiv {{\left( {\frac{{{{r}_{0}}}}{h}} \right)}^{2}}\frac{{{{r}_{{\text{g}}}}}}{{{{r}_{0}}}} = \frac{{2{{r}_{0}}}}{p}.$$

The shape of geodesics is determined by the roots of the function f(u). It is a polynomial of the third degree and hence has three roots. Obviously, the first root is x₁ = 1; the other two roots are

$$\begin{gathered} {{x}_{2}} = \frac{{{{r}_{0}}}}{p}(1 + e), \hfill \\ {{x}_{3}} = \frac{{{{r}_{0}}}}{p}(1 - e). \hfill \\ \end{gathered} $$

(24)

The first root (x₁) of the polynomial of the third degree is an orbit located at the WH throat, the second root (x₂) is the distance from the apocenter of the orbit in the Newtonian approximation, and the third root (x₃) is the distance from the pericenter. We represent the relation between the roots depending on the orbit parameter p. When p > 2r₀, the relation between the roots is x₁ > x₂ > x₃. When r₀ ≤ p ≤ 2r₀, the relation between the roots is x₂ > x₁ > x₃.

Decomposing the polynomial of the third degree into a product of linear terms, we obtain the equation

$${{\left( {\frac{{dx}}{{d\varphi }}} \right)}^{2}} = f(x) = (1 - x)({{x}_{2}} - x)(x - {{x}_{3}}).$$

(25)

The solution of this equation is an elliptic integral of the first kind.

Consider the solution for the orbit of a test particle around a WH. To this end, we make the following substitution in Eq. (23) [12]:

$$x = \frac{{{{r}_{0}}}}{p}(1 + e\cos \chi ).$$

Here χ is a new variable, which can be called the relativistic anomaly. The derivative of the function x with respect to χ is

$$\frac{{dx}}{{d\chi }} = - e\frac{{{{r}_{0}}}}{p}\sin \chi .$$

Let us introduce a new parameter, ρ = r₀/p. Then we can write the equations for the linear terms and the derivative as

$$1 - x = 1 - \rho - e\rho \cos \chi ,$$

$${{x}_{2}} - x = e\rho (1 - \cos \chi ),$$

$$x - {{x}_{3}} = e\rho (1 + \cos \chi ),$$

$${{\left( {\frac{{dx}}{{d\varphi }}} \right)}^{2}} = {{(e\rho )}^{2}}{{\sin }^{2}}\chi {{\left( {\frac{{d\chi }}{{d\varphi }}} \right)}^{2}}.$$

Now we obtain the following equation for the relativistic anomaly:

$${{\left( {\frac{{d\chi }}{{d\varphi }}} \right)}^{2}} = (1 - \rho - e\rho \cos \chi ).$$

This implies that the orbit parameter p in the case of eccentric orbits cannot be less than (1 + ecosξ)r₀. This inequality corresponds to the fact that in the case of eccentric orbits the distance from the center cannot be less than r₀:

$$r(\varphi ) > \frac{{1 + e}}{{1 + e\cos \chi }}{{r}_{0}}.$$

Let us denote α = π/2 – χ/2 and write the final equation for α:

$$\frac{{d\alpha }}{{d\varphi }} = \pm \frac{q}{2}\sqrt {1 - {{k}^{2}}{{{\sin }}^{2}}\alpha } ,$$

where

$${{k}^{2}} = \frac{{2e\rho }}{{1 + e\rho - \rho }},$$

$${{q}^{2}} = 1 + e\rho - \rho .$$

The solution of this equation is given by the elliptic integral of the first kind

$$\int\limits_0^\alpha {\frac{{d\tau }}{{\sqrt {1 - {{k}^{2}}{{{\sin }}^{2}}\tau } }} = \pm \frac{q}{2}\Delta \varphi (\alpha ),} $$

where Δφ(α) is the variation of the angular coordinate φ under the variation of the relativistic anomaly from π – 2α to π.

These solutions can be expressed in terms of Jacobi functions:

$$\sin \alpha = {\text{sn}}\left( {\frac{q}{2}\Delta \varphi (\alpha )} \right),\quad \cos \alpha = {\text{cn}}\left( {\frac{q}{2}\Delta \varphi (\alpha )} \right).$$

Accordingly, the expression for the relativistic anomaly is given by

$$\cos \chi = {\text{c}}{{{\text{n}}}^{2}}\left( {\frac{{q\varphi }}{2}} \right) - {\text{s}}{{{\text{n}}}^{2}}\left( {\frac{{q\varphi }}{2}} \right).$$

Here cn and sn are the elliptic cosine and sine, respectively.

Thus, the equation for the trajectory of a test particle in orbit around a WH has the form

$$r = \frac{p}{{1 + e\left( {{\text{c}}{{{\text{n}}}^{2}}\left( {\frac{{q\varphi }}{2}} \right) - {\text{s}}{{{\text{n}}}^{2}}\left( {\frac{{q\varphi }}{2}} \right)} \right)}}.$$

5.2 Energy of a Test Particle

Now let us derive a formula for the energy constant in the case of a test particle in orbit around a WH.

We use the first integrals of the problem of motion:

$$1 = \frac{{{{\epsilon }^{2}}}}{{1 - {{r}_{{\text{g}}}}{\text{/}}r}} - \frac{1}{{1 - {{r}_{0}}{\text{/}}r}}\frac{{d{{r}^{2}}}}{{d{{s}^{2}}}} - {{r}^{2}}\frac{{d{{\varphi }^{2}}}}{{d{{s}^{2}}}}.$$

Let us transform this equation as

$$\begin{gathered} \left( {{{\epsilon }^{2}} - 1 + \frac{{{{r}_{{\text{g}}}}}}{r}} \right)\left( {1 - \frac{{{{r}_{0}}}}{r}} \right) \\ = \left( {1 - \frac{{{{r}_{{\text{g}}}}}}{r}} \right)\frac{{d{{r}^{2}}}}{{d{{s}^{2}}}} - \left( {1 - \frac{{{{r}_{{\text{g}}}}}}{r}} \right)\left( {1 - \frac{{{{r}_{0}}}}{r}} \right){{r}^{2}}\frac{{d{{\varphi }^{2}}}}{{d{{s}^{2}}}}. \\ \end{gathered} $$

In this equation we can neglect the factor (1 – r_g/r) on the right-hand side to obtain the equation

$$\left( {{{\epsilon }^{2}} - 1 + \frac{{{{r}_{{\text{g}}}}}}{r}} \right)\left( {1 - \frac{{{{r}_{0}}}}{r}} \right) = \frac{{d{{r}^{2}}}}{{d{{s}^{2}}}} - \left( {1 - \frac{{{{r}_{0}}}}{r}} \right){{r}^{2}}\frac{{d{{\varphi }^{2}}}}{{d{{s}^{2}}}},$$

now we make the substitution

$${{r}^{2}}\frac{{d\varphi }}{{ds}} = h.$$

We also use the substitution for the trajectory, which we call, just as in [12], the relativistic ellipse in the case of e < 1,

$$\frac{1}{r} = \frac{1}{p}(1 + e\cos \chi )$$

and

$$\frac{{dr}}{{ds}} = {{r}^{2}}\frac{{e\sin \chi }}{p}\frac{{d\chi }}{{ds}},$$

as well as

$$\begin{gathered} {{\left( {\frac{{d\chi }}{{ds}}} \right)}^{2}} = (1 - \rho - e\rho \cos \chi ){{\left( {\frac{{d\varphi }}{{ds}}} \right)}^{2}} \\ = (1 - \rho - e\rho \cos \chi )\frac{{{{h}^{2}}}}{{{{r}^{4}}}}, \\ \end{gathered} $$

finally we obtain

$${{\epsilon }^{2}} - 1 + \frac{{{{r}_{{\text{g}}}}}}{p}(1 + e{\text{cos}}\chi ) = \frac{{{{h}^{2}}}}{{{{p}^{2}}}}(1 + {{e}^{2}}) + \frac{{2e{{h}^{2}}}}{{{{p}^{2}}}}{\text{cos}}\chi .$$

Equating the terms at the function cosχ, we obtain the following expressions for h(p):

$${{h}^{2}} = \frac{1}{2}{{r}_{g}}p,$$

(26)

and for $\epsilon$(e, p):

$${{\epsilon }^{2}} = 1 - \frac{{{{r}_{{\text{g}}}}}}{{2p}}(1 - {{e}^{2}}) = 1 - \frac{{GM}}{a}.$$

(27)

Here a is the semi-major axis of the relativistic ellipse. We have accepted that

$$p = a(1 - {{e}^{2}}).$$

In classical celestial mechanics [13], the quantity

$$W = - \frac{{GM}}{a}$$

is called the energy constant. In this case, W < 0 and e < 1 give an elliptic orbit, W = 0 and e = 1 give a parabolic orbit, and W > 0 and e > 1 give a hyperbolic orbit.

5.3 Analysis of the Shape of Finite Trajectories

Consider the shape of trajectories for various values of the orbit parameters (either $\epsilon$ and h or e and p) for an approximate solution. We will restrict ourselves to finite trajectories. Regardless of the properties of the WH throat (it can be either traversable or nontraversable), geodesics can always be constructed in this case.

The trajectories have simplest shape when

$$p \geqslant {{r}_{0}}(1 + e).$$

In this case, a trajectory lies completely in one fold of the space, touching the throat of the WH at a single point when p = r₀(1 + e). Finite trajectories have the shape of a relativistic ellipse; in other words, they have the shape of an almost elliptical trajectory with a shift in the pericenter of the orbit.

Let us write the inequalities that must be satisfied by the parameters of the geodesic. First of all, we give the expressions for the apocenter and pericenter of the trajectory:

$${{r}_{a}} = \frac{p}{{1 - e}},$$

$${{r}_{p}} = \frac{p}{{1 + e}}.$$

It follows from the inequality r ≥ r₀ that

$$p \geqslant {{r}_{0}}(1 + e\cos \chi ).$$

(28)

We also obtain

$$1 - \rho - e\rho \cos \chi \geqslant 0.$$

(29)

Note that inequalities (28) and (29) are equivalent.

Hence we obtain an inequality that determines the range of variation of χ:

$$\frac{{p - {{r}_{0}}}}{{e{{r}_{0}}}} \geqslant \cos \chi \geqslant - 1.$$

When

$$p = {{r}_{0}}(1 + e)$$

the angle χ lies in the interval π ≥ χ ≥ 0. For smaller values of p, the range of χ is less than π. A deficit angle arises, similar to that in the geometry of a space with a cosmic string [14]. For example, when

$$p = {{r}_{0}}\left( {1 + \frac{1}{2}e} \right)$$

the range of χ is π ≥ χ ≥ 60°.

We also assume that there are two universes: the first universe (or the upper fold of the space, or the upper universe) and the second universe (or the lower fold of the space, or the lower universe). We will also assume that the apocenter of the considered system (a trajectory or a geodesic) lies in the first universe. The position of the trajectory may be either only in the first universe, or partly in the first and partly in the second universe.

Let us consider these cases depending on the relationship between the parameters that determine the trajectories. The position of a trajectory depends on three parameters: p, r₀, and e. If p ≥ r₀(1 + e), then the trajectory lies entirely in the first universe, where it touches the WH throat at one point only when p = r₀(1 + e). Further, if p < r₀(1 + e), then a trajectory passes into the second universe. When the trajectory belongs to both universes (the first and the second), the angle χ varies in the interval

$$\frac{{p - {{r}_{0}}}}{{e{{r}_{0}}}} \geqslant \cos \chi \geqslant - 1,$$

or

$$\pi \geqslant \chi \geqslant \arccos \frac{{p - {{r}_{0}}}}{{e{{r}_{0}}}}.$$

When p < r₀, the interval is

$$\pi \geqslant \chi \geqslant \frac{\pi }{2}.$$

When r₀(1 + e) ≥ p ≥ r₀, the interval is

$$\frac{\pi }{2} \geqslant \chi \geqslant 0.$$

Numerically obtained examples of characteristic trajectories of a test particle near a WH are given in the Appendix.

6 ESTIMATES OF THE PERICENTER SHIFT

It is well known that the shift of the perihelion of Mercury was the first test of GR. There is a significant discrepancy between the predictions of the Newtonian theory of gravity and the observed shift of the perihelion. It amounts to approximately 43″ for 100 years.

Since then the shift of the pericenter of various relativistic objects in binary systems has become one of the most powerful tests in the study of binary star systems. In particular, after the discovery of the first binary pulsar PSR 1913 + 16, this test made it possible to accurately measure the masses of the components of a binary system.

To calculate the pericenter shift, we integrate Eq. (17):

$$\int\limits_{ - \pi }^\pi {\frac{{d\chi }}{{\sqrt {1 - \rho - e\rho \cos \chi } }} = \Delta \varphi .} $$

In the case of ρ ≪ 1, the integral has a simple form:

$$\int\limits_{ - \pi }^\pi {d\chi \left( {1 + \frac{\rho }{2} + \frac{{e\rho }}{2}\cos \chi } \right) = \Delta \varphi .} $$

The shift of the angular coordinate φ during a complete rotation along the relativistic anomaly –π ≤ χ ≤ π (here we count the relativistic anomaly from the apocenter) is

$$\Delta \varphi = 2\pi + \frac{{\pi {{r}_{0}}}}{p}.$$

Note that the relationship between the period of a test particle in orbit around a WH and its semi-major axis is determined by the gravitational radius of the WH, while the shift of the orbit pericenter is determined by the radius of the WH throat. In the case under consideration, when r₀ ≫ r_g, the shift of the pericenter can significantly exceed the value predicted by GR for a BH. This can serve as a criterion for distinguishing between WH and BH in astronomical observations.

If the throat radius is greater than three gravitational radii (r₀ > 3r_g,) and all circular orbits around a WH are stable, the shift of the pericenter of the test particle in orbit around the WH exceeds the shift of the pericenter of this particle in orbit around a BH with the same gravitational radius.

7 CONCLUSIONS

Due to the increasing accuracy of observations and the new observational channel—gravitational-wave astronomy—differences in the motion of matter near BHs and WHs can become discernible.

In future searches for observational effects that distinguish WHs, it is necessary to know the shapes of the characteristic trajectories of bodies (test particles) near WHs. In the present work, we have derived the equations of motion of a test particle in the WH metric and considered the most interesting properties of these motions. We have derived a general equation for geodesics in the WH metric and considered some properties of these geodesics. We have analyzed the exact solution for circular orbits of test particles around a WH and an approximate analytical solution of the geodesic equations. We have considered the shift of the pericenter of the orbit of a test particle in the WH field and discussed possible observational consequences. We have also presented examples of trajectories, obtained by numerical simulation, for test particles near a WH.

Appendices

APPENDIX

Simulation of Finite Trajectories

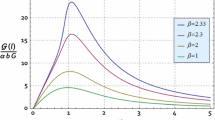
Consider the shape of trajectories for various values of the orbit parameters $\epsilon$ and h or e and p (see (26) and (27)).

The trajectories have the simplest shape when

$$p \geqslant {{r}_{0}}.$$

In this case, a trajectory lies completely in one fold of the space, touching the throat of the WH at a single point; the case when the parameter p = r₀ is illustrated in Fig. 1. In the finite case, the trajectories have the shape of a relativistic ellipse; in other words, they have the shape of an almost elliptical trajectory with a shift of the pericenter of the orbit.

Fig. 1.

The geodesic starts at the green dot, reaches the WH throat, passes to the second fold of the space (the second universe), and ends at the black dot. For such a trajectory, the angular momentum is h = 0; i.e., the test particle moves only along the radius. The total energy is $\epsilon$ ≈ 0.949, and the minimum distance from the center of the WH is r_min = r₀. The trajectory in the first universe is superimposed on the trajectory in the second universe; therefore, in the r–ϕ projection, the two parts of the trajectory merge.

Let us give several examples of finite trajectories near a WH (Figs. 2–7). All the pictures are drawn in the coordinates r and ϕ, except for Fig. 4, which presents a qualitative view of the trajectory when the line of sight lies in the plane of the WH throat. The trajectory parameters are given in units of the gravitational radius (r_g) of the WH. The maximum distance (apocenter) of the trajectory from the WH center is r_max = 10r_g for all trajectories. The black lines denote the BH horizon, and the purple lines show the position of the WH throat. The green dot is the starting point of the geodesic, and the black dot is the endpoint of the geodesic. The green dot corresponds to ϕ = 0.

Fig. 2.

The geodesic starts at the green dot and moves in the first universe. This section of the geodesic is indicated by the red line. It reaches the throat and passes through it. Then the geodesic moves in the second universe, which is indicated by the blue line, returns to the throat, passes through it again, and returns to the first universe (red line). The geodesic stops at the black point. The angular momentum is h = 0.1r_g, and the total energy is $\epsilon$ ≈ 0.949. The minimum distance from the WH center is r_min = r₀.

Fig. 3.

This trajectory is of most interest. It starts, as before, at the green point, reaches the throat, enters the second universe, makes half a revolution, returns to the throat, passes through it, and ends at the black point. A small gap in the trajectory corresponds to the shift of the apocenter. The angular momentum is h = r_g, the total energy is $\epsilon$ ≈ 0.953, and the minimum distance from the WH center is r_min = r₀.

Fig. 4.

The trajectory shown in Fig. 3 is represented in an artificial virtual projection that graphically separates the upper and lower folds of the space.

Fig. 5.

A trajectory similar to those shown in Figs. 1 and 2. The angular momentum is h = 1.9r_g, the total energy is $\epsilon$ ≈ 0.966, and the minimum distance from the WH center is r_min = r₀.

Fig. 6.

A trajectory similar to those shown in Figs. 1 and 2. The angular momentum is h = 2r_g, the total energy is $\epsilon$ ≈ 0.973, and the minimum distance from the WH center is r_min = r₀.

Fig. 7.

A trajectory similar to those shown in Figs. 1 and 2. The angular momentum is h = 2.3r_g, the total energy is $\epsilon$ ≈ 0.973, and the minimum distance from the WH center is r_min = r₀.