Optimal Control for Systems Modeled by the Diffusion-Wave Equation

Abstract

The article deals with an optimal control problem for the model system described by the one-dimensional inhomogeneous diffusion-wave equation that is a generalization of the wave equation to the case when the time derivative is replaced with the fractional Caputo derivative. In the general case, we consider both boundary and distributed controls which are Lebesgue $p$-summable functions, with $p>1$ and $p=\infty$. We state and study the two types of optimal control problems: The problem of finding a minimal norm control for a given control time and the performance problem of finding a control that brings the system to a given state in the minimal time for a given constraint on the control norm. The study bases on using an exact solution to the diffusion-wave equation, which allows us to reduce the optimal control problem to an infinite-dimensional $l$-moment problem. We also examine the similar finite-dimensional $l$-moment problem that uses an approximate solution to the diffusion-wave equation and analyze the well-posedness and solvability of this problem. Also, we exhibit some example of calculating the boundary control by using the finite-dimensional $l$-moment problem.

Introduction

The fractional order models of systems with distributed parameters as well as the questions of optimal control for these systems are topical. These models describe the processes of heat and mass transfer, diffusion in inhomogeneous media, diffusion-wave phenomena in complex multicomponent systems, oscillations in inhomogeneous media, and so on. Previously, optimal control problems for these systems were studied using variational methods with constraints on some quadratic functional presenting the sum of the system state and control (see, for instance, [1,2,3]).

In the present article, we study an optimal control problem with a constraint on the norm of a control for an inhomogeneous linear diffusion-wave equation. We consider the distributed and boundary control that are Lebesgue $p$-summable functions on an interval with $p>1$. The study of the optimal control problem is carried out by the method of moments.

1. Statement of the Problem

We consider the system whose state is described by the equation

$$ r(x)\,^{C}_{0}\!D_{t}^{\alpha}Q(x,t)=\frac{\partial}{\partial x}\left[w(x)\,\frac{\partial Q(x,t)}{\partial x}\right]-q(x)Q(x,t)+f(x,t)+u(x,t), $$

(1)

where $Q(x,t)$ is the state of the system, ${}^{C}_{0}\!D_{t}^{\alpha}$ is the left-sided operator of fractional time differentiation, where $\alpha\in(1,2)$, $t\geq 0$, $x\in[0,L]$, and $(x,t)\in\Omega=[0,L]\times[0,\infty)$. The fractional differentiation is understood in the Caputo sense [4, Section 2.4], i.e.,

$$ {}^{C}_{0}\!D_{t}^{\alpha}Q(x,t)={}^{RL}_{0}\!D_{t}^{\alpha}\Bigg{[}Q(x,t)-\sum_{k=0}^{[\alpha]}\frac{\partial^{k}Q(x,0+)}{\partial t^{k}}\,\frac{t^{k}}{k!}\Bigg{]}, $$

(2)

where ${}^{RL}_{0}\!D_{t}^{\alpha}$ is the left-sided operator of the fractional Riemann–Liouville differentiation and

$$ {}^{RL}_{0}\!D_{t}^{\alpha}Q(x,t)=\frac{1}{\Gamma(1-\{\alpha\})}\,\frac{\partial^{[\alpha]+1}}{\partial\tau^{[\alpha]+1}}\int\limits_{0}^{t}\frac{Q(x,\tau)\,d\tau}{(t-\tau)^{\{\alpha\}}}, $$

with $[\alpha]$ and $\{\alpha\}$ the respective integer and fractional parts of $\alpha$.

It is assumed that the function $Q(x,t)$ is time differentiable for $t\geq 0$ and twice space differentiable on $[0,L]$. The functions $r(x)>0$, $w(x)>0$, and $q(x)$ are continuous on $[0,L]$. A perturbation $f(x,t)$ is summable in both variables in $\Omega$. We also assume that a distributed control $u(x,t)$ belongs to the $L_{p_{1},p_{2}}(\Omega)$ space, with $p_{1,2}>1$.

Equation (1) is called a diffusion-wave equation.

The initial conditions for (1) are given in the form

$$ \frac{\partial^{k}Q(x,0+)}{\partial t^{k}}=\varphi^{k}(x),\quad x\in[0,L],\ k=0,1. $$

(3)

The boundary conditions for (1) are written as

$$ \left[b_{i}\,\frac{\partial Q(x,t)}{\partial x}+a_{i}Q(x,t)\right]_{x=x^{i}}=h_{i}(t)+u^{i}(t),\quad t\geq 0,\ i=1,2, $$

(4)

where $a_{i}$ and $b_{i}$ are coefficients, with $b_{1}\leq 0$ and $b_{2}\geq 0$, while $h_{i}(t)$ are some known functions, and ${x^{1}=0}$, $x^{2}=L$. The boundary controls $u^{1,2}(t)$ are assumed to be in $L_{p}[0,T]$, with $p>1$, and can be written as the vector $U(t)=(u^{1}(t),u^{2}(t))$.

The goal of an optimal control is to achieve a given state $Q^{*}(x)$ at a given time $T>0$ by a system

$$ Q(x,T)=Q^{*}(x),\quad T>0,\ x\in[0,L]. $$

(5)

The optimal control problem is stated in two forms as in [5]. To find controls $u(x,t)$ and/or $U(t)$ such that the system described by (1) with the initial conditions (3) and boundary conditions (4) achieves (5) at $t=T$ and the conditions hold:

$\bullet$ the norm of the controls $u(x,t)$ and/or $U(t)$ is minimal for a given $T$ (Problem A);

$\bullet$ the transition time at a given state (5) is minimal under given constraints on the norm of the controls $\|u(x,t)\|\leq l$ and $\|U(t)\|\leq l$ (with $l>0$ a given real) (Problem B).

2. Representation of an Optimal Control Problem in the Form of the Generalized $l$-Moment Problem

The exact solution is known to (1) with initial conditions (3) and boundary conditions (4) (see [6, formula (18)]). Write down the solution of (5) at $t=T$ as follows:

$$ \begin{gathered}\displaystyle Q(x,T)=Q^{*}(x)=R(x,T)+\sum_{n=1}^{\infty}X_{n}(x)\int\limits_{0}^{T}u_{n}(t)\,\frac{E_{\alpha,\alpha}[-\lambda_{n}(T-t)^{\alpha}]}{(T-t)^{1-\alpha}}\,dt+\sum_{n=1}^{\infty}X_{n}(x)\\ \displaystyle\times\int\limits_{0}^{T}dt\,\frac{E_{\alpha,\alpha}[-\lambda_{n}(T-t)^{\alpha}]}{(T-t)^{1-\alpha}}\left[\left(v^{\prime}_{1}w^{\prime}_{n}-(q(x)v_{1}(x))_{n}\right)u^{1}(t)+\left(v^{\prime}_{2}w^{\prime}_{n}-(q(x)v_{2}(x))_{n}\right)u^{2}(t)\right]\\ \displaystyle-\sum_{n=1}^{\infty}X_{n}(x)\!\int\limits_{0}^{T}\!\frac{E_{\alpha,\alpha}[-\lambda_{n}(T\!-\!t)^{\alpha}]\,[(r(x)v_{1}(x))_{n}\cdot{}^{C}_{0}\!D_{t}^{\alpha}u^{1}(t)+(r(x)v_{2}(x))_{n}\cdot{}^{C}_{0}\!D_{t}^{\alpha}u^{2}(t)]\,dt}{(T-t)^{1-\alpha}},\end{gathered} $$

(6)

where

$$ v_{1}(x)=\frac{a_{2}(x-L)-b_{2}}{a_{2}b_{1}-a_{1}b_{2}-a_{1}a_{2}L};\quad v_{2}(x)=\frac{b_{1}-a_{1}x}{a_{2}b_{1}-a_{1}b_{2}-a_{1}a_{2}L}; $$

$$ v^{\prime}_{1}=\frac{dv_{1}(x)}{dx}=\frac{a_{2}}{a_{2}b_{1}-a_{1}b_{2}-a_{1}a_{2}L};\quad v^{\prime}_{2}=\frac{dv_{2}(x)}{dx}=-\frac{a_{1}}{a_{2}b_{1}-a_{1}b_{2}-a_{1}a_{2}L}; $$

$$ \begin{gathered}\displaystyle R(x,T)=v_{1}(x)u^{1}(T)+v_{2}(x)u^{2}(T)+V(x,T)\\ \displaystyle+\sum\limits_{n=1}^{\infty}E_{\alpha}(-\lambda_{n}T^{\alpha})\left[\varphi^{0}_{n}-V_{n}(0+)-v_{1n}u^{1}(0+)-v_{2n}u^{2}(0+)\right]X_{n}(x)\\ \displaystyle+\,T\sum\limits_{n=1}^{\infty}E_{\alpha,2}(-\lambda_{n}T^{\alpha})X_{n}(x)\Bigg{[}\varphi^{1}_{n}-\left(\frac{\partial V(x,0+)}{\partial t}\right)_{n}-v_{1n}\left(\frac{\partial u^{1}(0+)}{\partial t}\right)_{n}\\ \displaystyle-\,v_{2n}\left(\frac{\partial u^{2}(0+)}{\partial t}\right)_{n}\Bigg{]}+\sum\limits_{n=1}^{\infty}X_{n}(x)\int\limits_{0}^{T}dt\,\frac{E_{\alpha,\alpha}[-\lambda_{n}(T-t)^{\alpha}]}{(T-t)^{1-\alpha}}\\ \displaystyle\times\,\left[f_{n}(t)+w^{\prime}_{n}\left(\frac{\partial V(x,t)}{\partial x}\right)_{n}-(q(x)V(x,t))_{n}-\left(r(x)\,{}^{C}_{0}\!D_{t}^{\alpha}V(x,t)\right)_{n}\right];\end{gathered} $$

where $\varphi^{0,1}_{n}$, $u_{n}(t)$, $f_{n}(t)$, $V_{n}(t)$, and $v_{(1,2)n}$ are coefficients of the expansion of $\varphi^{0,1}(x)$, $u(x,t)$, $f(x,t)$, $V(x,t)$, and $v_{1,2}(x)$ in the system of eigenfunctions $\{X_{n}(x)\}$; similarly, $(\,\dots)_{n}$ is the coefficient of the expansion of an expression in the brackets in the system of eigenfunctions $\{X_{n}(x)\}$; $V(x,t)=v_{1}(x)h_{1}(t)+v_{2}(x)h_{2}(t)$; $E_{\alpha,\beta}(t)$ is the two-parameter Mittag-Leffler function; and $E_{\alpha}(t)=E_{\alpha,1}(t)$. The eigenvalues $\lambda_{n}$ and eigenfunctions $X_{n}(x)$ are solutions to the Sturm–Liouville problem (see [6]):

$$ \left[\frac{\partial}{\partial x}\left(w(x)\frac{\partial}{\partial x}\right)-q(x)\right]X(x)+\lambda r(x)X(x)=0, $$

$$ \left[b_{i}\,\frac{\partial X(x)}{\partial x}+a_{i}X(x)\right]_{x=x^{i}}=0,\quad i=1,2. $$

State the $l$-moment problem [5]. A system of functions $g_{n}(t)\in L_{p^{\prime}}[0,T]$ and a collection of reals $c_{n}$ with at least one nonzero member are given as well as some $l>0$. We have to find $W(t)\in L_{p}(0,T]$ ($1/p+1/p^{\prime}=1$) such that

$$ \int\limits_{0}^{T}g_{n}(\tau)W(\tau)d\tau=c_{n},\quad n=1,2,\dots, $$

(7)

$$ \|W(t)\|\leq l. $$

(8)

Choose $W(t)$ and $g_{n}(t)$ in the form

$$ \begin{gathered}\displaystyle W(t)=u_{n}(t)+\left(v^{\prime}_{1}w^{\prime}_{n}-(q(x)v_{1}(x))_{n}+\lambda_{n}(r(x)v_{1}(x))_{n}\right)u^{1}(t)\\ \displaystyle+\,\left(v^{\prime}_{2}w^{\prime}_{n}-(q(x)v_{2}(x))_{n}+\lambda_{n}(r(x)v_{2}(x))_{n}\right)u^{2}(t),\end{gathered} $$

(9)

$$ g_{n}(t,T)=\frac{E_{\alpha,\alpha}[-\lambda_{n}(T-t)^{\alpha}]}{(T-t)^{1-\alpha}}. $$

(10)

The reals $c_{n}$ are chosen as

$$ \begin{gathered}\displaystyle c_{n}(T)\!=\!Q^{*}_{n}\!\!-\!V_{n}(T)\!-\!\left[\varphi^{0}_{n}\!-\!V_{n}(0+)\right]\!E_{\alpha}(-\lambda_{n}T^{\alpha})\!-\!\Bigg{[}\!\varphi^{1}_{n}\!-\!\left(\frac{\partial V(x,0+)}{\partial t}\right)_{n}\!\Bigg{]}TE_{\alpha,2}(-\lambda_{n}T^{\alpha})\\ \displaystyle-\int\limits_{0}^{T}g_{n}(t,T)\left[f_{n}(t)+w^{\prime}_{n}\left(\frac{\partial V(x,t)}{\partial x}\right)_{n}-(q(x)V(x,t))_{n}-\left(r(x)\,{}^{C}_{0}\!D_{t}^{\alpha}V(x,t)\right)_{n}\right]dt\\ \displaystyle+\,\big{[}(v_{1}(x)r(x))_{n}-v_{1n}\big{]}u^{1}(T)+\big{[}(v_{2}(x)r(x))_{n}-v_{2n}\big{]}u^{2}(T)\\ \displaystyle-\,\left(\big{[}(v_{1}(x)r(x))_{n}-v_{1n}\big{]}u^{1}(0+)+\big{[}(v_{2}(x)r(x))_{n}-v_{2n}\big{]}u^{2}(0+)\right)E_{\alpha}(-\lambda_{n}T^{\alpha})\\ \displaystyle-\,\left(\big{[}(v_{1}(x)r(x))_{n}-v_{1n}\big{]}\,\frac{\partial u^{1}(0+)}{\partial t}+\big{[}(v_{2}(x)r(x))_{n}-v_{2n}\big{]}\,\frac{\partial u^{2}(0+)}{\partial t}\right)TE_{\alpha,2}(-\lambda_{n}T^{\alpha}).\end{gathered} $$

(11)

Theorem 1

Assume that $W(t)$ in (9) is pointwise discontinuous and has at most countably many discontinuity points and continuity intervals. Moreover, suppose that $\alpha\in(1,2)$, the expression on the right-hand side of (11) is defined and bounded and does not vanish at least for one value of $n$. Then (6) is equivalent to (7), whenever (9)–(11) are taken into account.

Proof

Expand $Q^{*}(x)$ and $R(x,T)$ in the system of eigenfunctions $\{X_{n}(x)\}$ and insert the result in (6). Since the system $\{X_{n}(x)\}$ is complete by definition; therefore, (6) is equivalent to the corresponding collection of expressions for coefficients of the expansion for every $n$.

Consider the integral in (6) containing the fractional derivatives of boundary controls (which is a weighted sum of moments of the fractional derivatives of boundary controls relative $g_{n}(t)$). The formula of fractional integration by parts [7] and necessary calculations yield

$$ \begin{gathered}\displaystyle\int\limits_{0}^{T}g_{n}(t,T)\left[(r(x)v_{1}(x))_{n}\cdot{}^{C}_{0}\!D_{t}^{\alpha}u^{1}(t)+(r(x)v_{2}(x))_{n}\cdot{}^{C}_{0}\!D_{t}^{\alpha}u^{2}(t)\right]dt\\ \displaystyle=\int\limits_{0}^{T}\left[(r(x)v_{1}(x))_{n}u^{1}(t)+(r(x)v_{2}(x))_{n}u^{2}(t)\right]\cdot{}^{RL}_{t}\!D_{T}^{\alpha}g_{n}(t,T)\,dt\\ \displaystyle+\,\bigg{[}\bigg{[}(r(x)v_{1}(x))_{n}\,\frac{\partial u^{1}(t)}{\partial t}+(r(x)v_{2}(x))_{n}\,\frac{\partial u^{2}(t)}{\partial t}\bigg{]}\cdot{}^{RL}_{t}\!I_{T}^{1-\{\alpha\}}g_{n}(t,T)\bigg{]}\Bigg{|}_{0}^{T}\\ \displaystyle+\bigg{[}\bigg{[}(r(x)v_{1}(x))_{n}u^{1}(t)+(r(x)v_{2}(x))_{n}u^{2}(t)\bigg{]}\cdot{}^{RL}_{t}\!D_{T}^{\{\alpha\}}g_{n}(t,T)\bigg{]}\Bigg{|}_{0}^{T},\end{gathered} $$

(12)

where ${}^{RL}_{t}\!D_{T}^{\sigma}$ and ${}^{RL}_{t}\!I_{T}^{\sigma}$ are the respective right-sided operators of the fractional differentiation and integration of order $\sigma$ in the Riemann–Liouville sense.

The definitions of the right-sided Riemann–Liouville operators [4] and (10) imply that

$$ {}^{RL}_{t}\!D_{T}^{\alpha}g_{n}(t,T)=\frac{1}{\Gamma(1-\{\alpha\})}\,\frac{\partial^{2}}{\partial t^{2}}\int\limits_{t}^{T}(\tau-t)^{-\{\alpha\}}(T-\tau)^{\alpha-1}E_{\alpha,\alpha}\big{[}-\lambda_{n}(T-\tau)^{\alpha}\big{]}d\tau, $$

(13)

$$ {}^{RL}_{t}\!D_{T}^{\{\alpha\}}g_{n}(t,T)=-\frac{1}{\Gamma(1-\{\alpha\})}\,\frac{\partial}{\partial t}\int\limits_{t}^{T}(\tau-t)^{-\{\alpha\}}(T-\tau)^{\alpha-1}E_{\alpha,\alpha}\big{[}-\lambda_{n}(T-\tau)^{\alpha}\big{]}d\tau, $$

(14)

$$ {}^{RL}_{t}\!I_{T}^{1-\{\alpha\}}g_{n}(t,T)=\frac{1}{\Gamma(1-\{\alpha\})}\int\limits_{t}^{T}(\tau-t)^{-\{\alpha\}}(T-\tau)^{\alpha-1}E_{\alpha,\alpha}\big{[}-\lambda_{n}(T-\tau)^{\alpha}\big{]}d\tau. $$

(15)

The integral on the right-hand side of (13)–(15) can be calculated on using the representation of the Mittag-Leffler function in the form of a power series [4]:

$$ E_{\alpha,\beta}(t)=\sum_{k=0}^{\infty}\frac{t^{k}}{\Gamma(\alpha k+\beta)}. $$

(16)

Series (16) converges uniformly on the real axis and thereby we can change the order of summation and integration while calculating the integrals on the right-hand side of the expressions (13)–(15). Finally,

$$ \begin{gathered}\displaystyle K_{\alpha}(T,t)=\int\limits_{t}^{T}(\tau-t)^{-\{\alpha\}}(T-\tau)^{\alpha-1}E_{\alpha,\alpha}\big{[}-\lambda_{n}(T-\tau)^{\alpha}\big{]}\,d\tau\\ \displaystyle=\sum_{k=0}^{\infty}\frac{(-\lambda_{n})^{k}}{\Gamma[\alpha(k+1)]}\int\limits_{t}^{T}(\tau-t)^{-\{\alpha\}}(T-\tau)^{\alpha(k+1)-1}\,d\tau\\ \displaystyle=\sum_{k=0}^{\infty}\frac{(-\lambda_{n})^{k}}{\Gamma[\alpha(k+1)]}\,(T-t)^{\alpha k+[\alpha]}B(1-\{\alpha\},\alpha(k+1))\\ \displaystyle=\Gamma(1-\{\alpha\})(T-t)^{[\alpha]}E_{\alpha,1+[\alpha]}\big{[}-\lambda_{n}(T-t)^{\alpha}\big{]},\end{gathered} $$

(17)

where $B(\alpha,\beta)$ is the Euler beta function $B(\alpha,\beta)=\frac{\Gamma(\alpha)\Gamma(\beta)}{\Gamma(\alpha+\beta)}$.

In our case $\alpha\in(1,2)$, i.e., $[\alpha]=1$. Using (15), we infer from (17) that

$$ \begin{gathered}\displaystyle\left[\left[(r(x)v_{1}(x))_{n}\,\frac{\partial u^{1}(t)}{\partial t}+(r(x)v_{2}(x))_{n}\,\frac{\partial u^{2}(t)}{\partial t}\right]\cdot{}^{RL}_{t}\!I_{T}^{1-\{\alpha\}}g_{n}(t,T)\right]\Bigg{|}_{0}^{T}\\ \displaystyle=-TE_{\alpha,2}\big{[}-\lambda_{n}T^{\alpha}\big{]}\left[(r(x)v_{1}(x))_{n}\,\frac{\partial u^{1}(0+)}{\partial t}+(r(x)v_{2}(x))_{n}\,\frac{\partial u^{2}(0+)}{\partial t}\right].\end{gathered} $$

(18)

Calculate the first and second derivatives of (17) on using (16). The first derivative is written as

$$ \begin{gathered}\displaystyle-\frac{\partial}{\partial t}\,K_{\alpha}(T,t)=-\frac{\partial}{\partial t}\,\Gamma(1-\{\alpha\})(T-t)^{[\alpha]}\,E_{\alpha,1+[\alpha]}\big{[}-\lambda_{n}(T-t)^{\alpha}\big{]}\\ \displaystyle=\Gamma(1-\{\alpha\})\sum_{k=0}^{\infty}\frac{(-\lambda_{n})^{k}}{\Gamma[\alpha k+1+[\alpha]]}\,(\alpha k+[\alpha])(T-t)^{\alpha k+[\alpha]-1}\\ \displaystyle=\Gamma(1-\{\alpha\})(T-t)^{[\alpha]-1}\sum_{k=0}^{\infty}\frac{(-\lambda_{n})^{k}}{\Gamma[\alpha k+[\alpha]]}\,(T-t)^{\alpha k}\\ \displaystyle=\Gamma(1-\{\alpha\})(T-t)^{[\alpha]-1}E_{\alpha,[\alpha]}\big{[}-\lambda_{n}(T-t)^{\alpha}\big{]}.\end{gathered} $$

Using (14) and the equality $[\alpha]=1$, we infer that

$$ {}^{RL}_{t}\!D_{T}^{\{\alpha\}}g_{n}(t,T)=E_{\alpha}\big{[}-\lambda_{n}(T-t)^{\alpha}\big{]}. $$

Hence,

$$ \begin{gathered}\displaystyle\Big{[}\big{[}(r(x)v_{1}(x))_{n}u^{1}(t)+(r(x)v_{2}(x))_{n}u^{2}(t)\big{]}\cdot{}^{RL}_{t}\!D_{T}^{\{\alpha\}}g_{n}(t,T)\Big{]}\Big{|}_{0}^{T}\\ \displaystyle=(r(x)v_{1}(x))_{n}u^{1}(T)+(r(x)v_{2}(x))_{n}u^{2}(T)\\ \displaystyle-\left[(r(x)v_{1}(x))_{n}u^{1}(0+)+(r(x)v_{2}(x))_{n}u^{2}(0+)\right]E_{\alpha}\big{[}-\lambda_{n}T^{\alpha}\big{]}.\end{gathered} $$

(19)

Similar arguments justify the following formula for the second derivative of (17):

$$ \begin{gathered}\displaystyle\frac{\partial^{2}}{\partial t^{2}}\,K_{\alpha}(T,t)=\frac{\partial^{2}}{\partial t^{2}}\,\Gamma(1-\{\alpha\})(T-t)^{[\alpha]}E_{\alpha,1+[\alpha]}\big{[}-\lambda_{n}(T-t)^{\alpha}\big{]}\\ \displaystyle=\Gamma(1-\{\alpha\})\sum_{k=0}^{\infty}\frac{(-\lambda_{n})^{k}}{\Gamma[\alpha k+1+[\alpha]]}\,(\alpha k+[\alpha])(\alpha k+[\alpha]-1)(T-t)^{\alpha k+[\alpha]-2}\\ \displaystyle=\Gamma(1-\{\alpha\})(T-t)^{[\alpha]-2}\sum_{k=0}^{\infty}\frac{(-\lambda_{n})^{k}(\alpha k+[\alpha]-1)}{\Gamma[\alpha k+[\alpha]]}\,(T-t)^{\alpha k}\\ \displaystyle=\Gamma(1-\{\alpha\})(T-t)^{[\alpha]-2}\left[\frac{[\alpha]-1}{\Gamma([\alpha])}+\sum_{k=1}^{\infty}\frac{(-\lambda_{n})^{k}(\alpha k+[\alpha]-1)}{\Gamma[\alpha k+[\alpha]]}\,(T-t)^{\alpha k}\right].\end{gathered} $$

After the change $k=m+1$ of the index, we derive that

$$ \begin{gathered}\displaystyle\frac{\partial^{2}}{\partial t^{2}}\,K_{\alpha}(T,t)=\Gamma(1-\{\alpha\})(T-t)^{[\alpha]-2}\bigg{[}\frac{[\alpha]-1}{\Gamma([\alpha])}+\sum_{m=0}^{\infty}\frac{\left[-\lambda_{n}(T-t)^{\alpha}\right]^{m+1}}{\Gamma[\alpha(m+1)+[\alpha]-1]}\bigg{]}\\ \displaystyle=\Gamma(1-\{\alpha\})(T-t)^{[\alpha]-2}\left[\frac{[\alpha]-1}{\Gamma([\alpha])}-\lambda_{n}(T-t)^{\alpha}E_{\alpha,[\alpha]+\alpha-1}\big{[}-\lambda_{n}(T-t)^{\alpha}\big{]}\right].\end{gathered} $$

Inserting this expression into (13) and accounting for (10) and the equality $[\alpha]=1$, we conclude that

$$ {}^{RL}_{t}\!D_{T}^{\alpha}g_{n}(t,T)=-\lambda_{n}g_{n}(t,T). $$

(20)

Inserting (18)–(20) into the right-hand side of (12), we find that

$$ \begin{gathered}\displaystyle\int\limits_{0}^{T}g_{n}(t,T)\left[(r(x)v_{1}(x))_{n}\cdot{}^{C}_{0}\!D_{t}^{\alpha}u^{1}(t)+(r(x)v_{2}(x))_{n}\cdot{}^{C}_{0}\!D_{t}^{\alpha}u^{2}(t)\right]dt\\ \displaystyle=-\lambda_{n}\int\limits_{0}^{T}\left[(r(x)v_{1}(x))_{n}u^{1}(t)+(r(x)v_{2}(x))_{n}u^{2}(t)\right]g_{n}(t,T)\,dt-TE_{\alpha,2}\big{[}-\lambda_{n}(T-t)^{\alpha}\big{]}\\ \displaystyle\times\left[(r(x)v_{1}(x))_{n}\,\frac{\partial u^{1}(0+)}{\partial t}+(r(x)v_{2}(x))_{n}\,\frac{\partial u^{2}(0+)}{\partial t}\right]\\ \displaystyle+(r(x)v_{1}(x))_{n}u^{1}(T)+(r(x)v_{2}(x))_{n}u^{2}(T)\\ \displaystyle-\left[(r(x)v_{1}(x))_{n}u^{1}(0+)+(r(x)v_{2}(x))_{n}u^{2}(0+)\right]E_{\alpha}\big{[}-\lambda_{n}T^{\alpha}\big{]}.\end{gathered} $$

Inserting the above expression in (6) and accounting for (9), (11) and (10), we justify the desired expression (7).  ☐

Corollary 1

The optimal control problem considered in Section 1 for given $l$ and $T$ is equivalent to the one-dimensional countable $l$-moment problem (7)–(8) for (9), moments (11), and functions (10).

Remark 1

Expressions (11) contain the initial and final values of boundary controls and their derivatives. In the general case these values can be defined from some additional conditions or assumptions. For $r(x)=1$, the coefficients of these values vanish and we need not involve any additional information.

3. The Finite-Dimensional $l$-Moment Problem

As was shown in the previous section, the optimal control problem under consideration can be reduced to a countable $l$-moment problem. However, for this problem there are no lucid criteria that allow us to establish its solvability and find some algorithms for constructing a solution. For the finite-dimensional $l$-moment problem, such criteria algorithms exist [5]; and, thereby, it is reasonable to consider them. The finite-dimensional $l$-moment problem (7)–(8) can be obtained by analogy with the countable problem. Instead of the exact solution (6), we use an approximate one obtained by truncating the series in the formula. In this case a solution (6) in which the series is replaced with a partial sum contains not a finite rather than countable set of $N$ equalities for the expansion coefficients. This set can be rewritten as a finite-dimensional moment problem which in this case can be called an $\{l,N\}$-moment problem.

We say that a finite-dimensional $\{l,N\}$-moment problem is well-posed if the norms of the functions $g_{n}(t)$ in $L_{p^{\prime}}[0,T]$ are defined.

As is known, a finite-dimensional $l$-moment problem is solvable if $g_{n}(t)\in L_{p^{\prime}}[0,T]$ are linearly independent or have a subsystem of linearly independent functions among them [5].

Theorem 2

Problem (7)–(8) with (9)–(11) is well-posed and solvable for every fixed $N$ and every given $l>0$.

Proof

Estimate in $L_{p^{\prime}}[0,T]$ the norms of $g_{n}(t)$ given by (10) as follows:

$$ \big{\|}{\tilde{g}}_{n}(t,T)\big{\|}\leq\big{\|}E_{\alpha,\alpha}\big{[}-\lambda_{n}\left(T-\tau\right)^{\alpha}\big{]}\big{\|}\cdot\big{\|}(T-t)^{\alpha-1}\big{\|},\quad n=1,\dots,N. $$

The first factor on the right-hand side is bounded [4, p. 42]. The latter can be calculated explicitly for $p^{\prime}\geq 1$ and $\alpha\in(1,2)$, which yields

$$ \big{\|}(T-t)^{\alpha-1}\big{\|}=\frac{(T-t)^{[p^{\prime}(\alpha-1)+1]/p^{\prime}}}{[p^{\prime}(\alpha-1)+1]^{1/p^{\prime}}}\,\Bigg{|}_{0}^{T},\quad t\in[0,T]. $$

This norm is bounded for the real $T>0$, $p^{\prime}\geq 1$, and $\alpha\in(1,2)$. Hence, the $\{l,N\}$-moment problem is well-posed.

The functions, defined by (10), are linearly independent, which can be verified directly. Therefore, our problem of moments is solvable.  ☐

As is known, the finite-dimensional $l$-moment problem (which is well-posed and solvable) is equivalent the conditional optimization problem [5]: Find

$$ \min_{\xi_{1},\dots,\xi_{N}}\left(\int\limits_{0}^{T}\left|\sum_{i=1}^{N}\xi_{i}g_{i}(t)\right|^{p^{\prime}}dt\right)^{1/{p^{\prime}}}=\left(\int\limits_{0}^{T}\left|\sum_{i=1}^{N}\xi_{i}^{*}g_{i}(t)\right|^{p^{\prime}}dt\right)^{1/{p^{\prime}}} $$

(21)

satisfying

$$ \sum_{i=1}^{N}\xi_{i}c_{i}=\sum_{i=1}^{N}\xi_{i}^{*}c_{i}=1, $$

(22)

where $\xi_{i}$ are arbitrary reals, and the reals $\xi^{*}_{i}$ ($i=1,\dots,N$) correspond to a solution of the problem.

Employing a solution to problem (21)–(22), we can construct a solution to a finite-dimensional $l$-moment problem and thereby to the optimal control problem [5]. This solution for $W(t)\in L_{p}[0,T]$, $p>1$ is unique. In the case of Problem A, the function ${\widetilde{W}}(t)$, presenting a unique solution to the finite-dimensional $l$-moment problem, is defined as

$$ {\widetilde{W}}(t)=\Lambda_{N}^{p^{\prime}}\left|\sum_{i=1}^{N}\xi_{i}^{*}g_{i}(t)\right|^{p^{\prime}-1}\operatorname{sign}\left[\sum_{i=1}^{N}\xi_{i}^{*}g_{i}(t)\right],\quad t\in[0,T]. $$

(23)

Similarly, in the case of Problem B,

$$ {\widetilde{W}}(t)=l^{p^{\prime}}\left|\sum_{i=1}^{N}\xi_{i}^{*}g_{i}(t)\right|^{p^{\prime}-1}\operatorname{sign}\left[\sum_{i=1}^{N}\xi_{i}^{*}g_{i}(t)\right],\quad t\in[0,T^{*}], $$

(24)

where $T^{*}$ is a minimal nonnegative real satisfying the condition

$$ \Lambda_{N}(T)\leq l, $$

(25)

where

$$ \frac{1}{\Lambda_{N}(T)}=\left(\int\limits_{0}^{T}\left|\sum_{i=1}^{N}\xi_{i}^{*}g_{i}(t)\right|^{p^{\prime}}dt\right)^{1/{p^{\prime}}}. $$

Example 1

Consider an approximate solution to Problem B of an optimal boundary control for the superdiffusion equation to which (1) is reduced provided that

$$ \begin{gathered}\displaystyle f(x,t)=u(x,t)=0,\quad r(x)=w(x)=1,\quad q(x)=0,\\ \displaystyle b_{1}=b_{2}=0,\quad a_{1}=a_{2}=1,\quad h_{1}(t)=h_{2}(t)=0,\\ \displaystyle u^{1}(t)=u^{2}(t)=u(t),\quad\varphi^{0}(x)=Q^{0}=\operatorname{const},\quad\varphi_{1}(x)=Q^{1}=\operatorname{const}.\end{gathered} $$

We define the finite state (5) to which the system is transferred in the minimal time in the form

$$ Q^{*}(x)=Q^{T}=\operatorname{const},\quad Q^{T}>Q^{0}. $$

The eigenfunctions $X_{n}(x)$ and the eigenvalues $\lambda_{n}$ in our case are written as follows:

$$ X_{n}(x)=\sin\frac{\pi nx}{L},\quad\lambda_{n}=\left(\frac{\pi n}{L}\right)^{2}. $$

We consider essentially bounded boundary controls $u(t)\in L_{\infty}[0,T]$.

In general, the problem of this section is similar to the problem of optimal boundary control for the subdiffusion equation with $\alpha\in(0,1]$ which was studied in [8].

Moments (11) in this case are defined as

$$ c_{n}=\frac{2}{\pi n}\,\big{(}1-(-1)^{n}\big{)}\big{[}Q^{T}-Q^{0}E_{\alpha}(-\lambda_{n}T^{\alpha})-Q^{1}TE_{\alpha,2}(-\lambda_{n}T^{\alpha})\big{]}. $$

Function (9) is proportional to the boundary control

$$ W(t)=\frac{2\lambda_{n}}{\pi n}\,\big{(}1-(-1)^{n}\big{)}u(t). $$

The finite-dimensional $\{l,N\}$-moment problem in this case is written as follows:

$$ \int\limits_{0}^{T}g_{n}(t,T)u(t)\,dt=\frac{Q^{T}-Q^{0}E_{\alpha}(-\lambda_{n}T^{\alpha})-Q^{1}TE_{\alpha,2}(-\lambda_{n}T^{\alpha})}{\lambda_{n}}={\tilde{c}}_{n}. $$

(26)

Take $N=3$ and find a solution to (26). Using (22), we reduce the conditional minimization problem (21) to a similar problem of the form

$$ \frac{1}{\Lambda_{3}(T)}=\min_{\xi_{1},\,\xi_{2}}\rho_{\xi}(T), $$

and

$$ \rho_{\xi}(T)=\int\limits_{0}^{T}\left|\xi_{1}g_{1}(t)+\xi_{2}g_{2}(t)+\frac{1-\xi_{1}c_{1}-\xi_{2}c_{2}}{c_{3}}\,g_{3}(t)\right|dt. $$

This problem can be solved numerically, for instance, by using the algorithm of [5, Chapter 4, § 1]. The so-found estimates $T^{*}$ and $\xi^{*}_{1,2}$ allow us to calculate an optimal control and the state of the system at final moment of time by (24) and

$$ Q^{N}(x,T^{*})=\sum_{n=1}^{N}Q_{n}(T^{*})X_{n}(x), $$

where

$$ Q_{n}(T^{*})\!=\!\frac{2}{\pi n}\big{(}1-(-1)^{n}\big{)}\Bigg{[}\lambda_{n}\!\int\limits_{0}^{T^{*}}\!g_{n}(t,T^{*})u(t)dt\!+\!Q^{0}E_{\alpha}(-\lambda_{n}(T^{*})^{\alpha})\!+\!Q^{1}T^{*}E_{\alpha,2}(-\lambda_{n}(T^{*})^{\alpha})\Bigg{]}. $$

Fig. 1

An example of the optimal control calculated numerically (the upper picture) and the final state of the system (lower picture).

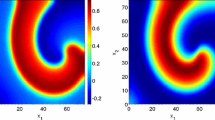
In Fig. 1 the optimal control and final state for the values of parameters $l=100$, $\alpha=1,8$, $Q^{0}=10$, $Q^{T}=30$, and $Q^{1}=0$ (the accuracy of numerical calculation of control time is equal to $5\times 10^{-2}$) are displayed. In Fig. 2 the dependence of control time $T^{*}$ on the exponent $\alpha$ for $Q^{1}=0$ (solid line) and $Q^{1}=5$ (dashed line) are displayed; the corresponding lines are very close.

Fig. 2

Dependence of the control time on the exponent $\alpha$.

Conclusion

In the present article, the optimal control problem is studied for a system whose behavior is described by a diffusion-wave equation containing the fractional Caputo time derivative. The problem reduces to a countable $l$-moment problem on the base of an exact solution to the diffusion-wave equation and to a finite-dimensional $l$-moment problem on the base of an approximate solution. In the latter case, we prove the well-posedness and solvability of the problem.