Introduction

Due to its substantial benefits, such as simple structure, lightweight, high-power factor and high efficiency, permanent magnet synchronous motor (PMSM) has broad application prospects in various fields. However, this also puts higher requirements for motor operation [1,2,3]. As one of the critical performance indices, vibration and noise issues have gradually become a research hotspot and have aroused extensive attention [4]. In the preliminary stage of motor design, parameters and excitation source design are closely related to vibration and noise level. An unreasonable design scheme is responsible for severe vibration and noise problems, degrading the motor’s efficiency. Therefore, it is crucial to discuss the noise excitation source of PMSM and related issues to improve motor performance [5,6,7].

Motor vibration mainly includes mechanical, aerodynamic and electromagnetic (EM) vibration. Since the radial EM force in the air-gap periodically deforms the stator core, this paper only concentrates on EM vibration caused by the interaction of radial EM force in the air-gap [8, 9]. Consequently, research on radial EM force and stator core deformation is the premise of motor vibration further analysis, revealing the inherent law of motor vibration and fundamentally realizing vibration and noise reduction.

Radial EM force is the dominating source of vibration. The analytical method was adopted in [10] to calculate the no-load magnetic field, armature reaction magnetic field, stator slotting magnetic field, and load magnetic field of surface-mounted PMSM, laying the foundation for radial EM force calculation. Then, the fast Fourier analysis method was employed to establish the relationship between frequency and order of radial EM force and illustrate the critical role of the spatial–temporal distribution [11]. The existing force analysis usually ignores the influence of tangential force, and the nonuniformly distributed radial EM force is equivalent to a concentrated force acting on the tooth center for calculation [12, 13]. This method does not consider additional bending moment contributed by the nonuniformly distributed force, inducing extra error. Meanwhile, in the spatial–temporal distribution of the radial EM force, the influence of the specific order force on vibration has been extensively studied [14,15,16,17]. Lin et al. [18] deduced the lowest spatial order force by an analytical model considering current harmonics and explained its influence on motor vibration and noise peak. Yang et al. [19] demonstrated that the force harmonics with low modal order could generate significant mechanical vibration. In [20], the relationship between the zero-order mode and lowest non-zero-order mode and the vibration was revealed based on the time variation of the spatial force distribution mean. However, there is a lack of research on the impact of specific order radial force on the total concentrated force and total additional bending moment.

These is a specific deformation law under the action of time-varying radial EM force called the vibration mode. Depending on the fixed support beam displacement equation, vibration mode directly determines the vibration amplitude, affecting the sound pressure level (SPL). Thus, in-depth research should be performed on the vibration mode to analyze the vibration and noise [21]. In [22], the vibration modes and excitation frequencies with different slot/pole combinations were converted into SPL for noise comparison. In [23,24,25], the motor vibration law was extensively studied under different conditions. In the existing stator vibration mode analysis, although more emphasis has been placed on noise prediction or influence, there is little research on the causes. Wang et al. [26] illustrated the causes of vibration mode through PMSM with a smooth stator surface and cogged rotor (SSCR) under the no-load condition. However, the analysis method could not be applied to PMSM with the cogged stator and smooth rotor surface (CSSR).

This paper presents a detailed analysis of the radial EM force by unit area and determines the low spatial order force wave that significantly influences the motor. The above conclusion is employed to establish a double equivalent model of air-gap permeance and air-gap flux density. It mainly comprises total concentrated force and additional bending moment applicable to PMSM with SSCR or CSSR. Besides, it can be utilized to analyze the action law, causes, and influencing factors of stator vibration mode. Taking an interior PMSM with a rated power of 3 kW with CSSR as the research object, the finite-element (FE) and experimental methods are employed to verify the radial EM force wave distribution and deformation law.

Study on Radial EM Force Wave with Significant Influence on Motor

The radial EM force wave comprises components with different frequencies and amplitudes. The components with low-order and high-amplitude characteristics are more likely to contribute significantly to vibration and noise levels. Besides, resonance will occur when the radial EM force wave frequencies are close to the modal frequencies. In this section, the radial EM force wave with significant influence can be acquired through spatial order, amplitude, and modal frequencies.

Basic Parameters and the FE Model

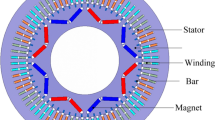
Taking an I-shaped interior PMSM with a rated power of 3 kW with CSSR as the research object, the basic parameters are shown in Table 1, and the two-dimensional FE model is shown in Fig. 1.

Table 1 Basic parameters
Full size table
Fig. 1
figure 1

Two-dimensional FE model

Full size image

Analytical and FE Analysis of Radial EM Force Wave with Low Spatial Order

Since the core permeability is much larger than the air gap, the tangential component of the EM force wave is much smaller than the radial one and can be neglected [27]. Thus, the EM force wave can be expressed as:

$$p_{\tt{r}} (\theta ,t) \approx \frac{{b_{\tt{r}}^{2} (\theta ,t)}}{{2\mu_{0} }} = \frac{{[f(\theta ,t)\lambda (\theta ,t)]^{2} }}{{2\mu_{0} }},$$
(1)

where br(θ,t) is radial air-gap flux density, μ0 is the vacuum permeability, f(θ,t) is the air-gap magnetic motive force (MMF), λ(θ, t) is air-gap permeance, θ is the mechanical angle, and t is time.

When the motor is in no-load condition, the permanent magnet (PM) provides the following air-gap MMF:

$$f_{1} (\theta ,t) = {\kern 1pt} {\kern 1pt} \sum\limits_{\mu } {F_{\mu } } \cos \left( {\frac{{\mu \omega_{1} }}{p}t - \mu \theta } \right),$$
(2)

where p is the number of pole-pairs, μ is the order of rotor harmonics, μ = (2r + 1)p, r = 0,1,2…, Fμ is the amplitude of MMF, which is related to the air-gap flux density amplitude Bμ and the air-gap length δ, and can be expressed as Fμ = Bμδ/μ0, and ω1 is the fundamental angular frequency.

When the motor is in rated-load condition, the following air-gap MMF is mainly provided by the PM and armature winding:

$$f_{2} (\theta ,t) = {\kern 1pt} {\kern 1pt} f_{1} (\theta ,t) + \sum\limits_{v} {F_{v} } \cos (\omega_{1} t - v\theta + \varphi_{v} ),$$
(3)

where ν is the order of stator harmonics, which are related to number of slots per pole and per phase q, when q is an integer, ν = (6 k + 1)p, k = 0, ± 1, ± 2, ± 3…, and φν is the initial phase angle of the air-gap MMF.

Assuming that the rotor has no eccentricity and a smooth surface, the air-gap permeance is expressed as:

$$\lambda (\theta ,t) = \Lambda_{0} + \sum\limits_{k = 1}^{\infty } {\Lambda_{k} } \cos (kZ_{1} \theta ),$$
(4)

where Λ0 is the constant component of air-gap permeance, Λk is the amplitude of the kth harmonic permeance, and Z1 is the stator slots number.

According to [27], taking μ0/δ as the base value, the air-gap permeance can be further expressed as:

$$\lambda^{\prime}(\theta ,t) = \frac{{\mu_{0} }}{\delta }\Lambda_{0}^{*} + \sum\limits_{k = 1}^{\infty } {( - 1)^{k + 1} \frac{{\mu_{0} }}{\delta }\Lambda_{{_{k} }}^{*} } \cos (kZ_{1} \theta ).$$
(5)

After sorting out Eqs. (1, 2, 3, 4, 5), the spatial–temporal distribution of radial EM force wave in no-load condition can be obtained, as shown in Table 2 as A–E, and the one in the rated-load condition is shown as A–I. Since the low spatial order in the spatial–temporal distribution significantly influences the motor, this part focuses on the analysis. When the motor is in no-load condition, the radial EM force wave can be classified into two types according to origin: one contributed by the main pole magnetic field and the other modulated by the tooth harmonic magnetic field, which are A, B and C, D, E, respectively. The spatial–temporal distribution of A and B are (2μ,2μ/p), [μ2 ± μ1,(μ2 ± μ1)/p], respectively, and can be further expressed as [2(2r + 1)p, 2(2r + 1)] and [(2r2 + 1)p ± (2r1 + 1)p,(2r2 + 1) ± (2r1 + 1)]. When r = 0, r2 − r1 = 1, the (2p,2) with low spatial order emerges. The low spatial orders of C, D, and E are generated by the interaction between the rotor main pole magnetic field (μ = p) and the tooth harmonic magnetic field (k = 1), which are expressed as r = μ ± (p ± Z1) = (2r + 1)p ± (p ± Z1). When the two “ ± ” take different signs, the r expression reaches the minimum recorded as r3 and r4, and the corresponding frequencies are fr3 and fr4, respectively:

$$r_{3} = \mu - (p + Z_{1} ) = 2rp - Z_{1} , f_{{r{3}}} = 2rf,$$
(6)
$$r_{4} = \mu + (p - Z_{1} ) = 2(r + 1)p - Z_{1} ,f_{r4} = 2(r + 1)f.$$
(7)
Table 2 Spatial–temporal distribution of radial EM force wave
Full size table

When the motor is in rated-load condition, the spatial orders μ include all spatial orders |ν|, so the spatial orders μ ± ν, 2ν, ν1 + ν2 are all within the range of 2μ, and orders μ ± ν ± kZ1 are all within the range of μ2 ± μ1 ± kZ1. Accordingly, the low spatial orders of radial EM force wave are precisely the same under no-load and rated-load conditions, and the corresponding spatial–temporal distribution is (2p, 2), (2rp − Z1, 2r), and [2(r + 1)p − Z1, 2(r + 1)]. Thus, the spatial–temporal distribution with low spatial orders of the 36-slot/6-pole combination can be arranged as (6, 2) and (0, 12), where k = 1, r = 5 or r = 6.

FE analysis is performed on the no-load and rated-load conditions to further verify the rationality of analytical derivation. When the motor runs at a steady state, each position's radial air-gap flux density at every moment is substituted into Eq. (1). Figure 2 shows the radial EM force wave curves, and Fig. 3 shows the spatial–temporal characteristics obtained by the Fourier decomposition.

Fig. 2
figure 2

Radial EM force wave curves under a no-load condition and b rated-load condition

Full size image
Fig. 3
figure 3

The spatial–temporal characteristics of radial EM force wave under a no-load condition and b rated-load condition

Full size image

As shown in Fig. 3, the spatial–temporal characteristics show the parallel diagonal distribution, in which the corresponding orders of the main pole magnetic field and the tooth harmonic magnetic field are (6, 2), (12, 4), (18, 6), (24, 8), (30, 10), (36, 12) and (0, 12), (6, 14), (12, 16), which is consistent with Table 4. Furthermore, the force amplitude of (6, 2) and (0, 12) is more prominent. (0, 12) is mainly generated by the interaction between the main pole magnetic field and the first-order tooth harmonic. The amplitude is more significant than other tooth harmonics and is dominant in tooth harmonics.

Modal Analysis

As the rotor and shaft have little influence on modal results, the established three-dimensional prototype model only includes the enclosure, end covers, stator core and winding. Nevertheless, the meshing quality of winding is poor in the FE analysis, leading to a considerable error. Therefore, appropriate simplifications are necessary for the modal analysis; that is, the winding of the loose wire and its end is equivalent to a bar and an end ring, respectively. Figure 4 shows the prototype model of 36-slot/6-pole and each component.

Fig. 4
figure 4

Prototype model of 36-slot/6-pole and each component

Full size image

Assuming that the equivalent winding mass is equal to the actual mass, and the equivalent density is the ratio of mass to equivalent volume, the elastic modulus can be calculated by slot fill factor and insulating material. Partial material parameters are presented in Table 3.

Table 3 Partial material parameters of the prototype
Full size table

Material parameters in Table 3 are added to the prototype, and the modal results are shown in Fig. 5. The second-order and third-order modal frequencies are 1015.1 Hz and 2267.1 Hz, respectively, which are far away from the force wave frequencies with significant influence on the motor so that no resonance will occur. Consider that the force wave frequencies with significant influence can be obtained from the temporal orders of (6,2) and (0,12), which are 2f = 75 Hz and 12f = 450 Hz, respectively.

Fig. 5
figure 5

The second-order and third-order modal frequencies and modal shapes

Full size image

Noise Calculation

The prototype under no-load and rated-load conditions is applied to attain multi-speed effective radiation power level curves, as shown in Fig. 6a and b.

Fig. 6
figure 6

Effective radiation power level curves under a no-load condition and b rated-load condition

Full size image

Figure 6 shows an apparent bright line in the 36th order, indicating its most contribution to the total SPL. The noise order is equal to the ratio of frequency to rotation frequency, and the rotation frequency is revolutions per second. At 750 rpm, the rotation frequency is 12.5 Hz, so the frequency corresponding to the 36th order is 450 Hz, which corresponds to 12f of the radial EM force wave. Therefore, the spatial–temporal distribution (0, 12) can lead to more severe vibration and noise, and at this time, k = 1.

Analysis of Stator Vibration Mode

Theoretical Analysis of the Vibration Mode

The vibration mode analysis is based on Fig. 1, where the stator inner radius is R, and the calculated polar distance is τα, where τ is the polar distance, α is the polar arc coefficient, and β is a central angle expressed as follows:

$$\beta = \frac{{\frac{\tau \alpha }{2}}}{R} = \frac{{\frac{{{\uppi }D\alpha }}{2p \times 2}}}{R} = \frac{{\alpha {\uppi }}}{2p}\;.$$
(8)

As shown in Fig. 7a, the radial EM force on each stator tooth has a nonuniform amplitude. For calculation convenience, the force on each tooth is equivalent to a concentrated force and an additional bending moment, as shown in Fig. 7b. The tooth corresponding to one pole is equivalent to a total concentrated force Fr and a total additional bending moment Mr by ignoring the slotted effect. This will be introduced in depth later.

Fig. 7
figure 7

The a nonuniform acting force and b equivalent concentrated force and bending moment

Full size image

Double equivalence is required before discussing the stator vibration mode:

  1. (1)

    As described in the previous section, when k = 1, the radial EM force significantly influences the vibration. Thus, Eq. (4) can only be considered as the superposition of constant and first-order tooth harmonic permeances, and the expression is:

$$\lambda^{\prime}(\theta ,t) = \Lambda_{0} + \Lambda_{1} \cos (Z_{1} \theta ),$$
(9)

where Λ1 is the first-order tooth harmonic permeance.

  1. (B)

    The air-gap flux density is a function of time and space. It is discretized in space and continuous in time to simplify the calculation. Also, it is equivalent to a square wave after ignoring the slotted effect in space, whose height is Bδ and width is τα, as shown in Fig. 8. After determining the spatial impact, the relationship between the air-gap flux density and time can be approximated with a cosine function. The polar coordinate system is established with the vertical y direction of Fig. 1 as the 0-degree reference direction. According to Fig. 1 and Fig. 8, the cosine relationship can be expressed as follows:

$$B = \left\{ {\begin{array}{*{20}c} {\begin{array}{*{20}c} {B_{\delta } \cos \omega t} & {\begin{array}{*{20}c} {\left[ { - \beta + i\frac{{2{\uppi }}}{2p},} \right.} & {\begin{array}{*{20}c} {\left. {\beta + i\frac{{2{\uppi }}}{2p}} \right]} & {i = 2m} \\ \end{array} } \\ \end{array} } \\ \end{array} } \\ {\begin{array}{*{20}c} 0 & {\begin{array}{*{20}c} {\left[ {\beta + i\frac{{2{\uppi }}}{2p},} \right.} & {\begin{array}{*{20}c} {\left. { - \beta + (i + 1)\frac{{2{\uppi }}}{2p}} \right]} & {i = n} \\ \end{array} } \\ \end{array} } \\ \end{array} } \\ {\begin{array}{*{20}c} { - B_{\delta } \cos \omega t} & {\begin{array}{*{20}c} {\left[ { - \beta + i\frac{{2{\uppi }}}{2p},} \right.} & {\begin{array}{*{20}c} {\left. {\beta + i\frac{{2{\uppi }}}{2p}} \right]} & {i = 2m + 1} \\ \end{array} } \\ \end{array} } \\ \end{array} } \\ \end{array} } \right.$$
$$m = 0,1,2{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} n = 0,1,2,3,4,5$$
(10)

where B is the equivalent air-gap flux density under the action of time and space, and ω is the angular frequency.

Fig. 8
figure 8

The spatial impact on air-gap flux density

Full size image

In the above equivalence, the calculation of air-gap permeance only considers the dominant harmonic. The air-gap flux density calculation ignores the slotted effect to realize waveform idealization. The two-point equivalent methods have little effect on the vibration amplitude and do not affect the vibration mode.

According to Eqs. (1, 2, 3, 5) and (8, 9, 10), the actual air-gap flux density can be expressed as:

$$\begin{aligned} b_{{\text{r}}}^{\prime } (\theta ,t) & = F_{\delta } \cos \omega t \times (\Lambda _{0} + \Lambda _{1} \cos Z_{1} \theta ) \\ & = B_{\delta } \Lambda _{0}^{*} \cos \omega t + \frac{1}{2}B_{\delta } \Lambda _{1}^{*} \cos (Z_{1} \theta \pm \omega t), \\ \end{aligned}$$
(11)

where Fδ is the amplitude of MMF corresponding to the equivalent air-gap flux density.

By substituting Eq. (11) into Eq. (1), the actual radial EM force wave is:

$$p_{\tt{r}}^{\prime } (\theta ,t) = \left[ {\frac{{\Lambda_{0}^{*2} \cos^{2} \omega t}}{{2\mu_{0} }} + \frac{{\Lambda_{1}^{*2} \cos^{2} (Z_{1} \theta \pm \omega t)}}{{8\mu_{0} }} + \frac{{\Lambda_{0}^{*} \Lambda_{1}^{*} \cos (Z_{1} \theta \pm \omega t)}}{{2\mu_{0} }}} \right] \times B{}_{\delta }^{2} .$$
(12)

The first two terms in Eq. (12) are small and can be ignored. Considering only the influence of the third term, Eq. (12) can be expressed as:

$$p^{\prime}_{\tt{r1}} (\theta ,t) = \frac{{B{}_{\delta }^{2} \Lambda_{0}^{*} \Lambda_{1}^{*} }}{{2\mu_{0} }}\cos (Z_{1} \theta \pm \omega t)\;.$$
(13)

Regardless of the stator slotted effect, considering the teeth corresponding to one pole, the Fr of this part can be calculated by integrating the radial EM force wave at a certain point. The equivalent integral diagram is shown in Fig. 9 (dθ is the unit angle), and the calculation formula is:

$$\begin{gathered} F_{{\text{r}}} = l_{{\text{p}}} \int_{{\frac{{ - \alpha {\uppi }}}{2p} + i\frac{{2{\uppi }}}{2p}}}^{{\frac{{\alpha {\uppi }}}{2p} + i\frac{{2{\uppi }}}{2p}}} {p^{\prime}_{{{\text{r}}1}} (\theta ,t)} R{\text{d}}\theta \hfill \\ {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} { = }\,\,\frac{{l_{{\text{p}}} B_{\delta }^{2} \Lambda_{0}^{*} \Lambda_{1}^{*} R}}{{\mu_{0} Z_{1} }}\sin \alpha_{1} {\uppi }\cos (i2{\uppi }Z_{{\text{p}}} \pm 2\omega t)\;, \hfill \\ \end{gathered}$$
(14)

where lp is the magnetic pole length, α1 = αZ1/2p, and Zp = Z1/2p.

Fig. 9
figure 9

Equivalent integral diagram

Full size image

The Mr also plays an essential role in the vibration mode, which can be acquired by multiplying the force by the force arm. The force expression is shown in Eq. (14). The force arm x (or \(x^{\prime}\)) is expressed as the distance from any action point to the central axis in Eq. (15), and the action mode is illustrated in Fig. 10.

$$x = R\sin \theta {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} (or{\kern 1pt} {\kern 1pt} x^{\prime} = R^{\prime}\sin \theta^{\prime}).$$
(15)
Fig. 10
figure 10

The action mode of the force arm

Full size image

By sorting out Eqs. (14) and (15), Mr can be expressed as follows:

$$\begin{gathered} M_{{\text{r}}} = l_{{\text{p}}} \int_{{\frac{{ - \alpha {\uppi }}}{2p} + i\frac{{2{\uppi }}}{2p}}}^{{\frac{{\alpha {\uppi }}}{2p} + i\frac{{2{\uppi }}}{2p}}} {P_{{{\text{r}}1}}^{\prime } (\theta ,t)} x{\text{d}}x \hfill \\ {\kern 1pt} { = }\,\,\frac{{l_{{\text{p}}} B_{\delta }^{2} \Lambda_{0}^{*} \Lambda_{1}^{*} R^{2} }}{{2\mu_{0} Z_{1} }}\sin \frac{{\alpha {\uppi }}}{2p}\cos \alpha_{1} {\uppi }\sin (i2{\uppi }Z_{{\text{p}}} \pm \omega t)\;. \hfill \\ \end{gathered}$$
(16)

Equations (14) and (16) show that the amplitude of Fr is twice that of Mr, both of which are sine and cosine functions and are all affected by lp, Bδ, Λ*0, Λ*1, R, Z1, Zp, etc. Among them, the sine relation sinα1π and the cosine relation cosα1π significantly influence Fr and Mr, respectively, and so does Zp. Based on the sine and cosine characteristics, α1 can be divided into integer and (integer + 0.5). Zp represents the stator teeth number under each magnetic pole. When integer teeth are included, the force arms on both sides are equal because the distance between each tooth under the left and right polar arcs and centerline is equal. The bending moment phases on the teeth corresponding to adjacent magnetic poles are also consistent. When (integer + 0.5) teeth are included, the phases are opposite. When α1 is an integer or (integer + 0.5) and Zp is an integer or (integer + 0.5), the action law of Fr and Mr can be expressed as follows:

  1. (1)

    When Zp and α1 are both integers, the bending moments on the teeth corresponding to adjacent magnetic poles are in phase, the Fr is the smallest, and the Mr is the largest. The vibration mode is 2p, as shown in Fig. 11a.

  2. (2)

    When Zp is an integer, and α1 is (integer + 0.5), the bending moments on the teeth corresponding to adjacent magnetic poles are in phase, the Mr is the smallest, and the Fr is the largest. The vibration mode is 0, as shown in Fig. 11b.

  3. (3)

    When Zp is (integer + 0.5), and α1 is an integer, the bending moments on the teeth corresponding to adjacent magnetic poles have opposite phases, the Fr is the smallest, and the Mr is the largest. The vibration mode is p, as shown in Fig. 11c.

  4. (4)

    When Zp and α1 are both (integer + 0.5), the bending moments on the teeth corresponding to adjacent magnetic poles have opposite phases, the Mr is the smallest, and the Fr is the largest. The vibration mode is p, as shown in Fig. 11d.

Fig. 11
figure 11

The stator vibration modes under four cases: a Zp=integer, α1=integer, b Zp=integer, α1=integer+0.5, c Zp=integer+0.5, α1=integer, and d Zp=integer+0.5, α1=integer+0.5

Full size image

The vibration mode law in four cases can be summarized in Table 4. Table 4 shows that the vibration mode is determined by the cooperation of bending moment phase direction and excitation source attribute, determined by Zp and α1, respectively. Since Zp = Z1/2p and α1 = αZ1/2p, the two are mainly affected by slot/pole combination, which is critical to stator vibration mode.

Table 4 The vibration mode law of stator core
Full size table

The above scheme employs the double equivalent method to analyze the causes and influencing factors of the vibration mode of PMSM with CSSR. This method can simplify the calculation and clarify the relationship between slot/pole combination and vibration mode. For PMSM with SSCR, the air-gap permeance is presented in Eq. (17). After applying the double equivalent method, the expression of \(F^{\prime}_{{\text{r}}}\) is shown in Eq. (18). This has only a phase change compared to the original Fr, while Mr is the same as the change. The phenomenon shows that the stator vibration mode law in Table 4 is satisfied. Thus, this scheme applies to PMSM with CSSR and PMSM with SSCR.

$$\lambda ^{\prime\prime}(\theta ,t) = \Lambda_{0} + \Lambda_{1} \cos (Z_{2} \theta - Z_{2} \Omega_{2} t),$$
(17)

where Z2 is the rotor slots number, and Ω2 is the rotor mechanical angular velocity.

$$F_{{\text{r}}}^{\prime } { = }\,\,\frac{{l_{{\text{p}}} B_{\delta }^{2} \Lambda_{0}^{*} \Lambda_{1}^{*} R}}{{\mu_{0} Z_{1} }}\sin \alpha_{1} {\uppi }\cos (i2{\uppi }Z_{{\text{p}}} \pm 2\omega t - Z_{2} \Omega_{2} t).$$
(18)

FE Simulation of the Vibration Mode

The radial EM force gained by two-dimensional FE analysis under no-load and rated-load conditions is circularly loaded on the stator core and the prototype, as shown in Fig. 12. In the model of Fig. 12a, 120° symmetrical bolt holes on the stator yoke are set, and cylindrical constraints are added. In the model of Fig. 12b, fixed constraints at the bottom corner of the prototype are added. The model in Fig. 12a is simulated to obtain stator vibration modes under no-load and rated-load conditions, as shown in Fig. 13. The model in Fig. 12b is established to capture the vibration curves at point a under two working conditions. Only the no-load working condition curve is shown in Fig. 14 due to the article length limitation.

Fig. 12
figure 12

FE model of a stator core and b prototype

Full size image
Fig. 13
figure 13

Stator vibration modes under a no-load condition and b rated-load condition

Full size image
Fig. 14
figure 14

Simulated curve of radial vibration displacement at point a under the no-load condition

Full size image

Figure 13 shows that Fr and Mr make the stator core fluctuate six times based on the original model for both no-load and rated-load conditions, which means that the stator vibration mode is 6. The result is consistent with theoretical analysis result. In theoretical analysis, Zp = 6, α1 = 4 for the 36-slot/6-pole combination. It is judged that the bending moments are in phase, and Mr is the main excitation source, so the vibration mode is 2p = 6. To further explore the vibration mode law in Fig. 11b, c, and d, three motors with different slot/pole combinations are selected, i.e., 36/6, 12/8, and 36/8. The FE simulation results are shown in Table 5, which are compatible with the theoretical derivation in Table 4. The above analysis further illustrates the rationality of the equivalence, the correctness of the theoretical analysis model, and the applicability to both no-load and rated-load conditions.

Table 5 Vibration mode law of three motors with different slot/pole combinations
Full size table

Under the constant consumption of PM and polar arc coefficient, the V-shaped PM arrangement motor replaces the I-shaped one. The two-dimensional model and simulation results are shown in Fig. 15. As shown in Fig. 15, the stator vibration mode of the V-shaped PM arrangement motor is 6, which is compatible with that of the I-shaped one, indicating the applicability of the theoretical analysis model.

Fig. 15
figure 15

Stator vibration mode of the V-shaped PM arrangement motor

Full size image

PMSM can be divided into interior and surface-mounted types. The section takes an 18/4 surface-mounted motor (Zp = 4.5, α1 = 4.5) as the research object to study the vibration mode law. The simulation result is presented in Fig. 16. It is identical to theoretical analysis, demonstrating the applicability of this scheme to a surface-mounted PMSM.

Fig. 16
figure 16

Stator vibration mode of surface-mounted PMSM

Full size image

Experimental Verification of the Vibration Mode

This section deals with the experimental validation of stator vibration mode through vibration displacement amplitude. The experimental platform is described in Fig. 17a, and the equipment parameters are shown in Table 6. After power-on, the frequency converter controls the motor to start until running stably, and the vibration response is captured by ICP unidirectional acceleration sensor and imported into AVANT MI-7008 dynamic data acquisition analyzer for further analysis. Figure 17b shows the experimental setup and connection schematic diagram.

Fig. 17
figure 17

The a experimental platform and equipment and b experimental schematic diagram for setup and connection

Full size image
Table 6 Main equipment parameters
Full size table

Since the prototype is three-phase symmetrical, all pairs of points between a, b, and c have the same stator vibration mode. This section only studies points a, b, and the central areas. The test indicates that 12 points can be continuously selected as measuring points on the central axis, as shown in Fig. 18.

Fig. 18
figure 18

Distribution of 12 continuous vibration measuring points along the central axis between points a and b

Full size image

The unidirectional acceleration sensor should be radially placed on the radiating fin during vibration measurement. After the stable operation, the acceleration sensor collects the vibration displacement of each measuring point. To save space, this paper only lists the vibration displacement curve at point a under no-load condition, as shown in Fig. 19.

Fig. 19
figure 19

Experimental curve of radial vibration displacement at point a under no-load condition

Full size image

Figures 14 and 19 show that the radial vibration displacement amplitude of the simulated curve is smaller than that of the experimental one due to the ideal simulation environment. However, the experimental environment should consider the EM and mechanical vibrations, equipment installation and fixation and other factors. Thus, the experimental displacement result is larger than the simulated one.

To further investigate the radial vibration displacement of 12 measuring points, the Fourier decomposition method performs frequency spectrum analysis on each point. Figure 20 compares the simulated and experimental frequency spectrum analysis at point a under the no-load condition. As shown in Fig. 20, simulated and experimental curves have the same trend. Besides, the radial vibration amplitude corresponding to 75 Hz is the largest, which can be an ideal reference point. The simulated and experimental displacement amplitude of 12 reference points between a and b is collected, as shown in Fig. 21.

Fig. 20
figure 20

Comparison of simulated and experimental frequency spectrum analysis at point a under no-load condition

Full size image
Fig. 21
figure 21

The fluctuation curves of simulated and experimental radial vibration displacement amplitude of 12 reference points

Full size image

As shown in Fig. 21, the overall trend of the simulated and experimental radial vibration displacement amplitude of 12 reference points is consistent. Moreover, Fig. 21 shows two stator core fluctuations between points a and b, leading two fluctuations between b and c, and c and a. Thus, the motor stator fluctuates six times. According to Fig. 21, the displacement amplitude of each reference point on the central axis of the motor is drawn in Fig. 22 to more intuitively analyze the vibration mode, where the experimental curve is the average value of five data groups. In Fig. 22, convex points of the simulated and experimental curves are connected for the convenience of analysis. The two connecting lines have a hexagon shape; that is, their vibration modes are 6. Besides, the shape of the simulated connecting line is relatively symmetrical due to the ideal environment, while there are some errors in the experiment. Through curve fitting and comparison, the maximum error of simulation and experiment is calculated as 7.41%. This is mainly due to the gravity component of the acceleration sensor and radial installation error caused by radiating fins in the experiment, while this does not affect the vibration mode results. Therefore, in the above analysis process, simulated and experimental results can further demonstrate the correctness of the theoretical analysis model.

Fig. 22
figure 22

The simulated and experimental radial vibration displacement amplitude distribution and vibration mode of the motor

Full size image

Conclusion

This paper analyzes radial EM force waves and determines the low spatial order. The double equivalence of air-gap permeance and air-gap flux density is realized using the above conclusion. A theoretical analysis model mainly comprises the total concentrated force and additional bending moment. This model is applied to PMSM with CSSR and PMSM with SSCR to explore the vibration mode law validated by FE and experimental methods. The conclusions are as follows:

  1. (1)

    Low spatial order force waves such as (6, 2) and (0, 12) can cause considerable vibration and noise. Among them, (0, 12) is mainly generated by the interaction between the rotor magnetic field and the first-order tooth harmonic magnetic field. Compared with other tooth harmonics, the one is dominant.

  2. (2)

    The vibration mode is determined by the Fr and Mr, which are affected by Zp and α1. Zp determines whether bending moment phases on the teeth corresponding to adjacent magnetic poles are in phase, and α1 determines the excitation source attribute. When Zp is an integer, the bending moment phases are the same; When Zp is (integer + 0.5), the bending moment phases are opposite; When α1 is an integer, the excitation source is Mr; When α1 is (integer + 0.5), the excitation source is Fr.

  3. (3)

    Zp and α1 are mainly affected by slot/pole combination, so the slot/pole combination indirectly affects the Fr and Mr, and its ratio plays an essential role in the vibration mode.

  4. (4)

    This paper proposes a theoretical analysis model suitable for both PMSM with CSSR and PMSM with SSCR to study the action law, causes, and influencing factors of stator vibration mode. It lays the foundation for the prediction and reduction of motor vibration.